Next Article in Journal
Distribution and Conservation Gaps of Nautilus pompilius: A Study Based on Species Distribution Models
Previous Article in Journal
Effect of Long-Term Set-Aside Management System on Soil Health by Evaluation of Biodiversity Indicators
Previous Article in Special Issue
The Herpetofauna of the Chihuahuan Desert Biogeographic Province of Mexico: Diversity, Similarity to Other Provinces, and Conservation Status
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Diversity
Volume 17
Issue 4
10.3390/d17040241
diversity-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Geometric Morphometric Analysis of Adult and Juvenile Turtle Shells: Directional Asymmetry and Fluctuating Asymmetry

by
Ece Oktay
1,
İlayda Boz Doğan
1,
Sokol Duro
2,
Gülsün Pazvant
3,
Funda Yiğit
4 and
Tomasz Szara
5,*
1
Institute of Graduate Studies, Istanbul University-Cerrahpasa, 34320 Istanbul, Türkiye
2
Department of Anatomy, Faculty of Veterinary Medicine, Agricultural University of Tirana, 1000 Tirana, Albania
3
Department of Anatomy, Faculty of Veterinary Medicine, Istanbul University-Cerrahpasa, 34320 Istanbul, Türkiye
4
Department of Histology and Embryology, Faculty of Veterinary Medicine, Istanbul University-Cerrahpasa, 34320 Istanbul, Türkiye
5
Department of Morphological Sciences, Institute of Veterinary Medicine, Warsaw University of Life Sciences-SGGW, 02-776 Warsaw, Poland
*
Author to whom correspondence should be addressed.
Diversity 2025, 17(4), 241; https://doi.org/10.3390/d17040241
Submission received: 27 February 2025 / Revised: 20 March 2025 / Accepted: 24 March 2025 / Published: 28 March 2025
(This article belongs to the Special Issue Biology and Evolutionary History of Reptiles)
Browse Figures
Versions Notes

Abstract

:
Bilateral symmetry is quite common in animals, but in some cases, asymmetry can be altered by hereditary or developmental processes. Symmetry may be preserved, or asymmetry may increase as the developmental stages progress. This study applied geometric morphometric analyses at the juvenile and adult stages to investigate directional asymmetry and fluctuating asymmetry in turtle shells. In total, 71 turtle shells (46 adults, 25 juveniles) of Testudo hermanni boettgeri were used. These turtle shells were recorded using the Generalized Procrustes method to interpret developmental asymmetry. A covariance matrix was then applied, followed by principal component analysis. Analysis of Variance (ANOVA) was used to explain individual variation. The procedures were applied and interpreted separately to the carapace and plastron. Specific structures, such as the nuchal and neural plates, exhibited a narrower shape than the mean shape configuration in directional asymmetry. The epiplastron region showed significant enlargement in juveniles compared to adults, potentially linked to developmental growth. This study investigated fluctuating asymmetry (FA) and directional asymmetry (DA) in turtle shells by analyzing the carapace and plastron. Although wavy asymmetry was not statistically significant overall, localized shape differences between the edges of the coastal and neural plates of the carapace and the edges of the plastron were observed. The side effects showed statistical significance (p = 0.0005). Environmental or developmental factors may have influenced these differences. Directional asymmetry was statistically significant for the carapace and plastron, indicating consistent shape changes associated with developmental growth. This study revealed significant directional asymmetry in the carapace and plastron of Testudo hermanni boettgeri, reflecting consistent developmental trends.

1. Introduction

Specimens of eastern Hermann’s tortoises (Testudo hermanni boettgeri) were used for research. This species is found in Mediterranean Europe, from the coast of northeastern Spain to southeastern France, as well as Mallorca (Spain), Menorca (Spain), Corsica (France), Sardinia (including Asinara Island), and Italy. It also inhabits the Croatian coasts, Bosnia and Herzegovina, Montenegro, central and southern Serbia, inland and southwestern Romania, most of Bulgaria, Macedonia, almost all of Albania, mainland Greece, and the European part of Türkiye [1]. Testudo hermanni hermanni and Testudo hermanni boettgeri (including Testudo hermanni hercegovinensis) are known subspecies [1]. T. h. hermanni is found in northeastern Spain, southeastern France, the Balearic Islands (Spain), Corsica (France), and Sardinia (Italy); Testudo hermanni boettgeri is found mainly in the Balkan region [1]. Although this species prefers Mediterranean oak forests, it can also inhabit maquis, seagrass meadows, agricultural areas, and railway sides [1].
The skull of this tortoise species articulates with the atlas through a single occipital condyle, as in other turtles. This species can retract its head into the shell through two strong muscles: m. retrahens capiti collique and m. carapacocervicocapitis medialis [2]. The carapace is a dermal bone structure covered with keratinized epithelium. The shell consists of a dorsal part, called the carapace, and a ventral part, flatter than the dorsal part, called the plastron. The shell comprises dermal bone, ribs, and thoracic and lumbar vertebrae [3]. The carapace has 5 vertebral scutes in the midline, 4 pleural scutes more laterally on each side, and 12 marginal scutes surrounding the first. In the plastron, the scutes are arranged to start from the cranial end: intergular, entoplastron, epiplastron, gular, humeral, pectoral, abdominal, femoral, and anal [4].
Geometric morphometrics methods as morphological methods have recently been preferred in anatomy and biology [5,6,7,8]. One of the reasons why such methods are preferred is that they can be used to explain differences regarding the averages of samples collected from one or more groups [9,10]. The differences and averages obtained from the samples can be interpreted functionally for studies. Geometric morphometric methods compare the crucial points determined during the survey by analyzing their spatial relationships through coordinate-based measurements. This contributes to seeing and interpreting the results individually and as a group [11,12].
In this study, a geometric morphometric method is used to place landmark points on the anatomical structures of specimens. These points are transferred to a coordinate system, forming a shape representation of the samples. The resulting shape is then analyzed in terms of distances, angles, centroid size, and asymmetry [13]. Since the shape is constructed by superimposing images of the samples in the coordinate plane, potential visual errors are minimized. This method allows for identifying shape differences and asymmetry levels between adults and juveniles [14].
Symmetry and asymmetry studies can provide fundamental insights into shape variations based on developmental, genetic, and environmental factors. These studies help to explain patterns of developmental stability and morphological integration. In short, they examine the quantitative characterization of the specimens used in these studies. As a result of these examinations, they support geometric morphometric arguments and improve the disciplines involved in these fields, such as biology and anatomy [15]. For this purpose, asymmetry can be analyzed in three categories: directional asymmetry, fluctuating asymmetry, and antisymmetry [16]. Directional asymmetry explains the stable differences shaped by the analyzed structures [17]. It reveals prominent characteristics [16]. Fluctuating asymmetry is used to find random differences [17] and may be attributed to environmental and developmental factors [16,18]. Antisymmetry is used to explain phenotypic differences [19]. However, antisymmetry is challenging to analyze when the number of samples is insufficient [17].
This study aimed to investigate the asymmetric factors between adult and juvenile turtle shells. Accordingly, the fluctuating and directional asymmetry between adult and juvenile turtle shells was investigated. In addition, by comparing the shells of adult and juvenile turtles, we tried to interpret the effect of environmental factors on the asymmetry in the shells by their habitat. Statistical information obtained from the geometric morphometric method was analyzed to determine the types of asymmetry in the shells.

2. Materials and Methods

2.1. Samples

In this study, 71 tortoises of the Testudo hermanni boettgeri species were collected and photographed from the hills and mountains around Tirana, Albania, between August and October 2019. Of these 71 turtles, 47 were adults and 24 were juveniles. Overall, 46 of the 71 turtles were females, and 25 were males. Ethical approval was obtained from the Ethics Committee of the Agricultural University of Tirana (Decision No. 143/15 April 2020). The external morphological characteristics of the turtles in their natural habitat were evaluated, subspecies and developmental levels were determined, and the individuals were classified as adults and juveniles. This classification was performed by counting the growth rings on the turtles’ shells [20]. The carapace and plastron were analyzed for symmetry and asymmetry. After the image-capturing phase, the animals were released back to their localities.

2.2. Digitization Process

Dorsal and ventral images of the shells were taken. The carapace and plastron structures were analyzed. All photographs were captured from a distance of 25 cm following the stabilization of the turtles. Photos were taken with a Nikon Digital Camera D7100 18-105 lens. The photographs were classified into two files, carapace and plastron, on a computer. Subsequently, the images were converted to ‘.tps’ format using tpsUtil (version 1.74) and introduced into tpsDig2 software (version 2.31) [21]. The tpsDig2 was used to designate the landmarks [22]. In total, 24 landmarks were used in the dorsal shell and 12 in the ventral shell. These landmarks were placed from the lateral edges of the peripheral bone for the dorsal shell to the corners of the neural, vertebral, and costal bones in the inner part. For the ventral shell, the lateral edges of the plastron were preferred to place the 12 landmarks [23]. The landmarks placed on both sides of the shell were type 1. This was because type 1 landmarks facilitate the identification of specific apparent anatomical structures [24]. The locations marked on the shells were predetermined, and the landmarks were placed using tpsDig2 software.
After landmarking, all images were saved as a text file, and the text’s numerical coordinate data were transferred to the MorphoJ (version 1.08.01) program for statistical analyses.
Each structure (carapace and plastron) was digitized twice to assess the measurement error [25].

2.3. Statistical Analysis

The “MorphoJ” program was used for geometric morphometric analyses [25]. Firstly, the Generalized Procrustes analysis was applied, and a covariance matrix was generated. With Procrustes, all points examined were superimposed as closely as possible to the average shape of the individuals. Landmark coordinate data were then standardized, and covariance matrices were generated from the shape data set [26]. Procrustes ANOVA test was applied to compare shape differences between adult and juvenile developmental levels and to assess the amount of measurement error associated with sources of variation [27]. MANOVA analyses were also performed for both shells described in the results.
Principal component analysis (PCA) was applied to show overall shape variation using multivariate analysis, and the main features of shape changes were visualized separately for both dorsal and ventral shells [28,29,30].
To examine the applicability of ANOVA for fluctuating asymmetry (FA), Levene’s statistic was applied between the Procrustes coordinates and individuals. For this purpose, the data were transferred to the SPSS program (version 29), and the homogeneity of variation was tested.
To examine the effect of size on shape variation, regression analysis was performed between the Procrustes coordinates and centroid size in MorphoJ.
In addition, sex-induced asymmetry (FA) between adult individuals was also considered to better explain the shape variation between juvenile and adult individuals. For this purpose, Principal Component Analysis (PCA) was also applied to male and female individuals.

3. Results

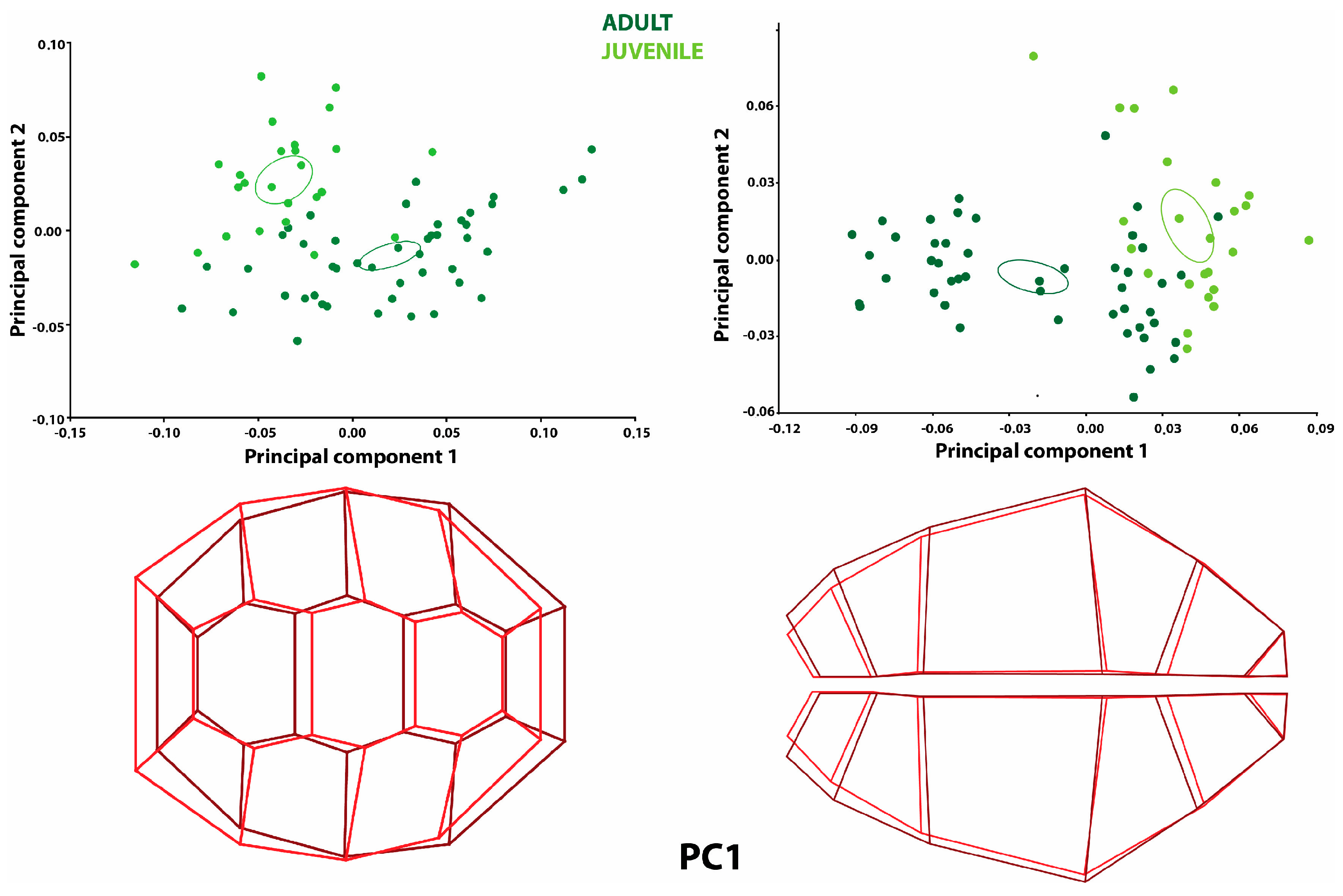
The degree to which the first five principal components explain the total variation is given in Table 1 for the carapace and Table 2 for the plastron. However, PC1 is considered in the figures and results (Figure 1 and Figure 2). PC1 explained the highest percentage of shape variance in the carapace and plastron among the principal components (Table 1 and Table 2).

3.1. Carapace

Twenty-four landmarks were superimposed on the images taken from the turtles’ shells. All landmarks were paired with each other. This ensured that no error was made in the symmetry of the selected points (landmarks) after the program transferred them to the coordinate plane.
The ANOVA test results showed that the size and shape variation were statistically significant (p < 0.0001; Table 3 and Table 4). Whether individual variation was significantly greater than error was determined by ANOVA analysis [29]. According to the ANOVA analysis results, the error value was low. As a result, for both the carapace and the plastron, the study was significant.
The difference between averages was also analyzed in this study. According to these results, the Procrustes distance was 0.06. Also, the T-square results, obtained to measure the difference between averages, were statistically significant (p < 0.0001).
The principal component analysis (PCA) results for the directional asymmetry of the carapace revealed that 50.9% of the total variation was explained by PC1 and 18.3% was explained by PC2 (Table 1). It was observed that the individuals representing the positive limit in PC1 were mostly adult turtles (Figure 1). The shape variation at the positive limit for PC1 was more significant than the mean shape configuration of the caudal side of the dorsal carapace. The cranial side of the carapace was wider than the mean shape configuration but narrowed as it progressed to the center of the carapace compared to the mean shape configuration. The mid-shell was more expansive than the average shape configuration, similar to the cranial and caudal sides.
Differences were also observed in the plates of the carapace for directional asymmetry. The first neural and nuchal plates showed a narrower shape variation in the area compared to the mean shape configuration. The second neural plate was narrower compared to the mean shape configuration. The fourth neural plate was more expansive than the average shape configuration. Therefore, the third neural plate was displaced cranially, although it did not show a different shape variation. The first costal plate was narrower in area compared to the average shape configuration. The second and third costal plates were shorter in width but longer in length. It was observed that these plates extended towards the second and third neural plates (Figure 1).
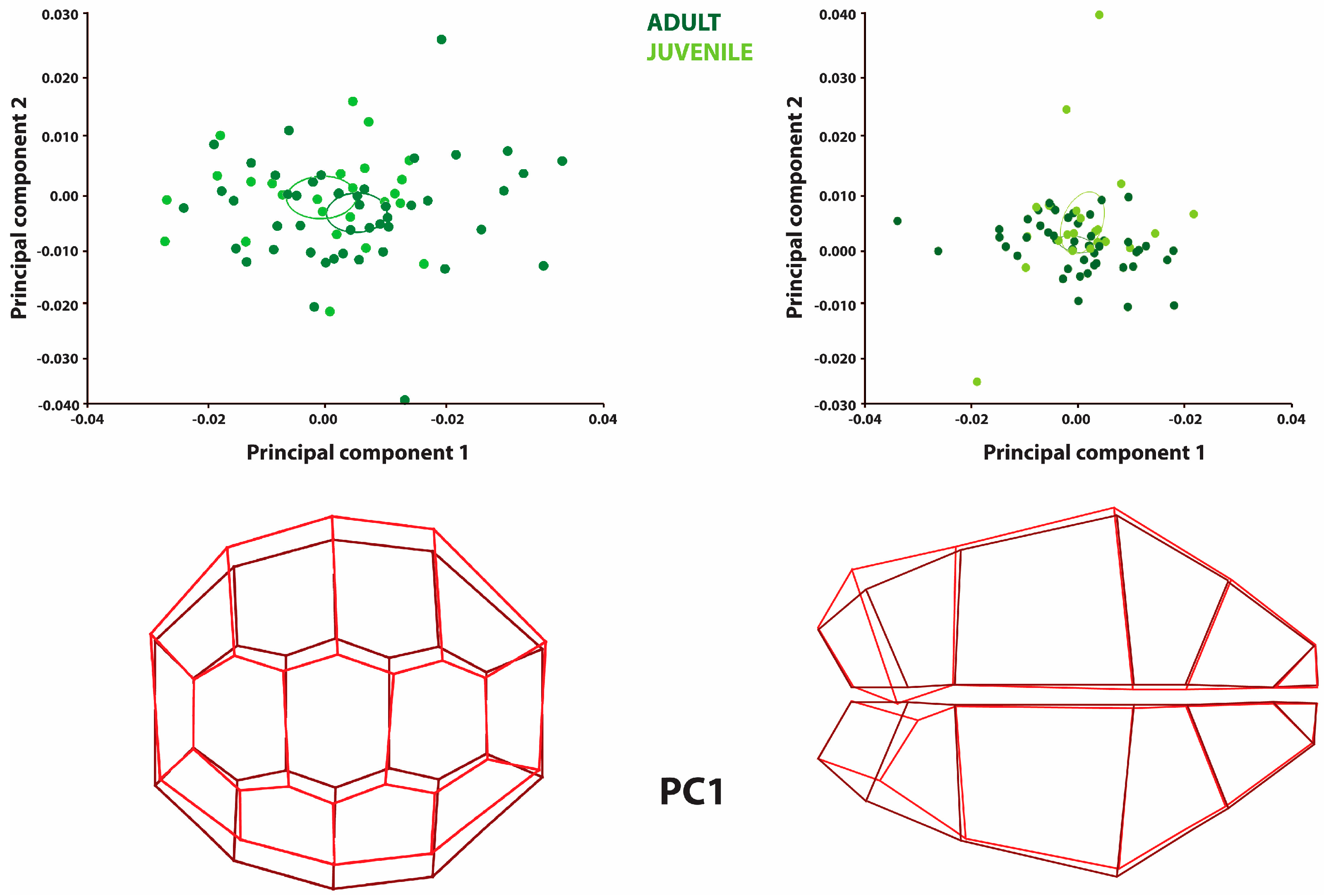
The principal component analysis (PCA) results for fluctuating asymmetry showed no difference between the groups (Figure 2). Also, the results were not statistically significant (Table 3). Nevertheless, PC1 explained 26.8% of the total variation, and PC2 explained 10.8% (Table 1). In the shape variation of fluctuating asymmetry in the PC1 graph, the shape variation shown by the costal plates located in the sinister was more expansive than the average shape configuration. As the positive PC1 value increased, the costal plates situated on the dexter became narrower. The landmarks on the neural plates were located at different points compared to the mean shape configuration (Figure 2).
In the MANOVA analysis of the carapace, the individual value for the symmetrical component was statistically significant (p < 0.0001). This supported the study’s other findings because directional asymmetry (DA) was statistically significant in different analyses.
As a result of the regression analyses for DA, the centroid size explained 5.97% of the shape variation (R2 = 0.0597). This relation was statistically significant (p = 0.0452). Although an effect of centroid size on the shape variation of the carapace was observed, this effect was not strong. The analysis for FA showed that size explained 3.99% of the shape variation (R2 = 0.0399). The result was not statistically significant (p > 0.05). As a result, the FA analysis revealed that centroid size has no significant effect on the shape variation of the carapace.

3.2. Plastron

Twenty-six landmarks were superimposed on the images taken from the plastron of the turtles. All the marks were paired with each other. This ensured that no error was made in the symmetry of the selected points (landmarks) after the program transferred them to the coordinate plane.
The results of the ANOVA revealed that the centroid size and shape variation were statistically significant (Table 5 and Table 6; p < 0.0001). The difference between the averages was also analyzed in this study. According to these results, the Procrustes distance was 0.06. In addition, the T-square results, obtained to measure the difference between the means, were statistically significant (p < 0.0001).
Principal component analysis (PCA) was applied to the samples. According to the results of this analysis, conclusions were made about the directional asymmetry and fluctuating asymmetry. When the directional asymmetry data in the plastron region were analyzed, PC1 explained 55.53% of the total variation, and PC2 explained 16.10% (Table 2). Figure 1 shows a significant narrowing in the caudal part of the plastron region for PC1. This was explained by the mean directional asymmetry of all offspring samples, which was higher than zero (Figure 1; graphical plot). The directional asymmetry (DA) was also statistically significant according to the analysis (Table 6).
According to the fluctuating asymmetry analysis, 30.60% of the total variation for the plastron was explained by PC1 and 14.88% was explained by PC2 (Table 2). In the PC1 plot, the most prominent feature observed is the expansion of the left side and the narrowing of the right side in the caudal region of the animals. A constriction was observed on the right side of the animals. On the left side, an expansion proportional to the constriction was found. For both PC1 and PC2, adult and juvenile turtles showed a similar distribution (Figure 2). The Side effect of directional asymmetry (DA) was statistically significant (p < 0.0001; Table 6). This indicates that deviations from symmetry and shape variation on both sides reflect directional asymmetry. In other words, shape differences between the right and left sides can be considered an indicator of directional asymmetry. The Side effect denotes directional asymmetry, and the Individual ∗ Side effect denotes fluctuating asymmetry. This result means that directional asymmetry is independent of individual differences and is only associated with a shape variation between both sides of symmetry. However, the Ind ∗ Side interaction was not statistically significant (p = 0.9979; Table 6). As a result, no statistically significant difference was observed for fluctuating asymmetry.
The results of the MANOVA analysis for the plastron revealed that the individual value of the symmetrical component was statistically significant (p < 0.0001), which is similar to the findings for the carapace. This supports the results of other analyses. Directional asymmetry (DA) was also found to be significant according to the results of different studies.
According to the results of the regression analysis for DA, the centroid size explained 19.10% of the shape variation (R2 = 0.190954). This relation was statistically significant (p = 0.0001). The findings showed that plastron shape variation was significantly affected by centroid size. Accordingly, it can be considered that centroid size contributes to changes in plastron morphology during the transition to adulthood. In the analysis for FA, size explained 5.07% of the shape variation (R2 = 0.0507), and this relation was statistically significant (p = 0.0188). The effect of centroid size on FA was not as strong as for DA, but its impact was still observed.

3.3. Sex-Based Fluctuating Asymmetry

Sex-based fluctuating asymmetry among adult specimens was also analyzed to elucidate the differences between the adult and juvenile individuals examined in this study. For this purpose, principal component analysis was applied to the samples (Figure 3, Table 7).
The PCA results indicated that PC1 accounted for 30% of the total variation in the carapace and 34% of the variation in the plastron. The individuals exhibited similar distribution patterns. The carapace’s left side was wider in male specimens, while the right side was narrower. A comparable pattern of shape variation was also noted in the plastron, where the right side exhibited more significant asymmetry, whereas the left side displayed more constrained variation.
Among adult individuals, the carapace asymmetry aligned with the shape variations observed when juvenile specimens were included. However, in the plastron, the asymmetry further supported the previously identified shape variation between adult and juvenile individuals, particularly in the caudal and epiplastron regions.

4. Discussion

This study used size and shape analyses to compare adult and juvenile groups regarding directional asymmetry and fluctuating asymmetry. Geometric morphometric methods are preferred in this respect [31,32]. These methods are particularly advantageous for quantifying morphological variations and have broad applications in evolutionary and developmental studies. Moreover, this developmental investigation demonstrates that they facilitate the assessment of how environmental factors influence asymmetry [33,34].
Fluctuating asymmetry refers to deviations from perfect bilateral symmetry and is often used to indicate developmental instability [31,35,36]. It is influenced by genetic and environmental factors, making it a valuable tool for interpreting developmental imbalances [34]. Statistically, FA encompasses all heterogeneous factors affecting symmetry [35]. In contrast, directional asymmetry represents consistent, non-random differences between sides, eliminating inter-individual heterogeneity in FA through techniques such as mixture analysis [37,38].
The DA value for the carapace was statistically significant (Table 4), indicating that as turtles mature, the dorsal carapace undergoes notable shape changes. Specifically, the carapace enlarges as turtles grow from juveniles to adults, consistent with findings from Ewing [39]. This enlargement is likely linked to age-related developmental processes, reflecting stable directional trends.
Similarly, the DA value for the plastron was statistically significant, as revealed by ANOVA (Table 6). This finding may be attributed to the unique developmental processes of the plastron. Earlier studies suggested the presence of cartilaginous tissue in the plastron, but recent histological analyses confirmed that its ossification occurs intramembranously, classifying it as a dermal, integumental, or exoskeletal structure [40,41,42]. Periodic and seasonal factors, rather than age alone, may contribute to FA in such exoskeletal structures. Supporting this hypothesis, Kotegov demonstrated that FA in fish exoskeletons varied periodically with seasonal changes [43].
The consistent detection of directional asymmetry (DA) in both the carapace (Side: SS = 0.002, F = 2.61, p = 0.0143; Table 4) and plastron (Side: SS = 0.002, F = 4.10, p = 0.0005; Table 6) underscores a systematic left–right shape divergence that transcends individual variation, as evidenced by its statistical significance across multiple analyses (e.g., MANOVA, p < 0.0001). This DA, explaining 50.9% of carapace variation (PC1, Table 1) and 55.53% of plastron variation (PC1, Table 2), manifests as distinct morphological shifts—such as the wider cranial and mid-shell regions of the carapace and the narrowed caudal region of the plastron—suggesting an adaptive or developmental response to external pressures. The regression analyses further revealed that centroid size significantly influences DA, particularly in the plastron (R2 = 0.190954, p = 0.0001), where it accounts for 19.10% of the shape variation, compared to a weaker effect in the carapace (R2 = 0.0597, p = 0.0452). This disparity implies that size-related growth dynamics may have a more pronounced role in driving plastron asymmetry, potentially linked to ontogenetic transitions from juvenile to adult stages, as visualized in the PCA scatterplots (Figure 1). These findings suggest that DA could serve as a reliable morphological marker for investigating biomechanical or ecological influences on turtle shell development, warranting further exploration into its functional consequences.
Anatomical analysis revealed that the cranial regions of animals with DA were more prominent, correlating with growth. Triangulation points in the cranial part of the plastron, located in the epiplastron region, showed significant developmental changes [23]. The epiplastron region showed significant juvenile enlargement, likely linked to developmental growth during early stages. This finding is consistent with previous studies, where the broader epiplastron in juvenile turtles was attributed to growth toward peripheral ossification centers during juvenile development [44].
The observed enlargement of the caudal region in adults compared to juveniles is likely associated with the onset of sexual maturity and growth patterns. This region remains smaller in juveniles, reflecting the developmental stages before sexual maturation [45].
In contrast, the persistent absence of significant fluctuating asymmetry (FA) in both structures (carapace: Ind × Side, F = 0.41, p = 0.9935; plastron: Ind × Side, F = 0.37, p = 0.9979) highlights a high degree of developmental stability, despite PC1 explaining 26.8% and 30.6% of FA variation in the carapace and plastron, respectively (Table 1 and Table 2). The lack of statistical significance, even with the sex-based analysis showing subtle differences (e.g., wider left carapace in males, Table 7), suggests that random deviations in symmetry are either biologically minimal or obscured by measurement error (Residual: carapace MS = 0.0001; plastron MS = 0.00007). This stability is notable given the significant individual variation (carapace: F = 881.6, p < 0.0001; plastron: F = 98.64, p < 0.0001), which dominates shape differences (97.05% and 97.47% of variance, respectively). The low error values from the ANOVA (Table 3, Table 4, Table 5 and Table 6) indicate methodological reliability, but the subtle FA signal (e.g., SS = 0.009 for carapace) remained below the detection threshold, possibly due to insufficient sample power or landmark resolution. These results contrast with the robustness of DA and suggest that while systematic asymmetry reflects consistent external or intrinsic drivers, random asymmetry may require enhanced precision—such as additional replicates or landmarks—to uncover potential stress-related signatures in this population.

5. Conclusions

As a result of this study, it was observed that DA was statistically significant in juvenile and adult turtle shells. The presence of substantial directional asymmetry in both the carapace and plastron suggests a consistent asymmetry in both shells. Hence, it can be argued that turtle shells exhibit a pattern within the same species. Fluctuating asymmetry was not statistically significant. However, shape variations were observed in this type of asymmetry. According to these results, it can be said that although individual differences may not be significant, environmental and developmental factors could play a significant role.

Author Contributions

Conceptualization, E.O., T.S., S.D. and G.P.; methodology, İ.B.D. and T.S.; software, E.O. and İ.B.D.; validation, T.S., S.D. and G.P; formal analysis, E.O.; investigation, F.Y.; resources, S.D.; data curation, S.D. and T.S.; writing—original draft preparation, E.O.; writing—review and editing, T.S.; visualization, İ.B.D.; supervision, G.P.; project administration, E.O.; funding acquisition, F.Y. and T.S. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Institutional Review Board Statement

The animal study protocol was approved by the Ethics Committee of the Tirana Faculty of Veterinary Medicine (Decision No. 143/15.04.2020).

Informed Consent Statement

Not applicable.

Data Availability Statement

The data presented in this study are available upon request from the corresponding author (E.O.).

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Van Dijk, P.P.; Corti, C.; Mellado, V.P.; Cheylan, M. Testudo Hermanni. IUCN Red List. Threat. Species 2004, 8235, e.T21648A176604335. [Google Scholar]
  2. Werneburg, I. The cranial musculature of turtles. Palaeontol. Electron. 2011, 14, 15A-99p. [Google Scholar]
  3. Girling, S.J. Veterinary Nursing of Exotic Pets; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
  4. Duro, S.; Gündemir, O.; Sönmez, B.; Jashari, T.; Szara, T.; Pazvant, G.; Kambo, A. A different perspective on sex dimorphism in the adult Hermann’s turtle: Geometric morphometry. Zool. Stud. 2021, 60, e9. [Google Scholar]
  5. Hadžiomerović, N.; Gundemir, O.; Tandir, F.; Avdić, R.; Katica, M. Geometric and morphometric analysis of the auditory ossicles in the red fox (Vulpes vulpes). Animals 2023, 13, 1230. [Google Scholar] [CrossRef] [PubMed]
  6. Manuta, N.; Çakar, B.; Gündemir, O.; Spataru, M.C. Shape and Size Variations of Distal Phalanges in Cattle. Animals 2024, 14, 194. [Google Scholar] [CrossRef]
  7. Gündemir, O.; Szara, T. Morphological patterns of the European bison (Bison bonasus) skull. Sci. Rep. 2025, 15, 1418. [Google Scholar] [CrossRef]
  8. Batur, B.; Kiliçli, İ.B.; Yunus, H.A.; Şahin, S.; Gündemir, O.; Özen, D.; Bakici, C. Geometric morphometric analysis of plastinated brain sections using computer-based methods: Evaluating shrinkage and shape changes. Ann. Anat.-Anat. Anz. 2025, 257, 152351. [Google Scholar] [CrossRef] [PubMed]
  9. Ağaç, D.K.; Onuk, B.; Gündemir, O.; Kabak, M.; Manuta, N.; Çakar, B.; Janeczek, M.; Crampton, D.A.; Szara, T. Comparative Cranial Geometric Morphometrics among Wistar Albino, Sprague Dawley, and WAG/Rij Rat Strains. Animals 2024, 14, 1274. [Google Scholar] [CrossRef]
  10. Eravci Yalin, E.; Gündemir, O.; Günay, E.; Vatansever Çelik, E.C.; Duro, S.; Szara, T.; Blagojevic, M.; Sonmez, B.; Spataru, M.C. Carapace Morphology Variations in Captive Tortoises: Insights from Three-Dimensional Analysis. Animals 2024, 14, 2664. [Google Scholar] [CrossRef]
  11. Korkmazcan, A.; Ünal, B.; Bakıcı, C.; Gündemir, O. Exploring skull shape variation and allometry across different chicken breeds. Ank. Üniv. Vet. Fakültesi Derg. 2025, 72, 1–7. [Google Scholar] [CrossRef]
  12. Slice, D.E. Geometric morphometrics. Annu. Rev. Anthropol. 2007, 36, 261–281. [Google Scholar] [CrossRef]
  13. Gündemir, O.; Özkan, E.; Dayan, M.O.; Aydoğdu, S. Sexual analysis in Turkey (Meleagris gallopavo) neurocranium using geometric morphometric methods. Turk. J. Vet. Anim. Sci. 2020, 44, 681–687. [Google Scholar]
  14. Gündemir, O. Shape analysis of fossa masseterica and processus coronoideus in domestic cats (Felis catus) and domestic dogs (Canis familiaris). Anat. Histol. Embryol. 2023, 52, 899–906. [Google Scholar] [PubMed]
  15. Savriama, Y. A step-by-step guide for geometric morphometrics of floral symmetry. Front. Plant Sci. 2018, 9, 1433. [Google Scholar]
  16. Siddiq, A.B.; Casanova, P.M.P.; Öncü, Ö.E.; Kar, H.; Onar, V. High level of fluctuating asymmetry in the Byzantine dogs from the Theodosius Harbor, Istanbul, Turkey. Turk. J. Vet. Anim. Sci. 2021, 45, 248–256. [Google Scholar]
  17. Klingenberg, C.P. A developmental perspective on developmental instability: Theory, models and mechanisms. Dev. Instab. Causes Conseq. 2003, 1, 14–34. [Google Scholar]
  18. Gündemir, O.; Michaud, M.; Altundağ, Y.; Karabağlı, M.; Onar, V.; Crampton, D. Chewing asymmetry in dogs: Exploring the importance of the fossa masseterica and first molar teeth morphology. Anat. Histol. Embryol. 2024, 53, e13050. [Google Scholar]
  19. Palmer, A.R. Antisymmetry. In Variation; Academic Press: Cambridge, UK, 2005; p. 359-XXIV. [Google Scholar]
  20. Bertolero, A.; Carretero, M.; Llorente, G. An assessment of the reliability of growth rings counts for age determination in the Hermann’s Tortoise Testudo hermanni. Amphib.-Reptil. 2005, 26, 17–23. [Google Scholar]
  21. Rohlf, F.J. TpsUtil Program Version 1.79. Ecology & Evolution; SUNY at Stone Brook: Stone Brook, NY, USA, 2019. [Google Scholar]
  22. Rohlf, F.J. The Tps Series of Software. Hystrix 2015, 26, 9–12. [Google Scholar]
  23. Moustakas-Verho, J.E.; Cebra-Thomas, J.; Gilbert, S.F. Patterning of the turtle shell. Curr. Opin. Genet. Dev. 2017, 45, 124–131. [Google Scholar]
  24. Boz, İ.; Manuta, N.; Özkan, E.; Kahvecioğlu, O.; Pazvant, G.; Ince, N.G.; Kahvecioglu, O.; Ozkan, E.; Manuta, N.; Gundemir, O. Geometric morphometry in veterinary anatomy. Veterinaria 2003, 72, 15–27. [Google Scholar]
  25. Klingenberg, C.P.; Barluenga, M.; Meyer, A. Shape analysis of symmetric structures: Quantifying variation among individuals and asymmetry. Evolution 2022, 56, 1909–1920. [Google Scholar]
  26. Klingenberg, C.P. MorphoJ: An integrated software package for geometric morphometrics. Mol. Ecol. Resour. 2011, 11, 353–357. [Google Scholar] [PubMed]
  27. Jojić, V.; Blagojević, J.; Vujošević, M. B chromosomes and cranial variability in yellow-necked field mice (Apodemus flavicollis). J. Mammal. 2011, 92, 396–406. [Google Scholar] [CrossRef]
  28. Valenzuela, N.; Adams, D.C.; Bowden, R.M.; Gauger, A.C. Geometric morphometric sex estimation for hatchling turtles: A powerful alternative for detecting subtle sexual shape dimorphism. Copeia 2004, 2004, 735–742. [Google Scholar]
  29. Kircher, L.; Wyneken, J. Sex Estimation by Geometric Morphometric Analysis of Loggerhead (Caretta caretta) Sea Turtle Hatchlings. Mar. Turt. Newsl. 2017, 154, 12–15. [Google Scholar]
  30. Sönmez, B.; Bağda, E.; Candan, O.; Yilmaz, H.E. Sex determination in green turtle hatchlings: Geometric morphometry and molecular sex markers. Nat. Eng. Sci. 2019, 4, 42–54. [Google Scholar]
  31. Parés Casanova, P.M.; Cardoner, M.; Raüll, C.; Martínez Silvestre, A. Plastral asymmetries in young European Pond Turtles, Emys orbicularis (Linnaeus 1758). Reptiles Amphib. 2020, 27, 496–498. [Google Scholar]
  32. Parés-Casanova, P.M. Directional asymmetry in the yellow-bellied slider turtles (Trachemys scripta scripta) (Schoepff 1792). Herpetol. Notes 2020, 13, 587–592. [Google Scholar]
  33. Palmer, A.R. Waltzing with asymmetry. BioScience 1996, 46, 518–532. [Google Scholar]
  34. Cherepanov, G.O. Patterns of scute development in turtle shell: Symmetry and asymmetry. Paleontol. J. 2014, 48, 1275–1283. [Google Scholar]
  35. González-Ruiz, J.M.; Pérez-Núñez, M.I.; García-Alfaro, M.D.; Bastir, M. How can directional and fluctuating asymmetry help in the prognosis of scoliosis along the course of the condition? J. Anat. 2021, 239, 1400–1408. [Google Scholar]
  36. Laia, R.C.; Pinto, M.P.; Menezes, V.A.; Rocha, C.F.D. Asymmetry in reptiles: What do we know so far? Springer Sci. Rev. 2015, 3, 13–26. [Google Scholar]
  37. Dongen, V.; Lens; Molenberghs. Mixture analysis of asymmetry: Modelling directional asymmetry, antisymmetry and heterogeneity in fluctuating asymmetry. Ecol. Lett. 1999, 2, 387–396. [Google Scholar]
  38. Graham, J.H.; Freeman, D.C.; Emlen, J.M. Antisymmetry, directional asymmetry, and dynamic morphogenesis. In Proceedings of the Developmental Instability: Its Origins and Evolutionary Implications: Proceedings of the International Conference on Developmental Instability: Its Origins and Evolutionary Implications, Tempe, AZ, USA, 14–15 June 1993; pp. 123–139. [Google Scholar]
  39. Ewing, H.E. Growth in the eastern box-turtle, with special reference to the dermal shields of the carapace. Copeia 1939, 1939, 87–92. [Google Scholar]
  40. Rathke, H. Über die Entwicklung der Schildkröten; Friederich Vieweg und Sohn: Braunschweig, Germany, 1848. [Google Scholar]
  41. Zangerl, R. The turtle shell. In The Biology of the Reptilia; Gans, C., Bellairs, A.d’A., Eds.; Academic Press: New York, NY, USA, 1969; Volume 1, pp. 311–319. [Google Scholar]
  42. Cebra-Thomas, J.A.; Betters, E.; Yin, M.; Plafkin, C.; McDow, K.; Gilbert, S.F. Evidence that a late-emerging population of trunk neural crest cells forms the plastron bones in the turtle Trachemys scripta. Evol. Dev. 2007, 9, 267–277. [Google Scholar]
  43. Kotegov, B.G. Fluctuating Asymmetry of the Traits of the Seismosensory System of the Roach Rutilus rutilus (L.) (Cyprinidae, Pisces) and the European Perch Perca fluviatilis L. (Percidae, Pisces) in Small Ponds with Various Water Mineralization. Biol. Bull. 2020, 47, 1372–1377. [Google Scholar]
  44. Gilbert, S.F.; Loredo, G.A.; Brukman, A.; Burke, A.C. Morphogenesis of the turtle shell: The development of a novel structure in tetrapod evolution. Evol. Dev. 2001, 3, 47–58. [Google Scholar]
  45. Ishihara, T.; Kamezaki, N. Size at maturity and tail elongation of loggerhead turtles (Caretta caretta) in the North Pacific. Chelonian Conserv. Biol. 2011, 10, 281–287. [Google Scholar]
Diversity 17 00241 g001
Figure 1. Principal component scatterplots and wireframe warp plots of the carapace and plastron for directional asymmetry. Above: Graphical plots displaying confidence ellipses for means; juveniles are represented by light green dots, and adults are represented by dark green dots. Below: Wireframe warp plots; brown outlines represent the mean shape configuration, while red outlines indicate shape changes associated with the positive ends of the PC1 axes).
Figure 1. Principal component scatterplots and wireframe warp plots of the carapace and plastron for directional asymmetry. Above: Graphical plots displaying confidence ellipses for means; juveniles are represented by light green dots, and adults are represented by dark green dots. Below: Wireframe warp plots; brown outlines represent the mean shape configuration, while red outlines indicate shape changes associated with the positive ends of the PC1 axes).
Diversity 17 00241 g001
Diversity 17 00241 g002
Figure 2. Principal component scatterplots and wireframe warp plots of carapace and plastron for fluctuating asymmetry. Above: Graphical plots showing confidence ellipses for means; juveniles are represented by light green dots, and adults are represented by dark green dots. Below: Wireframe warp plots; brown outlines represent the mean shape configuration, while red outlines indicate shape changes associated with the positive ends of the PC1 axes.
Figure 2. Principal component scatterplots and wireframe warp plots of carapace and plastron for fluctuating asymmetry. Above: Graphical plots showing confidence ellipses for means; juveniles are represented by light green dots, and adults are represented by dark green dots. Below: Wireframe warp plots; brown outlines represent the mean shape configuration, while red outlines indicate shape changes associated with the positive ends of the PC1 axes.
Diversity 17 00241 g002
Diversity 17 00241 g003
Figure 3. Principal component scatterplots and wireframe warp plots of carapace and plastron for fluctuating asymmetry. Above: Graphical plot with confidence ellipses for means; females are represented by red dots, and males by dark blue dots. Below: Wireframe warp plots; grey outlines represent the mean shape configuration, while blue outlines indicate shape changes associated with the positive ends of the PC1 axes.
Figure 3. Principal component scatterplots and wireframe warp plots of carapace and plastron for fluctuating asymmetry. Above: Graphical plot with confidence ellipses for means; females are represented by red dots, and males by dark blue dots. Below: Wireframe warp plots; grey outlines represent the mean shape configuration, while blue outlines indicate shape changes associated with the positive ends of the PC1 axes.
Diversity 17 00241 g003
Table 1. Explanation of the total variation of the first five principal components based on principal component analysis results (carapace).
Table 1. Explanation of the total variation of the first five principal components based on principal component analysis results (carapace).
Principal ComponentDirectional Asymmetry (DA)Fluctuating Asymmetry (FA)
Eigenvalues% VarianceEigenvalues% Variance
10.0027044650.9140.0001784926.802
20.0009747118.3500.0000722810.854
30.000526229.9070.000059218.891
40.000353306.6510.000046316.954
50.000138922.6150.000041036.161
Table 2. Explanation of the total variation of the first five principal components based on principal component analysis results (plastron).
Table 2. Explanation of the total variation of the first five principal components based on principal component analysis results (plastron).
Principal ComponentDirectional Asymmetry (DA)Fluctuating Asymmetry (FA)
Eigenvalues% VarianceEigenvalues% Variance
10.0022113155.530.0001657730.60
20.0006412416.10 0.0000806414.88
30.000196104.920.0000581110.72
40.000172954.340.000043508.03
50.000140853.530.000033886.25
Table 3. The results of ANOVA for centroid size (carapace).
Table 3. The results of ANOVA for centroid size (carapace).
EffectSSMSFp (Param.)
Individual428,967.063008428,967.0630087.290.0087
Residual4,059,101.55628858,827.558787
Table 4. The results of ANOVA for shape (carapace).
Table 4. The results of ANOVA for shape (carapace).
EffectSSMSFp (Param.)
Individual0.0760.0034881.6<0.0001
Side0.0020.00012.610.0143
Ind ∗ Side0.0090.000040.410.9935
Residual0.320.0001
Table 5. The results of ANOVA for centroid size (plastron).
Table 5. The results of ANOVA for centroid size (plastron).
EffectSSMSFp (Param.)
Individual1,193,844.4246011,193,844.42460117.20<0.0001
Residual4,789,357.07317069,410.972075
Table 6. The results of ANOVA for shape (plastron).
Table 6. The results of ANOVA for shape (plastron).
EffectSSMSFp (Param.)
Individual0.0650.002798.64<0.0001
Side0.0020.00014.100.0005
Ind ∗ Side0.00060.000020.370.9979
Residual0.2500.00007
Table 7. Explanation of the total variation in fluctuating asymmetry (FA) for the first five principal components based on principal component analysis results (female/male).
Table 7. Explanation of the total variation in fluctuating asymmetry (FA) for the first five principal components based on principal component analysis results (female/male).
Principal ComponentCarapacePlastron
Eigenvalues% VarianceEigenvalues% Variance
10.0002035330.860.0001156534.9
20.0000907013.750.0000416012.55
30.000058608.880.000024187.29
40.000048587.360.000023327.03
50.000042786.480.000019715.95
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Oktay, E.; Boz Doğan, İ.; Duro, S.; Pazvant, G.; Yiğit, F.; Szara, T. Geometric Morphometric Analysis of Adult and Juvenile Turtle Shells: Directional Asymmetry and Fluctuating Asymmetry. Diversity 2025, 17, 241. https://doi.org/10.3390/d17040241

AMA Style

Oktay E, Boz Doğan İ, Duro S, Pazvant G, Yiğit F, Szara T. Geometric Morphometric Analysis of Adult and Juvenile Turtle Shells: Directional Asymmetry and Fluctuating Asymmetry. Diversity. 2025; 17(4):241. https://doi.org/10.3390/d17040241

Chicago/Turabian Style

Oktay, Ece, İlayda Boz Doğan, Sokol Duro, Gülsün Pazvant, Funda Yiğit, and Tomasz Szara. 2025. "Geometric Morphometric Analysis of Adult and Juvenile Turtle Shells: Directional Asymmetry and Fluctuating Asymmetry" Diversity 17, no. 4: 241. https://doi.org/10.3390/d17040241

APA Style

Oktay, E., Boz Doğan, İ., Duro, S., Pazvant, G., Yiğit, F., & Szara, T. (2025). Geometric Morphometric Analysis of Adult and Juvenile Turtle Shells: Directional Asymmetry and Fluctuating Asymmetry. Diversity, 17(4), 241. https://doi.org/10.3390/d17040241

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop