State-specific Multi-reference Perturbation Theories with Relaxed Coefficients: Molecular Applications

Abstract

We present in this paper two new versions of Rayleigh-Schrödinger (RS) and the Brillouin-Wigner (BW) state-specific multi-reference perturbative theories (SS-MRPT) which stem from our state-specific multi-reference coupled-cluster formalism (SS-MRCC), developed with a complete active space (CAS). They are manifestly size-extensive and are designed to avoid intruders. The combining coefficients $c_{\mu }$ for the model functions ${\varphi }_{\mu }$ are completely relaxed and are obtained by diagonalizing an effective operator in the model space, one root of which is the target eigenvalue of interest. By invoking suitable partitioning of the hamiltonian, very convenient perturbative versions of the formalism in both the RS and the BW forms are developed for the second order energy. The unperturbed hamiltonians for these theories can be chosen to be of both Mφller-Plesset (MP) and Epstein-Nesbet (EN) type. However, we choose the corresponding Fock operator f_µ for each model function ${\varphi }_{\mu }$, whose diagonal elements are used to define the unperturbed hamiltonian in the MP partition. In the EN partition, we additionally include all the diagonal direct and exchange ladders. Our SS-MRPT thus utilizes a multi-partitioning strategy. Illustrative numerical applications are presented for potential energy surfaces (PES) of the ground (${}^1\sum ^{+}$) and the first delta (${}^1\mathsf{\Delta }$) states of ${\mathrm{CH}}^{+}$ which possess pronounced multi-reference character. Comparison of the results with the corresponding full CI values indicates the efficacy of our formalisms.

1 Introduction

One of the prime challenges in quantum chemistry lies in the development of formally rigorous models capable of reliable computations of potential energy surfaces (PES) of systems of arbitrary complexity and generality. Despite impressive methodological developments in correlated theories of electronic structure over the past two decades, major bottlenecks such as proper maintenance of size-consistency over a wide range of geometries, keeping the wavefunction of consistently good quality in the regions of real and/or avoided curve-crossings and formulating general strategies to bypass intruders have posed rather non-trivial theoretical problems. The effective hamiltonian based multi-reference (MR) methods [1,2,3,4] are often unsuitable for PES studies due to the perennial intruder state problem [5], though the intruders could be bypassed to a large extent at certain specific geometries by utilizing an incomplete model spaces (IMS) [6,7,8]. An inherently superior approach seems to be to work with a multi-determinantal reference space and to correlate only the target state of interest. Such strategies are generally referred to as the state-specific (SS) MR methods in the modern quantum chemical parlance.

Recently we have developed a rigorously size-consistent state-specific multi-reference coupled-cluster (SS-MRCC) theory [9,10] involving a CAS, which is designed to bypass the intruder problem. The pilot numerical applications of the formalism gave very encouraging results for electronic states possessing varying degrees of quasi-degeneracy and avoided crossings [10,11], which indicate its efficacy and viability. We also demonstrated that the SS-MRCC theory is quite rich in its structure in that it embodies in a natural manner some underlying Rayleigh-Schrödinger (RS) and Brillouin-Wigner (BW) type perturbation expansion with robust denominators, depending on the expansion strategy. The perturbative realizations of the formalism are quite worthwhile to explore, since any possible low order perturbation expansion – which captures such essence of the parent SS-MRCC as size-consistency and avoidance of intruders without sacrificing its accuracy significantly – will turn out to be potentially attractive in terms of the applicability to bigger systems. We have in fact recently suggested a specific way to generate such state-specific multi-reference perturbation theories (SS-MRPT) [12] and demonstrated their usefulness with some preliminary applications [11,13]. This mode of formulation has the limitation that only a very specific partitioning of the hamiltonian H could be supported for a consistent development. In this paper, we present an alternative viewpoint, which lends flexibility in the sense that several different partitionings can be used in formulating the perturbative versions. The objective of the this paper is to provide an account of the various facets of both the RS and BW versions of the new SS-MRPT, with several different partitionings of the hamiltonian. We also present numerical applications to typical multi-reference electronic states studied individually in a state-specific manner. We will discuss the first order RS and BW perturbative expansions of the wavefunction of our SS-MRCC formalism, which provides the second order energy. As a significant departure from the currently popular perturbative methods starting from a CAS, such as the CASPT2 [14,15], our formulation is intrinsically flexible in that it is designed to handle relaxed coefficients of the reference function. However, we can allow frozen coefficients as well in our formalism, and this feature can be utilized to explore the extent of accuracy gained if the coefficients are relaxed.

Successful implementation of all the traditional multi-reference many-body perturbation theories (MR-MBPT), which were developed within the effective hamiltonian framework [1], was always seriously affected due to the presence of the perennial intruder problem [5,16]. The zeroth-order energies appearing in the denominator of RS resolvent makes it explicit how the series becomes ill-conditioned if any of the virtual functions become quasi-degenerate in energy with any of the model functions. This drawback of the RS version of MR-MBPT is apparently not present in the corresponding BW type of resolvent, since the perturbed total energies figure in the denominators. Unfortunately, this has the disadvantage that a straightforward formulation leads to manifestly size-inextensive formalism, even for a CAS. The more sophisticated BW version developed by Bloch and Horowitz [17] scales somewhat better in the sense that it generates the shift of energy relative to the closed shell core, so that the error scales as number of valence occupancies. It is nevertheless still not fully extensive. The need to formulate comprehensive perturbative formalisms which can generate a size-consistent expansion of energy yet avoiding intruders was thus very much warranted.

Two fruitful avenues have been explored in recent times towards the realization of this goal. In one, an attempt is made to develop theories based on an (N × N) MR model space, but instead of targeting all the N eigenstates only a subset of them is targeted. The model space is partitioned into two subspaces, primary and secondary, where the latter may have energetic overlap with the virtual space. The idea is to define a pseudo-wave operator which acts on the entire model space, but generates exact states which are equal in number to the dimension of the primary model subspace and are dominated by the model functions spanning this subspace. This approach was advocated by Kirtman [18], but it was developed fully by Malrieu et al. [19] in the perturbative context, who termed this the intermediate hamiltonian (IH) approach. There have been important recent developments by Hoffmann [20], and Khait and Hoffmann [21] in this context. Coupled-cluster based IH formalisms [22,23,24], which are size-extensive, were also developed.

Another approach to circumvent the difficulty of intruders is to abandon the idea of partitioning of the CAS into primary and secondary subspaces, and define a wave operator which acts on just one reference function, which is a linear combination of all the functions of the CAS. The emphasis in this approach is to develop a state-specific theory, targeting only one state, rather than several states at the same time of the IH approach. An important advantage then is that it is not necessary for the exact function to be dominated by only certain model functions (which are taken to form the primary subspace in the IH methods).

There are various pro and contra issues for the different SS-MR based perturbation theories, which shape their numerical demands. There have been two distinct courses of development of the SS-MR theories. In one, to be hereafter called the frozen coefficients variety, the coefficients of the model functions forming the initial reference function are fixed by a prior diagonalization in the model space, and they are not revised or updated as a consequence of mixing with the virtual functions [14,15,25,26,27,28,29]. In another approach, the combining coefficients are iteratively updated, which lends an intrinsic accuracy to the perturbed functions [12,13,19,20,21,30]. We will henceforth call them as belonging to the relaxed coefficients variety.

We discuss the MRPTs with frozen coefficients first. There are usually two ways in which the virtual functions are handled. One way is to generate mutually non-orthogonal and linearly dependent set of functions obtained by the action of elementary excitation operators on the CAS-function, and selecting the linearly independent set by a Gram-Schmidt singular value decomposition procedure [14,15,25]. The hamiltonian matrix in the space of the virtual functions in this approach is non-diagonal, thus necessitating an implicit iterative inversion of a large matrix. Moreover, it requires the storage of upto four-body active densities, thus making it computationally expensive. There is, however, an intrinsic accuracy in the approach, since the projection manifold in the virtual space uses very elaborate multi-configuration functions.

There are also some hybrid solution strategies like the one suggested by Werner [31], where the double excitations are chosen as the composite functions as obtained by the first procedure, while the single excitations are taken as the singly excited determinants or CSF’s with respect to the model determinants.

It should be mentioned here that there are non-perturbative state-specific methods as well with frozen coefficients [9,32], which are explicitly size-extensive.

In the other strategy [26,27], the virtual functions used for projections are simple determinants or CSFs with given orbital occupancies, which results in the corresponding matrix in the space of virtual functions being diagonal. This lends a simpler structure to the working equations. In both these approaches, the functions used for projections to the virtual manifold are linearly independent.

The various CAS-based perturbative methods can also differ in their choice of unperturbed hamiltonian H₀, apart from the mode of representing the reference function relaxed or unrelaxed with respect to the coefficients. The original CASPT [14] was formulated with the generalized Fock operator as the unperturbed hamiltonian. This and the related formalisms [15,25,26,27,28,29] may be viewed as generalizations of MP perturbation theory to a CAS reference function. To take a better account of the orbital occupancy in non-singlet cases, a portion of the two-body terms of H has also been added to H₀, with varied degrees of success [29]. Obviously, the most elaborate choice of such H₀ would be to include the full two body active part of interaction, in addition to the standard generalized spin-average Fock operator [28]. Dyall [28] considered the entire active portion of H in H₀ in his development of CASPT. Some recent formulations [33] of effective hamiltonian perturbation theories have also used a multi-partitioning MP approach in the traditional effective hamiltonian framework. Also Heully et al. [34,35] discussed a partitioning of the hamiltonian where the size-consistency is imposed as an external constraint.

The various CASPT2 versions described above by and large avoids the size-consistency error, but not rigorously so. But, in order to reduce the artifacts stemming from the lack of relaxation of the coefficients, they advocate the use of rather large CAS, which may be fraught with intruders. For a critique along this line, see ref. [33]. As we will show in Sec.2.2, our SS-MRPT uses the best traits of the multi-partitioning strategy as well as of a rigorously size-extensive formulation.

We now discuss the SS-MR approaches with relaxed coefficients. There are both non-perturbative and perturbative developments. There have been three formalisms [9,10,30,36], based on this idea. One of them is our SS-MRCC formalism [9,10] on which the present SS-MRPT [12,13] are based. There are two other SS formalisms [30,36] which bear kinship with our SS-MRCC formulation. A common aspect of all these formalisms is the use of the same virtual determinant (or, the CSFs) to generate equations for excitation amplitudes for operators exciting from each model functions. This leads to redundancy of the excitation amplitudes. Malrieu and his group developed their SS-MR theories using a dressed-CI approach [30]. They eliminated the redundancy of their working equation in favor of some fractional parentage coefficients, and suggested expressions for determining them. The method is size-consistent with respect to fragment separations using localized orbitals. Our SS-MRCC theory, however, is invariant with respect to rotations with the active and virtual orbitals separately, and thus is size-consistent in either localized or delocalised orbitals. A SS Brillouin-Wigner type of MRCC approach, proposed by Hubač and co-workers [36], has a simpler structure compared to the more elaborate expression of our SS-MRCC theory. It is however not rigorously size-extensive or size-consistent. Hubač and co-workers [37], in a later development, sought to correct for the size-inextensivity by reverting to a RS type formulation. It was observed that the intruders would not show up if the inextensivity correction is incorporated by one iteration only. However, in general, this procedure does not ensure the removal of all the inextensive terms. Multiple iterations or the converged RS type of results will, however, unfortunately bring back the problem of potential intruders.

Since our SS-MRPT formalism is based on the relaxed coefficients approach, it might appear that this will involve very extensive computations because of the redundancy of the excitation amplitudes. As we will discuss in Sec.2.2, the additional effort due to redundancy can be largely minimized by an appropriate organization of the solution strategy.

2 Evolution of the RS and the BW state-specific perturbation theories from SS-MRCC theory with relaxed coefficients

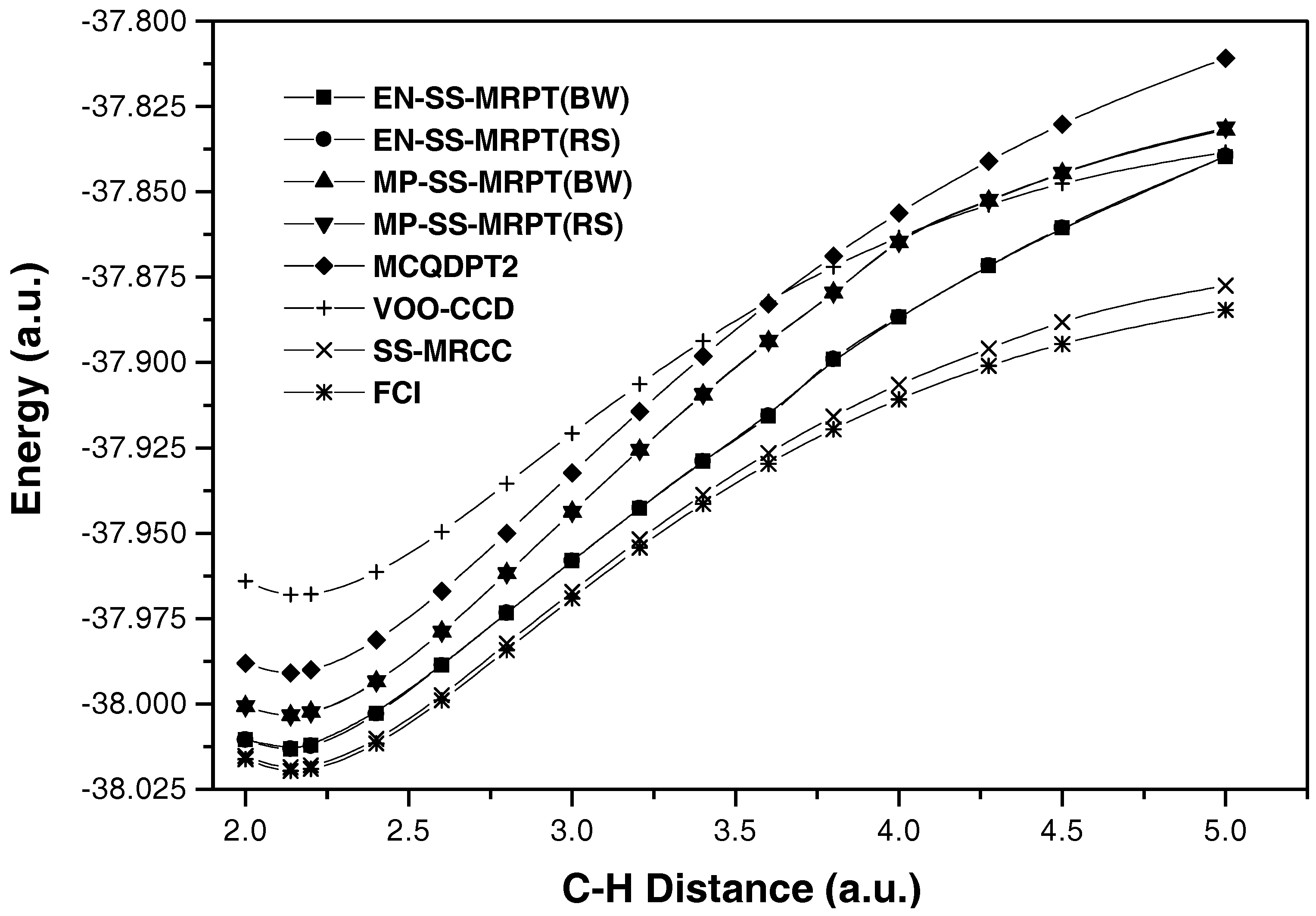
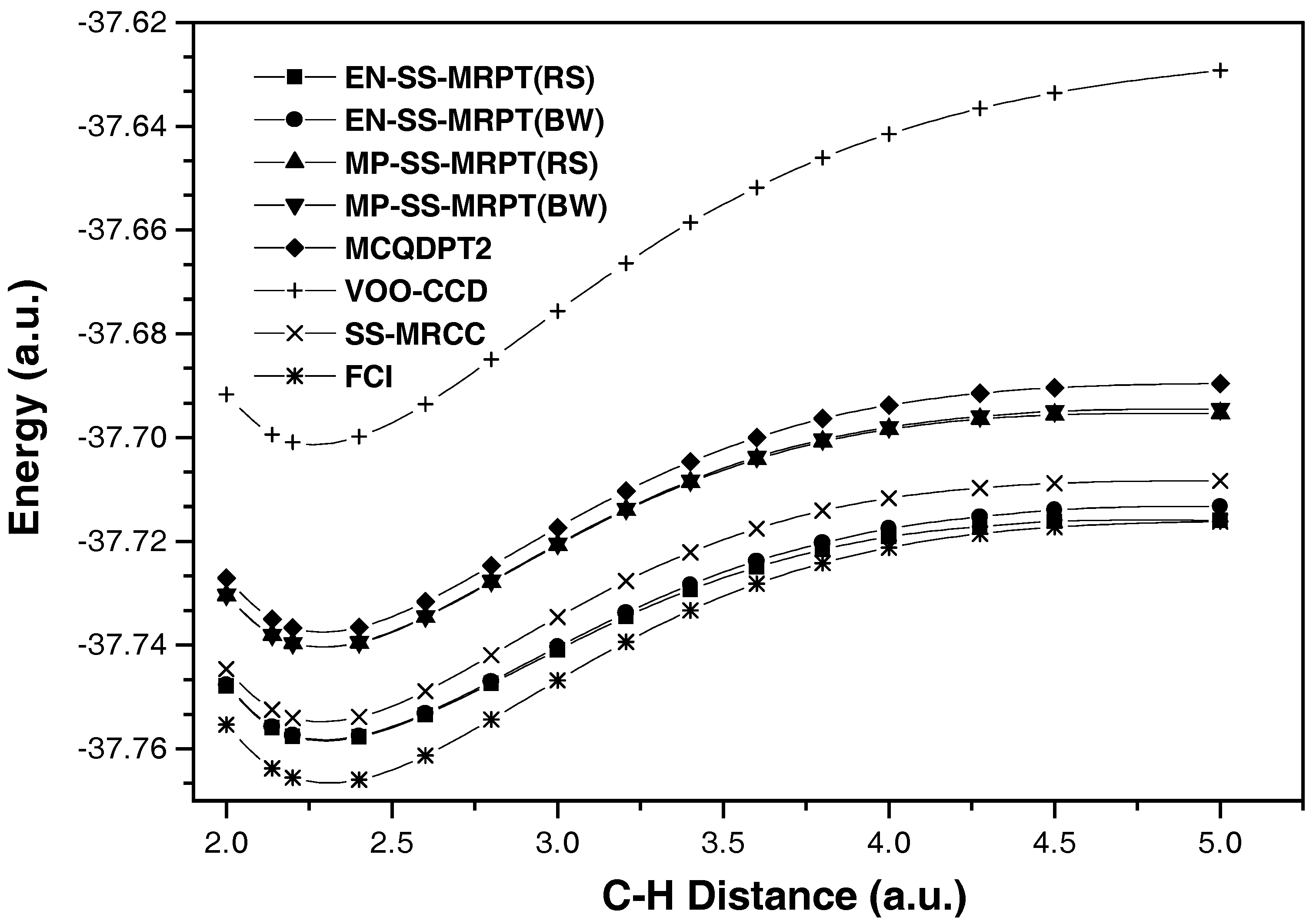
2.1 Preliminaries

We begin this section with a very brief summary of the essential ingredients of the SS-MRCC formalism. This will form the starting point for the perturbative approximations to follow. We write the reference function $|{\psi }_0\rangle$ as a combination of the reference determinants $|{\varphi }_{\mu }\rangle$ spanning the CAS:

$$|{\psi }_0\rangle =\sum _{\mu }|{\varphi }_{\mu }\rangle c_{\mu }$$

(1)

The exact function $|\psi \rangle$ is written as a cluster expansion involving cluster operators $T^{\mu }$ exciting from corresponding $|{\varphi }_{\mu }\rangle$’s:

$$|\psi \rangle =\sum _{\mu }\exp \left(T^{\mu }\right)|{\varphi }_{\mu }\rangle c_{\mu }$$

(2)

$|\psi \rangle$ is taken to satisfy the Schrödinger equation with the eigenvalue E:

$$H|\psi \rangle =H\sum _{\mu }\exp \left(T^{\mu }\right)|{\varphi }_{\mu }\rangle c_{\mu }=E|\psi \rangle$$

(3)

Each $T^{\mu }$ excites to all the virtual functions from ${\varphi }_{\mu }$ via the various n hole – n particle excitations, where the holes and particles are defined with respect to each ${\varphi }_{\mu }$. Such a cluster expansion Ansatz was first used by Jeziorski and Monkhorst in the context of the effective hamiltonian based state-universal multi-reference coupled-cluster (SU-MRCC) theory [2] and has later been exploited in the state-specific formulations too [9,10,36]. Since each ${\varphi }_{\mu }$ has different sets of active orbitals, any specific core-to-particle excitation would lead to a different virtual determinant from each ${\varphi }_{\mu }$. This is, however, not so in general for excitations involving active orbitals. Thus, we would encounter redundancy of the cluster operators involving active orbitals. To determine all of them, we have to invoke suitable sufficiency conditions. One may imagine that sufficiency conditions introduce a great degree of arbitrariness in a formalism. This is, however, not so if we want to exploit the arbitrariness in our choice to satisfy our twin desirable goals: to ensure that intruders are absent and to guarantee size-extensivity. It has been found that there are only two choices which naturally lead to MRCC equations which generate manifestly connected cluster operators. One set is just the SU-MRCC theory of Jeziorski and Monkhorst [2], which is known to encounter intruders. The other is our SS-MRCC formalism [9,10]. We present below, without the detailed derivation, the form of the working equations for the cluster amplitudes:

$$\langle {\chi }_l|{\overline{H}}_{\mu }|{\varphi }_{\mu }\rangle c_{\mu }+\sum _{\nu }\langle {\chi }_l|\exp (-T^{\mu })\exp (T^{\nu })|{\varphi }_{\mu }\rangle {\tilde{H}}_{\mu \nu }{c}_{\nu }=0\forall l,\mu$$

(4)

where

$${\overline{H}}_{\mu }=\overline{H\exp (T^{\mu })}$$

(5)

and

$${\tilde{H}}_{\mu \nu }=\langle {\varphi }_{\mu }|{\overline{H}}_{\nu }|{\varphi }_{\nu }\rangle$$

(6)

The model space coefficients $\left\{c_{\mu }\right\}$ are determined from

$${\displaystyle \sum _{\nu }{\tilde{H}}_{\mu \nu }}{c}_{\nu }=E{c}_{\mu }$$

(7)

The sets $\left\{T_{\mu }\right\}$ and $\left\{c_{\mu }\right\}$ are coupled through eq. (4) and eq. (7). Solving these coupled set of equation gives us the cluster amplitudes and the converged coefficients from the diagonalization.

For the detailed derivation and the proof of the extensivity of the SS-MRCC theory we refer to our recent papers [9,10]. What is pertinent for us here is the identification of one of the essential arguments leading to extensivity, since this will form the guideline of the perturbative approximations to follow. Dividing eq. (4) through by $c_{\mu }$, we have

$$\langle {\chi }_l|{\overline{H}}_{\mu }|{\varphi }_{\mu }\rangle +\sum _{\nu }\langle {\chi }_l|\exp (-T^{\mu })\exp (T^{\nu })|{\varphi }_{\mu }\rangle {\tilde{H}}_{\mu \nu }{c}_{\nu }/c_{\mu }=0\forall l,\mu$$

(8)

The first term of eq. (8) above is manifestly extensive, while the connectivity property of the second term requires a careful treatment, since this involves a product of two matrix-elements and may not have terms with common orbital labels in the two factors. Using the Baker-Campbell-Hausdorff formula for the product of exponentials, the second term can be written as

$$\begin{array}{c}{\displaystyle \sum _{\nu }}\langle {\chi }_l|\exp (-T^{\mu })\exp (T^{\nu })|{\varphi }_{\mu }\rangle {\tilde{H}}_{\mu \nu }{c}_{\nu }/c_{\mu }={\displaystyle \sum _{\nu }}\langle {\chi }_l|(T^{\nu }-T^{\mu }+\frac{1}{2}\left[T^{\nu },T^{\mu }\right]\\ +\frac{1}{12}[[T^{\nu },T^{\mu }],T^{\mu }]-\frac{1}{12}[[T^{\nu },T^{\mu }],T^{\nu }]+\cdots )|{\varphi }_{\mu }\rangle {\tilde{H}}_{\mu \nu }{c}_{\nu }/c_{\mu }\end{array}$$

(9)

Now, the second factor in eq. (9), ${\tilde{H}}_{\mu \nu }{c}_{\nu }/c_{\mu }$ is labeled by all the active orbitals which distinguish the determinants ${\varphi }_{\mu }$ and ${\varphi }_{\nu }$, and the first factor $\langle {\chi }_l|(T^{\nu }-T^{\mu }+\frac{1}{2}\left[T^{\nu },T^{\mu }\right]+\frac{1}{12}[[T^{\nu },T^{\mu }],T^{\mu }]-\frac{1}{12}[[T^{\nu },T^{\mu }],T^{\nu }]+\cdots )|{\varphi }_{\mu }\rangle$ should contain terms with some of these distinguishing active orbitals in ${\tilde{H}}_{\mu \nu }{c}_{\nu }/c_{\mu }$ for extensivity. While it is straightforward to show that the commutators and the multiple commutators generated by the Baker-Campbell-Hausdorff formula do have active orbital labels with this property, the individual terms linear in $T^{\mu }$ and $T^{\nu }$ do not. In fact there are excitation operators involving orbitals different from those active orbitals distinguishing ${\varphi }_{\mu }$ and ${\varphi }_{\nu }$. It was proved by us [9,10] that the term $\langle {\chi }_l|(T^{\nu }-T^{\mu })|{\varphi }_{\mu }\rangle$ containing the difference $(T^{\nu }-T^{\mu })$ is, however, labeled by some or all the active orbitals distinguishing ${\varphi }_{\mu }$ and ${\varphi }_{\nu }$ , and thus the two factors in the term in eq. (9) above have indeed some orbital labels in common. For any approximation of the SS-MRCC equations preserving the extensivity, it is mandatory to treat all the cluster amplitudes on an equal footing; otherwise the difference $(T^{\nu }-T^{\mu })$ will not be labeled by the active orbitals distinguishing ${\varphi }_{\mu }$ and ${\varphi }_{\nu }$. This aspect forms one guiding principle in our development of the perturbative approximations.

2.2 State-specific multi-reference perturbation theories: SS-MRPT

We wish to view the low order perturbative versions as a suite of quasi-linearized approximations of the SS-MRCC theory. Towards this end, we rewrite the leading terms of the cluster amplitude finding equations, eq. (4), of the parent SS-MRCC theory in the following form:

$$\begin{array}{c}[\langle {\chi }_l|H|{\varphi }_{\mu }\rangle ]c_{\mu }+\left[({\displaystyle \sum _m}\langle {\chi }_l|H|{\chi }_m\rangle -\langle {\varphi }_{\mu }|H|{\varphi }_{\mu }\rangle {\delta }_{lm})\langle {\chi }_m|T^{\mu }|{\varphi }_{\mu }\rangle \right]c_{\mu }\\ -\left[{\displaystyle \sum _{\nu }}\langle {\chi }_l|T^{\mu }|{\varphi }_{\mu }\rangle {\tilde{H}}_{\mu \nu }\right]c_{\nu }+\left[{\displaystyle \sum _{\nu }}\langle {\chi }_l|T^{\nu }|{\varphi }_{\mu }\rangle {\tilde{H}}_{\mu \nu }\right]c_{\nu }=0\forall l,\mu \end{array}$$

(10)

The four distinct terms in the above expression are separately shown under four brackets. The first term essentially corresponds to the coupling of a virtual function to a model function, and is akin to the numerator in a simple perturbation theory. The second term is a commutator of $T^{\mu }$ and $H$, and with $H_0$ approximating $H$ contributes an RS-like denominator of a traditional effective hamiltonian-based theory. The third and the fourth terms together perform two inter-related but distinct functions: (a) to convert the usual RS-like denominators into one containing the actual state energies, to bypass intruders – as befitting a state-specific theory, and (b) to supply counter-terms guaranteeing size-extensivity of the theory. The third term, in fact, supplies the term containing the state-energy, as shown below, while the fourth term, which couples the different model functions via the dressed hamiltonian ${\tilde{H}}_{\mu \nu }$ containing $T^{\nu }$, is, in conjunction with the third term, responsible to maintain size-extensivity.

Let us first briefly review our former perturbative formulations. This will help not only in emphasizing certain theoretical issues which any perturbative approximant has to satisfy, but also will serve to indicate where a more flexible approach can be taken. We recall at this point the observation noted earlier (after eq. (9)) that the term $\langle {\chi }_l|(T^{\nu }-T^{\mu })|{\varphi }_{\mu }\rangle {\tilde{H}}_{\mu \nu }{c}_{\nu }/c_{\mu }$ is connecte provided $T^{\nu }$ and $T^{\mu }$ are treated on the same footing. This aspect has a direct bearing on the structure of the RS and BW form of the working equations in any size-extensive perturbative formalism, viz. the last two terms in eq. (10) should be treated in the same approximation.

In the original formulation, we treated all the four terms consistently in the same partitioning scheme. This led to a rather inflexible approach, since this necessarily constrained us to use only a very specific partitioning strategy. Since it is natural to have the unperturbed state-energy $E_0$ appear in the denominator in the RS version, we approximated ${\tilde{H}}_{\mu \nu }$ by $H_{\mu \nu }$ in ${\sum }_{\nu }\langle {\chi }_l|T^{\mu (1)}|{\varphi }_{\mu }\rangle {\tilde{H}}_{\mu \nu }{c}_{\nu }$, since this leads to:

$${\displaystyle \sum _{\nu }}\langle {\chi }_l|T^{\mu (1)}|{\varphi }_{\mu }\rangle H_{\mu \nu }{c}_{\nu }=E_0\langle {\chi }_l|T^{\mu (1)}|{\varphi }_{\mu }\rangle c_{\mu }$$

(11)

To treat the term containing $\langle {\chi }_l|T^{\nu (1)}|{\varphi }_{\mu }\rangle$ on the same footing, it should thus appear multiplied by $H_{\mu \nu }{c}_{\nu }$ in the RS version. In a consistent perturbative approach, each term should be of first order in a first order RS formulation. Thus $H_{\mu \nu }$ in the last two terms in eq. (10) should be interpreted as $H_{0\mu \nu }$ . The partitioning of H in this approach is thus dictated by the necessity of keeping the full active portion of the hamiltonian in $H_0$. For the definition of $H_0$ for the virtual functions, it is natural to choose it as in the traditional EN partition. We thus advocated the following strategy in our earlier formulation: we partitioned the hamiltonian, H, into an unperturbed part, $H_0$, and a perturbation, V. We used a multi-partition strategy in that the unperturbed $H_{0\mu }$ was chosen as dependent on the ${\varphi }_{\mu }$ it acts upon, analogous to what was advocated in [33]. $H_0$ is a sum of ${\langle H\rangle }_{\mu }$, the diagonal part of the Fock operator, $f_{\mu }$, with respect to ${\varphi }_{\mu }$ as vacuum, when there is at least one inactive orbital, the whole active block of $f_{\mu }$, plus all the ladder operators of the two-body term which contains at least one inactive orbital and the entire active portion of the two-body term. Though this resembles the choice of Dyall [28] in the context of CASPT2, it is appropriately generalized in the context of multi-partitioning.

The eq. (10) was expanded in orders of perturbation to systematically generate the proper RS and BW versions of the perturbative expansion. While the RS version used E as a power series expansion, in the BW the E was kept unexpanded. We expanded each cluster operator $T^{\mu }$ that appear in the above equation as a power series in V. The same approximations were invoked while computing the third and the fourth terms. We should note here that $H_0$ in this formulation is non-diagonal in the active orbitals, which leads to coupling of various amplitudes of $T^{\mu }$ in the second term of eq. (10)

Instead of a strict perturbative analysis of all the four terms in the quasi linearized SS-MRCC theory, we want now to treat the third and the fourth terms of eq. (10) as something to be computed independently of the perturbative order. To motivate towards further development, we rewrite the third term explicitly in terms of the ‘state-energy’ $\epsilon$:

$$\begin{array}{c}[\langle {\chi }_l|H|{\varphi }_{\mu }\rangle ]c_{\mu }+[({\displaystyle \sum _m\langle {\chi }_l|H|{\chi }_m\rangle }-\langle {\varphi }_{\mu }|H|{\varphi }_{\mu }\rangle {\delta }_{lm})\langle {\chi }_m|T^{\mu }|{\varphi }_{\mu }\rangle ]c_{\mu }\\ -[\epsilon \langle {\chi }_l|T^{\mu }|{\varphi }_{\mu }\rangle ]c_{\mu }+[{\displaystyle \sum _{\nu }\langle {\chi }_l|T^{\nu }|{\varphi }_{\mu }\rangle }{\tilde{H}}_{\mu \nu }]c_{\nu }=0\forall l,\mu \end{array}$$

(12)

In the above expression, we treat $\epsilon$ as dependent on our choice of ${\tilde{H}}_{\mu \nu }$, depending on the RS or BW mode of formulation, but not on a specific partitioning strategy. We choose ${\tilde{H}}_{\mu \nu }$ simply as $H_{\mu \nu }$ for the RS version, or as the second order effective pseudo-operator, ${\tilde{H}}_{\mu \nu }^{(2)}$ for the BW version. The partitioning of H affects only the terms $\langle {\chi }_l|H_{\mu }|{\varphi }_{\nu }\rangle$ and $\langle {\chi }_l|[H_{\mu },T_{\mu }]|{\varphi }_{\nu }\rangle$. Since the partitioning of H and the treatment of the size-extensivity correction term are independent now, we can choose $H_0$ to be even a one-particle operator, reminiscent of a truly MP theory. We can also envision using an EN type of partition for H. In both the choices, $H_0$ is a diagonal operator, and this lends a simpler structure to our new perturbation theory. Expanding the first two terms of eq. (12) in orders of perturbation, and retaining only the terms of the first order, we have

$$[\langle {\chi }_l|V_{\mu }|{\varphi }_{\mu }\rangle ]c_{\mu }+[\langle {\chi }_l|[H_{0\mu },T_{\mu }]|{\varphi }_{\mu }\rangle ]c_{\mu }-[\epsilon \langle {\chi }_l|T^{\mu }|{\varphi }_{\mu }\rangle ]c_{\mu }+[{\displaystyle \sum _{\nu }\langle {\chi }_l}|T^{\nu }|{\varphi }_{\mu }\rangle {\tilde{H}}_{\mu \nu }]c_{\nu }=0\forall l,\mu$$

(13)

In our MP partition, we choose $H_{0\mu }$ to be a sum of the Fock operator for the function ${\varphi }_{\mu }$. This will correspond again to a multi-partitioning MP perturbation theory [33]. In the EN case, $H_0$ contains in addition all the diagonal direct and exchange ladders. In this paper we will explore the efficacy of the new multi-partitioned MP and EN type formulations only, as proposed above.

For actual applications, and to emphasize the organizational aspects of the theory, we rewrite the working equations, eq. (12), in the following form:

$$t_{\mu }^{l(1)}=\frac{H_{l\mu }+{\sum }_{\nu }^{\nu \ne \mu }\langle {\chi }_l|T^{\nu (1)}|{\varphi }_{\mu }\rangle {\tilde{H}}_{\mu \nu }(c_{\nu }/c_{\mu })}{[\epsilon -{\tilde{H}}_{\mu \mu }]+[H_{\mu \mu }^0-H_{ll}^0]}$$

(14)

We note that the only coupling between the various Ts are via the sum over ν appearing in the numerator of eq. (14) above. There is thus no coupling between the various excitation components in $T^{\mu }\mathrm{s}$, and the coupling is present with only those $T^{\nu }\mathrm{s}$ which lead to the same excitation as by the product of excitation operators for the specific $t_{\mu }^{l(1)}$ under consideration. This leads to a very attractive computational scheme, where we consider each type of excitation involving a specific set of orbitals, and compute all the T amplitudes for various µ with the same set of orbitals using eq. (14). The contributions of all these T amplitudes to the effective pseudo-operator $\tilde{H}$ are then computed, and a fresh set of excitations considered next. Thus, no T amplitudes need to be stored in this formulation, and the coupling is minimal.

It is interesting to compare the working equations of MRMP [26,27] and our SS-MRPT. Because of the sufficiency conditions stemming from the redundancy in our formulation, the projection on to the various virtual functions has to be considered for each model function. The minimal coupling in eq. (14) above take care of both the redundancy and size-extensivity. The solution of these equations require the storage of only those $t_{\mu }$ amplitudes for various µs which are labeled by the same spin-orbitals. In the first step of the iteration, if the couplings are ignored, we get almost the same working equations of the MRMP theory. Since in the MRMP formulation, the denominator for each ${\varphi }_{\mu }$ also requires a separate calculation, the extra work entailed in our theory as compared to the MRMP theory is not significantly large. Thus, by paying some extra computational price, we could ensure the rigorous size-extensivity of our formulation.

As emphasized earlier, for the RS theories $\epsilon =E_0$, corresponding to the CAS energy and the term ${\tilde{H}}_{\mu \nu }$ is just $H_{0\mu \nu }$. For the BW version $\epsilon =E^{(2)}$, the second-order energy obtained by diagonalizing ${\tilde{H}}_{\mu \nu }^{(2)}$. In both cases ${\tilde{H}}_{\mu \nu }^{(2)}$ is given by

$${\tilde{H}}_{\mu \nu }^{(2)}=H_{\mu \nu }+{\displaystyle \sum _l{H}_{\mu l}}{t}_{\nu }^{l(1)}$$

(15)

The second order energy $E^{(2)}$ is obtained from

$${\displaystyle \sum _{\nu }{\tilde{H}}_{\mu \nu }^{(2)}}{c}_{\nu }^{(2)}=E^{(2)}{c}_{\mu }^{(2)}$$

(16)

For the MP partitioning, the quantity $(H_{\mu \mu }^0-H_{ll}^0)$ would be the difference of the diagonal elements of $f_{\mu }$ containing the occupied and unoccupied orbitals of ${\varphi }_{\mu }$ involved in the excitation. For the EN partition, the corresponding term will involve, in addition, the diagonal direct and exchange ladders involving the same orbitals.

The eqs. (14), (15) and (16) are our principal working equations. It is noteworthy that in the SS-MRPT(RS) formalism the zeroth order coefficients $c_{\mu }^0$’s are used to evaluate the cluster operators in eq. (14), but the coefficients are relaxed during the computation of $E^{(2)}$, since this is obtained by diagonalization via eq. (16). On the other hand, in the BW context, the coefficients are iteratively updated.

The robustness of the energy denominators in the presence of intruders is quite manifest in our SS-MRPT formalisms: the denominators are of the form $[(\epsilon -H_{ll}^0)+(H_{\mu \mu }^0-{\tilde{H}}_{\mu \mu })]$. The difference $[H_{\mu \mu }^0-{\tilde{H}}_{\mu \mu }]$ is usually smaller than the term $[\epsilon -H_{ll}^0]$. The latter is never small as long as the unperturbed or the perturbed energy, $\epsilon$, is well-separated from the energies of the virtual functions. This holds true even if some of the $H_{\mu \mu }^0$’s are close to $H_{ll}^0$. The above arguments remain equally valid even in the case of EN partitioning. In this case, for the RS version, $H_{\mu \mu }^0=H_{\mu \mu }$, and ${\tilde{H}}_{\mu \mu }=H_{\mu \mu }$, and the denominator takes the simple form $[E_0-H_{ll}^0]$. Both the perturbation theories are thus intruder-free, and both are explicitly size-extensive. They are also size-consistent when we use orbitals localized on the separated fragments.

The above development has been in terms of spin-orbitals. The spin-adaptation of SS-MRCC or SS-MRPT for states of arbitrary spins is rather non-trivial, and requires quite extensive formal developments. We shall present the spin-adapted version in our future publications. For the CAS involving only closed-shell singlets, however, the spin-adaptation is very simple: we replace the spin-orbital indices by orbital indices, and assign a factor of 2 for each ‘loop’ (when the terms are expressed diagrammatically). These types of model spaces are the ones we will use in our applications, and will work with the spin-adapted expressions.

3 Size-consistency of the SS-MRPT formalisms: An illustrative example

Let us now illustrate size-consistency of the formalisms by demonstrating strict separability of the energies by considering an model problem of computing the interaction potential of two two-electron fragments. We show this for the EN version only, although the MP version has the same property. Let us denote the fragments as A and B. We use orbitals localized on the fragments. The fragment A has just one active orbital, which we denote as a. The fragment B has two active orbitals, which we denote as $b_1$ and $b_2$. The model space consists of ${\varphi }_1=a^2{b}_1^2,{\varphi }_2=a^2{b}_2^2$ in the localized orbital representation in infinite separation of the fragments. Obviously, we choose the fragment B to have a strong quasi-degeneracy to induce a MR character to our model. In view of the invariance of the formalisms under the localizing transformation of the active orbitals, our conclusions obtained from the localized representation will remain valid with delocalized orbitals as well. There are two types of excitations: a) excitation $a^2\to a_i^{\star 2}$ and b) excitations $b_1^2\to b_i^{\star 2}$ and $b_2^2\to b_i^{\star 2}$where $a_i^{\star }$ and $b_i^{\star }$ are the set of virtual orbitals in the fragments A and B respectively. Among all these, let us focus on just two virtual orbitals, viz. $a^{\star }$, and $b_1^{\star }$. In a), there is a pair of amplitudes $\langle a^{\star 2}|t^{1(1)}|a^2\rangle$ and $\langle a^{\star 2}|t^{2(1)}|a^2\rangle$. In b), there are two amplitudes $\langle b_1^{\star 2}|t^{1(1)}|b_1^2\rangle$ and $\langle b_1^{\star 2}|t^{2(1)}|b_2^2\rangle$, but there is only one virtual function $a^2{b}_1^{\star 2}$. However the sufficiency conditions allow us to generate precisely two equations for these two amplitudes.

We consider the separated fragment asymptotics. In the RS case, the unperturbed energy E₀ for the composite is obtained by diagonalizing the matrix $\langle {\varphi }_{\mu }|H|{\varphi }_{\nu }\rangle$ in the model space. In the separated limit, $E_0=E_{0A}+E_{0B}$ where $E_{0A}=\langle a^2|H|a^2\rangle$ and $E_{0B}$ is obtained by diagonalizing the hamiltonian of B in the space $b_1^2$ and $b_2^2$. For excitations to $a^{\star 2}{b}_1^2$ out of ${\varphi }_1$, the lowest order equation is

$$\begin{array}{l}\langle a^{\star 2}|H|a^2\rangle c_1^B+[\langle a^{\star 2}|H|a^{\star 2}\rangle -E_{0_A}]\langle a^{\star 2}|t^{1(1)}|a^2\rangle c_1^B-\langle a^{\star 2}|t^{1(1)}|a^2\rangle \langle b_1^2|H|b_2^2\rangle c_2^B\\ +\langle a^{\star 2}|t^{2(1)}|a^2\rangle \langle b_1^2|H|b_2^2\rangle c_2^B=0\end{array}$$

(17)

where we have explicitly used the properties of our H₀. For the excitation $a^2\to a^{\star 2}$ from ${\varphi }_2$, we likewise have

$$\begin{array}{c}\langle a^{\star 2}|H|a^2\rangle c_2^B+[\langle a^{\star 2}|H|a^{\star 2}\rangle -E_{0_A}]\langle a^{\star 2}|t^{2(1)}|a^2\rangle c_2^B-\langle a^{\star 2}|t^{2(1)}|a^2\rangle \langle b_2^2|H|b_1^2\rangle c_1^B\\ +\langle a^{\star 2}|t^{1(1)}|a^2\rangle \langle b_2^2|H|b_1^2\rangle c_1^B=0\end{array}$$

(18)

This leads to the solutions

$$\langle a^{\star 2}|t^{1(1)}|a^2\rangle =\frac{\langle a^{\star 2}|H|a^2\rangle }{(E_{0_A}-\langle a^{\star 2}|H|a^{\star 2}\rangle )}$$

(19)

$$\langle a^{\star 2}|t^{2(1)}|a^2\rangle =\frac{\langle a^{\star 2}|H|a^2\rangle }{(E_{0_A}-\langle a^{\star 2}|H|a^{\star 2}\rangle )}$$

(20)

and they are equal, as they should be, since the other fragment is far apart.

Now let us consider the situation b). In this case we have a redundancy, corresponding to the excitation to a virtual on B, viz. $b_1^{\star 2}$. For this, we have the following sufficiency condition for the excitation from $b_1^2$

$$\begin{array}{c}\langle b_1^{\star 2}|H|b_1^2\rangle c_1^B+[\langle b_1^{\star 2}|H|b_1^{\star 2}\rangle -\langle b_1^2|H|b_1^2\rangle ]\langle b_1^{\star 2}|t^{1(1)}|b_1^2\rangle c_1^B\\ -\langle b_1^{\star 2}|t^{1(1)}|b_1^2\rangle \langle b_1^2|H|b_2^2\rangle c_2^B=0\end{array}$$

(21)

leading to

$$\langle b_1^{\star 2}|t^{1(1)}|b_1^2\rangle =\frac{\langle b_1^{\star 2}|H|b_1^2\rangle }{(E_{0_B}-\langle b_1^{\star 2}|H|b_1^{\star 2}\rangle )}$$

(22)

and similarly for the excitation from $b_2^2$

$$\langle b_1^{\star 2}|t^{2(1)}|b_2^2\rangle =\frac{\langle b_1^{\star 2}|H|b_2^2\rangle }{(E_{0_B}-\langle b_1^{\star 2}|H|b_1^{\star 2}\rangle )}$$

(23)

This ensures separability of the total energy E as E = E_A + E_B There is an analogous situation for the BW case, with the corresponding fragment amplitudes emerging in the separated limit. The denominators in this case, however, have perturbed energies E⁽²⁾ for both composites and the fragments. The composite perturbative energies are additively separable into fragment energies in the asymptotic limit, since the pseudo-effective operator is an extensive operator.

We conclude this section by an analysis of a perturbative formulation of Heully et al. [35] where they attempted to correct for the size-inextensivity of a denominator that would appear in a naive state-specific perturbation theory. Starting with the second-order energy expression

$${\tilde{H}}_{\nu \mu }^{(2)}={\displaystyle \sum _l\frac{H_{\nu l}{H}_{lu}}{E_0-H_{ll}}}$$

(24)

the corresponding cluster-amplitude $t_{\mu }^l$ in eq. (24) given by

$$t_{\mu }^{l(1)}=\frac{H_{lu}}{E_0-H_{ll}}$$

(25)

The expression of ${\tilde{H}}_{\nu \mu }^{(2)}$ above will not separate correctly into non-interacting fragments A and B. Denoting the set of model functions on fragments A and B as $\{{\varphi }_{{\mu }_A}\}$ and $\{{\varphi }_{{\nu }_B}\}$the CAS functions for the composite will be spanned by $\{A[{\varphi }_{{\nu }_A}{\varphi }_{{\nu }_B}]\}$. The CAS energy E₀ separates correctly into fragments $E_{0_A}$ and $E_{0_B}$ in the non-interacting limit. $E_{0_A}$ and $E_{0_B}$ are respectively obtained by diagonalizing H in the space of functions $\{{\varphi }_{{\mu }_A}\}$ and $\{{\varphi }_{{\nu }_B}\}$. The coefficients $c_{{\mu }_A{\nu }_B}$ of the composite go over to the products $c_{{\mu }_A}{c}_{{\nu }_B}$ in the non-interacting limit. In this limit, where the fragment A excited from ${\mu }_A\to l_A$ and the other fragment B remaining unexcited in ${\nu }_B$ it is easy to show that the corresponding T amplitude goes over to

$$t_{\mu }^l\to t_{{\mu }_A{\nu }_B}^{l_A{\nu }_B}=\frac{{[H_A]}_{l_A{\mu }_A}}{E_{0_A}+E_{0_B}-H_{l_A{l}_A}-H_{{\nu }_B{\nu }_B}}$$

(26)

For a size-extensive theory this $T$ amplitude should in the limit behave just as $t_{{\mu }_A}^{l_A}$. This is not reached in eq. (26) above because of the B-dependent terms in the denominator. Heully et al. argued that one should replace the last term in the denominator by $E_{0_B}$ to effect the cancellation and concluded that the form for $t_{\mu }^l$ which ensures this is given by

$$t_{\mu }^{l(1)}=\frac{H_{l_{\mu }}}{E_0-E_{ll}-{\sum }_{\nu \ne \mu }^{\prime }{H}_{\mu \nu }(\frac{c_{\nu }}{c_{\mu }})}$$

(27)

where the prime indicates summation over only those ${\varphi }_{\nu }$’s for which the excitation $\mu \to l$ acting on ${\varphi }_{\nu }$ is non-zero. In fact, in the non-interacting limit the ${\varphi }_{\nu }$’s for which the excitations $t_{{\mu }_A}^{l_A}$ are non-zero, are the functions ${\varphi }_{{\mu }_A{\lambda }_B}$ with ${\lambda }_B\ne {\nu }_B$. In that case,

$$\begin{array}{ccc}\hfill t_{\mu }^l\to t_{{\mu }_A{\nu }_B}^{l_A{\nu }_B}& =& \frac{{[H_A]}_{l_A{\mu }_A}}{E_{0_A}+E_{0_B}-H_{l_A{l}_A}-H_{{\nu }_B{\nu }_B}-{\sum }_{{\lambda }_B\ne {\nu }_B}{H}_{{\mu }_A{\nu }_B,{\mu }_A{\lambda }_B}(\frac{c_{{\mu }_A{\lambda }_B}}{c_{{\mu }_A{\nu }_B}})}\hfill \\ \hfill & \equiv & \frac{{[H_A]}_{l_A{\mu }_A}}{E_{0_A}+E_{0_B}-H_{l_A{l}_A}-H_{{\nu }_B{\nu }_B}-{\sum }_{{\lambda }_B\ne {\nu }_B}{H}_{{\nu }_B{\lambda }_B}(\frac{c_{{\lambda }_B}}{c_{{\nu }_B}})}\hfill \end{array}$$

(28)

Using the relation

$$H_{{\nu }_B{\nu }_B}+{\displaystyle \sum _{{\lambda }_B\ne {\nu }_B}{H}_{{\nu }_B{\lambda }_B}}(c_{{\lambda }_B}/c_{{\nu }_B})\equiv {\displaystyle \sum _{{\lambda }_B}{H}_{{\nu }_B{\lambda }_B}}(c_{{\lambda }_B}/c_{{\nu }_B})=E_{0_B}$$

(29)

we have

$$t_{\mu }^l\to t_{{\mu }_A{\nu }_B}^{l_A{\nu }_B}=\frac{{[H_A]}_{l_A{\mu }_A}}{E_{0_A}-H_{l_A{l}_A}}\equiv t_{{\mu }_A}^{l_A}$$

(30)

as it should be. Thus Heully et al. advocated the form eq. (27) as the proper size-extensive expression for the cluster amplitudes.

In order to show the kinship of this theory with ours, we rewrite our RS expression, viz. eq. (14), with $\epsilon =E_0$, in the following manner:

$$t_{\mu }^{l\text{(1)}}=\frac{H_{l\mu }}{E_0-H_{ll}-{\sum }_{\nu \ne \mu }\frac{\langle {\chi }_l|T^{\nu (1)}|{\varphi }_{\mu }\rangle H_{\mu \nu }{c}_{\nu }}{\langle {\chi }_l|T^{\mu (1)}|{\varphi }_{\mu }\rangle c_{\mu }}}$$

(31)

For the same excitation ${\mu }_A{\nu }_B\to l_A{\nu }_B$, our expression reduces to

$$t_{\mu }^l\to t_{{\mu }_A{\nu }_B}^{l_A{\nu }_B}=\frac{{[H_A]}_{l_A{\mu }_A}}{E_{0_A}+E_{0_B}-H_{l_A{l}_A}-H_{{\nu }_B{\nu }_B}-\chi }$$

(32)

where $\chi$ is given by

$$\chi ={\displaystyle \sum _{{\lambda }_B\ne {\nu }_B}\frac{\langle {\chi }_{l_A}{\varphi }_{{\nu }_B}|T^{({\mu }_A{\lambda }_B)}|{\varphi }_{{\mu }_A{\nu }_B}\rangle H_{{\nu }_B{\lambda }_B}{c}_{{\lambda }_B}}{\langle {\chi }_{l_A}{\varphi }_{{\nu }_B}|T^{({\mu }_A{\nu }_B)}|{\varphi }_{{\mu }_A{\nu }_b}\rangle c_{{\nu }_B}}}$$

(33)

This differs from the expression ${\sum }_{{\lambda }_B\ne {\nu }_B}{H}_{{\nu }_B{\lambda }_B}(\frac{c_{{\lambda }_B}}{c_{{\nu }_B}})$ appearing in eq. (26) by the various ratios $\frac{\langle {\chi }_{l_A}{\varphi }_{{\nu }_B}|T^{({\mu }_A{\lambda }_B)}|{\varphi }_{{\mu }_A{\nu }_B}\rangle }{\langle {\chi }_{l_A}{\varphi }_{{\nu }_B}|T^{({\mu }_A{\nu }_B)}|{\varphi }_{{\mu }_A{\nu }_b}\rangle }$. In the non-interacting limit, the extensivity of our cluster amplitudes implies that all the cluster amplitudes involving ${\mu }_A\to l_A$ with the fragment B unexcited are equal to $t_{{\mu }_A}^{l_A}$ independent of ${\nu }_B:\chi ={\sum }_{{\lambda }_B\ne {\nu }_B}{H}_{{\nu }_B{\lambda }_B}(\frac{c_{{\lambda }_B}}{c_{{\nu }_B}})$ and the expression obtained by Heully et al. coincides with that of ours in this limit.

In the interacting situation, however, it is not physically sensible to assume that they would remain equal, and in fact, our theory would determine them self-consistently from the coupled set of perturbative equations. In contrast Heully et al. would still continue to treat these amplitudes equal even in the presence of interactions, which implies that their treatment amounts to the use of “anonymous parentage” approximation [38], where one equates the values of the cluster amplitudes inducing the same excitation irrespective of ${\varphi }_{\mu }$’s.

4 Applications

In our numerical applications we will consider a portion of the potential energy surface (PES) of the ground ${}^1\sum ^{+}$ and an excited ${}^1\mathsf{\Delta }$ states of CH⁺. They possess pronounced MR character and thus warrant an MR description to capture the non-dynamical correlation effect.

We use the RS and BW based SS-MRPT in both the MP and EN partitionings to study the PES of these states. The performance of our SS-MRPT using these partitionings have been assessed and compared with the corresponding results of the SS-MRCC theory, in the singles-doubles truncation scheme, and also the FCI values using the same basis, given in the paper of Krylov et al. [39] as the benchmark. The basis set comprises of the standard Dunning DZP basis functions [40] augmented with diffuse s and p functions on carbon and one diffuse s function on the hydrogen.

CH⁺ has a ground state electronic configuration of $1{\sigma }^{2}2{\sigma }^{2}3{\sigma }^2$ and a large non-dynamical correlation contribution originating from the $1{\sigma }^{2}2{\sigma }^{2}1{\pi }_x^2$ and $1{\sigma }^{2}2{\sigma }^{2}1{\pi }_y^2$ configurations, Z-axis being considered as the molecular axis. Due to symmetry reasons, the (3×3) model space spanned by these configurations is complete. Moreover, all the functions in the CAS are closed-shell singlets, allowing the use of our spin-adapted versions of the SS theories. The importance of the configurations $1{\sigma }^{2}2{\sigma }^{2}1{\pi }_x^2$ and $1{\sigma }^{2}2{\sigma }^{2}1{\pi }_y^2$ steadily increases with the increase in the C–H distance, and the importance of the virtual configurations arising out of single and double excitations from $1{\sigma }^{2}2{\sigma }^{2}3{\sigma }^2$ involving the low-lying ${\sigma }^{\star }$ and ${\pi }^{\star }$ orbitals becomes prominent. Our model space thus becomes somewhat inferior for large C–H separation, but we nevertheless use this model space to have only closed-shell configurations in our CAS. The ${}^1\mathsf{\Delta }$ state is dominated by the configurations $1{\sigma }^{2}2{\sigma }^{2}1{\pi }_x^2$ and $1{\sigma }^{2}2{\sigma }^{2}1{\pi }_y^2$, and our model space is quite adequate for its description. x y

We have employed the CAS-SCF orbitals for the lowest root of the (3×3) CAS for our treatment of the ground state ${}^1\sum ^{+}$, whereas for the first state ${}^1\mathsf{\Delta }$ – and excited state, we have used the CAS orbitals for the (2×2) CAS spanned by the two configurations $1{\sigma }^{2}2{\sigma }^{2}1{\pi }_x^2$ and $1{\sigma }^{2}2{\sigma }^{2}1{\pi }_y^2$ as our reference functions. The CAS spaces were kept the same throughout the PES for both the states.

We have displayed in Fig 1 and Fig 2 the PES for the ${}^1\sum ^{+}$ state and the ${}^1\mathsf{\Delta }$ state respectively, obtained with both MP and EN partitioning schemes using RS as well as BW versions of the new SS-MRPT, which we refer to as MP-SS-MRPT(RS) and MP-SS-MRPT(BW), and EN-SS-MRPT(RS) and EN-SS-MRPT(BW) respectively, for the two distinct partitioning schemes. For assessing the performance, the corresponding FCI results [39] with the same basis [40] have also been shown. Results from the parent SS-MRCC theory are also displayed to indicate the extent of correlation captured by its perturbative counterparts. We have also shown the results of the MCQDPT2 theory of Nakano [27] using the same CAS, and VOO-CCD values of Krylov et al. for both the ground as well as the ${}^1\mathsf{\Delta }$ state [39]. From the figures one could easily discern that our SS-MRPT theories, both RS and BW, using both MP and the EN perform very well. The EN partition works better for both RS and BW versions. The performance of the RS and BW methods is comparable with any given partition. For the ground state, the quality of the perturbative results go down in the large C–H distances, since here the (3×3) model space itself is not entirely adequate. We emphasize that this is no reflection of the limitation of our formalism – only an inadequacy of our choice of the model space in the present application. As mentioned above, we have kept our model space (3×3) to have a spin-free formulation just for convenience. The singles-doubles coupling involving the low-lying virtual orbitals becomes important at large C–H distance, which is a sort of Brueckner effect. This cannot be adequately captured by a first order theory such as SS-MRPT. The single reference Brueckner version VOO-CCD of Head-Gordon and co-workers [41] captures this effect in the sense that the relative quality of the results remains more or less similar throughout the PES, though the accuracy is much less as compared with those from SS-MRPT. It is interesting to note that the parent SS-MRCC, which captures the effects of singles in a non-perturbative manner captures the Brueckner effect quite adequately. This indicates that a viable strategy to include Brueckner effect will be to treat the low-lying singles and doubles in a non-perturbative manner, as in SS-MRCC, and treat the rest of excitations as in SS-MRPT. Such a development is in progress in our laboratory.

For the ${}^1\mathsf{\Delta }$ state, our model space is quite adequate, and very good performance of the SS-MRPT have been observed. Th SS-MRCC results are also quite good. The ${}^1\mathsf{\Delta }$ state was also studied by Krylov et al. [39] using the linear response version of the VOO-CCD. The excited states dominated by double excitations are not described well in any linear response theory because of

Figure 1. The ground state (${}^1\sum ^{+}$) PES of CH⁺

Figure 1. The ground state (${}^1\sum ^{+}$) PES of CH⁺

Figure 2. The first excited (${}^1\mathsf{\Delta }$) state PES of CH⁺

Figure 2. The first excited (${}^1\mathsf{\Delta }$) state PES of CH⁺

an insufficient inclusion of dynamical correlation and thus the PES of Krylov et al. [39] shows the deterioration of the quality at large C–H distance. We do not use linear response theories for this state here, and our SSMR theories remain very good throughout the PES.

As mentioned earlier, we have also displayed in the figures the MCQDPT2 results of Nakano [27], computed by us using the same model spaces with GAMESS code. The MCQDPT2 uses a single partitioning of the hamiltonian and chooses a generalized Fock operator for the entire CAS function in an multi-reference MP strategy. In contrast to our relaxed description, the MCQDPT2 uses a frozen coefficient description, and is thus inherently less flexible as compared to our approach. Its performance for the two states studied by us is not as good as SS-MRPT.

Since the computed PES using our SS-MR methods for both the ground and the first ${}^1\mathsf{\Delta }$ state of CH⁺ system are very close to the corresponding FCI results, it prompted us to compare the accuracy of the spectroscopic constants also using these PES. Using our SS-MRPT and SS-MRCC, we have calculated the spectroscopic constants, viz. the equilibrium distance $r_e$, the harmonic vibrational frequency ${\omega }_e$, the anharmonicity ${\omega }_e{x}_e$ the rotational constant $B_e$, the rovibronic coupling constant ${\alpha }_e$ and the centrifugal distortion constant ${\overline{D}}_e$ of ${\mathrm{CH}}^{+}$ via a Dunham analysis [42]. In Table 1 and Table 2, we provide respectively the values of these quantities for the ground as well as the first ${}^1\mathsf{\Delta }$ excited state of ${\mathrm{CH}}^{+}$ using the aforesaid suite of methods employing the same basis set, along with the FCI values. We also list the corresponding results obtained from VOO-CCD and MCQDPT2 methods. To have a better feeling of the magnitudes of the values, we provide the experimental values as well [43].

A comparison with the corresponding FCI values clearly demonstrates that the various versions of our state-specific perturbation theories along with their parent SS-MRCC serve as good theoretical models for the calculation of the various spectroscopic constants. The performance of VOO-CCD and MCQDPT2 are also good in this context.

Table 1. Spectroscopic constants for the ground electronic state of ${\mathrm{CH}}^{+}$.

Method	$r_e$	${\omega }_e$	${\omega }_e{x}_e$	${\overline{D}}_e$	$B_e$	${\alpha }_e$
EN-SS-MRPT(RS)	1.120	3006	64.9	1.33 × 10−3	14.436	0.466
EN-SS-MRPT(BW)	1.127	2846	63.4	1.40 × 10−3	14.167	0.471
MP-SS-MRPT(RS)	1.120	3092	63.2	1.23 × 10−3	14.341	0.439
MP-SS-MRPT(BW)	1.120	3118	59.7	1.21 × 10−3	14.340	0.411
MCQDPT2	1.132	2949	58.2	1.27 × 10−3	14.034	0.415
VOO-CCD	1.133	2910	62.6	1.30 × 10−3	14.021	0.451
SS-MRCC	1.132	2966	65.7	1.27 × 10−3	14.074	0.465
FCI	1.131	2921	64.4	1.31 × 10−3	14.072	0.463
Experiment	1.131	2740	64.0	1.40 × 10−3	14.177	0.492

$r_e$ in $\mathrm{\AA }$, and all other quantities in cm⁻¹. Experimental data from Ref. [43]

Table 1. Spectroscopic constants for the ground electronic state of ${\mathrm{CH}}^{+}$.

Method	$r_e$	${\omega }_e$	${\omega }_e{x}_e$	${\overline{D}}_e$	$B_e$	${\alpha }_e$
EN-SS-MRPT(RS)	1.120	3006	64.9	1.33 × 10−3	14.436	0.466
EN-SS-MRPT(BW)	1.127	2846	63.4	1.40 × 10−3	14.167	0.471
MP-SS-MRPT(RS)	1.120	3092	63.2	1.23 × 10−3	14.341	0.439
MP-SS-MRPT(BW)	1.120	3118	59.7	1.21 × 10−3	14.340	0.411
MCQDPT2	1.132	2949	58.2	1.27 × 10−3	14.034	0.415
VOO-CCD	1.133	2910	62.6	1.30 × 10−3	14.021	0.451
SS-MRCC	1.132	2966	65.7	1.27 × 10−3	14.074	0.465
FCI	1.131	2921	64.4	1.31 × 10−3	14.072	0.463
Experiment	1.131	2740	64.0	1.40 × 10−3	14.177	0.492

$r_e$ in $\mathrm{\AA }$, and all other quantities in cm⁻¹. Experimental data from Ref. [43]

Table 2. Spectroscopic constants for the first ${}^1\mathsf{\Delta }$ state of ${\mathrm{CH}}^{+}$.

Method	$r_e$	${\omega }_e$	${\omega }_e{x}_e$	${\overline{D}}_e$	$B_e$	${\alpha }_e$
EN-SS-MRPT(RS)	1.222	2135	82.1	1.53 × 10−3	12.044	0.656
EN-SS-MRPT(BW)	1.223	2124	84.4	1.55 × 10−3	12.034	0.674
MP-SS-MRPT(RS)	1.222	2154	82.8	1.51 × 10−3	12.044	0.655
MP-SS-MRPT(BW)	1.207	2237	88.4	1.51 × 10−3	12.364	0.685
MCQDPT2	1.204	2276	81.9	1.48 × 10−3	12.418	0.638
VOO-CCD	1.125	2710	81.2	1.57 × 10−3	14.230	0.622
SS-MRCC	1.217	2224	81.5	1.45 × 10−3	12.149	0.632
FCI	1.222	2188	83.4	1.46 × 10−3	12.044	0.649
Experiment	1.233	2075	76.3	1.30 × 10−3	11.940	0.620

$r_e$ in $\mathrm{\AA }$, and all other quantities in cm⁻¹. Experimental data from Ref. [43]

Table 2. Spectroscopic constants for the first ${}^1\mathsf{\Delta }$ state of ${\mathrm{CH}}^{+}$.

Method	$r_e$	${\omega }_e$	${\omega }_e{x}_e$	${\overline{D}}_e$	$B_e$	${\alpha }_e$
EN-SS-MRPT(RS)	1.222	2135	82.1	1.53 × 10−3	12.044	0.656
EN-SS-MRPT(BW)	1.223	2124	84.4	1.55 × 10−3	12.034	0.674
MP-SS-MRPT(RS)	1.222	2154	82.8	1.51 × 10−3	12.044	0.655
MP-SS-MRPT(BW)	1.207	2237	88.4	1.51 × 10−3	12.364	0.685
MCQDPT2	1.204	2276	81.9	1.48 × 10−3	12.418	0.638
VOO-CCD	1.125	2710	81.2	1.57 × 10−3	14.230	0.622
SS-MRCC	1.217	2224	81.5	1.45 × 10−3	12.149	0.632
FCI	1.222	2188	83.4	1.46 × 10−3	12.044	0.649
Experiment	1.233	2075	76.3	1.30 × 10−3	11.940	0.620

$r_e$ in $\mathrm{\AA }$, and all other quantities in cm⁻¹. Experimental data from Ref. [43]

5 Summarizing Remarks

In this paper, we have presented a new formulation of the state-specific multi-reference perturbation theories (SS-MRPT), which are designed to bypass intruders. The method is derived as a perturbative approximant from our size-extensive state-specific multi-reference coupled-cluster (SS-MRCC) theory. All these SSMR methods work with a complete active space (CAS). By emphasizing the role of certain specific terms in leading order in the SS-MRCC formalism, which simultaneously generate robust denominators free from intruders and maintain size-extensivity of the formalism, suitable perturbative approximations preserving these twin desirable features have been suggested. The present perturbative approach has the following advantages: (a) it allows arbitrary convenient partitioning of the hamiltonian H in the MP and the EN schemes; (b) it can be realized both in the RS and the BW forms and (c) it uses a completely relaxed description of the coefficients of the model space functions for computing the energy. The methods can be shown to be explicitly size-extensive. All these SSMR formulations treat each of the model space function on the same footing. We have illustrated the formalisms by applying it to study the PES of the ${}^1\sum ^{+}$ and ${}^1\mathsf{\Delta }$ states of ${\mathrm{CH}}^{+}$ using a CAS as the model space, and have shown that the method works well for both the RS and the BW versions, with the MP and EN partitions of H.