Next Article in Journal
Molecular Dynamics Simulation of the Rejuvenation Performance of Waste Cooking Oil with High Acid Value on Aged Asphalt
Next Article in Special Issue
Ruthenium p-Cymene Complexes Incorporating Substituted Pyridine–Quinoline-Based Ligands: Synthesis, Characterization, and Cytotoxic Properties
Previous Article in Journal
Composite 2D Material-Based Pervaporation Membranes for Liquid Separation: A Review
Previous Article in Special Issue
Structural Design, Anticancer Evaluation, and Molecular Docking of Newly Synthesized Ni(II) Complexes with ONS-Donor Dithiocarbazate Ligands
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Molecules
Volume 29
Issue 12
10.3390/molecules29122831
molecules-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Infrared Photodissociation Spectroscopy of Dinuclear Vanadium-Group Metal Carbonyl Complexes: Diatomic Synergistic Activation of Carbon Monoxide

by
Jin Hu
and
Xuefeng Wang
*
Shanghai Key Laboratory of Chemical Assessment and Sustainability, School of Chemical Science and Engineering, Tongji University, 1239 Siping Road, Shanghai 200092, China
*
Author to whom correspondence should be addressed.
Molecules 2024, 29(12), 2831; https://doi.org/10.3390/molecules29122831
Submission received: 12 May 2024 / Revised: 10 June 2024 / Accepted: 12 June 2024 / Published: 14 June 2024
(This article belongs to the Special Issue Recent Advance in Transition Metal Complexes and Their Applications II)
Browse Figures
Versions Notes

Abstract

:
The geometric structure and bonding features of dinuclear vanadium-group transition metal carbonyl cation complexes in the form of VM(CO)n+ (n = 9–11, M = V, Nb, and Ta) are studied by infrared photodissociation spectroscopy in conjunction with density functional calculations. The homodinuclear V2(CO)9+ is characterized as a quartet structure with CS symmetry, featuring two side-on bridging carbonyls and an end-on semi-bridging carbonyl. In contrast, for the heterodinuclear VNb(CO)9+ and VTa(CO)9+, a C2V sextet isomer with a linear bridging carbonyl is determined to coexist with the lower-lying CS structure analogous to V2(CO)9+. Bonding analyses manifest that the detected VM(CO)9+ complexes featuring an (OC)6M–V(CO)3 pattern can be regarded as the reaction products of two stable metal carbonyl fragments, and indicate the presence of the M–V d-d covalent interaction in the CS structure of VM(CO)9+. In addition, it is demonstrated that the significant activation of the bridging carbonyls in the VM(CO)9+ complexes is due in large part to the diatomic cooperation of M–V, where the strong oxophilicity of vanadium is crucial to facilitate its binding to the oxygen end of the carbonyl groups. The results offer important insight into the structure and bonding of dinuclear vanadium-containing transition metal carbonyl cluster cations and provide inspiration for the design of active vanadium-based diatomic catalysts.

1. Introduction

Transition metals, especially noble metals, are ubiquitous in catalysis and material chemistry and remain the indispensable catalysts in numerous chemical reactions. The scarcity, high cost, toxicity as well as concerns about the adverse environmental impacts from the extraction, purification, utilization and reclamation of transition metals have inspired efforts to find ways to reduce the use of transition metals. Single atom catalysts (SACs) are being pursued as attractive solutions to the aforementioned problem by virtue of their maximized metal atom utilization, well-defined active sites and exceptional selectivity compared with traditional bulk material and supported nano-particles [1,2,3]. However, despite a true boost in catalytic performance and the feasibility of further improving their intrinsic activity by careful tuning of their coordination and electronic configurations, the poor structural and compositional complexity of SACs renders them inadequate in the face of complicated reactions. In addition, the lack of diverse synergistic interactions in SAC materials with only a single type of active site presents an insurmountable obstacle to regulating activity and selectivity over a wide range [4]. This calls for the introduction of additional metal atoms in the vicinity of single active sites of SACs to form multi-atom cluster catalysts [5,6], typically such as dual atom catalysts (DACs), which allow for the inheritance of high atomic dispersity, high atomic utilization, high activity and selectivity of SACs, and the break of the intrinsic limitations as well. Studies of DACs to date have revealed opportunities in a broad variety of applications [7,8,9], but the molecular-level understanding of this diatomic synergistic effect remains elusive due to the herculean challenges to exploring the structure and properties of actual active sites fraught with complicated ambient perturbations through common characterization methods. Thus, a combination of spectroscopic methods and theoretical calculations to determine the interaction between diatomic metal clusters and small molecules is particularly meaningful.
Infrared photodissociation spectroscopy (IRPD) has proven to be a powerful tool allowing for structural identification of mass-selected charged clusters in the gas phase [10,11,12], as well as an understanding of bonding interactions between core atoms in conjunction with density functional calculations [13,14,15,16,17,18,19]. In addition, carbon monoxide (CO) acts as an ideal probe for detecting metal–ligand interactions owing to its strong infrared absorption and simple vibration pattern. Miscellaneous cationic and anionic carbonyl complexes of transition metals have been studied by combining infrared photodissociation spectroscopy with high-precision quantum computation over the past few decades [10,11,12]. The metal-carbonyl bonding is well-described as a combination of CO→M σ-donation and M→CO π-backdonation by the Dewar–Chatt–Duncanson (DCD) model [20,21]. The earlier transition metals are demonstrated to exhibit a stronger ability to activate CO due to their relatively smaller electron detachment energies, which is conducive to the π-backbonding. Zhou and coworkers systematically explored the structures and bonding of homodinuclear first-row transition metal carbonyl complex cations and found a predilection towards formation of (OC)xM–M(CO)y structure with terminally bound carbonyls for the saturated complexes of late transition metals such as Fe2(CO)9+ [22], Fe2(CO)8− [23], Ni2(CO)8+ [24] and Cu2(CO)6+ [25]. Similar structural and bonding features have also been shown to prevail in heterodinuclear late transition metal carbonyl complexes, including CuFe(CO)7− [26], FeZn(CO)5+ [27], CoZn(CO)7+ [27], FeM(CO)8+ (M = Co, Ni, Cu) [28] and MCu(CO)7+ (M = Co, Ni) [28]. The carbonyl groups in these complexes are separately tethered to the two metal centers with a small shift of the infrared frequency relative to the free CO, signaling the poor ability of two late-transition metal atoms to synergistically activate CO. Moreover, these complexes exhibit a preference for asymmetric structures, so as to give priority to ensuring that one of the metal centers meets the favorable 18-electron rule. In marked contrast, bridging CO ligands are presented in the homodinuclear carbonyl complexes of early transition metals. The Cr2(CO)9+ cation was characterized to have the (OC)5Cr–C–O–Cr(CO)3 configuration with a linear bridged carbonyl group bonded to one chromium site through its carbon atom and to the other chromium site through its oxygen atom [29]. The Ti2(CO)9+ cation was determined to be a doublet CS structure with two side-on bridging carbonyls and one semi-bridging carbonyl [30]. The carbonyl group serving as a four-electron donor in these complexes is simultaneously activated by two metal atoms, thus featuring an appreciably elongated C–O distance and a significant redshift in the stretching frequency.
Atomically dispersed vanadium-related catalysts are ubiquitous in various reactions including the oxidations of alkanes, alkenes, arenes, alcohols, as well as the activation and cleavage of C–C and C–O bonds [31]. Atomically precise small size clusters in the gas phase are ideal models for studying the cluster chemical bonding and the cluster–ligand interactions. Recently, clusters related to vanadium-group metals have also received special attention [32,33,34]. However, spectroscopic investigations involving multinuclear vanadium-containing complexes with metal–metal synergistic interactions are rarely available, since the formation and stabilization of pure vanadium-group all-metallic clusters in the gas phase is experimentally challenging due to the intense propensity of vanadium-group metals to form oxides [35,36,37,38,39].
In the present work, homo- and heterodinuclear vanadium-containing carbonyl complex cations in the form of VM(CO)n+ (M = V, Nb, and Ta; n = 9–11) are produced in the gas phase and studied by infrared photodissociation spectroscopy. The geometric structures of mass-selected complexes are determined by comparisons of their experimental infrared photodissociation spectra and simulated IR spectra from density functional calculations.

2. Results

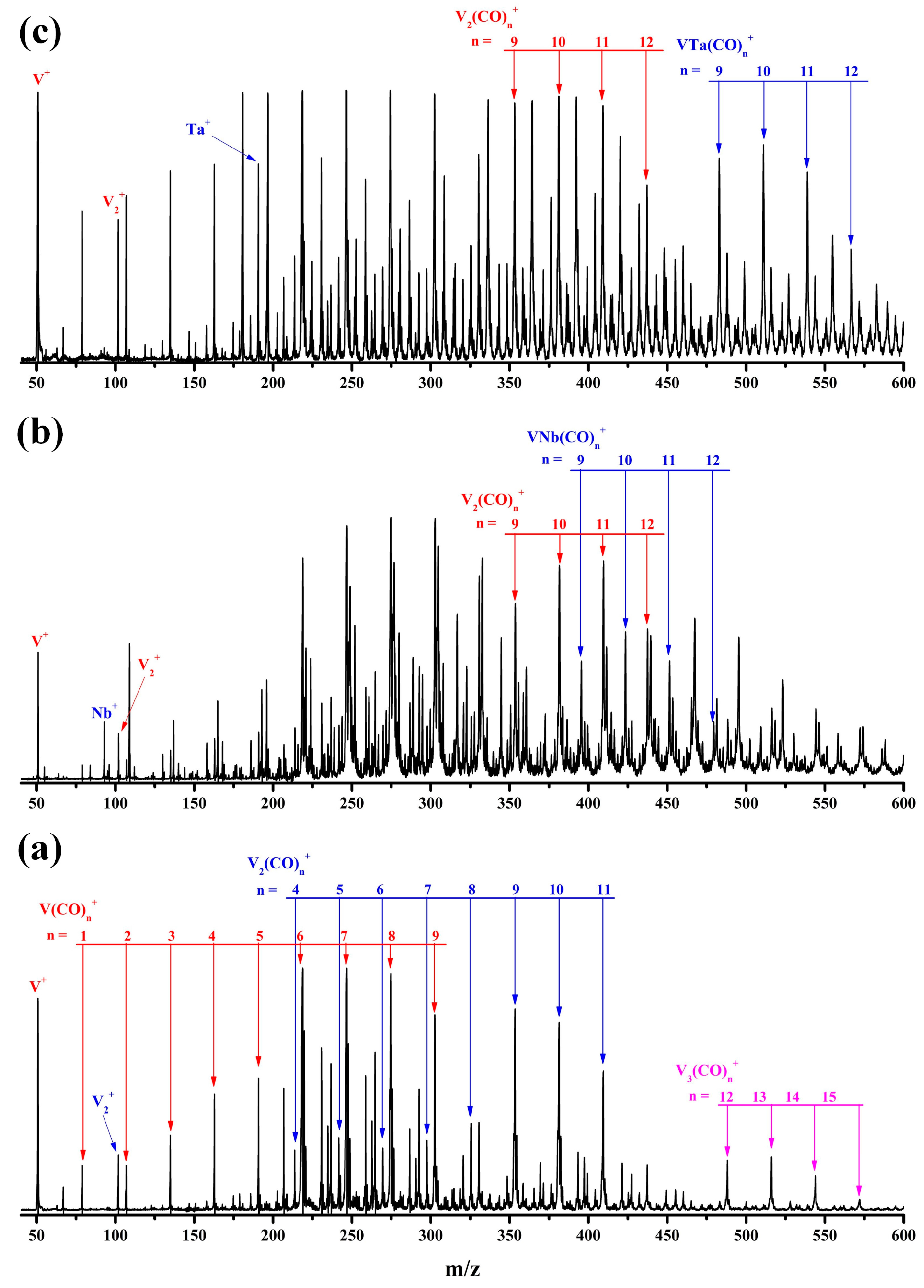
2.1. Mass Spectra

The representative mass spectrum of cations generated by the pulsed laser ablation of the pure bulk vanadium target in the expansion of a CO/He mixture is shown in Figure 1a. The product progressions are completely composed of the homonuclear carbonyl complexes Vm(CO)n+ with m = 1–3. For the mixed vanadium–niobium and vanadium–tantalum targets, aside from the exclusively vanadium-containing mono/multinuclear carbonyl complexes, the heteronuclear bimetallic carbonyl complexes VNb(CO)n+ and VTa(CO)n+ were observed (Figure 1b,c). The chemical formulas of each compound were determined by combining their mass-to-charge ratios with the most probable carbonyl coordination number at the metal centers.
Duncan and coworkers reported that for a single vanadium cation, the 16-electron V(CO)6+ is saturated and the formation of strongly bonded 18-electron V(CO)7+ is prohibited in the pulsed laser sputtering source [40,41]. As displayed in Figure 1a, the V(CO)6+ cation shows a sharp increase in the mass intensity relative to V(CO)1–5+, followed by a smooth decrease in the intensity of V(CO)7–9+, indicating that the current experimental conditions are biased in favor of the formation of saturated coordination compounds. Accordingly, the V2(CO)9+ and V3(CO)12+ cations with the highest abundance in their corresponding product sequences are expected to be the saturated homogeneous dinuclear and trinuclear vanadium carbonyl complexes, respectively. It is worth noting that from V(CO)6+ over V2(CO)9+ to V3(CO)12+, one V(CO)3 unit is progressively added, to some extent suggesting the possible presence of a certain rule in the growth process of multinuclear vanadium carbonyl complexes. Except for the heterodinuclear VNb(CO)10,11+ and VTa(CO)10,11+ cations, the similar mass intensity indicates that their saturated coordination numbers cannot be determined unilaterally by the mass spectrum.
The VM(CO)9+ (M = V, Nb, and Ta) complexes were mass-selected to interact with tunable infrared laser in the carbonyl stretching frequency region. However, no dissociation was observed, indicating that these nonacarbonyl compounds are strongly bonded substances. Further, the decacarbonyl and undecacarbonyl complexes VM(CO)10,11+ (M = V, Nb, and Ta) were mass-isolated and exposed to infrared laser radiation, and obvious laser-induced fragmentation occurred (Figure 2). The VM(CO)10+ cations rapidly dissociate through the immediate removal of a CO ligand at a laser energy of 1.0–1.2 mJ/pulse, resulting in the decomposition of about 20–30% of the parent ions into the nonacarbonyl species. The direct shedding of double CO ligands from the parent cation VM(CO)11+ with a higher dissociation at the same frequency of laser radiation was observed, indicating that the nonacarbonyl VM(CO)9+ complexes are probably fully coordinated and the outermost two CO ligands are exceedingly weakly attached to the periphery of the transition metal saturated coordination sphere.

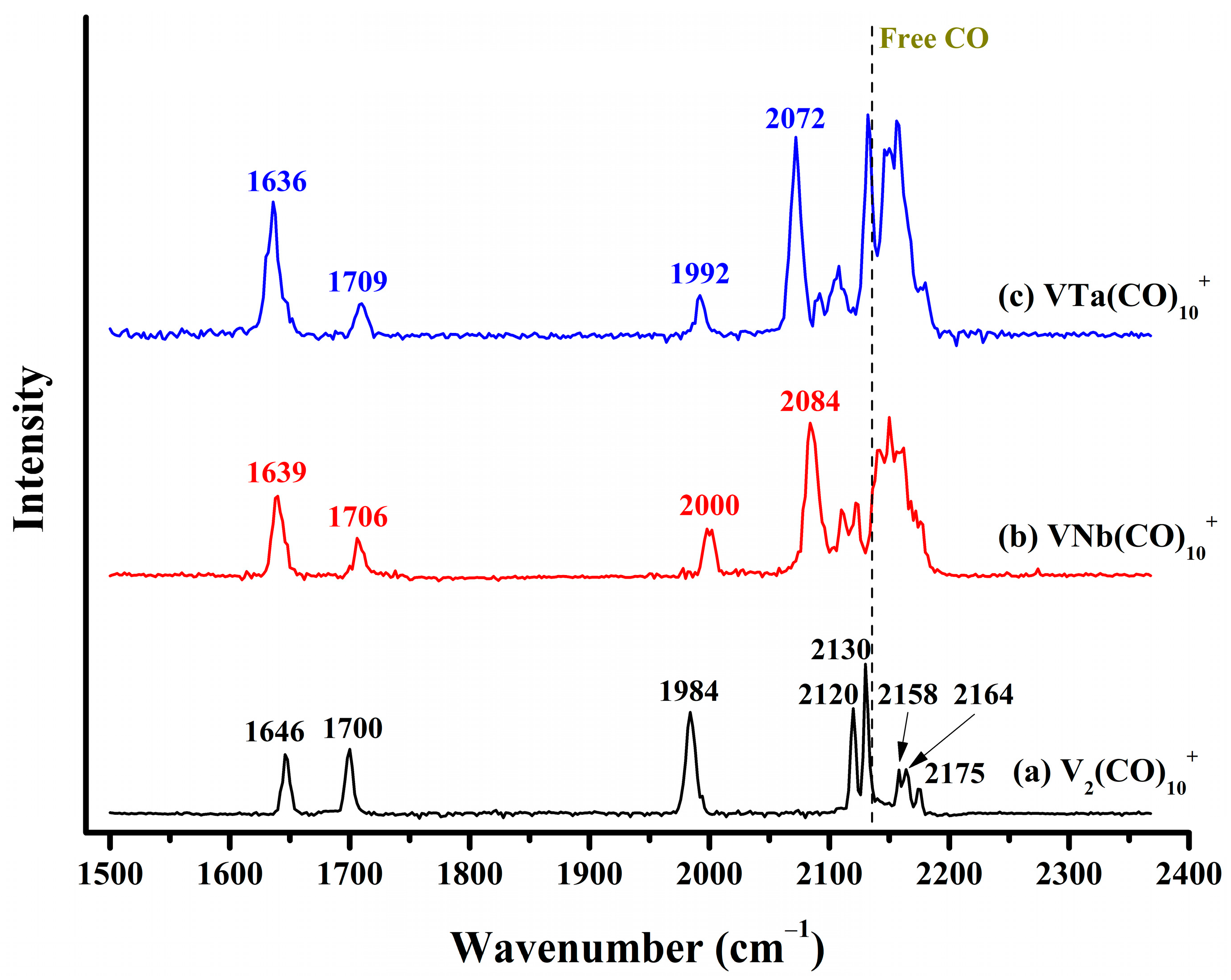
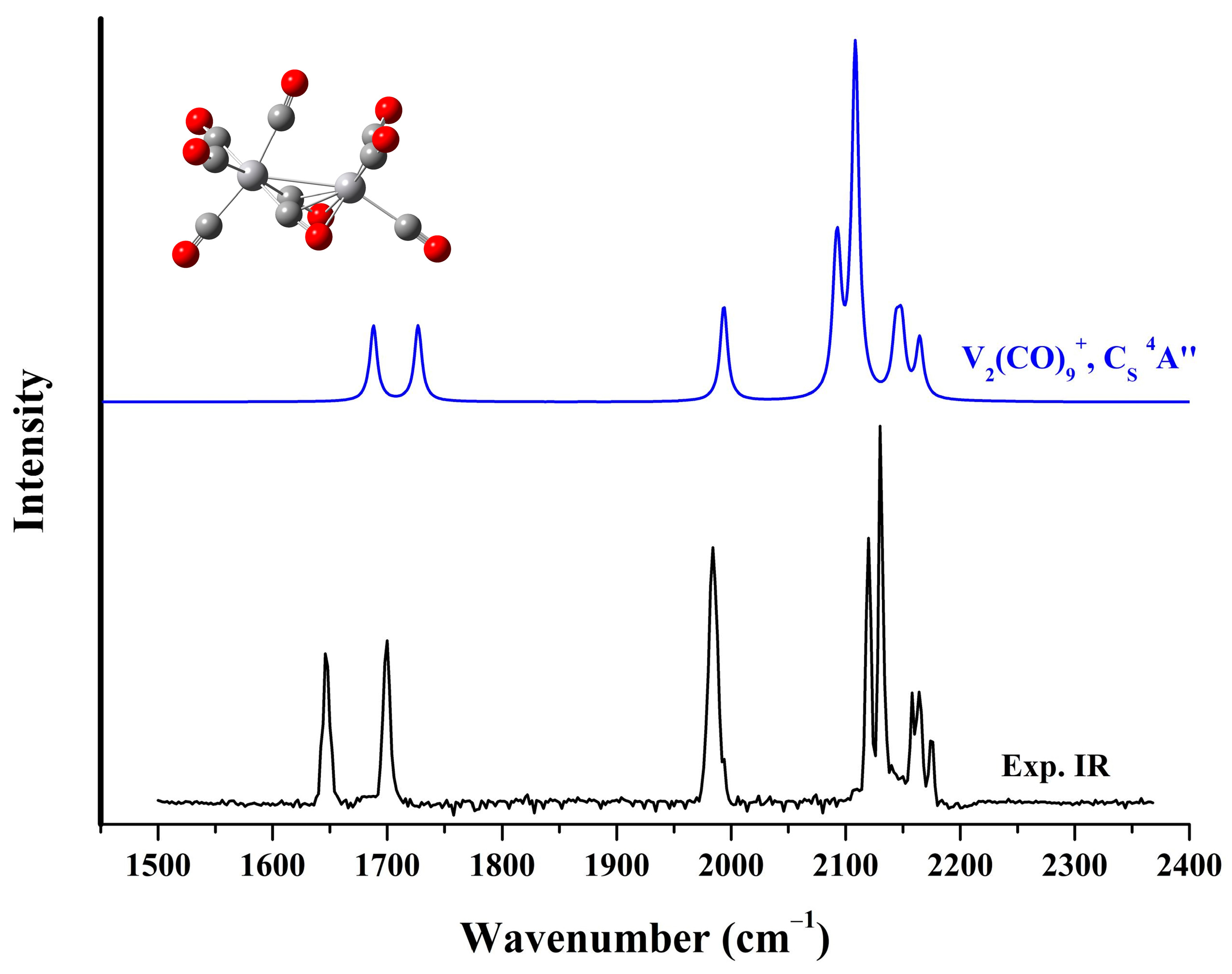
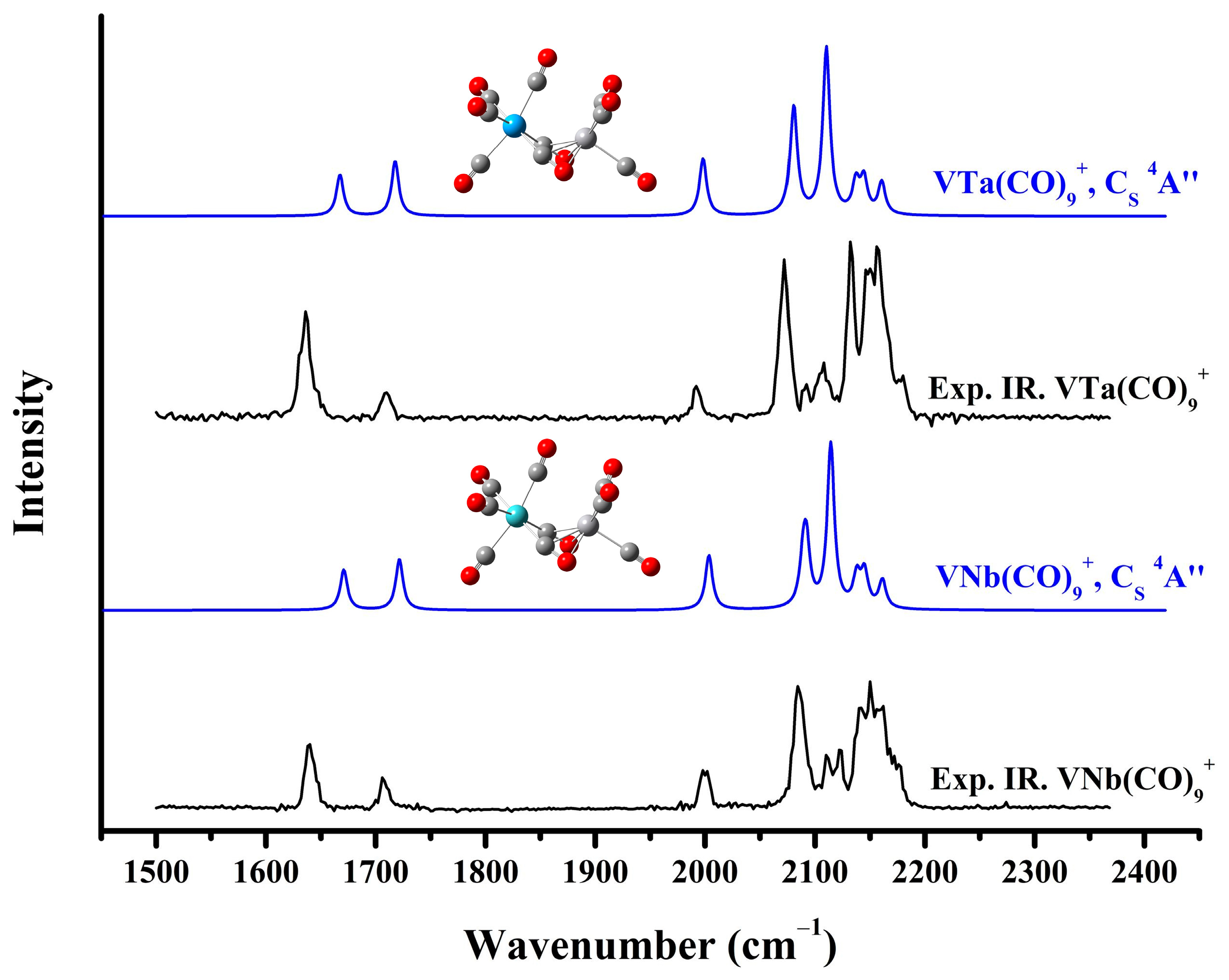
2.2. Infrared Photodissociation Spectra

Labeling techniques by tagging a weakly bound ligand can help indirectly obtain the infrared photodissociation spectra of strongly bonded substances. In this regard, the VM(CO)10,11+ cations generated under experimental conditions conducive to the formation of oversaturated complexes were selected for photodissociation. The infrared photodissociation spectra of V2(CO)10,11+ present totally identical number and location of adsorption peaks, despite slight shifts of some bands within 5 cm−1 as well as insignificant differences in intensity (Figure S1). Similarly, the resulting photodissociation spectra of VNb(CO)11+ and VTa(CO)11+ from monitoring the simultaneous volatilization of two labeling CO molecules are nearly identical to that of VNb(CO)10+ and VTa(CO)10+, respectively (Figures S2 and S3). The spectroscopic observations well justify the rationality of the presumption that VM(CO)9+ are saturated complexes.
The infrared photodissociation spectrum of the VM(CO)10+ (M = V, Nb, Ta) cations are collectively displayed in Figure 3. For V2(CO)10+, eight well-resolved absorption bands centered at 1646, 1700, 1984, 2120, 2130, 2158, 2164 and 2175 cm−1 were detected. The observation of two bands far below 2000 cm−1 and one band slightly below 2000 cm−1 indicates that this cation complex involves multiple bridge-bonded CO ligands in different forms. For VNb(CO)10+ and VTa(CO)10+, the three low-frequency absorption bands associated with bridged carbonyls were also detected at roughly similar frequency regions, suggesting some similarity between them and the ground state structure of V2(CO)10+. In contrast, in the frequency region between 2050 and 2200 cm−1, VNb(CO)10+ and VTa(CO)10+ exhibit more absorption bands compared to V2(CO)10+. On the whole, the profiles of infrared photodissociation spectra of VNb(CO)10+ and VTa(CO)10+ are highly consistent but slightly different from that of V2(CO)10+. One possible scenario is that in addition to the ground state structure shared with V2(CO)10+, VNb(CO)10+ and VTa(CO)10+ have other isomers that are allowed under our experimental conditions. Anyway, the high resemblance in the photodissociation spectra of the VM(CO)10+ cations implies similar geometric parameters and bonding situations of corresponding saturated coordination VM(CO)9+ complexes.

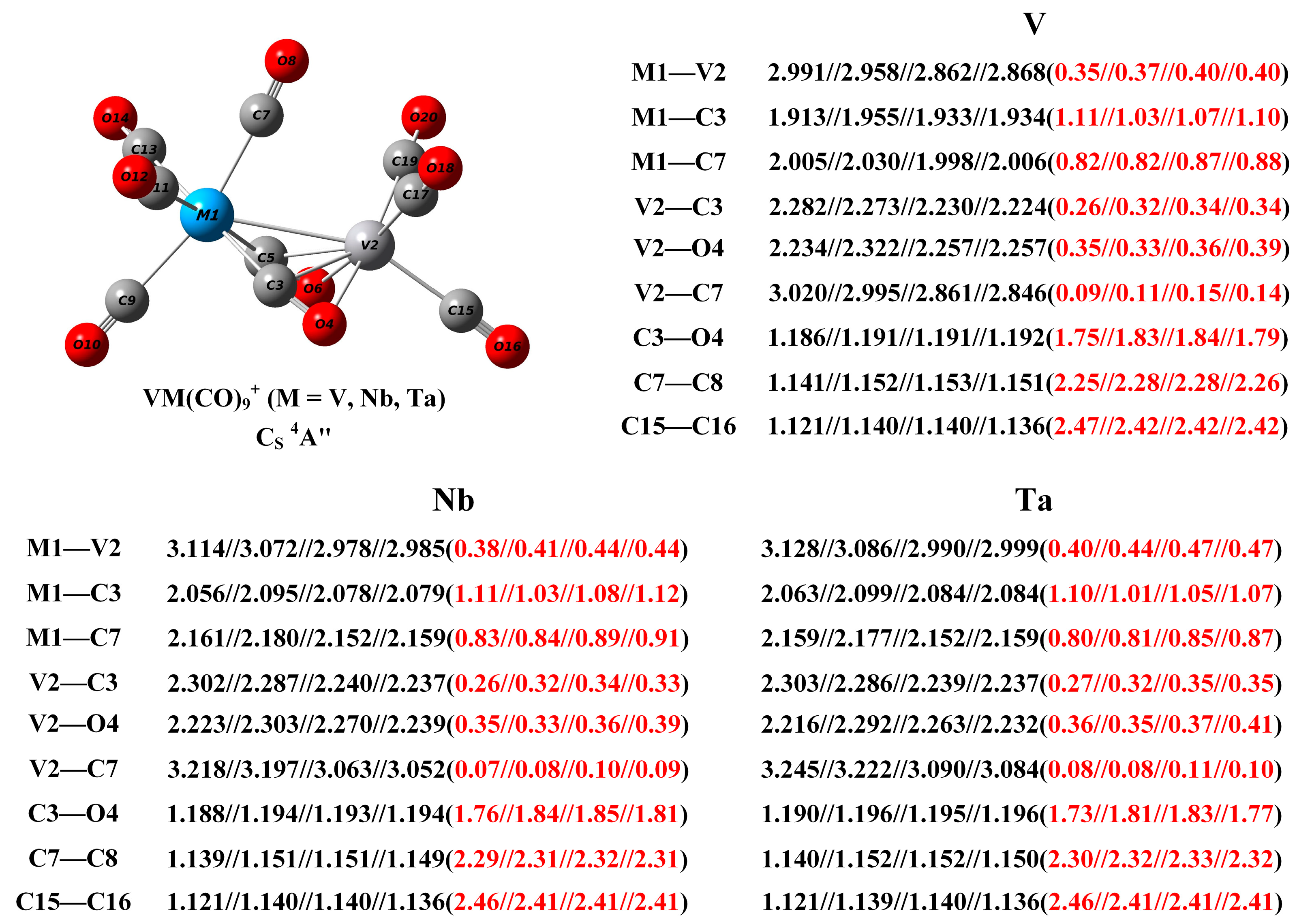
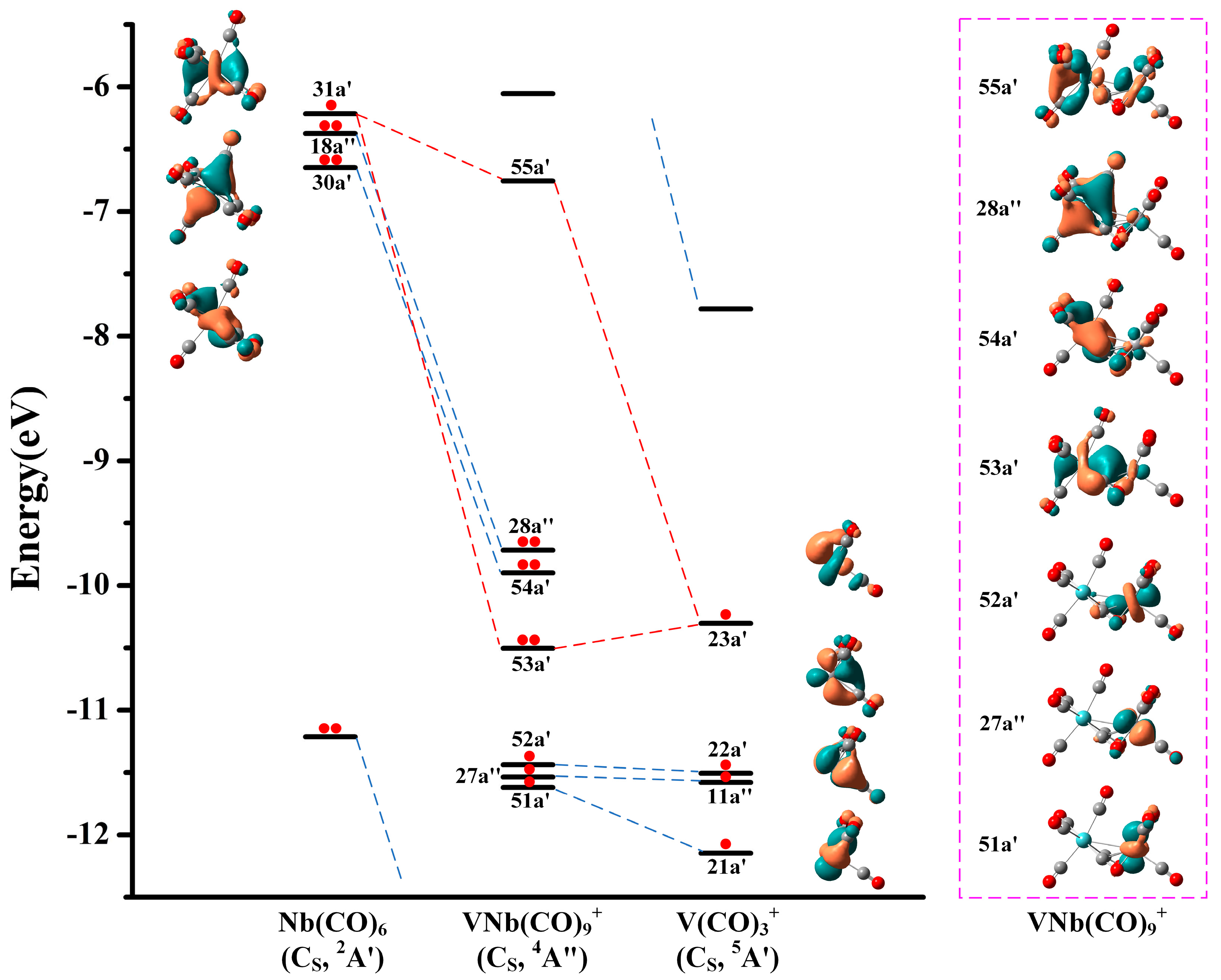
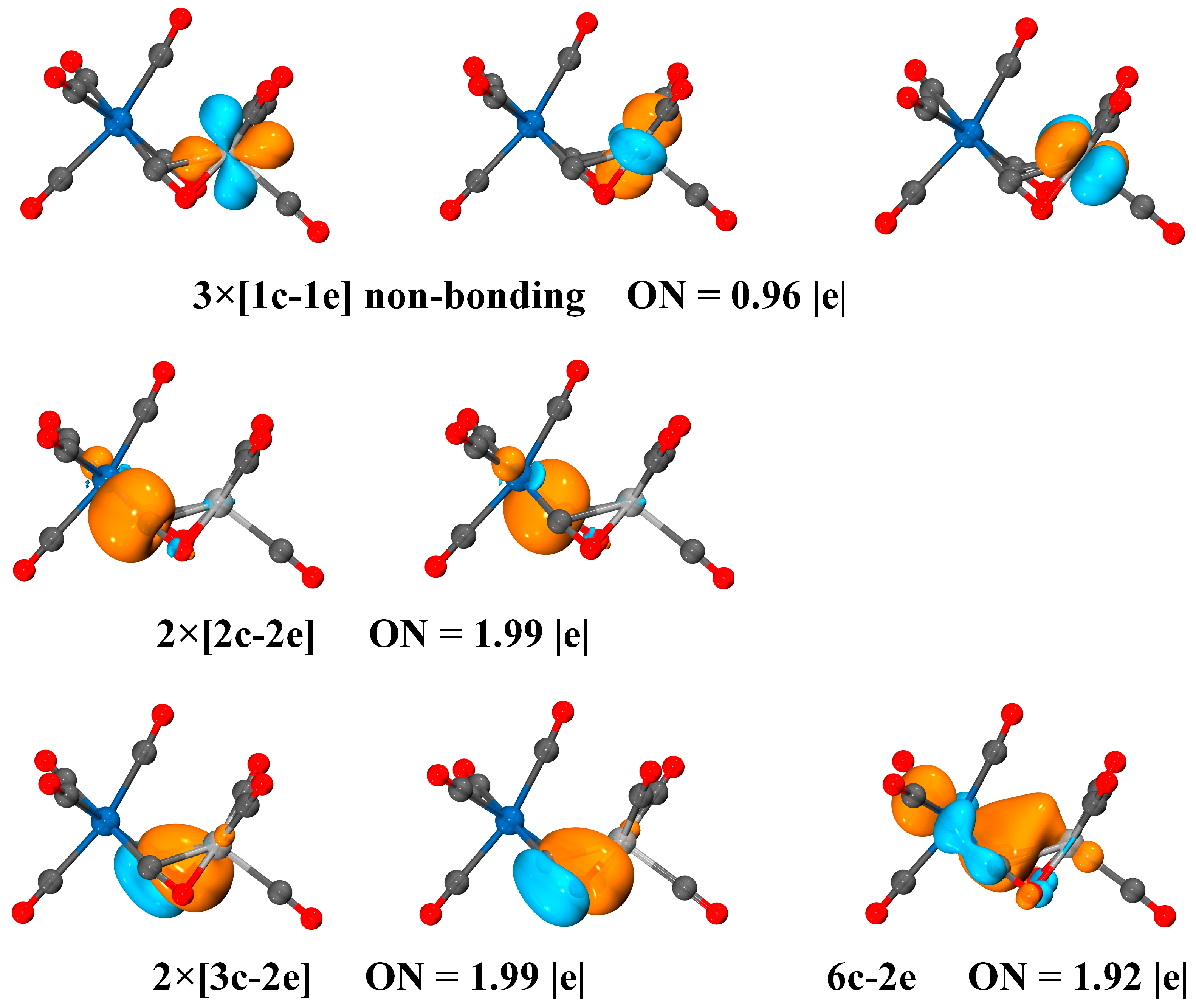
2.3. Structure of the VM(CO)9+ Cations

Quantum chemical calculations based on density functional theory were conducted to support the assignments of the vibrational spectra of the observed species and to examine the geometric and electronic structure of the carbonyl complexes. The calculated eight lowest-lying structural isomers of V2(CO)9+ at the B3LYP, BLYP, PBE, and TPSS level of theory are presented in Figure S4. And the comparison of the experimental photodissociation spectrum of the solvated V2(CO)10+ with the simulated spectra of these stable candidates derived from the B3LYP calculations is shown in Figure S5. The global minimum (a) has a 4E electronic state and C3V symmetry with three equivalent end-on semi-bridging carbonyl groups and six terminally bonded carbonyl groups at two vanadium centers. The second structure (b) has CS symmetry involving two side-on bridging CO ligands and one end-on semi-bridging CO ligand. Structure (c) is also a CS-symmetry structure, but has two end-on semi-bridging carbonyls and one side-on bridging carbonyl. In contrast to these three lowest-lying structures, all of which are quartet structures, the fourth structure (d) is a sextet structure that appears as a (OC)5V–C–O–V(CO)3 configuration with a linear-bridge carbonyl group. The subsequent isomers (e), (f), (g) and (h) are all characterized by one or more similar quasi-linear bridging carbonyls. The CS isomer (b) provides a simulated infrared spectrum that well agrees with the experimental photodissociation spectrum (Figure 4). In this structure, the two side-on bridging carbonyls are equivalent, with an appreciably elongated C–O distance relative to the end-on semi-bridging carbonyl and the other terminal CO ligands. The theoretical frequencies for the C–O stretching modes of the VM(CO)9+ cations, along with the experimental values are shown in Table 1. The calculated values are derived from the scaled harmonic frequencies from calculations at the B3LYP/def2-TZVP level with a factor of 0.968, which comes from the ratio of the fundamental frequency of free CO (2143 cm−1) to the calculated value (2214 cm−1). For V2(CO)9+, the very low CO stretching frequencies at 1646 and 1700 cm−1 are attributed to the antisymmetric and symmetric stretching vibrations of the two four-electron donor side-on bridging carbonyl groups, respectively. The single band centered at 1984 cm−1 is due to the stretching vibration of the end-on semi-bridging CO ligand. The 2120 and 2130 cm−1 bands originate from the coupled stretching vibrations of the three end-on carbonyl groups on the left-hand vanadium atom. In contrast, the blue-shifted bands at 2158, 2164 and 2175 cm−1 result from the three carbonyl groups terminally bonded on the right-hand vanadium atom.
As a further supplement, geometry optimizations were performed on various possible structures for V2(CO)10+. As shown in Figure S6, the most stable structure (a) at the B3LYP level can be viewed as the further reaction product of the fourth sextet V2(CO)9+ with a CO ligand, which maintains the linear (OC)5V–C–O–V(CO)4 structure. The sub-stable C2V-symmetry structure (b) can also be seen as the formation of the isomer (h) of V2(CO)9+ with an additional CO molecule, retaining the characteristic two quasi-linear bridging carbonyl groups as four-electron donors. The other isomers lie at least 4 kcal/mol higher in energy relative to the global minimum, all of which exhibit bridging carbonyls with specific patterns. As shown in Figure S7, none of the simulated infrared spectra of the eight lowest-lying isomers of V2(CO)10+ can match the experimental spectrum of V2(CO)10+, confirming that the V2(CO)10+ cation observed in the experiments is a loose adduct formed from the saturated coordination product V2(CO)9+ with a weakly adsorbed CO molecule. A comparison of the experimental photodissociation spectrum of V2(CO)10+ with the calculated infrared spectrum of the saturated V2(CO)9+ cation as well as that of the CO-tagged V2(CO)10+ is presented in Figure S8. The disturbance of the outlying CO ligand to the geometrical parameters and chemical bonding of the fully coordinated V2(CO)9+ core is negligible, except for slight shifts of the main absorption bands.
For the VNb(CO)9+ cation, eight low-lying structures and comparisons of their simulated spectra with the experimental infrared photodissociation spectrum of VNb(CO)10+ are displayed in Figure S9 and Figure S10, respectively. The lowest-lying structures (a), (b) and (c) share similar geometric parameters and electronic states with the three most stable isomers of V2(CO)9+, respectively. In fact, the geometric features of structures (d)–(g) are also consistent with several low-energy isomers of V2(CO)9+, despite a varying order of energies at the computation level with different functionals. Three low-frequency absorption bands below 2000 cm−1 firmly confirm the presence of structure (b) at the B3LYP level, which has the same CS symmetry and 4A” electron state as the structure (b) of V2(CO)9+. Unfortunately, the intensity of the two absorption bands near 1700 cm−1 predicted by theoretical calculation is roughly the same, while the experimental results clearly show that the absorption band centered at 1639 cm−1 is much more intense than the band at 1706 cm−1. In addition, the absorption bands attributed to terminal carbonyl groups between 2050 and 2200 cm−1 of structure (b) cannot match the experimental results very well. We noted that the throng of absorption bands of terminally bonded carbonyl groups of the sextet structure (e) provides a reasonable agreement with the experimental spectrum. However, according to the thermodynamic energies, the sextet isomer of VNb(CO)9+ stands more than 23 kcal/mol higher relative to the calculated CS-symmetry structure (b) at the PBE level with def2-TZVP basis set, as shown in Figure S9C. Such a significant energy difference suggests that the coexistence of these two structures is unlikely. Thus, as shown in Figure 5, the experimentally observed VNb(CO)9+ is attributed to the CS-symmetry structure. Figure S11 displays the eight lowest-lying isomers of the strongly bonded VNb(CO)10+ cation complex. The structures with thermodynamic advantages have a preference for the electronic states of quartet. As shown in Figure S12, none of the simulated spectra of isomers match the experimental spectra of VNb(CO)10+, showing no evidence for the formation of strongly bonded VNb(CO)10+ under our experimental conditions. Note that although the photofragmentation mass spectra of VM(CO)11+ (Figure 2) and their spectra show no evidence for the strongly bonded VM(CO)10+, their much lower energies relative to the solvated CO-tagged VM(CO)10+ still suggest that they may be available under altered experimental conditions.
The calculated lowest-lying structures of the strongly bonded VTa(CO)9+ and the comparison of their simulated vibrational spectra with the experimental infrared photodissociation spectrum of VTa(CO)10+ are presented in Figure S13 and Figure S14, respectively. As in the case of VNb(CO)9+, the VTa(CO)9+ cation generated in the experiment was determined to be the CS-symmetry quartet structure (Figure 5). Similarly, no strongly bonded VTa(CO)10+ complex provides a calculated spectrum matching the experimental spectrum (Figures S15 and S16), showing no evidence for its presence in this experiment. To sum up, based on experimental results and theoretical calculations, the structure of VM(CO)9+ (M = V, Nb, and Ta) complexes with CS symmetry and a 4A” electronic state is indisputably established (Figure 6). According to the calculated frequencies in Table 1, for VNb(CO)9+ and VTa(CO)9+, the two lowest-frequency bands detected in the experiment are mainly attributed to the antisymmetric and symmetric stretching vibration of the two side-on bridging carbonyls, respectively, and the single band close to 2000 cm−1 is due to the stretching vibration of the end-on semi-bridging carbonyl. Notably, VM(CO)9+ are all quartet complexes, while previous studies have shown that bare V2+ also has a quartet ground state [42]. This may mean that the CO ligands only coordinate into the unoccupied orbitals of V2+ over the course of the formation of saturated carbonyl complexes, without affecting the configuration of its unpaired electrons.

3. Discussion

The calculated bond distance and Mayer bond index for some bonds of interest, along with the geometrical structures of the VM(CO)9+ cations are shown in Figure 6. The V–V distance is predicted to be 2.868 Å with a Mayer bond index of 0.40 at the TPSS level, indicating the presence of a half-bond. The V1–C3 distance is estimated to be 1.934 Å, which is much shorter than the V1–C7 bond (2.006 Å), suggesting that the covalent interaction between the side-on bridging carbonyl and the V1 center is significantly enhanced compared to that of the end-on semi-bridging carbonyl and other terminal carbonyls. The C–O distance of the side-on bridging carbonyl is appreciably elongated to 1.192 Å, roughly 5% longer than the bond length of free CO (1.135 Å). And its Mayer bond index is calculated to be 1.79, unequivocally proving that this carbonyl group is highly activated and closer to a C=O double bond. The calculated Mayer bond orders of the V2–C3 bond (0.34) and V2–O4 bond (0.39) demonstrate that there exist strong chemical bonding interactions between the right-hand vanadium atom and the side-on bridging carbonyl. From V over Nb to Ta, the M1–V2 bond is much elongated, but the calculated bond index is monotonically increasing. The longer bond distance is attributed to the increasing radius of vanadium-group metals from top to bottom, while the increased bond order indicates an enhanced metal–metal interaction. The modest decrease in the bond index of the Ta1–C3 and Ta1–C7 bonds relative to the Nb1-C3 and Nb1-C7 bonds can be rationalized by the contraction of s and p orbitals due to the relativistic effect of heavy atoms [43]. Thus, the increase in the M1–V2 bond index from V over Nb to Ta suggests that this bond is mainly related to the d orbital in the metal center.
To gain deeper insight into the electronic structures and chemical bonding of the VM(CO)9+ cations, the natural population analysis was conducted. As displayed in Table S1, the M1 center of the quartet VM(CO)9+ is substantially negative, while the V2 center is relatively positive. More importantly, the spin population on the V2 center exceeds 2.50 e, indicating that all unpaired electrons are highly localized. Therefore, the experimentally determined VM(CO)9+ structures can be regarded as reaction products of a neutral M(CO)6 fragment with a cationic high-spin V(CO)3+ fragment. Note that Duncan and coworkers have reported the infrared photodissociation spectrum of the V(CO)3+ cation by argon labeling technique, and determined its T-shaped geometry and quintet electronic state in combination with density functional calculations [41]. Moreover, the evident abundance of V(CO)3+ in the mass spectra (Figure 1) also supports the assignment that it functions as a raw material for the generation of VM(CO)9+. The correlation diagram for the orbital interactions along with the frontier canonical Kohn–Sham valence molecular orbitals of VNb(CO)9+ is shown in Figure 7. The HOMO (28a″) and HOMO-1 (54a′) of VNb(CO)9+ principally originate from the 18a″ and 30a′ orbitals of the Nb(CO)6 fragment, respectively, representing two non-bonding orbitals. The Nb(CO)6 fragment possesses an electronic structure of 4d95s25p6 with a half-filled d-type orbital (SOMO, 31a′). This singly occupied orbital interacts with the SOMO (23a′) of the quintet V(CO)3+ fragment in a covalent manner, forming the bonding orbital 53a′ and the antibonding orbital 55a′ of VNb(CO)9+. Notably, the three singly occupied orbitals of VNb(CO)9+ (51a′, 27a″ and 52a′) energetically stand much lower than the doubly occupied 53a′, 54a′ and 28a″ orbitals, which seems unusual because unpaired electrons usually behave actively and therefore reside in the outermost orbitals. It can be rationalized by the description that these orbitals are derived from the 21a′, 11a″ and 22a′ orbitals of the V(CO)3+ fragment. The desire to minimize the thermodynamic energy of the product from the fragment reaction drives these single-occupied orbitals to remain in the inner orbitals. Therefore, the V–Nb interactions in the quartet VNb(CO)9+ cation can be described as a single electron-sharing bond, which renders the niobium center the favored 18-electron configuration. This bonding scheme is also established for the CS structures of V2(CO)9+ and VTa(CO)9+.
According to the AdNDP analysis shown in Figure 8, the bonding interaction related to the two side-on bridging carbonyls in VNb(CO)9+ consists of two two-center-two-electron (2c-2e) Nb–C bonds with occupation numbers of 1.99 |e|, two 3c-2e bonds with an occupation number of 1.99 |e|, and one 6c-2e bond with the occupation number of 1.92 |e|. The two 2c-2e Nb–C bonds represent the σ donation of the side-on bridging carbonyls to the Nb center. The two 3c-2e bonds illustrate the formation of π donation of the side-on bridging carbonyls to the V center. Accordingly, the two side-on bridging carbonyl groups act as both the classical σ donor and the less common π donor, essentially serving as four-electron donors. The 6c-2e bond depicts the interaction between the two metal centers, which principally consists of the dxy, dx2-y2 and dz2 orbitals of V/Nb and partly contains the contribution of the 2π* orbitals of the side-on bridging carbonyls (Table S2). This d-d bonding is consistent with the results of the fragment interaction analysis.

4. Methods

The homodinuclear vanadium carbonyl cluster cations were produced by the pulsed laser ablation of a pure vanadium target. And the heteronuclear vanadium–niobium, vanadium–tantalum carbonyl cluster cations were produced by the pulsed laser ablation of composite targets, which were prepared via direct compression of the mixture of vanadium powder and niobium/tantalum powder with a molar ratio of 1:3. The 1064 nm fundamental of a Nd: YAG laser (Continuum, Minilite II, 10 Hz repetition rate and 6 ns pulse width) was introduced to the rotating metal target by the optical lens, leading to the formation of a searing metallic plasma. Various carbonyl complexes were produced from the reactions of the hot plasma with 10% CO seeded in helium using a pulsed valve (General Valve, Series 9) at a background pressure of 1.0–1.5 MPa. The prepared products were cooled by supersonic adiabatic expansion and were skimmed to be analyzed using a Wiley–McLaren time-of-flight mass spectrometer. The ions of interest were mass-selected by a mass gate and forced to interact with a tunable infrared laser beam provided by an OPO/OPA system (Laser Vision) pumped by a Nd:YAG laser (Continuum, Surelite EX). Under the laser radiation with a frequency consistent with the vibration of cluster complex, the energy of the infrared photon is absorbed by the complex. Over the course of energy relaxation, the weakest links in the molecule may be broken. The bond energy of a typical chemical bond is generally higher than the energy of a single infrared photon, so it is difficult to directly measure the infrared photodissociation spectrum of strongly bonded species by using the infrared laser from the OPO/OPA optical system. The spectrum of the complex of interest can be obtained indirectly by the labelling technique via weakly bound messengers. Therefore, the solvated loose complex were mass-isolated for photodissociation in this experiment.
The dissociated fragments and parent cations were reaccelerated and mass-analyzed by the second tandem TOF mass spectrometer. The infrared photodissociation spectra were obtained by monitoring the yield of the fragment cations as a function of the infrared laser wavelength with a scan step of 2 cm−1. In this experiment, the used IR laser beam has an energy of 0.7–1.3 mJ/pulse.
First principle density functional theory (DFT) calculations were carried out using the Gaussian 09 computational package [44]. The hybrid B3LYP functional [45,46] is the most extensively used density functional for structural optimization and harmonic vibration frequency analysis, which has been demonstrated to be able to provide reliable predictions on the structures and vibrational frequencies of transition metal-containing compounds [47,48,49]. Therefore, the preliminary structure search for each carbonyl complex VM(CO)n+ was conducted using the def2-SVP basis set at the B3LYP level [50,51], which started from a plethora of initial structures generated by randomly putting the CO ligands around the exposed VM+ core. A large number of presupposed structures containing one/two/three bridging carbonyls sandwiched between the two metal centers in various binding patterns were considered as well [12]. The preliminary structure optimization yielded considerable candidates, in which the three lowest spin states, namely the doublet, quartet and sextet of each candidate isomer were considered. To the best of our knowledge, the B3LYP functional is capable of providing accurate vibrational spectra of carbonyl complexes involving transition metals [14,16,18,19], but is much inferior to the non-hybrid functionals in terms of energy calculation and bond length prediction [52,53,54]. Therefore, the resulting low-lying candidates from the preliminary structure search were further re-optimized at the B3LYP, BLYP, PBE, and TPSS level in conjunction with the def2-TZVP basis set to obtain more accurate energetical results and structural parameters [45,50,51,55,56,57,58]. We performed a calculation for the bond dissociation energy of the bare V2+ cation at the B3LYP, BLYP, PBE, and TPSS level with the def2-TZVP basis set. We found that the obtained results closest to the experimental value (3.140 eV ± 0.002 eV) are provided by the non-hybrid meta-GGA functional TPSS (3.970 eV) [42], as shown in Table S3. The non-hybrid BLYP and PBE calculations provide results that are more than 1 eV larger than the experimental value, while the hybrid functional B3LYP significantly underestimates the dissociation energy of V2+ (1.947 eV). Therefore, the thermodynamic energies derived from the recalculations at the non-hybrid TPSS/def2-TZVPP level are more reliable. After each optimization, the harmonic vibration frequency was carefully checked to confirm that a true minimum point was obtained, and the zero point vibration energy (ZPVE) was derived. The relative energies of various isomers were calculated based on the electron energies of related substances corrected by ZPVE. We have calculated the binding energy (in kcal/mol) of the weakly bound CO ligand in the solvated VM(CO)10+ at the B3LYP, BLYP, PBE, and TPSS level with the def2-TZVP basis set. As shown in Table S4, the binding energy predicted at all theoretical levels is within 2 kcal/mol, which is much smaller than the energy of a single infrared photon in our experiments (typically 1500–2300 cm−1, on the order of 7 kcal/mol), so the laser-induced CO dissociation from the solvated VM(CO)10+ is completely thermodynamically feasible. The computational spectra were scaled by a factor of 0.968 and widened by the Gaussian-type curve with a full width at half-maximum (FWHM) of 4 cm−1. The chemical bonding properties were analyzed by employing several different methods including Mayer bond order (MBO) [59], natural population analysis (NPA) [60], adaptive natural density partitioning (AdNDP) [61], orbital component analysis based on natural atomic orbital (NAO) [62] and orbital correlation based on charge decomposition analysis (CDA) [63]. All the bonding analyses were performed by using Multiwfn, which has proven to be a powerful wave function analysis software [64,65].

5. Conclusions

In summary, dinuclear vanadium-group transition metal carbonyl cation complexes in the form of VM(CO)n+ (M = V, Nb, and Ta) were produced in the gas phase via pulsed laser vaporization supersonic expansion and studied by infrared photodissociation spectroscopy in the carbonyl stretching frequency region. The geometric and electronic structures are assigned by comparison of the experimental spectra with simulated spectra derived from density functional calculations. The combination of laser-induced fragmentation mass spectra and infrared photodissociation spectra revealed that the observed VM(CO)9+ are saturated complexes, and there is no evidence to support the presence of the strongly bonded VM(CO)10+ under our experimental conditions. The dinuclear VM(CO)9+ cation can be irrefutably attributed to a quartet structure with CS symmetry, featuring two highly activated side-on bridging carbonyls and one slightly activated end-on semi-bridging carbonyl. It is worth emphasizing that the CS structure of VNb(CO)9+ and VTa(CO)9+ is characterized as the (OC)6M–V(CO)3 pattern, rather than the (OC)6V–M(CO)3 pattern, because the latter is much higher in energy. Accordingly, all the detected VM(CO)9+ complexes can be seen as the reaction products of two stable metal carbonyl fragments. Bonding analyses based on AdNDP and NAO methods along with the orbital correlation based on canonical fragment orbitals indicate the presence of the M–V d-d covalent interaction in VM(CO)9+, which renders the heavier metal center the favorable 18-electron configuration.
One of the striking results over the course of this work was the discovery of the vibrational bands below 1710 cm−1 of VM(CO)9+ cations, which represent highly activated CO ligands. DFT calculations manifest that the significant activation of the side-on bridging carbonyls in the VM(CO)9+ cations is due in large part to the diatomic cooperation of M–V, and the bridging carbonyls serve as both the classical σ donor and the less common π donor. The results offer important insight into the structure and bonding of dinuclear vanadium-containing transition metal carbonyl cluster cations and provide inspiration for the design of active vanadium-based diatomic catalysts.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/molecules29122831/s1, Figure S1: The comparison of experimental infrared photodissociation spectra of the V2(CO)10,11+ cation complexes; Figure S2: The comparison of experimental infrared photodissociation spectra of the VNb(CO)10,11+ cation complexes; Figure S3: The comparison of experimental infrared photodissociation spectra of the VTa(CO)10,11+ cation complexes; Figure S4: The calculated lowest-lying structures of the strongly bonded V2(CO)9+ cation at the (A) B3LYP, (B) BLYP, (C) PBE, and (D) TPSS level with the def2-TZVP basis set; Figure S5: The experimental infrared photodissociation spectrum of V2(CO)10+ and the simulated vibrational spectra of the eight lowest-lying V2(CO)9+ cation complexes at the B3LYP/def2-TZVP level in the carbonyl stretching frequency region; Figure S6: The calculated lowest-lying structures of the strongly bonded V2(CO)10+ cation; Figure S7: The experimental infrared spectrum of V2(CO)10+ and the simulated vibrational spectra of the low-lying strongly bonded V2(CO)10+ cation complexes in the carbonyl stretching frequency region; Figure S8: Comparisons of the experimental infrared spectrum of V2(CO)10+ and the simulated vibrational spectra of the saturated V2(CO)9+ cation and the low-lying CO-tagged V2(CO)10+ complexes in the carbonyl stretching frequency region; Figure S9: The calculated lowest-lying structures of the strongly bonded VNb(CO)9+ cation at the (A) B3LYP, (B) BLYP, (C) PBE, and (D) TPSS level with the def2-TZVP basis set; Figure S10: The experimental infrared photodissociation spectrum of VNb(CO)10+ and the simulated vibrational spectra of the eight lowest-lying VNb(CO)9+ cation complexes at the B3LYP/def2-TZVP level in the carbonyl stretching frequency region; Figure S11: The calculated lowest-lying structures of the strongly bonded VNb(CO)10+ cation; Figure S12: The experimental infrared spectrum of VNb(CO)10+ and the simulated vibrational spectra of the low-lying strongly bonded VNb(CO)10+ cation complexes in the carbonyl stretching frequency region; Figure S13: The calculated lowest-lying structures of the strongly bonded VTa(CO)9+ cation at the (A) B3LYP, (B) BLYP, (C) PBE, and (D) TPSS level with the def2-TZVP basis set; Figure S14: The experimental infrared photodissociation spectrum of VTa(CO)10+ and the simulated vibrational spectra of the eight lowest-lying VTa(CO)9+ cation complexes at the B3LYP/def2-TZVP level in the carbonyl stretching frequency region; Figure S15: The calculated lowest-lying structures of the strongly bonded VTa(CO)10+ cation; Figure S16: The experimental infrared spectrum of VTa(CO)10+ and the simulated vibrational spectra of the low-lying strongly bonded VTa(CO)10+ cation complexes in the carbonyl stretching frequency region; Figure S17: The comparison between the experimental vibrational spectrum of the solvated V(CO)7+ cluster cation and the simulated spectra of saturated V(CO)6+ cation derived from calculations at (a) B3LYP, (b) BLYP, (c) PBE, and (d) TPSSh level in conjunction with the def2-TZVP basis set; Figure S18: The comparison between the experimental vibrational spectrum of the solvated V2(CO)10+ cluster cation and the simulated spectra of saturated V2(CO)9+ cation derived from calculations at (a) B3LYP, (b) BLYP, (c) PBE, and (d) TPSSh level in conjunction with the def2-TZVP basis set; Table S1: The calculated charge and spin population of VM(CO)9+ based on natural population analysis; Table S2: Orbital composition of the AdNDP orbitals of the quartet VNb(CO)9+ complex cations based on natural atomic orbital method; Table S3: The calculated energy (in a.u.) of quartet V2+ dimer, quintet V+ cation (3d4), and quartet V atom (4s23d3), as well as the bond dissociation energy (BDE, in eV) at the B3LYP, BLYP, PBE, and TPSS level with the def2-TZVP basis set; Table S4: The calculated binding energy (in kcal/mol) of the weak bound CO ligand in the solvated VM(CO)10+ at the B3LYP, BLYP, PBE, and TPSS level with the def2-TZVP basis set; Table S5: The cartesian coordinates (Å) of the structures of VM(CO)9+ optimized at B3LYP/def2-TZVP level.

Author Contributions

Conceptualization, X.W. and J.H.; methodology, J.H.; formal analysis, J.H.; investigation, J.H.; resources, X.W.; data curation, J.H.; writing—original draft preparation, J.H.; writing—review and editing, J.H.; visualization, J.H.; supervision, X.W.; project administration, X.W.; funding acquisition, X.W. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by the National Natural Science Foundation of China, grant number 22273066.

Data Availability Statement

Data are contained within the article and Supplementary Materials.

Conflicts of Interest

The authors declare no conflicts of interest. The funders had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript; or in the decision to publish the results.

References

  1. Fei, H.; Dong, J.; Chen, D.; Hu, T.; Duan, X.; Shakir, I.; Huang, Y.; Duan, X. Single atom electrocatalysts supported on graphene or graphene-like carbons. Chem. Soc. Rev. 2019, 48, 5207–5241. [Google Scholar] [CrossRef]
  2. Zhang, T.; Walsh, A.G.; Yu, J.; Zhang, P. Single-atom alloy catalysts: Structural analysis, electronic properties and catalytic activities. Chem. Soc. Rev. 2021, 50, 569–588. [Google Scholar] [CrossRef] [PubMed]
  3. Lei, G.; Pan, H.; Mei, H.; Liu, X.; Lu, G.; Lou, C.; Li, Z.; Zhang, J. Emerging single atom catalysts in gas sensors. Chem. Soc. Rev. 2022, 51, 7260–7280. [Google Scholar] [CrossRef]
  4. An, Q.; Yang, C.; Xu, Y.; Yu, F.; Jiang, J.; Gong, C.; Li, B.; Zhang, J.; Liu, Q. The superiority and perspectives in single-atom site and multi-atom site catalysts for energy conversion. APL Mater. 2022, 10, 120901. [Google Scholar] [CrossRef]
  5. Sun, L.B.; Reddu, V.; Wang, X. Multi-atom cluster catalysts for efficient electrocatalysis. Chem. Soc. Rev. 2022, 51, 8923–8956. [Google Scholar] [CrossRef]
  6. Wang, Z.; Zou, G.; Park, J.H.; Zhang, K. Progress in design and preparation of multi-atom catalysts for photocatalytic CO2 reduction. Sci. China-Mater. 2024, 67, 397–423. [Google Scholar] [CrossRef]
  7. Ying, Y.; Luo, X.; Qiao, J.; Huang, H. “More is different”: Synergistic effect and structural engineering in double-atom catalysts. Adv. Funct. Mater. 2021, 31, 2007423. [Google Scholar] [CrossRef]
  8. Zhang, J.; Huang, Q.-a.; Wang, J.; Wang, J.; Zhang, J.; Zhao, Y. Supported dual-atom catalysts: Preparation, characterization, and potential applications. Chin. J. Catal. 2020, 41, 783–798. [Google Scholar] [CrossRef]
  9. Pedersen, A.; Barrio, J.; Li, A.; Jervis, R.; Brett, D.J.L.; Titirici, M.M.; Stephens, I.E.L. Dual-metal atom electrocatalysts: Theory, synthesis, characterization, and applications. Adv. Energy Mater. 2022, 12, 2102715. [Google Scholar] [CrossRef]
  10. Ricks, A.M.; Reed, Z.E.; Duncan, M.A. Infrared spectroscopy of mass-selected metal carbonyl cations. J. Mol. Spectrosc. 2011, 266, 63–74. [Google Scholar] [CrossRef]
  11. Frenking, G.; Fernandez, I.; Holzmann, N.; Pan, S.; Krossing, I.; Zhou, M. Metal-CO bonding in mononuclear transition metal carbonyl complexes. JACS Au 2021, 1, 623–645. [Google Scholar] [CrossRef] [PubMed]
  12. Wang, G.-J.; Zhou, M.-F. Infrared spectra, structures and bonding of binuclear transition metal carbonyl cluster ions. Chin. J. Chem. Phys. 2018, 31, 1–11. [Google Scholar] [CrossRef]
  13. Wang, G.J.; Zhou, M.F.; Goettel, J.T.; Schrobilgen, G.J.; Su, J.; Li, J.; Schloder, T.; Riedel, S. Identification of an iridium-containing compound with a formal oxidation state of IX. Nature 2014, 514, 475–478. [Google Scholar] [CrossRef] [PubMed]
  14. Chi, C.; Wang, J.-Q.; Qu, H.; Li, W.-L.; Meng, L.; Luo, M.; Li, J.; Zhou, M. Preparation and characterization of uranium-iron triple-bonded UFe(CO)3− and OUFe(CO)3− complexes. Angew. Chem. Int. Ed. 2017, 56, 6932–6936. [Google Scholar] [CrossRef] [PubMed]
  15. Wu, X.; Zhao, L.; Jin, J.; Pan, S.; Li, W.; Jin, X.; Wang, G.; Zhou, M.; Frenking, G. Observation of alkaline earth complexes M(CO)8 (M = Ca, Sr, or Ba) that mimic transition metals. Science 2018, 361, 912–916. [Google Scholar] [CrossRef]
  16. Chi, C.; Wang, J.-Q.; Hu, H.-S.; Zhang, Y.-Y.; Li, W.-L.; Meng, L.; Luo, M.; Zhou, M.; Li, J. Quadruple bonding between iron and boron in the BFe(CO)3− complex. Nat. Commun. 2019, 10, 4713. [Google Scholar] [CrossRef] [PubMed]
  17. Wang, J.-Q.; Chi, C.; Hu, H.-S.; Li, X.; Luo, M.; Li, J.; Zhou, M. Multiple bonding between group-3 metals and Fe(CO)3−. Angew. Chem. Int. Ed. 2020, 59, 2344–2348. [Google Scholar] [CrossRef] [PubMed]
  18. Jin, X.; Bai, Y.; Zhou, Y.; Wang, G.; Zhao, L.; Zhou, M.; Frenking, G. Highly coordinated heteronuclear calcium-iron carbonyl cation complexes CaFe(CO)n+ (n = 5–12) with d-d bonding. Angew. Chem. Int. Ed. 2021, 60, 13865–13870. [Google Scholar] [CrossRef] [PubMed]
  19. Wang, G.; Zhao, J.; Hu, H.-S.; Li, J.; Zhou, M. Formation and characterization of BeFe(CO)3− anion with beryllium-iron bonding. Angew. Chem. Int. Ed. 2021, 60, 9334–9338. [Google Scholar] [CrossRef]
  20. Wrighton, M. Photochemistry of metal carbonyls. Chem. Rev. 1974, 74, 401–430. [Google Scholar] [CrossRef]
  21. Aubke, F.; Wang, C. Carbon monoxide as a σ-donor ligand in coordination chemistry. Coord. Chem. Rev. 1994, 137, 483–524. [Google Scholar] [CrossRef]
  22. Wang, G.; Cui, J.; Chi, C.; Zhou, X.; Li, Z.H.; Xing, X.; Zhou, M. Bonding in homoleptic iron carbonyl cluster cations: A combined infrared photodissociation spectroscopic and theoretical study. Chem. Sci. 2012, 3, 3272–3279. [Google Scholar] [CrossRef]
  23. Chi, C.; Cui, J.; Li, Z.H.; Xing, X.; Wang, G.; Zhou, M. Infrared photodissociation spectra of mass selected homoleptic dinuclear iron carbonyl cluster anions in the gas phase. Chem. Sci. 2012, 3, 1698–1706. [Google Scholar] [CrossRef]
  24. Cui, J.; Wang, G.; Zhou, X.; Chi, C.; Hua Li, Z.; Liu, Z.; Zhou, M. Infrared photodissociation spectra of mass selected homoleptic nickel carbonyl cluster cations in the gas phase. Phys. Chem. Chem. Phys. 2013, 15, 10224–10232. [Google Scholar] [CrossRef]
  25. Cui, J.; Zhou, X.; Wang, G.; Chi, C.; Liu, Z.; Zhou, M. Infrared photodissociation spectroscopy of mass selected homoleptic copper carbonyl cluster cations in the gas phase. J. Phys. Chem. A 2013, 117, 7810–7817. [Google Scholar] [CrossRef]
  26. Zhang, N.; Luo, M.; Chi, C.; Wang, G.; Cui, J.; Zhou, M. Infrared photodissociation spectroscopy of mass-selected heteronuclear iron-copper carbonyl cluster anions in the gas phase. J. Phys. Chem. A 2015, 119, 4142–4150. [Google Scholar] [CrossRef] [PubMed]
  27. Qu, H.; Kong, F.; Wang, G.; Zhou, M. Infrared photodissociation spectroscopy of heterodinuclear iron-zinc and cobalt-zinc carbonyl cation complexes. J. Phys. Chem. A 2017, 121, 1628–1633. [Google Scholar] [CrossRef]
  28. Qu, H.; Kong, F.; Wang, G.; Zhou, M. Infrared photodissociation spectroscopic and theoretical study of heteronuclear transition metal carbonyl cluster cations in the gas phase. J. Phys. Chem. A 2016, 120, 7287–7293. [Google Scholar] [CrossRef]
  29. Zhou, X.; Cui, J.; Li, Z.H.; Wang, G.; Zhou, M. Infrared photodissociation spectroscopic and theoretical study of homoleptic dinuclear chromium carbonyl cluster cations with a linear bridging carbonyl group. J. Phys. Chem. A 2012, 116, 12349–12356. [Google Scholar] [CrossRef]
  30. Zhou, X.; Cui, J.; Li, Z.H.; Wang, G.; Liu, Z.; Zhou, M. Carbonyl bonding on oxophilic metal centers: Infrared photodissociation spectroscopy of mononuclear and dinuclear titanium carbonyl cation complexes. J. Phys. Chem. A 2013, 117, 1514–1521. [Google Scholar] [CrossRef]
  31. Langeslay, R.R.; Kaphan, D.M.; Marshall, C.L.; Stair, P.C.; Sattelberger, A.P.; Delferro, M. Catalytic applications of vanadium: A mechanistic perspective. Chem. Rev. 2019, 119, 2128–2191. [Google Scholar] [CrossRef] [PubMed]
  32. Chen, Q.; Zhao, Y.-X.; Jiang, L.-X.; Li, H.-F.; Chen, J.-J.; Zhang, T.; Liu, Q.-Y.; He, S.-G. Thermal activation of methane by vanadium boride cluster cations VBn+ (n = 3–6). Phys. Chem. Chem. Phys. 2018, 20, 4641–4645. [Google Scholar] [CrossRef] [PubMed]
  33. Zhang, M.-Y.; Liu, A.-A.; Jiao, L.; Zhang, S.; Jiang, L.-H.; Kong, X.; Pang, D.-W. Capture of small clusters by ligand-solvent interaction. J. Chem. Phys. 2023, 159, 064301. [Google Scholar] [CrossRef]
  34. Gan, W.; Geng, L.; Huang, B.; Hansen, K.; Luo, Z. Dehydrogenation of diborane on small Nbn+ clusters. Phys. Chem. Chem. Phys. 2024, 26, 9586–9592. [Google Scholar] [CrossRef]
  35. Brathwaite, A.D.; Ricks, A.M.; Duncan, M.A. Infrared photodissociation spectroscopy of vanadium oxide-carbonyl cations. J. Phys. Chem. A 2013, 117, 13435–13442. [Google Scholar] [CrossRef] [PubMed]
  36. Zhou, M.; Zhou, Z.; Zhuang, J.; Li, Z.H.; Fan, K.; Zhao, Y.; Zheng, X. Carbon dioxide coordination and activation by niobium oxide molecules. J. Phys. Chem. A 2011, 115, 14361–14369. [Google Scholar] [CrossRef]
  37. Iskra, A.; Gentleman, A.S.; Cunningham, E.M.; Mackenzie, S.R. Carbon dioxide binding to metal oxides: Infrared spectroscopy of NbO2+(CO2)n and TaO2+(CO2)n complexes. Int. J. Mass Spectrom. 2019, 435, 93–100. [Google Scholar] [CrossRef]
  38. Jian, X.; Xin, K.; Hu, J.; Zhang, L.; Wang, X.; Wang, G. Infrared spectroscopy of solvation in the TaO2+ hydrolysis reaction. J. Phys. Chem. A 2021, 125, 5054–5060. [Google Scholar] [CrossRef]
  39. Xin, K.; Chen, Y.; Zhang, L.; Xu, B.; Wang, X.; Wang, G. Infrared photodissociation spectroscopic investigation on VO+ and NbO+ hydrolysis catalyzed by water molecules. Phys. Chem. Chem. Phys. 2021, 23, 528–535. [Google Scholar] [CrossRef]
  40. Ricks, A.M.; Reed, Z.D.; Duncan, M.A. Seven-coordinate homoleptic metal carbonyls in the gas phase. J. Am. Chem. Soc. 2009, 131, 9176–9177. [Google Scholar] [CrossRef]
  41. Ricks, A.M.; Brathwaite, A.D.; Duncan, M.A. Coordination and spin states in vanadium carbonyl complexes V(CO)n+, n = 1–7 revealed with ir spectroscopy. J. Phys. Chem. A 2013, 117, 1001–1010. [Google Scholar] [CrossRef]
  42. Russon, L.M.; Heidecke, S.A.; Birke, M.K.; Conceicao, J.; Morse, M.D.; Armentrout, P.B. Photodissociation measurements of bond dissociation energies: Ti2+, V2+, Co2+, and Co3+. J. Chem. Phys. 1994, 100, 4747–4755. [Google Scholar] [CrossRef]
  43. Pyykko, P. Relativistic effects in structural chemistry. Chem. Rev. 1988, 88, 563–594. [Google Scholar] [CrossRef]
  44. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09, Revision a.2; Gaussian Inc.: Pittsburgh, PA, USA, 2009. [Google Scholar]
  45. Lee, C.; Yang, W.; Parr, R.G. Development of the colle-salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B Condens Matter. 1988, 37, 785–789. [Google Scholar] [CrossRef] [PubMed]
  46. Becke, A.D. Density-functional thermochemistry. 3. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
  47. Sousa, S.F.; Fernandes, P.A.; Ramos, M.J. General performance of density functionals. J. Phys. Chem. A 2007, 111, 10439–10452. [Google Scholar] [CrossRef] [PubMed]
  48. Cramer, C.J.; Truhlar, D.G. Density functional theory for transition metals and transition metal chemistry. Phys. Chem. Chem. Phys. 2009, 11, 10757–10816. [Google Scholar] [PubMed]
  49. Katari, M.; Nicol, E.; Steinmetz, V.; van der Rest, G.; Carmichael, D.; Frison, G. Improved infrared spectra prediction by DFT from a new experimental database. Chem. Eur. J. 2017, 23, 8414–8423. [Google Scholar] [CrossRef] [PubMed]
  50. Weigend, F.; Ahlrichs, R. Balanced basis sets of split valence, triple zeta valence and quadruple zeta valence quality for H to Rn: Design and assessment of accuracy. Phys. Chem. Chem. Phys. 2005, 7, 3297–3305. [Google Scholar] [CrossRef]
  51. Andrae, D.; Haussermann, U.; Dolg, M.; Stoll, H.; Preuss, H. Energy-adjusted ab initio pseudopotentials for the 2nd and 3rd row transition elements. Theor. Chim. Acta 1990, 77, 123–141. [Google Scholar] [CrossRef]
  52. Schultz, N.E.; Zhao, Y.; Truhlar, D.G. Databases for transition element bonding:  Metal–metal bond energies and bond lengths and their use to test hybrid, hybrid meta, and meta density functionals and generalized gradient approximations. J. Phys. Chem. A 2005, 109, 4388–4403. [Google Scholar] [CrossRef] [PubMed]
  53. Buehl, M.; Kabrede, H. Geometries of transition-metal complexes from density-functional theory. J. Chem. Theory Comput. 2006, 2, 1282–1290. [Google Scholar] [CrossRef] [PubMed]
  54. Aoto, Y.A.; de Lima Batista, A.P.; Köhn, A.; de Oliveira-Filho, A.G.S. How to arrive at accurate benchmark values for transition metal compounds: Computation or experiment? J. Chem. Theory Comput. 2017, 13, 5291–5316. [Google Scholar] [CrossRef]
  55. Becke, A.D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef]
  56. Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
  57. Ernzerhof, M.; Scuseria, G.E. Assessment of the perdew–burke–ernzerhof exchange-correlation functional. J. Chem. Phys. 1999, 110, 5029–5036. [Google Scholar] [CrossRef]
  58. Tao, J.; Perdew, J.P.; Staroverov, V.N.; Scuseria, G.E. Climbing the density functional ladder: Nonempirical meta--generalized gradient approximation designed for molecules and solids. Phys. Rev. Lett. 2003, 91, 146401. [Google Scholar] [CrossRef]
  59. Mayer, I. Charge, bond order and valence in the ab initio SCF theory. Chem. Phys. Lett. 1983, 97, 270–274. [Google Scholar] [CrossRef]
  60. Reed, A.E.; Weinstock, R.B.; Weinhold, F. Natural population analysis. J. Chem. Phys. 1985, 83, 735–746. [Google Scholar] [CrossRef]
  61. Zubarev, D.Y.; Boldyrev, A.I. Developing paradigms of chemical bonding: Adaptive natural density partitioning. Phys. Chem. Chem. Phys. 2008, 10, 5207–5217. [Google Scholar] [CrossRef]
  62. Glendening, E.D.; Landis, C.R.; Weinhold, F. Natural bond orbital methods. WIREs Comput. Mol. Sci. 2012, 2, 1–42. [Google Scholar] [CrossRef]
  63. Dapprich, S.; Frenking, G. Investigation of donor-acceptor interactions—A charge decomposition analysis using fragment molecular-orbitals. J. Phys. Chem. 1995, 99, 9352–9362. [Google Scholar] [CrossRef]
  64. Lu, T.; Chen, F. Multiwfn: A multifunctional wavefunction analyzer. J. Comput. Chem. 2012, 33, 580–592. [Google Scholar] [CrossRef]
  65. Lu, T.; Chen, F. Calculation of molecular orbital composition. Acta Chim. Sin. 2011, 69, 2393–2406. [Google Scholar]
Molecules 29 02831 g001
Figure 1. The representative mass spectrum of the cation complexes in the m/z range of 45–600 produced by pulsed laser ablation of a pure vanadium target (a), the mixed vanadium–niobium target (b), and vanadium–tantalum target (c) in an expansion of 10% CO seeded in helium at a background pressure of 1.2 MPa.
Figure 1. The representative mass spectrum of the cation complexes in the m/z range of 45–600 produced by pulsed laser ablation of a pure vanadium target (a), the mixed vanadium–niobium target (b), and vanadium–tantalum target (c) in an expansion of 10% CO seeded in helium at a background pressure of 1.2 MPa.
Molecules 29 02831 g001
Molecules 29 02831 g002
Figure 2. The laser-induced photofragmentation mass spectra of mass-selected V2(CO)10,11+ (a), VNb(CO)10,11+ (b), and VTa(CO)10,11+ (c). The laser used in (a) is set at 2130 cm−1 with an energy of 1.0 mJ/pulse, and the laser used in (b,c) is set at 2150 cm−1 with an energy of 1.2 mJ/pulse.
Figure 2. The laser-induced photofragmentation mass spectra of mass-selected V2(CO)10,11+ (a), VNb(CO)10,11+ (b), and VTa(CO)10,11+ (c). The laser used in (a) is set at 2130 cm−1 with an energy of 1.0 mJ/pulse, and the laser used in (b,c) is set at 2150 cm−1 with an energy of 1.2 mJ/pulse.
Molecules 29 02831 g002
Molecules 29 02831 g003
Figure 3. The experimental infrared photodissociation spectra of (a) V2(CO)10+, (b) VNb(CO)10+ and (c) VTa(CO)10+ via elimination of the outermost physically bound CO in the carbonyl stretching frequency region, leading to the formation of VM(CO)9+ (M = V, Nb, and Ta). The vertical dashed line indicates the C–O vibration frequency of free CO at 2143 cm−1.
Figure 3. The experimental infrared photodissociation spectra of (a) V2(CO)10+, (b) VNb(CO)10+ and (c) VTa(CO)10+ via elimination of the outermost physically bound CO in the carbonyl stretching frequency region, leading to the formation of VM(CO)9+ (M = V, Nb, and Ta). The vertical dashed line indicates the C–O vibration frequency of free CO at 2143 cm−1.
Molecules 29 02831 g003
Molecules 29 02831 g004
Figure 4. The experimental infrared photodissociation spectrum of V2(CO)10+ and the simulated vibrational spectrum of the saturated V2(CO)9+ cation complexes in the carbonyl stretching frequency region.
Figure 4. The experimental infrared photodissociation spectrum of V2(CO)10+ and the simulated vibrational spectrum of the saturated V2(CO)9+ cation complexes in the carbonyl stretching frequency region.
Molecules 29 02831 g004
Molecules 29 02831 g005
Figure 5. The experimental infrared photodissociation spectra of VNb(CO)10+ and VTa(CO)10+ and the simulated vibrational spectra of the saturated VNb(CO)9+ and VTa(CO)9+ cation complexes in the carbonyl stretching frequency region.
Figure 5. The experimental infrared photodissociation spectra of VNb(CO)10+ and VTa(CO)10+ and the simulated vibrational spectra of the saturated VNb(CO)9+ and VTa(CO)9+ cation complexes in the carbonyl stretching frequency region.
Molecules 29 02831 g005
Molecules 29 02831 g006
Figure 6. The geometric structure of the VM(CO)9+ (M = V, Nb, and Ta) cations, as well as the calculated bond distance (in Å, black font) and Mayer bond order (red font in parentheses) of selected bonds at the B3LYP, BLYP, PBE, and TPSS level with the def2-TZVP basis set.
Figure 6. The geometric structure of the VM(CO)9+ (M = V, Nb, and Ta) cations, as well as the calculated bond distance (in Å, black font) and Mayer bond order (red font in parentheses) of selected bonds at the B3LYP, BLYP, PBE, and TPSS level with the def2-TZVP basis set.
Molecules 29 02831 g006
Molecules 29 02831 g007
Figure 7. Bonding scheme for the orbital interactions between the doublet Nb(CO)6 fragment and the quintet V(CO)3+ fragment, as well as the canonical Kohn–Sham molecular orbitals of the CS structure of the quartet VNb(CO)9+ at the TPSS/def2-TZVP level. The electrons in the corresponding orbitals are marked with red dots. All the molecular orbitals are plotted with isosurfaces = 0.05 a.u.
Figure 7. Bonding scheme for the orbital interactions between the doublet Nb(CO)6 fragment and the quintet V(CO)3+ fragment, as well as the canonical Kohn–Sham molecular orbitals of the CS structure of the quartet VNb(CO)9+ at the TPSS/def2-TZVP level. The electrons in the corresponding orbitals are marked with red dots. All the molecular orbitals are plotted with isosurfaces = 0.05 a.u.
Molecules 29 02831 g007
Molecules 29 02831 g008
Figure 8. Localized nc-ne bonds and occupation numbers in the quartet VNb(CO)9+ obtained by AdNDP analyses at the TPSS/def2-TZVP level. All the molecular orbitals are plotted with isosurfaces = 0.05 a.u.
Figure 8. Localized nc-ne bonds and occupation numbers in the quartet VNb(CO)9+ obtained by AdNDP analyses at the TPSS/def2-TZVP level. All the molecular orbitals are plotted with isosurfaces = 0.05 a.u.
Molecules 29 02831 g008
Table 1. Comparison of the calculated carbonyl stretching frequencies (in cm−1) of the VM(CO)10+ (M = V, Nb, and Ta) cations at B3LYP/def2-TZVP level of theory with the experimental values in the present work. IR intensities are listed in parentheses in km/mol, and all frequencies are scaled with a factor of 0.968.
Table 1. Comparison of the calculated carbonyl stretching frequencies (in cm−1) of the VM(CO)10+ (M = V, Nb, and Ta) cations at B3LYP/def2-TZVP level of theory with the experimental values in the present work. IR intensities are listed in parentheses in km/mol, and all frequencies are scaled with a factor of 0.968.
ComplexExptl.Calc.Mode a
V2(CO)10+16461688 (501)C–O (side-on bridge) asym-str.
17001727 (503)C–O (side-on bridge) sym-str.
19841994 (627)C–O (end-on semi-bridge) str.
21202091 (478), 2094 (628)C–O (end-on at left-hand V) asym-str.
21302108 (2332)C–O (end-on at left-hand V) sym-str.
21582143 (397)C–O (end-on at right-hand V) asym-str.
21642149 (428)C–O (end-on at right-hand V) asym-str.
21752164 (387)C–O (end-on at right-hand V) sym-str.
VNb(CO)10+16391671 (531)C–O (side-on bridge) asym-str.
17061721 (673)C–O (side-on bridge) sym-str.
20002004 (618)C–O (end-on semi-bridge) str.
2084, 2111, 2123, 2142, 2151, 2161, 21782089 (524), 2092 (773), 2114 (2161), 2137 (409), 2145 (458), 2162 (367)C–O (terminal bound to V and Nb) asym-str./sym-str.
VTa(CO)10+16361668 (589)C–O (side-on bridge) asym-str.
17091718 (662)C–O (side-on bridge) sym-str.
19921994 (605)C–O (end-on semi-bridge) str.
2072, 2092, 2107, 2133, 2149, 2158, 21802084 (553), 2090 (759), 2111 (2184), 2136 (421), 2142 (441), 2159 (338)C–O (terminal bound to V and Nb) asym-str./sym-str.
a: The coupled carbonyl antisymmetric and symmetric stretching vibrations are marked as “asym-str.” and “sym-str.”, respectively, and the stretching vibration of a single carbonyl is indicated as “str.”.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Hu, J.; Wang, X. Infrared Photodissociation Spectroscopy of Dinuclear Vanadium-Group Metal Carbonyl Complexes: Diatomic Synergistic Activation of Carbon Monoxide. Molecules 2024, 29, 2831. https://doi.org/10.3390/molecules29122831

AMA Style

Hu J, Wang X. Infrared Photodissociation Spectroscopy of Dinuclear Vanadium-Group Metal Carbonyl Complexes: Diatomic Synergistic Activation of Carbon Monoxide. Molecules. 2024; 29(12):2831. https://doi.org/10.3390/molecules29122831

Chicago/Turabian Style

Hu, Jin, and Xuefeng Wang. 2024. "Infrared Photodissociation Spectroscopy of Dinuclear Vanadium-Group Metal Carbonyl Complexes: Diatomic Synergistic Activation of Carbon Monoxide" Molecules 29, no. 12: 2831. https://doi.org/10.3390/molecules29122831

APA Style

Hu, J., & Wang, X. (2024). Infrared Photodissociation Spectroscopy of Dinuclear Vanadium-Group Metal Carbonyl Complexes: Diatomic Synergistic Activation of Carbon Monoxide. Molecules, 29(12), 2831. https://doi.org/10.3390/molecules29122831

Article Metrics

Back to TopTop