Magnetic Property, Heat Capacity and Crystal Structure of Mononuclear Compounds Based on Substitute Tetrazole Ligand
Abstract
:1. Introduction
2. Results and Discussion
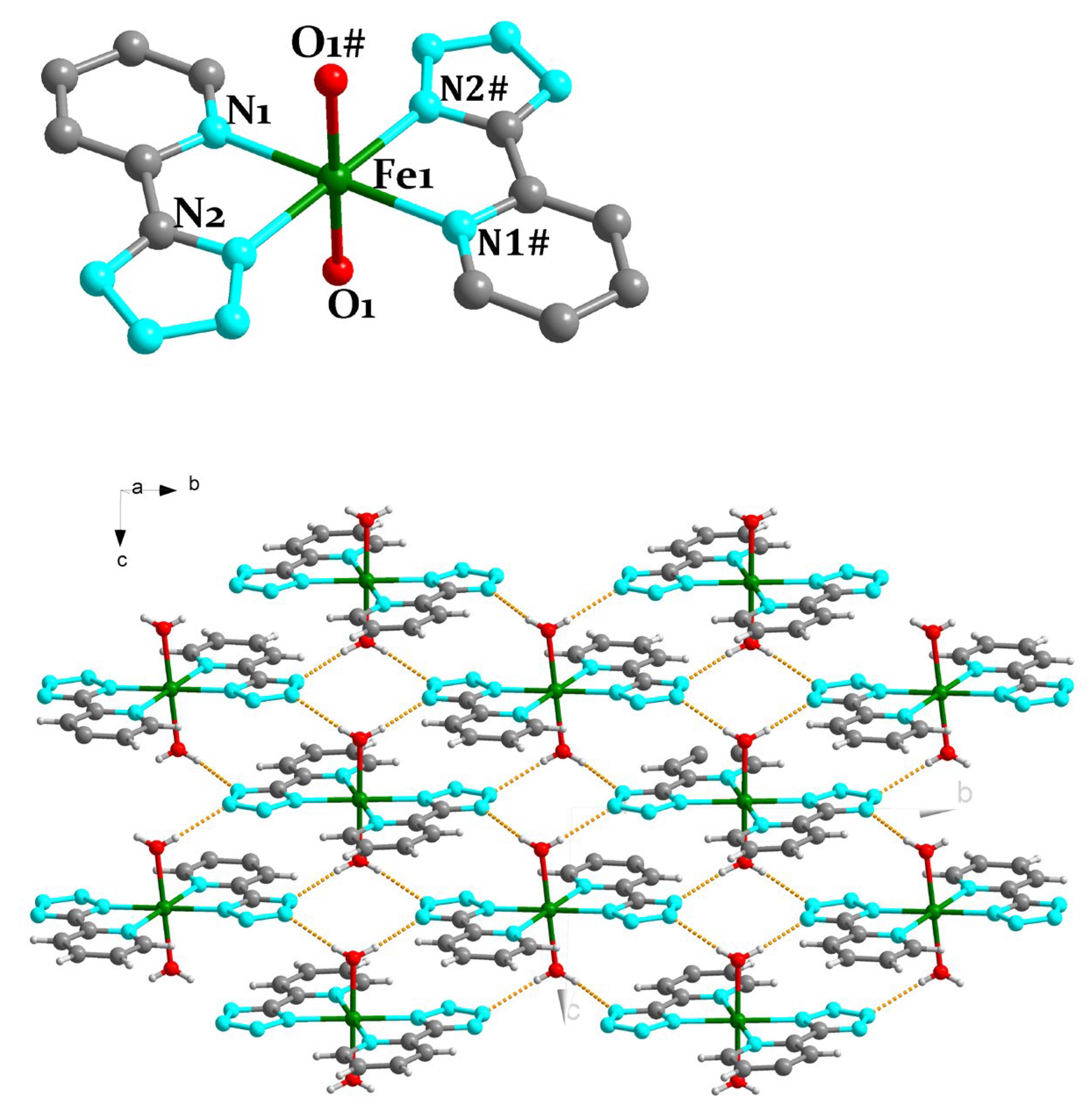
2.1. Crystal Structure
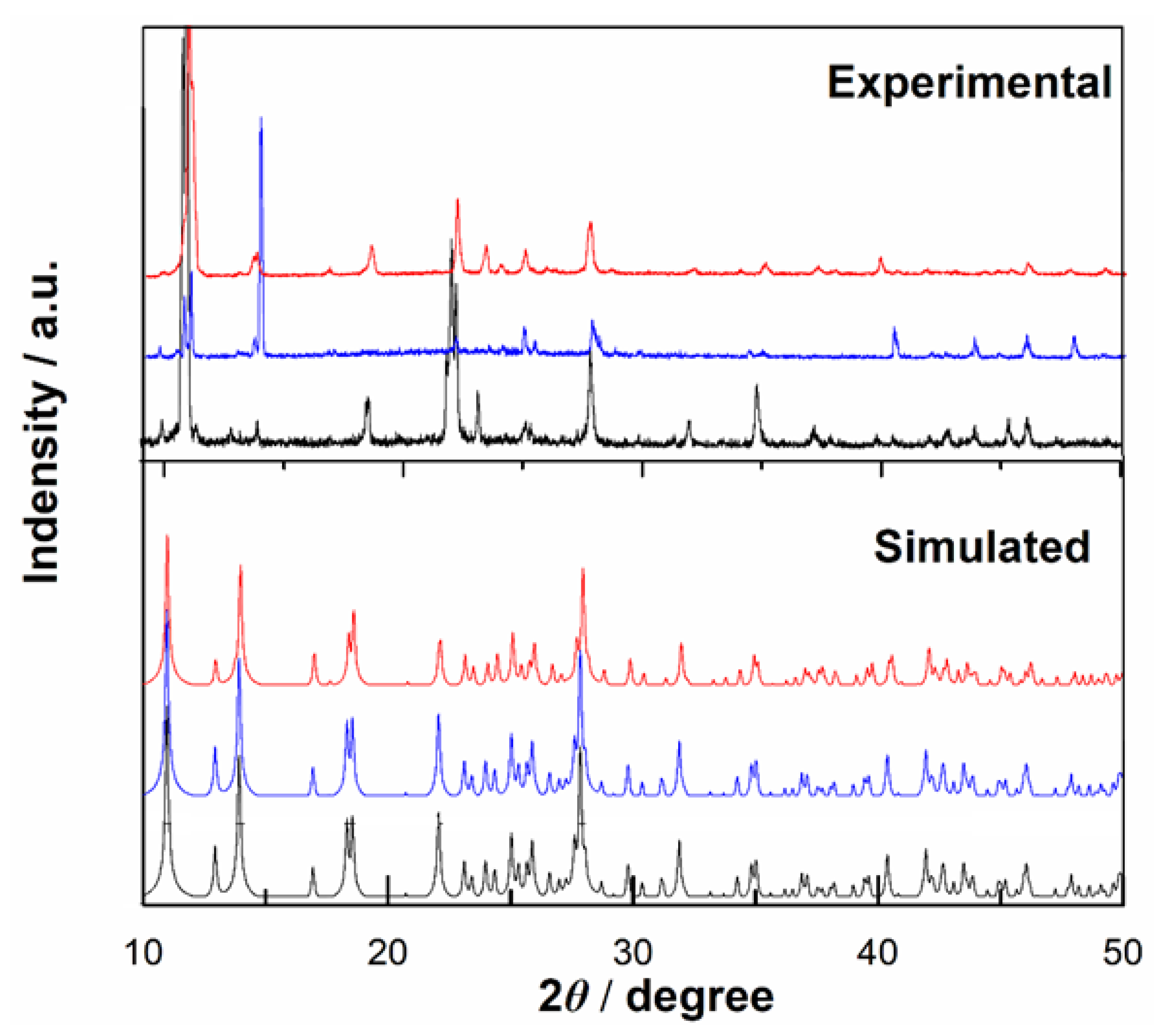
2.2. Powder X-ray Diffraction (XRD)
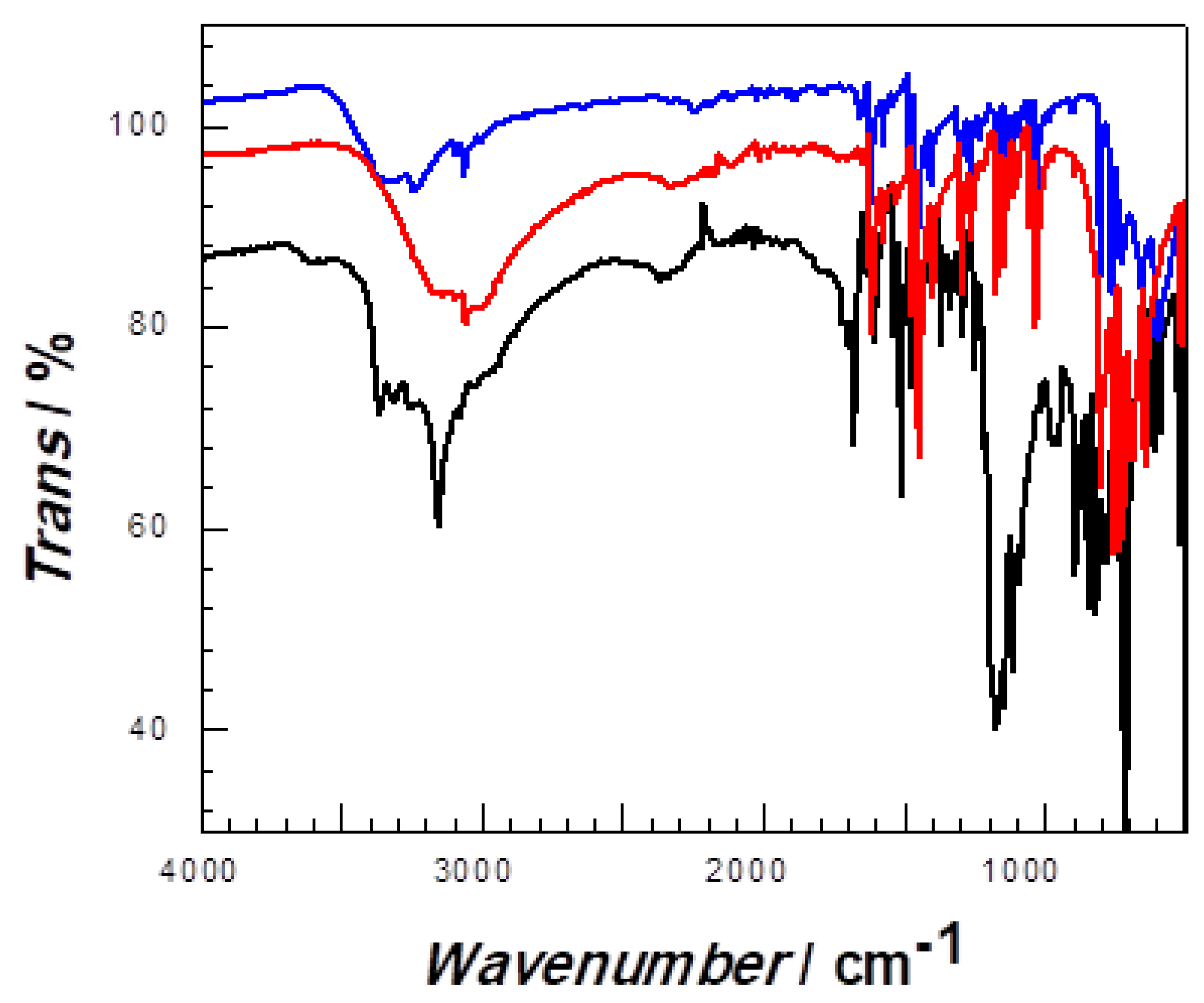
2.3. Fourier Transform Infrared (FTIR) Characterization
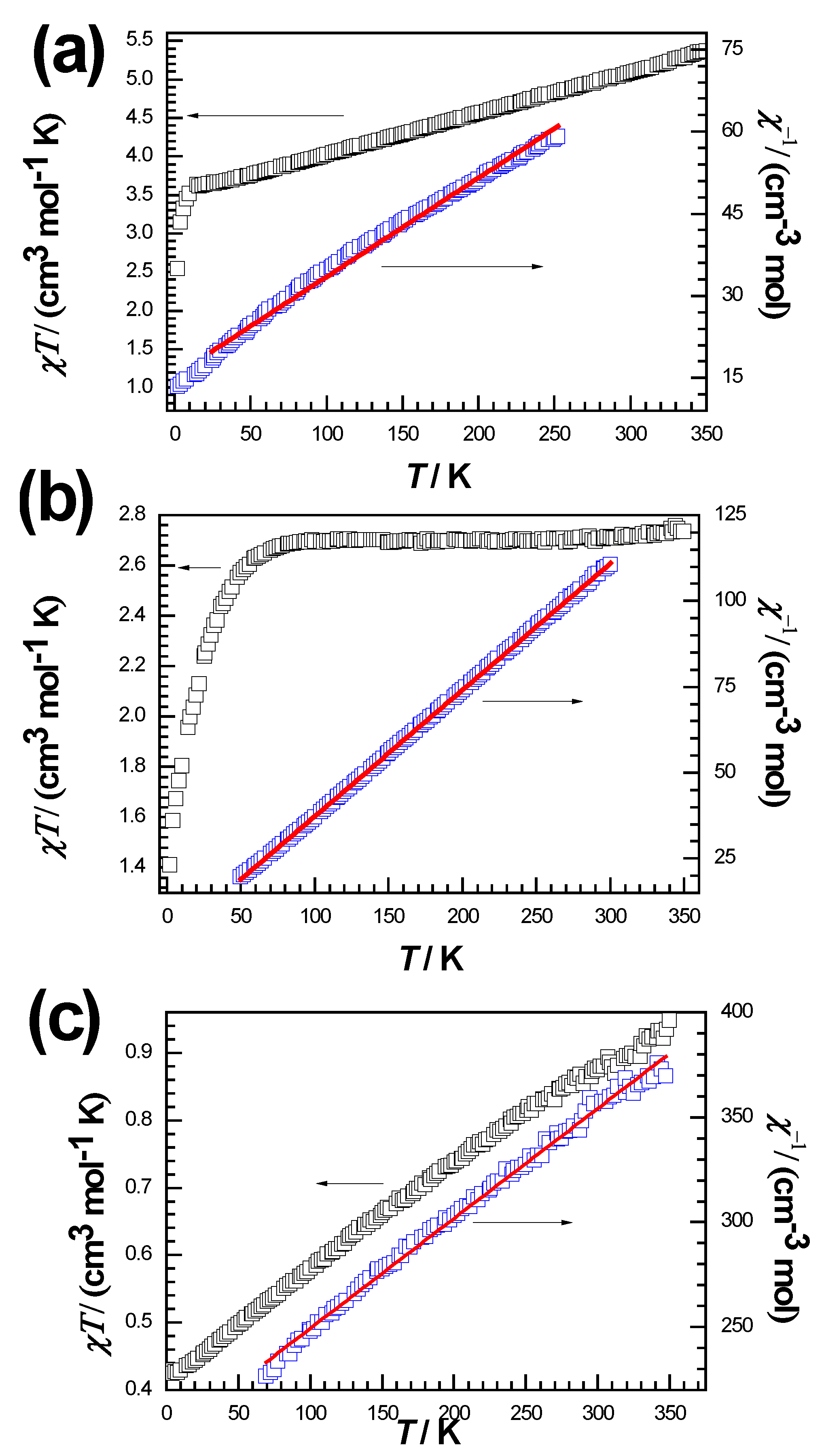
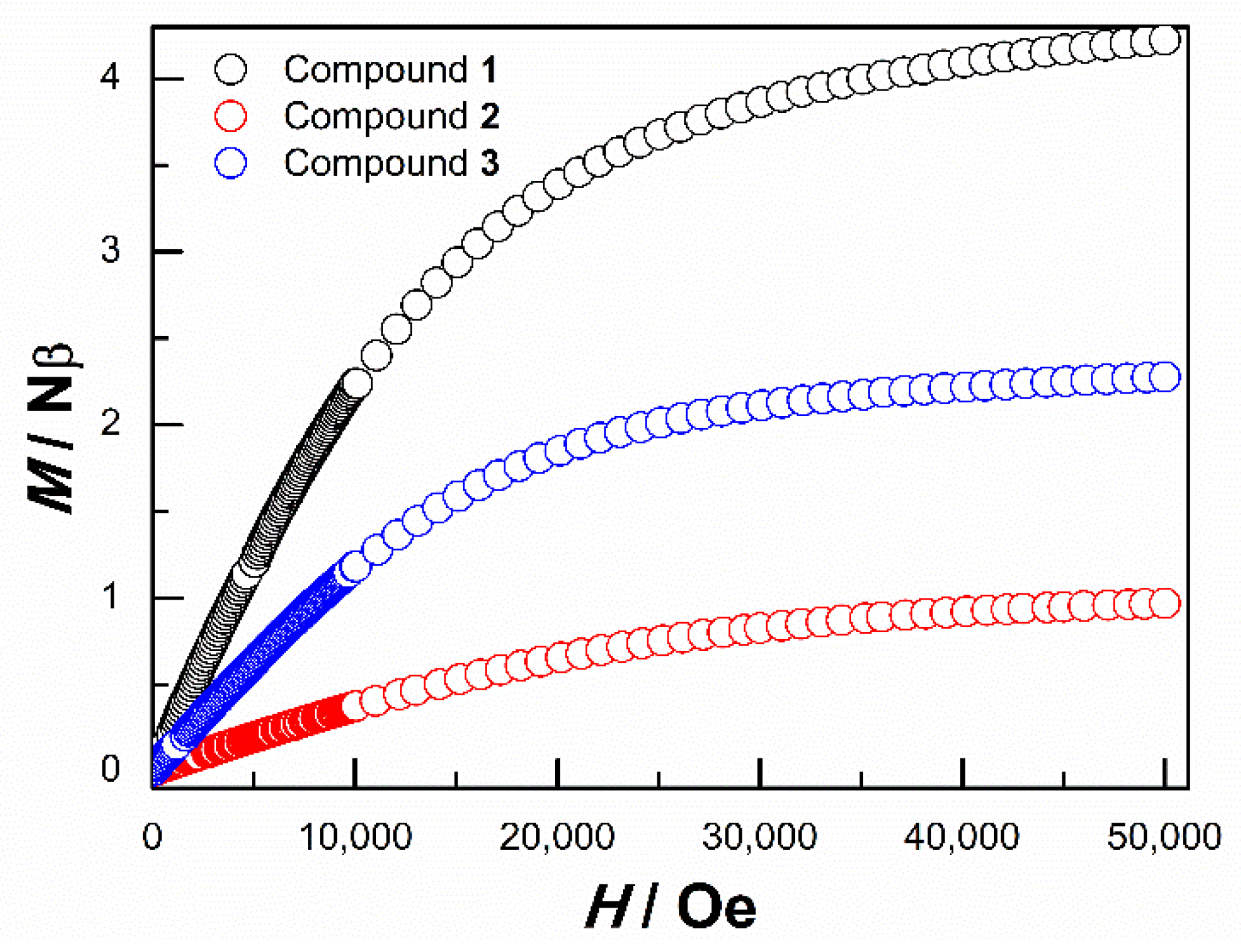
2.4. Magnetic Properties
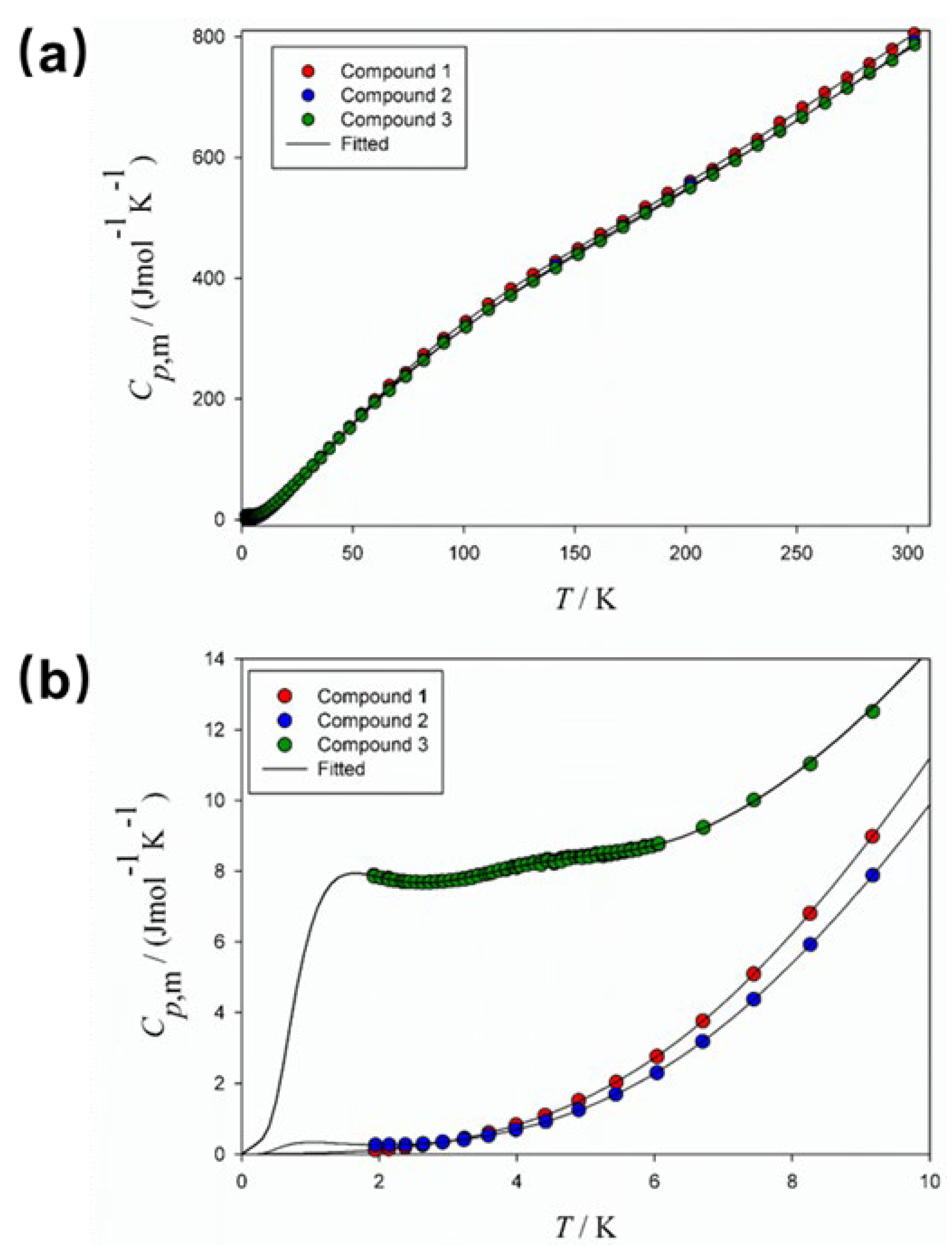
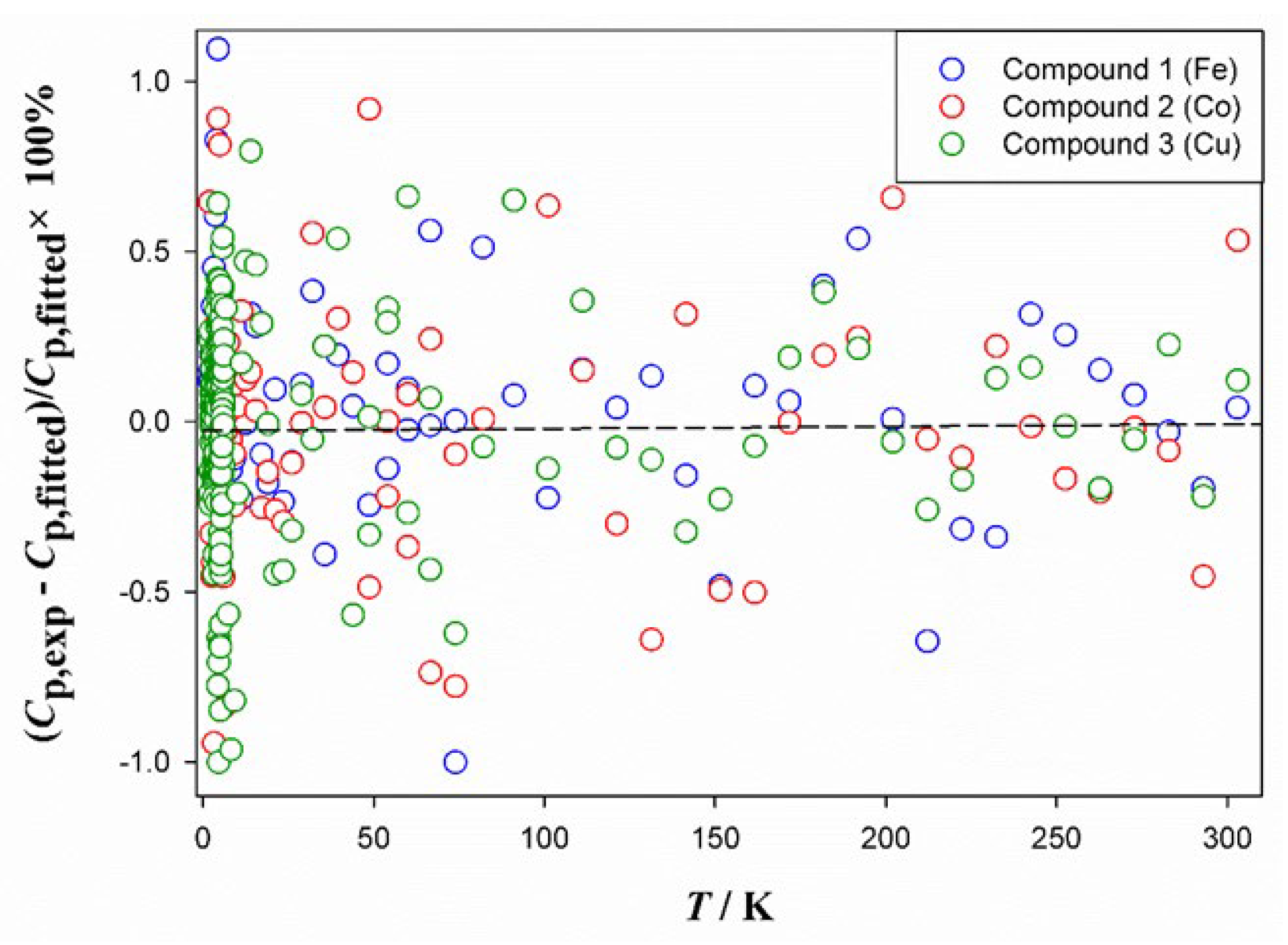
2.5. Heat Capacity and Thermodynamic Functions
3. Materials and Methods
3.1. Materials
3.2. Synthesis of [Fe{2-(1H-tetrazol-5-yl)pyridine}2(H2O)2] (1)
3.3. Synthesis of [Co{2-(1H-tetrazol-5-yl)pyridine}2(H2O)2] (2) and [Cu{2-(1H-tetrazol-5-yl)pyridine}2(H2O)2] (3)
3.4. Physical Characterization
3.5. X-ray Structure Determination
3.6. Magnetic and Calorimetric Measurements
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Gatteschi, D. Single molecule magnets: A new class of magnetic materials. J. Alloys Compd. 2001, 317–318, 8–12. [Google Scholar] [CrossRef]
- Sessoli, R.; Gatteschi, D.; Caneschi, A.; Novak, M.A. Magnetic bistability in a metal-ion cluster. Nature 1993, 365, 141–143. [Google Scholar] [CrossRef]
- Gatteschi, D.; Sessoli, R. Quantum Tunneling of Magnetization and related phenomena in molecular materials. Angew. Chem. Int. Ed. 2003, 42, 268–297. [Google Scholar] [CrossRef] [PubMed]
- Ding, Y.S.; Yu, K.X.; Reta, D.; Ortu, F.; Winpenny, R.E.P.; Zheng, Y.Z.; Chilton, N.F. Field- and temperature-dependent quantum tunnelling of the magnetisation in a large barrier single-molecule magnet. Nat. Commun. 2018, 9, 3134. [Google Scholar] [CrossRef] [PubMed]
- Gao, E.Q.; Liu, N.; Cheng, A.L.; Gao, S. Novel frustrated magnetic lattice based on triangular [Mn3(μ3-F)] clusters with tetrazole ligands. Chem. Commun. 2007, 2470–2472. [Google Scholar] [CrossRef]
- HHu, C.; Kang, X.M.; Cao, C.S.; Cheng, P.; Zhao, B. First tetrazole-bridged d–f heterometallic MOFs with a large magnetic entropy change. Chem. Commun. 2015, 51, 10850–10853. [Google Scholar] [CrossRef]
- Liu, N.; Yue, Q.; Wang, Y.Q.; Cheng, A.L.; Gao, E.Q. Coordination compounds of bis(5-tetrazolyl)amine with manganese(II), zinc(II) and cadmium(II): Synthesis, structure and magnetic properties. Dalton Trans. 2008, 34, 4621–4629. [Google Scholar] [CrossRef]
- Kang, X.M.; Tang, M.H.; Yang, G.L.; Zhao, B. Cluster/cage-based coordination polymers with tetrazole derivatives. Coord. Chem. Rev. 2020, 422, 213424. [Google Scholar] [CrossRef]
- Wurzenberger, M.H.H.; Lommel, M.; Gruhne, M.S.; Szimhardt, N.; Stierstorfer, J. Refinement of copper (II) azide with 1-Alkyl-5H-tetrazoles: Adaptable energetic complexes. Angew. Chem. Int. Ed. 2020, 59, 12367–12370. [Google Scholar] [CrossRef]
- Asgari, M.; Semino, R.; Schouwink, P.A.; Kochetygov, I.; Tarver, J.; Trukhina, O.; Krishna, R.; Brown, C.M.; Ceriotti, M.; Queen, W.L. Understanding how ligand functionalization influences CO2 and N2 adsorption in a sodalite metal–organic framework. Chem. Mater. 2020, 32, 1526–1536. [Google Scholar] [CrossRef]
- Demko, Z.P.; Sharpless, K.B. An Intramolecular [2+3] Cycloaddition Route to Fused 5-Heterosubstituted Tetrazoles. Org. Lett. 2001, 3, 4091–4094. [Google Scholar] [CrossRef] [PubMed]
- Xiong, R.G.; Xue, X.; Zhao, H.; You, X.Z.; Abrahams, B.F.; Xue, Z.L. Novel, Acentric Metal–Organic Coordination Polymers from Hydrothermal Reactions Involving In Situ Ligand Synthesis. Angew. Chem. Int. Ed. 2002, 41, 3800–3803. [Google Scholar] [CrossRef]
- Singhal, S.; Garg, A.N.; Chandra, K. Spin crossover studies in mixed ligand complexes of tris(N-ethyl, N′-n-butyldithiocarbamato)iron(III) with NN, NO and OO containing bidentate ligands and their thermal decomposition. J. Alloys Compd. 2007, 443, 53–60. [Google Scholar] [CrossRef]
- Zheng, H.; Meng, Y.S.; Zhou, G.L.; Duan, C.Y.; Sato, O.; Hayami, S.; Luo, Y.; Liu, T. Simultaneous Modulation of Magnetic and Dielectric Transition via Spin-Crossover-Tuned Spin Arrangement and Charge Distribution. Angew. Chem. Int. Ed. 2018, 57, 8468–8472. [Google Scholar] [CrossRef] [PubMed]
- Liu, Q.; Hu, J.X.; Meng, Y.S.; Jiang, W.J.; Wang, J.L.; Wen, W.; Wu, Q.; Zhu, H.L.; Zhao, L.; Liu, T. Asymmetric Coordination Toward a Photoinduced Single-Chain Magnet Showing High Coercivity Values. Angew. Chem. Int. Ed. 2021, 60, 10537–10541. [Google Scholar] [CrossRef] [PubMed]
- Yang, F.; Li, B.Y.; Xu, W.; Li, G.H.; Zhou, Q.; Hua, J.; Shi, Z.; Feng, S.H. Two Metal–Organic Frameworks Constructed from One-Dimensional Cobalt (II) Ferrimagnetic Chains with Alternating Antiferromagnetic/Ferromagnetic and AF/AF/FM Interaction: Synthesis, Structures, and Magnetic Properties. Inorg. Chem. 2012, 51, 6813–6820. [Google Scholar] [CrossRef]
- Nakamoto, K. Infrared and Raman Spectra of Inorganic and Coordination Compounds; Wiley: New York, NY, USA, 2008; ISBN 9780470405888. [Google Scholar]
- Cabrosi, D.; Cruz, C.; Paredes-Garcia, V.; Albores, P. A dinuclear Co(III)/Co(II) complex based on the H2pmide ligand showing field-induced SMM behavior. Dalton Trans. 2022, 52, 175–184. [Google Scholar] [CrossRef]
- Li, X.B.; Zhuang, G.M.; Wang, X.; Wang, K.; Gao, E.Q. Field-modified multiple slow relaxations in a metamagnet composed of cobalt(ii) chains with mixed azide and tetrazolate bridges. Chem. Commun. 2013, 49, 1814–1816. [Google Scholar] [CrossRef]
- Bauer, E.M.; Bellitto, C.; Imperatori, P.; Righini, G.; Colapietro, M.; Portalone, G.; Gomez-Garcıa, C.J. A Novel 1D-AF Hybrid Organic-Inorganic Chromium(II) Methyl Phosphonate Dihydrate: Synthesis, X-Ray Crystal and Molecular Structure, and Magnetic Properties. Inorg. Chem. 2010, 49, 7472–7477. [Google Scholar] [CrossRef]
- Dong, X.Y.; Shi, Z.; Li, D.Q.C.; Li, Y.Y.; An, N.; Shang, Y.J.; Sakiyama, H.; Muddassir, M.; Si, C.D. The regulation research of topology and magnetic exchange models of CPs through Co (II) concentration adjustment. J. Solid State Chem. 2023, 318, 123713. [Google Scholar] [CrossRef]
- Sorai, M.; Nakazawa, Y.; Nakano, M.; Miyazaki, Y. Update 1 of: Calorimetric investigation of phase transitions occurring in molecule-based magnets. Chem. Rev. 2013, 113, PR41–PR122. [Google Scholar] [CrossRef]
- Novikov, V.V.; Matovnikov, A.V.; Mitroshenkov, N.V.; Shevelkov, A.V. Negative thermal expansion and low-temperature heat capacity anomalies of Ge31P15Se8 semiclathrate. J. Alloys Compd. 2016, 684, 564–568. [Google Scholar] [CrossRef]
- Evangelisti, M.; Luis, F.; Jonghc, L.J.; Affronte, M. Magnetotherma properties of molecule-based materials. J. Mater. Chem. 2006, 16, 2534–2549. [Google Scholar] [CrossRef]
- Schliesser, J.M.; Woodfield, B.F. Lattice vacancies responsible for the linear dependence of the low-temperature heat capacity of insulating materials. Phys. Rev. B 2015, 91, 024109. [Google Scholar] [CrossRef]
- Li, R.C.; Luo, J.P.; Yan, H.M.; Zheng, H.; Wei, R.M.; Yang, M.; Gu, X.L.; Tan, Z.C.; Shi, Q. Low temperature heat capacity study of Co3(BTC)2·12H2O and Ni3(BTC)2·12H2O. Thermochim. Acta 2021, 699, 178909. [Google Scholar] [CrossRef]
- Dickson, M.S.; Rosen, P.F.; Neilsen, G.; Navrotsky, A.; Woodfield, B.F. Heat capacity and thermodynamic functions of transition metal ion (Cu2+, Fe2+, Mn2+) exchanged, partially dehydrated zeolite A (LTA). J. Chem. Therm. 2021, 161, 106556. [Google Scholar] [CrossRef]
- Sheldrick, G.M. SHELXS-97, Program for X-ray Crystal Structure Determination; University of Göttingen: Göttingen, Germany, 1997. [Google Scholar]
- Sheldrick, G.M. SHELXL-97, Program for X-ray Crystal Structure Refinement; University of Göttingen: Göttingen, Germany, 1997. [Google Scholar]
- Bain, G.A.; Berry, J.F. Diamagnetic corrections and Pascal’s constants. J. Chem. Educ. 2008, 85, 532–536. [Google Scholar] [CrossRef]
- Dai, R.; Zhang, S.; Yin, N.; Tan, Z.; Shi, Q. Low-temperature heat capacity and standard thermodynamic functions of β-d-(-)-arabinose (C5H10O5). J. Chem. Thermodyn. 2016, 92, 60–65. [Google Scholar] [CrossRef]
- Shi, Q.; Snow, C.L.; Boerio-Goates, J.; Woodfield, B.F. Accurate heat capacity measurements on powdered samples using a Quantum Design physical property measurement system. J. Chem. Thermodyn. 2010, 42, 1107–1115. [Google Scholar] [CrossRef]
- Shi, Q.; Boerio-Goates, J.; Woodfield, B.F. An improved technique for accurate heat capacity measurements on powdered samples using a commercial relaxation calorimeter. J. Chem. Thermodyn. 2011, 43, 1263–1269. [Google Scholar] [CrossRef]
- Luo, J.P.; Zheng, H.; Wang, X.Q.; Yin, N.; Meng, Y.S.; Tan, Z.C.; Shi, Q. Low temperature heat capacity, thermodynamic and magnetic property of several new dinuclear complexes. J. Chem. Thermodyn. 2022, 170, 106785–106793. [Google Scholar] [CrossRef]

| Compound | 1 | 2 | 3 |
|---|---|---|---|
| Empirical formula | C12H12N10O2Fe | C12H12N10O2Co | C12H12N10O2Cu |
| CCDC | 2,239,647 | 2,239,654 | 2,239,654 |
| Formula weight | 384.17 | 387.25 | 391.86 |
| Crystal system | Monoclinic | Monoclinic | Monoclinic |
| Space group | P21/c | P21/c | P21/c |
| Temperature/K | 296.15 ± 2 | 297.0 ± 2 | 296.15 ± 2 |
| a (Å) | 8.1032 ± 0.0009 | 8.0925 ± 0.0018 | 8.0925 ± 0.0018 |
| b (Å) | 12.9127 ± 0.0014 | 12.870 ± 0.003 | 12.870 ± 0.003 |
| c (Å) | 7.3470 ± 0.0008 | 7.3128 ± 0.0017 | 7.3128 ± 0.0017 |
| α (°) | 90.00 | 90.00 | 90.00 |
| β (°) | 96.092 ± 0.002 | 96.070 ± 0.004 | 96.070 ± 0.004 |
| γ (°) | 90.00 | 90.00 | 90.00 |
| V/Å3 | 764.41 ± 0.14 | 757.3 ± 0.3 | 757.3 ± 0.3 |
| Z | 2 | 2 | 2 |
| Density calculated/g cm−3 | 1.669 | 1.698 | 1.718 |
| µ/mm−1 | 1.019 | 1.166 | 1.475 |
| F(000) | 392.0 | 394.0 | 398 |
| Independent reflections | 4560 | 7905 | 10,938 |
| Data/restraint/parameters | 1765/0/120 | 1891/0/139 | 1949/0/139 |
| Goodness of fit on F2 | 0.819 | 1.059 | 1.196 |
| R1, ωR2 [I > 2σ(I)] a | 0.0372, 0.1125 | 0.0324, 0.0854 | 0.0424, 0.1226 |
| R1, ωR2 (all data) | 0.0582, 0.1285 | 0.0408, 0.0909 | 0.0463, 0.1282 |
| Compound 1 (FeII) | Compound 2 (CoII) | Compound 3 (CuII) | |
|---|---|---|---|
| M-N(1) | 2.201(2) | 2.146(16) | 2.041(13) |
| M-N(2) | 2.154(2) | 2.114(15) | 2.014(14) |
| M-O(1) | 2.146(2) | 2.126(16) | 2.420(18) |
| O(1)#1-Fe(1)-N(2) | 89.51(8) | 89.51(6) | 89.38(5) |
| O(1)#1-Fe(1)-N(1)#1 | 89.95(8) | 78.05(6) | 92.55(5) |
| N(2)-Fe(1)-N(1)#1 | 103.22(8) | 101.95(6) | 99.22(6) |
| O(1)#1-Fe(1)-N(1) | 90.05(8) | 89.73(6) | 87.45(5) |
| D–H···A | d(D–H) | d(H···A) | d(D···A) | <(DHA) |
|---|---|---|---|---|
| Compound 1 | ||||
| C(4)-H(4)···O(1)#1 | 0.93 | 3.06 | 3.830(2) | 142.2 |
| O(1)-H(1W)···N(4)#2 | 0.82(3) | 1.942 | 2.831(3) | 173(2) |
| O(1)-H(2W)···N(5)#3 | 0.69(3) | 2.139 | 2.757(3) | 171(2) |
| Compound 2 | ||||
| C(5)-H(5)···O(1)#1 | 0.93 | 2.56 | 3.343(3) | 142.2 |
| O(1)-H(1W)···N(4)#2 | 0.79(3) | 2.05(3) | 2.840(2) | 174(3) |
| O(1)-H(2W)···N(5)#3 | 0.79(3) | 1.99(3) | 2.781(2) | 176(3) |
| Compound 3 | ||||
| C(3)-H(3)···O(1)#1 | 0.92 | 2.68 | 3.883(3) | 145.5 |
| O(1)-H(1W)···N(4)#2 | 0.75(2) | 2.06(2) | 2.906(2) | 179(2) |
| O(1)-H(2W)···N(5)#3 | 0.78(2) | 2.16(2) | 2.835(2) | 171(2) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, H.; Luo, J.; Wang, X.; Yin, N.; Zhang, B.; Gao, X.; Zhang, Z.; Shi, Q.; Liu, J. Magnetic Property, Heat Capacity and Crystal Structure of Mononuclear Compounds Based on Substitute Tetrazole Ligand. Molecules 2023, 28, 6633. https://doi.org/10.3390/molecules28186633
Zheng H, Luo J, Wang X, Yin N, Zhang B, Gao X, Zhang Z, Shi Q, Liu J. Magnetic Property, Heat Capacity and Crystal Structure of Mononuclear Compounds Based on Substitute Tetrazole Ligand. Molecules. 2023; 28(18):6633. https://doi.org/10.3390/molecules28186633
Chicago/Turabian StyleZheng, Hui, Jipeng Luo, Xiaoqin Wang, Nan Yin, Beibei Zhang, Xuezhen Gao, Zongzheng Zhang, Quan Shi, and Junshen Liu. 2023. "Magnetic Property, Heat Capacity and Crystal Structure of Mononuclear Compounds Based on Substitute Tetrazole Ligand" Molecules 28, no. 18: 6633. https://doi.org/10.3390/molecules28186633
APA StyleZheng, H., Luo, J., Wang, X., Yin, N., Zhang, B., Gao, X., Zhang, Z., Shi, Q., & Liu, J. (2023). Magnetic Property, Heat Capacity and Crystal Structure of Mononuclear Compounds Based on Substitute Tetrazole Ligand. Molecules, 28(18), 6633. https://doi.org/10.3390/molecules28186633





