Thermodynamic and Thermal Analyze of N,N-Dimethylformamide + 1-Butanol Mixture Properties Based on Density, Sound Velocity and Heat Capacity Data
Abstract
1. Introduction
2. Results and Discussion
2.1. Volumetric Properties
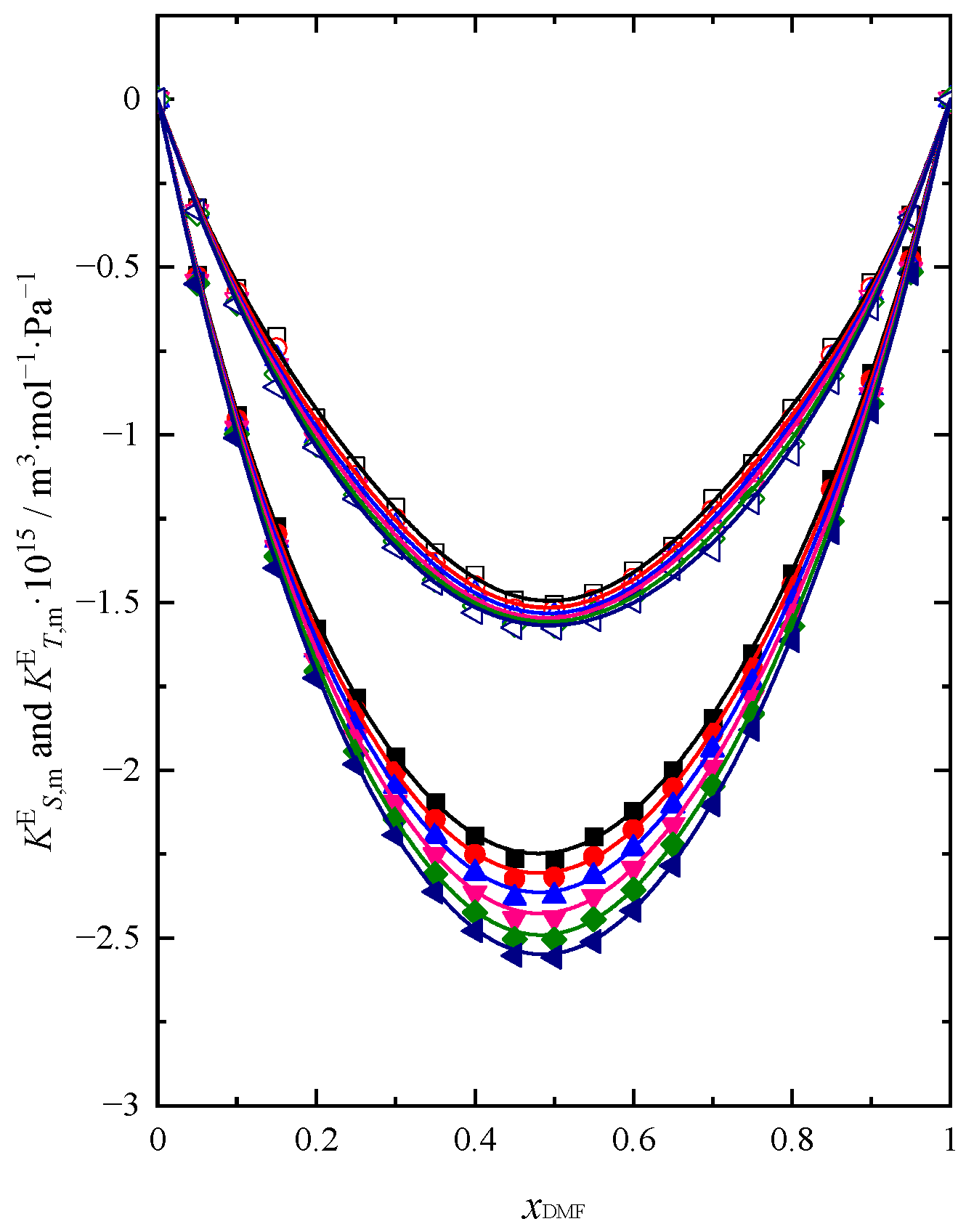
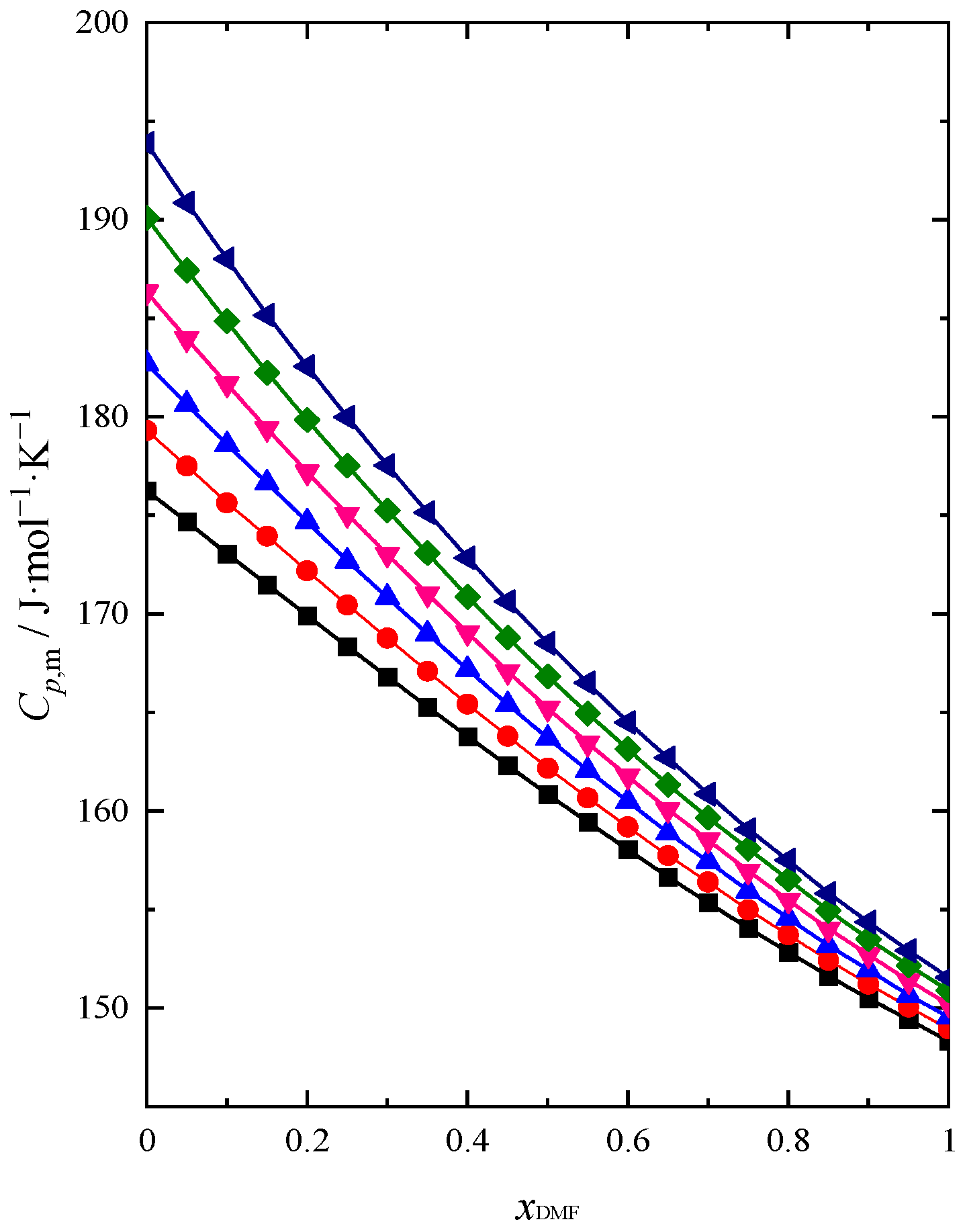
2.2. Sound Velocity and Heat Capacity
3. Experimental
3.1. Materials
3.2. Method
3.2.1. Density and Speed of Sound
3.2.2. Heat Capacity
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Potvin, J.; Péringer, P. Influence of n-propanol on growth and antibiotic production by an industrial strain of streptomyces erythreus under different nutritional conditions. Biotechnol. Lett. 1993, 15, 455–460. [Google Scholar] [CrossRef]
- Rotter, M.L.; Koller, W.; Neumann, R. The influence of cosmetic additives on the acceptability of alcohol-based hand disinfectants. J. Hosp. Infect. 1991, 18, 57–63. [Google Scholar] [CrossRef] [PubMed]
- Das, R.K.; Roy, M.N. Study on solution—Solution interactions prevailing in some liquid mixtures by volumetric, viscometric and acoustic measurements. Phys. Chem. Liq. 2014, 52, 55–77. [Google Scholar] [CrossRef]
- Thirumaran, S.; Alli, T.; Priya, D.; Selvi, A. Ultrasonic studies of molecular interactions in organic binary liquid mixtures. J. Chem. 2010, 7, 217–222. [Google Scholar] [CrossRef]
- Gautan, R.K.; Saharan, A.; Wilson, K.; Kaur, K.P. Properties and uses of butanol. In Properties and Uses of Butanol; Artois, A.M., Ed.; Nova Science Publishers: New York, NY, USA, 2020; pp. 1–26. [Google Scholar]
- Žagar, E.; Žigon, M. Solution properties of carboxylated polyurethanes and related ionomers in polar solvents (DMF and LiBr/DMF). Polymer 2000, 41, 3513–3521. [Google Scholar] [CrossRef]
- Kang, Y.K.; Park, H.S. Internal rotation about the C–N bond of amides. J. Mol. Struct. THEOCHEM 2004, 676, 171–176. [Google Scholar] [CrossRef]
- Howard, P.H. Handbook of Environmental Fate and Exposure Data for Organic Chemicals, Solvents 2; Lewis Publishers Inc.: Chelsea, MI, USA, 1993; Volume 4. [Google Scholar]
- Gescher, A. Metabolism of N,N-dimethylformamide: Key to the understanding of its toxicity. Chem. Res. Toxicol. 1993, 6, 245–251. [Google Scholar] [CrossRef]
- Jankowska, A.; Czerczak, S. N,N-dimetyloformamid. Dokumentacja dopuszczalnych wielkości narażenia zawodowego. Podstawy I Metod. Oceny Sr. Pr. 2010, 4, 55–92. [Google Scholar]
- Jödecke, M.; Pérez-Salado Kamps, Á.; Maurer, G. An experimental investigation of the solubility of CO2 in (N,N-dimethylmethanamide + water). J. Chem. Eng. Data 2012, 57, 1249–1266. [Google Scholar] [CrossRef]
- Byun, H.-S.; Kim, N.-H.; Kwak, C. Measurements and modeling of high-pressure phase behavior of binary CO2–amides systems. Fluid Phase Equilib. 2003, 208, 53–68. [Google Scholar] [CrossRef]
- Chang, C.J.; Chen, C.-Y.; Lin, H.-C. Solubilities of carbon dioxide and nitrous oxide in cyclohexanone, toluene, and N,N-dimethylformamide at elevated pressures. J. Chem. Eng. Data 1995, 40, 850–855. [Google Scholar] [CrossRef]
- Rao, K.P.; Reddy, K.S. Excess volumes and excess isentropic compressibility of binary mixtures of N,N-dimethylformamide with n-alcohols at 303.15 K. Phys. Chem. Liq. 1985, 15, 147–154. [Google Scholar] [CrossRef]
- Iloukhani, H.; Rostami, Z. Measurement of some thermodynamic and acoustic properties of binary solutions of N,N-dimethylformamide with 1-alkanols at 30°C and comparison with theories. J. Solut. Chem. 2003, 32, 451–462. [Google Scholar] [CrossRef]
- Dakua, V.K.; Sinha, B.; Roy, M.N. Thermophysical properties of binary mixtures of N,N-dimethylformamide with isomeric butanols at 298.15, 308.15 and 318.15 K. J. Indian Chem. Soc. 2007, 84, 37–45. [Google Scholar] [CrossRef]
- El-Dossoki, F.I. Refractive index and density measurements for selected binary protic-protic, aprotic-aprotic, and aprotic-protic systems at temperatures from 298.15 K to 308.15 K. JCCS 2007, 54, 1129–1137. [Google Scholar] [CrossRef]
- Sagar, S.; Kumari, L.; Gupta, M. Thermoacoustical analysis of binary mixtures of N,N-dimethylformamide (DMF) with BAE and 1-BuOH. J. Pure Appl. Ultrason. 2017, 39, 71–78. [Google Scholar]
- Meza, M.; Lans Ceballos, E.; Cantero-López, P. Densities and volumetric properties of the mixture N,N-dimethylformamide (DMF) + 1-butanol at several temperatures. DYNA 2013, 80, 132–141. [Google Scholar]
- Uddin, H.; Khan, M.Z.H.; Rahman, H.; Shahriar, A.M.; Al Mashud, A. Volumetric and viscometric properties observed for the mixtures of dmf (N,N-dimethylformamide) and other alcohols (butanol and 1-propanol). Phys. Chem. Liq. 2014, 52, 251–261. [Google Scholar] [CrossRef]
- García, B.; Alcalde, R.; Leal, J.M.; Trenzado, J.L. Volumetric behaviour of N-methylformamide–(C1–C10)alkan-1-ol and N,N-dimethylformamide–(C1–C10)alkan-1-ol solvent systems. JPOC 1997, 10, 138–144. [Google Scholar] [CrossRef]
- Zegers, H.C.; Somsen, G. Partial molar volumes and heat capacities in (dimethylformamide + an n-alkanol). J. Chem. Thermodyn. 1984, 16, 225–235. [Google Scholar] [CrossRef]
- Khursan, S.L. Heat capacity estimation using a complete set of homodesmotic reactions for organic compounds. Molecules 2022, 27, 7814. [Google Scholar] [CrossRef]
- Kumari, S.; Chauhan, S.; Singh, K.; Umar, A.; Fouad, H.; Alissawi, M.S.; Akhtar, M.S. Volumetric, Compressibility and viscometric approach to study the interactional behaviour of sodium cholate and sodium deoxycholate in aqueous glycyl glycine. Molecules 2022, 27, 8998. [Google Scholar] [CrossRef] [PubMed]
- Douhéret, G.; Davis, M.I.; Reis, J.C.R.; Blandamer, M.J. Isentropic compressibilities—Experimental origin and the quest for their rigorous estimation in thermodynamically ideal liquid mixtures. ChemPhysChem 2001, 2, 148–161. [Google Scholar] [CrossRef] [PubMed]
- Tyczyńska, M.; Jóźwiak, M.; Komudzińska, M.; Majak, T. Effect of temperature and composition on the volumetric, acoustic and thermal properties of N,N-dimethylformamide + propan-1-ol Mixture. J. Mol. Liq. 2019, 290, 111124. [Google Scholar] [CrossRef]
- Kato, M.; Suzuki, N. Excess volumes of binary mixtures containing acetylacetone. J. Chem. Thermodyn. 1978, 10, 435–440. [Google Scholar] [CrossRef]
- Smith, F.B.I. Thermodynamic properties of alcohol + alkane mixtures. I. Theoretical results for the hydrogen bond contribution to the excess energies. Aust. J. Chem. 1973, 26, 691–704. [Google Scholar] [CrossRef]
- Franks, F.; Ives, D.J.G. The structural properties of alcohol–water mixtures. Q. Rev. Chem. Soc. 1966, 20, 1–44. [Google Scholar] [CrossRef]
- Prajapati, A.N. Dielectric study of binary mixtures of 1-butanol with N,N-dimethylformamide. AIP Conf. Proc. 2020, 2220, 040030. [Google Scholar] [CrossRef]
- Chao, J.P.; Wang, Y.X.; Dai, M. Studies of thermodynamic properties of binary mixtures containing an alcohol XVII. excess molar enthalpies of each of (one of the four butanols + N,N-dimethylacetamide or N,N-dimethylformamide or dimethyl sulfoxide) at the temperature 298.15 K. J. Chem. Thermodyn. 1991, 23, 163–168. [Google Scholar] [CrossRef]
- Sivagurunathan, P.; Ramachandran, K.; Dharmalingam, K. Hydrogen bonding between alcohols and N,N-dimethylformamide: An FTIR study. Main Group Chem. 2006, 5, 89–94. [Google Scholar] [CrossRef]
- Komudzińska, M.; Tyczyńska, M.; Jóźwiak, M.; Burakowski, A.; Gliński, J. Volumetric, acoustic and thermal properties of aqueous N,N-dimethylformamide system. effect of temperature and composition. J. Mol. Liq. 2020, 300, 112321. [Google Scholar] [CrossRef]
- Klimaszewski, K.; Stronka-Lewkowska, E.; Soliwoda, K.; Bald, A. Acoustic and volumetric studies on water+diethylene glycol mixtures in a wide temperature range. comparison with mixtures of water with tri- and tetraethylene glycol. J. Mol. Liq. 2016, 215, 520–533. [Google Scholar] [CrossRef]
- Acree, W.E. Excess Isentropic Compressibilities of binary mixtures of N,N-dimethylformamide with n-alcohols at 303.1 5 K. Phys. Chem. Liq. 1986, 16, 113–116. [Google Scholar] [CrossRef]
- Sridevi, U.; Samatha, K.; Sarma, A.V. Excess thermodynamic properties in binary liquids. J. Pure Appl. Ultrason. 2004, 26, 1–11. [Google Scholar]
- Yasmin, M.; Gupta, M.; Shukla, J.P. Acoustical study of molecular interactions in polymer solutions through various thermodynamical parameters and Flory’s theory at 298.15 K. Phys. Chem. Liq. 2010, 48, 682–697. [Google Scholar] [CrossRef]
- Fortin, T.J.; Laesecke, A.; Freund, M.; Outcalt, S. Advanced calibration, adjustment, and operation of a density and sound speed analyzer. J. Chem. Thermodyn. 2013, 57, 276–285. [Google Scholar] [CrossRef]
- Nain, A.K. Densities and volumetric properties of binary mixtures of formamide with 1-butanol, 2-butanol, 1,3-butanediol and 1,4-butanediol at temperatures between 293.15 and 318.15 K. J. Solution Chem. 2007, 36, 497–516. [Google Scholar] [CrossRef]
- Singh, S.; Parveen, S.; Shukla, D.; Gupta, M.; Shukla, J.P. Volumetric, optical, acoustical and viscometric study of molecular association in binary mixtures of butylamine with 1-butanol and tert-butanol. Acta Phys. Pol. A 2007, 111, 847–858. [Google Scholar] [CrossRef]
- Jóźwiak, M.; Tyczyńska, M. Volumetric properties of urea in the mixture of N,N-dimethylformamide with water. J. Chem. Eng. Data 2012, 57, 2067–2075. [Google Scholar] [CrossRef]
- Rodríguez, A.; Canosa, J.; Tojo, J. Density, refractive index, and speed of sound of binary mixtures (diethyl carbonate + alcohols) at several temperatures. J. Chem. Eng. Data 2001, 46, 1506–1515. [Google Scholar] [CrossRef]
- Tyczyńska, M.; Jóźwiak, M. Apparent and partial molar volumes of 18-crown-6 ether in the mixture of N,N-dimethylformamide with water. J. Mol. Liq. 2014, 195, 80–86. [Google Scholar] [CrossRef]
- Calvar, N.; González, E.J.; Domínguez, Á.; Macedo, E.A. Acoustic, volumetric and osmotic properties of binary mixtures containing the ionic liquid 1-butyl-3-methylimidazolium dicyanamide mixed with primary and secondary alcohols. J. Chem. Thermodyn. 2012, 50, 19–29. [Google Scholar] [CrossRef]
- Dzida, M. Thermophysical properties of 1-butanol at high pressures. Energies 2020, 13, 5046. [Google Scholar] [CrossRef]
- Tyczyńska, M.; Jóźwiak, M. Partial molar volumes of 15-crown-5 ether in mixtures of N,N,-dimethylformamide with water. J. Solut. Chem. 2014, 43, 388–403. [Google Scholar] [CrossRef] [PubMed]
- Olivieri, G.V.; da Cunha, C.S.; dos Santos Martins, L.; Paegle, P.A.M.; Nuncio, S.D.; de Araújo Morandim-Giannetti, A.; Torres, R.B. thermodynamic and spectroscopic study of binary mixtures of n-butylammonium oleate ionic liquid + alcohol at T = 288.15–308.15 K. J. Therm. Anal. Calorim. 2018, 131, 2925–2942. [Google Scholar] [CrossRef]
- Warmińska, D.; Krakowiak, J.; Grzybkowski, W. Thermodynamic properties of inorganic salts in nonaqueous solvents. iii. apparent molar volumes and compressibilities of divalent transition-metal chlorides in N,N-dimethylformamide. J. Chem. Eng. Data 2007, 52, 2099–2104. [Google Scholar] [CrossRef]
- Płaczek, A.; Koziel, H.; Grzybkowski, W. Apparent molar compressibilities and volumes of some 1,1-electrolytes in N,N-dimethylacetamide and N,N-dimethylformamide. J. Chem. Eng. Data 2007, 52, 699–706. [Google Scholar] [CrossRef]
- Safarov, J.; Ahmadov, B.; Mirzayev, S.; Shahverdiyev, A.; Hassel, E.P. Thermophysical properties of 1-butanol over a wide range of temperatures and pressures up to 200MPa. J. Mol. Liq. 2015, 209, 465–479. [Google Scholar] [CrossRef]
- Boruń, A.; Bald, A. Conductometric studies of 1-ethyl-3-methylimidazolium tetrafluoroborate and 1-butyl-3-methylimidazolium tetrafluoroborate in N,N-dimethylformamide at temperatures from (283.15 to 318.15) K. J. Chem. Eng. Data 2012, 57, 475–481. [Google Scholar] [CrossRef]
- Riddick, J.A.; Bunger, W.B.; Sakano, T.K. Organic Solvents: Physical Properties and Methods of Purification. In Techniques of Chemistry, 4th ed.; Wiley Interscience: New York, NY, USA, 1986. [Google Scholar]
- Warmińska, D.; Krakowiak, J.; Grzybkowski, W. Thermodynamic properties of inorganic salts in nonaqueous solvents. I. Apparent molar volumes and compressibilities of divalent transition-metal perchlorates in N,N-dimethylformamide. J. Chem. Eng. Data 2005, 50, 221–225. [Google Scholar] [CrossRef]
- Iloukhani, H.; Zarei, H.A. Excess molar enthalpies of N,N-dimethylformamide + alkan-1-ols (C1−C6) at 298.15 K. J. Chem. Eng. Data 2002, 47, 195–197. [Google Scholar] [CrossRef]
- Chabouni, Y.; Amireche, F. Some physicochemical properties of binary mixtures of glycerol with butanol isomers in the temperature range 293.15–318.15 K and ambient pressure. J. Chem. Eng. Data 2020, 65, 1679–1694. [Google Scholar] [CrossRef]
- Singh, S.; Aznar, M.; Deenadayalu, N. Densities, speeds of sound, and refractive indices for binary mixtures of 1-butyl-3-methylimidazolium methyl sulphate ionic liquid with alcohols at T = (298.15, 303.15, 308.15, and 313.15) K. J. Chem. Thermodyn. 2013, 57, 238–247. [Google Scholar] [CrossRef]
- Nain, A.K. Densities, ultrasonic speeds, viscosities and excess properties of binary mixtures of methyl methacrylate with N,N-dimethylformamide and N,N-dimethylacetamide at different temperatures. J. Chem. Thermodyn. 2013, 60, 105–116. [Google Scholar] [CrossRef]
- Papari, M.M.; Ghodrati, H.; Fadaei, F.; Sadeghi, R.; Behrouz, S.; Rad, M.N.S.; Moghadasi, J. Volumetric and ultrasonic study of mixtures of 2-phenylethanol with 1-butanol, 2-butanol, and 2-methyl-1-butanol at T = (298.15–323.15) K and atmospheric pressure: Measurement and prediction. J. Mol. Liq. 2013, 180, 121–128. [Google Scholar] [CrossRef]
- AlTuwaim, M.S.; Alkhaldi, K.H.A.E.; Al-Jimaz, A.S.; Mohammad, A.A. Comparative Study of physico-chemical properties of binary mixtures of N,N-dimethylformamide with 1-alkanols at different temperatures. J. Chem. Thermodyn. 2012, 48, 39–47. [Google Scholar] [CrossRef]
- Segade, L.; Jiménez de Llano, J.; Domínguez-Pérez, M.; Cabeza, Ó.; Cabanas, M.; Jiménez, E. Density, surface tension, and refractive index of octane + 1-alkanol mixtures at T = 298.15 K. J. Chem. Eng. Data 2003, 48, 1251–1255. [Google Scholar] [CrossRef]
- Roy, M.N.; Chanda, R.; Ghosh, G. Molar volumes, viscosity, and isentropic compressibility of some primary monoalkanols in aqueous N,N-dimethylformamide solutions. Russ. J. Phys. Chem. 2009, 83, 1331–1341. [Google Scholar] [CrossRef]
- Sharma, V.K.; Dua, R. Topological and thermodynamic investigations of mixtures containing o-chlorotoluene and lower amides. J. Chem. Thermodyn. 2014, 71, 182–195. [Google Scholar] [CrossRef]
- Varfolomeev, M.A.; Zaitseva, K.V.; Rakipov, I.T.; Solomonov, B.N.; Marczak, W. Speed of sound, density, and related thermodynamic excess properties of binary mixtures of butan-2-one with C1–C4 n-alkanols and chloroform. J. Chem. Eng. Data 2014, 59, 4118–4132. [Google Scholar] [CrossRef]
- Papamatthaiakis, D.; Aroni, F.; Havredaki, V. Isentropic compressibilities of (amide + water) mixtures: A comparative study. J. Chem. Thermodyn. 2008, 40, 107–118. [Google Scholar] [CrossRef]
- Ivanov, E.V.; Abrosimov, V.K.; Lebedeva, E.Y. Apparent molar volumes and expansibilities of H2O and D2O in N,N-dimethylformamide and N,N-dimethylacetamide in the range of T = (278.15 to 318.15)K at p = 0.1MPa: A Comparative Analysis. J. Chem. Thermodyn. 2012, 53, 131–139. [Google Scholar] [CrossRef]
- Neeti; Jangra, S.K.; Yadav, J.S.; Dimple; Sharma, V.K. Thermodynamic investigations of ternary o-toluidine+tetrahydropyran+N,N-dimethylformamide mixture and its binaries at 298.15, 303.15 and 308.15 K. J. Mol. Liq. 2011, 163, 36–45. [Google Scholar] [CrossRef]
- Alavianmehr, M.M.; Hemmati, N.; Ghodrati, H. Excess molar volumes, excess thermal expansion coefficients and isentropic compressibility deviations for binary mixtures of benzyl alcohol + (1-butanol, 2-butanol, 2-methyl-1-butanol and tert-butanol) at T = (298.15-328.15) K and ambient pressure. Phys. Chem. Liq. 2017, 55, 85–99. [Google Scholar] [CrossRef]
- Abidi, R.; Artal, M.; Hichri, M.; Lafuente, C. Experimental and modelled thermophysical behaviour of methyl levulinate (methyl 4-oxopentanoate) and n-alkanol systems. J. Mol. Liq. 2021, 339, 116739. [Google Scholar] [CrossRef]
- Iglesias, M.; Orge, B.; Canosa, J.M.; Rodríguez, A.; Domínguez, M.; Piñeiro, M.M.; Tojo, J. Thermodynamic behaviour of mixtures containing methyl acetate, methanol, and 1-butanol at 298.15 K: Application of the eras model. Fluid Phase Equilib. 1998, 147, 285–300. [Google Scholar] [CrossRef]
- Zhu, L.; Li, H.; Wang, C.; Han, S. Isothermal and isobaric (vapour + liquid) equilibria of (N,N-dimethylformamide + 2-propanol+ 1-butanol). J. Chem. Thermodyn. 2001, 33, 1535–1543. [Google Scholar] [CrossRef]
- Chandra Sekhar, M.; Madhu Mohan, T.; Vijaya Krishna, T. Excess thermodynamic and FT-IR spectroscopic studies on binary liquid mixtures of 2-chloroaniline with isomeric butanols at T = (303.15 to 318.15) K. J. Mol. Liq. 2014, 200, 263–272. [Google Scholar] [CrossRef]
- Palani, R.; Geetha, A. Acoustical and excess thermodynamic studies of molecular interaction in aqueous mixed solvent systems at 303, 308 and 313 K. Phys. Chem. Liq. 2009, 47, 542–552. [Google Scholar] [CrossRef]
- Oswal, S.L.; Desai, H.S. Studies of viscosity and excess molar volume of binary mixtures: 4. 1-alkanol + tri-n-butylamine mixtures at 303.15 and 313.15 K. Fluid Phase Equilib. 2003, 204, 281–294. [Google Scholar] [CrossRef]
- Mehta, S.K.; Ram, G.; Mani, C.; Bhasin, K.K. A Comparative study of thermophysical and spectroscopic properties in mixtures of isomeric butanediol and N,N-dimethylformamide. J. Chem. Thermodyn. 2006, 38, 836–848. [Google Scholar] [CrossRef]
- Nikam, P.S.; Shirsat, L.N.; Hasan, M. Density and viscosity studies of binary mixtures of acetonitrile with methanol, ethanol, propan-1-ol, propan-2-ol, butan-1-ol, 2-methylpropan-1-ol, and 2-methylpropan-2-ol at (298.15, 303.15, 308.15, and 313.15) K. J. Chem. Eng. Data 1998, 43, 732–737. [Google Scholar] [CrossRef]
- Zorębski, E.; Deć, E. Speeds of sound and isentropic compressibilities for binary mixtures of 1,2-ethanediol with 1-butanol, 1-hexanol, or 1-octanol in the temperature range from 293.15 to 313.15K. J. Mol. Liq. 2012, 168, 61–68. [Google Scholar] [CrossRef]
- Outcalt, S.L.; Laesecke, A.; Fortin, T.J. Density and speed of sound measurements of 1- and 2-butanol. J. Mol. Liq. 2010, 151, 50–59. [Google Scholar] [CrossRef]
- Bebek, K.; Strugała-Wilczek, A. Acoustic and thermophysical properties of binary liquid mixtures of primary butanols with hexane and cyclohexane at 293.15 K. Int. J. Thermophys. 2010, 31, 8–15. [Google Scholar] [CrossRef]
- Bernal-García, J.M.; Guzmán-López, A.; Cabrales-Torres, A.; Estrada-Baltazar, A.; Iglesias-Silva, G.A. Densities and viscosities of (N,N-dimethylformamide + water) at atmospheric pressure from (283.15 to 353.15) K. J. Chem. Eng. Data 2008, 53, 1024–1027. [Google Scholar] [CrossRef]
- Gadžurić, S.; Tot, A.; Zec, N.; Papović, S.; Vraneš, M. Volumetric properties of binary mixtures of 1-butyl-1-methylpyrrolidinium tris(pentafluoroethyl)trifluorophosphate with N-methylformamide, N-ethylformamide, N,N-dimethylformamide, N,N-dibutylformamide, and N,N-dimethylacetamide from (293.15 to 323.15) K. J. Chem. Eng. Data 2014, 59, 1225–1231. [Google Scholar] [CrossRef]
- Scharlin, P.; Steinby, K.; Domańska, U. Volumetric properties of binary mixtures of N,N-dimethylformamide with water or water- d2 at temperatures from 277.13 K to 318.15 K. J. Chem. Thermodyn. 2002, 34, 927–957. [Google Scholar] [CrossRef]
- Góralski, P.; Tkaczyk, M.; Chora̧żewski, M. Heat capacities of α,ω-dichloroalkanes at temperatures from 284.15 K to 353.15 K and a group additivity analysis. J. Chem. Eng. Data 2003, 48, 492–496. [Google Scholar] [CrossRef]
- Zábranský, M.; Růžička, V., Jr.; Domalski, E.S. Heat capacity of liquids: Critical review and recommended values. supplement I. J. Phys. Chem. Ref. Data 2001, 30, 1199–1689. [Google Scholar] [CrossRef]
- Zorębski, E.; Góralski, P. Molar heat capacities for (1-butanol+1,4-butanediol, 2,3-butanediol, 1,2-butanediol, and 2-methyl-2,4-pentanediol) as function of temperature. J. Chem. Thermodyn. 2007, 39, 1601–1607. [Google Scholar] [CrossRef]
- Smirnova, N.N.; Tsvetkova, L.Y.; Bykova, T.A.; Marcus, Y. Thermodynamic properties of N,N-dimethylformamide and N,N-dimethylacetamide. J. Chem. Thermodyn. 2007, 39, 1508–1513. [Google Scholar] [CrossRef]
- Checoni, R.F.; Volpe, P.L.O. Measurements of the molar heat capacities and excess molar heat capacities for water + organic solvents mixtures at 288.15 K to 303.15 K and atmospheric pressure. J. Solution Chem. 2010, 39, 259–276. [Google Scholar] [CrossRef]
- Shokouhi, M.; Jalili, A.H.; Hosseini-Jenab, M.; Vahidi, M. Thermo-physical properties of aqueous solutions of N,N-dimethylformamide. J. Mol. Liq. 2013, 186, 142–146. [Google Scholar] [CrossRef]
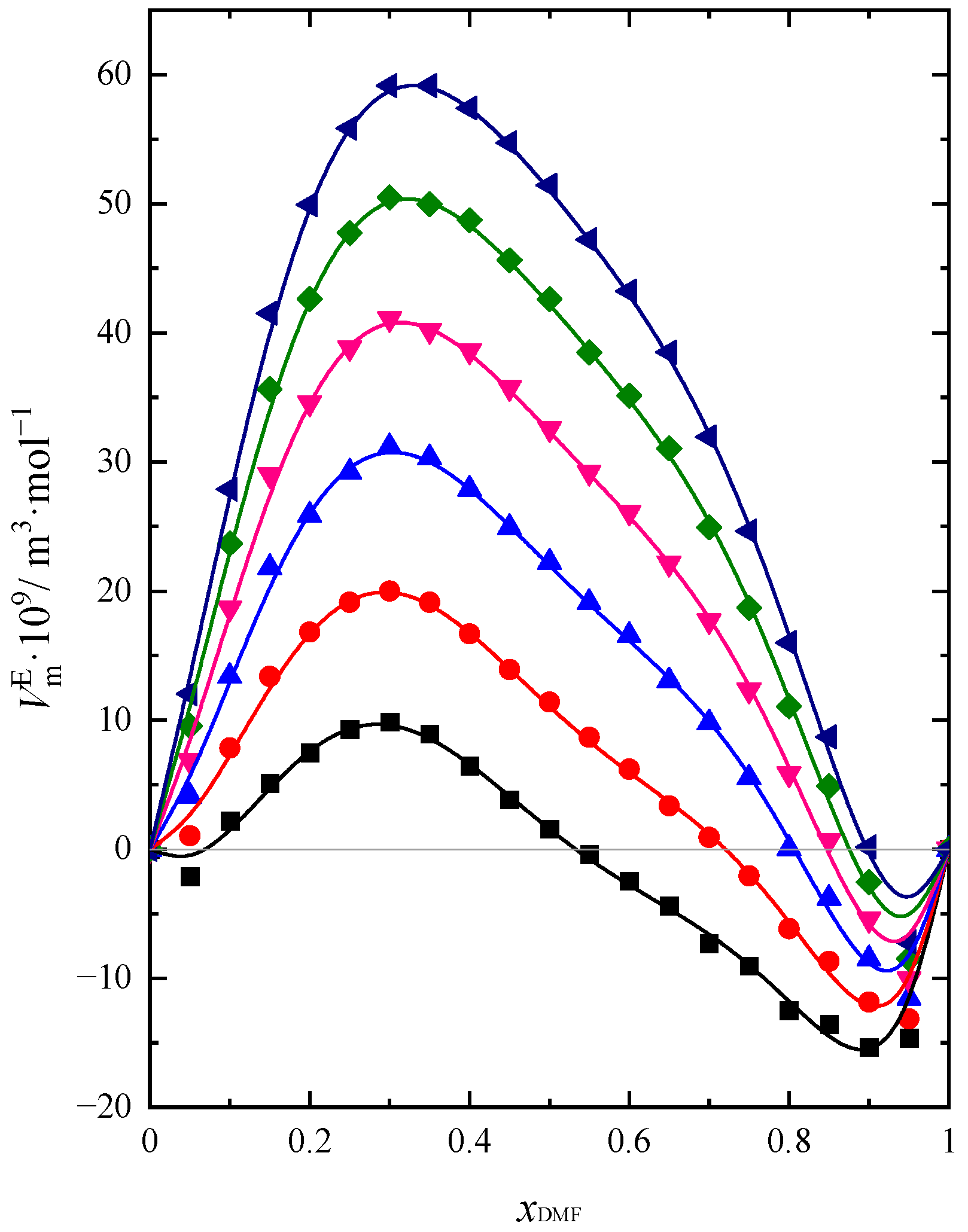
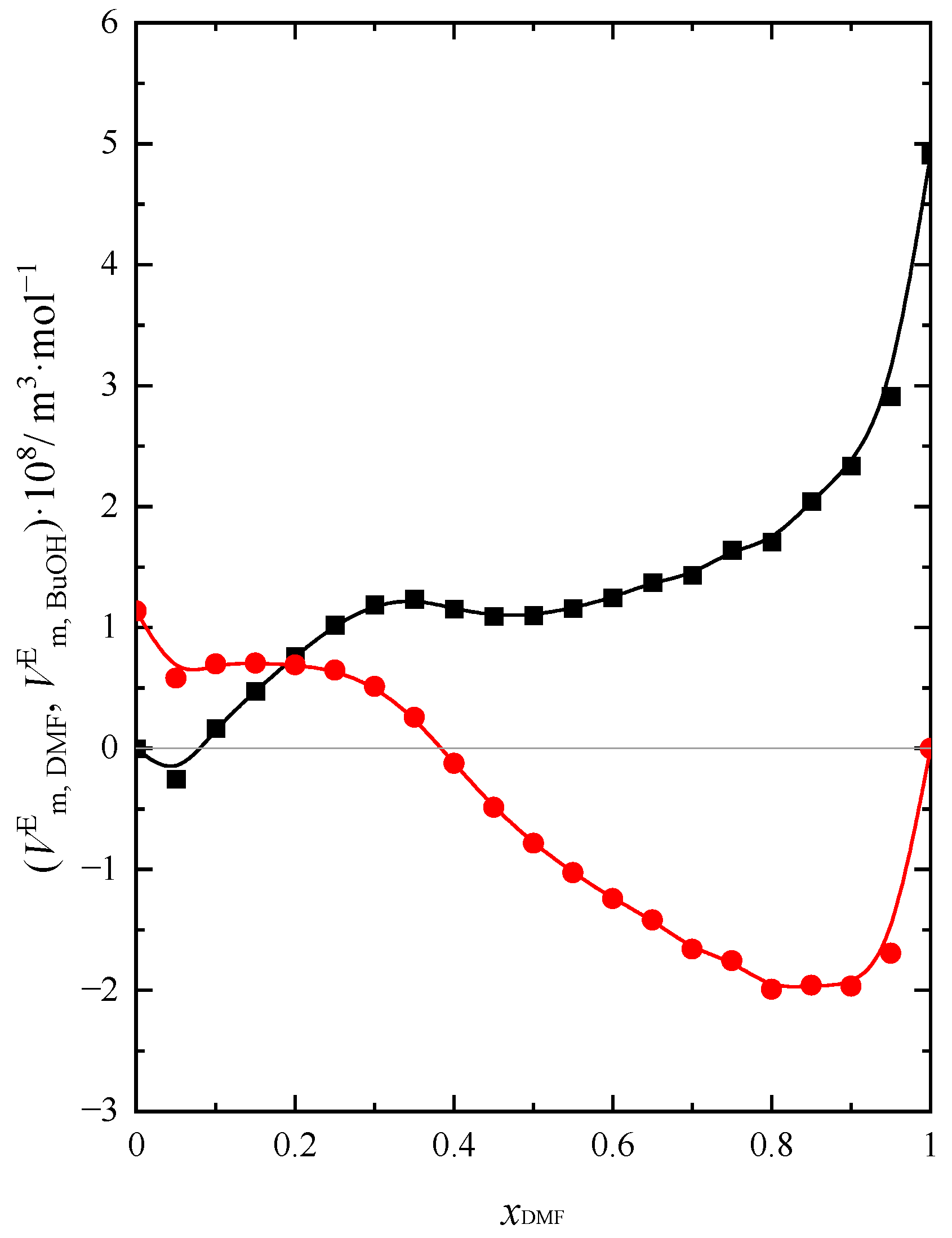
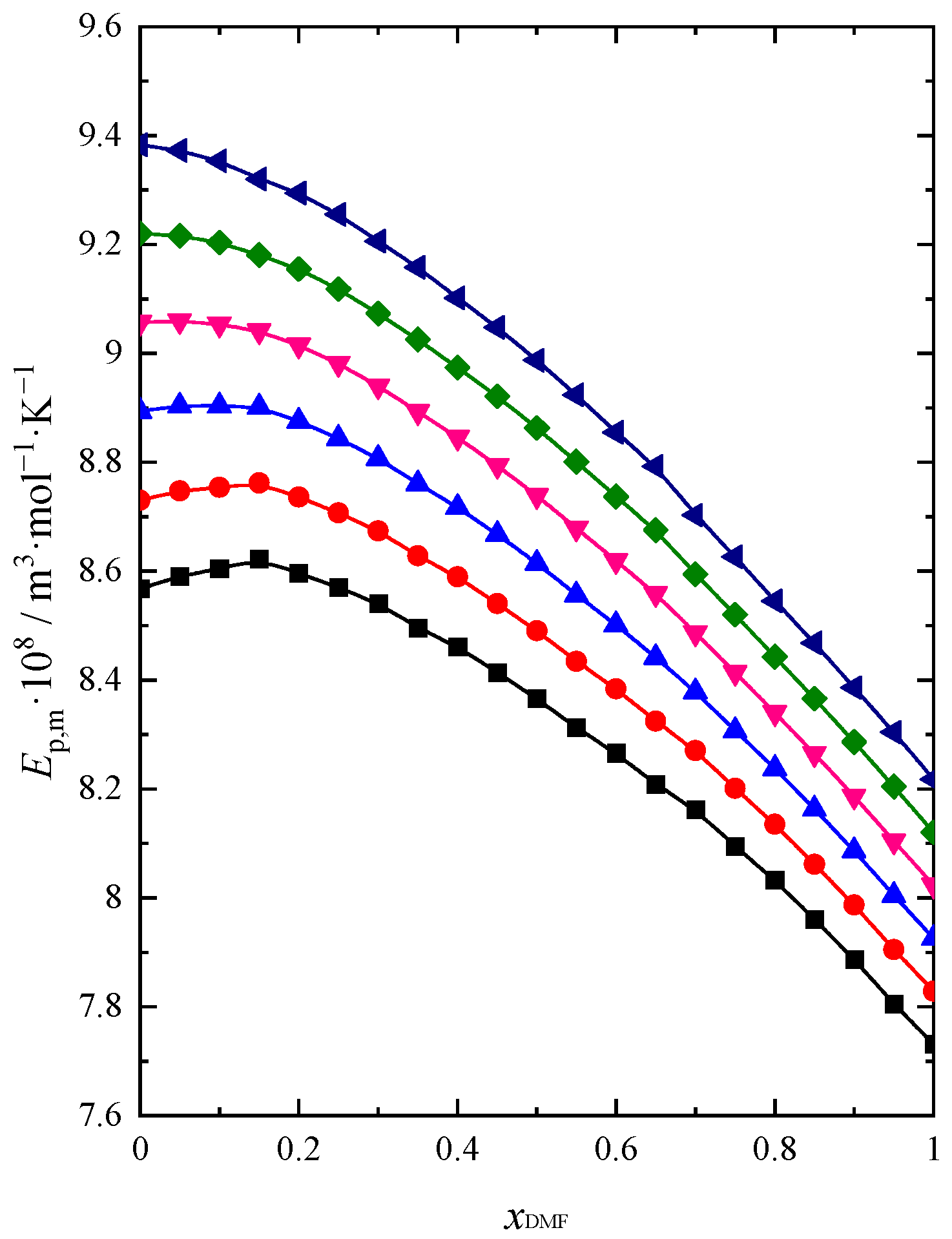
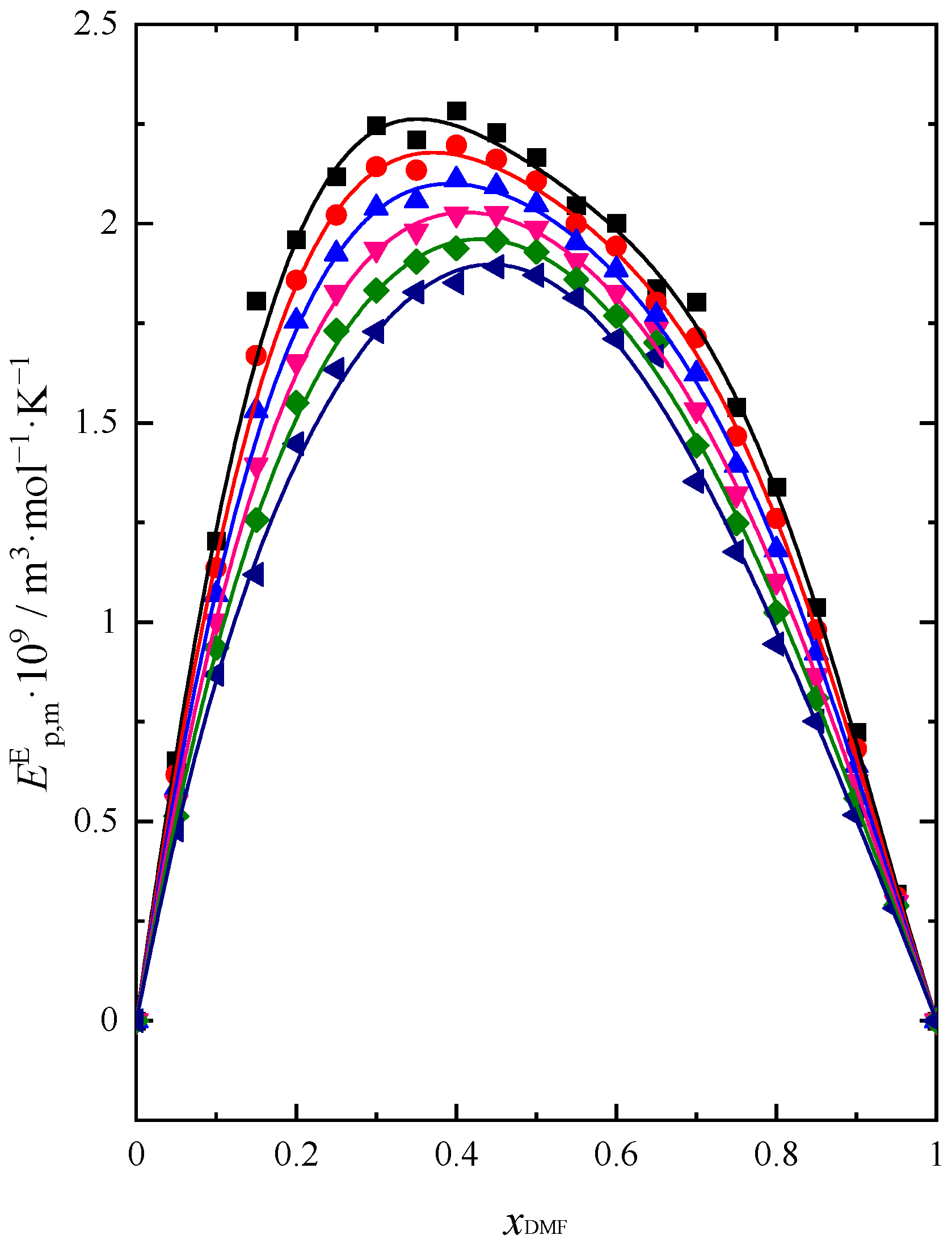


| Name of Compound | Source | Purity a | Purification Method | Mass Fraction of Water b |
|---|---|---|---|---|
| 1-butanol (BuOH) | Sigma-Aldrich (Poznan-Poland) | >0.998 | 3 × 10−3 | |
| N,N-dimethylformamide (DMF) | Sigma-Aldrich (Poznan-Poland) | 0.998 | Distillation under reduced pressure | 2 × 10−4 |
| BuOH | DMF | |||||||
|---|---|---|---|---|---|---|---|---|
| T/K | ρ (kg·m−3) | u (m·s−1) | ρ (kg·m−3) | u (m·s−1) | ||||
| Exp. | Lit. | Exp. | Lit. | Exp. | Lit. | Exp. | Lit. | |
| 293.15 | 809.55 | 809.17 [39] | 1256.4 | 1256 [40] | 948.69 | 948.742 [41] | 1477.0 | 1499 [18] |
| 809.5 [42] | 1256 [42] | 948.747 [43] | ||||||
| 809.53 [44] | 1256.33 [45] | 948.737 [46] | ||||||
| 809.54 [19] | 1256.55 [47] | 948.611 [48] | ||||||
| 809.58 [45] | 1256.57 [44] | 948.546 [49] | ||||||
| 809.64 [50] | 1260.4 [18] | 948.584 [51] | ||||||
| 809.664 [47] | 948.67 [19] | |||||||
| 809.7 [52] | 948.73 [52] | |||||||
| 809.78 [15] | 948.653 [53] | |||||||
| 809.79 [54] | ||||||||
| 809.8 [40] | ||||||||
| 809.94 [55] | ||||||||
| 298.15 | 805.74 | 805.54 [39] | 1239.5 | 1239.28 [56] | 943.92 | 943.976 [41] | 1457.4 | 1469.5 [57] |
| 805.6 [16] | 1239.29 [45] | |||||||
| 805.73 [19] | 1240.09 [58] | 943.981 [43] | 1457.69 [59] | |||||
| 805.76 [60] | 1240.2 [61] | 943.971 [46] | 1458.5 [62] | |||||
| 805.77 [50] | 1240.37 [63] | 944.290 [59] | 1457.49 [64] | |||||
| 805.79 [45] | 1240.52 [47] | 943.869 [65] | 1458 [66] | |||||
| 805.806 [67] | 1240.55 [68] | 943.817 [51] | 1457.13 [49] | |||||
| 805.81 [63] | 1241 [42] | 944.603 [66] | 1478 [18] | |||||
| 805.851 [47] | 1241.6 [18] | 943.90 [19] | 1465.2 [61] | |||||
| 805.89 [69] | 1277.55 [50] | 943.97 [52] | ||||||
| 805.94 [70] | 944.09 [70] | |||||||
| 806 [52] | ||||||||
| 806.06 [68] | ||||||||
| 303.15 | 801.90 | 801.90 [39] | 1222.7 | 1222.36 [45] | 939.14 | 939.201 [41] | 1438.0 | 1438.23 [59] |
| 801.90 [19] | 1222.5 [71] | 939.206 [43] | 1440.2 [62] | |||||
| 801.92 [71] | 1223 [40] | 939.196 [46] | 1476.2 [72] | |||||
| 801.94 [73] | 1223.69 [47] | 939.047 [59] | 1458.8 [18] | |||||
| 801.95 [45] | 1224 [42] | 939.073 [48] | ||||||
| 801.970 [67] | 1229.2 [18] | 939.042 [51] | ||||||
| 802.009 [47] | 939.13 [19] | |||||||
| 802.04 [15] | 939.8 [73] | |||||||
| 802.28 [55] | ||||||||
| 802.31 [14] | ||||||||
| 308.15 | 798.03 | 789.03 [19] | 1206.0 | 1206.5 [44] | 934.36 | 934.425 [41] | 1418.6 | 1421.95 [59] |
| 789.25 [39] | 1206.5 [71] | 934.430 [43] | 1420.8 [62] | |||||
| 798 [61] | 1205.54 [45] | 934.420 [46] | 1426.03 [74] | |||||
| 798.06 [44] | 1206.95 [47] | 934.721 [59] | 1464.6 [72] | |||||
| 798.10 [45] | 1215.6 [18] | 934.298 [65] | 1432.4 [18] | |||||
| 798.101 [67] | 934.255 [51] | |||||||
| 798.133 [47] | 934.34 [19] | |||||||
| 798.21 [75] | 941.2 [52] | |||||||
| 798.25 [39] | ||||||||
| 798.41 [55] | ||||||||
| 313.15 | 794.13 | 794.12 [19] | 1189.4 | 1188.65 [76] | 929.56 | 929.478 [59] | 1399.4 | 1395.69 [59] |
| 794.195 [67] | 1188.81 [45] | 929.458 [51] | 1408.8 [18] | |||||
| 794.22 [45] | 1189.6 [77] | 929.55 [19] | ||||||
| 794.42 [68] | 1190.48 [68] | |||||||
| 794.49 [55] | 1193 [78] | |||||||
| 794.60 [39] | 1196.0 [18] | |||||||
| 318.15 | 790.18 | 790.24 [45] | 1173.2 | 1172.19 [45] | 924.76 | 924.683 [65] | 1380.4 | 1453.5 [72] |
| 790.248 [55] | 1173.0 [77] | 925.49 [79] | ||||||
| 790.5 [61] | 924.04 [80] | |||||||
| 790.53 [67] | 924.6 [81] | |||||||
| 790.97 [39] | ||||||||
| T/K | Cp,m/(J·mol−1·K−1) | |||
|---|---|---|---|---|
| BuOH | DMF | |||
| This Paper | Literature | This Paper | Literature | |
| 293.15 | 176.3 | 173.80 [84] 174.00 [50] 176.8 [83] b 177.6 [83] a | 148.3 | 147.16 [63] 147.3 [62] a 147.5 [62] b |
| 298.15 | 179.3 | 177.10 [84] 177.47 [50] 180.9 [83] b 181.4 [83] a | 148.9 | 148.0 [83] b 148.1 [83] a 148.16 [85] 148.2 [85] 148.54 [62] 150.16 [86] |
| 303.15 | 182.7 | 180.61 [84] 185.2 [83] a 185.3 [83] b | 149.5 | 148.5 [83] b 148.9 [83] a 150.41 [62] 151.3 [87] 153.32 [86] |
| 308.15 | 186.3 | 184.28 [84] 189.2 [83] a 189.8 [83] b | 150.2 | 149.1 [83] b 149.8 [83] a 152.65 [62] |
| 313.15 | 190.1 | 188.02 [84] 193.2 [83] a 194.4 [83] b | 150.9 | 149.7 [83] b 150.7 [83] a 152.9 [87] |
| 318.15 | 193.9 | 192.10 [84] 197.3 [83] a 199.1 [83] b | 151.6 | 150.4 [83] b 151.5 [83] a |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tyczyńska, M.; Dentkiewicz, A.; Jóźwiak, M. Thermodynamic and Thermal Analyze of N,N-Dimethylformamide + 1-Butanol Mixture Properties Based on Density, Sound Velocity and Heat Capacity Data. Molecules 2023, 28, 4698. https://doi.org/10.3390/molecules28124698
Tyczyńska M, Dentkiewicz A, Jóźwiak M. Thermodynamic and Thermal Analyze of N,N-Dimethylformamide + 1-Butanol Mixture Properties Based on Density, Sound Velocity and Heat Capacity Data. Molecules. 2023; 28(12):4698. https://doi.org/10.3390/molecules28124698
Chicago/Turabian StyleTyczyńska, Magdalena, Aleksandra Dentkiewicz, and Małgorzata Jóźwiak. 2023. "Thermodynamic and Thermal Analyze of N,N-Dimethylformamide + 1-Butanol Mixture Properties Based on Density, Sound Velocity and Heat Capacity Data" Molecules 28, no. 12: 4698. https://doi.org/10.3390/molecules28124698
APA StyleTyczyńska, M., Dentkiewicz, A., & Jóźwiak, M. (2023). Thermodynamic and Thermal Analyze of N,N-Dimethylformamide + 1-Butanol Mixture Properties Based on Density, Sound Velocity and Heat Capacity Data. Molecules, 28(12), 4698. https://doi.org/10.3390/molecules28124698





