Mathematical Geometry and Groups for Low-Symmetry Metal Complex Systems
Abstract
1. Introduction
2. Symmetry and Structural Inorganic Chemistry
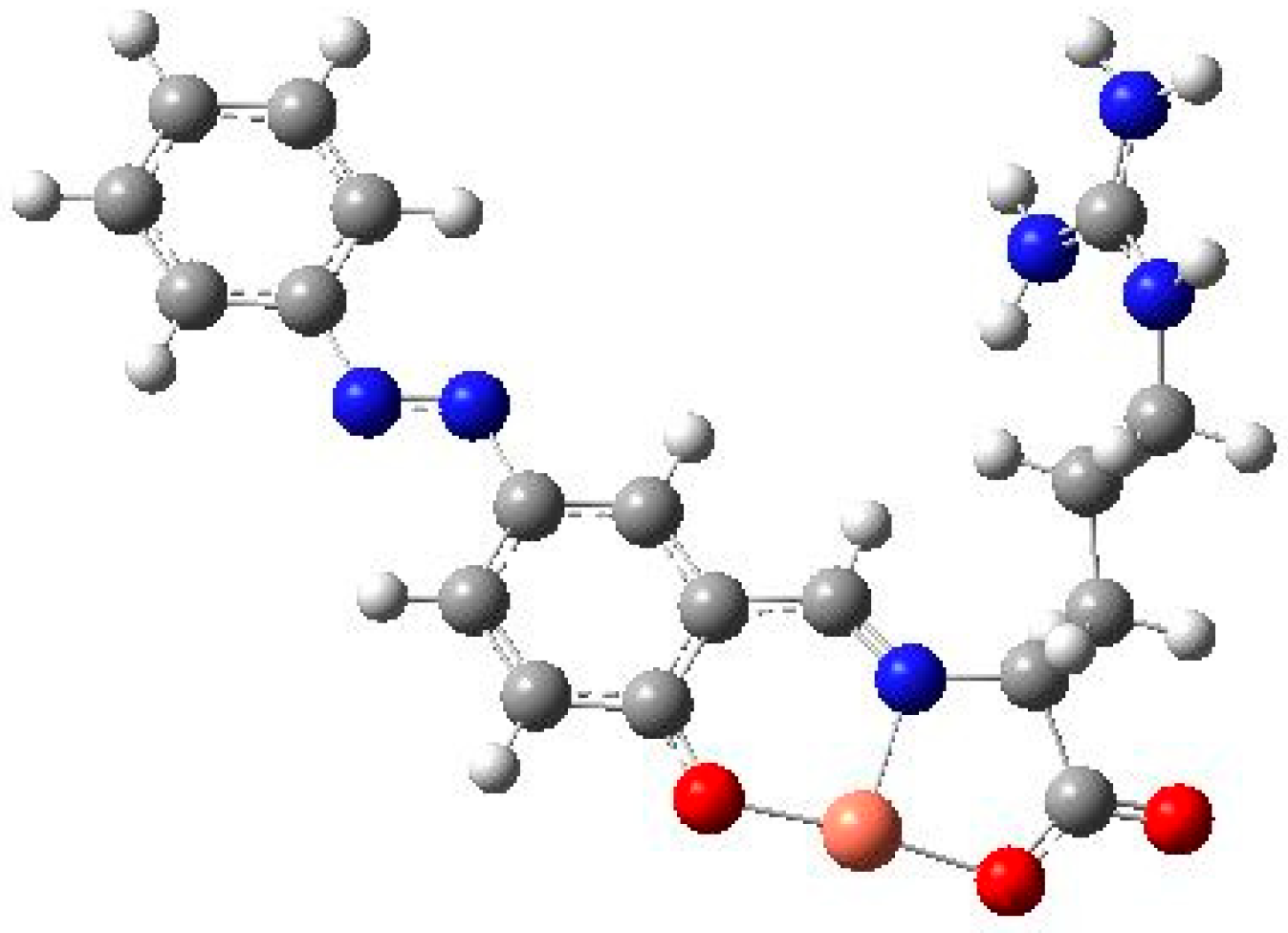
3. Limitations of Group Theory and ‘Actual’ Low-Symmetry Compounds
4. Conclusions and Future Perspectives
Funding
Conflicts of Interest
Sample Availability
References
- Kotani, M. Mathematics and Chemistry-Exploring New Collaborations in a Digital Society. Chem. Chem. Ind. 2018, 71, 457–458. Available online: https://www.chemistry.or.jp/opinion/ronsetsu1806.pdf (accessed on 29 May 2023).
- Kotani, M. A Challenge by Mathematics-Materials Science Collaboration. Hyomen Kagaku 2013, 34, 3–8. [Google Scholar] [CrossRef]
- Hirata, A.; Kang, L.J.; Klumov, B.; Matsue, K.; Kotani, M.; Yavari, A.R.; Chen, M.W. Geometric Frustration of Icosahedron in Metallic Glasses. Science 2013, 341, 376–379. [Google Scholar] [CrossRef] [PubMed]
- Sunada, T. Lecture on topological crystallography. Jpn. J. Math. 2012, 7, 1–39. [Google Scholar] [CrossRef]
- Sunada, T. Why do diamonds look so beautiful? Crystal lattices with big symmetry. Expo. Curr. Math. 2006, 2006, 26–35. [Google Scholar] [CrossRef]
- Flapan, E. When Topology Meets Chemistry: A Topological Look at Molecular Chirality; Cambridge University Press: Cambridge, UK, 2000; ISBN 0-521-66482-9. [Google Scholar]
- Tagami, M.; Liang, Y.; Naito, H.; Kawazoe, Y.; Kotani, M. Negatively curved cubic carbon crystals with octahedral symmetry. Carbon 2014, 76, 266–274. [Google Scholar] [CrossRef]
- Wadachi, M. Differential & Topological Geometry; Iwanami Shoten: Tokyo, Japan, 1996; ISBN 4-00-007980-8. [Google Scholar]
- Nagaosa, N. Differential Geometry and Topology; Maruzen: Tokyo, Japan, 2016; ISBN 978-4-621-30067-1. [Google Scholar]
- Nakauchi, N. Geometry Must be Differentiated–Introduction to Differential Geometry; Gendaisugakusha: Kyoto, Japan, 2011; ISBN 978-4-7687-0570-0. [Google Scholar]
- Tachibana, A.; Fukui, K. Differential Geometry of Chemically Reacting Systems. Thoret. Chim. Acta 1978, 49, 321–347. [Google Scholar] [CrossRef]
- Louie, A.H.; Somorjai, R.L. Differential Geometry of Proteins: A Structural and Dynamical Representation of Patterns. J. Theor. Biol. 1982, 98, 189–209. [Google Scholar] [CrossRef] [PubMed]
- Blum, Z.; Lidin, S. The Carcerand, an Organic Structure on a Minimal Surface. Org. Chem. Biochem. 1988, 42, 332–335. [Google Scholar] [CrossRef]
- Blum, Z.; Lidin, S.; Eberson, L. Differential Geometry and Organic Chemistry; the Bonnet Transformation Applied to the Racemization of Tri-o-thymotide and Isomerization of Cyclophenes. Acta Chem. Scandinavica. Ser. B Org. Chem. Biochem. 1988, 42, 484–488. [Google Scholar] [CrossRef]
- Zimpel, Z.; Mezey, P.G. Molecular Geometry and Symmetry from a Differential Geometry Viewpoint. Int. J. Quantum Chem. 1997, 64, 669–678. [Google Scholar] [CrossRef]
- Kocian, P.; Schenk, K.; Chapuis, G. Differential geometry: A natural tool for describing symmetry operations. Acta Cryst. A 2009, 65, 329–341. [Google Scholar] [CrossRef] [PubMed]
- Kocian, P.; Schenk, K.; Chapuis, G. The role of the tangent bundle for symmetry operations and modulated structures. Acta Cryst. A 2010, 66, 394–406. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, D.D.; Wei, G.-W. DG-GL: Differential geometry-based geometric learning of molecular datasets. Int. J. Numer. Methods Biomed. Eng. 2019, 35, e3179. [Google Scholar] [CrossRef] [PubMed]
- Spackman, M.A.; Jayatilaka, D. Hirshfeld surface analysis. CrystEngComm 2009, 11, 19–32. [Google Scholar] [CrossRef]
- Banaru, A.; Kochnev, A. The minimal set of intermolecular interactions in the structures of substituted prolines. Stud. UBB Chem. LXII 2017, 1, 121–128. [Google Scholar] [CrossRef]
- Yuasa, M.; Akitsu, T. Fundamental and Applications of Coordination Chemistry; Corona Publishing Co., Ltd.: Tokyo, Japan, 2014; pp. 30–32. [Google Scholar]
- Akitsu, T. Lecture Note on Inorganic Chemistry, 2nd ed.; Gendaitsho: Sagamihara, Japan, 2019; p. 136. [Google Scholar]
- Kuroda, Y.; Miyazaki, R.; Shimonishi, D.; Nakane, D.; Akitsu, T. Coordination and Photoisomerization of Azobenzene-Amino Acid Schiff Base Copper(II) Complexes to Lysozyme. J. Mater. Sci. Chem. Eng. 2023, 11, 34–44. [Google Scholar] [CrossRef]
- Cotton, F.A. Chemical Applications of Group Theory, 3rd ed.; Wiley-Interscience: Hoboken, NJ, USA, 1990. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akitsu, T. Mathematical Geometry and Groups for Low-Symmetry Metal Complex Systems. Molecules 2023, 28, 4509. https://doi.org/10.3390/molecules28114509
Akitsu T. Mathematical Geometry and Groups for Low-Symmetry Metal Complex Systems. Molecules. 2023; 28(11):4509. https://doi.org/10.3390/molecules28114509
Chicago/Turabian StyleAkitsu, Takashiro. 2023. "Mathematical Geometry and Groups for Low-Symmetry Metal Complex Systems" Molecules 28, no. 11: 4509. https://doi.org/10.3390/molecules28114509
APA StyleAkitsu, T. (2023). Mathematical Geometry and Groups for Low-Symmetry Metal Complex Systems. Molecules, 28(11), 4509. https://doi.org/10.3390/molecules28114509





