Comparison of Proton Acceptor and Proton Donor Properties of H2O and H2O2 in Organic Crystals of Drug-like Compounds: Peroxosolvates vs. Crystallohydrates
Abstract
:1. Introduction
- The H-bond enthalpy in crystalline hydrates ([2-amino-nicotinic acid+maleic acid+H2O] (1:1:1), [N-(5-Nitro-2-furfurylidene)-1-aminohydantoin+H2O] (1:1)) and peroxosolvates ([2-amino-nicotinic acid+maleic acid+H2O2] (1:1:1), [N-(5-Nitro-2-furfurylidene)-1-aminohydantoin+H2O2] (1:1)) (Scheme 1) was determined using periodic DFT calculations [30] followed by Rosenberg’s equation [23,24]. The structures of the crystalline hydrates were studied by X-ray diffraction [35,36]. The isomorphic or isostructural peroxosolvates were purposefully prepared for this study.
- The spectroscopic features of the considered crystals were studied by low-frequency Raman spectroscopy followed by periodic DFT computations.
2. Results and Discussion
2.1. Features of H-Bonded Networks in Hydrates and Isomorphic Peroxosolvates
2.2. The H-Bond Enthalpy in the Selected Crystalline Hydrates and Peroxosolvates
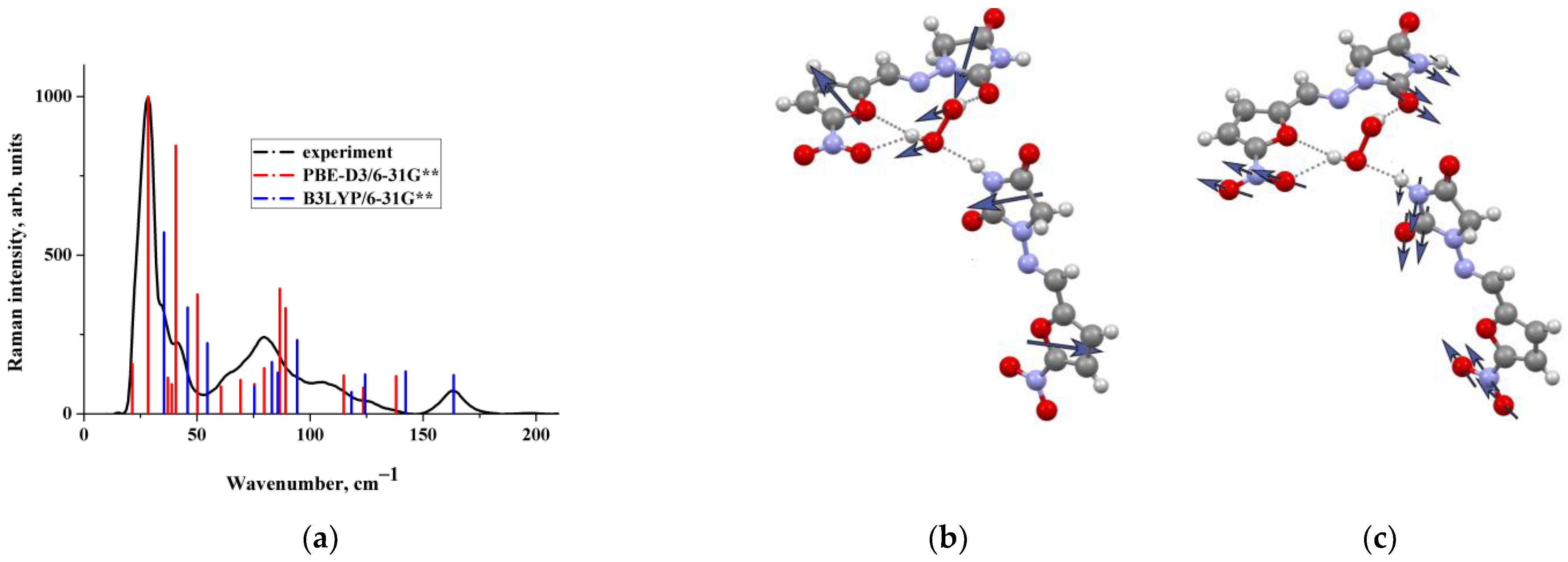
2.3. Low-Frequency Raman Spectra of the Considered Crystals
3. Materials and Methods
3.1. Compounds and Solvents
3.2. Cocrystal Preparation
3.3. Single Crystal X-ray Diffraction
3.4. Raman Spectroscopy
3.5. Periodic (Solid-State) DFT Computations
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Evora, A.O.L.; Castro, R.A.E.; Maria, T.M.R.; Rosado, M.T.S.; Silva, M.R.; Beja, A.M.; Canotilho, J.; Eusebio, M.E.S. Pyrazinamide-Diflunisal: A New Dual-Drug Co-Crystal. Cryst. Growth Des. 2011, 11, 4780–4788. [Google Scholar] [CrossRef]
- Grobelny, P.; Mukherjee, A.; Desiraju, G.R. Drug-drug co-crystals: Temperature-dependent proton mobility in the molecular complex of isoniazid with 4-aminosalicylic acid. CrystEngComm 2011, 13, 4358–4364. [Google Scholar] [CrossRef]
- Kaur, R.; Cavanagh, K.L.; Rodriguez-Hornedo, N.; Matzger, A.J. Multidrug Cocrystal of Anticonvulsants: Influence of Strong Intermolecular Interactions on Physiochemical Properties. Cryst. Growth Des. 2017, 17, 5012–5016. [Google Scholar] [CrossRef] [PubMed]
- Sekhon, B.S. Drug-drug co-crystals. DARU J. Pharm. Sci. 2012, 20, 45. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Linley, E.; Denyer, S.P.; McDonnell, G.; Simons, C.; Maillard, J.Y. Use of hydrogen peroxide as a biocide: New consideration of its mechanisms of biocidal action. J. Antimicrob. Chemotherm. 2012, 67, 1589–1596. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kersten, K.M.; Breen, M.E.; Mapp, A.K.; Matzger, A.J. Pharmaceutical solvate formation for the incorporation of the antimicrobial agent hydrogen peroxide. Chem. Commun. 2018, 54, 9286–9289. [Google Scholar] [CrossRef] [PubMed]
- Ahn, S.H.; Cluff, K.J.; Bhuvanesh, N.; Blümel, J. Hydrogen Peroxide and Di(hydroperoxy)propane Adducts of Phosphine Oxides as Stoichiometric and Soluble Oxidizing Agents. Angew. Chem. Int. Ed. 2015, 54, 13341–13345. [Google Scholar] [CrossRef] [PubMed]
- Hilliard, C.R.; Bhuvanesh, N.; Gladysz, J.A.; Blümel, J. Synthesis, purification, and characterization of phosphine oxides and their hydrogen peroxide adducts. Dalton Trans. 2012, 41, 1742–1754. [Google Scholar] [CrossRef] [PubMed]
- Churakov, A.V.; Grishanov, D.A.; Medvedev, A.G.; Mikhaylov, A.A.; Vener, M.V.; Navasardyan, M.A.; Tripol’skaya, T.A.; Lev, O.; Prikhodchenko, P.V. Stabilization of hydrogen peroxide by hydrogen bonding in the crystal structure of 2-aminobenzimidazole perhydrate. Cryst. Eng. Comm. 2020, 22, 2866–2872. [Google Scholar] [CrossRef]
- Foroughi, L.M.; Metzger, A.J. From Hydrate to Peroxosolvate: A Test of Prediction with Cyclic N-Oxides. Cryst. Growth Des. 2021, 21, 5873–5879. [Google Scholar] [CrossRef]
- Luo, J.; Xia, H.; Zhang, W.; Song, S.; Zhang, Q. A promising hydrogen peroxide adduct of ammonium cyclopentazolate as a green propellant component. J. Mater. Chem. A 2020, 8, 12334–12338. [Google Scholar] [CrossRef]
- Gillon, A.L.; Feeder, N.; Davey, R.J.; Storey, R. Hydration in Molecular Crystals-A Cambridge Structural Database Analysis. Cryst. Growth Des. 2003, 3, 663–673. [Google Scholar] [CrossRef]
- Infantes, L.; Fabian, L.; Motherwell, W.D.S. Organic crystal hydrates: What are the important factors for formation. CrystEngComm 2007, 9, 65–71. [Google Scholar] [CrossRef]
- Chernyshov, I.Y.; Vener, M.V.; Prikhodchenko, P.V.; Medvedev, A.G.; Lev, O.; Churakov, A.V. Peroxosolvates: Formation Criteria, H2O2 Hydrogen Bonding, and Isomorphism with the Corresponding Hydrates. Cryst. Growth Des. 2017, 17, 214–220. [Google Scholar] [CrossRef]
- Vener, M.V.; Medvedev, A.G.; Churakov, A.V.; Prikhodchenko, P.V.; Tripol’skaya, T.A.; Lev, O. H-Bond Network in Amino Acid Cocrystals with H2O or H2O2. The DFT Study of Serine–H2O and Serine–H2O2. J. Phys. Chem. A 2011, 115, 13657–13663. [Google Scholar] [CrossRef] [PubMed]
- Medvedev, A.G.; Shishkina, A.V.; Prikhodchenko, P.V.; Lev, O.; Vener, M.V. The Applicability of the Dimeric Heterosynthon Concept to Molecules with Equivalent Binding Sites. A DFT Study of Crystalline Urea–H2O2. RSC Adv. 2015, 5, 29601–29608. [Google Scholar] [CrossRef] [Green Version]
- Churakov, A.V.; Prikhodchenko, P.V.; Howard, J.A.K.; Lev, O. Glycine and L-serine Crystalline Perhydrates. Chem. Commun. 2009, 4224–4226. [Google Scholar] [CrossRef]
- Prikhodchenko, P.V.; Medvedev, A.G.; Tripol’skaya, T.A.; Churakov, A.V.; Wolanov, Y.; Howard, J.A.K.; Lev, O. Crystal Structures of Natural Amino Acid Perhydrates. CrystEngComm 2011, 13, 2399–2407. [Google Scholar] [CrossRef]
- Yamaguchi, R.; Tanaka, R.; Maetani, M.; Tabe, H.; Yamada, Y. Efficient capturing of hydrogen peroxide in dilute aqueous solution by co-crystallization with amino acids. CrystEngComm 2021, 23, 5456–5462. [Google Scholar] [CrossRef]
- Steiner, T. The Hydrogen Bond in the Solid State. Angew. Chem. Int. Ed. 2002, 41, 48–76. [Google Scholar] [CrossRef]
- Herschlag, D.; Pinney, M.M. Hydrogen Bonds: Simple after All? Biochemistry 2018, 57, 3338–3352. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Musin, R.N.; Mariam, Y.H. An integrated approach to the study of intramolecular hydrogen bonds in malonaldehyde enol derivatives and naphthazarin: Trend in energetic versus geometrical consequences. J. Phys. Org. Chem. 2006, 19, 425–444. [Google Scholar] [CrossRef]
- Vener, M.V.; Levina, E.O.; Astakhov, A.A.; Tsirelson, V.G. Specific Features of the Extra Strong Intermolecular Hydrogen Bonds in Crystals: Insights from the Theoretical Charge Density Analysis. Chem. Phys. Lett. 2015, 638, 233–236. [Google Scholar] [CrossRef]
- Iogansen, A.V. Direct Proportionality of the Hydrogen Bonding Energy and the Intensification of the Stretching XH Vibration in Infrared Spectra. Spectrochim. Acta A 1999, 55, 1585–1612. [Google Scholar] [CrossRef]
- Rozenberg, M.; Loewenschuss, A.; Marcus, Y. An Empirical Correlation Between Stretching Vibration Redshift and Hydrogen Bond Length. Phys. Chem. Chem. Phys. 2000, 2, 2699–2702. [Google Scholar] [CrossRef]
- Rozenberg, M.; Shoham, G.; Reva, I.; Fausto, R. A correlation between the proton stretching vibration red shift and the hydrogen bond length in polycrystalline amino acids and peptides. Phys. Chem. Chem. Phys. 2005, 7, 2376–2383. [Google Scholar] [CrossRef] [Green Version]
- Mata, I.; Alkorta, I.; Espinosa, E.; Molins, E. Relationships Between Interaction Energy, Intermolecular Distance and Electron Density Properties in Hydrogen Bonded Complexes under External Electric Fields. Chem. Phys. Lett. 2011, 507, 185–189. [Google Scholar] [CrossRef]
- Medvedev, A.G.; Churakov, A.V.; Prikhodchenko, P.V.; Lev, O.; Vener, M.V. Crystalline Peroxosolvates: Nature of the Coformer, Hydrogen-Bonded Networks and Clusters, Intermolecular Interactions. Molecules 2021, 26, 26. [Google Scholar] [CrossRef]
- Yukhnevich, G.V. Relationship Between the Lengths of Covalent and Intermolecular Bonds in X-HY bridges. Crystallogr. Rep. 2010, 55, 377–380. [Google Scholar] [CrossRef]
- Evarestov, R.A. Quantum Chemistry of Solids; Springer: Berlin/Heidelberg, Germany, 2012; pp. 1–734. [Google Scholar] [CrossRef] [Green Version]
- Deringer, V.L.; George, J.; Dronskowski, R.; Englert, U. Plane-Wave Density Functional Theory Meets Molecular Crystals: Thermal Ellipsoids and Intermolecular Interactions. Acc. Chem. Res. 2017, 50, 1231–1239. [Google Scholar] [CrossRef]
- Churakov, A.V.; Grishanov, D.A.; Medvedev, A.G.; Mikhaylov, A.A.; Tripol’skaya, T.A.; Vener, M.V.; Navasardyan, M.A.; Lev, O.; Prikhodchenko, P.V. Cyclic Dipeptide Peroxosolvates: First Direct Evidence for Hydrogen Bonding Between Hydrogen Peroxide and a Peptide Backbone. CrystEngComm 2019, 21, 4961–4968. [Google Scholar] [CrossRef]
- Groom, C.R.; Bruno, I.J.; Lightfoot, M.P.; Ward, S.C. The Cambridge Structural Database. Acta Cryst. 2016, B72, 171–179. [Google Scholar] [CrossRef]
- Belsky, A.; Hellenbrandt, M.; Karen, V.L.; Luksch, P. New developments in the Inorganic Crystal Structure Database (ICSD): Accessibility in support of materials research and design. Acta Cryst. 2002, B58, 364–369. [Google Scholar] [CrossRef] [Green Version]
- Pienaar, E.W.; Caira, M.R.; Lotter, A.P. Polymorphs of nitrofurantoin. I. Preparation and X-ray crystal structures of two monohydrated forms of nitrofurantoin. J. Crystallogr. Spectrosc. Res. 1993, 23, 739–744. [Google Scholar] [CrossRef]
- Surov, A.O.; Vasilev, N.A.; Churakov, A.V.; Parashchuk, O.D.; Artobolevskii, S.V.; Alatortsev, O.A.; Makhrov, D.E.; Vener, M.V. Two Faces of Water in the Formation and Stabilization of Multicomponent Crystals of Zwitterionic Drug-Like Compounds. Symmetry 2021, 13, 425. [Google Scholar] [CrossRef]
- Tupikina, E.Y.; Bodensteiner, M.; Tolstoy, P.M.; Denisov, G.S.; Shenderovich, I.G. P=O Moiety as an Ambidextrous Hydrogen Bond Acceptor. J. Phys. Chem. C 2018, 122, 1711–1720. [Google Scholar] [CrossRef]
- Bennion, J.C.; Chowdhury, N.; Kampf, J.W.; Matzger, A.J. Hydrogen Peroxide Solvates of 2,4,6,8,10,12-Hexanitro-2,4,6,8,10,12-hexaazaisowurtzitane. Angew. Chem. Int. Ed. 2016, 55, 13118–13121. [Google Scholar] [CrossRef]
- Manin, A.N.; Voronin, A.P.; Shishkina, A.V.; Vener, M.V.; Churakov, A.V.; Perlovich, G.L. Influence of Secondary Interactions on the Structure, Sublimation Thermodynamics, and Solubility of Salicylate:4-Hydroxybenzamide Cocrystals. Combined Experimental and Theoretical Study. J. Phys. Chem. B 2015, 119, 10466–10477. [Google Scholar] [CrossRef] [PubMed]
- Taylor, R. It Isn’t, It Is: The C–H···X (X = O, N, F, Cl) Interaction Really Is Significant in Crystal Packing. Cryst. Growth Des. 2016, 16, 4165–4168. [Google Scholar] [CrossRef]
- Tuma, C.; Boese, D.A.; Handy, N.C. Predicting the binding energies of H-bonded complexes: A comparative DFT study. Phys. Chem. Chem. Phys. 1999, 1, 3939–3947. [Google Scholar] [CrossRef]
- Vener, M.V.; Levina, E.O.; Koloskov, O.A.; Rykounov, A.A.; Voronin, A.P.; Tsirelson, V.G. Evaluation of the lattice energy of the two-component molecular crystals using solid-state density functional theory. Cryst. Growth Des. 2014, 14, 4997–5003. [Google Scholar] [CrossRef]
- Medvedev, A.G.; Mikhaylov, A.A.; Chernyshov, I.Y.; Vener, M.V.; Lev, O.; Prikhodchenko, P.V. Effect of aluminum vacancies on the H2O2 or H2O interaction with a gamma-AlOOH surface. A solid-state DFT study. Int. J. Quantum Chem. 2019, 119, e25920. [Google Scholar] [CrossRef]
- Parrott, E.P.J.; Zeitler, J.A. Terahertz Time-Domain and Low-Frequency Raman Spectroscopy of Organic Materials. Appl. Spectros. 2015, 69, 1–25. [Google Scholar] [CrossRef] [PubMed]
- King, M.D.; Buchanan, W.D.; Korter, T.M. Identification and Quantification of Polymorphism in the Pharmaceutical Compound Diclofenac Acid by Terahertz Spectroscopy and Solid-State Density Functional Theory. Anal. Chem. 2011, 83, 3786–3792. [Google Scholar] [CrossRef]
- Zhang, F.; Wang, H.-W.; Tominaga, K.; Hayashi, M. Mixing of intermolecular and intramolecular vibrations in optical phonon modes: Terahertz spectroscopy and solid-state density functional theory. WIREs Comput. Mol. Sci. 2016, 6, 386–409. [Google Scholar] [CrossRef]
- Vener, M.V.; Parashchuk, O.D.; Kharlanov, O.G.; Maslennikov, D.R.; Dominskiy, D.I.; Chernyshov, I.Y.; Paraschuk, D.Y.; Sosorev, A.Y. Non-Local Electron-Phonon Interaction in Naphthalene Diimide Derivatives, its Experimental Probe and Impact on Charge-Carrier Mobility. Adv. Electron. Mater. 2021, 7, 2001281. [Google Scholar] [CrossRef]
- Takahashi, M. Terahertz Vibrations and Hydrogen-Bonded Networks in Crystals. Crystals 2014, 4, 74–103. [Google Scholar] [CrossRef]
- Surov, A.O.; Vasilev, N.A.; Vener, M.V.; Parashchuk, O.D.; Churakov, A.V.; Magdysyuk, O.V.; Perlovich, G.L. Pharmaceutical Salts of Fenbendazole with Organic Counterions: Structural Analysis and Solubility Performance. Cryst. Growth Des. 2021, 21, 4516–4530. [Google Scholar] [CrossRef]
- Vener, M.V.; Chernyshov, I.Y.; Rykounov, A.A.; Filarowski, A. Structural and Spectroscopic Features of Proton Hydrates in the Crystalline State. Solid-state DFT Study on HCl and Triflic Acid Hydrates. Mol. Phys. 2018, 116, 251–262. [Google Scholar] [CrossRef]
- Scott, A.P.; Radom, L.J. Harmonic Vibrational Frequencies: An Evaluation of Hartree–Fock, Møller–Plesset, Quadratic Configuration Interaction, Density Functional Theory, and Semiempirical Scale Factors. J. Phys. Chem. 1996, 100, 16502–16513. [Google Scholar] [CrossRef]
- Sosorev, A.Y.; Maslennikov, D.; Chernyshov, I.Y.; Dominskiy, D.I.; Bruevich, V.V.; Vener, M.V.; Paraschuk, D.Y. Relationship between electron–phonon interaction and low-frequency Raman anisotropy in high-mobility organic semiconductors. Phys. Chem. Chem. Phys. 2018, 20, 18912–18918. [Google Scholar] [CrossRef] [PubMed]
- Grishanov, D.A.; Navasardyan, M.A.; Medvedev, A.G.; Lev, O.; Prikhodchenko, P.V.; Churakov, A.V. Hydrogen Peroxide Insular Dodecameric and Pentameric Clusters in Peroxosolvate Structures. Angew. Chem. Int. Ed. 2017, 56, 15241–15245. [Google Scholar] [CrossRef] [PubMed]
- Bi, J.; Tao, Y.; Hu, J.-Y.; Wang, H.; Zhou, M. High-pressure investigations on urea hydrogen peroxide. Chem. Phys. Lett. 2022, 787, 139230. [Google Scholar] [CrossRef]
- Giguere, P.A.; Geoffrion, P. Refractive index of hydrogen peroxide solutions. A revision. Can. J. Res. 1949, 27B, 168–173. [Google Scholar] [CrossRef]
- Sheldrick, G.M. SADABS, Program for Scaling and Correction of Area Detector Data; University of Göttingen: Göttingen, Germany, 1997. [Google Scholar]
- Sheldrick, G.M. Crystal structure refinement with SHELXL. Acta. Crystallogr. 2015, C71, 3–8. [Google Scholar]
- Fonari, A.; Corbin, N.S.; Vermeulen, D.; Goetz, K.P.; Jurchescu, O.D.; McNeil, L.E.; Bredas, J.L.; Coropceanu, V. Vibrational properties of organic donor-acceptor molecular crystals: Anthracene-pyromellitic-dianhydride (PMDA) as a case study. J. Chem. Phys. 2015, 143, 224503. [Google Scholar] [CrossRef] [Green Version]
- Mazurek, A.H.; Szeleszczuk, Ł.; Pisklak, D.M. Periodic DFT Calculations—Review of Applications in the Pharmaceutical Sciences. Pharmaceutics 2020, 12, 415. [Google Scholar] [CrossRef] [PubMed]
- Voronin, A.P.; Surov, A.O.; Churakov, A.V.; Parashchuk, O.D.; Rykounov, A.A.; Vener, M.V. Combined X-ray Crystallographic, IR/Raman Spectroscopic, and Periodic DFT Investigations of New Multicomponent Crystalline Forms of Anthelmintic Drugs: A Case Study of Carbendazim Maleate. Molecules 2020, 25, 2386. [Google Scholar] [CrossRef] [PubMed]
- Dovesi, R.; Erba, A.; Orlando, R.; Zicovich-Wilson, C.M.; Civalleri, B.; Maschio, L.; Rérat, M.; Casassa, S.; Baima, J.; Salustro, S.; et al. Quantum-mechanical condensed matter simulations with CRYSTAL. WIREs Comput. Mol. Sci. 2018, 8, e1360. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef] [Green Version]
- Vosko, S.H.; Wilk, L.; Nusair, M. Accurate spin-dependent electron liquid correlation energies for local spin density calculations: A critical analysis. Can. J. Phys. 1980, 58, 1200–1211. [Google Scholar] [CrossRef] [Green Version]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Grimme, S.; Ehrlich, S.; Goerigk, L. Effect of the damping function in dispersion corrected density functional theory. J. Comput. Chem. 2011, 32, 1456–1465. [Google Scholar] [CrossRef] [PubMed]
- Wiscons, R.A.; Bellas, M.K.; Bennion, J.C.; Matzger, A.J. Detonation Performance of Ten Forms of 5,5′-Dinitro-2H,2H′-3,3′-bi-1,2,4-triazole (DNBT). Cryst. Growth Des. 2018, 18, 7701–7707. [Google Scholar] [CrossRef]

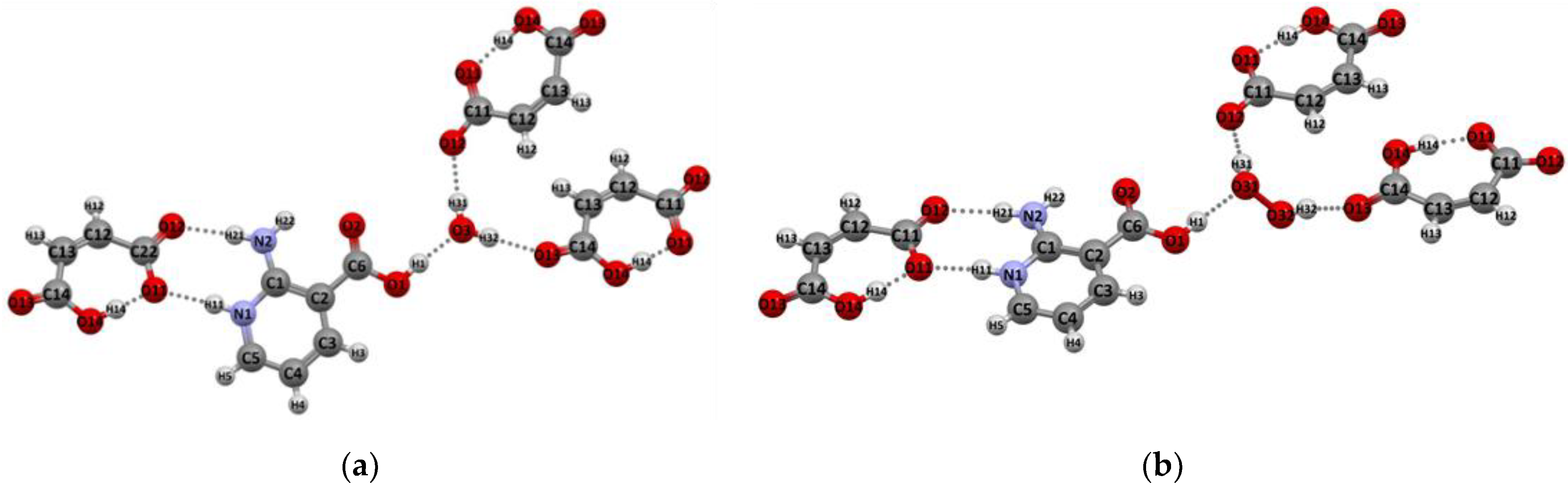
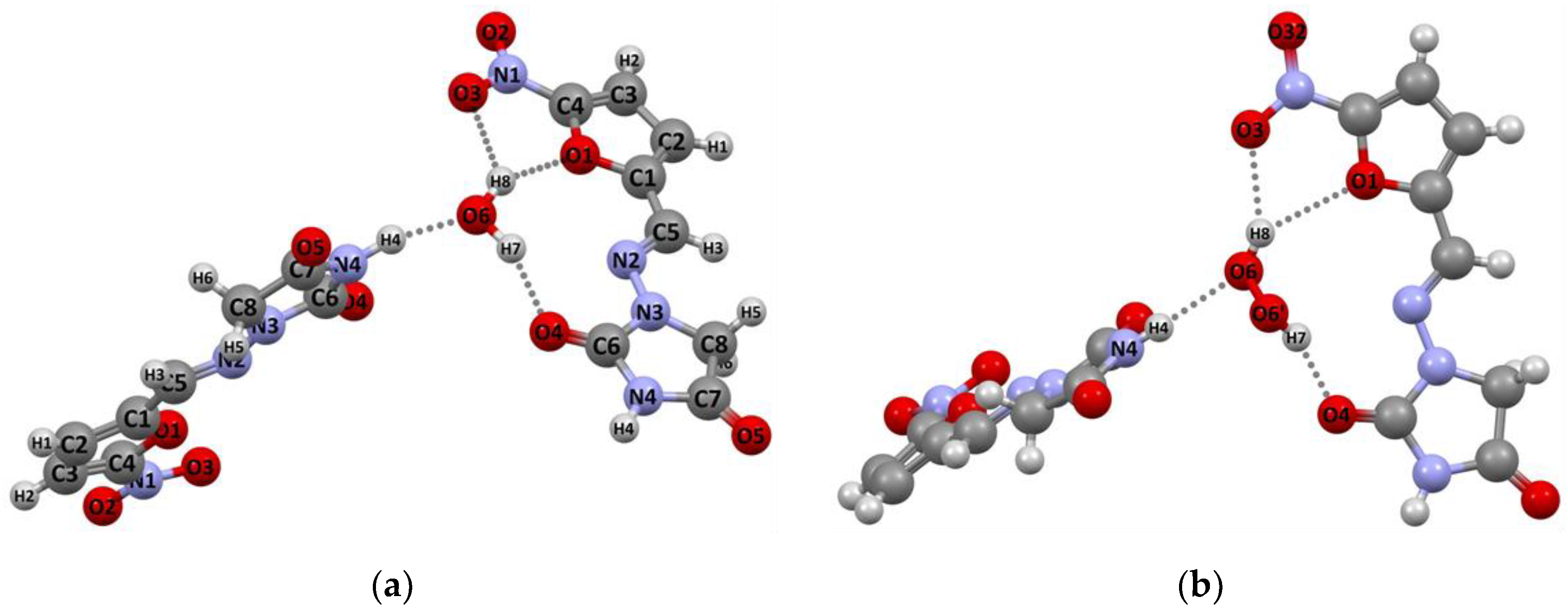


| Fragment 1 | R(O∙∙∙N)/R(O∙∙∙O) 2, Å | R(H∙∙∙O), Å | ∆HHB, kJ/mol |
|---|---|---|---|
| [2AmNic+Mle+H2O] (1:1:1) | |||
| O12…H21-N2 | 2.804 (2.816) | 1.777 (1.928) | 26.0 |
| O11…H11-N1 | 2.816 (2.816) | 1.770 (1.905) | 26.4 |
| O12…H31-O3 | 2.701 (2.717) | 1.707 (1.860) | 29.4 |
| O13…H32-O3 | 2.765 (2.771) | 1.783 (1.900) | 25.8 |
| O3…H1-O1 | 2.536 (2.567) | 1.484 (1.641) | 45.1 |
| [2AmNic+Mle+H2O2] (1:1:1) | |||
| O12…H21-N2 | 2.846 (2.831) | 1.810 (1.955) | 24.6 |
| O11…H11-N1 | 2.849 (2.735) | 1.805 (1.803) | 24.8 |
| O12…H31-O31 | 2.658 (2.636) | 1.648 (1.759) | 32.8 |
| O13…H32-O32 | 2.774 (2.698) | 1.841 (1.767) | 23.3 |
| O31…H1-O1 | 2.726 (2.646) | 1.738 (1.769) | 27.9 |
| [NFA+H2O] | |||
| N4-H4…O6 | 2.689 (2.763) | 1.653 (1.782) | 32.5 |
| O6-H7…O4 | 2.834 (2.961) | 1.938 (2.015) | 20.0 |
| O6-H8…O1 | 3.080 (3.148) | 2.338 (2.245) | 11.3 |
| O6-H8…O3 | 2.966 (3.172) | 2.100 (2.392) | 15.6 |
| [NFA+H2O2] | |||
| N4-H4…O6 | 2.807 (2.905) | 1.822 (2.098) | 24.1 |
| O6’-H7…O4 | 2.735 (2.737) | 1.786 (1.894) | 25.6 |
| O6-H8…O1 | 3.175 (3.128) | 2.496 (2.477) | 9.2 |
| O6-H8…O3 | 2.871 (2.907) | 1.935 (2.144) | 20.1 |
| [2AmNic+Mle+H2O2] | [NFA+H2O2] | |
|---|---|---|
| Empirical formula | C10H12N2O8 | C8H8N4O7 |
| Fw | 288.22 | 272.18 |
| color, habit | colorless, prism | light-yellow, prism |
| crystal size (mm) | 0.25 × 0.20 × 0.15 | 0.25 × 0.15 × 0.10 |
| crystal system | monoclinic | orthorhombic |
| space group | P21/n | Pbca |
| a (Å) | 9.5451(4) | 13.0154(7) |
| b (Å) | 11.7871(4) | 9.4659(7) |
| c (Å) | 11.0780(4) | 17.9512(10) |
| β (deg) | 105.089(1) | 90 |
| V (Å3) | 1203.40(8) | 2211.6(2) |
| Z | 4 | 8 |
| Dc (g·cm−3) | 1.591 | 1.635 |
| μ (mm−1) | 0.140 | 0.146 |
| F(000) | 600 | 1120 |
| θ range (deg) | 2.51 to 29.00 | 2.27 to 28.00 |
| refl collcd | 11941 | 19769 |
| indep reflns/Rint | 3189/0.0219 | 2671/0.0650 |
| reflns I > 2σ(I) | 2742 | 1995 |
| No of param | 229 | 205 |
| GooF on F2 | 1.047 | 1.025 |
| R1 (I > 2σ(I)) | 0.0345 | 0.0371 |
| wR2(all data) | 0.0954 | 0.0882 |
| largest diff peak/hole (e·Å−3) | 0.368/−0.191 | 0.250/−0.244 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vener, M.V.; Churakov, A.V.; Voronin, A.P.; Parashchuk, O.D.; Artobolevskii, S.V.; Alatortsev, O.A.; Makhrov, D.E.; Medvedev, A.G.; Filarowski, A. Comparison of Proton Acceptor and Proton Donor Properties of H2O and H2O2 in Organic Crystals of Drug-like Compounds: Peroxosolvates vs. Crystallohydrates. Molecules 2022, 27, 717. https://doi.org/10.3390/molecules27030717
Vener MV, Churakov AV, Voronin AP, Parashchuk OD, Artobolevskii SV, Alatortsev OA, Makhrov DE, Medvedev AG, Filarowski A. Comparison of Proton Acceptor and Proton Donor Properties of H2O and H2O2 in Organic Crystals of Drug-like Compounds: Peroxosolvates vs. Crystallohydrates. Molecules. 2022; 27(3):717. https://doi.org/10.3390/molecules27030717
Chicago/Turabian StyleVener, Mikhail V., Andrei V. Churakov, Alexander P. Voronin, Olga D. Parashchuk, Sergei V. Artobolevskii, Oleg A. Alatortsev, Denis E. Makhrov, Alexander G. Medvedev, and Aleksander Filarowski. 2022. "Comparison of Proton Acceptor and Proton Donor Properties of H2O and H2O2 in Organic Crystals of Drug-like Compounds: Peroxosolvates vs. Crystallohydrates" Molecules 27, no. 3: 717. https://doi.org/10.3390/molecules27030717
APA StyleVener, M. V., Churakov, A. V., Voronin, A. P., Parashchuk, O. D., Artobolevskii, S. V., Alatortsev, O. A., Makhrov, D. E., Medvedev, A. G., & Filarowski, A. (2022). Comparison of Proton Acceptor and Proton Donor Properties of H2O and H2O2 in Organic Crystals of Drug-like Compounds: Peroxosolvates vs. Crystallohydrates. Molecules, 27(3), 717. https://doi.org/10.3390/molecules27030717









