Development of Azo Dye Immobilized Sulfonated Poly (Glycidyl Methacrylate) Polymer Composite as Novel Adsorbents for Water Treatment Applications: Methylene Blue Immobilization Isotherm, Kinetic, Thermodynamic, and Simulations Studies
Abstract
1. Introduction
2. Results and Discussion
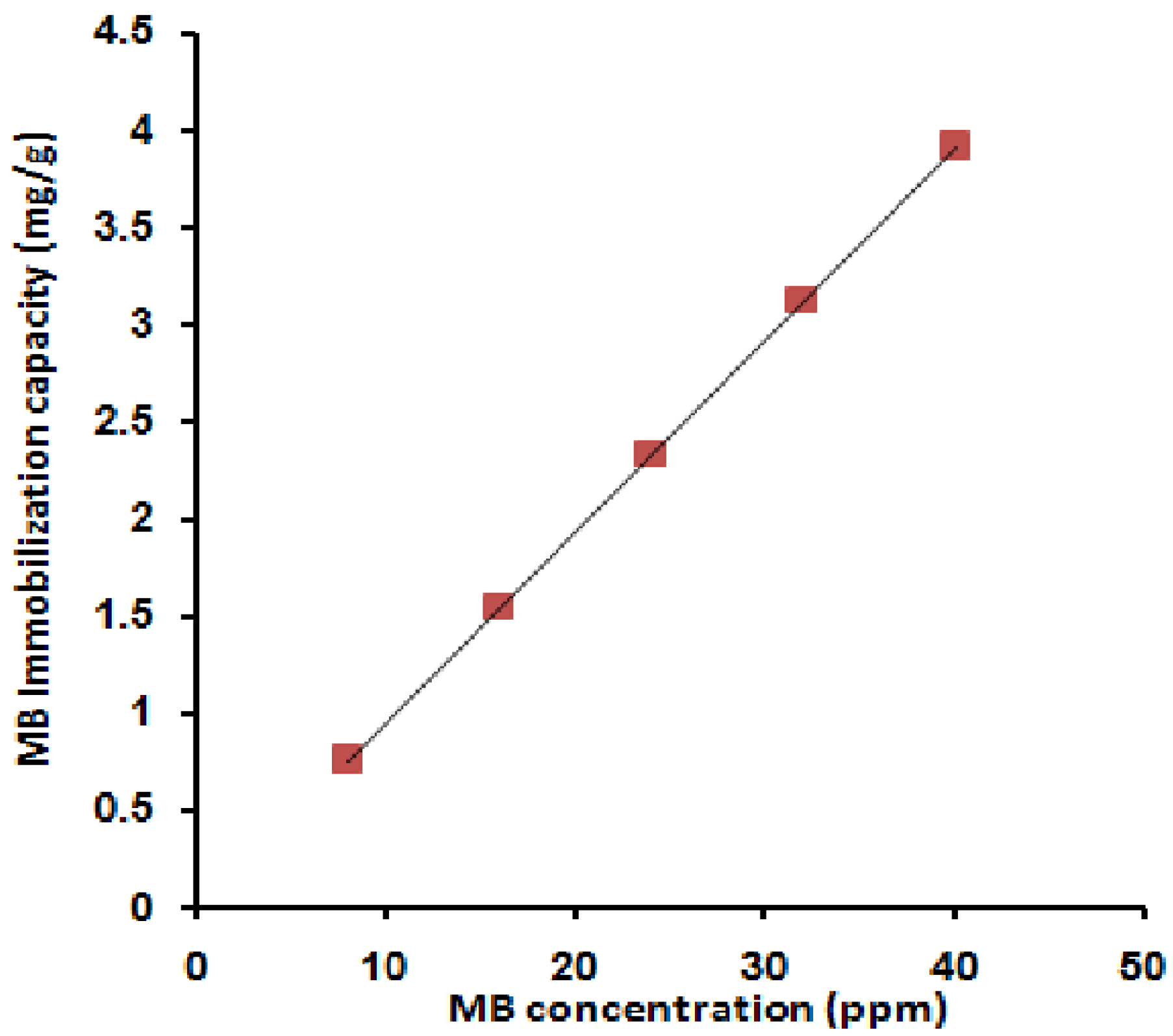
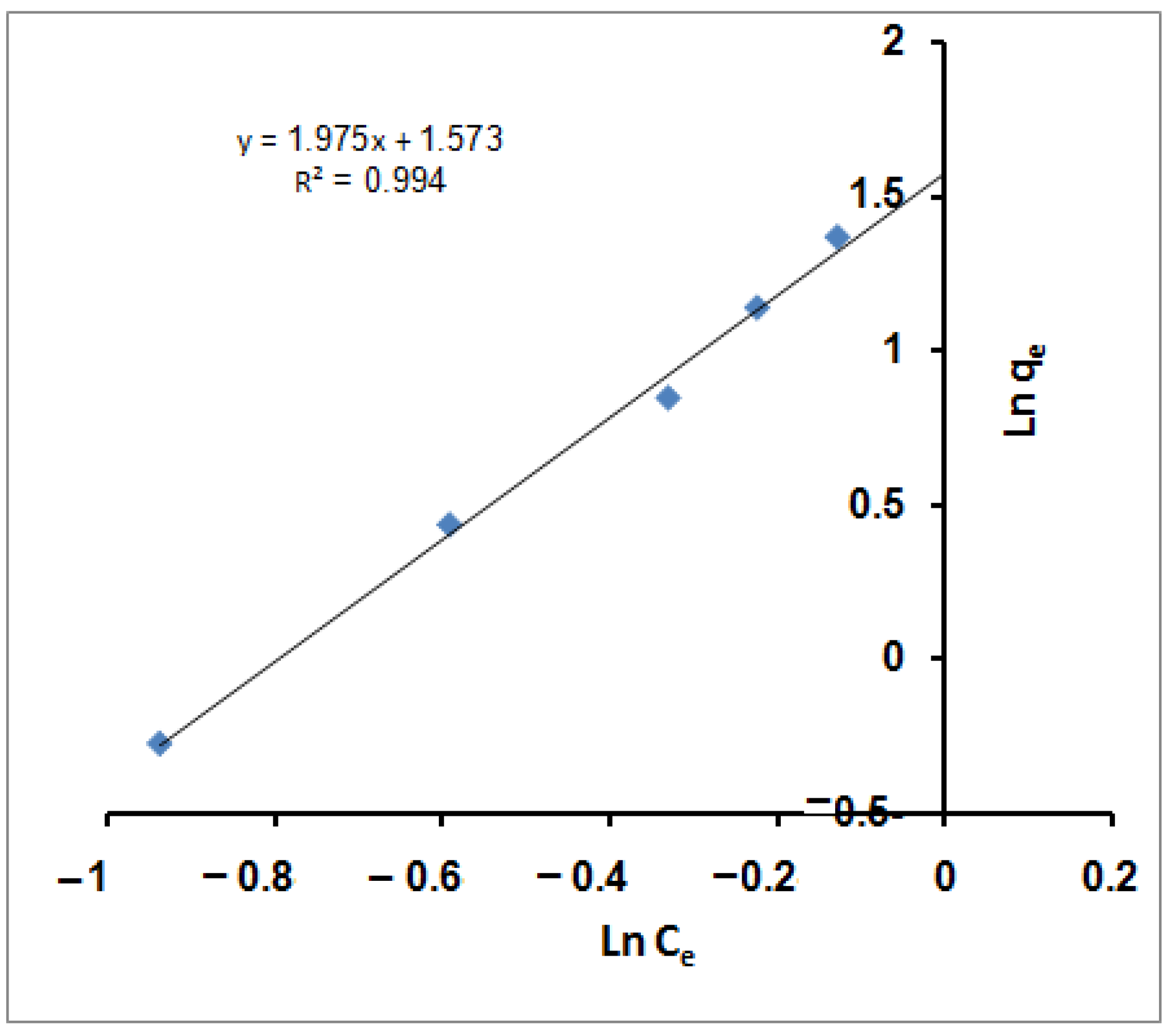
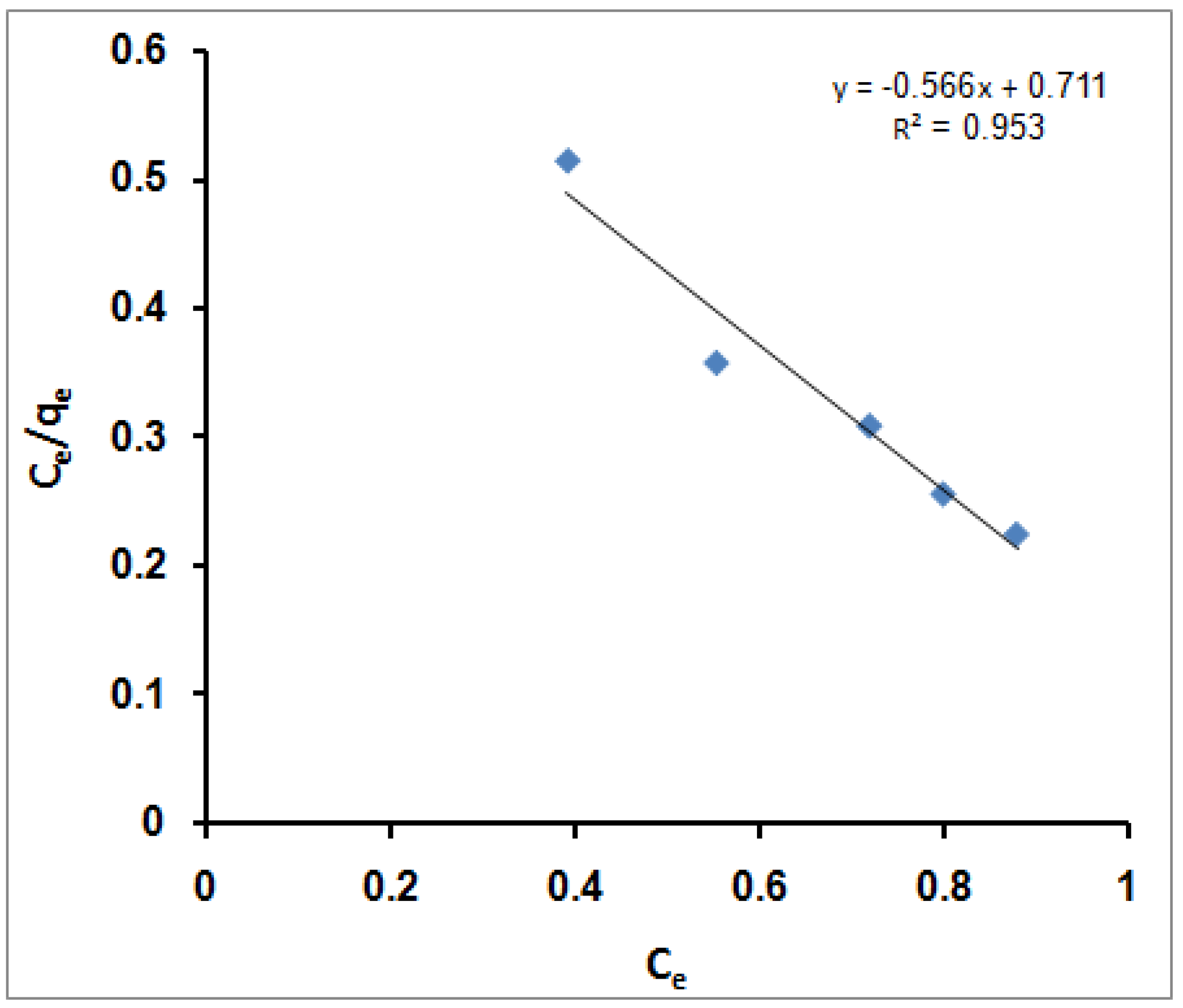
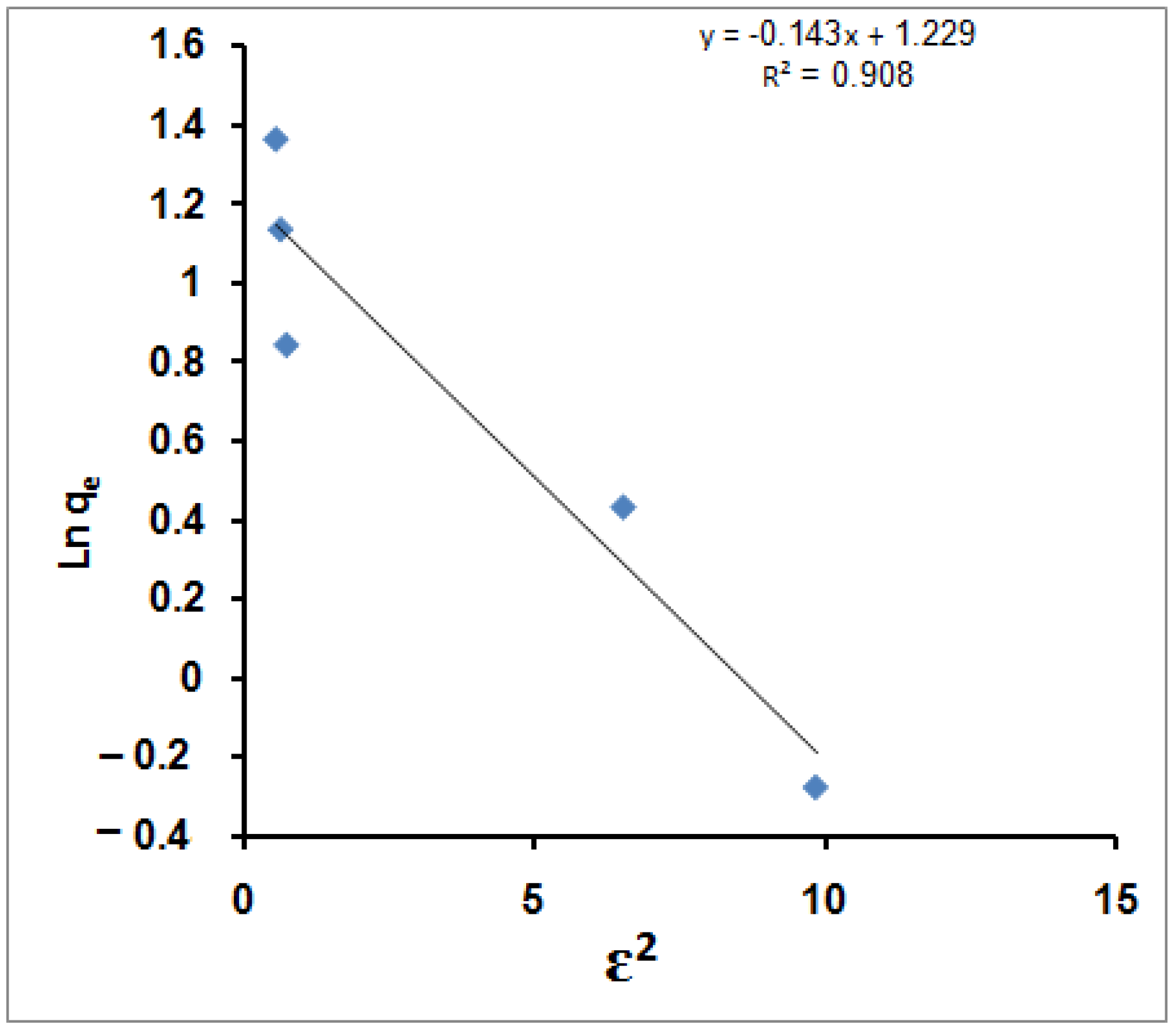
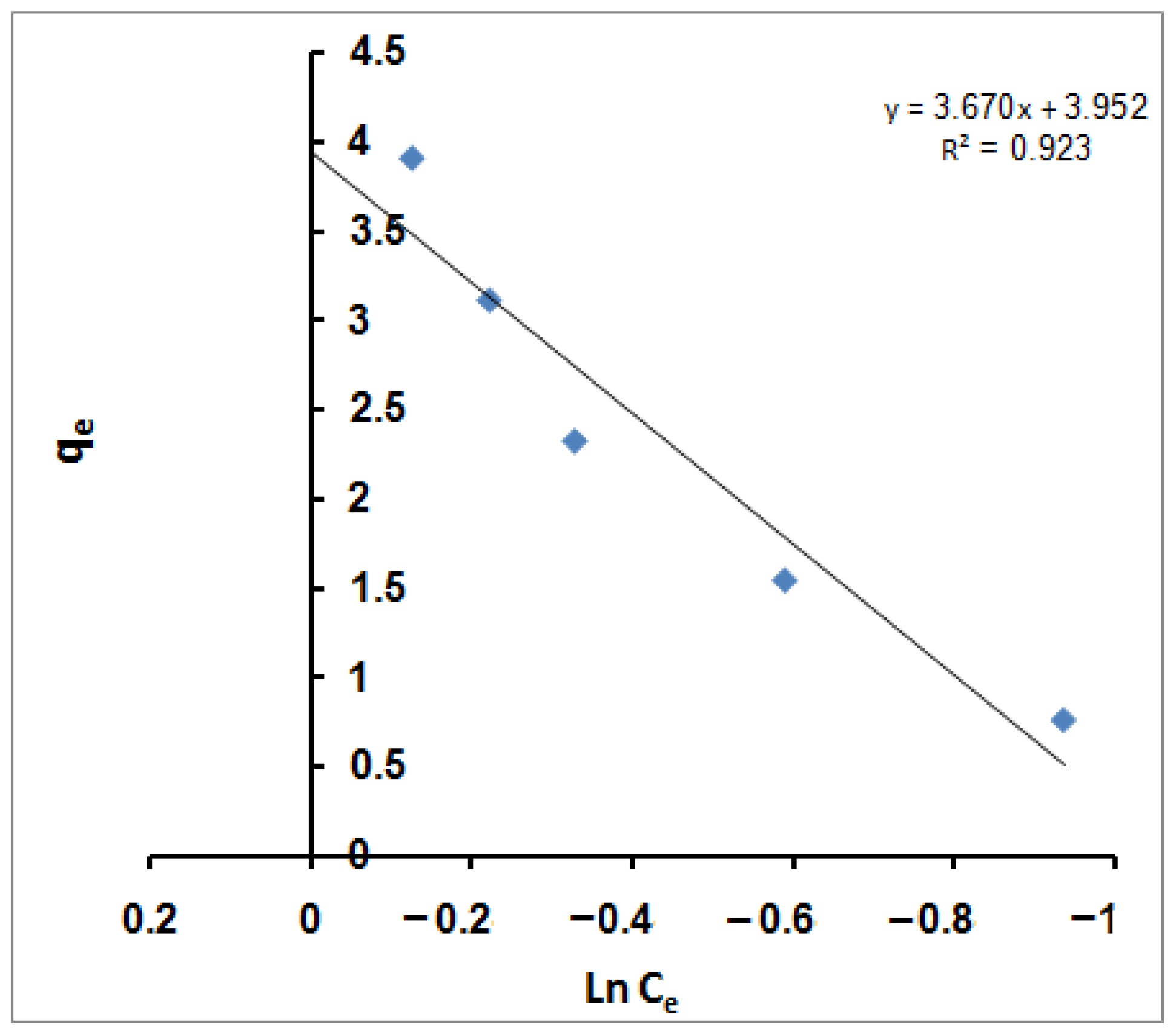
2.1. Methylene Blue Concentration and Adsorption Isotherms
2.2. Methylene Blue Immobilization Time and Adsorption Kinetics
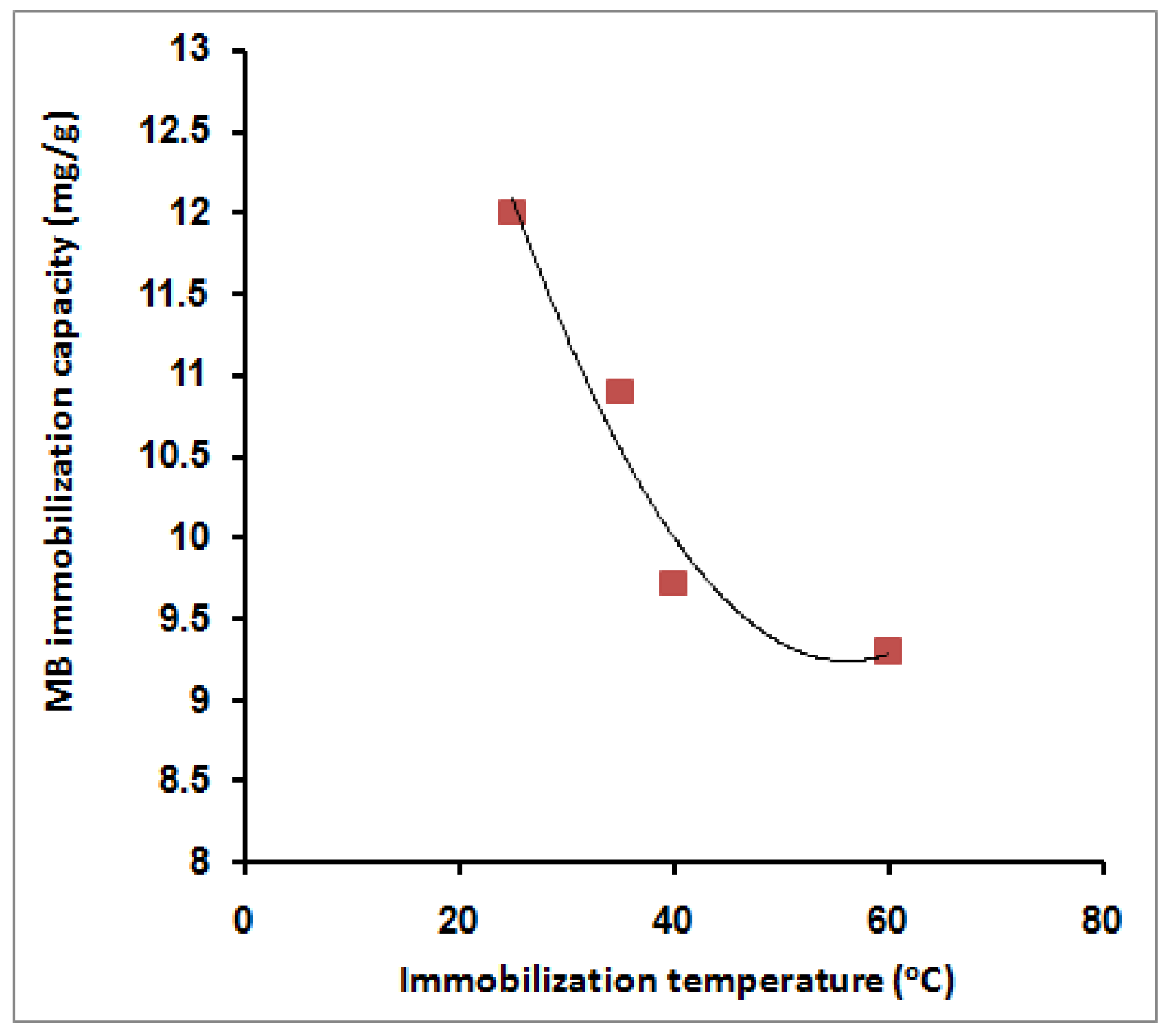
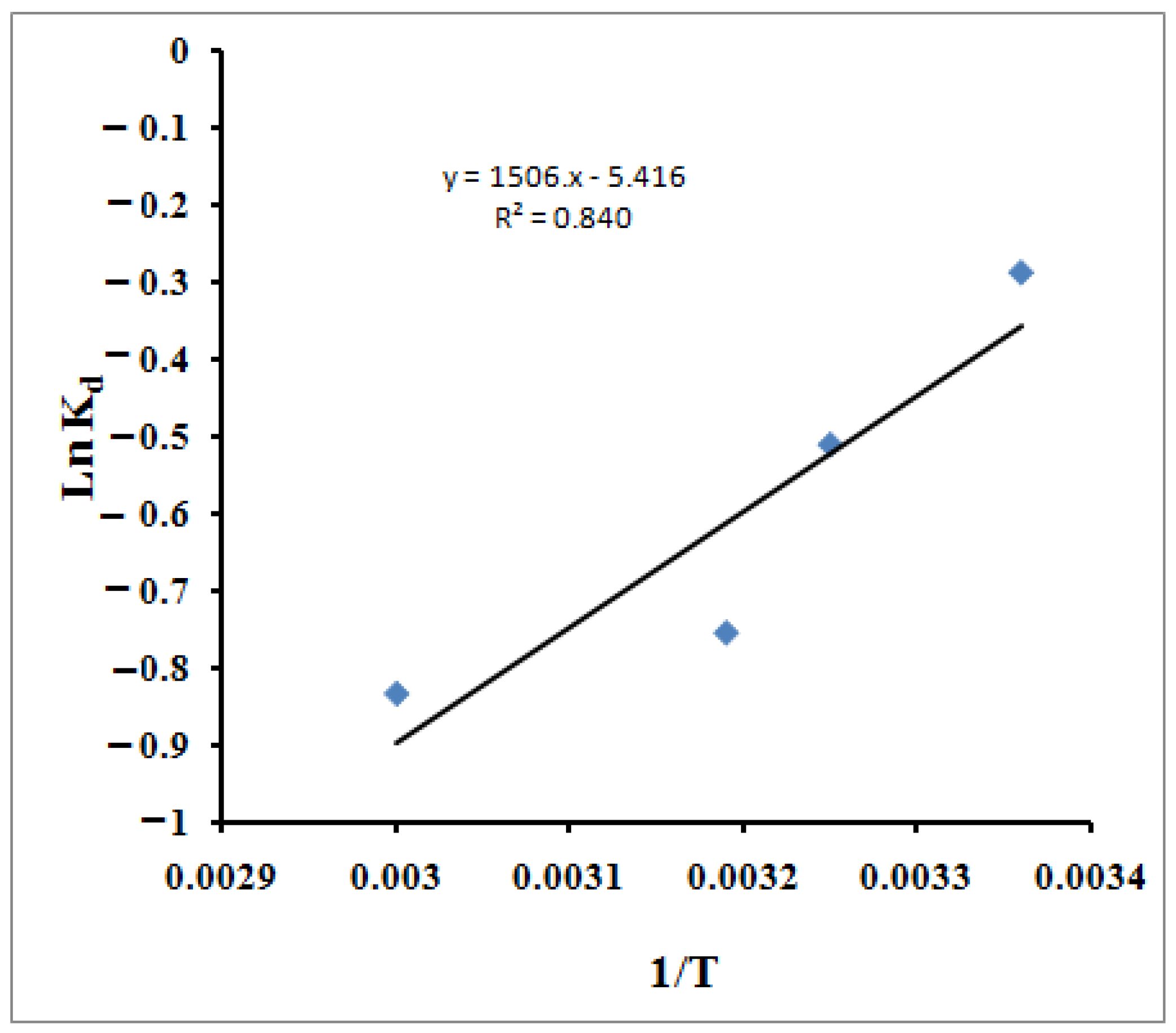
2.3. MB Immobilization Temperature and Adsorption Thermodynamics
2.4. Simulation Mathematical Model
2.5. Metal Ions Removal from Wastewater
3. Materials and Methods
3.1. Materials
3.2. Polymerization Process
3.3. Sulphonation Process
3.4. Preparation of Basic Methylen Blue Solution
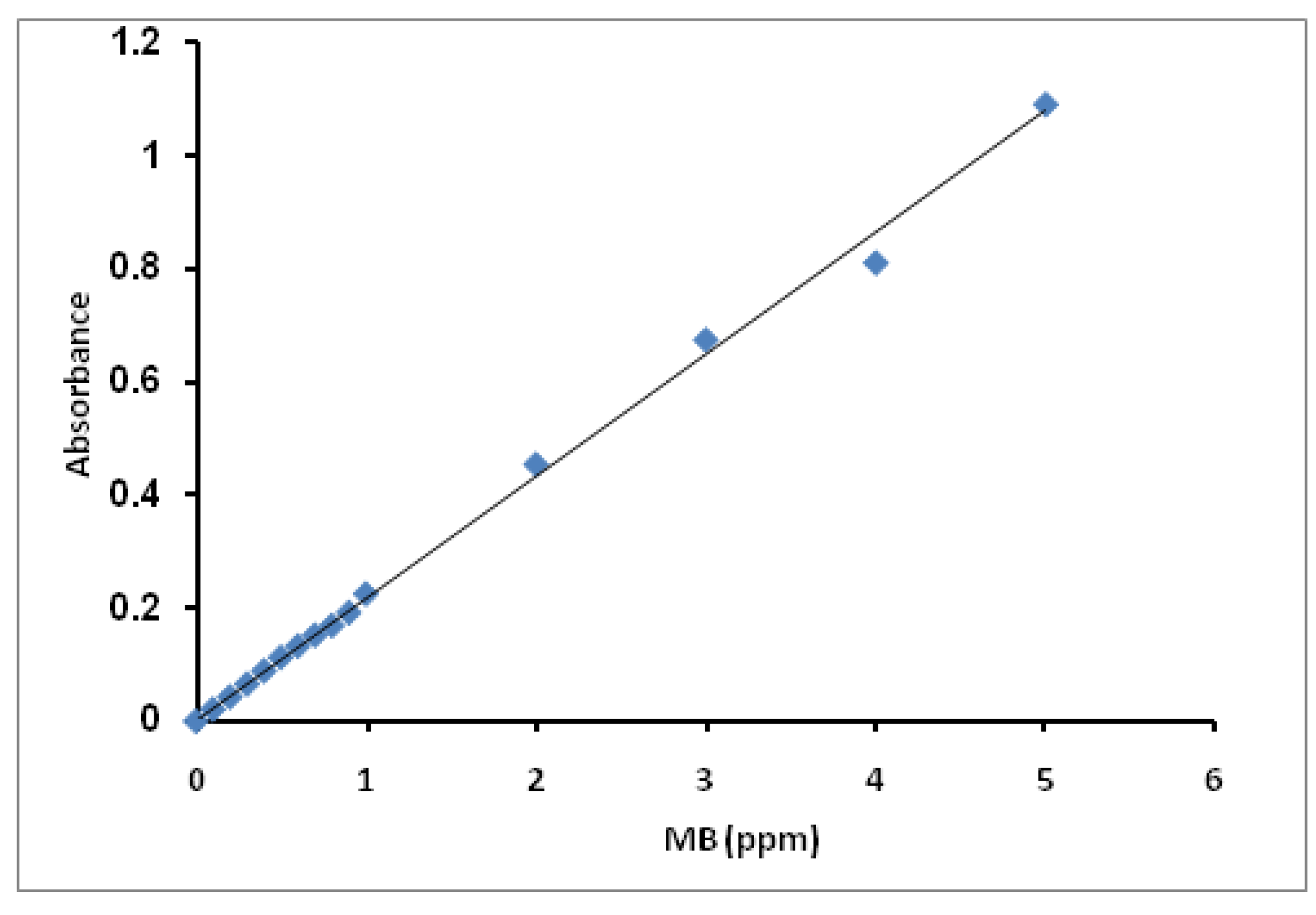
3.5. Standard Curve of MB Concentration
3.6. Methylene Blue–Polymers Composite Formation (Immobilization Process)
3.7. Isotherm, Kinetic, and Thermodynamic Studies
3.8. Mathematical Model of the MB Immobilization Process [95]
3.9. Chromium (VI) and Manganese (VII) Ion Removal [70,71]
4. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Gómez, V.; Larrechi, M.; Callao, M. Kinetic and adsorption study of acid dye removal using activated carbon. Chemosphere 2007, 69, 1151–1158. [Google Scholar] [CrossRef] [PubMed]
- Gupta, V.K.; Kumar, R.; Nayak, A.; Saleh, T.A.; Barakat, M.A. Adsorptive removal of dyes from aqueous solution onto carbon nanotubes: A review. Adv. Colloid Interface Sci. 2013, 193–194, 24–34. [Google Scholar] [CrossRef] [PubMed]
- Ayad, M.M.; Abo El-Nasr, A. Adsorption of cationic dye (methylene blue) from water using polyaniline nano-tubes base. J. Phys. Chem. C 2010, 114, 14377–14383. [Google Scholar] [CrossRef]
- Wong, Y.C.; Szeto, Y.S.; Cheung, W.H.; McKay, G. Equilibrium Studies for Acid Dye Adsorption onto Chitosan. Langmuir 2003, 19, 7888–7894. [Google Scholar] [CrossRef]
- Baybars, A.F.; Cengiz, Q.; Mustafa, K. Cationic dye (methylene blue) removal from aqueous solution by montmorillonite. Bull. Korean Chem. Soc. 2012, 33, 3184–3190. [Google Scholar]
- Yagub, M.T.; Sen, T.K.; Ang, H.M. Equilibrium, Kinetics, and Thermodynamics of Methylene Blue Adsorption by Pine Tree Leaves. Water Air Soil Pollut. 2012, 223, 5267–5282. [Google Scholar] [CrossRef]
- Sen, T.K.; Afroze, S.; Ang, H.M. Equilibrium, kinetics and mechanism of removal of methylene blue from aqueous solution by adsorption onto pine cone biomass of Pinus radiate. Water Air Soil Pollut. 2011, 218, 499–515. [Google Scholar] [CrossRef]
- Mohammad, M.; Maitra, S.; Ahmad, N.; Bustam, A.; Sen, T.; Dutta, B.K. Metal ion removal from aqueous solution using physic seed hull. J. Hazard. Mater. 2010, 179, 363–372. [Google Scholar] [CrossRef]
- Abd EI-Latif, M.M.; Ibrahim, A.M.; EI-Kady, M.F. Adsorption equilibrium, kinetics and thermodynamics of methylene blue from aqueous solutions using biopolymer oak sawdust composite. J. Am. Sci. 2010, 6, 267–283. [Google Scholar]
- Yao, Z.; Wang, L.; Qi, J. Biosorption of Methylene Blue from Aqueous Solution Using a Bioenergy Forest Waste: Xanthoceras sorbifoliaSeed Coat. Clean. Soil Air Water 2009, 37, 642–648. [Google Scholar] [CrossRef]
- Yagub, M.T.; Sen, T.K.; Afroze, S.; Ang, H.M. Dye and its removal from aqueous solution by adsorption: A review. Adv. Colloid Interface Sci. 2014, 209, 172–184. [Google Scholar] [CrossRef] [PubMed]
- Salleh, M.A.M.; Mahmoud, D.K.; Karim, W.A.W.A.; Idris, A. Cationic and anionic dye adsorption by agricultural solid wastes: A comprehensive review. Desalination 2011, 280, 1–13. [Google Scholar] [CrossRef]
- Srinivasan, A.; Viraraghavan, T. Decolorization of dye wastewaters by biosorbents: A review. J. Environ. Manag. 2010, 91, 1915–1929. [Google Scholar] [CrossRef] [PubMed]
- Leszczynska, M.; Hubicki, Z. Application of weakly and strongly basic anion exchangers for the removal of brilliant yellow from aqueous solutions. Desalin. Water Treat. 2009, 2, 156–161. [Google Scholar] [CrossRef]
- Purkait, M.; Maiti, A.; DasGupta, S.; De, S. Removal of congo red using activated carbon and its regeneration. J. Hazard. Mater. 2007, 145, 287–295. [Google Scholar] [CrossRef]
- Hernández-Montoya, V.; Pérez-Cruz, M.; Mendoza-Castillo, D.; Moreno-Virgen, M.; Bonilla-Petriciolet, A. Competitive adsorption of dyes and heavy metals on zeolitic structures. J. Environ. Manag. 2013, 116, 213–221. [Google Scholar] [CrossRef]
- Errais, E.; Duplay, J.; Elhabiri, M.; Khodja, M.; Ocampo, R.; Baltenweck-Guyot, R.; Darragi, F. Anionic RR120 dye adsorption onto raw clay: Surface properties and adsorption mechanism. Colloids Surf. A Physicochem. Eng. Asp. 2012, 403, 69–78. [Google Scholar] [CrossRef]
- Ofomaja, A.E. Equilibrium sorption of methylene blue using mansonia wood sawdust as biosorbent. Desalin. Water Treat. 2009, 3, 1–10. [Google Scholar] [CrossRef]
- Reddy, M.S.; Sivaramakrishna, L.; Reddy, A.V. The use of an agricultural waste material, Jujuba seeds for the removal of anionic dye (Congo red) from aqueous medium. J. Hazard. Mater. 2012, 203–204, 118–127. [Google Scholar] [CrossRef]
- Sarioglu, M.; Atay, U.A. Removal of Methylene blue by using biosolid. Global Nest J. 2006, 8, 113–120. [Google Scholar]
- Deniz, F.; Karaman, S. Removal of Basic Red 46 dye from aqueous solution by pine tree leaves. Chem. Eng. J. 2011, 170, 67–74. [Google Scholar] [CrossRef]
- Dawood, S.; Sen, T.K. Removal of anionic dye Congo red from aqueous solution by raw pine and acid-treated pine cone powder as adsorbent: Equilibrium, thermodynamic, kinetics, mechanism and process design. Water Res. 2012, 46, 1933–1946. [Google Scholar] [CrossRef] [PubMed]
- Auta, M.; Hameed, B. Coalesced chitosan activated carbon composite for batch and fixed-bed adsorption of cationic and anionic dyes. Colloids Surf. B Biointerfaces 2013, 105, 199–206. [Google Scholar] [CrossRef] [PubMed]
- Poinern, G.E.J.; Senanayake, G.; Shah, N.; Thi-Le, X.N.; Parkinson, G.M. Adsorption of the aurocyanide, View the MathML source complex on granular activated carbons derived from macadamia nut shells—A preliminary study. Miner. Eng. 2011, 24, 1694–1702. [Google Scholar] [CrossRef]
- Garba, A.; Al-Qalam University Katsina; Tahir, A.; Yusuf, A.K. Adsorption of Methylene Blue Using Activated Carbon Made from Watermelon Rinds. J. Sustain. Mater. Process. Manag. 2021, 1, 38–43. [Google Scholar] [CrossRef]
- Eldin, M.M.; Gouda, M.; Abu-Saied, M.; El-Shazly, Y.M.; Farag, H. Development of grafted cotton fabrics ions exchanger for dye removal applications: Methylene blue model. Desalination Water Treat. 2016, 57, 22049–22060. [Google Scholar] [CrossRef]
- Mohy Eldin, M.S.; Elkady, M.F.; Abdel Rahman, A.M.; Soliman, E.A.; Elzatahry, A.A.; Youssef, M.E.; Eweida, B.Y. Preparation and characterization of imino diacetic acid functionalized alginate beads for removal of contaminates from waste water: I. methylene blue cationic dye model. Desali. Water Treat. 2012, 40, 15–23. [Google Scholar] [CrossRef]
- Mohy, E.M.S.; Aly, K.; Khan, Z.A.; Meky, A.E.; Saleh, T.S.; Elbogamy, A.S. Development of Novel Acid-Base Ions Exchanger for Basic Dye Removal: Phosphoric Acid Doped Pyrazole-g-Polyglycidyl Methacrylate. Desali. Water Treat. 2016, 57, 24047–24055. [Google Scholar] [CrossRef]
- Elkady, M.F.; Mohy Eldin, M.S.; Abu-Saied, M.A.; Abdel Rahman, A.M.; Soliman, E.A.; Elzatahry, A.A.; Youssef, M.E. Novel nano-sulphonated polyglycidyl methacrylate cation exchanger for removal of heavy metals: Optimization of the operational conditions. Desalination 2011, 279, 152–162. [Google Scholar] [CrossRef]
- Mohy Eldin, M.S.; El-Sakka, S.A.; El-Masry, M.M.; Abdel-Gawad, I.I.; Garybe, S.S. Removal of methylene blue dye from aqueous medium by nano-polyacrylonitrile particles. Desalin. Water Treat. 2012, 44, 151–160. [Google Scholar] [CrossRef]
- Mohy Eldin, M.S.; Aggour, Y.A.; El-Aassar, M.R.; Beghet, G.E.; Atta, R.R. Development of nano-crosslinked polyacrylonitrile ions exchanger particles for dyes removal. Desalin. Water Treat. 2016, 57, 4255–4266. [Google Scholar] [CrossRef]
- Eldin, M.M.; Abu-Saied, M.; Tamer, T.; Youssef, M.; Hashem, A.; Sabet, M. Development of polystyrene based nanoparticles ions exchange resin for water purification applications. Desalin. Water Treat. 2016, 57, 14810–14823. [Google Scholar] [CrossRef]
- Aksu, Z. Algae for wastewater treatment. In Biosorption of Heavy Metals by Microalgae in Batch and Continuous Systems; Wong, Y.-S., Tam, N.F.Y., Eds.; Springer: Berlin/Heidelberg, Germany, 1998; pp. 37–53. [Google Scholar]
- Dönmez, G.; Aksu, Z. The effect of copper(II) ions on the growth and bioaccumulation properties of some yeasts. Process Biochem. 1999, 35, 135–142. [Google Scholar] [CrossRef]
- Das, J.; Dangar, T.K.; Panigrahy, M. Bioremediation of Heavy Metals: A Substantive Potential for Clean Earth. J. Sustain. Mater. Process. Manag. 2022, 2, 80–89. [Google Scholar] [CrossRef]
- Ahmaruzzaman Adsorption of phenolic compounds on low-cost adsorbents: A review. Adv. Colloid Interface Sci. 2008, 143, 48–67. [CrossRef] [PubMed]
- Waldemer, R.H.; Tratnyek, P.G. Kinetics of Contaminant Degradation by Permanganate. Environ. Sci. Technol. 2005, 40, 1055–1061. [Google Scholar] [CrossRef]
- Gupta, V.; Kumari, S.; Virvadiya, C. Adsorption Analysis of Mn(VII) from Aqueous Medium by Activated Orange Peels Powder. Int. Res. J. Pure Appl. Chem. 2015, 9, 1–8. [Google Scholar] [CrossRef]
- Zhang, K.; Li, C.; He, J.; Liu, R. Adsorption of permanganate onto activated carbon particles. Hua Xi Yi Ke Da Xue Xue Bao J. West China Univ. Med Sci. Huaxi Yike Daxue Xuebao 1997, 28, 344–346. [Google Scholar]
- Mahmoud, M.E.; Yakout, A.A.; Saad, S.R.; Osman, M.M. Removal of potassium permanganate from water by modified carbonaceous materials. Desalination Water Treat. 2015, 57, 1–11. [Google Scholar] [CrossRef]
- Virvadiya, C.; Kumari, S.; Choudhary, V.; Gupta, V. Combined bio- and chemosorption of Mn(VII) from aqueous solution by PROSOPIS CINERARIA leaf powder. Eur. Chem. Bull 2014, 3, 315–318. [Google Scholar]
- Chaudhary, M. Use of Millet Husk as a Biosorbent for the Removal of chromium and Manganese Ions from the Aqueous Solutions. Int. J. Chem. Environ. Pharm. Res. 2011, 2, 30–33. [Google Scholar]
- Wan Ngah, W.S.; Hanafiah, M.A.K.M. Removal of heavy metal ions from wastewater by chemically modified plant wastes as adsorbents: A review. Bioresour. Technol. 2007, 99, 3935–3948. [Google Scholar] [CrossRef]
- Waranusantigula, P.; Pokethitiyook, P.; Kruatrachue, M.; Upatham, E.S. Kinetics of cbasic dye (methylene blue) biosorption by giant duckweed (Spirodela polyrrhiza). Environ. Pollut. 2003, 125, 385–392. [Google Scholar] [CrossRef]
- Mohan, D.; Pittman, C.U. Activated carbons and low cost adsorbents for remediation of tri- and hexavalent chromium from water. J. Hazard. Mater. 2006, 137, 762–811. [Google Scholar] [CrossRef] [PubMed]
- Varga, M.; Takács, M.; Záray, G.; Varga, I. Comparative study of sorption kinetics and equilibrium of chromium (VI) on charcoals prepared from different low-cost materials. Microchem. J. 2013, 107, 25–30. [Google Scholar] [CrossRef]
- Srinivasan, K. Evaluation of Rice husk carbon for the removal of trace inorganic form water. Ph.D. Thesis, Indian Institute of Technology—Madras I.I.T., Madras, India, 1986. [Google Scholar]
- Iqbal, M.; Saeed, A.; Zafar, S.I. Hybrid biosorbent: An innovative matrix to enhance the biosorption of Cd(II) from aqueous solution. J. Hazard. Mater. 2007, 148, 47–55. [Google Scholar] [CrossRef]
- Malathi, S.; Srinivasan, K.; Gomathi, M. Studies on the removal of Cr (VI) from aqueous solution by activated carbon developed from Cottonseed activated withsulphuric acid. Int. J. Chem. Tech. Res. 2015, 8, 795–802. [Google Scholar]
- Ansari, R. Application of Polyaniline and its Composites for adsorption/Recovery of Chromium (VI) from Aqueous Solutions. Acta Chim. Slov. 2006, 53, 88–94. [Google Scholar]
- Jassal, P.S.; Raut, V.P.; Anand, N. Removal of Chromium (VI) ions from Aqueous solution onto Chitosan and Cross-linked Chitosan Beads. Proc. Indian Natn. Sci. Acad. 2010, 76, 1–6. [Google Scholar]
- Marin, N.M. Natural and Synthetic Polymers Modified with Acid Blue 113 for Removal of Cr3+, Zn2+ and Mn2+. Polymers 2022, 14, 2139. [Google Scholar] [CrossRef]
- Gupta, A.; Jain, R.; Gupta, D.C. Studies on uptake behavior of Hg (II) and Pb(II) by amine modified glycidyl methacrylate-styrene-N, N’-methylene bis-acrylamide ter- polyme. React. Funct. Polym. 2015, 93, 22–29. [Google Scholar] [CrossRef]
- Dou, X.B.; Chai, M.Y.; Zhu, Y.; Yang, W.T.; Xu, F.J. Aminated Poly(glycidyl methacrylate)s for Constructing Efficient Gene Carriers. ACS Appl. Mater. Interfaces 2013, 5, 3212–3218. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Li, F.; Yao, M.; Qiu, T.; Jiang, W.; Fan, L.-J. Atom transfer radical polymerization of glycidyl methacrylate followed by amination on the surface of monodispersed highly crosslinked polymer microspheres and the study of cation adsorption. React. Funct. Polym. 2014, 82, 66–71. [Google Scholar] [CrossRef]
- Younis, S.A.; Ghobashy, M.M.; Samy, M. Development of aminated poly(glycidyl methacrylate) nanosorbent by green gamma radiation for phenol and malathion contaminated wastewater treatment. J. Environ. Chem. Eng. 2017, 5, 2325–2336. [Google Scholar] [CrossRef]
- Aversa, T.M.; da Silva, C.M.F.; da Rocha, P.C.S.; Lucas, E.F. Influence of exchange group of modified glycidyl methacrylate polymer on phenol removal: A study by batch and continuous flow processes. J. Environ. Manag. 2016, 182, 301–307. [Google Scholar] [CrossRef]
- Yu, Y.; Su, J.; Liu, J.; Li, W. Magnetic Poly(glycidyl methacrylate) Microspheres with Grafted Polypyrrole Chains for the High-Capacity Adsorption of Congo Red Dye from Aqueous Solutions. Coatings 2022, 12, 168. [Google Scholar] [CrossRef]
- Chen, W.; Liu, Y.; Liu, C. Preparation and use of magnetic poly(glycidyl methacrylate) resin in drinking water treatment. J. Appl. Polym. Sci. 2013, 130, 106–112. [Google Scholar] [CrossRef]
- Cheng, L.; Wu, J.; Liang, H.; Yuan, Q. Preparation of Poly(glycidyl methacrylate) (PGMA) and Amine Modified PGMA Adsorbents for Purification of Glucosinolates from Cruciferous Plants. Molecules 2020, 25, 3286. [Google Scholar] [CrossRef]
- Benaglia, M.; Alberti, A.; Giorgini, L.; Magnoni, F.; Tozzi, S. Poly(glycidyl methacrylate): A highly versatile polymeric building block for post-polymerization modifications. Polym. Chem. 2013, 4, 124–132. [Google Scholar] [CrossRef]
- Waly, A.I.; Khedr, M.A.; Ali, H.M.; Ahmed, I.M. Application of amino-functionalized cellulose-poly(glycidyl methacrylate) graft copolymer (AM-Cell-g-PGMA)adsorbent for dyes removal from wastewater. Clean. Eng. Technol. 2022, 6, 100374. [Google Scholar] [CrossRef]
- Mohy Eldin, M.S.; Elkady, M.F.; Abu-Saied, M.A.; Abdel Rahman, A.M.; Soliman, E.A.; Elzatahry, A.A.; Youssef, M.E. Removal of cadmium ions from synthetic aqueous solutions using a novel nano-sulphonated poly glycidylmethacrylate cation exchanger: Kinetic and equilibrium studies. J. App. Poly Sci. 2010, 118, 3111–3122. [Google Scholar] [CrossRef]
- Abu-Saied, M.; Fontana Nova, E.; Drioli, E.; Mohy Eldin, M. Sulphonated poly (glycidyl methacrylate) grafted cellophane membranes: Novel application in polyelectrolyte membrane fuel cell (PEMFC). J. Polym. Res. 2013, 20, 1–13. [Google Scholar] [CrossRef]
- Mohy Eldin, M.S.; Nassr, A.A.; Kashyout, A.B.E.; Hassan, A. Novel Sulphonated Poly (Glycidyl Methacrylate) Grafted Nafion Membranes for Fuel Cell Applications. Polym. Bull. 2017, 74, 5195–5220. [Google Scholar] [CrossRef]
- El-Aassar, M.R.; Tamer, T.M.; El-Sayed, M.Y.; Omer, A.M.; Althobaiti, I.O.; Youssef, M.E.; Alolaimi, R.F.; El-Agammy, E.F.; Alruwaili, M.S.; Mohy-Eldin, M.S. Development of Azo Dye Immobilized Poly (Glycidyl Methacrylate-Co-Methyl Methacrylate) Polymers Composites as Novel Adsorbents for Water Treatment Applications: Methylene Blue-Polymers Composites. Polymers 2022, 14, 4672. [Google Scholar] [CrossRef]
- Bulut, Y.; Aydin, A. A kinetics and thermodynamics study of methylene blue adsorption on wheat shells. Desalination 2006, 194, 259–267. [Google Scholar] [CrossRef]
- Gode, F.; Pehlivan, E. A comparative study of two chelating ion-exchange resins for the removal of chromium(III) from aqueous solution. J. Hazard. Mater. 2003, 100, 231–243. [Google Scholar] [CrossRef] [PubMed]
- Gode, G.; Pehlivan, E. Removal of Cr (III) ions by Turkish brown coals. Fuel Process Technol. 2005, 86, 875–884. [Google Scholar] [CrossRef]
- Ho, Y.-S. Effect of pH on lead removal from water using tree fern as the sorbent. Bioresour. Technol. 2005, 96, 1292–1296. [Google Scholar] [CrossRef]
- Dubinin, M.M.; Zaverina, E.D.; Radushkevich, L.V. Sorption, and structure of activated carbons, I. investigation of organic vapor removal. Zh Fiz Khim 1947, 21, 1351–1362. [Google Scholar]
- Unlu, N.; Ersoz, M. Removal characteristics of heavy metal ions onto a low-cost biopolymeric sorbents from aqueous solution. J. Hazard. Mater. 2006, 136, 272–280. [Google Scholar] [CrossRef]
- Mohammad, A.; Rifaqat, A.K.R.; Rais, A.; Jameel, A. Removal studies on Citrus reticulata (fruit peel of orange): Removal and recovery of Ni(II) from electroplating wastewater. J. Hazard. Mater. 2000, 79, 117–131. [Google Scholar]
- Ho, Y.S.; Porter, J.F.; McKay, G. Equilibrium Isotherm Studies for the Sorption of Divalent Metal Ions onto Peat: Copper, Nickel and Lead Single Component Systems. Water Air Soil Pollut. 2002, 141, 1–33. [Google Scholar] [CrossRef]
- Tan IA, W.; Ahmad, A.L.; Hameed, B.H. Removal of basic dye using activated carbon prepared from oil palm shell: Batch and fixed bed studies. Desalination 2008, 225, 13–28. [Google Scholar] [CrossRef]
- Şeker, A.; Shahwan, T.; Eroğlu, A.E.; Yılmaz, S.; Demirel, Z.; Dalay, M.C. Equilibrium, thermodynamic and kinetic studies for the biosorption of aqueous lead(II), cadmium(II) and nickel(II) ions on Spirulina platensis. J. Hazard. Mater. 2008, 154, 973–980. [Google Scholar] [CrossRef]
- Helfferich, F. Ion Exchange; McGraw-Hill: New York, NY, USA, 1962. [Google Scholar]
- Malik, U.; Hasany, S.; Subhani, M. Sorptive potential of sunflower stem for Cr(III) ions from aqueous solutions and its kinetic and thermodynamic profile. Talanta 2005, 66, 166–173. [Google Scholar] [CrossRef]
- Smith, J.M. Chemical Engineering Kinetics; McGraw-Hill: New York, NY, USA, 1981. [Google Scholar]
- Anari-Anaraki, M.; Nezamzadeh-Ejhieh, A. Modification of clinoptilolite nanoparticles by a cationic surfactant and dithizone for removal of Pb(II) from aqueous solution. J. ColloidInterf. Sci. 2015, 440, 272–281. [Google Scholar] [CrossRef]
- Heidari-Chaleshtori, M.; Nezamzadeh-Ejhieh, A. Modified clinoptilolite nano-particles with Aspartic acid for removal of Cu(II) from aqueous solutions: Isotherms and kinetic aspects. New J. Chem. 2015, 39, 9396–9406. [Google Scholar] [CrossRef]
- Hameeda, B.H.; China, L.H.; Rengarajb, S. Removal of 4-chlorophenol onto activated carbon prepared from rattan sawdust. Desalination 2008, 225, 185–198. [Google Scholar] [CrossRef]
- Temkin, M.I.; Pyzhev, V. Kinetics of ammonia synthesis on promoted iron catalysts. Acta Physicochim. 1940, 12, 327–356. [Google Scholar]
- Langergren, S.; Svenska, B.K. Zur theorie der sogenannten adsorption geloester. Stoffe Veternskapsakad Handl. 1898, 24, 1–39. [Google Scholar]
- Ho, Y.S.; Mckay, G. The kinetics of sorption of basic dyes from aqueous solution by sphagnum moss peat. Can. J. Chem. Eng. 1998, 76, 822–827. [Google Scholar] [CrossRef]
- Ozacar, M.; Sengil, I.A. A kinetic study of metal complex dye sorption onto pinedust. Proc. Biochem. 2005, 40, 565–572. [Google Scholar] [CrossRef]
- Ho, Y.S.; McKay, G. Pseudo-second order model for sorption processes. Proc. Biochem. 1999, 34, 451–465. [Google Scholar] [CrossRef]
- Tseng, R.L. Mesopore control of high surface area NaOH-activated carbon. J. Coll. Interf. Sci. 2006, 303, 494–502. [Google Scholar] [CrossRef]
- Khan, T.A.; Dahiya, S.; Ali, I. Use of kaolinite as adsorbent: Equilibrium, dynamics and thermodynamic studies on the adsorption of Rhodamine B from aqueous solution. Appl. Clay Sci. 2012, 69, 58–66. [Google Scholar] [CrossRef]
- Zhao, G.; Li, J.; Wang, X. Kinetic and thermodynamic study of 1-naphthol adsorption from aqueous solution to sulfonated graphene nanosheets. Chem. Eng. J. 2011, 173, 185–190. [Google Scholar] [CrossRef]
- Alkan, M.; Demirbaş, Ö.; Çelikçapa, S.; Doğan, M. Sorption of acid red 57 from aqueous solution onto sepiolite. J. Hazard. Mater. 2004, 116, 135–145. [Google Scholar] [CrossRef]
- Kifuani, K.M.; Mayeko AK, K.; Lopaka, B.I.; Bokolombe, P.N.; Ondongo, T.M.; Bakambo, G.E.; Lunguya, J.M. Kinetic and thermodynamic studies adsorption of Methylene Blue (MB) in aqueous solution on a bioadsorbent from Cu-cumeropsis mannii Naudin waste seeds. Int. J. Biol. Chem. Sci. 2018, 12, 2412–2423. [Google Scholar] [CrossRef]
- Wu, J.; Xia, A.; Chen, C.; Feng, L.; Su, X.; Wang, X. Adsorption Thermodynamics and Dynamics of Three Typical Dyes onto Bio-adsorbent Spent Substrate of Pleurotus eryngii. Int. J. Environ. Res. Public Health 2019, 16, 679. [Google Scholar] [CrossRef]
- Yagub, M.T.; Sen, T.K.; Ang, M. Removal of cationic dye methylene blue (MB) from aqueous solution by ground raw and base modified pine cone powder. Environ. Earth Sci. 2014, 71, 1507–1519. [Google Scholar] [CrossRef]
- Eldin, M.S.M.; Gouda, M.; Youssef, M.E.; El-Shazly, Y.M.S.; Farag, H.A. Removal of methylene blue by amidoxime polyacrylonitrile-grafted cotton fabrics: Kinetic, equilibrium, and simulation studies. Fibers Polym. 2016, 17, 1884–1897. [Google Scholar] [CrossRef]






| Co | RL |
|---|---|
| 5 | 0.137 |
| 10 | 0.0737 |
| 15 | 0.0504 |
| 20 | 0.0382 |
| 30 | 0.0258 |
| Adsorbent | Pseudo-First-Order | Pseudo-Second-Order | Elovich | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| qe,exp, (mg/g) | qe,cal, (mg/g) | K1 (min−1) | R2 | qe,cal (mg/g) | K2 (g mg−1min−1) | R2 | β (g/mg) | α (mg/g min) | R2 | |
| SPGMA | 3.94 | 0.277 | 0.048 | 0.493 | 4.00 | 0.1422 | 0.999 | 0.137 | 3.449 | 0.829 |
| 1/T | ΔG (kJ/mol) | ΔH (kJ/mol) | ΔS (J·mol−1·K−1) |
|---|---|---|---|
| 0.00336 | 0.713 | −12.52 | −45.03 |
| 0.00325 | 1.309 | ||
| 0.00319 | 1.965 | ||
| 0.00300 | 2.309 |
| Metal Ions Concentration (ppm) | Metal Ions Removal Percentage (%) | |
|---|---|---|
| Cr6+ | Mn7+ | |
| 2 | 16.5 | 11 |
| 4 | 20 | 22 |
| 6 | 25 | 38 |
| 8 | 36 | 55 |
| MB (ppm) | qe (mg/g) | Ce (mg/L) |
|---|---|---|
| 8 | 0.761 | 0.392 |
| 16 | 1.545 | 0.5536 |
| 24 | 2.328 | 0.72 |
| 32 | 3.118 | 0.80 |
| 40 | 3.916 | 0.88 |
| Time (t) | lnt | Capacity (qt) | t/qt | ln(qe − qt) |
|---|---|---|---|---|
| 5 | 1.61 | 3.65 | 1.37 | −1.24 |
| 10 | 2.3 | 3.83 | 2.61 | −2.21 |
| 15 | 2.71 | 3.8 | 3.95 | −1.97 |
| 20 | 3 | 3.82 | 5.24 | −2.12 |
| 30 | 3.4 | 3.94 | 7.61 |
| Temperature (°C)/(K) | qe | Ce | Kd | ln Kd |
|---|---|---|---|---|
| 25/298 | 12 | 16 | 0.75 | −0.288 |
| 35/308 | 10.9 | 18.2 | 0.6 | −0.511 |
| 40/313 | 9.71 | 20.576 | 0.47 | −0.755 |
| 60/333 | 9.3 | 21.412 | 0.43 | −0.834 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
El-Aassar, M.R.; Tamer, T.M.; El-Sayed, M.Y.; Omer, A.M.; Althobaiti, I.O.; Youssef, M.E.; Alolaimi, R.F.; El-Agammy, E.F.; Alruwaili, M.S.; Rabhy, O.O.; et al. Development of Azo Dye Immobilized Sulfonated Poly (Glycidyl Methacrylate) Polymer Composite as Novel Adsorbents for Water Treatment Applications: Methylene Blue Immobilization Isotherm, Kinetic, Thermodynamic, and Simulations Studies. Molecules 2022, 27, 8418. https://doi.org/10.3390/molecules27238418
El-Aassar MR, Tamer TM, El-Sayed MY, Omer AM, Althobaiti IO, Youssef ME, Alolaimi RF, El-Agammy EF, Alruwaili MS, Rabhy OO, et al. Development of Azo Dye Immobilized Sulfonated Poly (Glycidyl Methacrylate) Polymer Composite as Novel Adsorbents for Water Treatment Applications: Methylene Blue Immobilization Isotherm, Kinetic, Thermodynamic, and Simulations Studies. Molecules. 2022; 27(23):8418. https://doi.org/10.3390/molecules27238418
Chicago/Turabian StyleEl-Aassar, Mohamed R., Tamer M. Tamer, Mohamed Y. El-Sayed, Ahmed M. Omer, Ibrahim O. Althobaiti, Mohamed E. Youssef, Rawan F. Alolaimi, Emam F. El-Agammy, Manar S. Alruwaili, Omar O. Rabhy, and et al. 2022. "Development of Azo Dye Immobilized Sulfonated Poly (Glycidyl Methacrylate) Polymer Composite as Novel Adsorbents for Water Treatment Applications: Methylene Blue Immobilization Isotherm, Kinetic, Thermodynamic, and Simulations Studies" Molecules 27, no. 23: 8418. https://doi.org/10.3390/molecules27238418
APA StyleEl-Aassar, M. R., Tamer, T. M., El-Sayed, M. Y., Omer, A. M., Althobaiti, I. O., Youssef, M. E., Alolaimi, R. F., El-Agammy, E. F., Alruwaili, M. S., Rabhy, O. O., & Mohy-Eldin, M. S. (2022). Development of Azo Dye Immobilized Sulfonated Poly (Glycidyl Methacrylate) Polymer Composite as Novel Adsorbents for Water Treatment Applications: Methylene Blue Immobilization Isotherm, Kinetic, Thermodynamic, and Simulations Studies. Molecules, 27(23), 8418. https://doi.org/10.3390/molecules27238418










