A Path Integral Molecular Dynamics Simulation of a Harpoon-Type Redox Reaction in a Helium Nanodroplet
Abstract
1. Introduction
2. Potential Energy Surfaces and Hopping Neutral/Ionic Probabilities
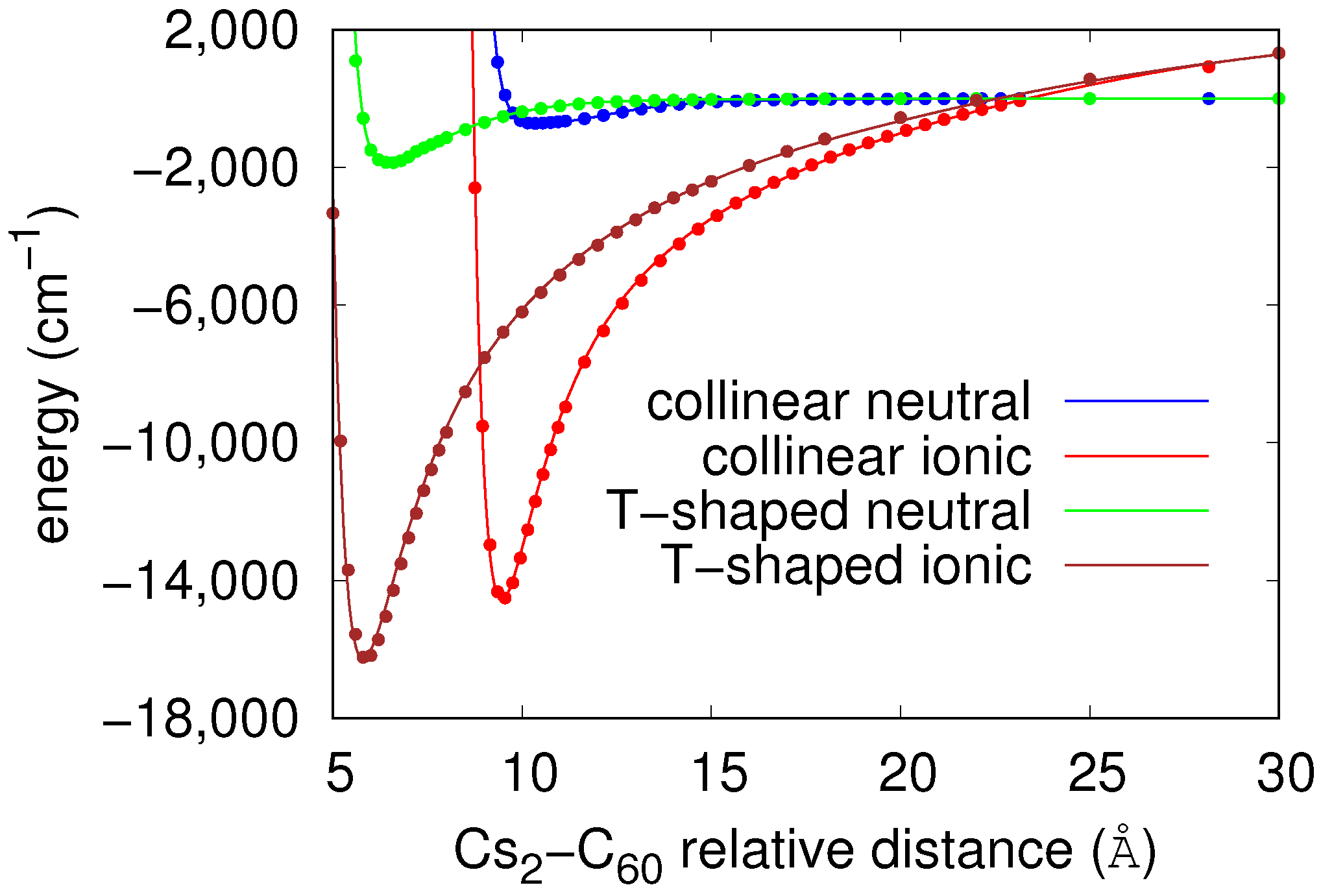
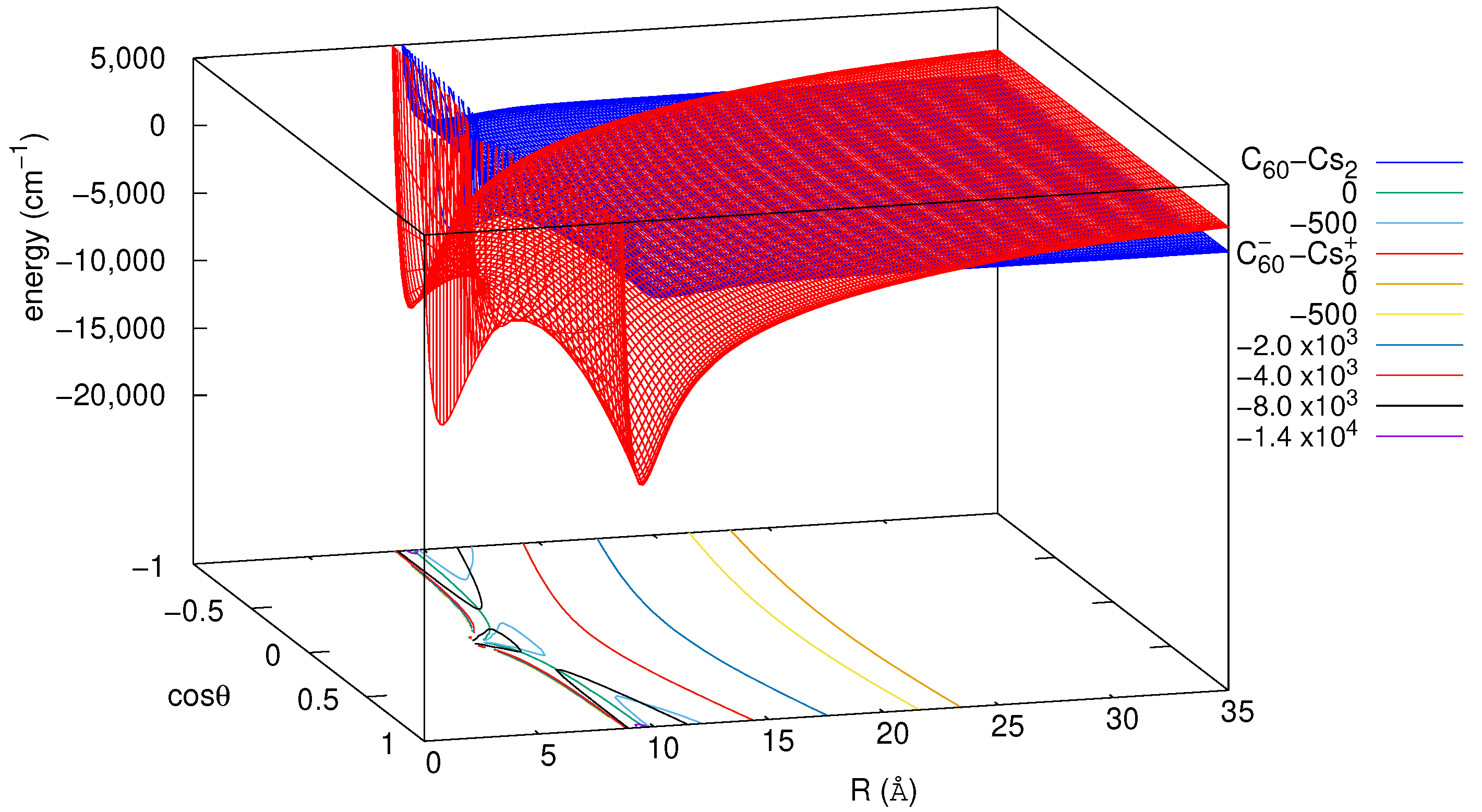
2.1. Analytic Potential Energy Surfaces
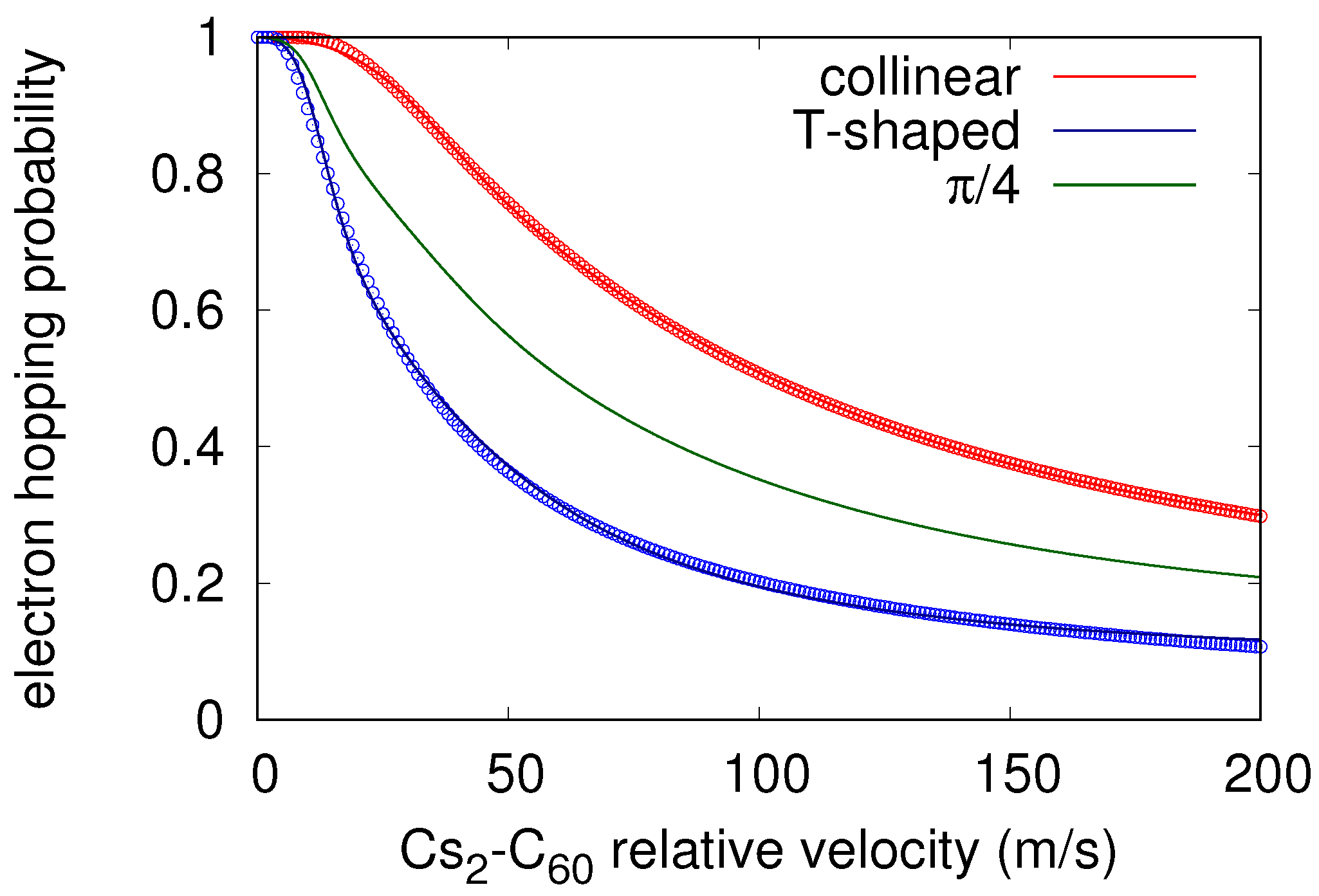
2.2. Hopping Neutral/Ionic Probabilities
3. Computational Method and Details
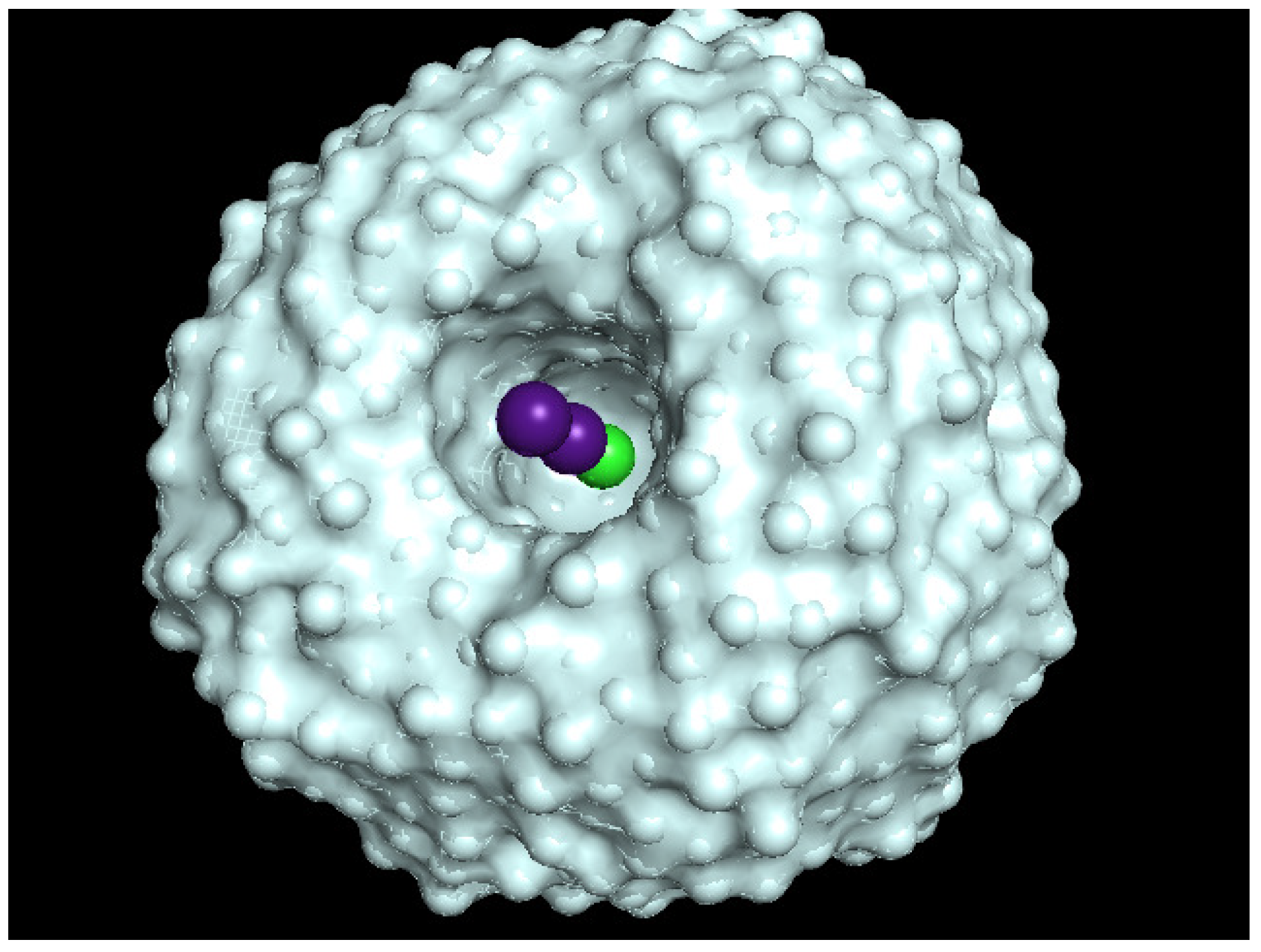
3.1. Initial Arrangement
3.2. PIMD Simulation
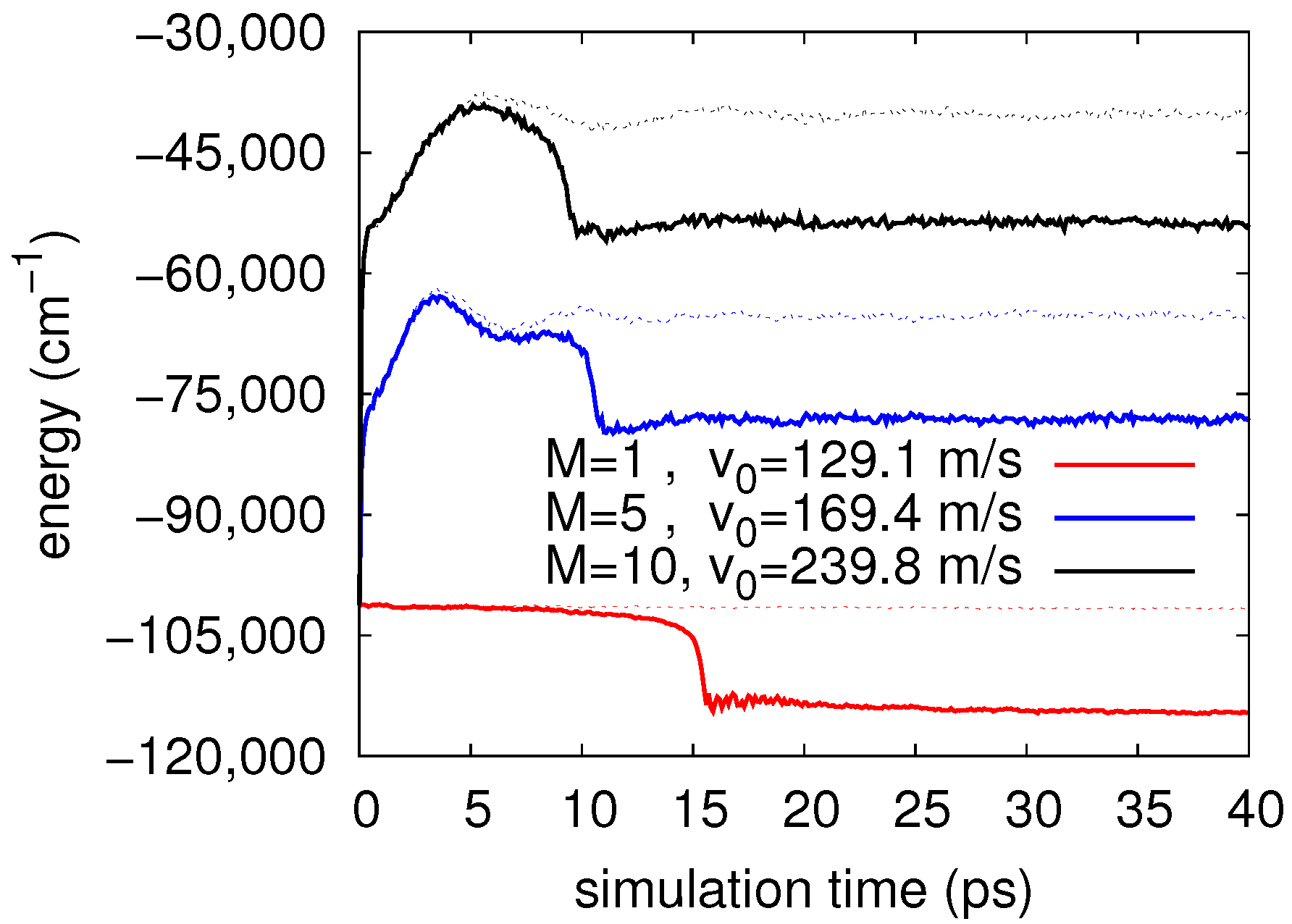
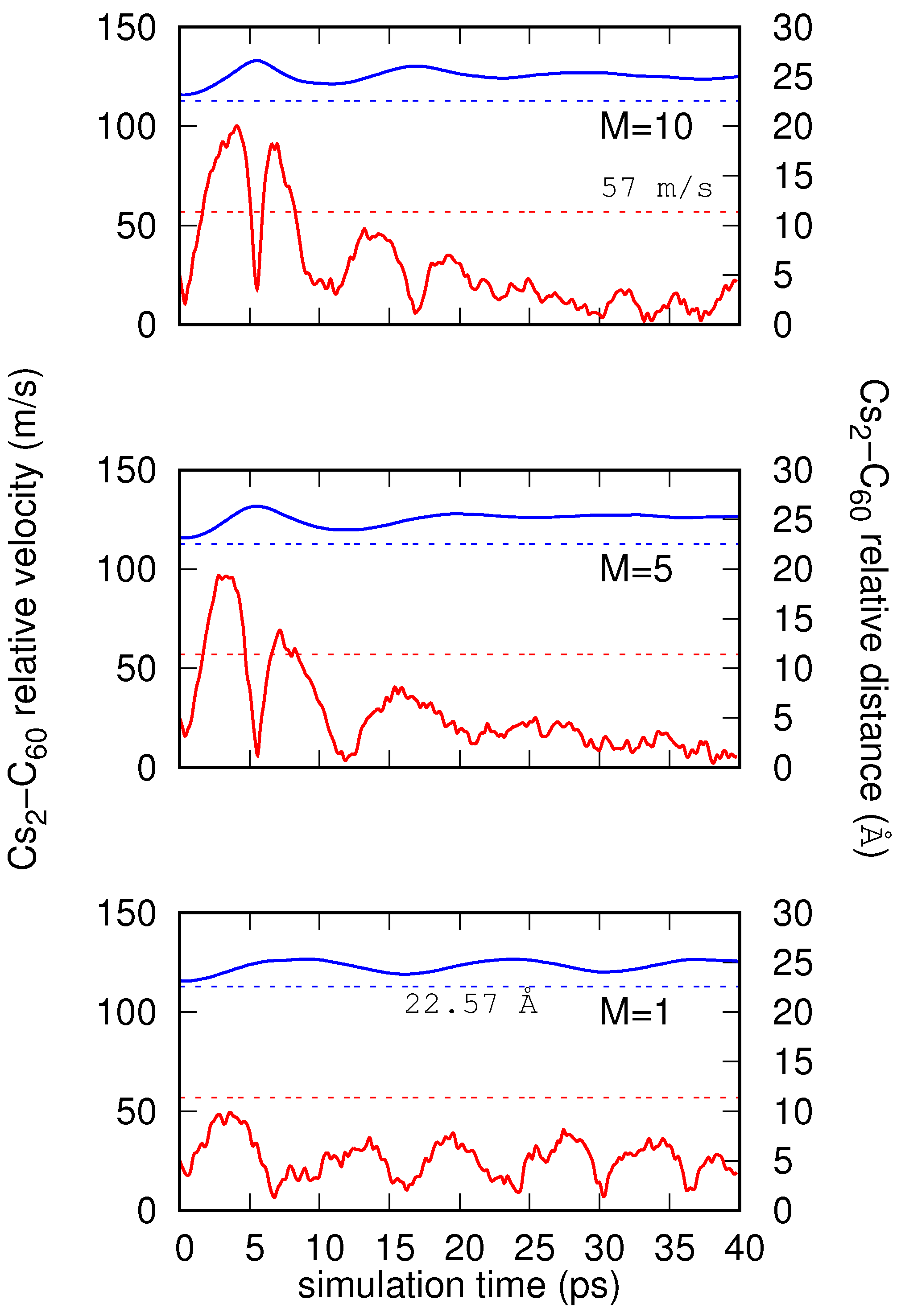
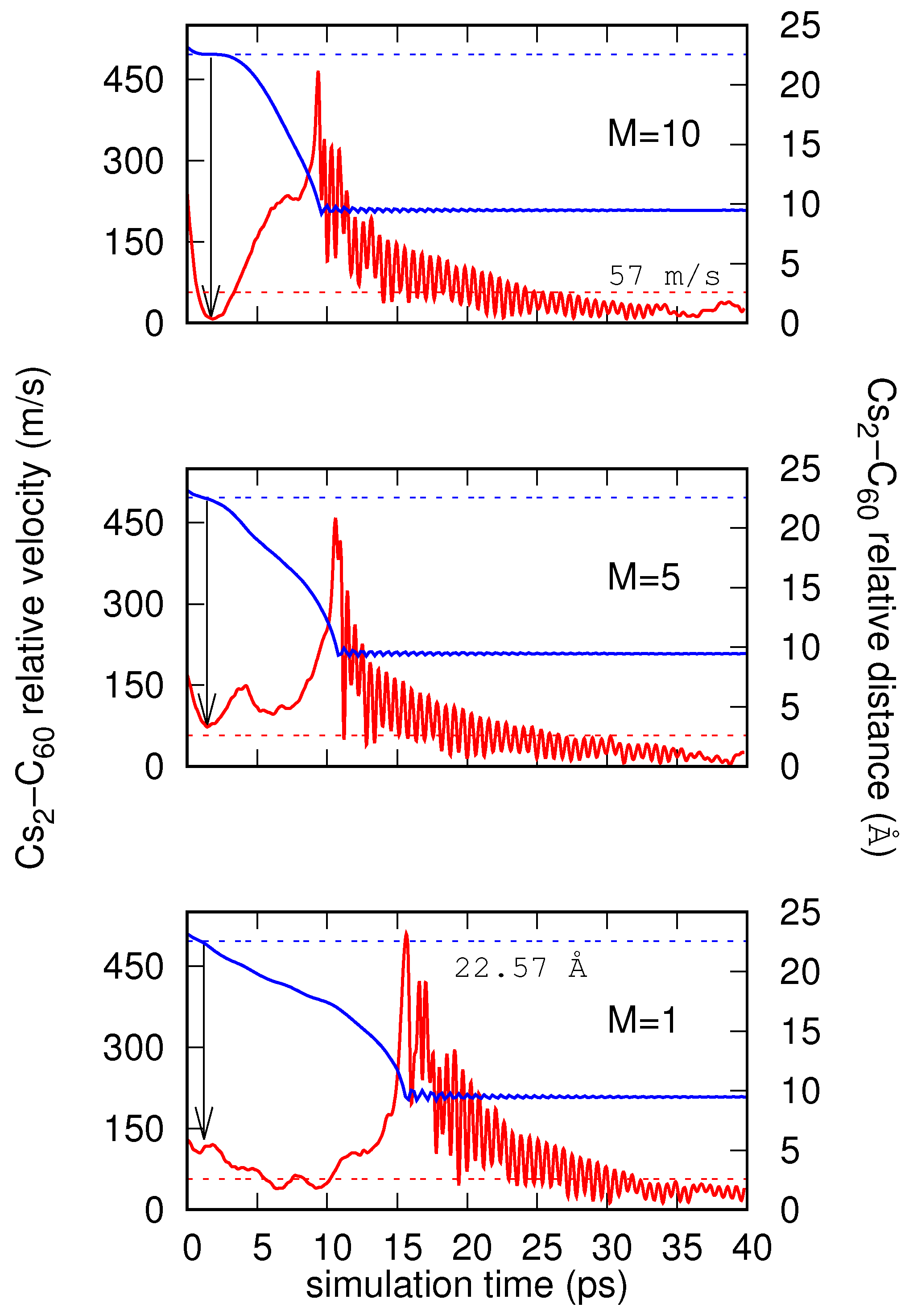
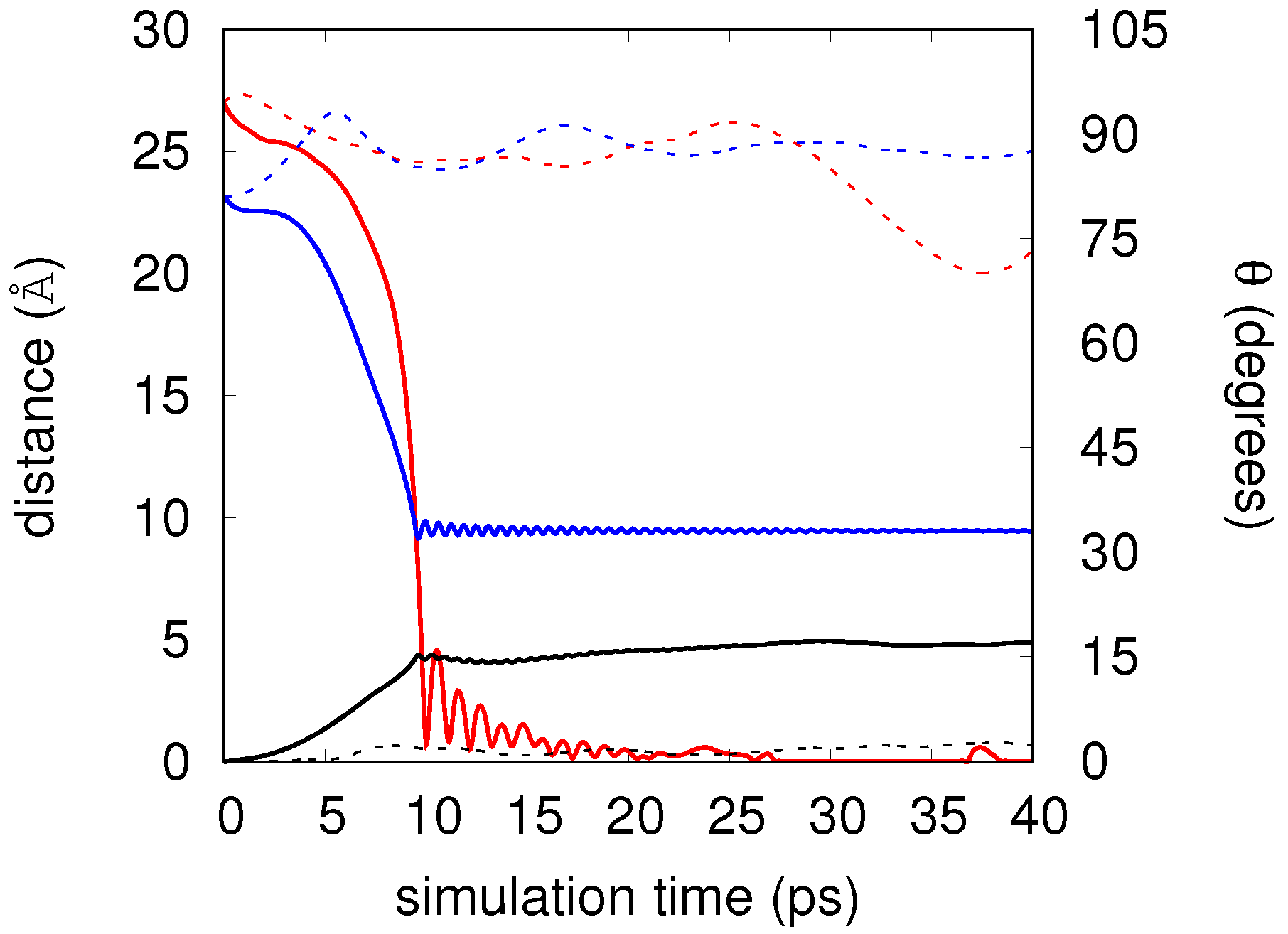
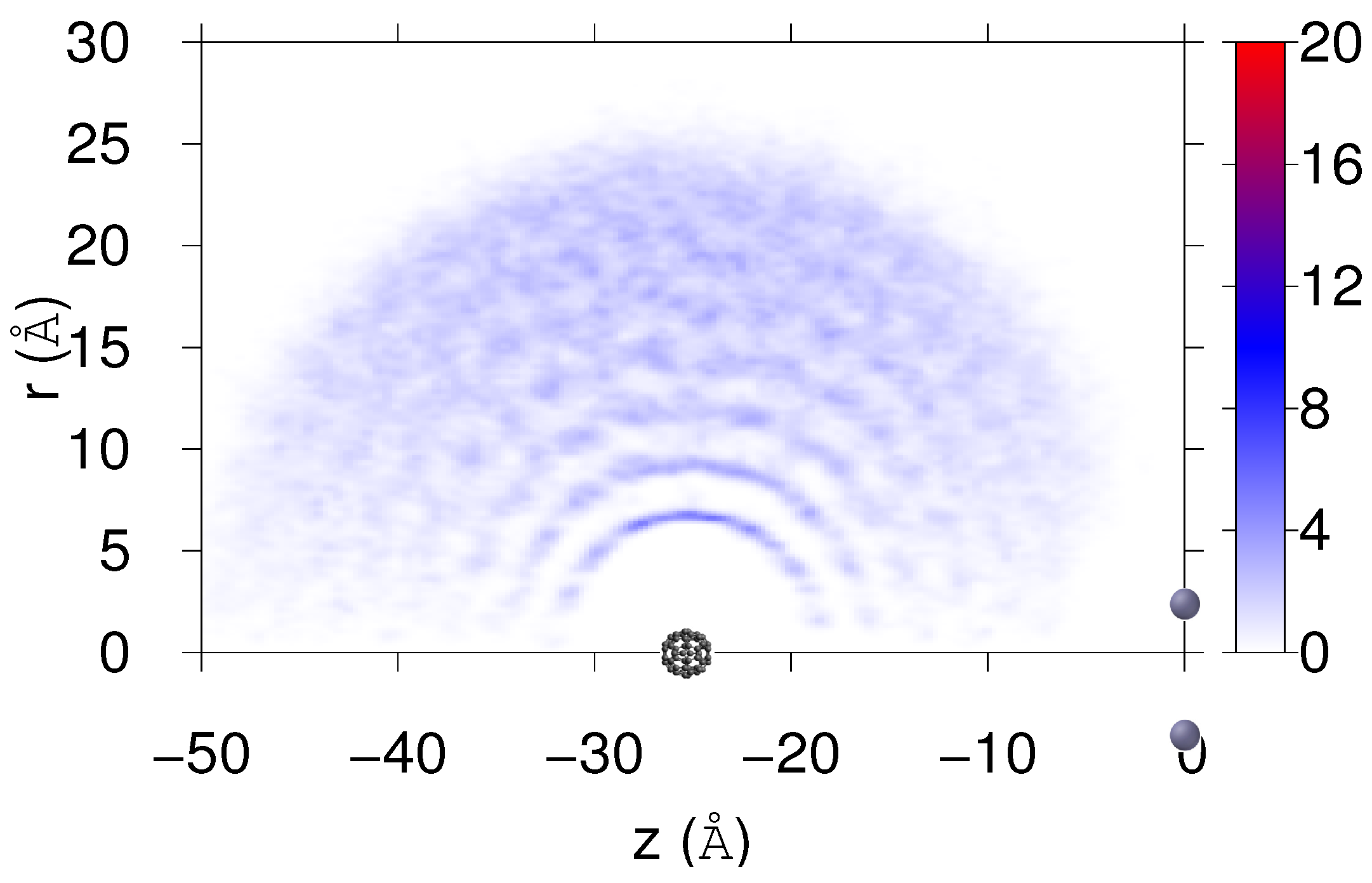
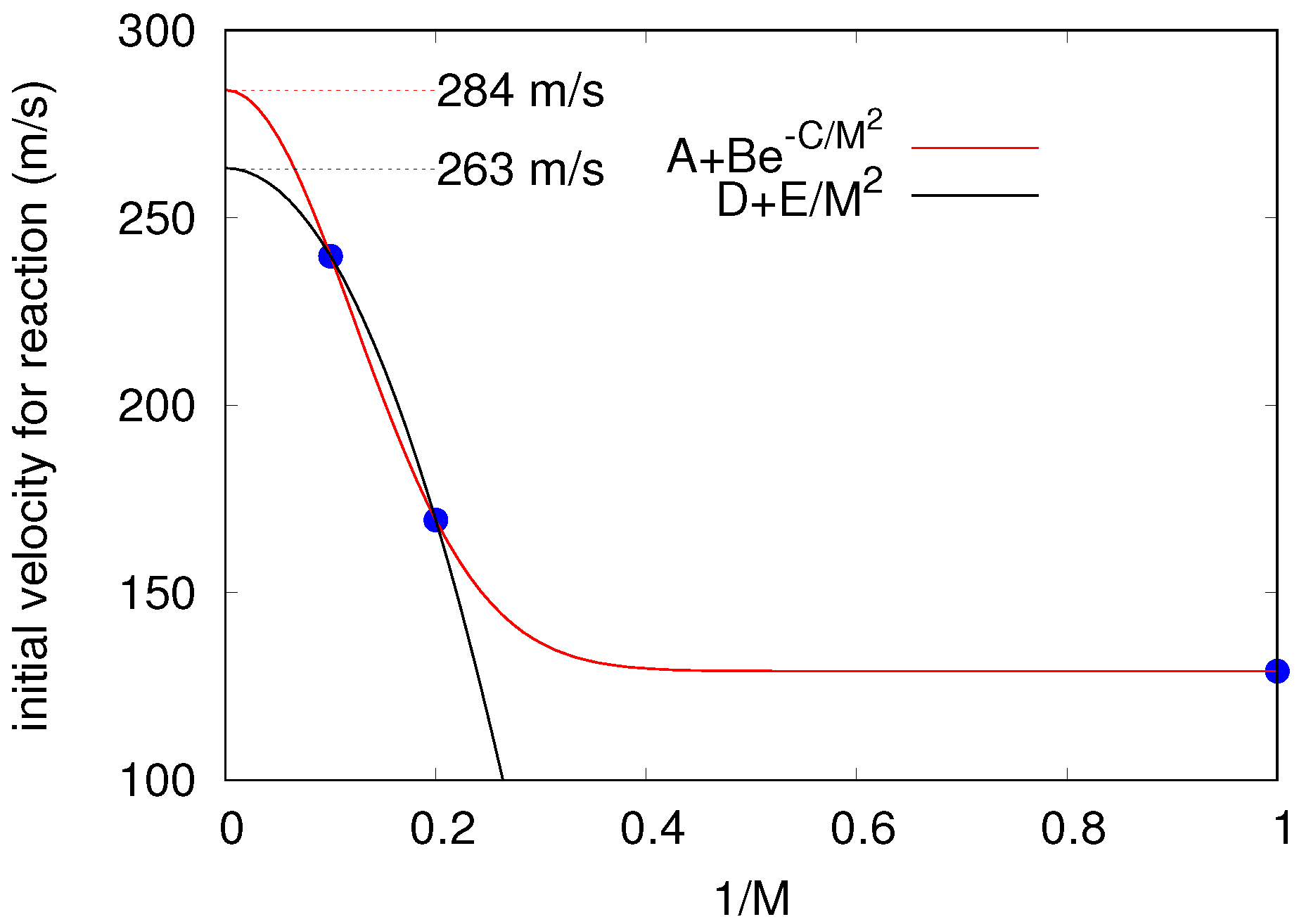
4. Results
Real-Time Simulations in the NVE Ensemble
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Toennies, J.P.; Vilesov, A.F. Spectroscopy of atoms and molecules in liquid helium. Annu. Rev. Phys. Chem. 1998, 49, 1–41. [Google Scholar] [CrossRef] [PubMed]
- Grebenev, S.; Toennies, J.P.; Vilesov, A.F. Superfluidity Within a Small Helium-4 Cluster: The Microscopic Andronikashvili Experiment. Science 1998, 279, 2083–2086. [Google Scholar] [CrossRef] [PubMed]
- Higgins, J.; Callegari, C.; Reho, J.; Stienkemeier, F.; Ernst, W.E.; Gutowski, M.; Scoles, G. Helium Cluster Isolation Spectroscopy of Alkali Dimers in the Triplet Manifold. J. Phys. Chem. A 1998, 102, 4952. [Google Scholar] [CrossRef]
- Goyal, S.; Schutt, D.L.; Scoles, G. Vibrational spectroscopy of sulfur hexafluoride attached to helium clusters. Phys. Rev. Lett. 1992, 69, 933–936. [Google Scholar] [CrossRef]
- Toennies, J.P.; Vilesov, A.F. Superfluid Helium Droplets: A Uniquely Cold Nanomatrix for Molecules and Molecular Complexes. Angew. Chem., Int. Ed. 2004, 43, 2622. [Google Scholar] [CrossRef] [PubMed]
- Choi, M.Y.; Douberly, G.E.; Falconer, T.M.; Lewis, W.K.; Lindsay, C.M.; Merritt, J.M.; Stiles, P.L.; Miller, R.E. Infrared spectroscopy of helium nanodroplets: Novel methods for physics and chemistry. Int. Rev. Phys. Chem. 2006, 25, 15–75. [Google Scholar] [CrossRef]
- Stienkemeier, F.; Lehmann, K.K. Spectroscopy and dynamics in helium nanodroplets. J. Phys. B Atom. Mol. Opt. Phys. 2006, 39, 127. [Google Scholar] [CrossRef]
- Mudrich, M.; Stienkemeier, F. Photoionisaton of pure and doped helium nanodroplets. Int. Rev. Phys. Chem. 2014, 33, 301. [Google Scholar] [CrossRef]
- An der Lan, L.; Bartl, P.; Leidlmair, C.; Schöbel, H.; Jochum, R.; Denifl, S.; Märk, T.D.; Ellis, A.M.; Scheier, P. The submersion of sodium clusters in helium nanodroplets: Identification of the surface → interior transition. J. Chem. Phys. 2011, 135, 044309. [Google Scholar] [CrossRef]
- An der Lan, L.; Bartl, P.; Leidlmair, C.; Schöbel, H.; Denifl, S.; Märk, T.D.; Ellis, A.M.; Scheier, P. Submersion of potassium clusters in helium nanodroplets. Phys. Rev. B 2012, 85, 115414. [Google Scholar] [CrossRef]
- Mauracher, A.; Daxner, M.; Postler, J.; Huber, S.E.; Denifl, S.; Scheier, P.; Toennies, J.P. Detection of Negative Charge Carriers in Superfluid Helium Droplets: The Metastable Anions He*− and He2*−. J. Phys. Chem. Lett. 2014, 5, 2444. [Google Scholar] [CrossRef] [PubMed]
- Mauracher, A.; Echt, O.; Ellis, A.M.; Yang, S.; Bohme, D.K.; Postler, J.; Kaiser, A.; Denifl, S.; Scheier, P. Cold physics and chemistry: Collisions, ionization and reactions inside helium nanodroplets close to zero K. Phys. Rep. 2018, 751, 1. [Google Scholar] [CrossRef]
- Hernando, A.; Barranco, M.; Mayol, R.; Pi, M.; Ancilotto, F.; Bünermann, O.; Stienkemeier, F. Absorption spectrum of Na atoms attached to helium nanodroplets. J. Low Temp. Phys. 2010, 158, 105. [Google Scholar] [CrossRef]
- Allard, O.; Nagl, J.; Auböck, G.; Callegari, C.; Ernst, W.E. Investigation of KRb and Rb2 formed on cold helium nanodroples. J. Phys. B At. Mol. Opt. Phys. 2006, 39, 1169–1181. [Google Scholar] [CrossRef]
- Claas, P.; Droppelmann, G.; Schulz, C.P.; Mudrich, M.; Stienkemeier, F. Wave Packet Dynamics in Triplet States of Na2 Attached to Helium Nanodroplets. J. Phys. Chem. A 2007, 111, 7537–7541. [Google Scholar] [CrossRef]
- Pifrader, A.; Allard, O.; Auböck, G.; Callegari, C.; Ernst, W.E.; Huber, R.; Ancilotto, F. One- and two-photon spectroscopy of highly excited states of alkali-metal atoms on helium nanodroplets. J. Chem. Phys. 2010, 133, 164502. [Google Scholar] [CrossRef]
- Auböck, G.; Nagl, J.; Callegari, C.; Ernst, W.E. Triplet state excitation of alkali molecules on helium droplets: Experiments and theory. J. Phys. Chem. A 2007, 111, 7404–7410. [Google Scholar] [CrossRef]
- Brühl, F.R.; Miron, R.A.; Ernst, W.E. Triplet states of rubidium dimers on helium nanodroplets. J. Chem. Phys. 2001, 115, 10275. [Google Scholar] [CrossRef]
- Theisen, M.; Lackner, F.; Ernst, W. Rb and Cs oligomers in different spin configurationson helium nanodroplet. J. Phys. Chem. A 2011, 115, 7005–7009. [Google Scholar] [CrossRef]
- Fechner, L.; Gruner, B.; Sieg, A.; Callegari, C.; Ancilotto, F.; Stienkemeier, F.; Mudrich, M. Photoionization and imaging spectroscopy of rubidium atoms attached to helium nanodroplets. Phys. Chem. Chem. Phys. 2012, 14, 3843. [Google Scholar] [CrossRef] [PubMed]
- von Vangerow, J.; Sieg, A.; Stienkemeier, F.; Mudrich, M.; Leal, A.; Mateo, D.; Hernando, A.; Barranco, M.; Pi, M. Desorption Dynamics of Heavy Alkali Metal Atoms (Rb, Cs) Off the Surface of Helium Nanodroplets. J. Phys. Chem. A 2014, 118, 6604. [Google Scholar] [CrossRef]
- Stienkemeier, F.; Higgins, J.; Ernst, W.E.; Scoles, G. Laser Spectroscopy of Alkali-Doped Helium Clusters. Phys. Rev. Lett. 1995, 74, 3592–3595. [Google Scholar] [CrossRef]
- Ernst, W.E.; Huber, R.; Jiang, S.; Beuc, R.; Movre, M.; Pichler, G. Cesium dimer spectroscopy on helium droplets. J. Chem. Phys. 2006, 124, 024313. [Google Scholar] [CrossRef]
- Rodríguez-Cantano, R.; González-Lezana, T.; Prosmiti, R.; Delgado-Barrio, G.; Villarreal, P.; Jellinek, J. Reactive scattering calculations for 87Rb+87RbHe–Rb2(3,v)+He from ultralow to intermediate energies. J. Chem. Phys. 2015, 142, 164304. [Google Scholar] [CrossRef]
- Renzler, M.; Daxner, M.; Kranabetter, L.; Kaiser, A.; Hauser, A.W.; Ernst, W.E.; Lindinger, A.; Zillich, R.; Scheier, P.; Ellis, A.M. Communication: Dopant-induced solvation of alkalis in liquid helium nanodroplets. J. Chem. Phys. 2016, 145, 181101. [Google Scholar] [CrossRef]
- Ancilotto, F.; Barranco, M.; Coppens, F.; Eloranta, J.; Halberstadt, N.; Hernando, A.; Mateo, D.; Pi, M. Density functional theory of doped superfluid liquid helium and nanodroplets. Int. Rev. Phys. Chem. 2017, 36, 621. [Google Scholar] [CrossRef]
- Coppens, F.; Ancilotto, F.; Barranco, M.; Halberstadt, N.; Pi, M. Capture of Xe and Ar atoms by quantized vortices in 4He nanodroplets. Phys. Chem. Chem. Phys. 2017, 19, 24805. [Google Scholar] [CrossRef] [PubMed]
- Coppens, F.; Ancilotto, F.; Barranco, M.; Halberstadt, N.; Pi, M. Dynamics of impurity clustering in superfluid 4He nanodroplets. Phys. Chem. Chem. Phys. 2019, 21, 17423. [Google Scholar] [CrossRef] [PubMed]
- Vilà, A.; González, M. Reaction dynamics inside super-fluid helium nanodroplets: The formation of the Ne2 molecule from Ne + Ne@(4He)N. Phys. Chem. Chem. Phys. 2016, 18, 31869. [Google Scholar] [CrossRef]
- Blancafort-Jorquera, M.; Vilà, A.; González, M. Quantum–classical approach to the reaction dynamics in a superfluid helium nanodroplet. The Ne2 dimer and Ne–Ne adduct formation reaction Ne + Ne-doped nanodroplet. Phys. Chem. Chem. Phys. 2019, 21, 24218. [Google Scholar] [CrossRef] [PubMed]
- Ceperley, D.M. Path integrals in the theory of condensed helium. Rev. Mod. Phys. 1995, 67, 279–355. [Google Scholar] [CrossRef]
- Kwon, Y.; Ceperley, D.M.; Whaley, K.B. Path integral Monte Carlo study of SF6-doped helium clusters. J. Chem. Phys. 1996, 104, 2341–2348. [Google Scholar] [CrossRef][Green Version]
- Pérez de Tudela, R.; López-Durán, D.; González-Lezana, T.; Delgado-Barrio, G.; Villarreal, P.; Gianturco, F.A.; Yurtsever, E. Quantum Features of a Barely Bound Molecular Dopant: Cs2(3Σu) in Bosonic Helium Droplets of Variable Size. J. Phys. Chem. A 2011, 115, 6892. [Google Scholar] [CrossRef] [PubMed]
- Rodríguez-Cantano, R.; López-Durán, D.; Pérez de Tudela, R.; González-Lezana, T.; Delgado-Barrio, G.; Villarreal, P.; Gianturco, F.A. Helium aggregates doped with alkali dimer impurities: A finite temperature study of complexes. Comput. Theor. Chem. 2012, 990, 106. [Google Scholar] [CrossRef]
- Rodríguez-Cantano, R.; Pérez de Tudela, R.; López-Durán, D.; González-Lezana, T.; Gianturco, F.A.; Delgado-Barrio, G.; Villarreal, P. Quantum rotation of Rb2 (3) attached to HeN droplets: A path-integral Monte Carlo study. Eur. Phys. J. D 2013, 67, 119. [Google Scholar] [CrossRef]
- Rodríguez-Cantano, R.; González-Lezana, T.; Villarreal, P. Path integral Monte Carlo investigations on doped helium clusters. Int. Rev. Phys. Chem. 2016, 35, 37. [Google Scholar] [CrossRef]
- Rodríguez-Cantano, R.; Pérez de Tudela, R.; Bartolomei, M.; Hernández, M.I.; Campos-Martínez, J.; González-Lezana, T.; Villarreal, P.; Hernández-Rojas, J.; Bretón, J. Coronene molecules in helium clusters: Quantum and classical studies of energies and configurations. J. Chem. Phys. 2015, 143, 224306. [Google Scholar] [CrossRef]
- Rodríguez-Cantano, R.; Bartolomei, M.; Hernández, M.I.; Campos-Martínez, J.; González-Lezana, T.; Villarreal, P.; de Tudela, R.P.; Pirani, F.; Hernández-Rojas, J.; Bretón, J. Comparative investigation of pure and mixed rare gas atoms on coronene molecules. J. Chem. Phys. 2017, 146, 034302. [Google Scholar] [CrossRef]
- Rastogi, M.; Leidlmair, C.; An der Lan, L.; Ortiz de Zárate, J.; Pérez de Tudela, R.; Bartolomei, M.; Hernández, M.I.; Campos-Martínez, J.; González-Lezana, T.; Hernández-Rojas, J.; et al. Lithium ions solvated in helium. Phys. Chem. Chem. Phys. 2018, 20, 25569. [Google Scholar] [CrossRef]
- Ceriotti, M.; Parrinello, M.; Markland, T.E.; Manolopoulos, D.E. Efficient stochastic thermosthatting of path integral molecular dynamics. J. Chem. Phys. 2010, 133, 124104. [Google Scholar] [CrossRef]
- Castillo-García, A.; González-Lezana, T.; Delgado-Barrio, G.; Villarreal, P. Formation of rubidium dimers on the surface of helium clusters: A first step through quantum molecular dynamics simulations. Eur. Phys. J. D 2018, 72, 102. [Google Scholar] [CrossRef]
- de Lara-Castells, M.P.; Hauser, A.W.; Mitrushchenkov, A.O. Ab Initio Confirmation of a Harpoon-Type Electron Transfer in a Helium Droplet. J. Phys. Chem. Lett. 2017, 8, 4284. [Google Scholar] [CrossRef]
- Hauser, A.W.; de Lara-Castells, M.P. Spatial quenching of a molecular charge-transfer process in a quantum fluid: The Csx-C60 reaction in supefluid helium nanodroplets. Phys. Chem. Chem. Phys. 2017, 19, 1342. [Google Scholar] [CrossRef]
- Smolarek, N.B.B.S.; Loginov, E.; Mateo, D.; Hernando, A.; Pi, M.; Barranco, M.; Buma, W.J.; Drabbels, M. Critical Landau Velocity in Helium Nanodroplets. Phys. Rev. Lett. 2013, 111, 153002. [Google Scholar]
- Walewski, L.; Forbert, H.; Marx, D. Reactive path integral quantum simulations of molecules solvated in superfluid helium. Comput. Phys. Commun. 2014, 185, 844. [Google Scholar] [CrossRef]
- Aziz, R.A.; Slaman, M.J. An examination of ab initio results for the helium potential energy curve. J. Chem. Phys. 1991, 94, 8047–8053. [Google Scholar] [CrossRef]
- Prosmiti, R.; Delgado-Barrio, G.; Villarreal, P.; Yurtsever, E.; Coccia, E.; Gianturco, F.A. Structuring a Quantum Solvent around a Weakly Bound Dopant: The He–Cs2(3Σu) Complex. J. Phys. Chem. A 2009, 113, 14718–14729. [Google Scholar] [CrossRef] [PubMed]
- Hesselmann, A.; Korona, T. On the accuracy of DFT-SAPT, MP2, SCS-MP2, MP2C, and DFT+Disp methods for the interaction energies of endohedral complexes of the C60 fullerene with a rare gas atom. Phys. Chem. Chem. Phys. 2011, 13, 732–743. [Google Scholar] [CrossRef] [PubMed]
- Gronowski, M.; Koza, A.M.; Tomza, M. Ab initio properties of the NaLi molecule in the 3Σ+ electronic state. Phys. Rev. A 2020, 102, 020801. [Google Scholar] [CrossRef]
- Leininger, T.; Nicklass, A.; Küchle, W.; Stoll, H.; Dolg, M.; Berhner, A. The accuracy of the pseudopotential approximation: Non-frozen-core effects for spectroscopic constants of alkali fluorides XF (X = K, Rb, Cs). Chem. Phys. Lett. 1996, 255, 274–280. [Google Scholar] [CrossRef]
- Jansen, G.; Hess, B.A. Revision of the Douglas-Kroll transformation. Phys. Rev. A 1989, 39, 6016–6017. [Google Scholar] [CrossRef]
- de Lara-Castells, M.P.; Mitrushchenkov, A.O.; Stoll, H. Combining density functional and incremental post-Hartree-Fock approaches for van der Waals dominated adsorbate-surface interactions: Ag2/graphene. J. Chem. Phys. 2015, 143, 102804. [Google Scholar] [CrossRef]
- Rodríguez-Cantano, R.; López-Durán, D.; González-Lezana, T.; Delgado-Barrio, G.; Villarreal, P.; Yurtsever, E.; Gianturco, F.A. Spin-Polarized Rb2 Interacting with Bosonic He Atoms: Potential Energy Surface and Quantum Structures of Small Clusters. J. Phys. Chem. A 2012, 116, 2394. [Google Scholar] [CrossRef] [PubMed]
- de Lara-Castells, M.P.; Krause, J.L. Theoretical study of the UV-induced desorption of molecular oxygen from the reduced TiO2 (110) surface. J. Chem. Phys. 2003, 118, 5098–5105. [Google Scholar] [CrossRef]
- de Lara-Castells, M.P.; Mitrushenkov, A.O.; Roncero, O.; Krause, J.L. Adsorption and nonadiabatic processes in the photodesorption of molecular oxygen from the reduced TiO2 (110) surface. Israel J. Chem. 2005, 45, 59–76. [Google Scholar] [CrossRef]
- Habershon, S.; Manolopoulos, D.E.; Markland, T.E.; Miller, T.F., III. Ring-Polymer Molecular Dynamics: Quantum Effects in Chemical Dynamics from Classical Trajectories in an Extended Phase Space. Annu. Rev. Phys. Chem. 2013, 64, 387. [Google Scholar] [CrossRef]
- Ceriotti, M.; More, J.; Manolopoulos, D.E. i-PI: A Python interface for ab initio path integral molecular dynamics. Comput. Phys. Commun. 2014, 185, 1019. [Google Scholar] [CrossRef]
- Bussi, G.; Parrinello, M. Accurate sampling using Langevin dynamics. Phys. Rev. E 2007, 75, 056707. [Google Scholar] [CrossRef] [PubMed]
- Baym, G.; Pethick, C.J. Landau critical velocity in weakly interacting Bose gases. Phys. Rev. A 2012, 86, 023602. [Google Scholar] [CrossRef]
- Zillich, R.E.; Whaley, K.B. Solvation Structure and Rotational Dynamics of LiH in 4He Clusters. J. Phys. Chem. A 2007, 111, 7489. [Google Scholar] [CrossRef] [PubMed]
- Halberstadt, N.; Bonhommeau, D.A. Fragmentation dynamics of Ar4He1000 upon electron impact ionization: Competition between ion ejection and trapping. J. Chem. Phys. 2020, 152, 234305. [Google Scholar] [CrossRef] [PubMed]
- Bonhommeau, D.; Lake, P.T.; Le Quiniou, C.; Lewerenz, M.; Halberstadt, N. Modeling the fragmentation dynamics of ionic clusters inside helium nanodroplets: The case of He100. J. Chem. Phys. 2007, 126, 051104. [Google Scholar] [CrossRef] [PubMed]
- Fernández-Perea, R.; Gómez, L.F.; Cabrillo, C.; Pi, M.; Mitrushchenkov, A.O.; Vilesov, A.F.; de Lara-Castells, M.P. Helium Droplet-Mediated Deposition and Aggregation of Nanoscale Silver Clusters on Carbon Surfaces. J. Phys. Chem. C 2017, 121, 22248–22257. [Google Scholar] [CrossRef]


| Cs-C | Cs-C | He-C | |||||
|---|---|---|---|---|---|---|---|
| C | T | C | T | ||||
| −2.22575d05 | 0 | 5.89589d03 | 4.52665d03 | ||||
| 9.66771d07 | 0 | −1.37653d05 | −1.03277d05 | ||||
| −1.60255d10 | 9.598560d7 | −3.91681d08 | −3.39629d8 | −1.90652d07 | |||
| 1.18367d12 | −1.37271d11 | 6.40779d12 | 8.58655d12 | 3.76378d09 | |||
| −4.10087d13 | 1.26854d13 | 5.14755d03 | 5.14755d03 | −2.79654d11 | |||
| 5.48817d14 | −4.37303d14 | 1.16141d05 | 1.16141d05 | 6.20350d12 | |||
| 0 | 5.43005d15 | , | 0 | 0 | |||
| −2.71882d08 | −2.71882d08 | , | −3.9 | 0 | |||
| 12.5 | 12.5 | 28.0 | 20.0 | ||||
| C | T | |
|---|---|---|
| a | 7047.479 | 1343.188 |
| b | 10,489.841 | 2226.989 |
| c | 469,477.985 | 13,407.221 |
| d | 2,797,586.355 | 43,037.030 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Castillo-García, A.; Hauser, A.W.; de Lara-Castells, M.P.; Villarreal, P. A Path Integral Molecular Dynamics Simulation of a Harpoon-Type Redox Reaction in a Helium Nanodroplet. Molecules 2021, 26, 5783. https://doi.org/10.3390/molecules26195783
Castillo-García A, Hauser AW, de Lara-Castells MP, Villarreal P. A Path Integral Molecular Dynamics Simulation of a Harpoon-Type Redox Reaction in a Helium Nanodroplet. Molecules. 2021; 26(19):5783. https://doi.org/10.3390/molecules26195783
Chicago/Turabian StyleCastillo-García, Alvaro, Andreas W. Hauser, María Pilar de Lara-Castells, and Pablo Villarreal. 2021. "A Path Integral Molecular Dynamics Simulation of a Harpoon-Type Redox Reaction in a Helium Nanodroplet" Molecules 26, no. 19: 5783. https://doi.org/10.3390/molecules26195783
APA StyleCastillo-García, A., Hauser, A. W., de Lara-Castells, M. P., & Villarreal, P. (2021). A Path Integral Molecular Dynamics Simulation of a Harpoon-Type Redox Reaction in a Helium Nanodroplet. Molecules, 26(19), 5783. https://doi.org/10.3390/molecules26195783







