Application of Ionic Liquids in Pot-in-Pot Reactions
Abstract
:1. Introduction
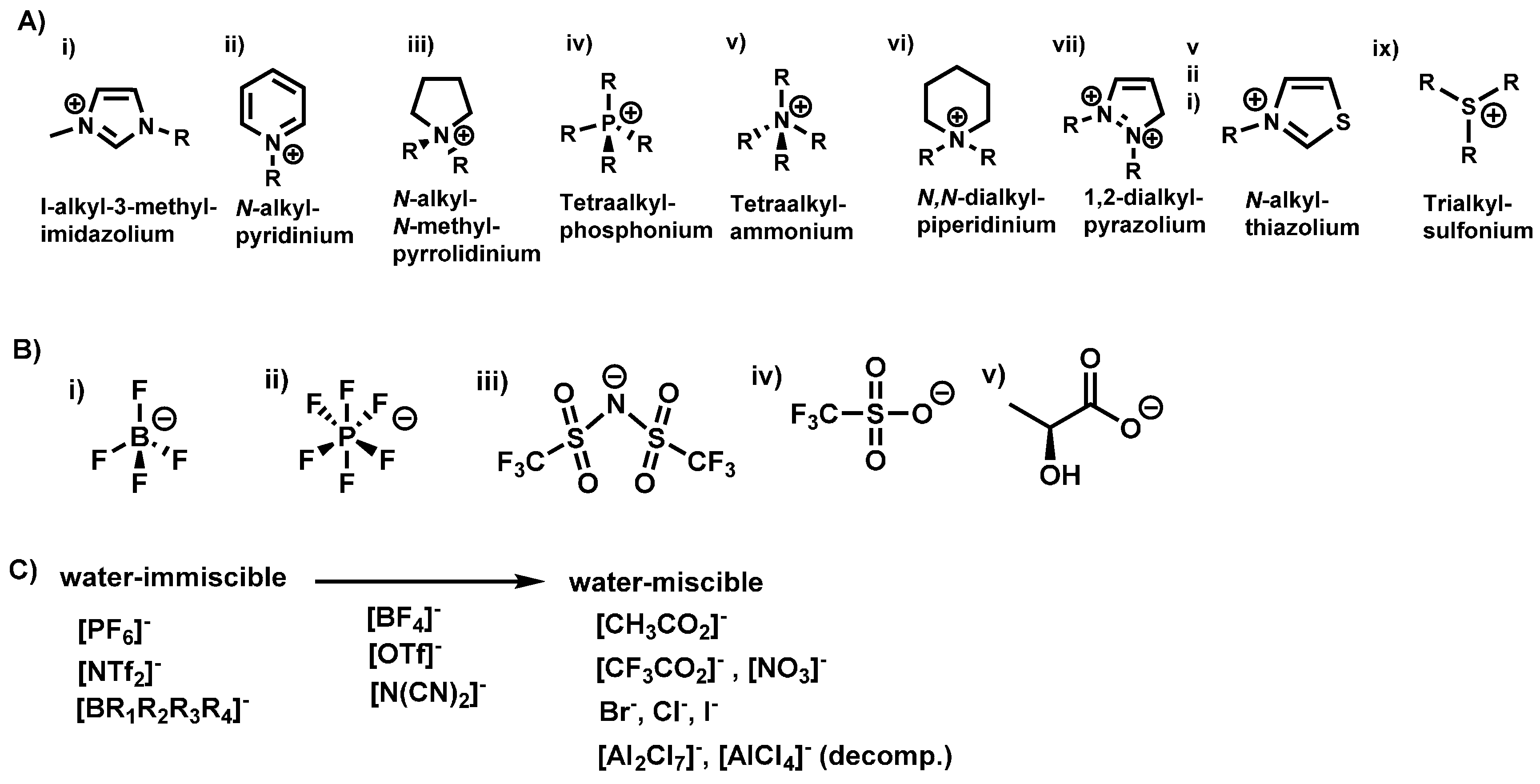
Unique Properties of Ionic Liquids
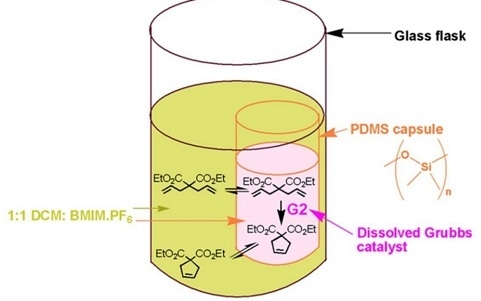
2. Ionic Liquids in Pot-in-Pot Reactions
3. Diffusion and Flux in Polymeric Membranes
3.1. Theories on Diffusion and Flux
3.2. General Transport Across Membranes
3.3. Role of Ionic Liquids in Selective Uni-Directional Flux in Polymer Membranes
4. Examples of Pot-in-pot Reactions
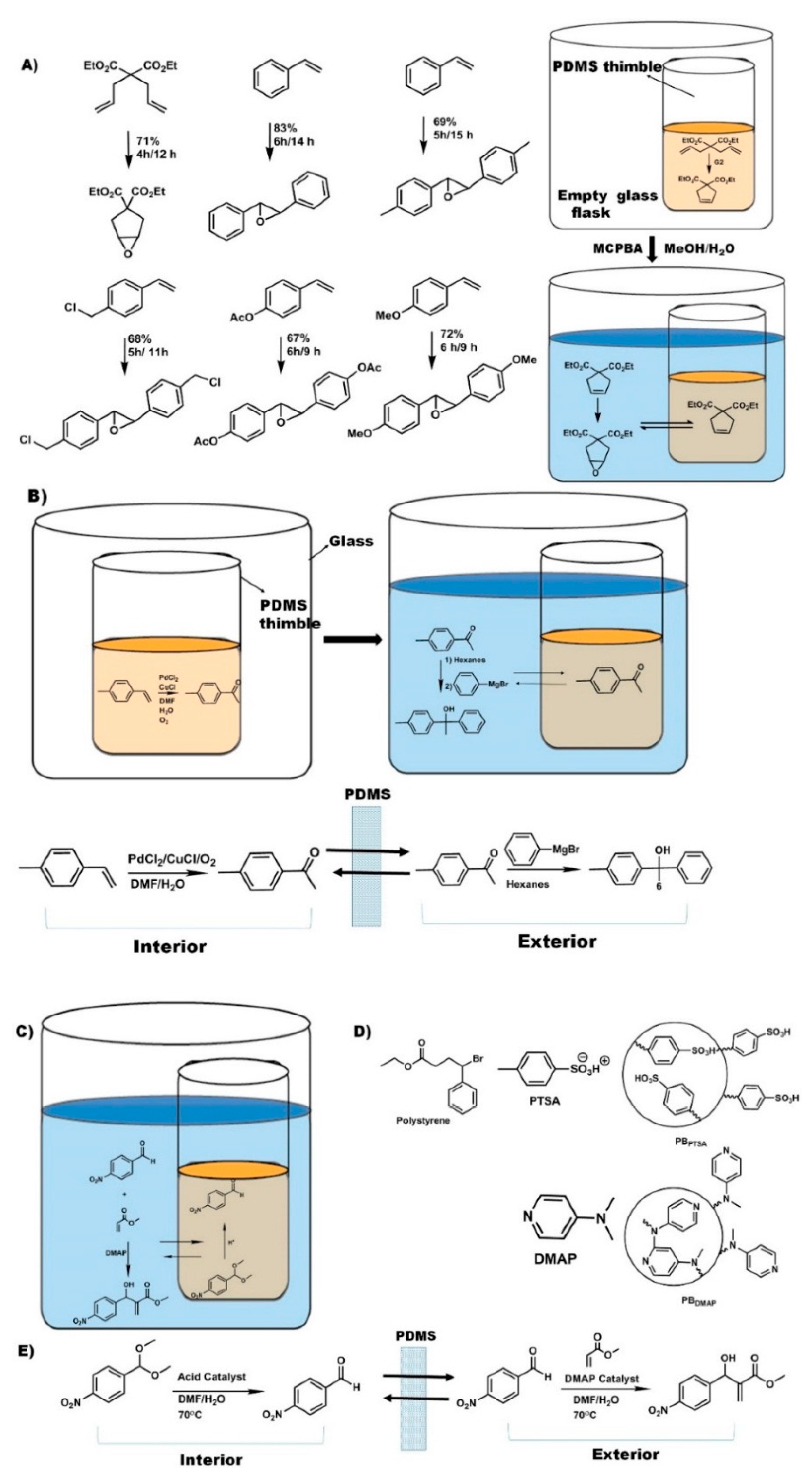
4.1. Site-Isolation of Incompatible Reactions
4.2. Pot-in-Pot Reactions for Difficult to Handle Reagents and as Solution to Simple But Tedious Synthesis
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Aerts, S.; Buekenhoudt, A.; Weyten, H.; Gevers, L.E.M.; Vankelecom, I.F.J.; Jacobs, P.A. The use of solvent resistant nanofiltration in the recycling of the co-jacobsen catalyst in the hydrolytic kinetic resolution (hkr) of epoxides. J. Membr. Sci. 2006, 280, 245–252. [Google Scholar] [CrossRef]
- Dijkstra, H.P.; van Klink, G.P.M.; van Koten, G. The use of ultra- and nanofiltration techniques in homogeneous catalyst recycling. Acc. Chem. Res. 2002, 35, 798–810. [Google Scholar] [CrossRef] [PubMed]
- Luo, Y.; Tan, S.; Wang, H.; Wu, F.; Liu, X.; Li, L.; Zhang, Z. Ppms composite membranes for the concentration of organics from aqueous solutions by pervaporation. Chem. Eng. J. 2008, 137, 496–502. [Google Scholar] [CrossRef]
- Narayanaswamy, R. Diffusion of Aliphatic Molecules through Polydimethylsiloxane Membrane: Predictive Approaches; University of Iowa: Iowa City, IA, USA, 1992. [Google Scholar]
- Watson, J.M.; Zhang, G.S.; Payne, P.A. The diffusion mechanism in silicone rubber. J. Membr. Sci. 1992, 73, 55–71. [Google Scholar] [CrossRef]
- Zhang, X.; Poojari, Y.; Drechsler, L.; Kuo, C.; Fried, J.; Clarson, S. Pervaporation of organic liquids from binary aqueous mixtures using poly(trifluoropropylmethylsiloxane) and poly(dimethylsiloxane) dense membranes. J. Inorg. Organomet. Polym. Mater. 2008, 18, 246–252. [Google Scholar] [CrossRef]
- Hasanoğlu, A.; Salt, Y.; Keleşer, S.; Özkan, S.; Dinçer, S. Pervaporation separation of organics from multicomponent aqueous mixtures. Chem. Eng. Process. Process Intensif. 2007, 46, 300–306. [Google Scholar] [CrossRef]
- Ki Hong, Y.; Hi Hong, W. Influence of ceramic support on pervaporation characteristics of ipa/water mixtures using pdms/ceramic composite membrane. J. Membr. Sci. 1999, 159, 29–39. [Google Scholar] [CrossRef]
- Kujawski, W.; Krajewski, S.R. Influence of inorganic salt on the effectiveness of liquid mixtures separation by pervaporation. Sep. Purif. Technol. 2007, 57, 495–501. [Google Scholar] [CrossRef]
- Watson, J.M.; Payne, P.A. A study of organic compound pervaporation through silicone rubber. J. Membr. Sci. 1990, 49, 171–205. [Google Scholar] [CrossRef]
- Xiangli, F.; Wei, W.; Chen, Y.; Jin, W.; Xu, N. Optimization of preparation conditions for polydimethylsiloxane (pdms)/ceramic composite pervaporation membranes using response surface methodology. J. Membr. Sci. 2008, 311, 23–33. [Google Scholar] [CrossRef]
- Handy, S.T. Grignard reactions in imidazolium ionic liquids. J. Org. Chem. 2006, 71, 4659–4662. [Google Scholar] [CrossRef] [PubMed]
- Schenzel, A.; Hufendiek, A.; Barner-Kowollik, C.; Meier, M.A.R. Catalytic transesterification of cellulose in ionic liquids: Sustainable access to cellulose esters. Green Chem. 2014, 16, 3266–3271. [Google Scholar] [CrossRef]
- Shen, Z.-L.; Zhou, W.-J.; Liu, Y.-T.; Ji, S.-J.; Loh, T.-P. One-pot chemoenzymatic syntheses of enantiomerically-enriched o-acetyl cyanohydrins from aldehydes in ionic liquid. Green Chem. 2008, 10, 283–286. [Google Scholar] [CrossRef]
- D’Arrigo, P.; Cerioli, L.; Chiappe, C.; Panzeri, W.; Tessaro, D.; Mele, A. Improvements in the enzymatic synthesis of phosphatidylserine employing ionic liquids. J. Mol. Catal. B Enzym. 2012, 84, 132–135. [Google Scholar] [CrossRef]
- Migowski, P.; Dupont, J. Catalytic applications of metal nanoparticles in imidazolium ionic liquids. Chem. Eur. J. 2007, 13, 32–39. [Google Scholar] [CrossRef] [PubMed]
- Cevasco, G.; Chiappe, C. Are ionic liquids a proper solution to current environmental challenges? Green Chem. 2014, 16, 2375–2385. [Google Scholar] [CrossRef]
- Hallett, J.P.; Welton, T. Room-temperature ionic liquids: Solvents for synthesis and catalysis. 2. Chem. Rev. 2011, 111, 3508–3576. [Google Scholar] [CrossRef] [PubMed]
- Plechkova, N.V.; Seddon, K.R. Applications of ionic liquids in the chemical industry. Chem. Soc. Rev. 2008, 37, 123–150. [Google Scholar] [CrossRef] [PubMed]
- Welton, T. Room-temperature ionic liquids. Solvents for synthesis and catalysis. Chem. Rev. 1999, 99, 2071–2084. [Google Scholar] [CrossRef] [PubMed]
- Shah, J.K.; Brennecke, J.F.; Maginn, E.J. Thermodynamic properties of the ionic liquid 1-n-butyl-3-methylimidazolium hexafluorophosphate from monte carlo simulations. Green Chem. 2002, 4, 112–118. [Google Scholar] [CrossRef]
- Hapiot, P.; Lagrost, C. Electrochemical reactivity in room-temperature ionic liquids. Chem. Rev. 2008, 108, 2238–2264. [Google Scholar] [CrossRef] [PubMed]
- Ho, T.D.; Zhang, C.; Hantao, L.W.; Anderson, J.L. Ionic liquids in analytical chemistry: Fundamentals, advances, and perspectives. Anal. Chem. 2014, 86, 262–285. [Google Scholar] [CrossRef] [PubMed]
- Huddleston, J.G.; Willauer, H.D.; Swatloski, R.P.; Visser, A.E.; Rogers, R.D. Room temperature ionic liquids as novel media for “clean” liquid-liquid extraction. Chem. Commun. 1998, 1765–1766. [Google Scholar] [CrossRef]
- Allen, C.; Sambasivarao, S.V.; Acevedo, O. An ionic liquid dependent mechanism for base catalyzed β-elimination reactions from qm/mm simulations. J. Am. Chem. Soc. 2013, 135, 1065–1072. [Google Scholar] [CrossRef] [PubMed]
- Sambasivarao, S.V.; Acevedo, O. Development of opls-aa force field parameters for 68 unique ionic liquids. J. Chem. Theory Comput. 2009, 5, 1038–1050. [Google Scholar] [CrossRef] [PubMed]
- Zahn, S.; Uhlig, F.; Thar, J.; Spickermann, C.; Kirchner, B. Intermolecular forces in an ionic liquid ([mmim][cl]) versus those in a typical salt (nacl). Angew. Chem. Int. Ed. 2008, 47, 3639–3641. [Google Scholar] [CrossRef] [PubMed]
- Wu, W.; Lu, Y.; Liu, Y.; Li, H.; Peng, C.; Liu, H.; Zhu, W. Structures and electronic properties of transition metal-containing ionic liquids: Insights from ion pairs. J. Phys. Chem. A 2014, 118, 2508–2518. [Google Scholar] [CrossRef] [PubMed]
- Tsuzuki, S.; Tokuda, H.; Hayamizu, K.; Watanabe, M. Magnitude and directionality of interaction in ion pairs of ionic liquids: Relationship with ionic conductivity. J. Phys. Chem. B 2005, 109, 16474–16481. [Google Scholar] [CrossRef] [PubMed]
- Atkinson, M.B.J.; Oyola-Reynoso, S.; Luna, R.E.; Bwambok, D.K.; Thuo, M.M. Pot-in-pot reactions: A simple and green approach to efficient organic synthesis. RSC Adv. 2015, 5, 597–607. [Google Scholar] [CrossRef]
- Mwangi, M.T.; Runge, M.B.; Hoak, K.M.; Schulz, M.D.; Bowden, N.B. A materials approach to site-isolation of grubbs catalysts from incompatible solvents and m-chloroperoxybenzoic acid. Chem. Eur. J. 2008, 14, 6780–6788. [Google Scholar] [CrossRef] [PubMed]
- Couto, R.; Neves, L.; Simoes, P.; Coelhoso, I. Supported ionic liquid membranes and ion-jelly(r) membranes with [bmim][dca]: Comparison of its performance for co2 separation. Membranes (Basel) 2015, 5, 13–21. [Google Scholar] [CrossRef] [PubMed]
- Scovazzo, P.; Visser, A.E.; Davis, J.H.; Rogers, R.D.; Koval, C.A.; DuBois, D.L.; Noble, R.D. Supported ionic liquid membranes and facilitated ionic liquid membranes. ChemInform 2002, 818, 69–87. [Google Scholar]
- De los Ríos, A.P.; Hernández-Fernández, F.J.; Lozano, L.J.; Godínez, C. Supported ionic liquid membranes: Preparation, stability and applications. In Green Solvents II; Ali Mohammad, I., Ed.; Springer: Dordrecht, The Netherlands, 2012; pp. 275–288. [Google Scholar]
- Fick, A.V. On liquid diffusion. Philos. Mag. Ser. 4 1855, 10, 30–39. [Google Scholar] [CrossRef]
- Deen, W.M. Analysis of Transport Phenomena; Oxford University Press: New York, NY, USA, 1998. [Google Scholar]
- Cussler, E.L. Diffusion: Mass Transfer in Fluid Systems; Cambridge University Press: New York, NY, USA, 1997. [Google Scholar]
- Miller, C.C. The stokes-einstein law for diffusion in solution. P. R. Soc. Lond. A Conta 1924, 106, 724–749. [Google Scholar] [CrossRef]
- Truskey, G.A.; Yuan, F.; Katz, D.F. Transport Phenomena in Biological Systems; Pearson/Prentice Hall: Upper Saddle River, NJ, USA, 2004. [Google Scholar]
- Nicolaou, K.C.; Chen, J.S. The art of total synthesis through cascade reactions. Chem. Soc. Rev. 2009, 38, 2993–3009. [Google Scholar] [CrossRef] [PubMed]
- Nicolaou, K.C.; Edmonds, D.J.; Bulger, P.G. Cascade reactions in total synthesis. Angew. Chem. Int. Ed. 2006, 45, 7134–7186. [Google Scholar] [CrossRef] [PubMed]
- Munnik, P.; de Jongh, P.E.; de Jong, K.P. Recent developments in the synthesis of supported catalysts. Chem. Rev. 2015, 115, 6687–6718. [Google Scholar] [CrossRef] [PubMed]
- Bonhôte, P.; Dias, A.-P.; Papageorgiou, N.; Kalyanasundaram, K.; Grätzel, M. Hydrophobic, highly conductive ambient-temperature molten salts. Inorg. Chem. 1996, 35, 1168–1178. [Google Scholar] [CrossRef] [PubMed]
- Ding, X.; Lv, X.; Hui, B.; Chen, Z.; Xiao, M.; Guo, B.; Tang, W. Olefin self-cross-metathesis catalyzed by the second-generation grubbs carbene complex in room temperature ionic liquids. Tetrahedron Lett. 2006, 47, 2921–2924. [Google Scholar] [CrossRef]
- Nockemann, P.; Binnemans, K.; Driesen, K. Purification of imidazolium ionic liquids for spectroscopic applications. Chem. Phys. Lett. 2005, 415, 131–136. [Google Scholar] [CrossRef]
- Schafer, T.; Paolo, R.E.D.; Franco, R.; Crespo, J.G. Elucidating interactions of ionic liquids with polymer films using confocal raman spectroscopy. Chem. Commun. 2005, 2594–2596. [Google Scholar]
- Runge, M.B.; Mwangi, M.T.; Miller, A.L.; Perring, M.; Bowden, N.B. Cascade reactions using lialh4 and grignard reagents in the presence of water. Angew. Chem. Int. Ed. 2008, 47, 935–939. [Google Scholar] [CrossRef] [PubMed]
- Kobayashi, S.; Akiyama, R. Renaissance of immobilized catalysts. New types of polymer-supported catalysts, “microencapsulated catalysts”, which enable environmentally benign and powerful high-throughput organic synthesis. Chem. Commun. 2003, 21, 449–460. [Google Scholar] [CrossRef]
- Yu, W.; Porosoff, M.D.; Chen, J.G. Review of pt-based bimetallic catalysis: From model surfaces to supported catalysts. Chem. Rev. 2012, 112, 5780–5817. [Google Scholar] [CrossRef] [PubMed]
- Mizuno, N.; Misono, M. Heterogeneous catalysis. Chem. Rev. 1998, 98, 199–218. [Google Scholar] [CrossRef] [PubMed]
- Zaera, F. Nanostructured materials for applications in heterogeneous catalysis. Chem. Soc. Rev. 2013, 42, 2746–2762. [Google Scholar] [CrossRef] [PubMed]
- La Sorella, G.; Strukul, G.; Scarso, A. Recent advances in catalysis in micellar media. Green Chem. 2015, 17, 644–683. [Google Scholar] [CrossRef]
- Orlich, B.; Schomäcker, R. Enzyme catalysis in reverse micelles. In History and Trends in Bioprocessing and Biotransformation; Dutta, N.N., Hammar, F., Haralampidis, K., Karanth, N.G., König, A., Krishna, S.H., Kunze, G., Nagy, E., Orlich, B., Osbourn, A.E., et al., Eds.; Springer: Berlin, Germany; Heidelberg, Germany, 2002; Volume 75, pp. 185–208. [Google Scholar]
- Zhang, Q.; Shu, X.-Z.; Lucas, J.M.; Toste, F.D.; Somorjai, G.A.; Alivisatos, A.P. Inorganic micelles as efficient and recyclable micellar catalysts. Nano Lett. 2014, 14, 379–383. [Google Scholar] [CrossRef] [PubMed]
- Gonzalez, R.D.; Lopez, T.; Gomez, R. Sol—Gel preparation of supported metal catalysts. Catal. Today 1997, 35, 293–317. [Google Scholar] [CrossRef]
- Reetz, M.T.; Tielmann, P.; Wiesenhöfer, W.; Könen, W.; Zonta, A. Second generation sol-gel encapsulated lipases: Robust heterogeneous biocatalysts. Adv. Synth. Catal. 2003, 345, 717–728. [Google Scholar] [CrossRef]
- Silva, C.R.; Airoldi, C. Acid and base catalysts in the hybrid silica sol–gel process. J. Colloid Interface Sci. 1997, 195, 381–387. [Google Scholar] [CrossRef] [PubMed]
- Ward, D.A.; Ko, E.I. Preparing catalytic materials by the sol-gel method. Ind. Eng. Chem. Res. 1995, 34, 421–433. [Google Scholar] [CrossRef]
- Astruc, D.; Lu, F.; Aranzaes, J.R. Nanoparticles as recyclable catalysts: The frontier between homogeneous and heterogeneous catalysis. Angew. Chem. Int. Ed. 2005, 44, 7852–7872. [Google Scholar] [CrossRef] [PubMed]
- Bania, K.K.; Deka, R.C. Zeolite-y encapsulated metal picolinato complexes as catalyst for oxidation of phenol with hydrogen peroxide. J. Phys. Chem. C 2013, 117, 11663–11678. [Google Scholar] [CrossRef]
- Chang, W.; Ahn, B.J. Catalytic organic reactions using mesostructured materials. Int. J. Nanotechnol. 2006, 3, 181–193. [Google Scholar] [CrossRef]
- Chassaing, S.; Kumarraja, M.; Pale, P.; Sommer, J. Zeolite-directed cascade reactions: Cycliacyarylation versus decarboxyarylation of α,β-unsaturated carboxylic acids. Org. Lett. 2007, 9, 3889–3892. [Google Scholar] [CrossRef] [PubMed]
- Derouane, E.G.; Chang, C.D. Confinement effects in the adsorption of simple bases by zeolites. Microporous Mesoporous Mater. 2000, 35–36, 425–433. [Google Scholar] [CrossRef]
- Derouane, E.G.; Dillon, C.J.; Bethell, D.; Derouane-Abd Hamid, S.B. Zeolite catalysts as solid solvents in fine chemicals synthesis: 1. Catalyst deactivation in the friedel–crafts acetylation of anisole. J. Catal. 1999, 187, 209–218. [Google Scholar] [CrossRef]
- Deshpande, S.; Srinivas, D.; Ratnasamy, P. Epr and catalytic investigation of cu(salen) complexes encapsulated in zeolites. J. Catal. 1999, 188, 261–269. [Google Scholar] [CrossRef]
- Heinrichs, C.; Hölderich, W. Novel zeolitic hosts for “ship-in-a-bottle” catalysts. Catal. Lett. 1999, 58, 75–80. [Google Scholar] [CrossRef]
- Herron, N.; Stucky, G.D.; Tolman, C.A. Shape selectivity in hydrocarbon oxidations using zeolite encapsulated iron phthalocyanine catalysts. J. Chem. Soc. Chem. Commun. 1986, 1521–1522. [Google Scholar] [CrossRef]
- Parton, R.F.; Vankelecom, I.F.J.; Tas, D.; Janssen, K.B.M.; Knops-Gerrits, P.-P.; Jacobs, P.A. Membrane occluded catalysts: A higher order mimic with improved performance. J. Mol. Catal. A Chem. 1996, 113, 283–292. [Google Scholar] [CrossRef]
- Mwangi, M.T.; Schulz, M.D.; Bowden, N.B. Sequential reactions with grubbs catalyst and ad-mix-alpha/beta using pdms thimbles. Org. Lett. 2009, 11, 33–36. [Google Scholar] [CrossRef] [PubMed]
- Hunsen, M. Carboxylic acids from primary alcohols and aldehydes by a pyridinium chlorochromate catalyzed oxidation. Synthesis 2005, 2487–2490. [Google Scholar] [CrossRef]
- Gunanathan, C.; Ben-David, Y.; Milstein, D. Direct synthesis of amides from alcohols and amines with liberation of h2. Science 2007, 317, 790–792. [Google Scholar] [CrossRef] [PubMed]



© 2016 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Çınar, S.; Schulz, M.D.; Oyola-Reynoso, S.; Bwambok, D.K.; Gathiaka, S.M.; Thuo, M. Application of Ionic Liquids in Pot-in-Pot Reactions. Molecules 2016, 21, 272. https://doi.org/10.3390/molecules21030272
Çınar S, Schulz MD, Oyola-Reynoso S, Bwambok DK, Gathiaka SM, Thuo M. Application of Ionic Liquids in Pot-in-Pot Reactions. Molecules. 2016; 21(3):272. https://doi.org/10.3390/molecules21030272
Chicago/Turabian StyleÇınar, Simge, Michael D. Schulz, Stephanie Oyola-Reynoso, David K. Bwambok, Symon M. Gathiaka, and Martin Thuo. 2016. "Application of Ionic Liquids in Pot-in-Pot Reactions" Molecules 21, no. 3: 272. https://doi.org/10.3390/molecules21030272
APA StyleÇınar, S., Schulz, M. D., Oyola-Reynoso, S., Bwambok, D. K., Gathiaka, S. M., & Thuo, M. (2016). Application of Ionic Liquids in Pot-in-Pot Reactions. Molecules, 21(3), 272. https://doi.org/10.3390/molecules21030272