On Optimization of a Non-Endorreversible Curzon-Ahlborn Cycle
Abstract
:1. Introduction
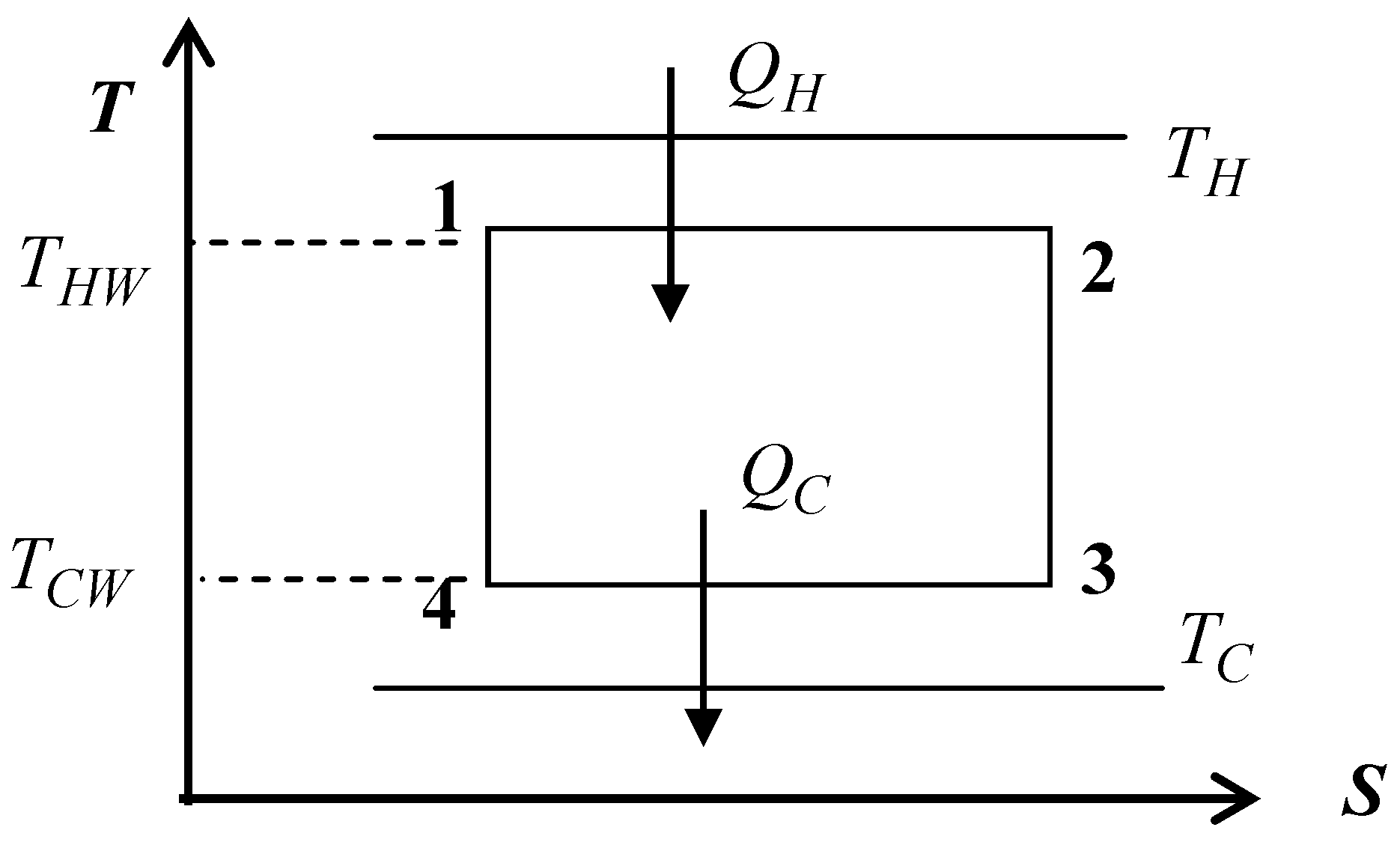
2. Power output and ecological function for instantaneous adiabats

3. Curzon and Ahlborn efficiency and ecological efficiency
| Power Plant | (K) | (K) | , | , | |
| Doel 4 (Belgium), 1985. | 283 | 566 | 0.297 to 0.357 | 0.35000 | 0.31535 to 0.3545 |
| Almaraz II (nuclear pressurized water reactor) Spain | 290 | 600 | 0.315 to 0.373 | 0.34500 | 0.3306 to 0.36889 |
| Sizewell B (nuclear pressurized water reactor) U. K. | 288 | 581 | 0.302 to 0.361 | 0.36300 | 0.3198 to 0.35821 |
| Cofrentes (nuclear boiling water reactor) Spain | 289 | 562 | 0.282 to 0.343 | 0.34000 | 0.30238 to 0.34228 |
| Heysham (nuclear advanced gas cooled reactor) U. K. | 288 | 727 | 0.410 to 0.460 | 0.40000 | 0.41206 to 0.44568 |
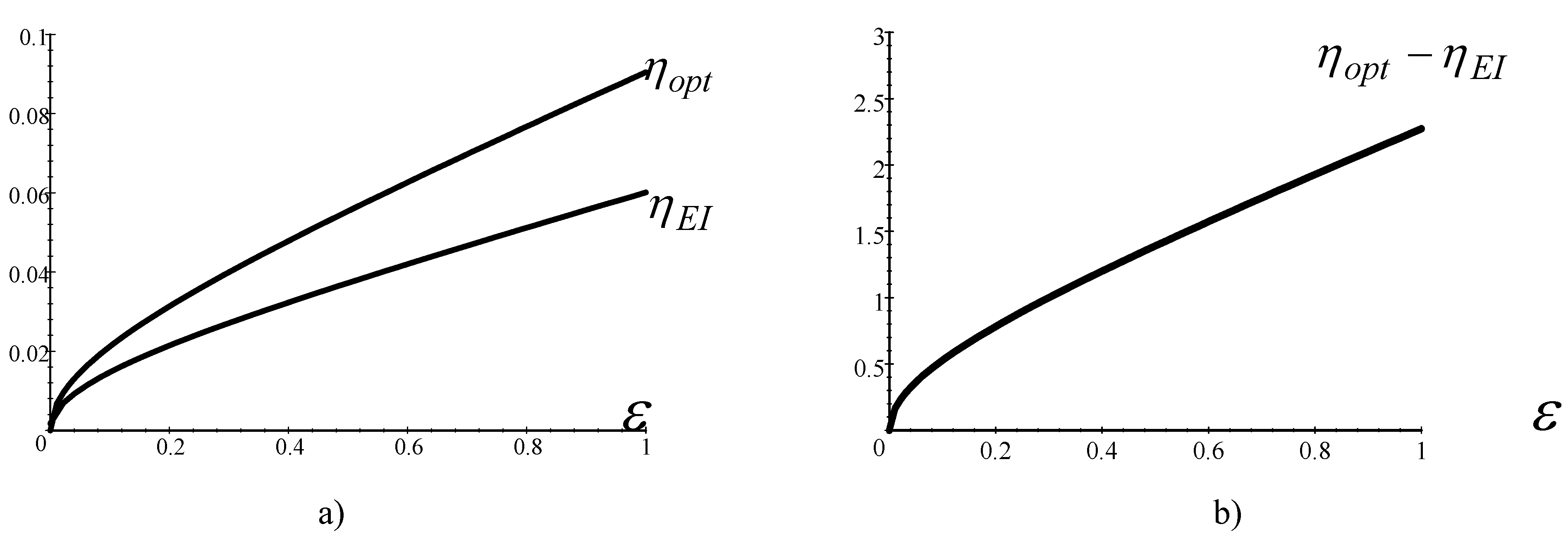
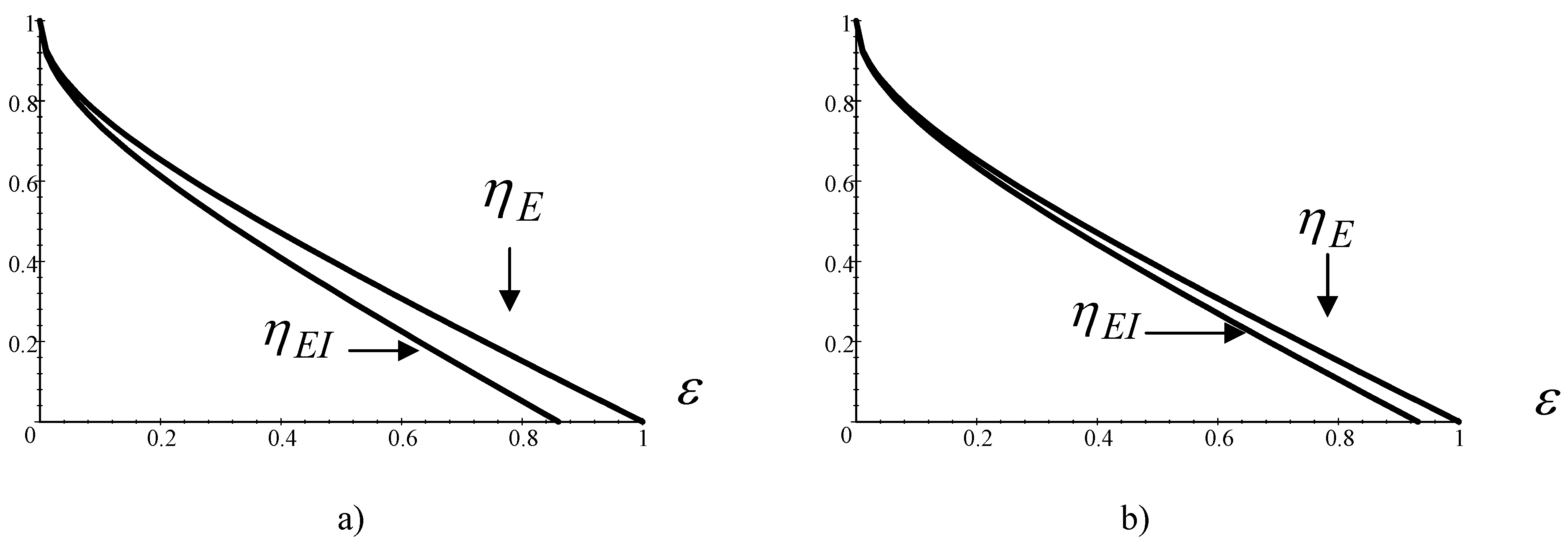
4. More general expressions for power output and for ecological function
5. Concluding remarks
Acknowledgements
References
- Curzon, F. L.; Ahlborn, B. Efficiency of a Carnot Engine at Maximum Power Output. Am. J. Phys. 1975, 43, 22–24. [Google Scholar] [CrossRef]
- Salamon, P.; Andresen, B.; Berry, R.S. Thermodynamics in Finite Time. I. Potencials for Finite-time processes. Phys. Rev. A 1976, 15, 2094–2101. [Google Scholar] [CrossRef]
- Rubin, M. Optimal Configuration of a Class of Irreversible Heat Engines. I. Phys. Rev. A 1979, 19, 1272–1276. [Google Scholar]Optimal Configuration of a Class of Irreversible Heat Engines. II. Phys. Rev. A 1979, 19, 1277–1288.Optimal Configuration of an Irreversible Heat Engine with Fixed Compression Ratio. Phys. Rev. A 1980, 22, 1741–1752.
- De Vos, A. Efficiency of some Heat Engines at Maximum-power Conditions. Am. J. Phys. 1985, 53, 570–573. [Google Scholar]
- Chen, J.; Yan, Z. Unified Description of Endoreversible Cycles. Phys. Rev. A 1989, 39, 4140–4147. [Google Scholar] [CrossRef] [PubMed]
- Yan, Z.; Chen, J. A Class of Irreversible Carnot Refrigeration Cycles with a General heat Transfer Law. J. Phys D: Appl. Phys. 1990, 23, 136–141. [Google Scholar] [CrossRef]
- Rebhan, E. Efficiency of Nonideal Carnot Engines with Friction and Heat Losses. Am. J. Phys. 2002, 70, 1143–1149. [Google Scholar] [CrossRef]
- Bejan, A. Entropy Generation Minimization: The New Thermodynamics of Finite-size Devices and Finite-time processes. J. Appl. Phys. 1996, 79, 1191–1218. [Google Scholar] [CrossRef]
- Gutkowics-Krusin, D.; Procaccia, I.; Ross, J. On the Efficiency of Rate Processes. Power and Effiency of Heat Engines. J. Chem. Phys. 1978, 69, 3898–3906. [Google Scholar] [CrossRef]
- Badescu, V. Optimal paths for minimizing lost avilable work during usual heat transferprocess. J. Non-Equilib. Thermodyn. 2004, 29, 53–73. [Google Scholar] [CrossRef]
- Amelkin, S.A.; Andresen, B.; Burzler, J.M.; Hoffman, K.H.; Tsirlin, A.M. Maximum power processfor multi-source endoreversible heat engines. J. Phys. D: Appl. Phys. 2004, 37, 1400–1404. [Google Scholar] [CrossRef]
- Amelkin, S.A.; Andresen, B.; Burzler, J.M.; Hoffman, K.H.; Tsirlin, A.M. Thermo-mechanical systems with several heat reservoirs: maximum power processes. J. Non-Equilib. Thermodyn. 2005, 30, 67–80. [Google Scholar] [CrossRef]
- Song, H.; Chen, L.; Sun, F.; Wu, C. Optimal configuraction of a clas of endoreversibleheat engines with linear phenomenological heat transfer law [q ∝ ∆(T−1)]. J. Appl. Phys. 2006, 100, 124907. [Google Scholar] [CrossRef]
- Song, H.; Chen, L.; Sun, F. Endoreversible heat engines for maximum power output with fixed duration and radiative heat transfer law. Applied Energy 2007, 84, 374–388. [Google Scholar] [CrossRef]
- Ladino-Luna, D.; de la Selva, S.M.T. The Ecological Efficiency of a Thermal Finite Time Engine. Rev. Mex. Fís. 2000, 46, 52–56. [Google Scholar]
- Ladino-Luna, D. Van der Waals gas as working substance in a Curzon and Ahlborn-Novikov engine. Entropy 2005, 7, 108–121. [Google Scholar] [CrossRef]Ladino-Luna, D. Efficiency of a Curzon and Ahlborn engine with Dulong-Petit heat transfer law. Rev. Mex. Fís. 2003, 49, 87–91. [Google Scholar] [CrossRef]
- Angulo-Brown, F. An Ecological Optimization Criterion for Finite-time Heat Engines. J. Appl. Phys. 1991, 69, 7465–7469. [Google Scholar] [CrossRef]
- Yan, Z. Commente on “Ecological optimization criterion for finite time engines”. J. Appl. Phys. 1993, 73, 3583. [Google Scholar] [CrossRef]
- Angulo-Brown, F.; Árias-Hernández, F.A.; Páez-Hernández, R.T. A General Property of Non-endoreversible Thermal Cycles. J. Phys. D: Appl. Phys. 1999, 32, 1415–1420. [Google Scholar]
- Wu, C.; Kiang, R.L. Finite-time thermodynamic analisys of a Carnot engine with internal irreversibility. Int. J. Energy 1992, 17, 1173–1178. [Google Scholar] [CrossRef]
- Chen, J. The Maximum Power Output and Maximum Efficiency of an Irreversible Carnot Heat Engine. J. Phys. D: Appl. Phys. 1994, 27, 1144–1149. [Google Scholar]The Efficiency of an Irreversible Combined Cycle at Maximum Specific Power Output. J. Phys. D: Appl. Phys. 1996, 29, 2818–2822.
- Chen, L.; Zhou, J.; Sun, F.; Wu, C. Ecological optimization of generalized irreversible Carnot engines. Applied Energy 2004, 77, 327–338. [Google Scholar] [CrossRef]
- Chen, L.; Zhu, X.; Sun, F.; Wu, C. Exergy-based ecological optimization of linear phenomenological heat transfer law irreversible Carnot engines. Applied Energy 2006, 83, 573–582. [Google Scholar] [CrossRef]
- Zhu, X.; Chen, L.; Sun, F.; Wu, C. The ecological optimization of generalized irreversible Carnot engine with generalized heat transfer law. Int. J. Ambient Energy 2003, 24, 189–194. [Google Scholar]
- Zhu, X.; Chen, L.; .Sun, F.; Wu, C. Effect of heat transfer law on the ecological optimization of a generalized irreversible Carnot engine. Open System & Information Dynamics 2005, 12, 249–260. [Google Scholar]
- Chen, L.; Zhang, W.; Sun, F. Power, efficiency, entropy generation rate and ecological optimization for a class of generalized universal heat engine cycles. Applied Energy 2007, 84, 512–525. [Google Scholar] [CrossRef]
- Árias-Hernández, L.A.; Angulo-Brown, F. A General Property of Endoreversible Termal Engines. J. Appl. Phys. 1997, 81, 2973–2979. [Google Scholar] [CrossRef]
- Velasco, S.; Roco, J.M.M.; Medina, A.; White, J.A.; Calvo-Hernández, A. Optimization of Heat Engines Including the Saving of Natural Resources and the Reduction of Termal Pollution. J. Phys. D: Appl. Phys. 2000, 33, 355–359. [Google Scholar]
- Angulo-Brown, F.; Ares de Parga, G.; Arias-Hernández, L.A. A Variational Approach to Ecological-type Optimization Criteria for Finite-time Termal Engine Models. J. Phys. D: Appl. Phys. 2002, 35, 1089–1093. [Google Scholar]
© 2007 by MDPI (http://www.mdpi.org). Reproduction is permitted for noncommercial purposes.
Share and Cite
Ladino-Luna, D. On Optimization of a Non-Endorreversible Curzon-Ahlborn Cycle. Entropy 2007, 9, 186-197. https://doi.org/10.3390/e9040186
Ladino-Luna D. On Optimization of a Non-Endorreversible Curzon-Ahlborn Cycle. Entropy. 2007; 9(4):186-197. https://doi.org/10.3390/e9040186
Chicago/Turabian StyleLadino-Luna, Delfino. 2007. "On Optimization of a Non-Endorreversible Curzon-Ahlborn Cycle" Entropy 9, no. 4: 186-197. https://doi.org/10.3390/e9040186
APA StyleLadino-Luna, D. (2007). On Optimization of a Non-Endorreversible Curzon-Ahlborn Cycle. Entropy, 9(4), 186-197. https://doi.org/10.3390/e9040186




