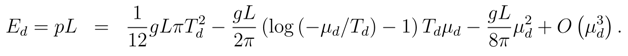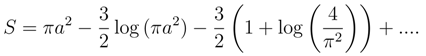1. Introduction
There is an intriguing analogy between gravitational dynamics of horizons and thermodynamics (for a recent review, see ref. [
1]). We do not have fundamental understanding of this though most people believe this indicates a deep and as yet undiscovered aspect of quantum gravity. The first results, of course, were that of black hole horizon [
2], Rindler horizon [
3] and De-Sitter horizon [
4]. In the case of these (and other horizons) one can associate a temperature fairly unambiguously. In the case of blackhole horizon, one also associates an entropy. In the case of Rindler and De-Sitter horizon, the observer dependence of the horizon makes people uneasy as regard associating entropy to the horizon (and most people try not to take a clear stand on this matter!). On the other hand there are strong arguments suggesting
all horizons have thermodynamic variables associated with them and entropy of horizons
is an observer dependent construct [
5]. If so, one can associate thermodynamic laws with all horizons and – in fact – this appears to offer an entirely new perspective on gravity [
6,
7].
In conventional systems, one can derive the laws of thermodynamics from a more fundamental theory — statistical mechanics. In such systems, entropy can be defined as the logarithm of total number of accessible microstates corresponding to the same macrostate. The existence of an entropy associated with any horizon provides a strong motivation for one to look for certain microstates of the underlying quantum theory. Although there have been several attempts to derive black hole entropy formula from counting the possible microstates in both string and loop formalisms, a comprehensive understanding of this issue remain elusive.
Given this state of affairs, there is justification to study various aspects of the horizon thermodynamics and try to construct phenomenological models based on them. Recently, Balazs et. al.[
8] has proposed an intriguing model in which they have considered a “dual thermodynamics” corresponding to isolated Schwarzschild black hole and have tried to obtain the entropy from a dual theory, to which standard statistical mechanics is applicable. They show that the standard (black hole) thermodynamic relations are invariant under the transformations
E′ → A/4,
S′ → M, and
T′ → T−1 where
A,
M and
T are the horizon area, mass and Hawking temperature of a black hole and
E′,
S′ and
T′ are the energy, entropy and temperature of the corresponding dual quantum system [
8]. After working out the standard thermodynamics of the dual system, they apply the inverse transformations to get standard horizon thermodynamics as well as the logarithmic corrections to the original Bekenstein-Hawking entropy formula. This approach seem to provide a description of a strongly interacting gravitational system like black hole in terms of a weakly interacting quantum mechanical dual system.
The result is sufficiently intriguing that one would like to understand its origin and domain of applicability. In particular one would like to know whether it generalizes for an arbitrary horizon (like e.g., De-Sitter horizon, which will play an important role in the study of cosmological constant [
9]). We will address several aspects of this question in this paper in an attempt to understand this result.
Any attempt to generalize these result beyond the Schwarzschild blackhole raises an operational issue: While one can define the temperature for a sufficiently general class of horizons (Schwarschild, Reissner-Nordstrom, De-Sitter, Rindler .....), and even take entropy per unit transverse area to be (1
/4) (so that non-compact horizons are also taken care of) it is not easy to define the
energy associated with arbitrary horizons! Even for Reissner-Nordstrom blackhole there are different expressions for energy available in the literature (see e.g., [
10,
11] and references cited therein). Fortunately, for all spherically symmetric metrics, there is a natural way of defining this quantity. This is explained in detail in ref.[
6] and is essentially |
U| =
a/2 in geometric units where
a is the horizon radius. However, we shall show below that, with this definition, one can
not generalize the idea of duality to spherically symmetric spacetime in a consistent manner.
On the other hand, one can define another expression for energy
E which is the source of gravitational acceleration using the Tolman-Komar integral (see [
12,
19]). It was shown in ref.[
12] that this expression for energy also arises naturally in the case of any static horizon and allows one to obtain an “equation of state” between entropy, temperature and energy in the form
S =
E/2
T. Surprisingly, this result arises directly from the duality model! We shall now describe these results in detail and provide a brief discussion. Consider a static, spherically symmetric horizon, in the spacetime described by a metric (in which we have adopted natural units, with
ℏ =
c =
G = 1):
We assume that the function
f(
r) has a simple zero at
r =
a with finite
f′(
a) so that the spacetime has a horizon at
r =
a. Periodicity in Eulerian time allows us to associate a temperature
T =
f′(
a)
/4
π with the horizon. (Even for spacetimes with multi-horizons this prescription is locally valid for each horizon surface [
6,
13]). The Bekenstein-Hawking entropy is equal to the one quarter of the horizon area and therefore is given by,
These correspondences have been worked out in detail in [
6]; when
f′(
a) < 0, like in the case of De-Sitter, one needs to make appropriate sign changes, which is also explained in [
6]. For our purpose we will ignore these complications.
The real ambiguity is in giving a proper prescription for the energy — a well known problem in general relativity. For the Schwarzschild black hole it is obviously equal to the total mass
M , but for De-Sitter spacetime or even in the apparently simpler (asymptotically flat) case of a charged black hole, the definition of energy is non-trivial. We will handle this difficulty by not assuming a-priori any prescription for energy
E; instead we will find its generic form if the duality principle is valid. Now, the duality transformation considered by in ref.[
8] is ,
Where
µ is the chemical potential. Such a transformation will preserve the form of the first law of thermodynamics for both the black hole and the dual system. If we assume its validity and map a general spherically symmetric horizon to its dual system, we get the expressions for energy, entropy and temperature for the dual system to be:
(Note that we are not assuming any specific form for the energy
E of the horizon.) Taking the dual system as a one dimensional Bose gas, the expressions for energy and entropy are given by [
8],
(One can easily perform all the calculations for the Fermi gas.) The coefficient
g is the internal degrees of freedom of the gas particles and
L is the size of the system. Consistency requires that the leading order term of the entropy of the dual system gives correct value of of the black hole energy under the reverse transformation. This fixes the value of the quantity
gL is uniquely as
gL = 3
Ef′(
a)
/2
π2, With this value of
gL, the
leading term in the expression of energy
Ed of the dual system becomes,
If under a reverse transformation this expression gives the correct black hole entropy, one must have,
πa2 = 2
Eπ/f′(
a) thereby allowing us to determine the energy associated with the horizon
The above analysis is exactly the same as the one performed in ref.[
8] but for general context, keeping
E unspecified but
S =
πa2,
T =
f′(
a)
/4
π. We stress that we have not done anything drastic or unconventional except to adhere to the duality prescription.
For Schwarzschild spacetime
a = 2
M , and
f′(
a) = 1
/2
M and the above equation will give correct value of energy
E =
M if we have
af′(
a) = 1. This condition is trivially satisfied for Schwarzschild blackhole. This is essentially the result of [
8].
Let us next try Reissner-Nordstrom blackhole. Then
f (
r) = (1 − 2
M/r +
Q2/r2) and the horizon is at
. The temperature associated with this outer horizon is
T =
κ/2
π where
κ is the surface gravity of the outer horizon. In this case it is easy to see that
af′(
a) = 2
M/a − 2
Q2/a2 (so that
af′(
a) ≠ 1 unless
Q = 0). If we require this analysis to be applicable for charged black holes also, the energy
E should be given by Eq. (8):
In the literature several expressions are given for energy of Reissner-Nordstrom metric and this one corresponds to Moller energy for Reissner-Nordstrom black hole [
10,
11]. However, since our motivation is to study the thermodynamics of the horizons, any definition of energy should come from a thermodynamic consideration. But the expression of the energy in Eq. (9) does not agree with this prescription. For example, if we drop an uncharged particle of mass
M into a Reissner-Nordstrom blackhole, its energy should change by
dM and the resulting change in entropy should be related to this by
dE =
TdS. But if we start with the expression of energy in Eq. (9), then the change in mass will result in a change in energy
. On the other hand
TdS = (
M/a −
Q2/a2)
da =
dM which does not match
dE. Therefore the energy expression in Eq. (9)
does not have a proper thermodynamic interpretation.
There is, however, another interesting interpretation possible for this result, which is based on the formalism developed in ref.[
12]. We shall briefly recall this result, which is based on the point of view that the entropy associated with any horizon is due to the information which are hidden by the horizon. In order to formulate this idea mathematically, we need to set up the geometrical framework which is adapted to a congruence of observers who sees a horizon. The metric of a static spacetime can always be put in the form
ds2 = −
N2dt2 +
γµνdxµdxν, where
N and
γµν are independent of time
t (Greek indices cover 1,2,3 and Latin indices cover 0-3). The comoving observer at
xµ =constant have the four velocity
and the four acceleration
ai = (0,
∂µN/N). If
N → 0 on a two-surface and
Na = (
γµν∂µN∂νN)
1/2 is finite (say
κ, the surface gravity), then the coordinate system has a horizon. Regularity in the Euclidean sector requires the periodicity in Euclidean time with the period |
β| = 2
π/
κ, allowing us to define a temperature
T = |
β−1| in terms of the derivatives of
N, whenever there is a horizon. The expression for entropy associated with this horizon is given as [
12],
Obviously, the entropy defined by the above expression depends on the choice of the congruence, through the four vector
ui, but is
generally covariant. (For justification behind this definition of gravitational entropy, see ref.[
12]). Now, in any spacetime, there is a differential geometric identity [
14],
where
Kab is the extrinsic curvature of spatial hypersurfaces and
K is its trace. In static spacetime we have
Kab = 0 and when combined with Einstein’s equation we can write:
This equation relates the integrand of Eq. (10) to the matter stress tensor
Tab. We next note that the source for gravitational acceleration is the covariant combination
, and the corresponding energy
E is given by Tolman-Komar integral [
19],
From Eq. (10), Eq. (8) and Eq. (12), we can easily find that,
A closer inspection of the Eq. (8) and Eq. (2) reveals that there exits a exactly the same relationship between entropy
S and energy
E defined by the dual system approach!
All these results can be easily extended to a general
D dimension by noting the fact even in
D dimension the entropy is still one quarter of the area of the black hole horizon and therefore for a
D dimensional spherically symmetric spacetime , the entropy of the horizon is given by,
where
AD−2 is the area of a (
D − 2) dimensional unit sphere. The temperature of the horizon is still
T =
f′(
a)/4
π and let the relevant energy is
E(D). Then the dual transformations are,
Therefore for the dual system we have:
Taking the dual system as a one dimensional Bose gas, and using the expressions for energy and entropy in Eq. (6), it is straightforward to determine the energy associated with the horizon as,
This reduces to Eq. (8) for the case of
D = 4. On the other hand, for the case of general
D dimension the entropy definition in Eq. (10) should be modified as,
The differential identity in Eq. (11) is valid in any dimension. Therefore we will have in
D dimensions,
The Komar integral in
D dimension is,
where
dSαβ is the surface element in (
D − 2) dimensional hypersurface and
is the spacetime’s timelike killing vector. We also have Stokes theorem for antisymmetric tensor field
Bαβ given by [
14]
In our case
Bαβ = ∇
α . Then using killing equation we can write,
Now all killing vectors satisfies the identity
![Entropy 09 00100 i024]()
Using this identity along with Einstein equations, it is straightforward to prove that,
Therefore, ‘2’ factor in the front is universal and does not depend on the dimensions. Hence from Eq. (12) and Eq. (24) we can easily show that the original relationship S = βE/2 prevails in case of general D dimensions also.
Therefore, the natural conclusion of our analysis is that, the generalization of this duality transformations in [
8] for any spherically symmetric horizon is
only possible when the entropy and the energy associated with the horizon are defined in accordance with ref.[
12]. That is, the energy-entropy dual- ity in general should be taken as a dual transformation between the
entropy S in Eq. (10) and energy E in Eq. (13), so that the relationship Eq. (14) will always be satisfied. This will ensure the consistency of the entire approach and give the correct value of the Bekenstein-Hawking entropy under reverse transformation.
For the sake of completeness we also calculate quantum corrections to the horizon entropy for a general spherically symmetric horizon, using corrections to the energy of the dual quantum gas. The energy of the Bose model is
Using
gL = 3
Ef′(
a)
/2
π2, and performing the reverse transformation, the entropy of the horizon is obtained as,
Quantum corrections to the entropy of various black holes were determined using the Cardy formula [
15,
17,
18]. In order to obtain the correct coefficient of the logarithmic quantum correction term [
16] we need to fix the value of
µ as,
With this choice of
µ the entropy of the horizon becomes,
For Schwarzschild case
a = 2
M , and we recover the correct expression for the black hole entropy with logarithmic quantum corrections as shown in [
8]. The actual value is, of course, obtained by adjusting the free parameter
µ in the theory and hence is not so important; but the fact that the nature of the corrections have the logarithmic form is interesting and is worthy of futher investigation. If the duality ideas are correct, these terms are also universal and is applicable to all horizons.
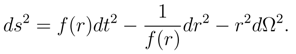

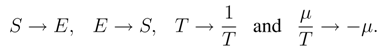







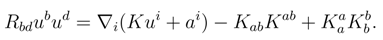
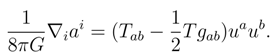




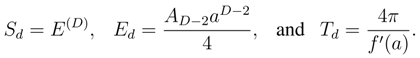
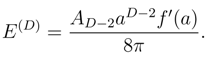

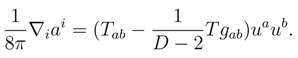


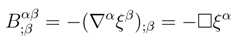
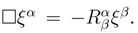 Using this identity along with Einstein equations, it is straightforward to prove that,
Using this identity along with Einstein equations, it is straightforward to prove that,