Entropy Production and Irreversible Processes -from the perspective of continuous topological evolution.
Abstract
:1 A Topological Perspective
1.1 The fundamental axioms
1.2 Notable Results
- Thermodynamics is a topological theory.
- Topological change is a necessary condition for thermodynamic irreversibility.
- When the Pfaff topological dimension of the 1-form of Action that encodes a physical system is 2, or less, the system is topologically isolated. The topological structure on domains of topological dimension n ≤ 2 never admit a continuous process which is thermodynamically irreversible. Non-equilibrium systems are of Pfaff topological dimension > 2.
- A 1-form of Action, A, with Pfaff topological dimension equal to 1, defines an equilibrium isolated system which has representation as a Lagrangian submanifold.
- The topological structure of physical systems on domains (contact manifolds) of odd topological dimension n = 3, 5, 7.. > 2 are non-equilibrium systems. On such systems there exists (to within a factor) a unique continuous extremal process, VE, which may be chaotic, but nevertheless is thermodynamically reversible, and has a Hamiltonian generator.
- The topological structure of physical systems on domains of even topological dimension n = 4, 6, 8... > 2 (symplectic manifolds) are non equilibrium systems. Such systems support (to within a factor) a unique continuous process, VT, related to the concept of Topological Torsion. Continuous evolution in the direction of the topological torsion vector is thermodynamically irreversible. In this sense, thermodynamic irreversibility is an artifact of topological dimension n ≥ 4.
- The change of the Pfaff topological dimension will produce topological defects and thermodynamic phase changes.
- The assumption of uniqueness of evolutionary solutions (which implies a Pfaff Topological dimension equal to 2 or less), and homeomorphic evolution, are different, but ubiquitous, constraints imposed upon classical mechanics that eliminate any time asymmetry.
- All Hamiltonian, Symplectic-Bernoulli and Helmholtz processes are thermodynamically reversible. In particular, the work 1-form, W, created by Hamiltonian processes is of Pfaff topological dimension 1 or less.
- The functional forms of tensor fields with arguments in terms of the base variables of the final state are not deterministically predictable in terms of the functional forms of tensor fields with arguments in terms of the base variables of the initial state, unless the map from initial to final state is a diffeomorphism (which preserves topology) [17] . On the other hand, the functional forms of those alternating tensor fields which are coefficients of exterior differential forms, and with arguments in terms of the base variables of the initial state, are well defined in terms of the functional forms of tensor fields with arguments in terms of the base variables of the final state, even when the (C1) map from initial to final state describes topological evolution. In other words, retrodiction of differential forms is possible when topology changes, but prediction is impossible. Hence an Arrow of Time asymmetry is a logical result [15] when topological evolution is admitted, but does not appear if the evolution is restricted to be a homeomorphism or a diffeomorphism, for then the topology is invariant.
- The topological structure of domains of Pfaff dimension 2 or less creates a connected, but not necessarily simply connected, topology. Evolutionary solution uniqueness is possible, to within a projective factor. Such a topological structure can be thought of as a topologically homogeneous object.
- The topological structure of domains of Pfaff dimension 3 or more creates a disconnected topology of multiple components. If solutions to a particular evolutionary problem exist, then the solutions are not unique. Envelope solutions, such as Huygen wavelets and propagating discontinuities (called signals) are classic examples of solution non-uniqueness. Such structures can be thought of as inhomogeneous topological objects.
- Cartan’s Magic formula, in terms of the Lie differential acting on exterior differential 1-forms establishes the long sought for combination of dynamics and thermodynamics, enabling non-equilibrium systems and many irreversible processes to be computed in terms of continuous topological evolution, without resort to probability theory and statistics. The technique goes beyond the usual constraints embedded in a variational principle.
- The Lie differential acting on differential forms is not necessarily the same as a linear affine covariant differential acting on differential forms. It is possible to demonstrate that if the process is locally adiabatic (no heat flow in the direction of the evolutionary process), then the Lie differential and the covariant differential can be made to coincide, as they both satisfy the Koszul axioms for an affine connection (see section 2.5). This is a surprising result, for, when the argument is reversed, the theorem implies that the ubiquitous affine covariant differential of tensor analysis, acting on a 1-form of Action, can always be cast into a form representing an adiabatic process. However, such adiabatic processes need not be reversible. Not all thermodynamic processes are adiabatic; the covariant derivative of tensor analysis does not capture such processes.
- The Lie differential can describe evolutionary processes which are not C2 differentiable, leading to a better understanding of wakes and shocks. On odd dimensional spaces, sequential C1 (translational) processes can be thermodynamically reversible, while intransitive C2 processes (rotation and expansion with a fixed point) will be thermodynamically irreversible.
- If the evolutionary process described by the Lie differential, affine equivalent or not, leaves the 1-form of Action invariant, then the process is thermodynamically reversible. If the affine covariant differential of tensor analysis induces parallel transport (the covariant differential is zero), then the affine process is adiabatic and reversible. Parallel transport does not encode irreversible processes.
- On spaces of Pfaff topological dimension 4, the Cayley-Hamilton theorem produces a characteristic polynomial with similarity invariant coefficients which will generate the format of the Gibbs function for a (universal) van der Waals gas, with a well defined critical point and binodal and spinodal lines. The same technique can be applied to dynamical systems. The fourth order polynomial indicates that the concept of phase change can be related to a 4th order "Higgs" potential.
1.3 Entropy and Topology

1.4 Topological Torsion
2 Fundamentals of non-equilibrium thermodynamics from a perspective of continuous topological evolution.
2.1 The Pfaff Topological Dimension
2.2 Physical Systems: Equilibrium, Isolated, Closed and Open
2.2.1 Connected Topology: Topological Torsion = AˆdA = 0
- Equilibrium physical systems are elements such that the Pfaff topological dimension is 1.
- Isolated physical systems are elements such that the Pfaff topological dimension is 2, or less [22]. Isolated systems of Pfaff dimension 2 need not be in equilibrium, but do not exchange radiation or mass with the environment.Systems : Pfaff topological dimension when: AˆdA = 0dA = 0 Equilibrium - Pfaff dimension 1AˆdA = 0 Isolated - Pfaff dimension 2Both systems have a connected topology, but these domains need not be simply connected.
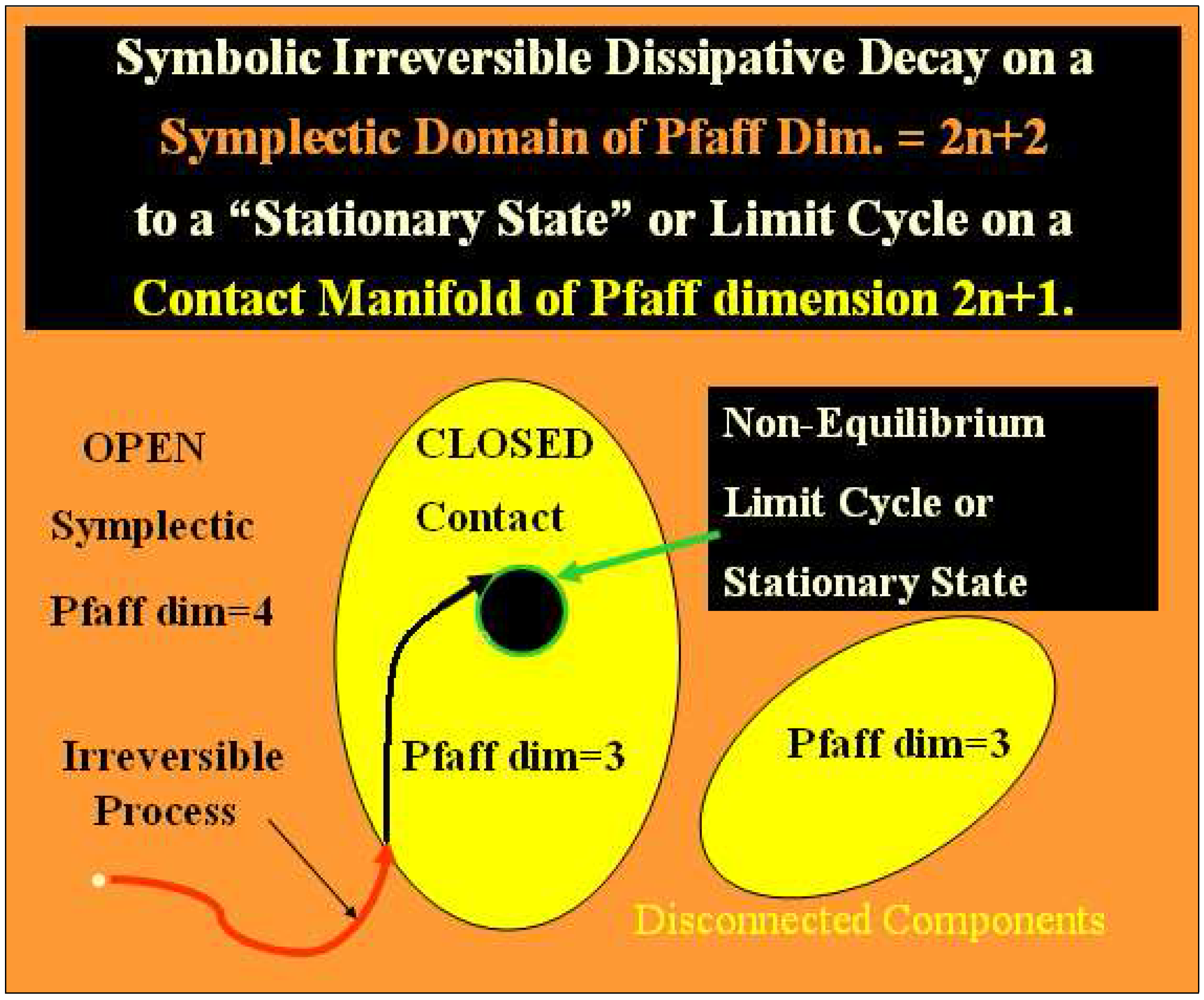
2.2.2 Disconnected Topology: Topological Torsion = AˆdA ≠ 0
- Closed physical systems are elements such that the Pfaff topological dimension is 3. Closed systems can exchange radiation, but not mass, with the environment.
- Open physical systems are such that the Pfaff topological dimension is 4. Open physical systems can exchange both radiation and mass with the environment.Systems : Pfaff topological dimension when: AˆdA ≠ 0d(AˆdA) = 0 Closed - Pfaff topological dimension 3dAˆdA ≠ 0. Open - Pfaff topological dimension 4.
2.3 Reversible and Irreversible Processes

2.4 A Non-Equilibrium Physical System
2.4.1 Topological Torsion
2.4.2 An Electromagnetic Example

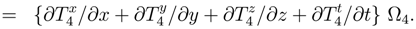
2.5 The Lie differential L(V) and the Covariant differential ∇(V)
3 The basic idea: Topological Fluctuations lead to a concept of an entropy production relative to continuous topological evolution.
3.1 Extensions of the Cartan-Hilbert Action 1-form
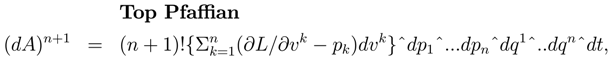
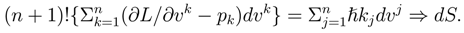

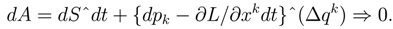

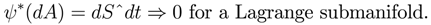
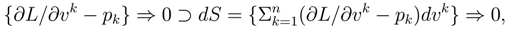
4 An Irreversible Example: The Sliding Bowling Ball
4.1 The Observation

4.2 The Analysis


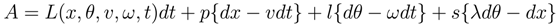

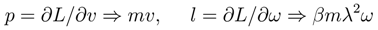

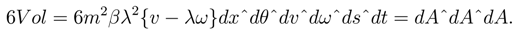

5 Summary
References
- Antonelli, P. L.; Ingarden, R.S.; Matsumoto, M. The Theory of Sprays and Finsler Spaces with applications to Biology and Physics; Kluwer: Dordrecht, Nd, 1993. [Google Scholar]
- Baldwin, P.; Kiehn, R. M. Cartan’s Topological Structure, Poster presented at the summer workshop. In Topology in Fluid Dynamics, Inst. for Th. Phy. UCSB, August 1991. Also see arXiv math-ph/0101033, or http://www22.pair.com/csdc/pdf/topstru4.pdf.
- Bamberg, P.; Sternberg, S. A Course in Mathematics for students of Physics, 2; Cambridge University press: Cambridge, 1992; pp. 775–780. [Google Scholar]
- Bowen, Rufus. Entropy for group endomorphisms and homogeneous spaces. Trans. Amer. Math. Soc. 1971, 153, 401–414, MR 43 #469. [Google Scholar] [CrossRef]
- Bott, R; Tu, L. W. Differential Forms in Algebraic Topology; Springer Verlag, N.Y, 1994. [Google Scholar]
- Cartan, E. Systems Differentials Exterieurs et leurs Applications Geometriques; Hermann: Paris, 1922. [Google Scholar]
- Cartan, E. Sur certaines expressions differentielles et le systeme de Pfaff. Ann Ec. Norm. 1899, 16, 329+. [Google Scholar]
- Chern, S. S. Annals of Math 1944, 45, 747–752, also Science Reports Nat. Tsing Hua Univ. 1948, 5; pp 95-121..
- Eckart, C. The thermodynamics of irreversible processes I: The simple fluid, II: Fluid mixtures, III: Relativistic theory of the simple fluid”. Phys. Rev 1940, 58. [Google Scholar]
- Flanders, H. Differential Forms; Academic Press: New York, 1963; p. 126. [Google Scholar]
- Forsyth, A.R. Theory of differential equations V1 and V2; Dover, N.Y., 1959. [Google Scholar]
- Goldenblat, I.I. Some problems of the mechanics of continuous media; Noordhoff: Holland, 1962; p. 193, “The quantity of heat in a reversible process always has an integrating factor”. [Google Scholar]
- Hermann, R. Differential Geometry and the Calculus of Variations. Academic Press: New York, 1968. [Google Scholar]
- Kiehn, R. M. D turbulence is a Myth, (invited speaker EGS XXIV General Assembly IUTAM, the Hague, 1999). http://www22.pair.com/csdc/pdf/hague6.pdf.
- Kiehn, R. M. Thermodynamic Irreversibility and the Arrow of Time. In The Nature of Time: Geometry, Physics and Perception; Bucher, R., et al., Eds.; Kluwer: Dordrecht, Netherland, 2003; pp. 243–250, (http://www22.pair.com/csdc/pdf/arwfinal.pdf). [Google Scholar]
- Kiehn, R. M. Topological evolution of classical electromagnetic fields and the photon. In Photon and Poincaré Group; Dvoeglazov, V., Ed.; Nova Science Publishers, Inc.: Commack, New York, 1999; pp. 246–262, ISBN 1-56072-718-7. Also see (http://www22.pair.com/csdc/pdf/photon5.pdf). [Google Scholar]
- Kiehn, R.M. Retrodictive Determinism. Int. J. of Eng. Sci. 1976, 4, 749+, (http://www22.pair.com/csdc/pdf/retrodic.pdf). [Google Scholar] [CrossRef]
- Kiehn, R. M. ...3.5 Mb). see the forthcoming book “Non-equilibrium thermodynamics and Irrversible Processes — from the Perspective of Topological Evolution” (pre-publication copy is available at http://www22.pair.com/csdc/download/topthermo.pdf.
- Kiehn, R. M. Some Closed Form Solutions to the Navier Stokes Equations, LANL arXiv/physics/0102002. 1991; (http://www22.pair.com/csdc/pdf/nvsol.pdf). [Google Scholar]
- Kiehn, R. M. Coherent Structures in Fluids are Topological Torsion Defects. In IUTAM Symposium on Simulation and Identification of Organized Structures in Flows; Sørensen, N., et al., Eds.; Kluwer Academic Publishers: Dordrecht, 1999; pp. 239–248, See (http://www22.pair.com/csdc/pdf/copen5.pdf). Presented at the IUTAMSIMFLO Conference at DTU, Denmark, May 25-29, (1997). [Google Scholar]
- Kiehn, R.M. Topological evolution of classical electromagnetic fields and the photon. In Photon and Poincaré Group; Dvoeglazov, V., Ed.; Nova Science Publishers, Inc.: Commack, New York, 1999; pp. 246–262, ISBN 1-56072-718-7. Also see (http://www22.pair.com/csdc/pdf/photon5.pdf). [Google Scholar]
- Lipschutz, S. General Topology; Schaum: New York, 1965; p. 88. [Google Scholar]
- Marsden, J.E.; Riatu, T. S. ntroduction to Mechanics and Symmetry; Springer-Verlag, 1994; p. 122. [Google Scholar]
- Martinet, J. On the Singularities of Differential Forms. Ann. Inst. Fourier, Grenoble 1970, 20(1), 95–178. [Google Scholar] [CrossRef]
- Morse, P. M. Thermal Physics, Benjamin, NY. 1964; 60.
- Pittie, H. V. Characteristic classes of Foliations; Res Notes in Math; Ditman: London, 1976. [Google Scholar]
- Libermann, P.; Marle, C.-M. Symplectic Geometry and Analytical Mechanics. Riedel, Dordrecht/Boston. 1987; 65. [Google Scholar]
- Rodriguez, C. C. From Euclid to Entropy. In Maximum Entropy and Bayesian Methods; Grandy, W. T., Jr., Ed.; Kluwer Academic Publishers, 1991; pp. 1–6. [Google Scholar]
- Schouten, J. A.; Van der Kulk, W. Pfaff’s Problem and its Generalizations; Oxford Clarendon Press, 1949. [Google Scholar]
- Abe, S. Tsallis entropy: How Unique? arXiv: cond-mat/0305087.
- Zhitomirski, M. Typical Singularities and Differential 1-forms and Pfaffian Equations, Translations of Mathematical Monographs, 113, AMS Rhode Island 1991.
- Ward, T. Valuations and Hyperbolicity in Dynamics, PROYDYN Summer School June-July 2001. Georg-August-University at Göttingen. http://www.mth.uea.ac.uk/~h720/lecture_notes/valuations.pdf.
- 1The term "Pfaff topological dimension" (instead of class) was introduced by the present author in order to emphasize the topological foundations of the concept.
- 2A direction field is defined by the components of a vector field which establish the "line of action" of the vector in a projective sense. An arbitrary factor times the direction field defines the same projective line of action, just reparameterized. In metric based situations, the arbitrary factor can be interpreted as a renormalization factor.
- 3The bold letter A represents the first 3 components of the 4 vector of potentials, with the order in agreement with the ordering of the independent variables. The letter A represents the 1-form of Action.
© CSDC Inc. CSDC gives MDPI (http://www.mdpi.org) the right to publish and distribute the article freely for non commercial purposes. CSDC retains the right to publish the article on the web and to use all or part of the contents in future publications created by or for CSDC.
Share and Cite
Kiehn, R.M. Entropy Production and Irreversible Processes -from the perspective of continuous topological evolution. Entropy 2004, 6, 262-292. https://doi.org/10.3390/e6030262
Kiehn RM. Entropy Production and Irreversible Processes -from the perspective of continuous topological evolution. Entropy. 2004; 6(3):262-292. https://doi.org/10.3390/e6030262
Chicago/Turabian StyleKiehn, Robert M. 2004. "Entropy Production and Irreversible Processes -from the perspective of continuous topological evolution." Entropy 6, no. 3: 262-292. https://doi.org/10.3390/e6030262
APA StyleKiehn, R. M. (2004). Entropy Production and Irreversible Processes -from the perspective of continuous topological evolution. Entropy, 6(3), 262-292. https://doi.org/10.3390/e6030262


