Transient Thermal Energy Harvesting at a Single Temperature Using Nonlinearity
Abstract
1. Introduction
2. Results and Discussion
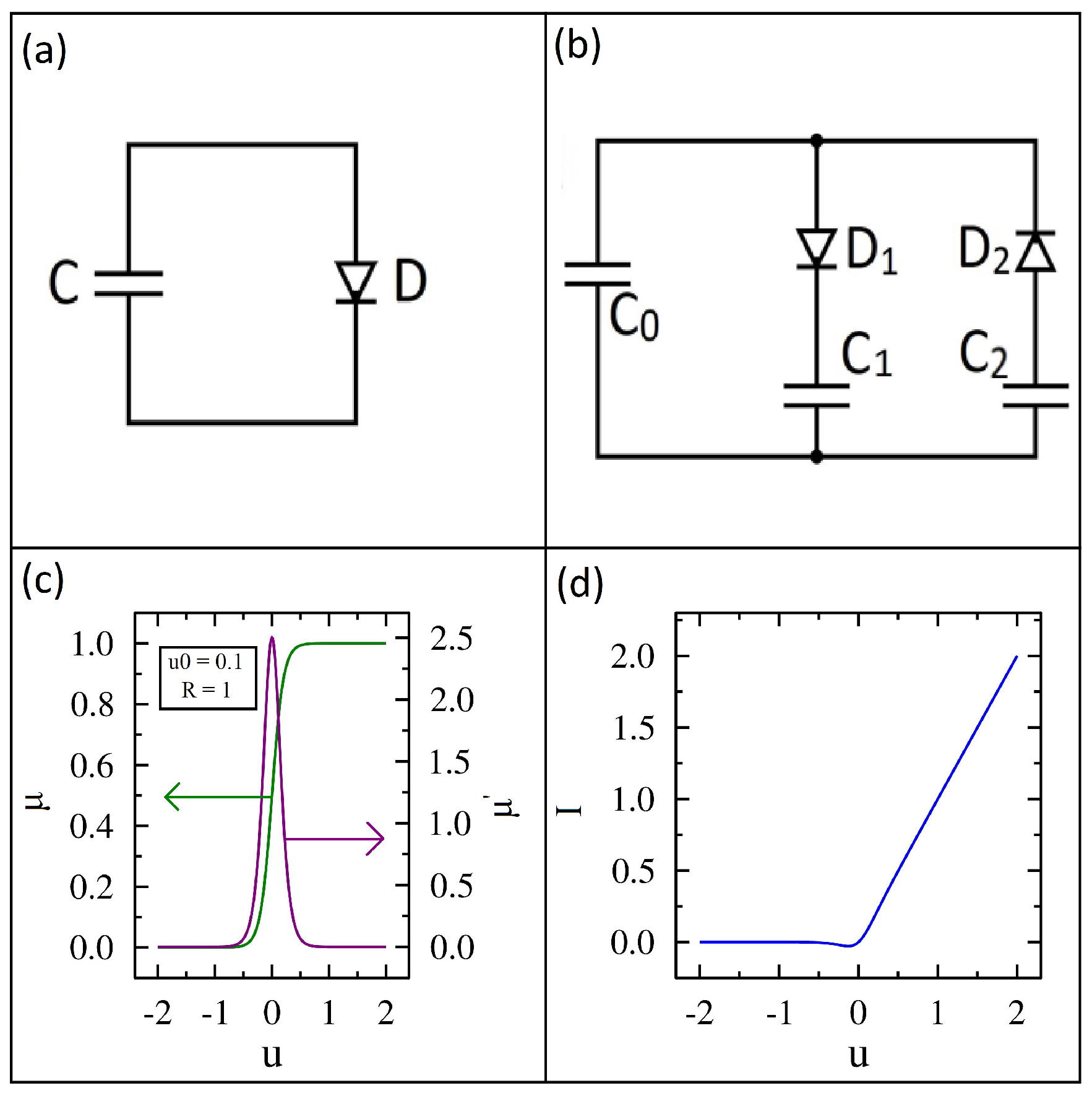
2.1. Single Loop Circuit
2.1.1. Differential Equations
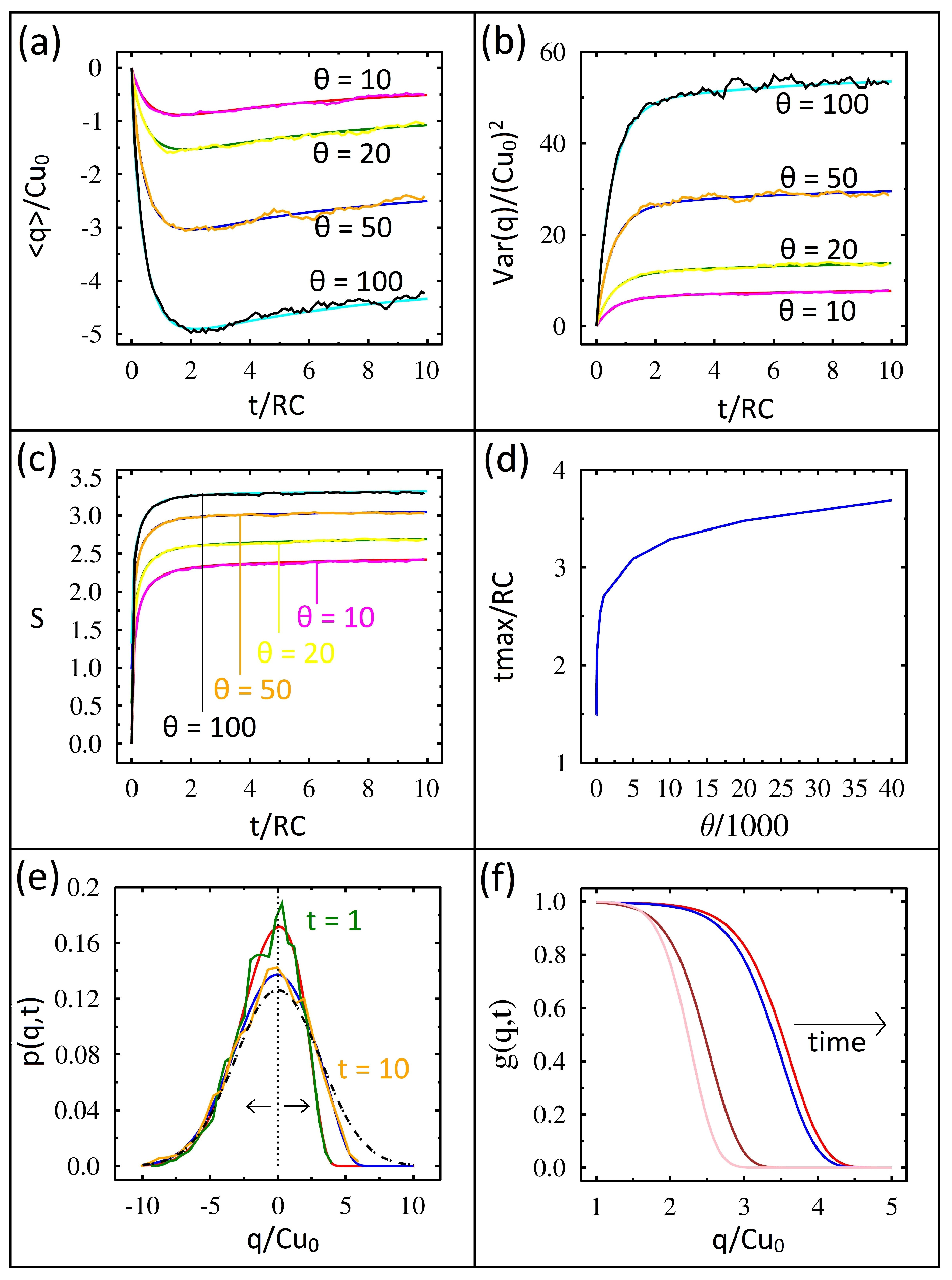
2.1.2. Simulations
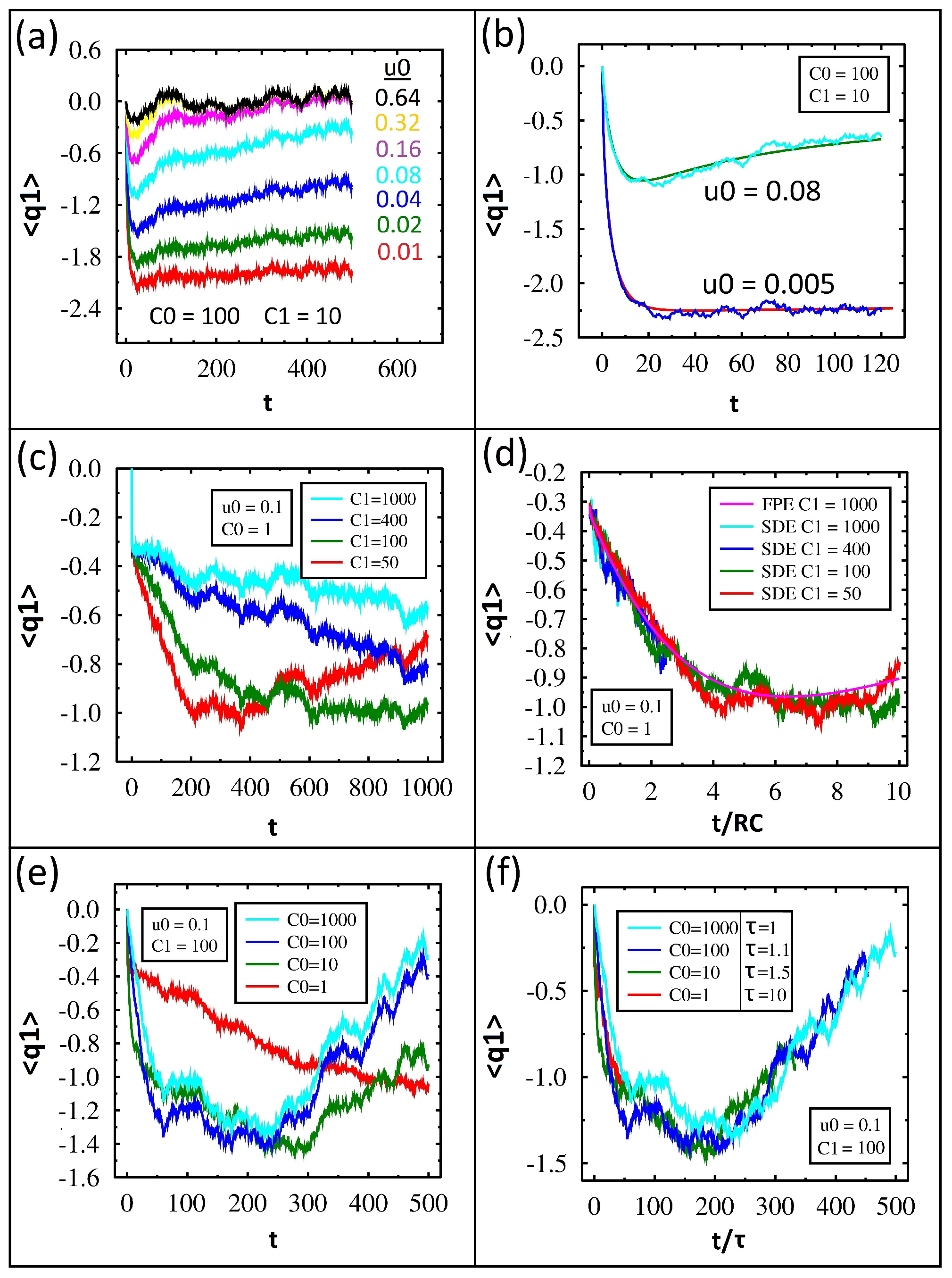
2.2. Two Loop Circuit
2.2.1. Differential Equations
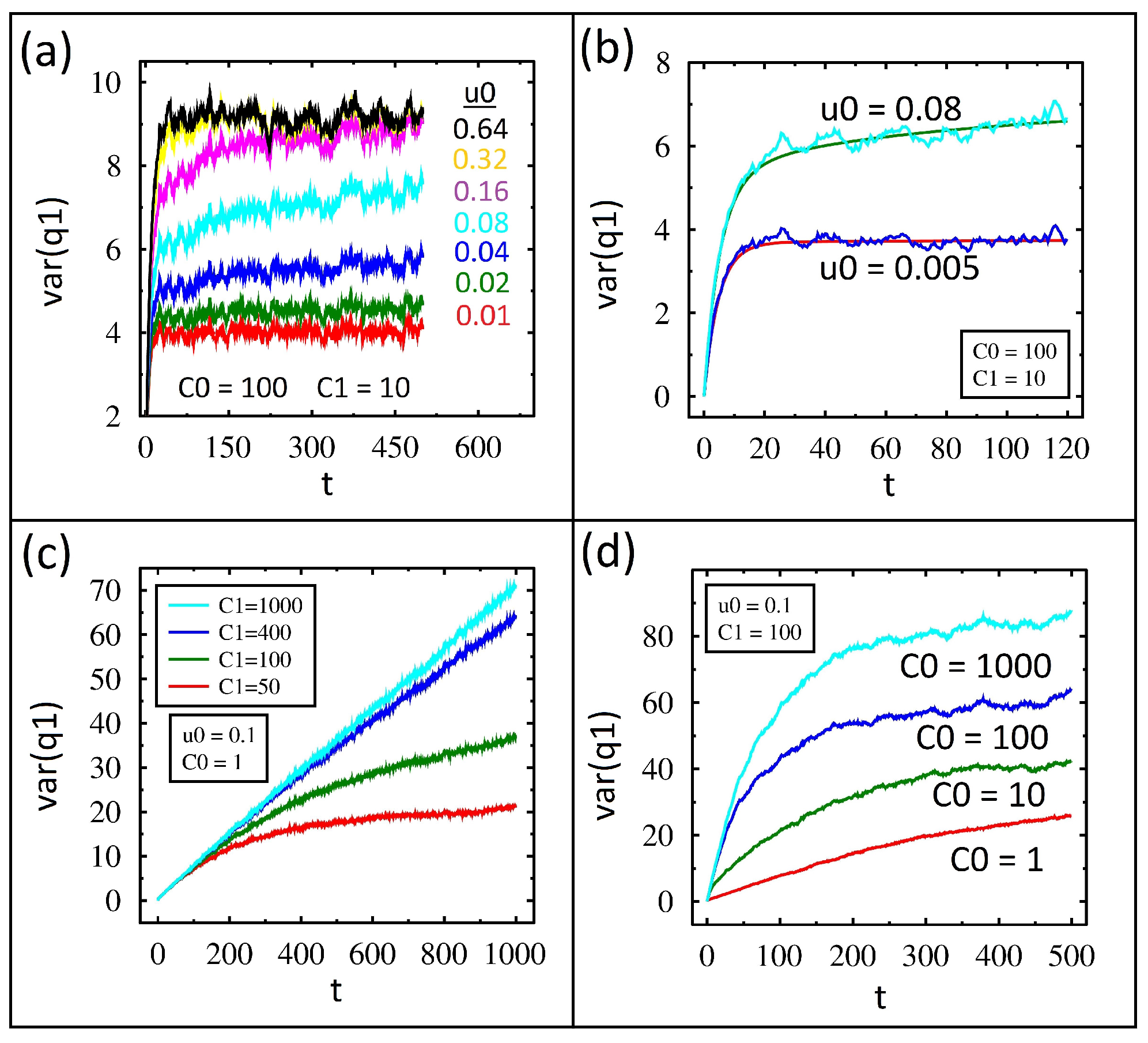
2.2.2. Simulations—Charge Variance
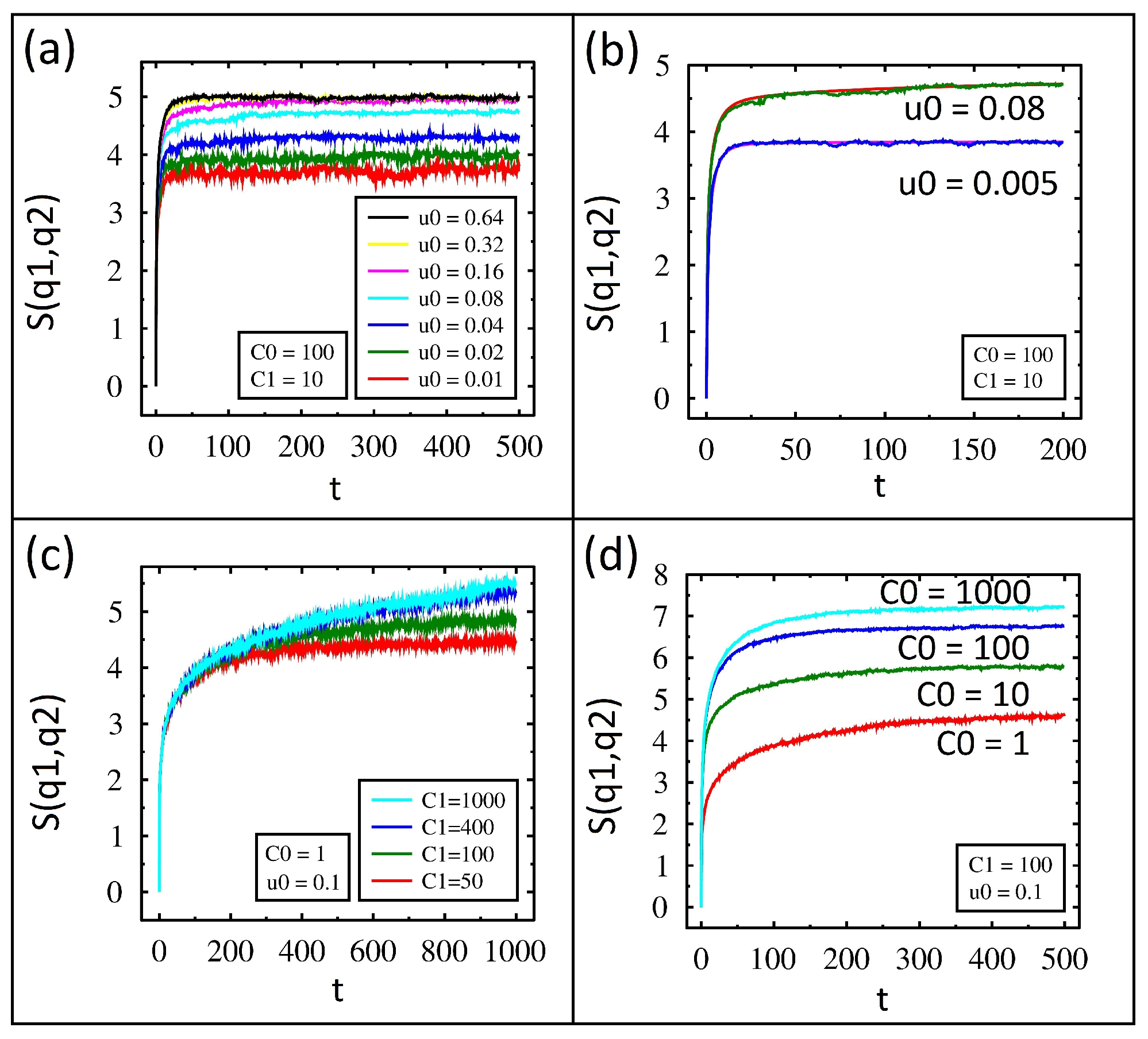
2.2.3. Simulations—Entropy
2.2.4. Simulations—Average Charge
3. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
References
- Banerjee, A.; Farhoudi, N.; Ghosh, C.; Mastrangelo, C.H.; Kim, H.; Broadbent, S.J.; Looper, R. Picowatt gas sensing and resistance switching in gunneling nano-gap electrodes. In Proceedings of the 2016 IEEE SENSORS, Orlando, FL, USA, 30 October–3 November 2016; pp. 1–3. [Google Scholar]
- Hanson, S.; Seok, M.; Lin, Y.-S.; Foo, Z.; Kim, D.; Lee, Y.; Liu, N.; Sylvester, D.; Blaauw, D. A low-voltage processor for sensing applications with picowatt standby mode. IEEE J. Solid-State Circuits 2009, 44, 1145–1155. [Google Scholar]
- Lee, Y.; Seok, M.; Sylvester, S.; Blaauw, D. Achieving ultralow standby power with an efficient SCCMOS bias generator. IEEE Trans. Circuits Syst. II Express Briefs 2013, 60, 842–846. [Google Scholar]
- Basu, J.; Ali, K.; Lin, L.; Alito, M. Picowatt-pwer analog gain stages in super-cutoff region with purely-harvested demonstration. IEEE-Solid-State Circuits Lett. 2022, 5, 226–229. [Google Scholar]
- Gupta, N.; Makosiej, A.; Anghel, C.; Amara, A.; Vladimirescu, A. CMOS sensor nodes with sub-picowatt TFET memory. IEEE Sens. J. 2016, 16, 8255–8262. [Google Scholar]
- Costanzo, L.; Schiavo, A.L.; Sarracino, A.; Vitelli, M. Stochastic thermodynamics of an electromagnetic energy harvester. Entropy 2024, 24, 1222. [Google Scholar]
- Johnson, J.B. Thermal agitation of electricity in conductors. Phys. Rev. 1928, 32, 97. [Google Scholar]
- Nyquist, H. Thermal agitation of electric charge in conductors. Phys. Rev. 1928, 32, 110. [Google Scholar] [CrossRef]
- Brillouin, L. Can the rectifier become a thermodynamical demon? Phys. Rev. 1950, 78, 627. [Google Scholar]
- Gunn, J.B. Thermodynamics of nonlinearity and noise in diodes. J. Appl. Phys. 1968, 39, 5357. [Google Scholar] [CrossRef]
- Gunn, J.B.; Staples, J.L. Spontaneous reverse current due to the Brillouin EMF in a diode. Appl. Phys. Lett. 1969, 14, 54. [Google Scholar]
- van Kampen, N.G. Derivation of the phenomenological equations from the master equation: I. Even variables only. Physica 1957, 23, 707–719. [Google Scholar]
- van Kampen, N.G. Non-linear thermal fluctuations in a diode. Physica 1960, 26, 585. [Google Scholar]
- Feynman, R.P.; Leighton, R.B.; Sands, M. The Feynman Lectures on Physics; Chapter 46; Addison-Wesley: Reading, MA, USA, 1966; Volume 1. [Google Scholar]
- Sokolov, I.M. On the energetics of a nonlinear system rectifying thermal fluctuations. Europhys. Lett. 1998, 44, 278. [Google Scholar] [CrossRef]
- Sokolov, I.M. Reversible fluctuation rectifier. Phys. Rev. E 1999, 60, 4946. [Google Scholar]
- Magnasco, M.O. Forced Thermal Ratchets. Phys. Rev. Lett. 1993, 71, 1477. [Google Scholar]
- Doering, C.R.; Horsthemke, W.; Riordan, J. Nonequilibrium fluctuation-induced transport. Phys. Rev. Lett. 1994, 72, 2984. [Google Scholar] [PubMed]
- Filliger, R.; Reimann, P. Brownian gyrator: A minimal heat engine on the nanoscale. Phys. Rev. Lett. 2007, 99, 230602. [Google Scholar]
- Chiang, K.-H.; Lee, C.-L.; Lai, P.-Y.; Chen, Y.-F. Electrical autonomous Brownian gyrator. Phys. Rev. E 2017, 96, 032123. [Google Scholar] [CrossRef]
- Gonzalez, J.P.; Neu, J.C.; Teitsworth, S.W. Experimental metrics for detection of detailed balance violation. Phys. Rev. E 2019, 99, 022143. [Google Scholar]
- Ackerman, M.L.; Kumar, P.; Neek-Amal, M.; Thibado, P.M.; Peeters, F.M.; Singh, S. Anomalous Dynamical Behavior of Freestanding Graphene Membranes. Phys. Rev. Lett. 2016, 117, 126801. [Google Scholar]
- Thibado, P.M.; Kumar, P.; Singh, S.; Ruiz-Garcia, M.; Lasanta, A.; Bonilla, L.L. Fluctuation-induced current from freestanding graphene. Phys. Rev. E 2020, 102, 042101. [Google Scholar] [CrossRef] [PubMed]
- Thibado, P.M.; Neu, J.C.; Kumar, P.; Singh, S.; Bonilla, L.L. Charging capacitors from thermal fluctuations using diodes. Phys. Rev. E 2023, 108, 024130. [Google Scholar] [CrossRef]
- Nguyen, V.H.; Kim, M.; Suleman, M.; Nasir, N.; Park, H.M.; Lee, S.; Elahi, E.; Noh, H.; Kumar, S.; Seo, Y. Thermal noise rectification with graphene. Nano Energy 2025, 136, 110687. [Google Scholar] [CrossRef]
- Fokker, A.D. Die mittlere Energie rotierender Elektrischer dipole im Strahlungsfeld. Ann. Phys. 1914, 348, 110. [Google Scholar] [CrossRef]
- Planck, V.M. Über Einen Satz der Statistischen Dynamik und Seine Erweiterung in der Quantentheorie; Reimer: Cleveland, OH, USA, 1917. [Google Scholar]
- Hjelmfelt, A.; Ross, J. Thermodynamics and stochastic theory of electrical circuits. Phys. Rev. A 1992, 45, 2201. [Google Scholar] [CrossRef] [PubMed]
- Sekimoto, K. Stochastic Energetics; Springer: New York, NY, USA, 2010. [Google Scholar]
- Seifert, U. Stochastic thermodynamics, fluctuation theorems and molecular machines. Rep. Prog. Phys. 2012, 75, 126001. [Google Scholar] [CrossRef]
- Durbin, J.; Mangum, J.M.; Gikunda, M.N.; Harerimana, F.; Amin, T.; Kumar, P.; Bonilla, L.L.; Thibado, P.M. Freestanding graphene heat engine analyzed using stochastic thermodynamics. AIP Adv. 2023, 13, 075217. [Google Scholar] [CrossRef]
- Sze, S.M. Physics of Semiconductor Devices, 2nd ed.; Wiley: New York, NY, USA, 1981. [Google Scholar]
- Gardiner, C.W. Stochastic Methods: A Handbook for the Natural and Social Sciences, 4th ed.; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
| 100 | 10 | 9 | 9 | 5 |
| 1 | 50 | 25 | 1 | 25 |
| 1 | 100 | 50 | 1 | 50 |
| 1 | 400 | 190 | 1 | 200 |
| 1 | 1000 | 490 | 1 | 500 |
| 1 | 100 | 50 | 1 | 50 |
| 10 | 100 | 52 | 9 | 50 |
| 100 | 100 | 66 | 50 | 50 |
| 1000 | 100 | 92 | 91 | 50 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amin, T.B.; Mangum, J.M.; Kabir, M.R.; Rahman, S.M.; Ashaduzzaman; Kumar, P.; Bonilla, L.L.; Thibado, P.M. Transient Thermal Energy Harvesting at a Single Temperature Using Nonlinearity. Entropy 2025, 27, 374. https://doi.org/10.3390/e27040374
Amin TB, Mangum JM, Kabir MR, Rahman SM, Ashaduzzaman, Kumar P, Bonilla LL, Thibado PM. Transient Thermal Energy Harvesting at a Single Temperature Using Nonlinearity. Entropy. 2025; 27(4):374. https://doi.org/10.3390/e27040374
Chicago/Turabian StyleAmin, Tamzeed B., James M. Mangum, Md R. Kabir, Syed M. Rahman, Ashaduzzaman, Pradeep Kumar, Luis L. Bonilla, and Paul M. Thibado. 2025. "Transient Thermal Energy Harvesting at a Single Temperature Using Nonlinearity" Entropy 27, no. 4: 374. https://doi.org/10.3390/e27040374
APA StyleAmin, T. B., Mangum, J. M., Kabir, M. R., Rahman, S. M., Ashaduzzaman, Kumar, P., Bonilla, L. L., & Thibado, P. M. (2025). Transient Thermal Energy Harvesting at a Single Temperature Using Nonlinearity. Entropy, 27(4), 374. https://doi.org/10.3390/e27040374









