On the Resistance Coefficients for Heat Conduction in Anisotropic Bodies at the Limit of Linear Extended Thermodynamics
Abstract
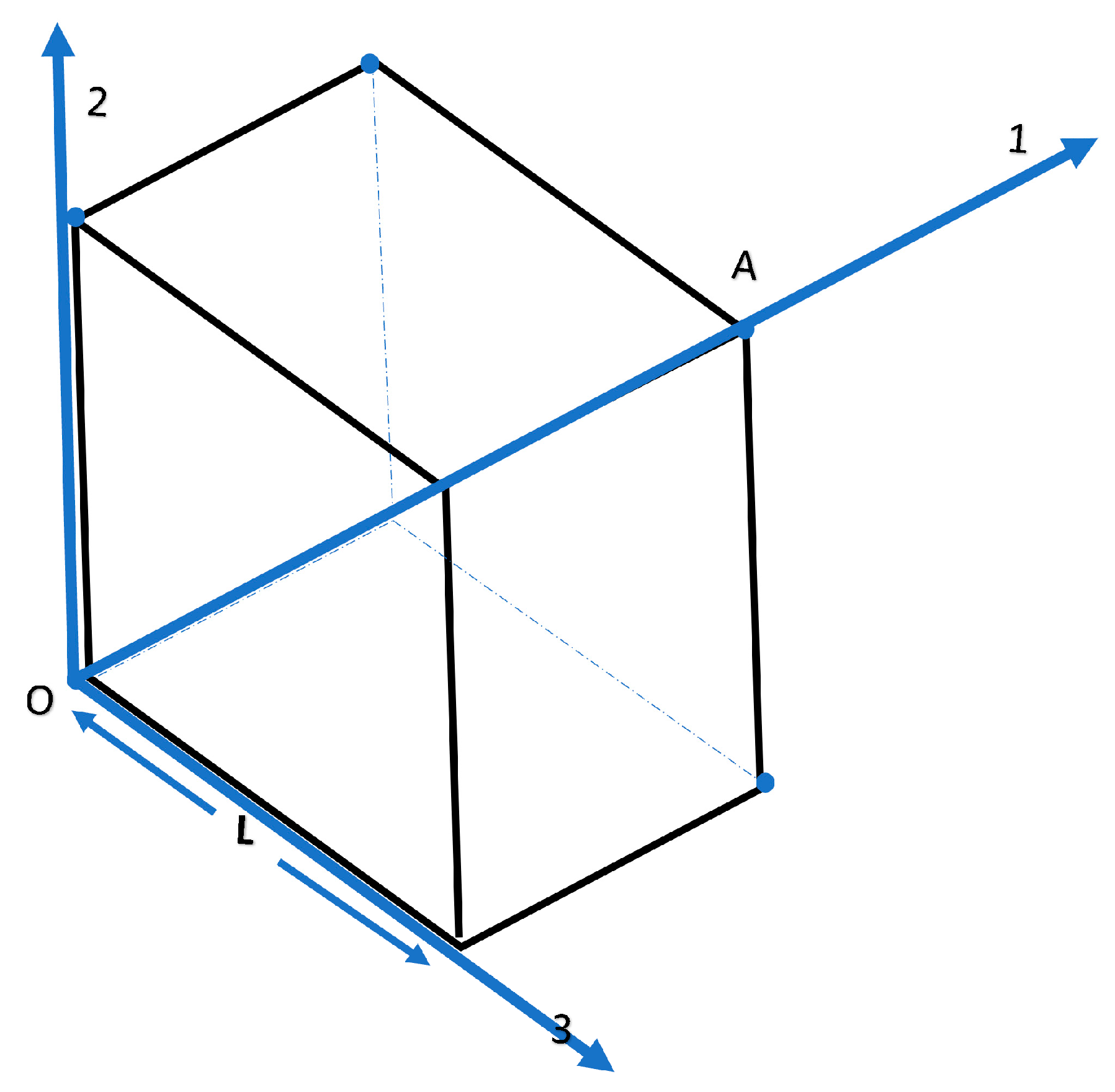
1. Introduction
2. Theoretical Section
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| adi | auxiliary parameter of the system |
| ci | molar concentration |
| h | overall heat transfer coefficient |
| hloc | close to equilibrium local heat transfer coefficients |
| hres | residual heat transfer coefficients |
| molar flux of the i-th substance relative to the velocity of the centre of mass | |
| Jq | heat flux |
| K | thermal conductivity coefficient |
| Li | distance between the point where T0j is located and the point having |
| temperature T. | |
| R | resistance coefficients |
| T | absolute temperature |
| T0 | reference absolute temperature |
| t | time |
| vi | velocity of the i-th substance |
| xj | space coordinate |
| X | thermodynamic driving force |
| w | weighting factors |
| Greek Letters | |
| δ | unit vector |
| λi | auxiliary parameter |
| chemical potential of the i-th substance | |
| σ | entropy production rate per unit volume |
| Τ | relaxation time. |
| Subscripts | |
| d | diffusion |
References
- Li, S.-N.; Cao, B.-Y. Generalized variational principles for heat conduction models based on Laplace transform. Int. J. Heat Mass Transf. 2016, 103, 1176–1180. [Google Scholar]
- Li, S.-N.; Cao, B.-Y. On Entropic Framework Based on Standard and Fractional Phonon Boltzmann Transport Equations. Entropy 2019, 21, 204. [Google Scholar] [CrossRef] [PubMed]
- Parker, M.C.; Jeynes, C. A Relativistic Entropic Hamiltonian–Lagrangian Approach to the Entropy Production of Spiral Galaxies in Hyperbolic Spacetime. Universe 2021, 7, 325. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes I. Phys. Rev. 1931, 37, 405–426. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes II. Phys. Rev. 1931, 37, 2265–2279. [Google Scholar] [CrossRef]
- Casimir, H.B.G. On Onsager Principle of Microscopic Reversibility. Rev. Mod. Phys. 1945, 17, 343–350. [Google Scholar] [CrossRef]
- Miller, D.G. Ternary Isothermal Diffusion and the Validity of the Onsager Reciprocity Relations. J. Phys. Chem. 1959, 63, 570–578. [Google Scholar] [CrossRef]
- Miller, D.G. Thermodynamics of Irreversible Processes: The Experimental Verification of the Onsager Reciprocal Relations. Chem. Rev. 1960, 60, 15–37. [Google Scholar] [CrossRef]
- Coleman, B.D.; Truesdel, C. On the Reciprocal Relations of Onsager. J. Chem. Phys. 1960, 33, 28–31. [Google Scholar] [CrossRef]
- Truesdell, C.A. Rational Thermodynamics; Springer: New York, NY, USA, 1984. [Google Scholar]
- Tyrrell, H.J.V.; Harris, K.R. Diffusion in Liquids: A Theoretical and Experimental Study; Butterworths: London, UK, 1984. [Google Scholar]
- de Groot, S.R.; Mazur, P. Non-Equilibrium Thermodynamics, 2nd ed.; Dover Publications: New York, NY, USA, 1984; pp. 11–82. [Google Scholar]
- Kuiken, G.D.C. Thermodynamics of Irreversible Processes—Applications to Diffusion and Rheology, 1st ed.; Wiley: New York, NY, USA, 1994; pp. 1–135. [Google Scholar]
- Jou, D.; Lebon, G.; Casas-Vazquez, J. Extended Irreversible Thermodynamics, 3rd ed.; Springer: Berlin, Germany, 2001; pp. 39–70. [Google Scholar]
- Bird, R.B.; Stewart, W.E.; Lightfoot, E.N. Transport Phenomena, 2nd ed.; Wiley: New York, NY, USA, 2002; pp. 764–798. [Google Scholar]
- Kondepudi, D.; Prigogine, I. Thermodynamics Nonequilibrium. In Encyclopedia of Applied Physics, 2nd ed.; Trigg, G.L., Ed.; Wiley-VCH: Weinheim, Germany, 2003. [Google Scholar]
- Demirel, Y.A.; Sandler, S.I. Nonequilibrium Thermodynamics in Engineering and Science. J. Phys. Chem. B 2004, 108, 31–43. [Google Scholar] [CrossRef]
- Öttinger, H.C. Beyond Equilibrium Thermodynamics, 1st ed.; Wiley: New York, NY, USA, 2005. [Google Scholar]
- Demirel, Y. Nonequilibrium Thermodynamics, Transport and Rate Processes in Physical, Chemical and Biological Systems, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2007; pp. 1–144. [Google Scholar]
- Rastogi, R.P. Introduction to Non-Equilibrium Physical Chemistry, 1st ed.; Elsevier: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Müller, I.; Müller, W.H. Fundamentals of Thermodynamics and Applications; Springer: Heidelberg, Germany, 2009. [Google Scholar]
- Michaelides, E.E. Transport Properties of Nanofluids. A Critical Review. J. Non Equil. Thermodyn. 2023, 38, 1–79. [Google Scholar] [CrossRef]
- Kondepudi, D.; Prigogine, I. Modern Thermodynamics: From Heat Engines to Dissipative Structures, 2nd ed.; Wiley: New York, NY, USA, 2015; pp. 333–455. [Google Scholar]
- Arya, R.K.; Verros, G.D. On the validity of Onsager Reciprocal Relations (ORR) for heat transfer in anisotropic solids. Axioms 2022, 11, 104. [Google Scholar] [CrossRef]
- Reinecke, B.N.; Shan, J.W.K.; Suabedissen, K.; Cherkasova, A.S. On the anisotropic thermal conductivity of magnetorheological suspensions. J. Appl. Phys. 2008, 104, 023507. [Google Scholar] [CrossRef]
- Li, M.-G.; Zheng, C.; Zhao, Q.; Chen, X.; Wu, W.-T. Anisotropic heat transfer of ferro-nanofluid in partially heated rectangular enclosures under magnetic field. Case Stud. Therm. Eng. 2021, 26, 101145. [Google Scholar] [CrossRef]
- Kim, S.; Menabde, S.G.; Brar, V.W.; Jang, M.S. Functional Mid-Infrared Polaritonics in van der Waals Crystals. Adv. Optical Mater. 2020, 8, 1901194. [Google Scholar] [CrossRef]
- Gonzalez-Munoz, S.; Agarwal, K.; Castanon, E.G.; Kudrynskyi, Z.R.; Kovalyuk, Z.D.; Spièce, J.; Kazakova, O.; Patanè, A.; Kolosov, O.V. Direct Measurements of Anisotropic Thermal Transport in γ-InSe Nanolayers via Cross-Sectional Scanning Thermal Microscopy. Adv. Mater. Interfaces 2023, 10, 2300081. [Google Scholar] [CrossRef]
- Cai, Y.; Faizan, M.; Mu, H.; Zhang, Y.; Zou, H.; Zhao, H.J.; Fu, Y.; Zhang, L. Anisotropic phonon thermal transport in two-dimensional layered materials. Front. Phys. 2023, 18, 43303. [Google Scholar] [CrossRef]
- Olson, D.H.; Angelici Avincola, V.; Parker, C.G.; Braun, J.L.; Gaskins, J.T.; Tomko, J.A.; Opila, E.J.; Hopkins, P.E. Anisotropic thermal conductivity tensor of β-Y2Si2O7 for orientational control of heat flow on micrometer scales. Acta Mater. 2020, 189, 299–305. [Google Scholar] [CrossRef]
- Chen, H.; Liu, D. Formulation of a nonlocal discrete model for anisotropic heat conduction problems. Int. J. Therm. Sci. 2022, 182, 107816. [Google Scholar] [CrossRef]
- Dong, H.; Cao, C.; Ying, P.; Fan, Z.; Qian, P.; Su, Y. Anisotropic and high thermal conductivity in monolayer quasi-hexagonal fullerene: A comparative study against bulk phase fullerene. Int. J. Heat Mass Transf. 2023, 206, 123943. [Google Scholar] [CrossRef]
- Zhao, Y.; Yan, F.; Liu, X. Thermal transport characteristics of diamond under stress. Diam. Relat. Mater. 2023, 136, 110016. [Google Scholar] [CrossRef]
- Sanamaría-Holek, I.; Gadomski, A.; Rubí, J.M. Controlling protein crystal growth rate by means of temperature. J. Phys. Condens. Matter 2011, 23, 235101. [Google Scholar] [CrossRef]
- Gadomski, A.; Kruszewska, N. Thermodiffusion as a close-to-interface effect that matters in non-isothermal (dis)orderly protein aggregations. Phys. Lett. Sect. A Gen. At. Solid State Phys. 2014, 378, 2881–2887. [Google Scholar] [CrossRef]
- Thapliyal, D.; Arya, R.K.; Verros, G.D. On the multi-component diffusion in the linear region of the extended thermodynamics framework. Eur. Phys. J. Plus 2021, 136, 1138. [Google Scholar] [CrossRef]
- Agren, J. The Onsager Reciprocity Relations Revisited. J. Phase Equilib. Diffus. 2022, 43, 640–647. [Google Scholar] [CrossRef]
- Liu, Z.-K. Theory of cross phenomena and their coefficients beyond Onsager theorem. Mater. Res. Lett. 2022, 10, 393–439. [Google Scholar] [CrossRef]
- Liu, Z.-K. Thermodynamics and its prediction and CALPHAD modeling: Review, state of the art, and perspectives. Calphad 2023, 82, 102580. [Google Scholar] [CrossRef]
- Verros, G.D. Comprehensive Criteria for the Extrema in Entropy Production Rate for Heat Transfer in the Linear Region of Extended Thermodynamics Framework. Axioms 2020, 9, 113. [Google Scholar] [CrossRef]
- Verros, G.D. On the Validity of the Onsager Reciprocal Relations in Multi-component Diffusion. Phys. Lett. A 2007, 365, 34–38. [Google Scholar] [CrossRef]
- Arya, R.K.; Thapliyal, D.; Verros, G.D.; Singh, N.; Singh, D.; Kumar, R.; Srivastava, R.K.; Tiwari, A.K. On the Validity of a Linearity Axiom in Diffusion and Heat Transfer. Coatings 2022, 12, 1582. [Google Scholar] [CrossRef]
- Verros, G.D. On the Validity of the Onsager Reciprocal Relations in Simultaneous Heat and Multi-component Diffusion. Phys. A Stat. Mech. Its Appl. 2007, 385, 487–492. [Google Scholar] [CrossRef]
- Glansdorff, P.; Nicolis, G.; Prigogine, I. The thermodynamic stability theory of non-equilibrium States. Proc. Natl. Acad. Sci. USA 1974, 71, 197–199. [Google Scholar] [CrossRef] [PubMed]
- Vilar, J.M.G.; Rubí, J.M. Thermodynamics “beyond” local equilibrium. Proc. Natl. Acad. Sci. USA 2001, 98, 11081–11084. [Google Scholar] [CrossRef]
- Stewart, W.E.; Caracotsios, M.; Sørensen, J.P. Parameter estimation from multiresponse data. AIChE J. 1992, 38, 641–650. [Google Scholar] [CrossRef]
- Gavassino, L. Relativistic Heat Conduction in the Large-Flux Regime. Entropy 2024, 26, 147. [Google Scholar] [CrossRef] [PubMed]
| Physical Quantity | Value | Units |
|---|---|---|
| T | 290 | K |
| Jq1 | 0.53 | W.m−2 |
| Jq2 | 0.6 | W.m−2 |
| Jq3 | −0.51 | W.m−2 |
| T01 | 270 | K |
| T02 | 280 | K |
| w1 | 0.1 | dimensionless |
| w2 | 0.1 | dimensionless |
| w3 | 0.8 | dimensionless |
| Physical Quantity | Value | Units |
|---|---|---|
| 0.234 | K.m. W−1 | |
| −0.03322 | K.m. W−1 | |
| 0.0434 | K.m. W−1 | |
| h1 | 0.0265 | Wm−2K−1 |
| h2 | 0.06 | Wm−2K−1 |
| h3 | 0.136 | Wm−2K−1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thapliyal, D.; Arya, R.K.; Achilias, D.S.; Verros, G.D. On the Resistance Coefficients for Heat Conduction in Anisotropic Bodies at the Limit of Linear Extended Thermodynamics. Entropy 2025, 27, 314. https://doi.org/10.3390/e27030314
Thapliyal D, Arya RK, Achilias DS, Verros GD. On the Resistance Coefficients for Heat Conduction in Anisotropic Bodies at the Limit of Linear Extended Thermodynamics. Entropy. 2025; 27(3):314. https://doi.org/10.3390/e27030314
Chicago/Turabian StyleThapliyal, Devyani, Raj Kumar Arya, Dimitris S. Achilias, and George D. Verros. 2025. "On the Resistance Coefficients for Heat Conduction in Anisotropic Bodies at the Limit of Linear Extended Thermodynamics" Entropy 27, no. 3: 314. https://doi.org/10.3390/e27030314
APA StyleThapliyal, D., Arya, R. K., Achilias, D. S., & Verros, G. D. (2025). On the Resistance Coefficients for Heat Conduction in Anisotropic Bodies at the Limit of Linear Extended Thermodynamics. Entropy, 27(3), 314. https://doi.org/10.3390/e27030314









