Occupation Times on the Legs of a Diffusion Spider
Abstract
1. Introduction
It is a diffusion which, when away from the origin, is a Brownian motion along a ray, but which has what might be called a roundhouse singularity at the origin: when the process enters it, it, like Stephen Leacock’s hero, immediately rides off in all directions at once.
2. Preliminaries
2.1. Linear Diffusions
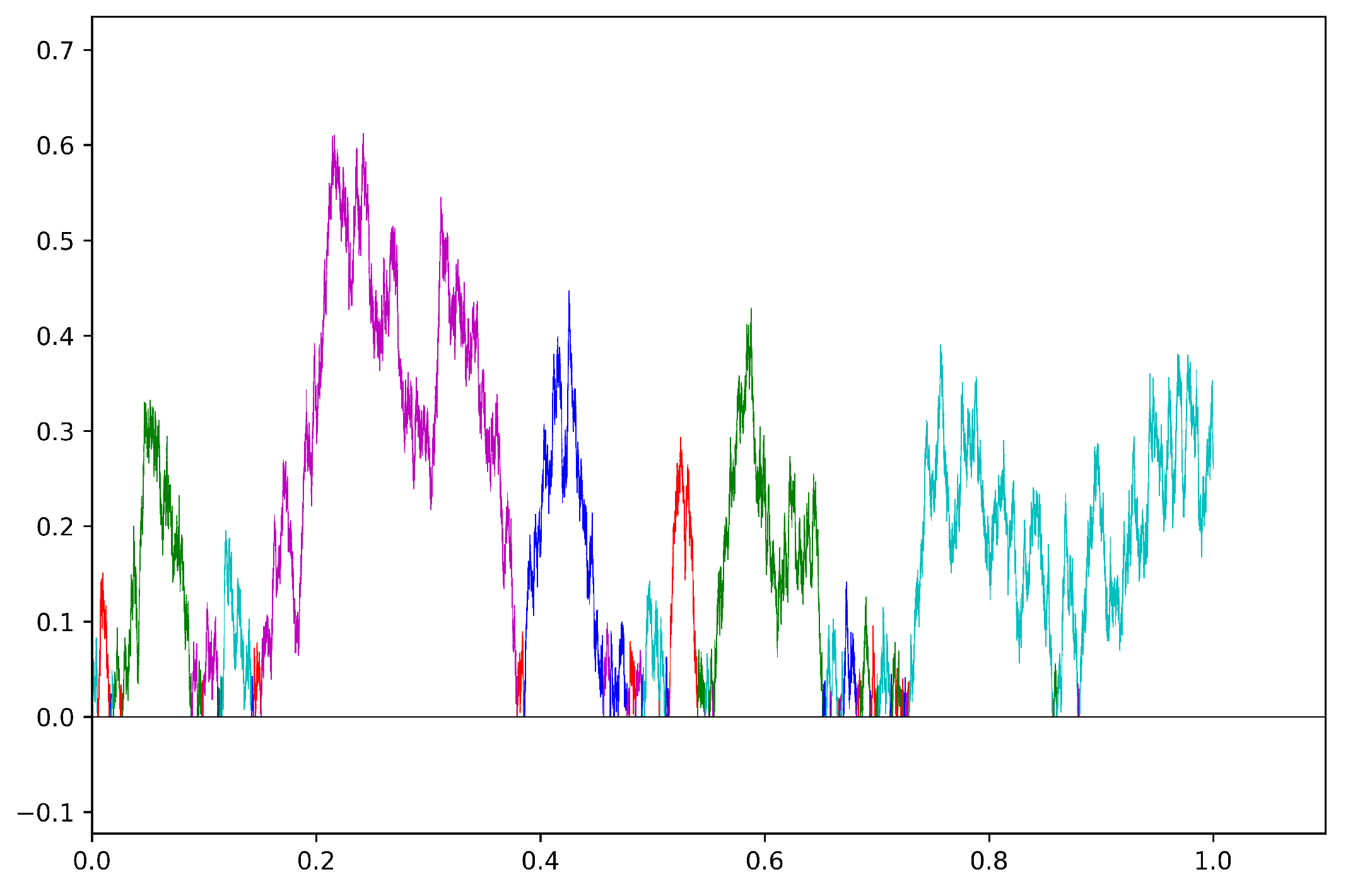
2.2. Diffusion Spider
3. Kac’s Moment Formula
4. Main Results
4.1. Moments of the Occupation Time on a Single Leg
- (1)
- (2)
- (3)
4.2. Joint Moments
5. Examples
5.1. Bessel Spider
5.2. Brownian Spider
5.3. Walsh Brownian Motion
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix C
Appendix D
Appendix E
References
- Walsh, J.B. A diffusion with a discontinuous local time. In Temps locaux; Société Mathématique de France: Marseille, France, 1978; Volume 52–53, pp. 37–45. [Google Scholar]
- Barlow, M.; Pitman, J.; Yor, M. On Walsh’s Brownian motions. In Séminaire de Probabilités, XXIII; Lecture Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 1989; Volume 1372, pp. 275–293. [Google Scholar] [CrossRef]
- Salisbury, T.S. Construction of right processes from excursions. Probab. Theory Related Fields 1986, 73, 351–367. [Google Scholar] [CrossRef]
- Yano, Y. On the joint law of the occupation times for a diffusion process on multiray. J. Theoret. Probab. 2017, 30, 490–509. [Google Scholar] [CrossRef]
- Freidlin, M.I.; Wentzell, A.D. Diffusion processes on graphs and the averaging principle. Ann. Probab. 1993, 21, 2215–2245. [Google Scholar] [CrossRef]
- Weber, M. On occupation time functionals for diffusion processes and birth-and-death processes on graphs. Ann. Appl. Probab. 2001, 11, 544–567. [Google Scholar] [CrossRef]
- Barlow, M.; Pitman, J.; Yor, M. Une extension multidimensionnelle de la loi de l’arc sinus. In Séminaire de Probabilités, XXIII; Lecture Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 1989; Volume 1372, pp. 294–314. [Google Scholar] [CrossRef]
- Papanicolaou, V.; Papageorgiou, E.; Lepipas, D. Random motion on simple graphs. Methodol. Comput. Appl. Probab. 2012, 14, 285–297. [Google Scholar] [CrossRef]
- Vakeroudis, S.; Yor, M. A scaling proof for Walsh’s Brownian motion extended arc-sine law. Electron. Commun. Probab. 2012, 17, 63. [Google Scholar] [CrossRef]
- Fitzsimmons, P.J.; Kuter, K.E. Harmonic functions on Walsh’s Brownian motion. Stoch. Process. Appl. 2014, 124, 2228–2248. [Google Scholar] [CrossRef]
- Csáki, E.; Csörgő, M.; Földes, A.; Révész, P. Some limit theorems for heights of random walks on a spider. J. Theoret. Probab. 2016, 29, 1685–1709. [Google Scholar] [CrossRef]
- Csáki, E.; Csörgő, M.; Földes, A.; Révész, P. Limit theorems for local and occupation times of random walks and Brownian motion on a spider. J. Theoret. Probab. 2019, 32, 330–352. [Google Scholar] [CrossRef]
- Ernst, P. Exercising control when confronted by a (Brownian) spider. Oper. Res. Lett. 2016, 44, 487–490. [Google Scholar] [CrossRef]
- Karatzas, I.; Yan, M. Semimartingales on rays, Walsh diffusions, and related problems of control and stopping. Stoch. Process. Appl. 2019, 129, 1921–1963. [Google Scholar] [CrossRef]
- Bayraktar, E.; Zhang, X. Embedding of Walsh Brownian motion. Stoch. Process. Appl. 2021, 134, 1–28. [Google Scholar] [CrossRef]
- Lempa, J.; Mordecki, E.; Salminen, P. Diffusion spiders: Green kernel, excessive functions and optimal stopping. Stoch. Process. Appl. 2024, 167, 104229. [Google Scholar] [CrossRef]
- Bednarz, E.; Ernst, P.A.; Osękowski, A. On the diameter of the stopped spider process. Math. Oper. Res. 2024, 49, 346–365. [Google Scholar] [CrossRef]
- Csáki, E.; Földes, A. In memoriam Pál Révész (1934–2022). Period. Math. Hungar. 2024, 89, 201–229. [Google Scholar] [CrossRef]
- Lejay, A. On the constructions of the skew Brownian motion. Probab. Surv. 2006, 3, 413–446. [Google Scholar] [CrossRef]
- Ramirez, J.M.; Thomann, E.A.; Waymire, E.C. Advection-dispersion across interfaces. Statist. Sci. 2013, 28, 487–509. [Google Scholar] [CrossRef]
- Appuhamillage, T.; Bokil, V.; Thomann, E.; Waymire, E.; Wood, B. Occupation and local times for skew Brownian motion with applications to dispersion across an interface. Ann. Appl. Probab. 2011, 21, 183–214. [Google Scholar] [CrossRef]
- Lejay, A.; Pichot, G. Simulating diffusion processes in discontinuous media: Numerical scheme with constant time step. J. Comput. Phys. 2012, 231, 7299–7314. [Google Scholar] [CrossRef]
- Lejay, A.; Mordecki, E.; Torres, S. Is a Brownian motion skew? Scand. J. Statist. 2014, 41, 346–364. [Google Scholar] [CrossRef]
- Alvarez, L.; Salminen, P. Timing in the presence of directional predictability: Optimal stopping of skew Brownian motion. Math. Meth. Oper. Res. 2017, 86, 377–400. [Google Scholar] [CrossRef][Green Version]
- Rossello, D. Arbitrage in skew Brownian motion models. Insur. Math. Econom. 2012, 50, 50–56. [Google Scholar] [CrossRef]
- Hussain, J.; Soomro, M.A.; Dahri, S.A.; Memon, K.N.; Bano, M.; Awwad, F.A.; Ismail, E.A.A.; Ahmad, H. A study of maximizing skew Brownian motion with applications to option pricing. J. Radiat. Res. Appl. Sci. 2024, 17, 100732. [Google Scholar] [CrossRef]
- Dassios, M.; Zhang, J. First Hitting Time of Brownian Motion on Simple Graph with Skew Semiaxes. Methodol. Comput. Appl. Probab. 2022, 24, 1805–1831. [Google Scholar] [CrossRef]
- Atar, R.; Cohen, A. Serve the shortest queue and Walsh Brownian motion. Ann. Appl. Probab. 2019, 29, 613–651. [Google Scholar] [CrossRef]
- Salminen, P.; Stenlund, D. On occupation times of one-dimensional diffusions. J. Theoret. Probab. 2021, 34, 975–1011. [Google Scholar] [CrossRef]
- Itô, K.; McKean, H.P., Jr. Diffusion Processes and Their Sample Paths; Springer: Berlin/Heidelberg, Germany, 1974. [Google Scholar]
- Borodin, A.N.; Salminen, P. Handbook of Brownian Motion—Facts and Formulae, 2nd ed.; Probability and Its Applications; Birkhäuser Verlag: Basel, Switzerland, 2015. [Google Scholar] [CrossRef]
- Kac, M. On some connections between probability theory and differential and integral equations. In Proceedings of the Second Berkeley Symposium on Mathematical Statistics and Probability, Berkeley/Los Angeles, CA, USA, 31 July–12 August 1950; University of California Press: Berkeley, LA, USA, 1951; pp. 189–215. [Google Scholar]
- Stenlund, D. On the connection between Stirling numbers and Bessel numbers. Electron. J. Combin. 2022, 29, P1.40. [Google Scholar] [CrossRef]
- Yang, S.L.; Qiao, Z.K. The Bessel numbers and Bessel matrices. J. Math. Res. Expo. 2011, 31, 627–636. [Google Scholar] [CrossRef]
- Graham, R.L.; Knuth, D.E.; Patashnik, O. Concrete Mathematics, 2nd ed.; Addison-Wesley Publishing Company: Reading, MA, USA, 1994. [Google Scholar]


| Moment/Statistic | Value |
|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salminen, P.; Stenlund, D. Occupation Times on the Legs of a Diffusion Spider. Entropy 2025, 27, 179. https://doi.org/10.3390/e27020179
Salminen P, Stenlund D. Occupation Times on the Legs of a Diffusion Spider. Entropy. 2025; 27(2):179. https://doi.org/10.3390/e27020179
Chicago/Turabian StyleSalminen, Paavo, and David Stenlund. 2025. "Occupation Times on the Legs of a Diffusion Spider" Entropy 27, no. 2: 179. https://doi.org/10.3390/e27020179
APA StyleSalminen, P., & Stenlund, D. (2025). Occupation Times on the Legs of a Diffusion Spider. Entropy, 27(2), 179. https://doi.org/10.3390/e27020179






