Ising Paradigm in Isobaric Ensembles
Abstract
1. Background and Scope
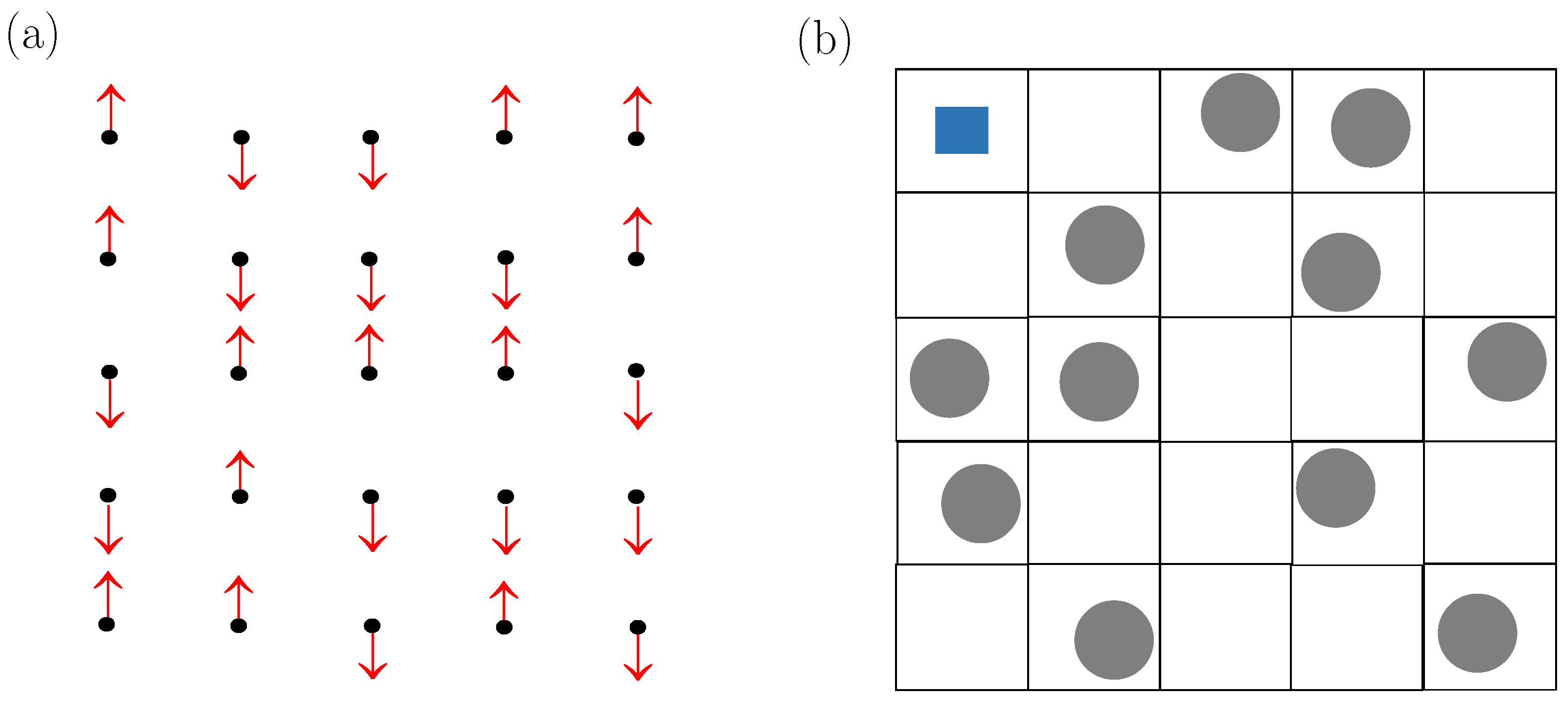
1.1. Ising Model and Standard Lattice Gas
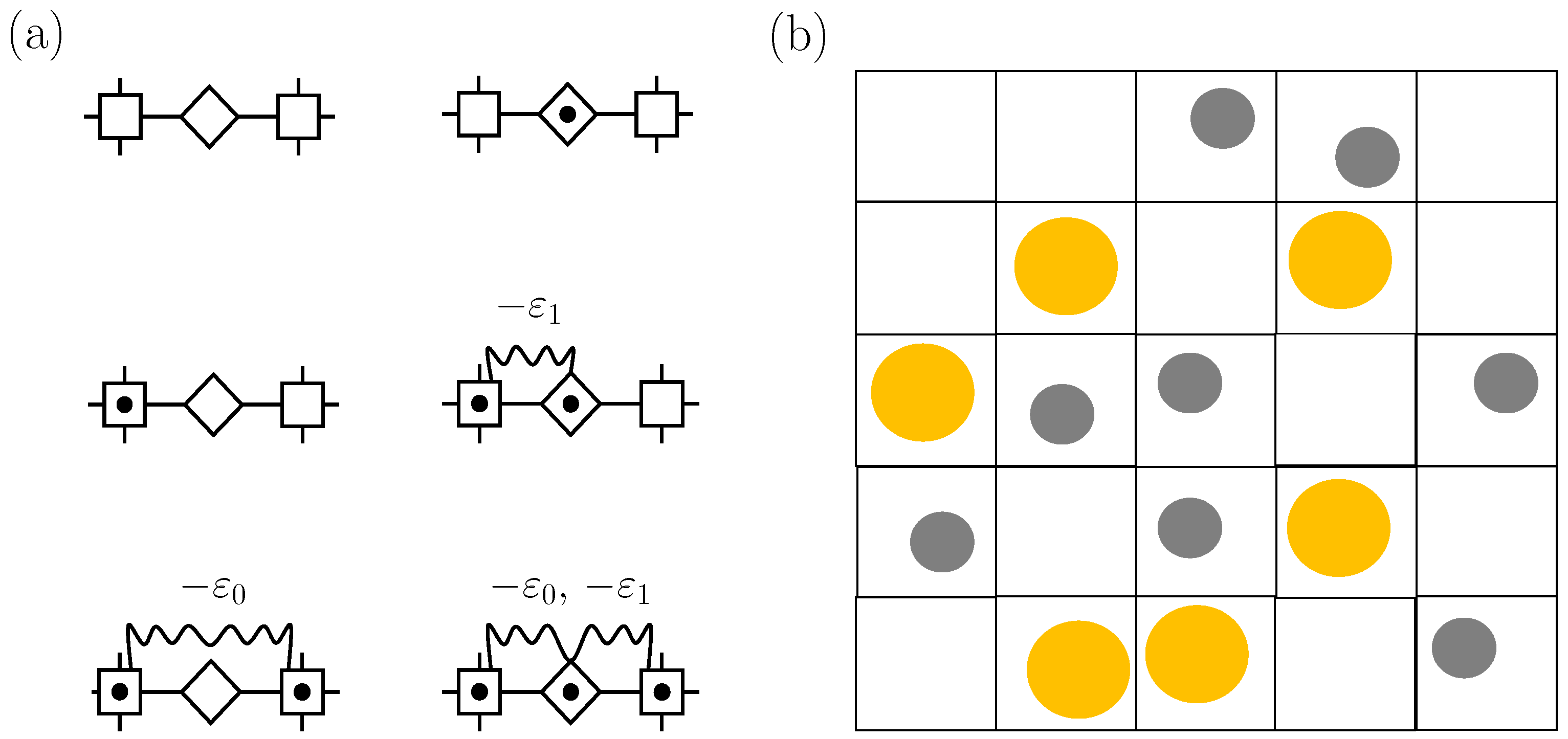
1.2. Decorated and BEG-like Models
1.3. Compressible Cell Models
2. Asymmetric Fluid Criticality
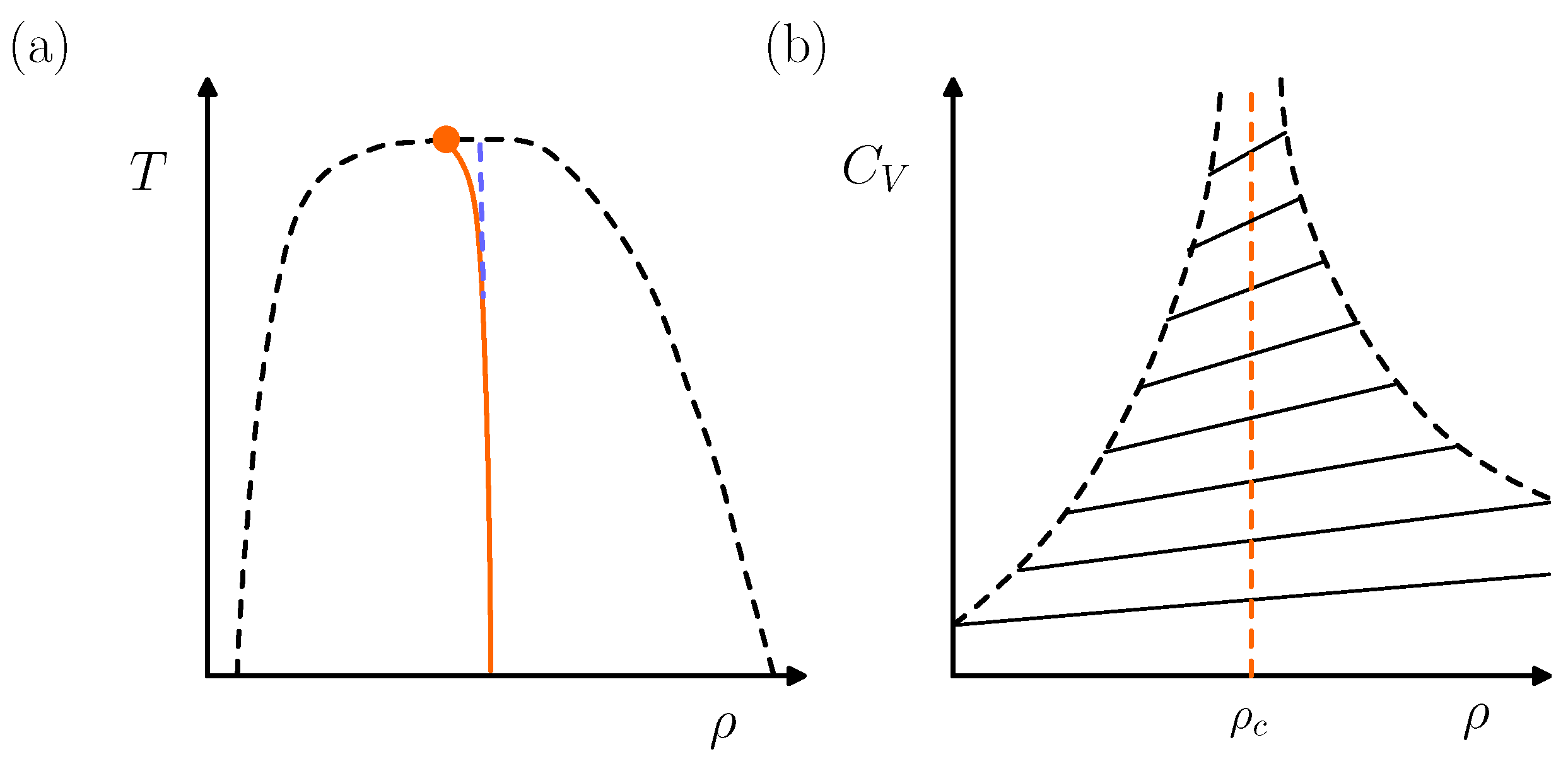
2.1. Complete Scaling with Singular Diameters and Yang–Yang Features
2.2. Most Basic Compressible Cell Gases
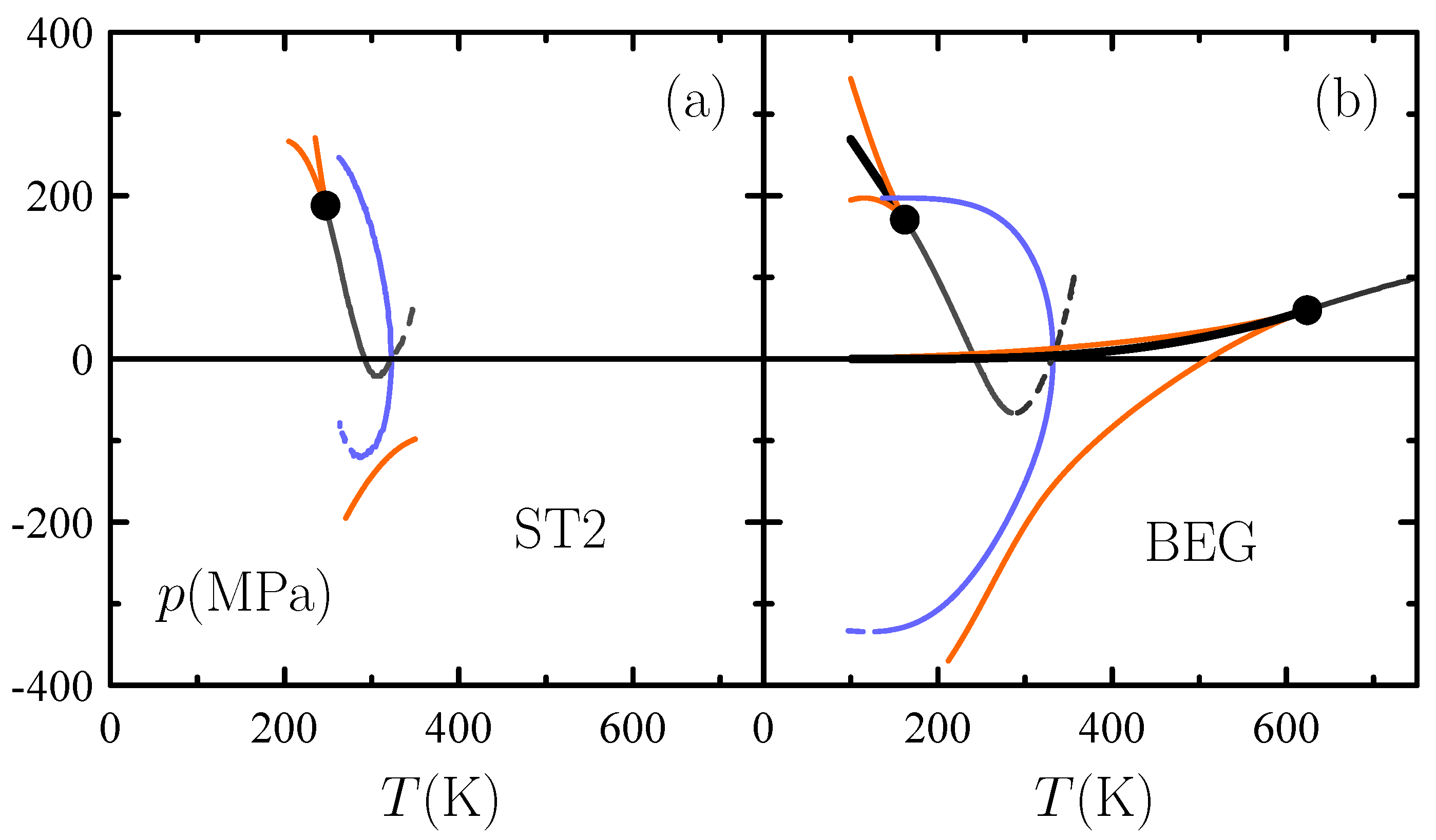
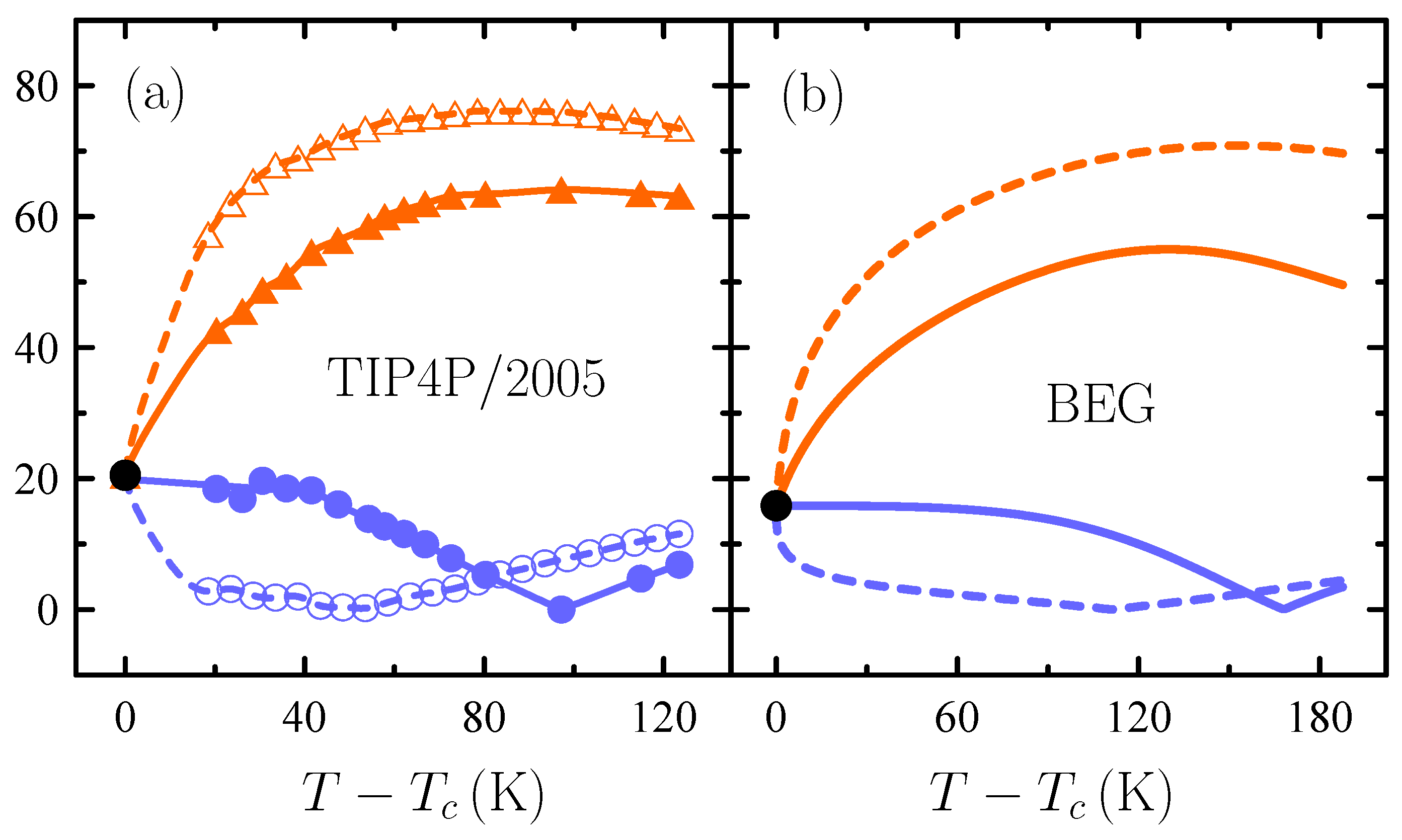
3. Water’s Unusual Thermodynamics
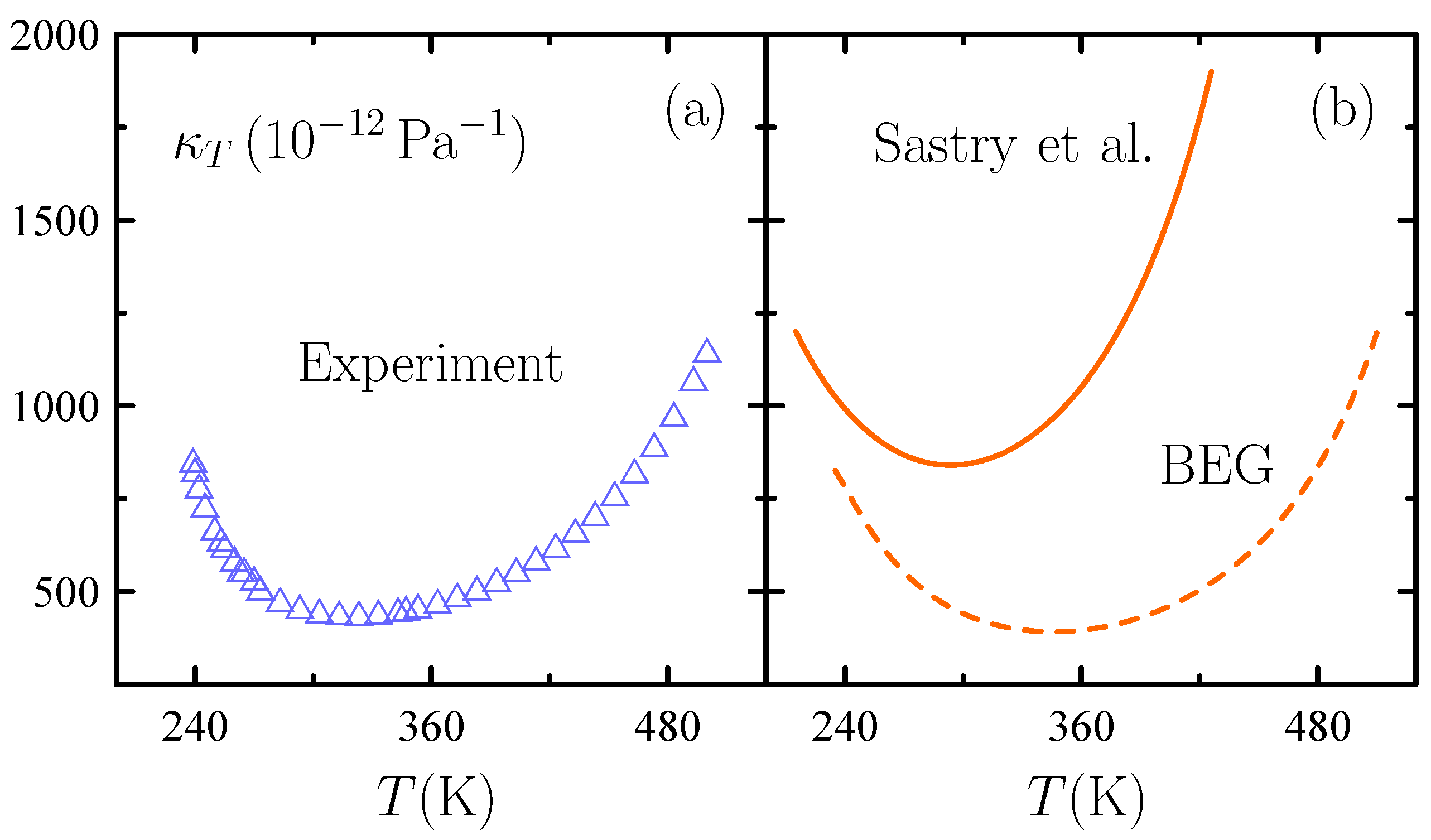
3.1. Singularity-Free Scenario Versus Second Critical Point
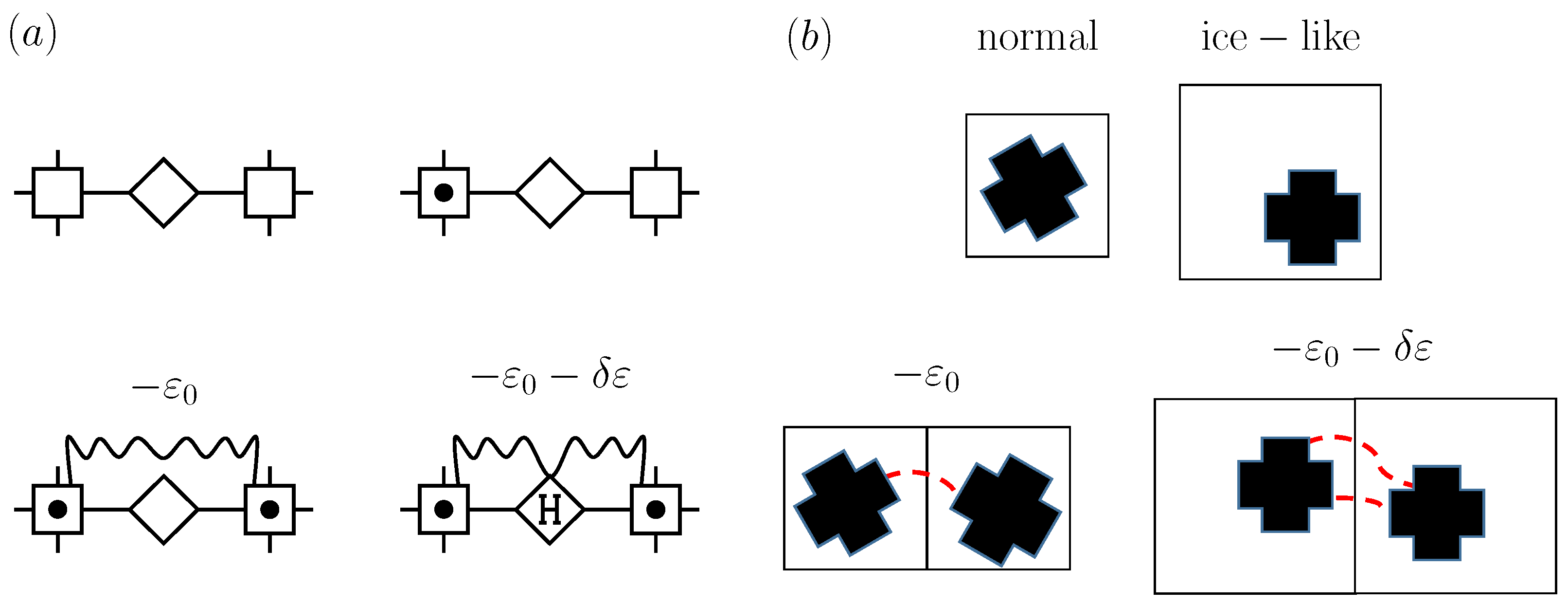
3.2. Compressible Cell Gas with “Ice-like” Hydrogen Bonding
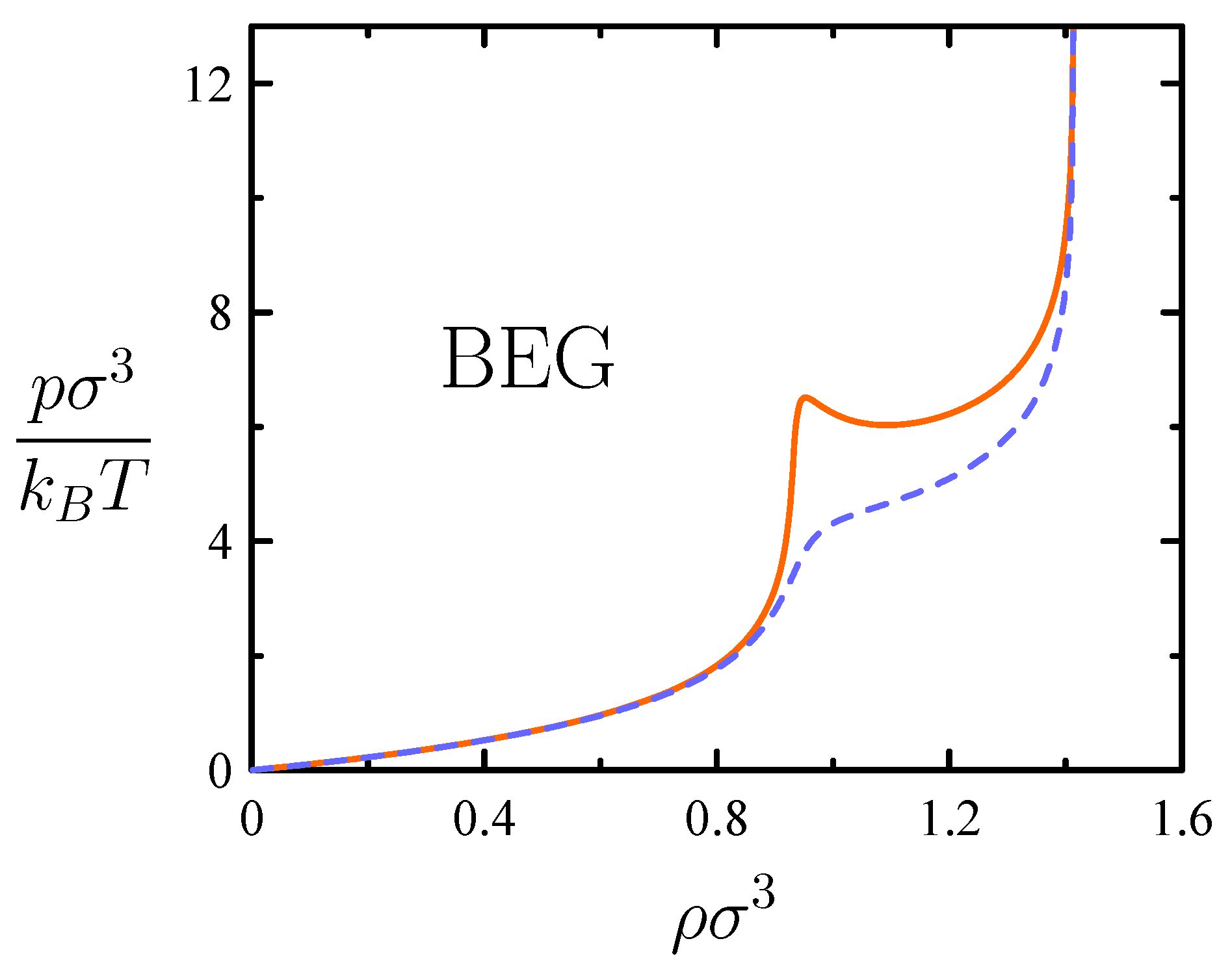
3.3. Compressible Cell Liquid with “Ice-like” Hydrogen Bonding and BEG Water-like Model
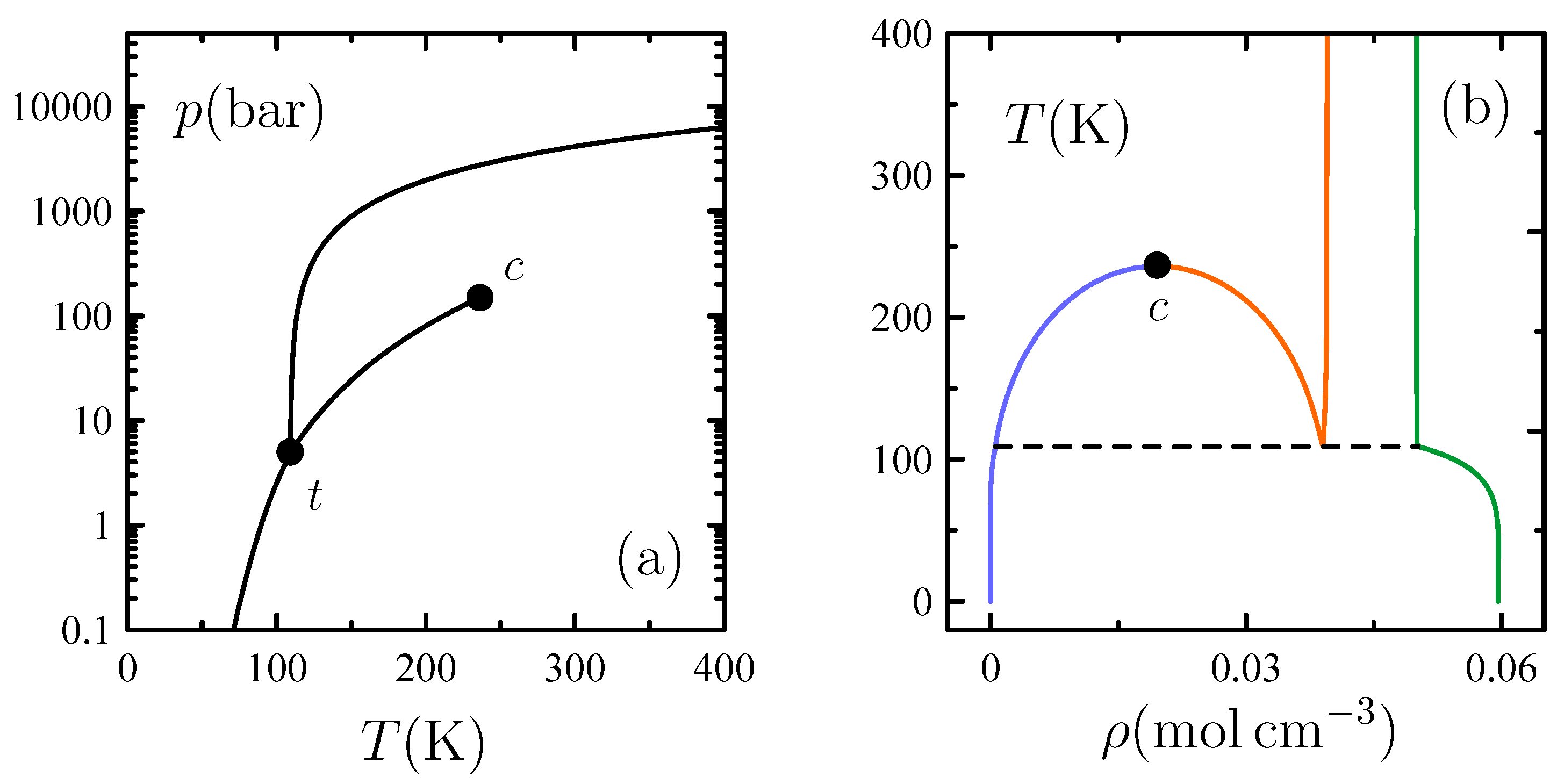
4. Freezing
4.1. An Open Problem to Statistical Mechanics
4.2. Compressible Cell Liquid with Molecular Packing
4.3. BEG-like Model and Van Der Waals Picture
5. Future Work
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| SLG | Standard lattice gas |
| CCG | Compressible cell gas |
| CCL | Compressible cell liquid |
| BEG | Blume–Emery–Griffiths |
References
- Ising, I. Report on the theory of ferromagnetism. Z. Phys. 1925, 31, 253–258. [Google Scholar] [CrossRef]
- Kramers, H.A.; Wannier, G.H. Statistics of the two-dimensional ferromagnet. Part I. Phys. Rev. 1960, 60, 252–262. [Google Scholar] [CrossRef]
- Onsager, L. Crystal statistics. I. A two-dimensional model with an order-disorder transition. Phys. Rev. 1944, 65, 117–149. [Google Scholar] [CrossRef]
- Yang, C.N. The spontaneous magnetization of a two-dimensional Ising model. Phys. Rev. 1952, 385, 808–816. [Google Scholar] [CrossRef]
- Domb, C. On the theory of cooperative phenomena in crystals. Adv. Phys. 1960, 9, 149–361. [Google Scholar] [CrossRef]
- Fisher, M.E. Theory of critical phenomena. Rep. Prog. Phys. 1967, 30, 615–730. [Google Scholar] [CrossRef]
- Kadanoff, L.P.; Gotze, W.; Hamblen, D.; Hecht, R.; Lewis, E.A.S.; Palciauskas, M.R.; Swift, J.; Aspnes, D.; Kane, J. Static phenomena near critical points: Theory and experiment. Rev. Mod. Phys. 1967, 39, 395–430. [Google Scholar] [CrossRef]
- Wilson, K.G. The renormalization group and critical phenomena. Rev. Mod. Phys. 1983, 55, 583–600. [Google Scholar] [CrossRef]
- Vaks, V.G.; Larkin, A.I. On phase transitions of second order. Soviet Phys. JETP 1966, 22, 678. [Google Scholar]
- Kosterlitz, J.M. Nobel lecture: Topological defects in two dimensions. Rev. Mod. Phys. 2017, 89, 040501. [Google Scholar] [CrossRef]
- Sherrington, D.; Kirkpatrick, S. Solvable model of a spin-glass. Phys. Rev. Lett. 1975, 35, 1792–1796. [Google Scholar] [CrossRef]
- Parisi, G. Nobel lecture: Multiple equilibria. Rev. Mod. Phys. 2023, 31, 030501. [Google Scholar] [CrossRef]
- Fisher, M.E. Simple Ising models still thrive! A review of some recent progress. Phys. A 1981, 106, 28–47. [Google Scholar] [CrossRef]
- Lee, T.D.; Yang, C.N. Statistical theory of equations of state and phase transitions. II. Lattice gas and Ising model. Phys. Rev. 1952, 87, 410–419. [Google Scholar] [CrossRef]
- Cernushi, F.; Eyring, H. An elementary theory of condensation. J. Chem. Phys. 1939, 7, 547–551. [Google Scholar] [CrossRef]
- Fisher, M.E. Transformations of Ising models. Phys. Rev. 1959, 113, 969–981. [Google Scholar] [CrossRef]
- Wheeler, J.C. Decorated lattice-gas models of critical phenomena on fluids and fluid mixtures. Annu. Rev. Phys. Chem. 1977, 28, 411–443. [Google Scholar] [CrossRef]
- Schouten, J.A.; Ten Seldam, C.A.; Trappeniers, N.J. The two-component lattice-gas model. Physica 1974, 73, 556–572. [Google Scholar] [CrossRef]
- Mukamel, D.; Blume, M. Ising model for tricritical points in ternary mixtures. Phys. Rev. A 1974, 10, 610–617. [Google Scholar] [CrossRef]
- Furman, D.; Dattagupta, S.; Griffiths, R.B. Global phase diagram for a three-component model. Phys. Rev. B 1977, 15, 441–464. [Google Scholar] [CrossRef]
- Blume, M.; Emery, V.J.; Griffiths, R.B. Ising model for the λ transition and phase separation in He3–He4 mixtures. Phys. Rev. A 1971, 4, 1071–1077. [Google Scholar] [CrossRef]
- Sastry, S.; Debenedetti, P.G.; Sciortino, F.; Stanley, H.E. Singularity-free interpretation of the thermodynamics of supercooled water. Phys. Rev. E 1996, 53, 6144–6154. [Google Scholar] [CrossRef] [PubMed]
- Rebelo, L.P.N.; Sastry, S.; Debenedetti, P.G. Singularity-free interpretation of the thermodynamics of supercooled water. II. Thermal and volumetric behavior. J. Chem. Phys. 1998, 109, 626–633. [Google Scholar] [CrossRef]
- Cerdeiriña, C.A.; Orkoulas, G.; Fisher, M.E. Soluble model fluids with complete scaling and Yang-Yang features. Phys. Rev. Lett. 2016, 116, 040601. [Google Scholar] [CrossRef] [PubMed]
- Cerdeiriña, C.A.; Orkoulas, G. Compressible cell models for asymmetric fluid criticality. Phys. Rev. E 2017, 95, 032105. [Google Scholar] [CrossRef] [PubMed]
- Cerdeiriña, C.A.; Stanley, H.E. Ising-like models with energy-volume coupling. Phys. Rev. Lett. 2018, 120, 120603. [Google Scholar] [CrossRef]
- Troncoso, J.; Cerdeiriña, C.A. Ising model for the freezing transition. Phys. Rev. E 2024, 109, 014123. [Google Scholar] [CrossRef]
- Cerdeiriña, C.A.; Troncoso, J.; González-Salgado, D.; Debenedetti, P.G.; Stanley, H.E. Water’s two-critical-point scenario in the Ising paradigm. J. Chem. Phys. 2019, 150, 244509. [Google Scholar] [CrossRef] [PubMed]
- James, H.M.; Guth, E. Theory of the elastic properties of rubber. J. Chem. Phys. 1943, 11, 455–481. [Google Scholar] [CrossRef]
- Sack, R.A. Pressure-dependent partition functions. Mol. Phys. 1959, 2, 8–22. [Google Scholar] [CrossRef]
- Fisher, M.E.; Orkoulas, G. The Yang-Yang anomaly in fluid criticality: Experiment and scaling theory. Phys. Rev. Lett. 2000, 85, 696–699. [Google Scholar] [CrossRef]
- Kim, Y.C.; Fisher, M.E.; Luijten, E. Orkoulas, G. Precise simulation of near-critical fluid coexistence. Phys. Rev. Lett. 2003, 91, 065701. [Google Scholar] [CrossRef]
- Kim, Y.C.; Fisher, M.E.; Orkoulas, G. Asymmetric fluid criticality. I. Scaling with pressure mixing. Phys. Rev. E 2000, 67, 061506. [Google Scholar] [CrossRef] [PubMed]
- Yang, C.N.; Yang, C.P. Critical point in liquid-gas transitions. Phys. Rev. Lett. 1964, 13, 303–305. [Google Scholar] [CrossRef]
- Mermin, N.D. Lattice gas with short-range pair interactions and a singular coexistence-curve diameter. Phys. Rev. Lett. 1971, 26, 957–959. [Google Scholar] [CrossRef]
- Widom, B.; Rowlinson, J.S. New model for study of liquid-vapor phase transitions. J. Chem. Phys. 1970, 52, 1670–1684. [Google Scholar] [CrossRef]
- Mermin, N.D. Solvable model of a vapor-liquid transition and a singular coexistence-curve diameter. Phys. Rev. Lett. 1971, 26, 169–172. [Google Scholar] [CrossRef]
- Speedy, R.J.; Angell, C.A. Isothermal compressibility of supercooled water and evidence for a thermodynamic singularity at −45∘C. J. Chem. Phys. 1976, 65, 851–858. [Google Scholar] [CrossRef]
- Angell, C.A.; Oguni, M.; Sichina, W.J. Heat capacity of water at the extremes of supercooling and superheating. J. Phys. Chem. 1982, 86, 998–1002. [Google Scholar] [CrossRef]
- Hare, D.E.; Sorensen, C.M. The density of supercooled water. II. Bulk samples cooled to the homogeneous nucleation limit. J. Chem. Phys. 1987, 87, 4840–4845. [Google Scholar] [CrossRef]
- Mishima, O.; Stanley, H.E. The relationship between liquid, supercooled and glassy water. Nature 1998, 396, 329–335. [Google Scholar] [CrossRef]
- Debenedetti, P.G. Supercooled and glassy water. J. Phys. Condens. Matter 2003, 15, R1669–R1726. [Google Scholar] [CrossRef]
- Poole, P.H.; Sciortino, F.; Essmann, U.; Stanley, H.E. Phase behavior of metastable water. Nature 1992, 360, 324–328. [Google Scholar] [CrossRef]
- Stanley, H.E.; Teixeira, J. Interpretation of the unusual behavior of H2O and D2O at low temperatures: Tests of a percolation model. J. Chem. Phys. 1980, 73, 3404–3422. [Google Scholar] [CrossRef]
- Kim, K.H.; Amann-Winkel, K.; Giovambattista, N.; Späh, A.; Perakis, F.; Pathak, H.; Parada, M.L.; Yang, C.; Mariedahl, D.; Eklund, T.; et al. Experimental observation of the liquid–liquid transition in bulk supercooled water under pressure. Science 2020, 370, 978–982. [Google Scholar] [CrossRef] [PubMed]
- Palmer, J.C.; Poole, P.H.; Sciortino, F.; Debenedetti, P.G. Advances in computational studies of the liquid–liquid transition in water and water-like models. Chem. Rev. 2018, 118, 9129–9151. [Google Scholar] [CrossRef] [PubMed]
- Debenedetti, P.G.; Sciortino, F.; Zerze, G.H. Second critical point in two realistic models of water. Science 2020, 369, 289–292. [Google Scholar] [CrossRef] [PubMed]
- Parisi, G.; Urbani, P.; Zamponi, F. Theory of Simple Glasses; Cambridge University Press: Cambridge, UK, 2020. [Google Scholar]
- Peter H. Poole kindly provided us with simulation data for ST2 water.
- Ciach, A.; Gozdz, W.; Perera, A. Simple three-state lattice model for liquid water. Phys. Rev. E 2008, 78, 021203. [Google Scholar] [CrossRef] [PubMed]
- Cerdeiriña, C.A.; Troncoso, J.; González-Salgado, D. Liquid–liquid criticality in TIP4P/2005 and three-state models of water. J. Phys. Chem. 2023, 127, 3902–3910. [Google Scholar] [CrossRef]
- de With, G. Melting is well-known, but is it also well-understood? Chem. Rev. 2023, 123, 13713–13795. [Google Scholar] [CrossRef]
- Kosterlitz, J.M.; Thouless, D.J. Early work on defect-mediated phase transitions. Int. J. Mod. Phys. 2016, 30, 1630018. [Google Scholar] [CrossRef]
- Baus, M. Present status of the density functional theory of the liquid–solid transition. J. Phys. Condens. Matter 1990, 2, 2111–2126. [Google Scholar] [CrossRef]
- Lennard-Jones, J.E.; Devonshire, A.F. Critical and co-operative phenomena III. A theory of melting and the structure of liquids. Proc. R. Soc. 1990, 1939, 317–338. [Google Scholar]
- Barker, J.A. Lattice Theories of the Liquid State; Pergamon Press: Oxford, UK, 2008; p. 163. [Google Scholar]
- Rowlinson, J.S. The rise and fall of lattice theories of the liquid state. Mol. Phys. 2015, 113, 2393–2402. [Google Scholar] [CrossRef]
- Wood, W.W.; Jacobson, J.D. Preliminary results from a recalculation of the Monte Carlo equation of state of hard spheres. J. Chem. Phys. 1957, 27, 1207–1208. [Google Scholar] [CrossRef]
- Alder, B.; Wainwright, T. Phase transition for a hard sphere system. J. Chem. Phys. 1957, 27, 1208–1209. [Google Scholar] [CrossRef]
- Domb, C. Some theoretical aspects of melting. Nuovo Cimento 1958, 9, 9. [Google Scholar] [CrossRef]
- Gaunt, D.S.; Fisher, M.E. Hard-sphere lattice gases. I. Plane-square lattice. J. Chem. Phys. 1965, 43, 2840–2863. [Google Scholar] [CrossRef]
- Bellemans, A.; Nigam, R.K. Phase transitions in two-dimensional lattice gases of hard-square molecules. J. Chem. Phys. 1967, 46, 2922–2935. [Google Scholar] [CrossRef]
- Baxter, R.J. Hard hexagons: Exact solution. J. Phys. A Math. Gen. 1980, 13, L61–L70. [Google Scholar] [CrossRef]
- Panagiotopoulos, A.Z. Thermodynamic properties of lattice hard-sphere models. J. Chem. Phys. 2005, 123, 104504. [Google Scholar] [CrossRef] [PubMed]
- Jauslin, I.; Lebowitz, J.L. High-fugacity expansion, Lee-Yang zeros, and order-disorder transitions in hard-core lattice systems. Commun. Math. Phys. 2018, 364, 655–682. [Google Scholar] [CrossRef]
- Vigneshwar, N.; Mandal, D.; Damle, K.; Dhar, D.; Rajesh, R. Phase diagram of a system of hard cubes on the cubic lattice. Phys. Rev. E 2019, 99, 052129. [Google Scholar] [CrossRef] [PubMed]
- Jaleel, A.A.A.; Mandal, D.; Thomas, J.E.; Rajesh, R. Freezing phase transition in hard-core lattice gases on the triangular lattice with exclusion up to seventh next-nearest neighbor. Phys. Rev. E 2022, 106, 044136. [Google Scholar] [CrossRef]
- Longuet-Higgins, H.C.; Widom, B. A rigid sphere model for melting of argon. Mol. Phys. 1964, 8, 549–556. [Google Scholar] [CrossRef]
- Widom, B. Intermolecular forces and the nature of the liquid state. Science 1967, 157, 375–382. [Google Scholar] [CrossRef]
- Chandler, D.; Weeks, J.D.; Andersen, H.C. van der Waals picture of liquids, solids, and phase transformations. Science 1983, 220, 787–794. [Google Scholar] [CrossRef]
- Binder, K. Theory of first-order phase transitions. Rep. Prog. Phys. 1987, 50, 783–859. [Google Scholar] [CrossRef]
- Debenedetti, P.G. Metastable Liquids. Concepts and Principles; Princeton University Press: Princeton, NJ, USA, 1996. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cerdeiriña, C.A.; Troncoso, J. Ising Paradigm in Isobaric Ensembles. Entropy 2024, 26, 438. https://doi.org/10.3390/e26060438
Cerdeiriña CA, Troncoso J. Ising Paradigm in Isobaric Ensembles. Entropy. 2024; 26(6):438. https://doi.org/10.3390/e26060438
Chicago/Turabian StyleCerdeiriña, Claudio A., and Jacobo Troncoso. 2024. "Ising Paradigm in Isobaric Ensembles" Entropy 26, no. 6: 438. https://doi.org/10.3390/e26060438
APA StyleCerdeiriña, C. A., & Troncoso, J. (2024). Ising Paradigm in Isobaric Ensembles. Entropy, 26(6), 438. https://doi.org/10.3390/e26060438





