1. Introduction
An oscillating neutral object in vacuum emits photons, the so-called dynamical Casimir effect (DCE)—see [
1,
2,
3,
4] and references therein. Photons are produced in pairs that satisfy the condition
where
denote the frequencies of the generated photons and
is the mechanical frequency. The rate of photon production and its angular distribution were evaluated analytically for a perfectly conducting plate of infinite transverse size [
5]. For a macroscopic mirror,
is typically below the MHz range, hence implying that the produced photons have wavelengths of the order or greater than
Higher mechanical frequencies, in the GHz range, are achievable with thin-film resonators [
6] and with plasmonic nanoantennas [
7]. Recently, rotation frequencies beyond
were demonstrated with optically levitated nanoparticles [
8]. In all cases, the transverse size of the movable surface is typically much smaller than
. The dipole approximation then provides a much more realistic description than usual approaches based on the assumption of an infinite transverse size [
5,
9,
10,
11,
12,
13].
In this situation, the most convenient way to address the photon production is not by imposing boundary conditions on the moving plate [
14] and deriving a relation between output and input fields [
15,
16,
17] (for instance in terms of a Bogoliubov transformation [
18]), but rather to employ directly a Hamiltonian approach [
19,
20,
21,
22]. Within the dipolar approximation, this strategy was successfully applied to evaluate the generation of photon pairs by an oscillating atom [
23] in the microscopic dynamical Casimir effect (MDCE) [
24,
25,
26,
27,
28,
29,
30,
31]. In the present paper, we first revisit the MDCE effect by providing an alternative derivation of the associated Hamiltonian where the dipole motion gives rise to time-dependent higher-order multipole moments (
Section 2). Our result allows us to consider anisotropic scatterers, which turns out to be a key element for extending the Hamiltonian approach into the macroscopic domain. General results for the DCE are then derived in
Section 3, which are later applied to obtain the radiation emitted by a macroscopic metallic disk (
Section 4). A comparison with the usual paradigm of an infinite oscillating surface reveals that finite-size effects can play a predominant role in the DCE. Indeed, the usual model of an infinite plate leads to an overestimation of the photon production rate by many orders of magnitude for any realistic configuration. Therefore, it is crucial to take into account the finite size of the oscillating mirror when estimating the required sensitivity in future DCE experiments with single objects in vacuum, which is precisely the main purpose of the present work. We also consider, in
Section 5, an oscillating rod as a simpler illustration of the symmetries underlying the dynamical Casimir photons. Final remarks are presented in
Section 6.
2. Effective Multipolar Hamiltonian for Moving Scatterers
In this Section, we shall consider a neutral polarizable object composed of several charged particles, with no permanent dipole, whose center-of-mass (CM) is set into a non-relativistic but otherwise arbitrarily prescribed motion . The CM motion is always treated classically, and the electromagnetic response of the object may be strongly anisotropic. The considered object may be either microscopic (e.g., a molecule), or macroscopic (e.g., a disk mirror). Our approach rests on the electric dipole approximation, and thus applies as long as the relevant electromagnetic wavelengths are much larger than the system size—this will be the case for all examples considered throughout this paper. As mentioned in the introduction, this condition is also largely satisfied in realistic DCE experiments.
In an inertial frame where the dipole is instantaneously at rest, the Hamiltonian describing the interaction in the Schrödinger picture is , where is the dipole operator of the system and is the electric field operator evaluated at the dipole CM position. We may obtain the corresponding laboratory-frame Hamiltonian by using a Lorentz boost to relate the field to different frames, as is usually carried out in the literature. However, here we shall follow an alternative path by recognizing that a moving dipole can always be described in terms of multipoles which are fixed at the origin of the coordinate system. In order to do so, we express the Hamiltonian by considering the expansion up to the electric quadrupole and magnetic dipole order. We start with a classical description and subsequently follow a standard procedure to quantize it.
We start with the usual minimal coupling framework, where the Lagrangian density for the matter–light interaction is given by
where
is the four-charge current and
is the four potential in Gaussian units. Let us assume that our charge distribution is neutral and may be treated within the multipolar approximation up to the electric quadrupole and dipole magnetic terms. We may derive the multipolar Lagrangian from Equation (
1) following standard procedures [
32] of adding a total time derivative, but it will be simpler to follow an alternative route by explicitly employing the charge and current density associated with the multipoles [
33]:
where, from now on, we use Einstein’s notation by summing over repeated indices.
and
represent the components of the electric dipole and quadrupole moments, respectively, while
contains the second-order correction to the electric current. Its symmetric part
captures the electric quadrupole’s contribution to the current, while its antisymmetric part can be written as the dual tensor to the magnetic dipole moment
, namely
(
is the Levi-Civita symbol).
Let us evaluate the multipole moments of our moving system. We seek a general description which is valid for any motion compatible with the dipole approximation, i.e., such that the system emits mostly in wavelengths much larger than its size. We thus take as our exact charge distribution , where represents the position of an arbitrary point attached to the system and is the electric dipole moment of our moving system with respect to . As a consistency check, this charge distribution yields an electric dipole moment , independently of the system position as expected for a neutral system. Consequently, higher-order multipole moments carry all the signatures of dynamical motion. For the same reason we do not have to worry about the intrinsic electric quadrupole and magnetic dipole moments of our system as their dynamical corrections will manifest only in the electric octopole and magnetic quadrupole terms, and thus can be neglected.
In order to evaluate the tensor
, we first write the electric current. For the considered model, it is given by
, where
is the moving dipole velocity. The first term is nothing but the usual
contribution to the current. The second term is the polarization current, originating from the time variation in the electric dipole, which ensures consistency with the local charge conservation
. One obtains
We work in the Coulomb gauge and set
which amounts to discard the self-energies of the multipoles [
34]. We note from now on that
and integrate Equation (
1) to write the interaction Lagrangian as
by virtue of Equations (
3) and (
4). Adding a total time derivative to the Lagrangian does not affect the equations of motion for the field. Thus, the transformation
with
generates the equivalent Lagrangian
where
is the electric field. By combining the last two terms (with an exchange of the summation indices), one obtains a magnetic contribution of the form
. One can then express the Lagrangian in terms of the EM fields as
We may now follow the usual quantization procedure [
34] and write the interaction Hamiltonian in the Schrödinger picture
From now on
is an operator, as well as the electric and magnetic fields, given explicitly by
where
V is the quantization volume (we shall take
in the end),
,
(
) is the annihilation (creation) operator for the mode
, and
is the unit vector for polarization
, assumed to be real without the loss of generality.
The Hamiltonian (
8) acts on a quantum system composed of the internal degrees of freedom (dofs) of the scatterer and of the electromagnetic field’s dofs. Its explicit time dependence accounts for the coupling of this quantum system with the center-of-mass motion (treated here classically), which drives the DCE radiation process and provides the corresponding energy. We consider from now on a prescribed harmonic motion of the form
The relevant (i.e., significantly populated) DCE mode frequencies lie in the interval
.
Let us discuss the magnitude of the successive terms of the interaction Hamiltonian (
8), noting for convenience
,
and
. One finds that
. Thus, the term
appears as a first-order relativistic correction in the scatterer velocity
to the static dipole interaction. A similar argument holds for the Röntgen term
:
. Similarly, one finds that the successive multipole contributions (capturing the dynamical motion of the dipole) are scaled as increasing powers of
. Working up to the first nonrelativistic order, one retrieves the usual interaction Hamiltonian
corresponding to a Lorentz transformation of the electric field to the instantaneous rest frame.
Let us now comment on the specific internal quantum structure of the moving scatterer. For atoms and molecules, the frequency of the prescribed mechanical harmonic motion is typically too small to excite internal transitions. On the other hand, low-frequency internal excitation channels are usually available in macroscopic scatterers made of a dissipative material, as for instance, a metallic mirror. Such internal dissipation channels allow for a quantum friction between scatterers in relative motion in a vacuum [
35], which we disregard since our focus is the dynamical Casimir effect for a single moving scatterer. Thus, we assume that the internal dofs remain in their ground state throughout the motion so that photons can only be produced in pairs due to the second order of perturbation theory in the Hamiltonian (
8).
Alternatively, we can build up an effective Hamiltonian which takes into account, by construction, the virtual internal transitions of the system. Using Equations (
9) and (
10), we write the interaction Hamiltonian (
8) as
with the field operator
given in terms of time-dependent coefficients
as follows:
This allows us to perform the same unitary transform as in Ref. [
36] and work with the effective Hamiltonian
where
is the polarizability of the scatterer:
Here,
and
denote the (internal) ground and excited states, respectively, and
stands for the transition frequency.
The effective Hamiltonian (
12) generalizes the approach of Refs. [
36,
37,
38] to the case of moving scatterers. It has the convenience of capturing two-photon processes within a first-order perturbation theory description of the coupling between a moving neutral quantum system, polarizable but with no permanent dipole, and the quantum electromagnetic field. As mechanical frequencies are typically much smaller than the internal transition frequencies, we are entitled to neglect dispersion and take the static polarizability:
. With this approximation and employing Equation (
11) we obtain
where we have neglected higher-order contributions involving second spatial derivatives of order
In the last term we have used the symmetry of the polarizability tensor as well as the fact that fields components at the same point commute with each other, as can be appreciated in Equations (
9) and (
10). The effective Hamiltonian (
14) is not restricted to microscopic quantum systems. It also captures the radiation of moving macroscopic scatterers treated in the dipole approximation, and will be our departure point for investigating illustrative examples of DCE in the next sections.
3. Photon Emission Rate
In this section we derive the dynamical Casimir photon emission rate from the effective Hamiltonian (
14). As already mentioned in the previous section, we consider that our scatterer is moving harmonically according to
, where
is a constant vector denoting the amplitude of the motion. We choose to express the amplitude in the form
, where the constant
v denotes the maximum velocity achieved by the scatterer. The Hamiltonian (
14) then assumes the form
The operators
and
capture the spatial variation in the electric field and the Röntgen current, respectively. As derived in the previous section, they arise as combinations of magnetic dipole and electric quadrupole contributions resulting from the motion of the scatterer considered within the electric dipole approximation and are given by
Finally, note that, in the particular case of isotropic scatters,
(
15)–(17) agree with Ref. [
23].
A great convenience of our effective Hamiltonian is that it involves only field dofs. Within first-order perturbation theory, only two-photon states can be generated from the initial vacuum state; a well-known feature of DCE: photons are generated in pairs. More concretely, the field state reads as
where
denotes the vacuum state of the electromagnetic field, while
denotes a state with two photons; one in mode
and the other in mode
. We are interested in the long time limit
, where Fermi’s golden rule implies that
connects only states differing by
in energy. Denoting the frequencies of the produced photons by
and
, we have
This is a remarkable feature of a sinusoidal perturbation, since the center of mass is here treated as classical and nonetheless exchanges energy only in the
quanta of
, which is analogous to what happens in the photoelectric effect, which can be explained by a classical description of the electromagnetic field [
39]. We find the photon emission rate for a given pair of photons
from the corresponding amplitude for pair production:
Providing that the polarizability tensor is symmetric, we find
where
and
The first term within brackets in (
21), arising from the operator
contains a non-trivial frequency dependence associated with
x and
that results from taking the gradient of the electric field operators in (
16). The second term, proportional to
captures the contribution of the Röntgen operator
and does not introduce any additional frequency dependence apart from the prefactor
which comes from the density of states. In a case where the scatterer is randomly rotating in a time scale much smaller than the typical emission time, we may substitute the polarizability tensor with its average
, with
. In this case, we reobtain the result for an isotropic system [
23].
We may integrate Equation (
21) with
and sum with
in order to obtain the angular spectral distribution of the DCE radiation. This can be readily carried out by employing the symmetrical properties of the rotation group, as detailed in appendix B of ref. [
23], which yields the result
where we have written
and
We emphasize that expression (
22) is valid only for
. Indeed, the photon production rate vanishes outside this interval to become first order in the perturbation as it is not possible to satisfy condition (
19). Summation over the polarization and integration over the solid angle yields the frequency spectral rate for photon production:
As expected, since the photons are generated in pairs satisfying condition (
19), the spectrum is symmetrical around
Indeed, the spectrum (
24) is invariant under the exchange
. Also, it vanishes at
, as it should since the electromagnetic field density of states vanishes in this case. Given the symmetry of the spectrum, it also vanishes at
Naturally, the right-hand-side of Equation (
24) is positive definite in the physical region
, as can be immediately verified by noticing that
(this inequality is readily established once the (symmetrical) polarizability tensor is expressed in its principal-axes basis). Photon production is maximized when the vector
has the smallest magnitude, which happens when the motion is aligned along the principal axis of the polarizability tensor with the smallest eigenvalue.
Finally, the total photon production rate is given by
Let us consider an almost isotropic scatterer, where the principal values of the polarizability are given by
, with
and
. We consider the motion to be parallel to the principal axis
j of the scatterer, so that to first-order expression (
25) simplifies to
We see that photon production is enhanced by a small anisotropy which diminishes the polarization along the direction of motion (
). In the following sections we shall examine in more detail two cases in which the anisotropy is not small.
5. Thin Cylindrical Metallic Rod
As a final example we shall consider a thin cylindrical metallic rod of length
L and small radius
, as depicted in
Figure 5. Denoting its symmetry axis by
z, the dominant contribution to the polarizability comes from the element [
40]
. Note that even this element vanishes in the limit
, indicating that the DCE in this situation is far smaller than in the case of a flat mirror discussed in
Section 4, as expected, since we now have essentially a one-dimensional scatterer. As in the previous section, we first evaluate the angular distribution from the general result (
22).
As usual when dealing with cylindrical symmetry, we define the transverse electric polarization with the condition that the electric field is orthogonal to the rod’s symmetry axis. We then define the unit vectors
and
as the unit vectors for TE and TM polarizations, respectively. The angular spectra are generally given by
When the motion is perpendicular to the rod’s symmetry axis (see
Figure 5a), the angular dependence is captured by the functions
where
is the angle between
and the rod’s symmetry axis (
z-axis), while
denotes the angle between the direction of motion (unit vector
) and the projection of
on the plane perpendicular to the rod (
plane).
At
, the spectra for TE and TM polarizations are proportional to
and
, respectively. Analogous with the case of a disk moving sideways discussed in
Section 4.2, such dependence on
results from a vector decomposition on the polarization basis in the limit
The unit vector along the direction of motion reads
Thus, Equations (44) and (45) show that the DCE radiation emitted parallel to the symmetry axis (
) is linearly polarized along the direction of motion, in contrast with the analog case for a disk, for which the radiation is partially polarized along the direction perpendicular to the motion.
As
does not depend on
x, the frequency dependence of the spectrum for TE polarization is entirely contained in the pre-factor appearing in (
43), which arises from the electromagnetic density of states. Such a property can also be seen in Equation (
21) since
. In other words, only the Röntgen current contributes to the emission of TE-polarized photons.
The plane
defined by
and by the rod’s axis, is a symmetry plane with respect to space reflection. Therefore, dynamical Casimir photons emitted at
are necessarily TM-polarized by symmetry (see
Section 4.2 for a similar discussion concerning a flat mirror moving sideways).
Equation (44) also shows that TE-polarized photons are mostly emitted close to the direction of the rod’s axis, as illustrated by
Figure 6a for the case
. In addition, for any given
, the emission is maximal along the direction perpendicular to the motion (
).
As for the TM angular spectrum, it has a maximum at
for any given value of
On the plane
, the TM angular spectrum for frequencies
is maximal along the direction parallel to the rod’s axis, as for example in the case
shown in
Figure 6b, while lower frequency photons are mostly emitted parallel to the direction of motion (
).
Finally, when the rod is moving parallel to its axis (see
Figure 5b), the angular spectrum is axially symmetric and hence independent of
In this configuration, any plane containing the rod is a symmetry plane, thus explaining the complete absence of TE photons. Note that the angular spectrum vanishes at
as expected since the results for TM and TE polarizations must coincide in this limit. Low-frequency photons are mostly emitted perpendicularly to the motion (
). As the frequency increases, the angle of maximum emission decreases and approaches
as
Figure 6c shows the angular spectrum for
Note that the angular distributions obtained for both the disk and the rod, illustrated by
Figure 2 and
Figure 6, respectively, are combinations of the familiar patterns for dipole and quadrupole radiation. The derivation of the Hamiltonian presented in
Section 2 explains the origin of this resemblance. Indeed, the motion of the scatterer can be represented in terms of time-dependent electric quadrupole and magnetic dipole contributions.
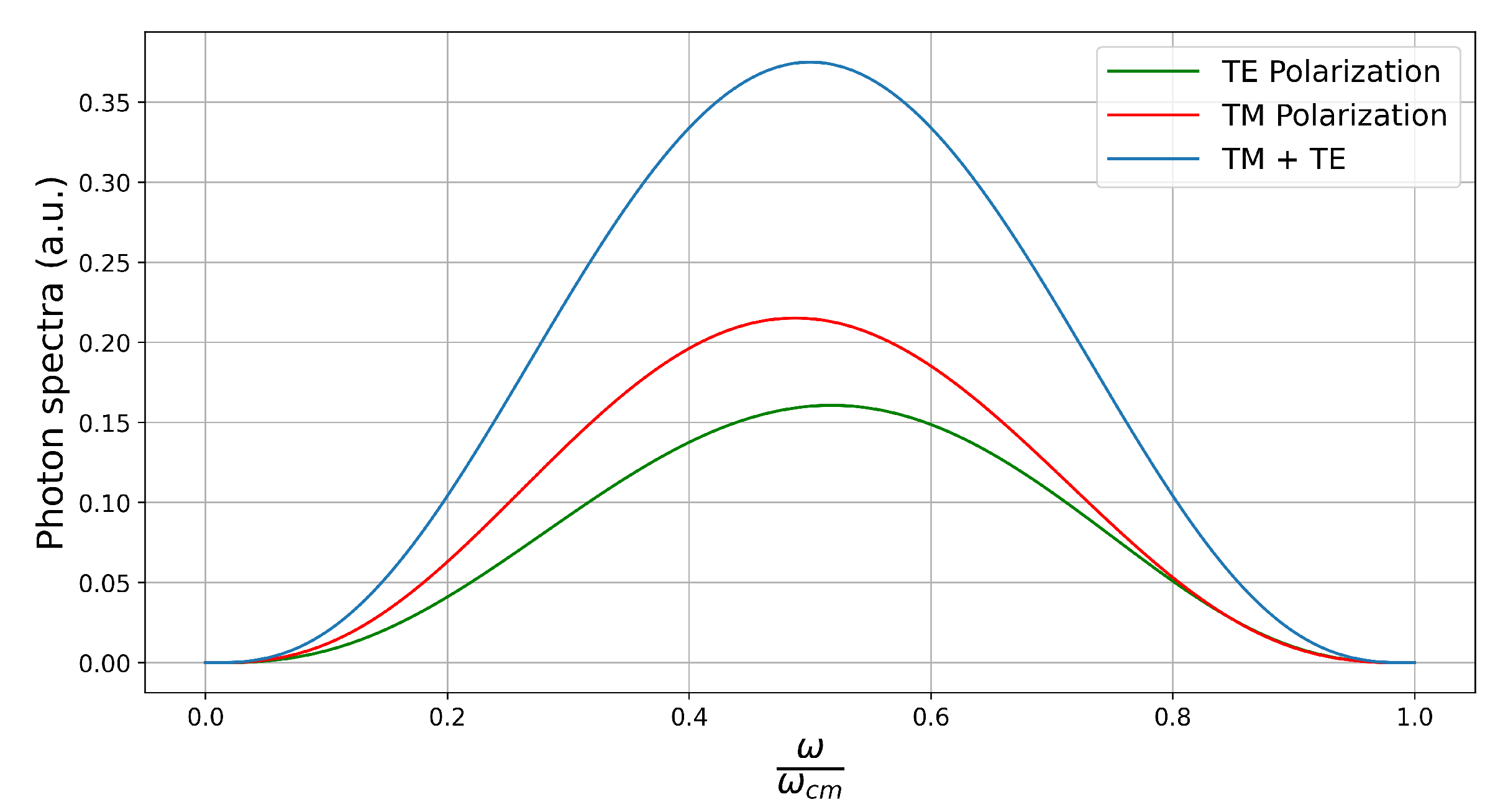
The frequency spectra for both perpendicular and parallel motions have the form
with
In contrast to the case of a flat mirror discussed in
Section 4, we see that each polarization spectrum is separately symmetrical with with respect to
since
is frequency independent. This property holds for any direction of motion and results from the fact that only the Röntgen current contributes to TE emission.
The total photon production rates are given by
By comparing (
53) with (54), we conclude that a motion parallel to the rod’s axis leads to 11 times less photons than a motion perpendicular to the axis. Such reduction factor can also be derived directly from the general result (
25).
6. Final Remarks
We have analyzed the dynamical Casimir radiation produced by anisotropic scatterers moving in the quantum vacuum. For the paradigmatic case of an oscillating mirror, the values for the photon wavelength are typically much larger than the transverse size of the mirror. Thus, modelling the mirror as a surface of infinite extension is not realistic.
Here, we have taken the opposite limit, corresponding to the electric dipole approximation, which provides a much more accurate description in most cases. We have analyzed the DCE for macroscopic neutral bodies without a permanent electric dipole, which emit no classical radiation, and for which the electromagnetic response is fully captured by their (generally anisotropic) polarizability tensor. Following these considerations, we have built up an effective Hamiltonian to capture the DCE radiation by macroscopic and anistropic scatterers. This effective Hamiltonian is obtained through a multipolar expansion involving electric quadrupole and magnetic dipole contributions that result from the motion of the scatterer. Such Hamiltonian formalism for anisotropic scatterers enabled us to extend into the macroscopic realm a previous approach of DCE restricted so far to microscopic systems [
23].
Considering a harmonic motion specifically, we obtained a general result for the DCE emission rate in terms of the polarizability tensor of the moving body. We find that the photon emission is maximum when the motion occurs along the principal axis corresponding to the smallest eigenvalue of this polarizability tensor. This result confirms the common intuition that DCE is maximal when the body moves along the direction of its smallest spatial extension, e.g., the normal to the surface of a moving disk.
We illustrated this approach in two examples that correspond to distinct geometries, namely a circular disk and a cylindrical rod. We have obtained the separate DCE contributions corresponding to the motion either parallel or perpendicular to the symmetry axis of each system. In particular, we have analyzed the most typical DCE configuration corresponding to the motion of a circular disk along its normal and compared the predictions of our dipole-approximation approach with the usual infinite plate model. Our findings reveal that the latter overestimates the DCE photon production rate by many orders of magnitude for any realistic configuration. There are also qualitative and conceptual differences: a finite-size disk can emit cross-polarized photon pairs, whereas photon pairs are necessarily co-polarized for an infinite plate model due to its translation symmetry. We have also discussed the manifestations of the translation symmetry breaking in the DCE angular and frequency spectra.
Finally, our Hamiltonian approach holds as long as the dipole approximation is applicable, which is typically the case in configurations involving finite-size objects undergoing a non-relativistic motion. Thus, our approach can be applied not only to DCE with an isolated single scatterer, but also to analyze more general QED effects involving the non-relativistic motion of one or several bodies.