Interplay of Spin Nernst Effect and Entanglement Negativity in Layered Ferrimagnets: A Study via Exact Diagonalization
Abstract
1. Introduction
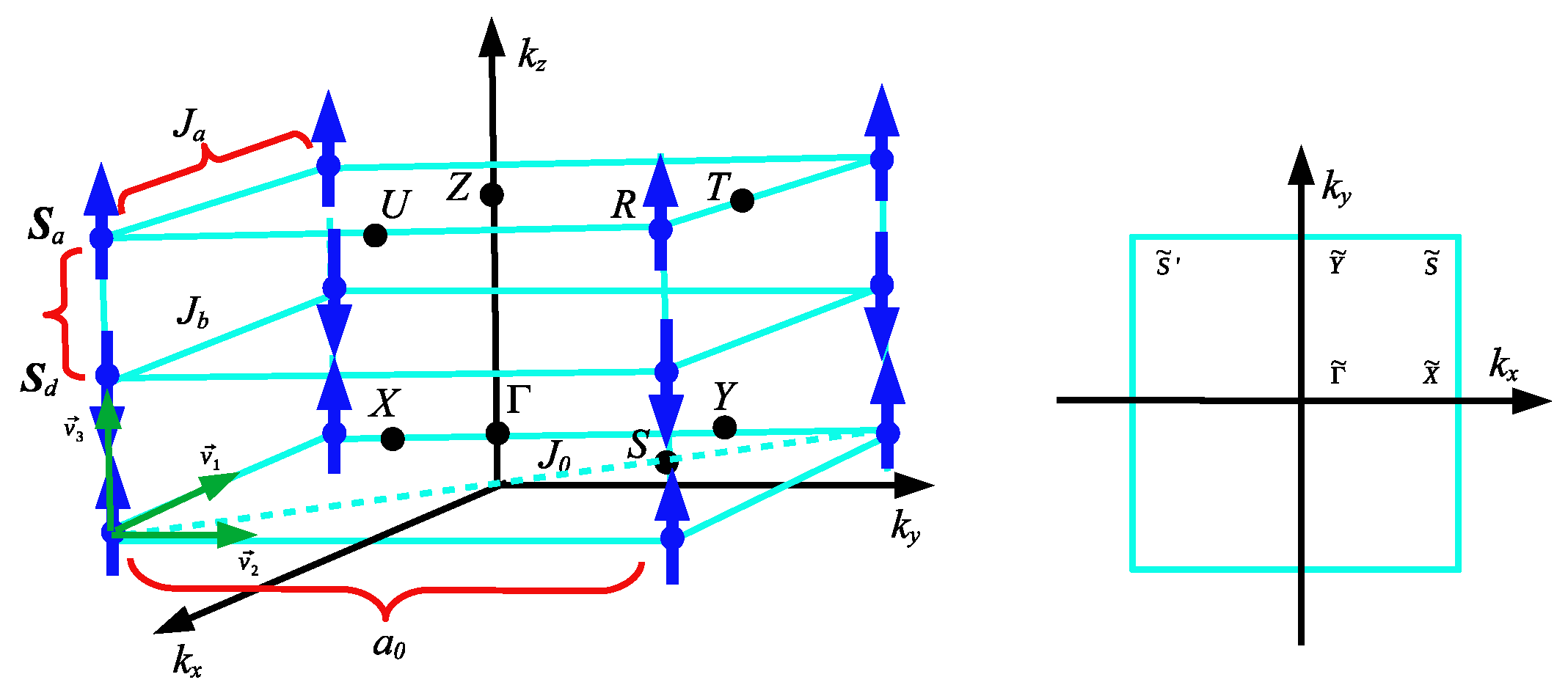
2. Model
3. Results
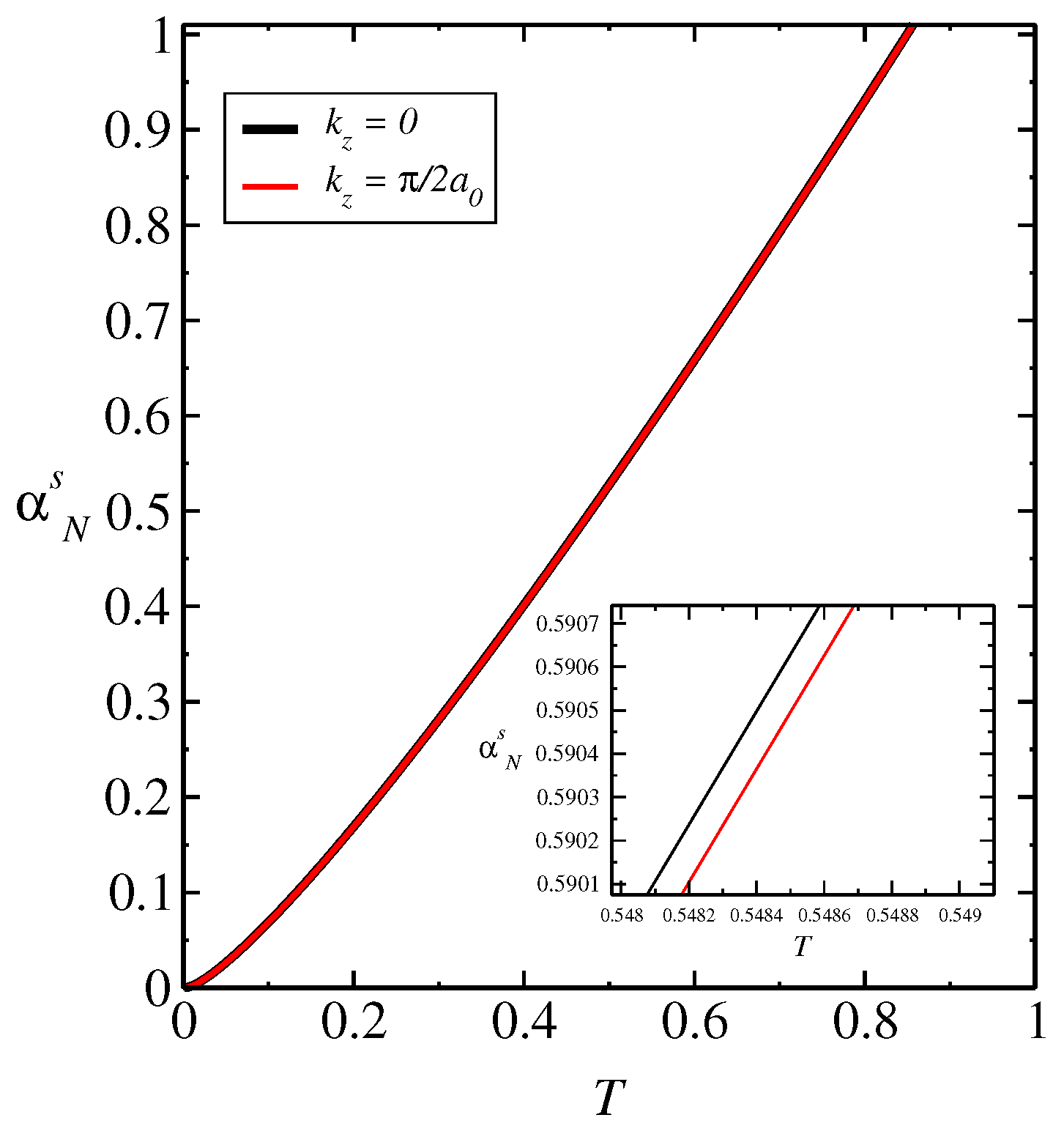
3.1. Negativity
3.2. Lanczos Algorithm and Exact Diagonalization
3.3. Analysis by SWT Approach
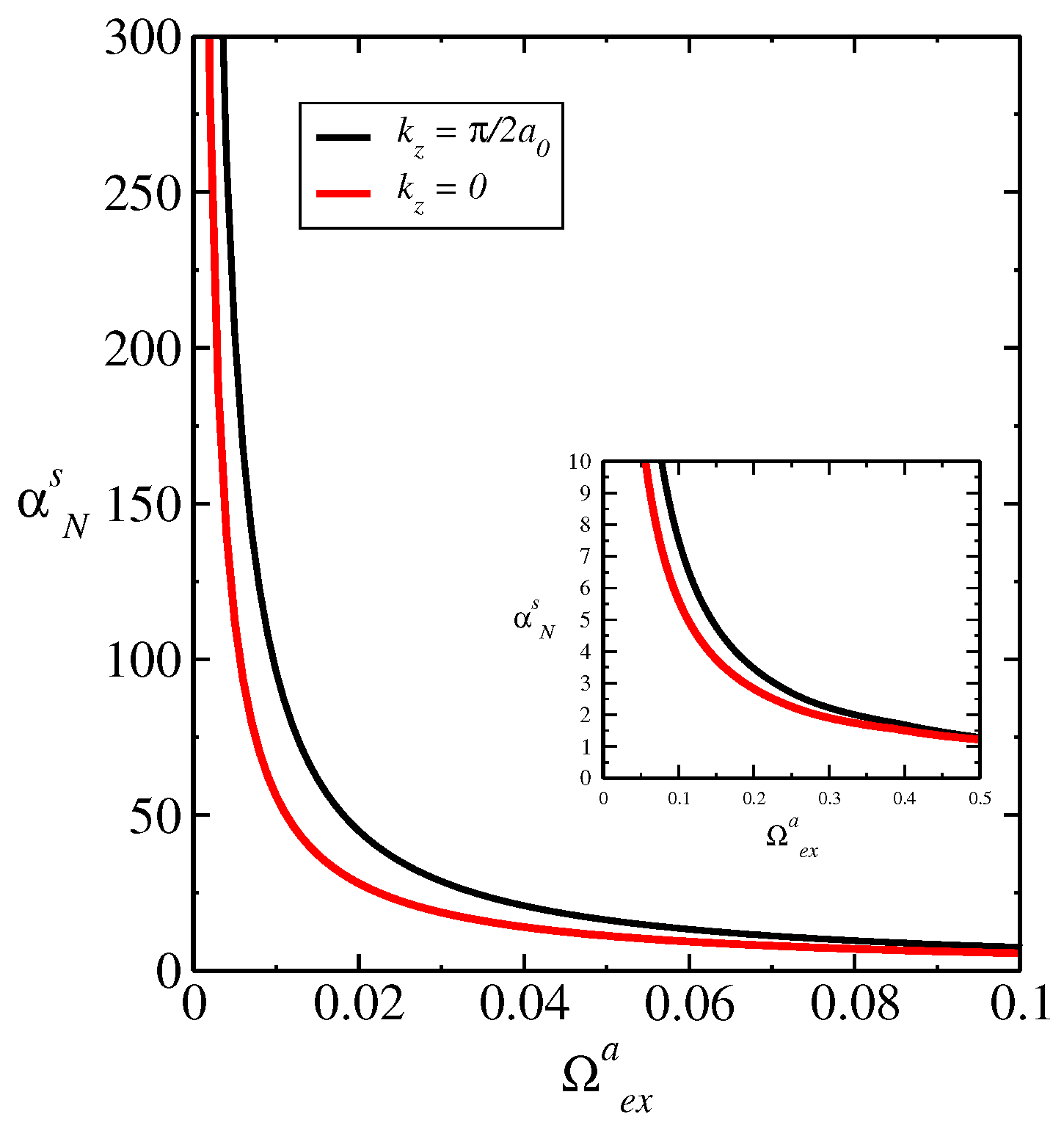
3.4. Magnon Nernst Effect
4. Outlook
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Fradkin, E. Field Theories of Condensed Matter Physics; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Laflorencie, N. Quantum entanglement in condensed matter systems. Phys. Rep. 2016, 646, 1–59. [Google Scholar] [CrossRef]
- Lacroix, C.; Mendels, P.; Mila, F. Introduction to Frustrated Magnetism; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Altland, A.; Simons, B. Condensed Matter Field Theory; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Liu, J.; Wang, L.; Shen, K. Tunable topological magnon phases in layered ferrimagnets. Phys. Rev. B 2023, 107, 174404. [Google Scholar] [CrossRef]
- Lima, L.S. Topological magnon bands, quantum correlation and spin conductivity in layered ferrimagnets. J. Magn. Magn. Mater 2024, 590, 171673. [Google Scholar] [CrossRef]
- Owerre, S.A. Topological thermal Hall effect in frustrated kagome antiferromagnets. Phys. Rev. B 2017, 95, 014422. [Google Scholar] [CrossRef]
- Owerre, S.A. Magnon Hall effect in AB-stacked bilayer honeycomb quantum magnets. Phys. Rev. B 2016, 94, 094405. [Google Scholar] [CrossRef]
- Owerre, S.A. A first theoretical realization of honeycomb topological magnon insulator. J. Phys. Condens. Matter 2016, 28, 386001. [Google Scholar] [CrossRef]
- Lima, L.S. Spin Nernst effect and quantum entanglement in two-dimensional antiferromagnets on checkerboard lattice. Phys. E 2021, 128, 114580. [Google Scholar] [CrossRef]
- Katsura, H.; Nagaosa, N.; Lee, P.A. Theory of the thermal Hall effect in quantum magnets. Phys. Rev. Lett. 2010, 104, 066403. [Google Scholar] [CrossRef] [PubMed]
- Zhang, L.; Ren, J.; Wang, J.-S.; Li, B. Topological magnon insulator in insulating ferromagnet. Phys. Rev. B 2013, 87, 144101. [Google Scholar] [CrossRef]
- Mook, A.; Henk, J.; Mertig, I. Magnon Hall effect and topology in kagome lattices: A theoretical investigation. Phys. Rev. B 2014, 89, 134409. [Google Scholar] [CrossRef]
- Matsumoto, R.; Shindou, R.; Murakami, S. Thermal Hall effect of magnons in magnets with dipolar interaction. Phys. Rev. B 2014, 89, 054420. [Google Scholar] [CrossRef]
- Laurell, P.; Fiete, G.A. Magnon thermal Hall effect in kagome antiferromagnets with Dzyaloshinskii-Moriya interactions. Phys. Rev. B 2018, 98, 094419. [Google Scholar] [CrossRef]
- Kim, K.-S.; Lee, K.H.; Chung, S.B.; Park, J.-G. Magnon topology and thermal Hall effect in trimerized triangular lattice antiferromagnet. Phys. Rev. B 2019, 100, 064412. [Google Scholar] [CrossRef]
- Chen, L. Topological magnon insulator with Dzyaloshinskii–Moriya interaction under the irradiation of light. Chin. Phys. B 2019, 28, 078503. [Google Scholar] [CrossRef]
- Mook, A.; Henk, J.; Mertig, I. Thermal Hall effect in noncollinear coplanar insulating antiferromagnets. Phys. Rev. B 2019, 99, 014427. [Google Scholar] [CrossRef]
- Li, Z.-X.; Cao, Y.; Yan, P. Topological insulators and semimetals in classical magnetic systems. Phys. Rep. 2021, 915, 1–64. [Google Scholar] [CrossRef]
- Cai, Z.; Bao, S.; Gu, Z.; Gao, Y.; Ma, Z.; Shangguan, Y.; Si, W.; Dong, Z.; Wang, W.; Wu, Y.; et al. Topological magnon insulator spin excitations in the two-dimensional ferromagnet CrBr3. Phys. Rev. B 2021, 104, L020402. [Google Scholar] [CrossRef]
- Zhu, F.; Zhang, L.; Wang, X.; dos Santos, F.J.; Song, J.; Mueller, T.; Schmalzl, K.; Schmidt, W.F.; Ivanov, A.; Park, J.T.; et al. Topological magnon insulators in two-dimensional van der Waals ferromagnets CrSiTe3 and CrGeTe3: Toward intrinsic gap-tunability. Sci. Adv. 2021, 7, eabi7532. [Google Scholar] [CrossRef]
- Mook, A.; Plekhanov, K.; Klinovaja, J.; Loss, D. Interaction-stabilized topological magnon insulator in ferromagnets. Phys. Rev. X 2021, 11, 021061. [Google Scholar] [CrossRef]
- Zhang, Z.; Feng, W.; Yao, Y.; Tang, B. Photoinduced Floquet topological magnons in a ferromagnetic checkerboard lattice. Phys. Lett. A 2021, 414, 127630. [Google Scholar] [CrossRef]
- McClarty, P.A. Topological magnons: A review. Annu. Rev. Condens. Matter Phys. 2022, 13, 171. [Google Scholar] [CrossRef]
- Zyuzin, V.A.; Kovalev, A.A. Magnon spin Nernst effect in antiferromagnets. Phys. Rev. Lett. 2016, 117, 217203. [Google Scholar] [CrossRef] [PubMed]
- Cheng, R.; Okamoto, S.; Xiao, D. Spin Nernst effect of magnons in collinear antiferromagnets. Phys. Rev. Lett. 2016, 117, 217202. [Google Scholar] [CrossRef] [PubMed]
- Ma, B.; Fiete, G.A. Intrinsic magnon Nernst effect in pyrochlore iridate thin films. Phys. Rev. B 2021, 104, 174410. [Google Scholar] [CrossRef]
- Holstein, T.; Primakoff, H. Field dependence of the intrinsic domain magnetization of a ferromagnet. Phys. Rev. 1940, 58, 1098. [Google Scholar] [CrossRef]
- White, S.R. Density matrix formulation for quantum renormalization groups. Phys. Rev. Lett. 1992, 69, 2863. [Google Scholar] [CrossRef]
- White, S.R. Density-matrix algorithms for quantum renormalization groups. Phys. Rev. B 1993, 48, 10345. [Google Scholar] [CrossRef]
- Syljnásen, O.F.; Sandvik, A.W. Quantum Monte Carlo with directed loops. Phys. Rev. E 2002, 66, 046701. [Google Scholar] [CrossRef]
- Bauer, B.; Carr, L.D.; Evertz, H.G.; Feiguin, A.; Freire, J.; Fuchs, S.; Gamper, L.; Gukelberger, J.; Gull, E.; Guertler, S.; et al. The ALPS project release 2.0: Open source software for strongly correlated systems. J. Stat. Mech. 2011, 2011, P05001. [Google Scholar] [CrossRef]
- Lanczos, C. An Iteration Method for the Solution of the Eigenvalue Problem of Linear Differential and Integral Operators. J. Res. Nat. Bur. Stand. 1950, 49, 255. [Google Scholar] [CrossRef]
- Golub, G.H.; van Loan, C.F. Matrix Computations; Johns Hopkins University Press: Baltimore, MD, USA, 1996. [Google Scholar]
- Arnoldi, W.E. The Principle of Minimized Iteration in the Solution of the Matrix Eigen problem. Q. Appl. Math. 1951, 9, 17. [Google Scholar] [CrossRef]
- Lima, L.S. Quantum correlation and entanglement in the Heisenberg model with biquadratic interaction on square lattice. Eur. Phys. J. D 2021, 75, 28. [Google Scholar] [CrossRef]
- Lima, L.S. Effect of Dzyaloshinskii–Moriya interaction on quantum entanglement in superconductors models of high Tc. Eur. Phys. J. D 2019, 73, 6. [Google Scholar] [CrossRef]
- Lima, L.S. Quantum correlation in the bilinear–biquadratic model for iron-based superconductors. Eur. Phys. J. Plus 2021, 136, 789. [Google Scholar] [CrossRef]
- Lima, L.S. Quantum Phase Transition and Quantum Correlation in the Two-dimensional Honeycomb-bilayer Lattice Antiferromagnet. J. Low Temp. Phys. 2021, 205, 112–125. [Google Scholar] [CrossRef]
- Lima, L.S. Thermal entanglement in the quantum XXZ model in triangular and bilayer honeycomb lattices. J. Low Temp. Phys. 2020, 198, 241–251. [Google Scholar] [CrossRef]
- Lima, L.S. Influence of Topological Phase Transition on Entanglement in the Spin-1 Antiferromagnetic XX Model in Two Dimensions. J. Low Temp. Phys. 2020, 201, 515. [Google Scholar] [CrossRef]
- Lima, L.S. Entanglement and quantum phase transition in the anisotropic two-dimensional XXZ model. Solid State Commun. 2020, 309, 113836. [Google Scholar] [CrossRef]
- Lima, L.S. Quantum entanglement and magnon Hall effect on the Lieb lattice model. Physica E 2022, 141, 115235. [Google Scholar] [CrossRef]
- Lima, L.S. Bipartite entanglement in non-Hermitian quantum spin systems. Physica E 2023, 148, 115659. [Google Scholar] [CrossRef]
- Lima, L.S. Effect of magnon bands on quantum entanglement in two-dimensional ferromagnets in the checkerboard lattice. Eur. Phys. J. Plus 2022, 137, 552. [Google Scholar] [CrossRef]
- Lima, L.S. Entanglement Negativity and Concurrence in Some Low-Dimensional Spin Systems. Entropy 2022, 24, 1629. [Google Scholar] [CrossRef] [PubMed]
- Ballentine, L.E. Resource letter IQM-2: Foundations of quantum mechanics since the Bell inequalities. Am. J. Phys. 1986, 55, 785. [Google Scholar] [CrossRef]
- DiVincenzo, D.P. Quantum computation. Science 1995, 270, 255. [Google Scholar] [CrossRef]
- Marinescu, D.C.; Marinescu, G.M. Approaching Quantum Computing; Pearson Prentice Hall: Upper Saddle River, NJ, USA, 2004. [Google Scholar]
- Nielsen, M.A.; Chuang, I.L. Quantum Computing and Quantum Information; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Its, A.R.; Jin, B.-Q.; Korepin, V.E. Entanglement in the XY spin chain. J. Phys. A Math. Gen. 2005, 38, 2975. [Google Scholar] [CrossRef]
- Latorre, J.I.; Rico, E.; Vidal, G. Ground state entanglement in quantum spin chains. Quant. Inf. Comput. 2004, 4, 48–92. [Google Scholar] [CrossRef]
- Bianchini, D.; Castro-Alvaredo, O.A.; Doyon, B.; Levi, E.; Ravanini, F. Entanglement entropy of non-unitary conformal field theory. J. Phys. A Math. Theor. 2014, 48, 04FT01. [Google Scholar] [CrossRef]
- Vidal, G.; Latorre, J.L.; Rico, E.I.; Kitaev, A. Entanglement in quantum critical phenomena. Phys. Rev. Lett. 2003, 90, 227902. [Google Scholar] [CrossRef]
- Latorre, J.I.; Riera, A. A short review on entanglement in quantum spin systems. J. Phys. A Math. Theor. 2009, 42, 504002. [Google Scholar] [CrossRef]
- Zyczkowski, K.; Horodecki, P.; Sanpera, A.; Lewenstein, M. Volume of the set of separable states. Phys. Rev. A 1998, 58, 883. [Google Scholar] [CrossRef]
- Vidal, G.; Werner, R.F. Computable measure of entanglement. Phys. Rev. A 2002, 65, 032314. [Google Scholar] [CrossRef]
- Plenio, M.B. Logarithmic negativity: A full entanglement monotone that is not convex. Phys. Rev. Lett. 2005, 95, 090503. [Google Scholar] [CrossRef] [PubMed]
- Castelnovo, C. Negativity and topological order in the toric code. Phys. Rev. A 2013, 88, 042319. [Google Scholar] [CrossRef]
- Lee, Y.A.; Vidal, G. Entanglement negativity and topological order. Phys. Rev. A 2013, 88, 042318. [Google Scholar] [CrossRef]
- Shen, K. Magnon spin relaxation and spin Hall effect due to the dipolar interaction in antiferromagnetic insulators. Phys. Rev. Lett. 2020, 124, 077201. [Google Scholar] [CrossRef]
- Shindou, R.; Ohe, J.-I.; Matsumoto, R.; Murakami, S.; Saitoh, E. Chiral spin-wave edge modes in dipolar magnetic thin films. Phys. Rev. B 2013, 87, 174402. [Google Scholar] [CrossRef]
- Hu, Z.; Fu, L.; Liu, L. Tunable magnonic chern bands and chiral spin currents in magnetic multilayers. Phys. Rev. Lett. 2022, 128, 217201. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lima, L.S. Interplay of Spin Nernst Effect and Entanglement Negativity in Layered Ferrimagnets: A Study via Exact Diagonalization. Entropy 2024, 26, 1060. https://doi.org/10.3390/e26121060
Lima LS. Interplay of Spin Nernst Effect and Entanglement Negativity in Layered Ferrimagnets: A Study via Exact Diagonalization. Entropy. 2024; 26(12):1060. https://doi.org/10.3390/e26121060
Chicago/Turabian StyleLima, Leonardo S. 2024. "Interplay of Spin Nernst Effect and Entanglement Negativity in Layered Ferrimagnets: A Study via Exact Diagonalization" Entropy 26, no. 12: 1060. https://doi.org/10.3390/e26121060
APA StyleLima, L. S. (2024). Interplay of Spin Nernst Effect and Entanglement Negativity in Layered Ferrimagnets: A Study via Exact Diagonalization. Entropy, 26(12), 1060. https://doi.org/10.3390/e26121060





