Evolution of Robustness in Growing Random Networks
Abstract
1. Introduction
2. Kirchhoff Index
2.1. Definitions
2.2. Lower Bound on
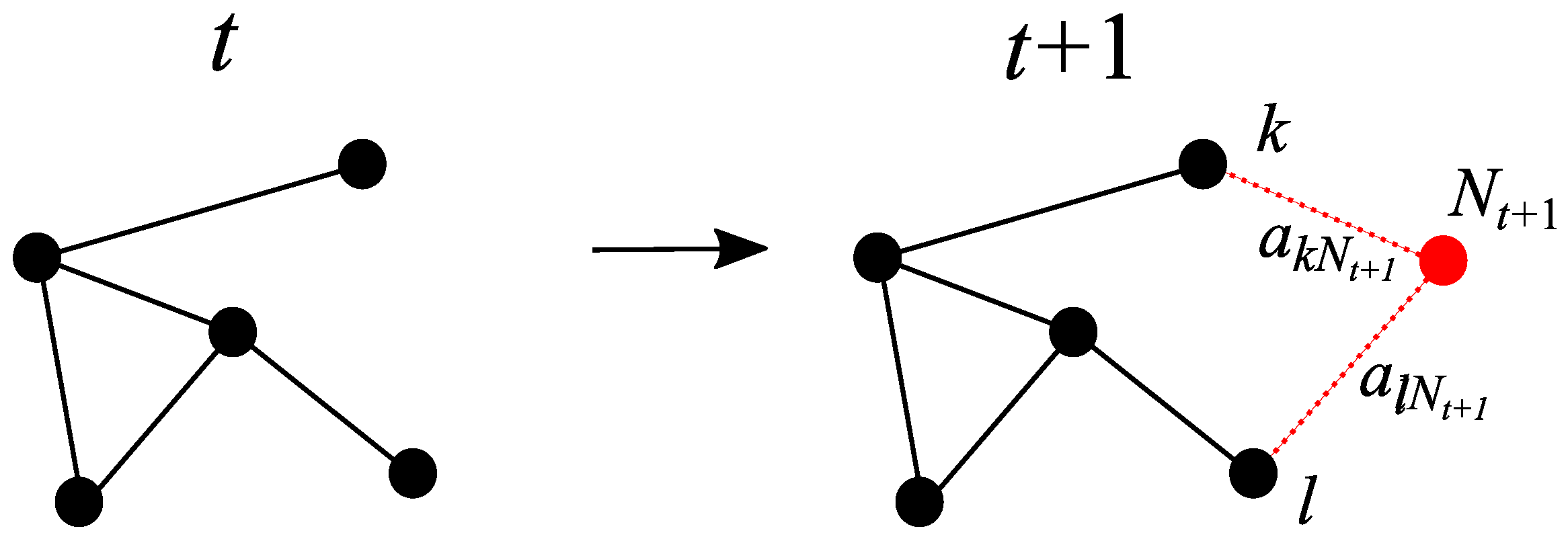
3. Robustness of Growing Networks
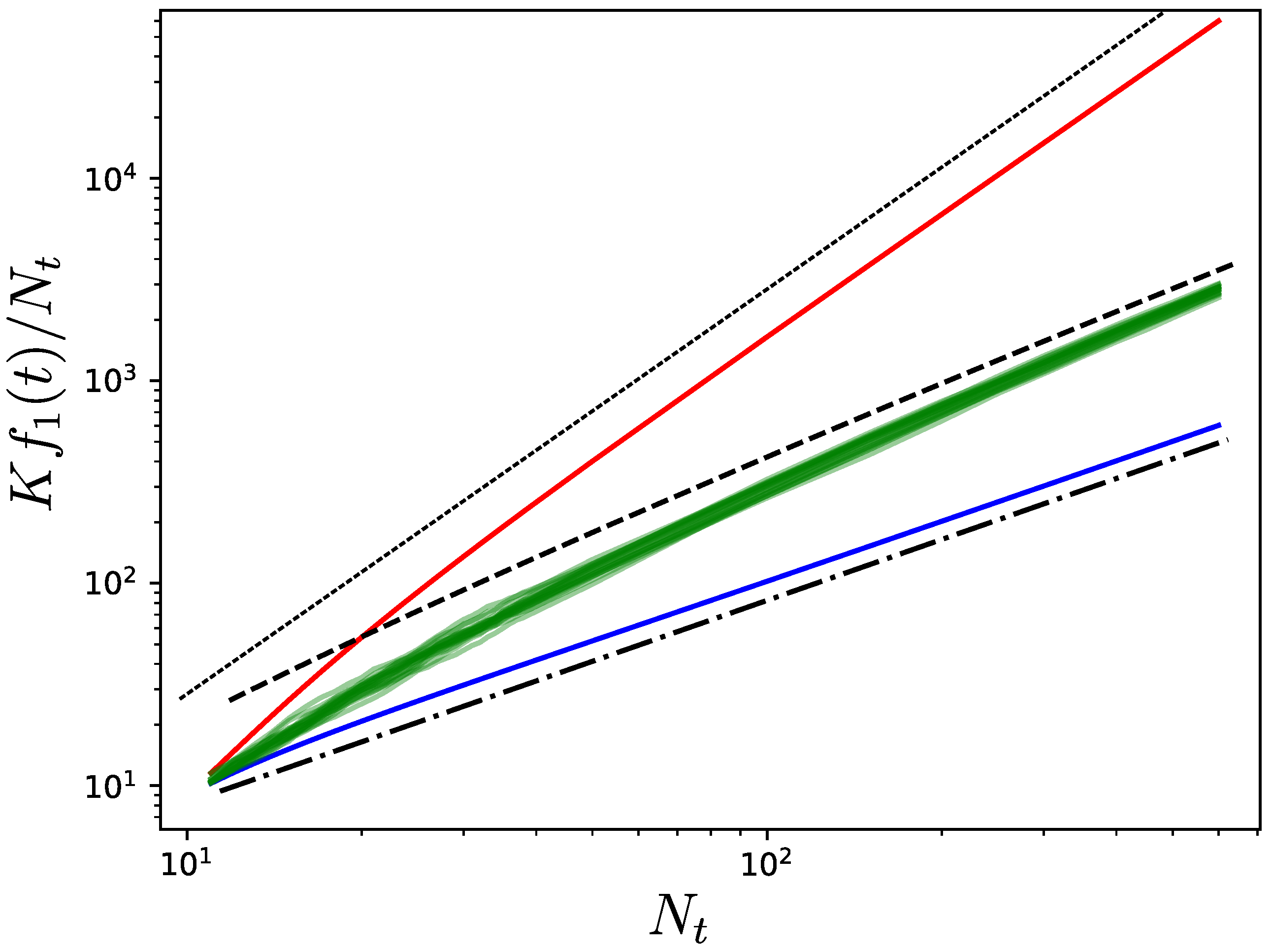
3.1. One New Node with a Single Connection ()
Discussion
3.2. One New Node with Two Connections ()
3.2.1. Discussion
3.2.2. Remark
4. Conclusions
Outlook
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Strogatz, S.H. Exploring complex networks. Nature 2001, 410, 268–276. [Google Scholar] [CrossRef] [PubMed]
- Pikovsky, A.; Rosenblum, M.; Kurths, J. Synchronization: A Universal Concept in Nonlinear Science; Cambridge University Press & Assessment: Cambridge, UK, 2001. [Google Scholar]
- Newman, M. Networks; Oxford University Press: Oxford, UK, 2018. [Google Scholar]
- Arenas, A.; Díaz-Guilera, A.; Kurths, J.; Moreno, Y.; Zhou, C. Synchronization in complex networks. Phys. Rep. 2008, 469, 93–153. [Google Scholar] [CrossRef]
- Boccaletti, S.; Latora, V.; Moreno, Y.; Chavez, M.; Hwang, D.U. Complex networks: Structure and dynamics. Phys. Rep. 2006, 424, 175–308. [Google Scholar] [CrossRef]
- Dorogovtsev, S.N.; Mendes, J.F. Evolution of Networks: From Biological Nets to the Internet and WWW; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Newman, M.E. Clustering and preferential attachment in growing networks. Phys. Rev. E 2001, 64, 025102. [Google Scholar] [CrossRef] [PubMed]
- Zhao, K.; Halu, A.; Severini, S.; Bianconi, G. Entropy rate of nonequilibrium growing networks. Phys. Rev. E 2011, 84, 066113. [Google Scholar] [CrossRef] [PubMed]
- Porter, M.A.; Gleeson, J.P. Dynamical Systems on Dynamical Networks. In Dynamical Systems on Networks: A Tutorial; Springer International Publishing: Cham, Switzerland, 2016; pp. 49–51. [Google Scholar]
- Della Rossa, F.; De Lellis, P. Synchronization and pinning control of stochastic coevolving networks. Annu. Rev. Control 2022, 53, 147–160. [Google Scholar] [CrossRef]
- Krapivsky, P.L.; Redner, S.; Leyvraz, F. Connectivity of growing random networks. Phys. Rev. Lett. 2000, 85, 4629. [Google Scholar] [CrossRef]
- Dorogovtsev, S.N.; Mendes, J.F.F.; Samukhin, A.N. Structure of growing networks with preferential linking. Phys. Rev. Lett. 2000, 85, 4633. [Google Scholar] [CrossRef]
- Neininger, R. The Wiener index of random trees. Comb. Probab. Comput. 2002, 11, 587–597. [Google Scholar] [CrossRef]
- Klein, D.J.; Randić, M. Resistance distance. J. Math. Chem. 1993, 12, 81–95. [Google Scholar] [CrossRef]
- Bonchev, D.; Balaban, A.T.; Liu, X.; Klein, D.J. Molecular cyclicity and centricity of polycyclic graphs. I. Cyclicity based on resistance distances or reciprocal distances. Int. J. Quantum Chem. 1994, 50, 1–20. [Google Scholar] [CrossRef]
- Babić, D.; Klein, D.J.; Lukovits, I.; Nikolić, S.; Trinajstić, N. Resistance-distance matrix: A computational algorithm and its application. Int. J. Quantum Chem. 2002, 90, 166–176. [Google Scholar] [CrossRef]
- Hosoya, H. Topological Index. A Newly Proposed Quantity Characterizing the Topological Nature of Structural Isomers of Saturated Hydrocarbons. Bull. Chem. Soc. Jpn. 1971, 44, 2332–2339. [Google Scholar] [CrossRef]
- Mohar, B.; Babic, D.; Trinajstic, N. A novel definition of the Wiener index for trees. J. Chem. Inf. Comput. Sci. 1993, 33, 153–154. [Google Scholar] [CrossRef]
- Tyloo, M.; Coletta, T.; Jacquod, P. Robustness of synchrony in complex networks and generalized Kirchhoff indices. Phys. Rev. Lett. 2018, 120, 084101. [Google Scholar] [CrossRef]
- Baumann, F.; Sokolov, I.M.; Tyloo, M. A laplacian approach to stubborn agents and their role in opinion formation on influence networks. Phys. A Stat. Mech. Its Appl. 2020, 557, 124869. [Google Scholar] [CrossRef]
- Tyloo, M.; Pagnier, L.; Jacquod, P. The key player problem in complex oscillator networks and electric power grids: Resistance centralities identify local vulnerabilities. Sci. Adv. 2019, 5, eaaw8359. [Google Scholar] [CrossRef]
- Ronellenfitsch, H.; Dunkel, J.; Wilczek, M. Optimal noise-canceling networks. Phys. Rev. Lett. 2018, 121, 208301. [Google Scholar] [CrossRef]
- Tyloo, M. Layered complex networks as fluctuation amplifiers. J. Phys. Complex. 2022, 3, 03LT01. [Google Scholar] [CrossRef]
- Lukovits, I.; Nikolić, S.; Trinajstić, N. Resistance distance in regular graphs. Int. J. Quantum Chem. 1999, 71, 217–225. [Google Scholar] [CrossRef]
- Zhou, B.; Trinajstić, N. A note on Kirchhoff index. Chem. Phys. Lett. 2008, 455, 120–123. [Google Scholar] [CrossRef]
- Golub, G.H.; Van Loan, C.F. Matrix Computations; JHU Press: Baltimore, MD, USA, 2013. [Google Scholar]
- Li, H.; Zhang, Z. Kirchhoff Index as a Measure of Edge Centrality in Weighted Networks: Nearly Linear Time Algorithms. In Proceedings of the 2018 Annual ACM-SIAM Symposium on Discrete Algorithms (SODA), New Orleans, LA, USA, 7–10 January 2018; pp. 2377–2396. [Google Scholar]
- Wagner, S. On the Wiener index of random trees. Part of special issue “Recent Trends in Graph Theory and Combinatorics”. Discret. Math. 2012, 312, 1502–1511. [Google Scholar] [CrossRef]
- Watts, D.J.; Strogatz, S.H. Collective dynamics of ‘small-world’ networks. Nature 1998, 393, 440–442. [Google Scholar] [CrossRef] [PubMed]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tyloo, M. Evolution of Robustness in Growing Random Networks. Entropy 2023, 25, 1340. https://doi.org/10.3390/e25091340
Tyloo M. Evolution of Robustness in Growing Random Networks. Entropy. 2023; 25(9):1340. https://doi.org/10.3390/e25091340
Chicago/Turabian StyleTyloo, Melvyn. 2023. "Evolution of Robustness in Growing Random Networks" Entropy 25, no. 9: 1340. https://doi.org/10.3390/e25091340
APA StyleTyloo, M. (2023). Evolution of Robustness in Growing Random Networks. Entropy, 25(9), 1340. https://doi.org/10.3390/e25091340






