Statistical Topology—Distribution and Density Correlations of Winding Numbers in Chiral Systems
Abstract
1. Introductory Remarks
2. Winding Numbers and Chirality
2.1. A Simple Topological Invariant in Complex Analysis
2.2. Kitaev Chain and Winding Numbers
2.3. Chirality, Random Winding Numbers and Modelling Aspects
2.4. Connections to Quantum Chromodynamics and Condensed Matter Physics
3. Formulation of the Problem and Mathematical Setup
3.1. Chiral Random Matrix Ensembles with Parametric Dependence
3.2. Statistical Quantities Considered
3.3. Mapping a Topological to a Spectral Problem
4. Results
4.1. Winding Number Correlators in the Chiral Unitary Case
4.2. Winding Number Distribution
4.3. Aspects of Universality
4.4. Generators in the Chiral Unitary and Symplectic Cases
5. Discussion and Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| RMT | Random Matrix Theory |
| QCD | Quantum Chromodynamics |
| chGUE | chiral Gaussian Unitary Ensemble |
| chGSE | chiral Gaussian Symplectic Ensemble |
| permutation group of N objects |
References
- Braun, P.; Hahn, N.; Waltner, D.; Gat, O.; Guhr, T. Winding number statistics of a parametric chiral unitary random matrix ensemble. J. Phys. A Math. Theor. 2022, 55, 224011. [Google Scholar] [CrossRef]
- Hahn, N.; Kieburg, M.; Gat, O.; Guhr, T. Winding Number Statistics for Chiral Random Matrices: Averaging Ratios of Determinants with Parametric Dependence. arXiv 2022, arXiv:2207.08612. [Google Scholar] [CrossRef]
- Kitaev, A.Y. Unpaired Majorana fermions in quantum wires. Physics-Uspekhi 2001, 44, 131–136. [Google Scholar] [CrossRef]
- Kitaev, A.Y. Anyons in an exactly solved model and beyond. Ann. Phys. 2006, 321, 2–111. [Google Scholar] [CrossRef]
- Pattanayak, A.; Pujari, S.; Dixit, G. Role of Majorana fermions in high-harmonic generation from Kitaev chain. Sci. Rep. 2022, 12, 6722. [Google Scholar] [CrossRef]
- Mehta, M.L. Random Matrices; Academic Press: Cambridge, MA, USA, 2004. [Google Scholar]
- Guhr, T.; Müller-Groeling, A.; Weidenmüller, H.A. Random-matrix theories in quantum physics: Common concepts. Phys. Rep. 1998, 299, 189–425. [Google Scholar] [CrossRef]
- Simons, B.D.; Altshuler, B.L. Universal velocity correlations in disordered and chaotic systems. Phys. Rev. Lett. 1993, 70, 4063–4066. [Google Scholar] [CrossRef]
- Simons, B.D.; Altshuler, B.L. Universalities in the spectra of disordered and chaotic systems. Phys. Rev. B 1993, 48, 5422–5438. [Google Scholar] [CrossRef]
- Verbaarschot, J. Spectrum of the QCD Dirac operator and chiral random matrix theory. Phys. Rev. Lett. 1994, 72, 2531–2533. [Google Scholar] [CrossRef]
- Verbaarschot, J.; Wettig, T. Random Matrix Theory and Chiral Symmetry in QCD. Annu. Rev. Nucl. Part. Sci. 2000, 50, 343–410. [Google Scholar] [CrossRef]
- Shuryak, E.; Verbaarschot, J. Random matrix theory and spectral sum rules for the Dirac operator in QCD. Nucl. Phys. A 1993, 560, 306–320. [Google Scholar] [CrossRef]
- Wettig, T.; Schäfer, A.; Weidenmüller, H. The chiral phase transition and random matrix models. Nucl. Phys. A 1996, 610, 492–499. [Google Scholar] [CrossRef]
- Wettig, T.; Schäfer, A.; Weidenmüller, H. The chiral phase transition in a random matrix model with molecular correlations. Phys. Lett. B 1996, 367, 28–34. [Google Scholar] [CrossRef]
- Jackson, A.D.; Verbaarschot, J.J.M. Random matrix model for chiral symmetry breaking. Phys. Rev. D 1996, 53, 7223–7230. [Google Scholar] [CrossRef] [PubMed]
- Verbaarschot, J.J.M.; Zahed, I. Spectral density of the QCD Dirac operator near zero virtuality. Phys. Rev. Lett. 1993, 70, 3852–3855. [Google Scholar] [CrossRef]
- Guhr, T.; Wilke, T.; Weidenmüller, H.A. Stochastic Field Theory for a Dirac Particle Propagating in Gauge Field Disorder. Phys. Rev. Lett. 2000, 85, 2252–2255. [Google Scholar] [CrossRef]
- Altland, A.; Zirnbauer, M.R. Nonstandard symmetry classes in mesoscopic normal-superconducting hybrid structures. Phys. Rev. B 1997, 55, 1142–1161. [Google Scholar] [CrossRef]
- Heinzner, P.; Huckleberry, A.; Zirnbauer, M.R. Symmetry Classes of Disordered Fermions. Commun. Math. Phys. 2005, 257, 725–771. [Google Scholar] [CrossRef]
- Kitaev, A. Periodic table for topological insulators and superconductors. AIP Conf. Proc. 2009, 1134, 22–30. [Google Scholar] [CrossRef]
- Schnyder, A.P.; Ryu, S.; Furusaki, A.; Ludwig, A.W.W. Classification of topological insulators and superconductors in three spatial dimensions. Phys. Rev. B 2008, 78, 195125. [Google Scholar] [CrossRef]
- Chiu, C.K.; Teo, J.C.Y.; Schnyder, A.P.; Ryu, S. Classification of topological quantum matter with symmetries. Rev. Mod. Phys. 2016, 88, 035005. [Google Scholar] [CrossRef]
- Oppermann, R. Anderson localization problems in gapless superconducting phases. Phys. A Stat. Mech. Its Appl. 1990, 167, 301–312. [Google Scholar] [CrossRef]
- Zirnbauer, M.R. Particle–hole symmetries in condensed matter. J. Math. Phys. 2021, 62, 021101. [Google Scholar] [CrossRef]
- Gade, R. Anderson localization for sublattice models. Nucl. Phys. B 1993, 398, 499–515. [Google Scholar] [CrossRef]
- Prodan, E.; Schulz-Baldes, H. Bulk and Boundary Invariants for Complex Topological Insulators: From K-Theory to Physics; Mathematical Physics Studies; Springer International Publishing: Cham, Switzerland, 2016. [Google Scholar] [CrossRef]
- Chen, B.H.; Chiou, D.W. An elementary rigorous proof of bulk-boundary correspondence in the generalized Su-Schrieffer-Heeger model. Phys. Lett. A 2020, 384, 126168. [Google Scholar] [CrossRef]
- Shapiro, J. The bulk-edge correspondence in three simple cases. Rev. Math. Phys. 2020, 32, 2030003. [Google Scholar] [CrossRef]
- Alicea, J. New directions in the pursuit of Majorana fermions in solid state systems. Rep. Prog. Phys. 2012, 75, 076501. [Google Scholar] [CrossRef] [PubMed]
- Walker, P.N.; Wilkinson, M. Universal Fluctuations of Chern Integers. Phys. Rev. Lett. 1995, 74, 4055–4058. [Google Scholar] [CrossRef] [PubMed]
- Gat, O.; Wilkinson, M. Correlations of quantum curvature and variance of Chern numbers. SciPost Phys. 2021, 10, 149. [Google Scholar] [CrossRef]
- Lu, L.; Joannopoulos, J.; Soljačić, M. Topological Photonics. Nat. Photonics 2014, 8, 821–829. [Google Scholar] [CrossRef]
- Maffei, M.; Dauphin, A.; Cardano, F.; Lewenstein, M.; Massignan, P. Topological characterization of chiral models through their long time dynamics. New J. Phys. 2018, 20, 013023. [Google Scholar] [CrossRef]
- Asbóth, J.K.; Oroszlány, L.; Pályi, A. A Short Course on Topological Insulators; Springer International Publishing: Berlin/Heidelberg, Germany, 2016. [Google Scholar] [CrossRef]
- Forrester, P.J.; Krishnapur, M. Derivation of an eigenvalue probability density function relating to the Poincaré disk. J. Phys. Math. Theor. 2009, 42, 385204. [Google Scholar] [CrossRef]
- Mays, A. A Real Quaternion Spherical Ensemble of Random Matrices. J. Stat. Phys. 2013, 153, 48–69. [Google Scholar] [CrossRef]
- Beenakker, C.; Rejaei, B. Random-matrix theory of parametric correlations in the spectra of disordered metals and chaotic billiards. Phys. A Stat. Mech. Its Appl. 1994, 203, 61–90. [Google Scholar] [CrossRef]
- Kieburg, M.; Guhr, T. Derivation of determinantal structures for random matrix ensembles in a new way. J. Phys. A 2010, 43, 075201. [Google Scholar] [CrossRef]
- Kieburg, M.; Guhr, T. A new approach to derive Pfaffian structures for random matrix ensembles. J. Phys. A 2010, 43, 135204. [Google Scholar] [CrossRef]


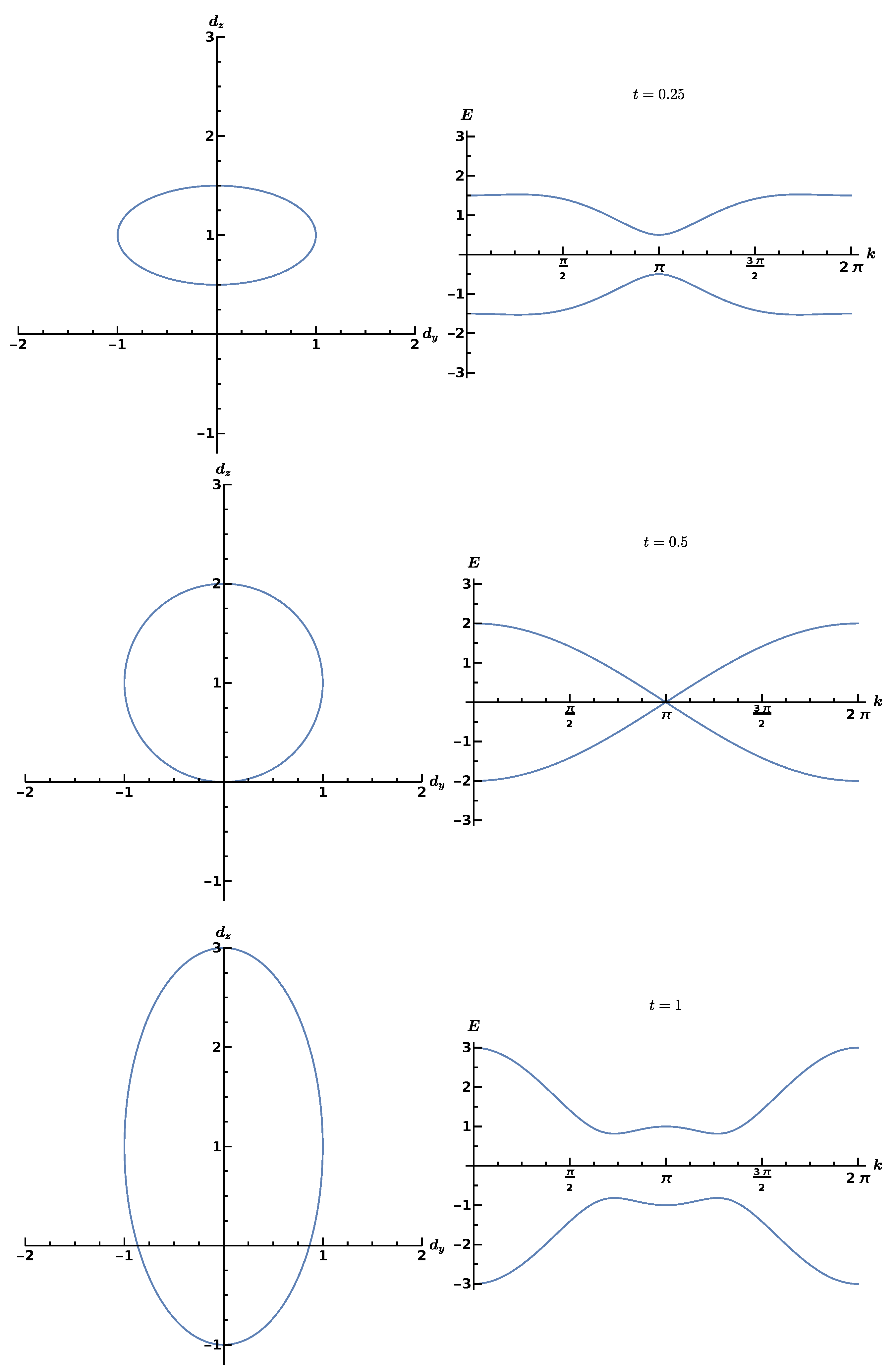
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guhr, T. Statistical Topology—Distribution and Density Correlations of Winding Numbers in Chiral Systems. Entropy 2023, 25, 383. https://doi.org/10.3390/e25020383
Guhr T. Statistical Topology—Distribution and Density Correlations of Winding Numbers in Chiral Systems. Entropy. 2023; 25(2):383. https://doi.org/10.3390/e25020383
Chicago/Turabian StyleGuhr, Thomas. 2023. "Statistical Topology—Distribution and Density Correlations of Winding Numbers in Chiral Systems" Entropy 25, no. 2: 383. https://doi.org/10.3390/e25020383
APA StyleGuhr, T. (2023). Statistical Topology—Distribution and Density Correlations of Winding Numbers in Chiral Systems. Entropy, 25(2), 383. https://doi.org/10.3390/e25020383






