Accurate Thermodynamic Properties of Ideal Bosons in a Highly Anisotropic 2D Harmonic Potential
Abstract
:1. Introduction
2. Thermodynamic Potential of the Ideal Boson System
2.1. Many-Particle State in the Particle-Number Representation
2.2. Thermodynamic Potential
3. Thermodynamic Properties of the Ideal Boson System
3.1. Ushering of the Reduced Chemical Potential
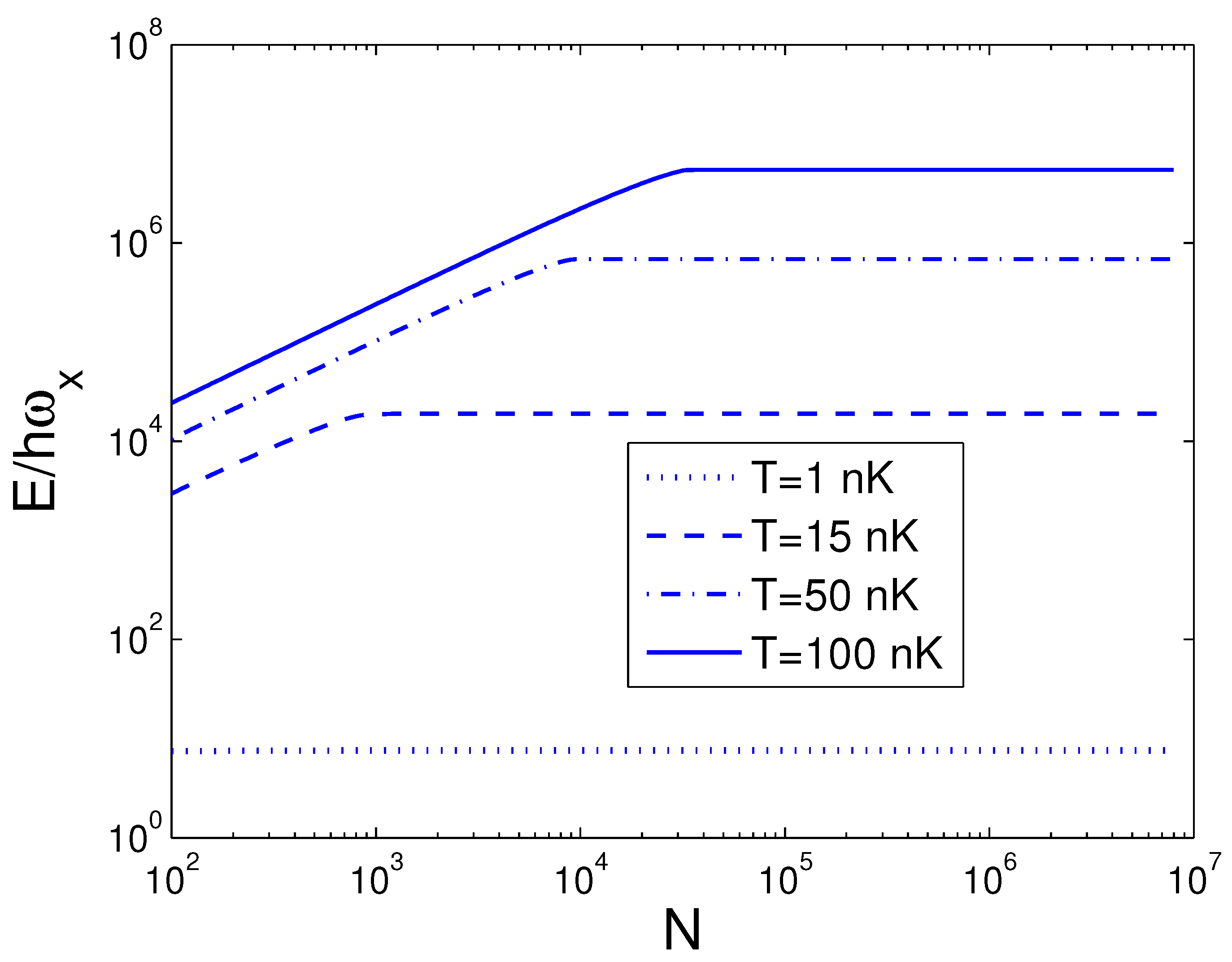
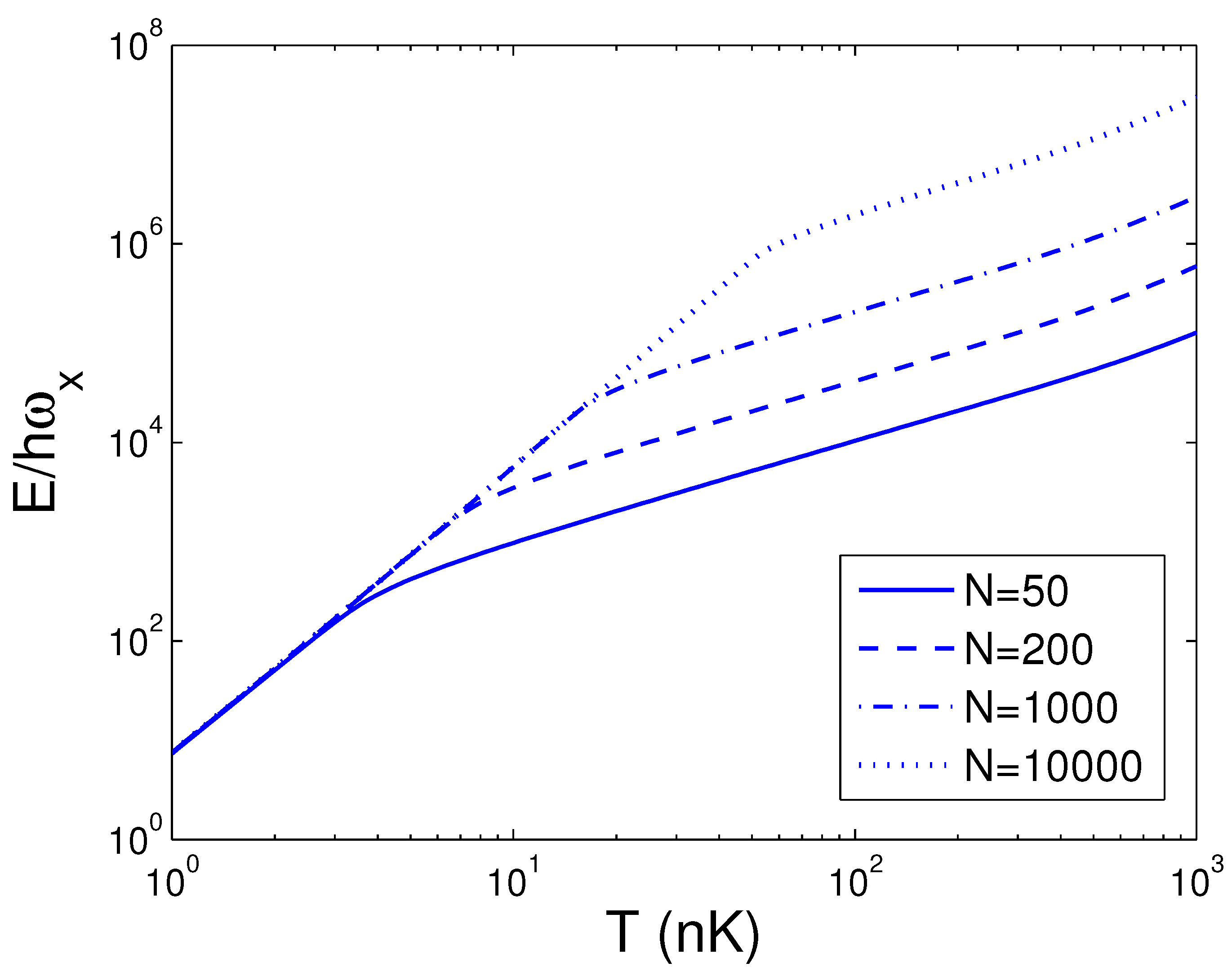
3.2. Internal Energy
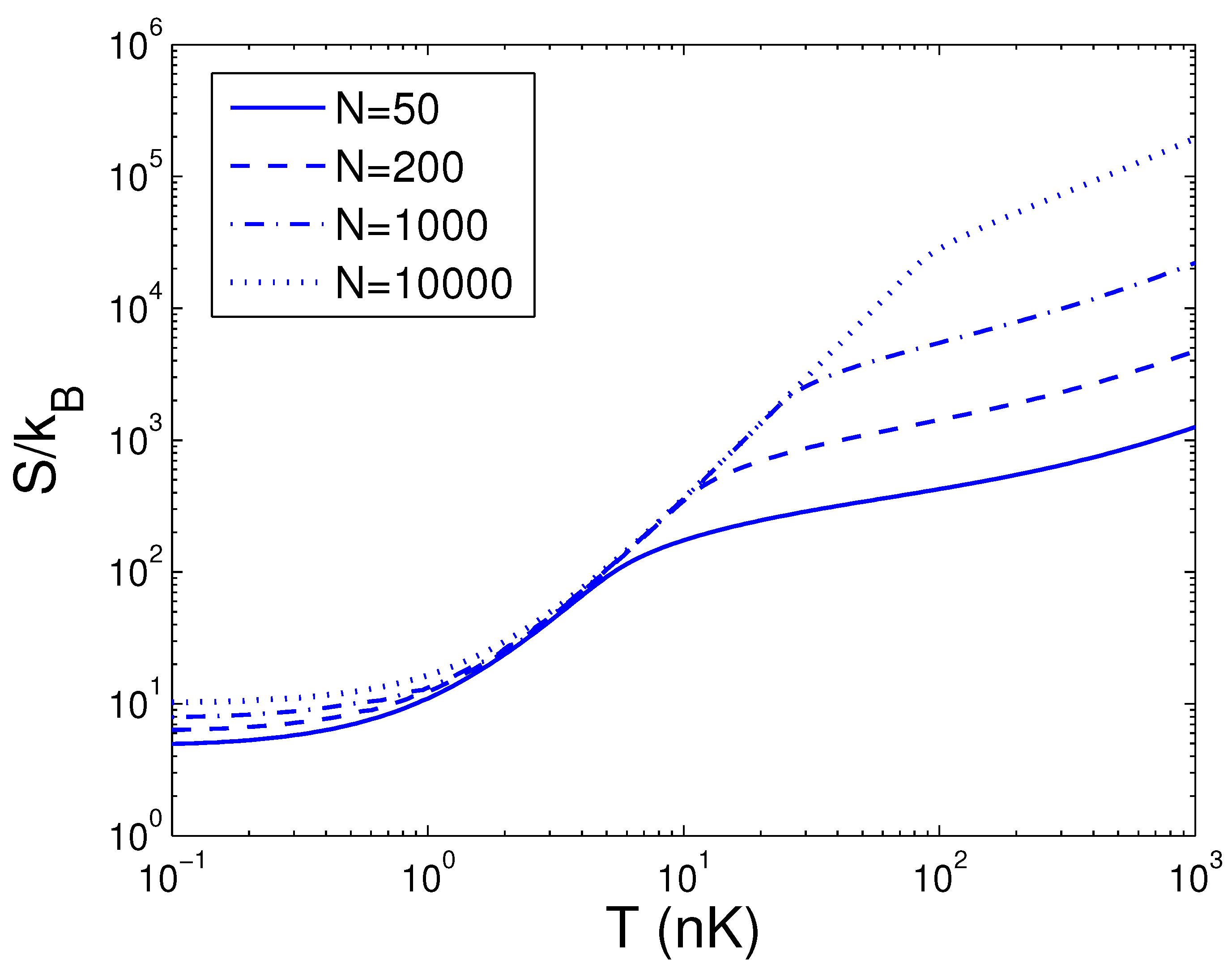
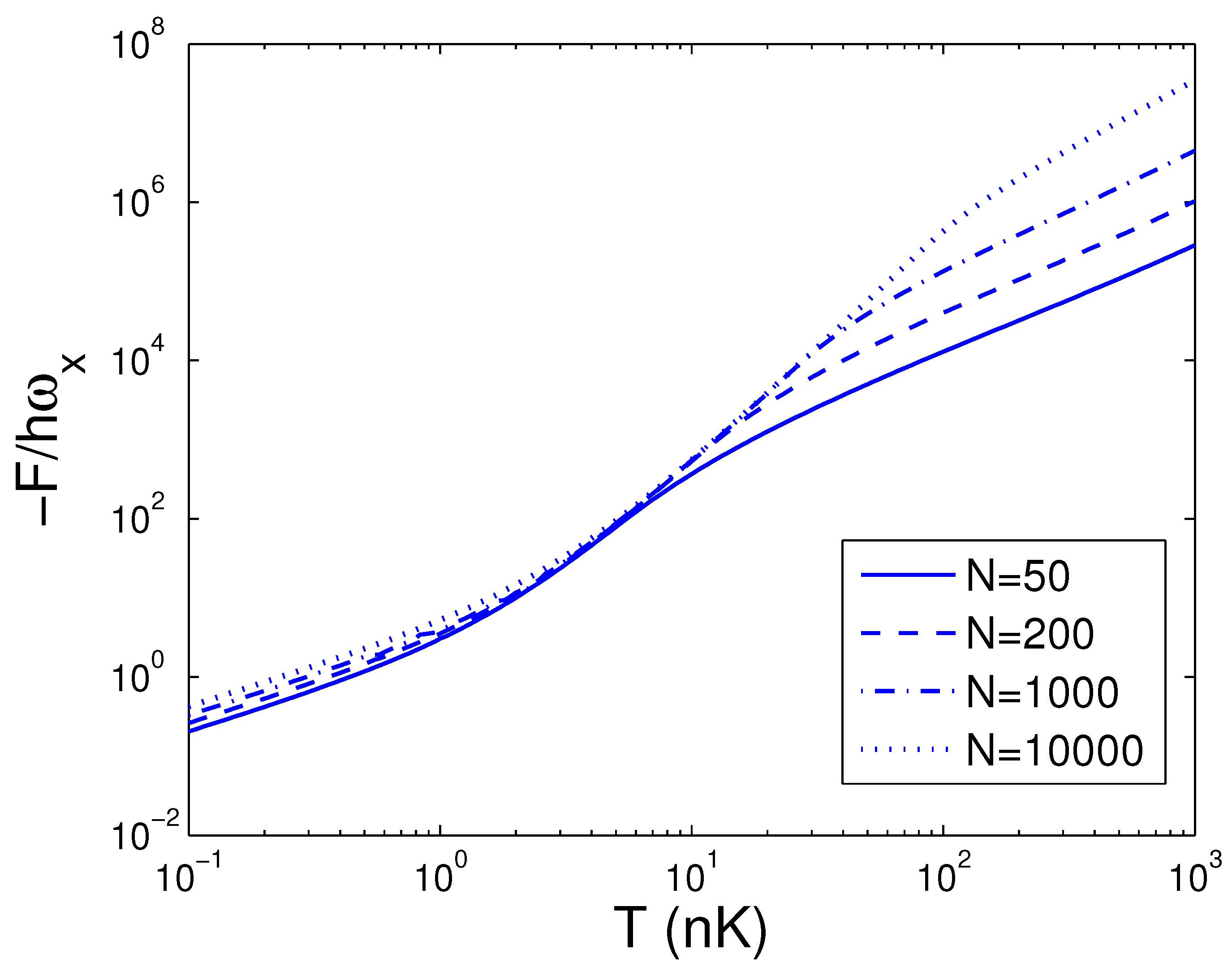
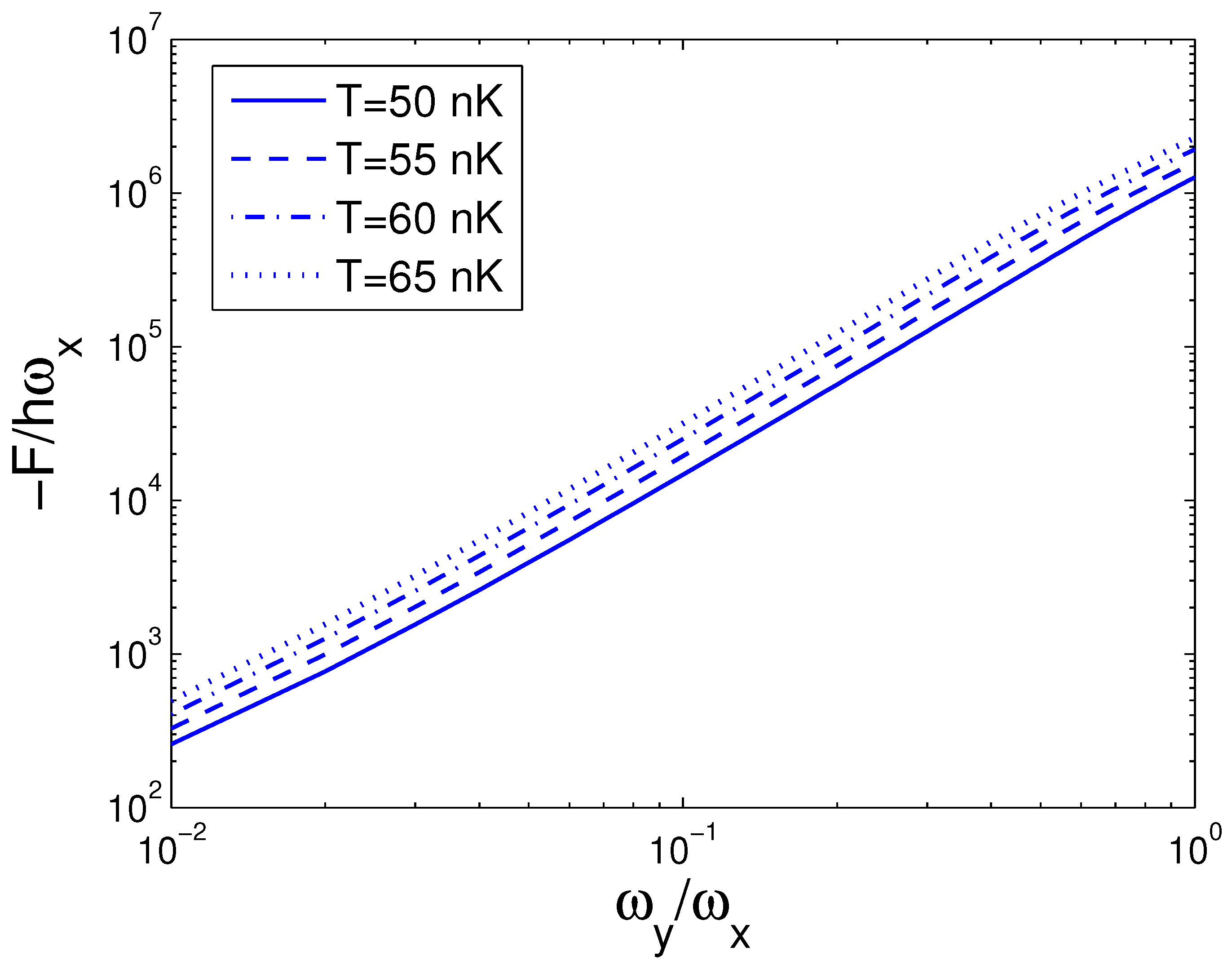
3.3. Entropy and Helmholtz Free Energy
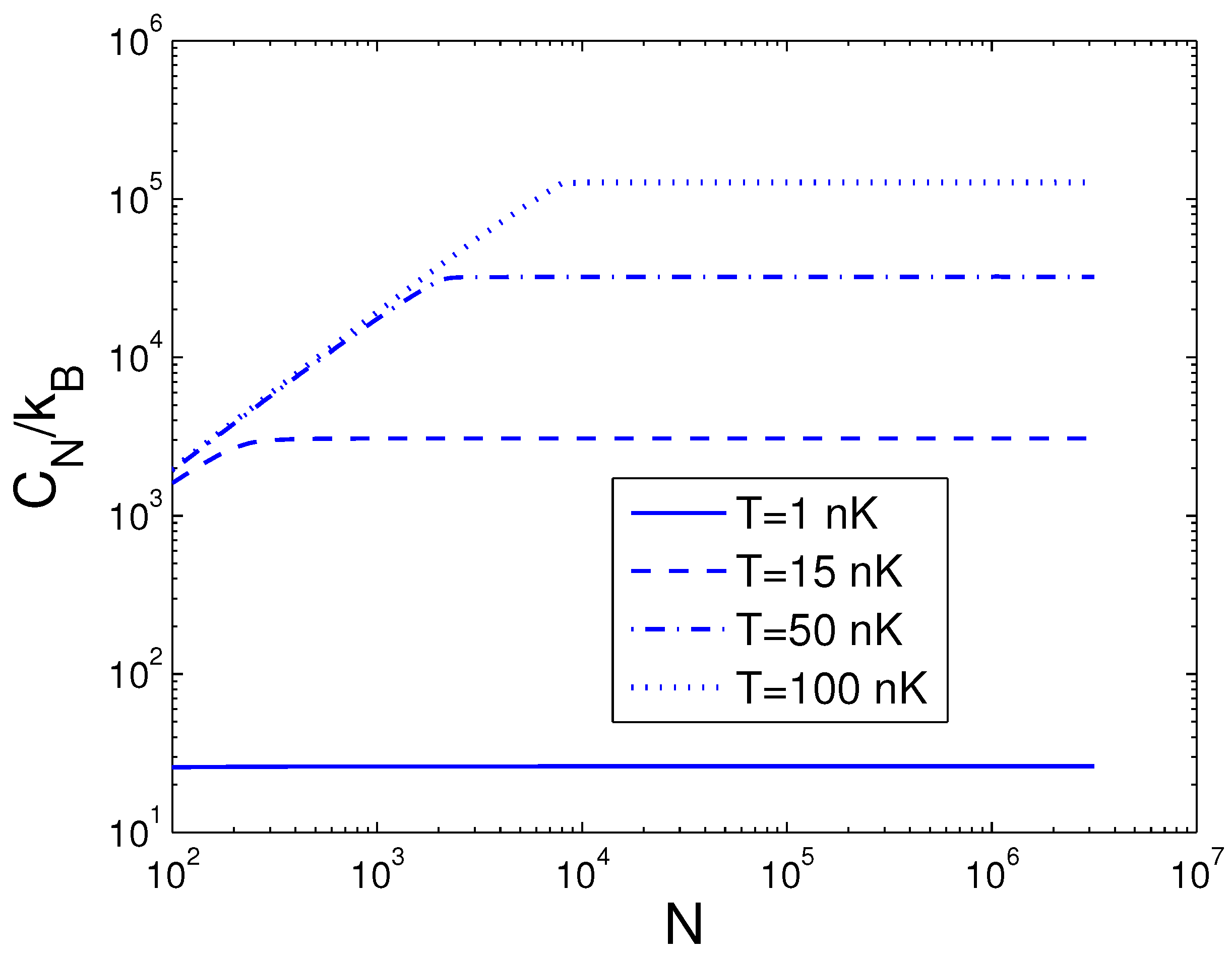
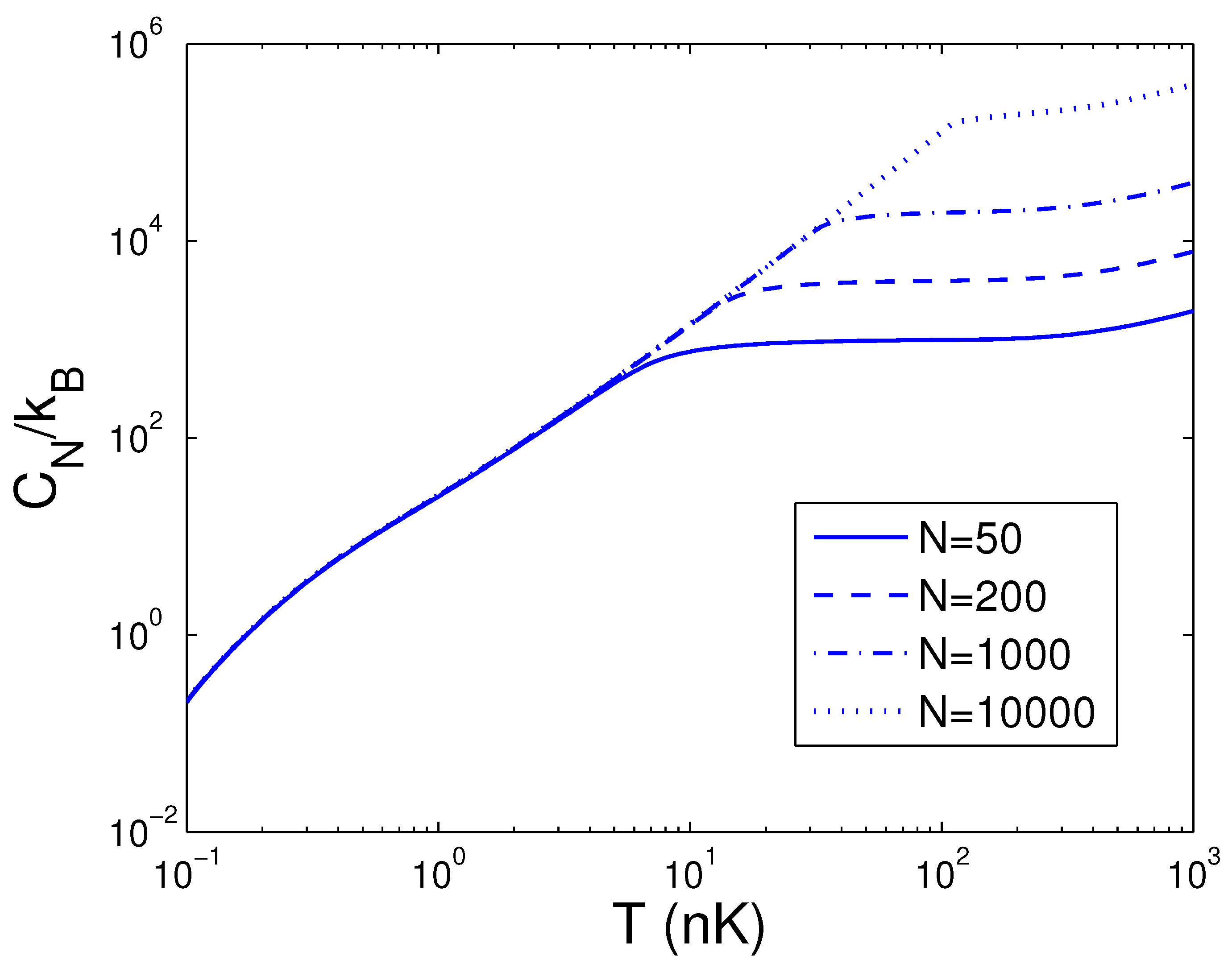
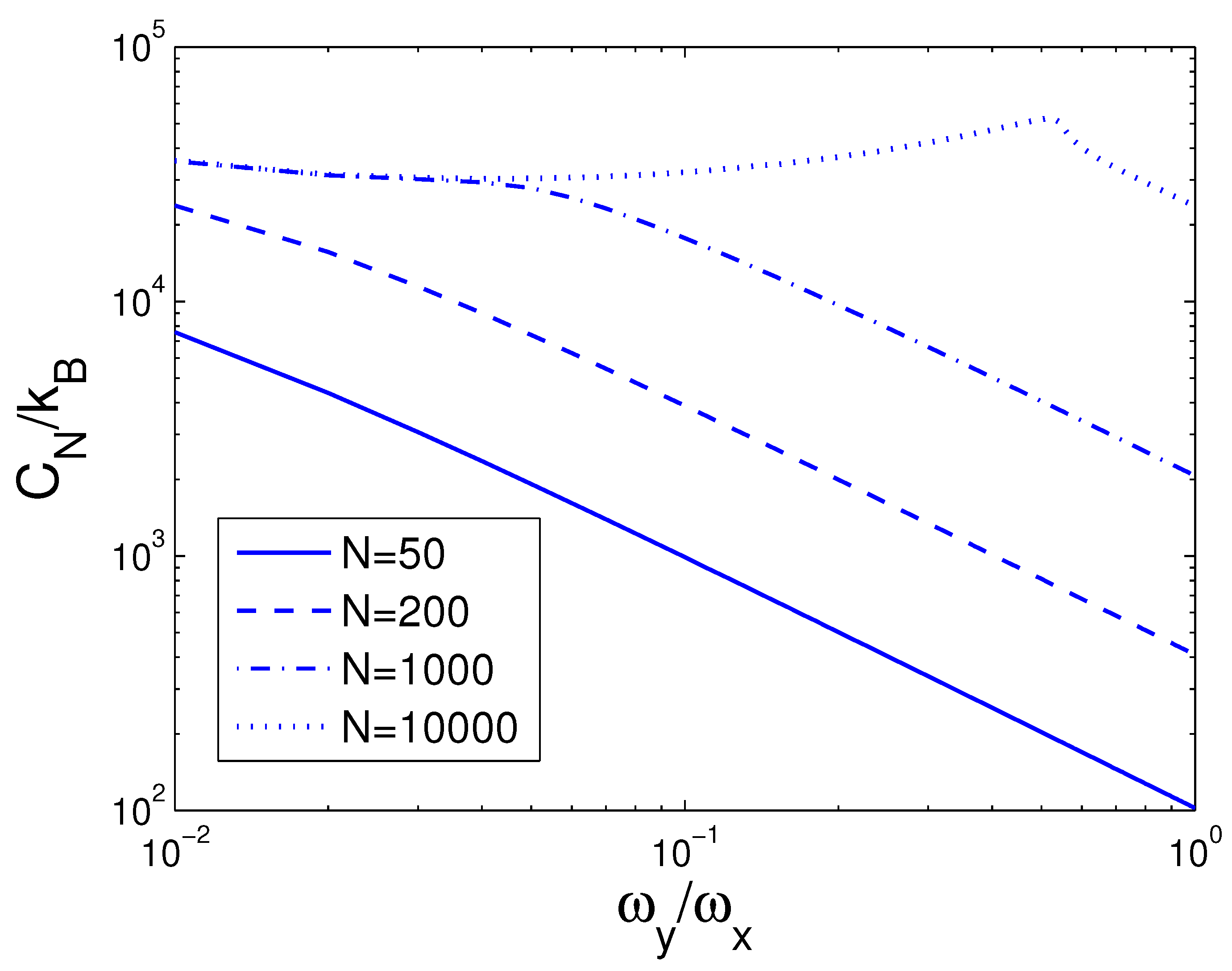
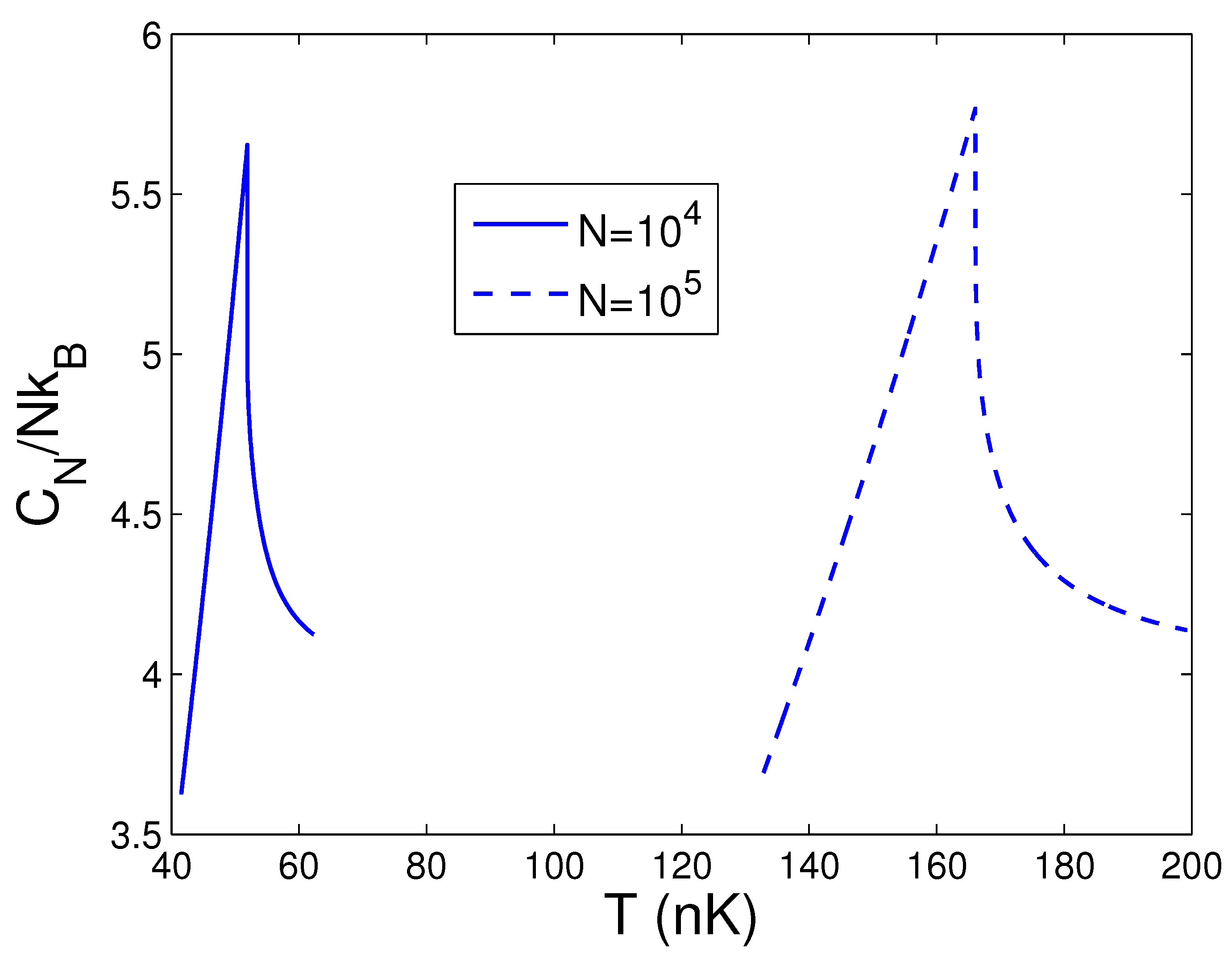
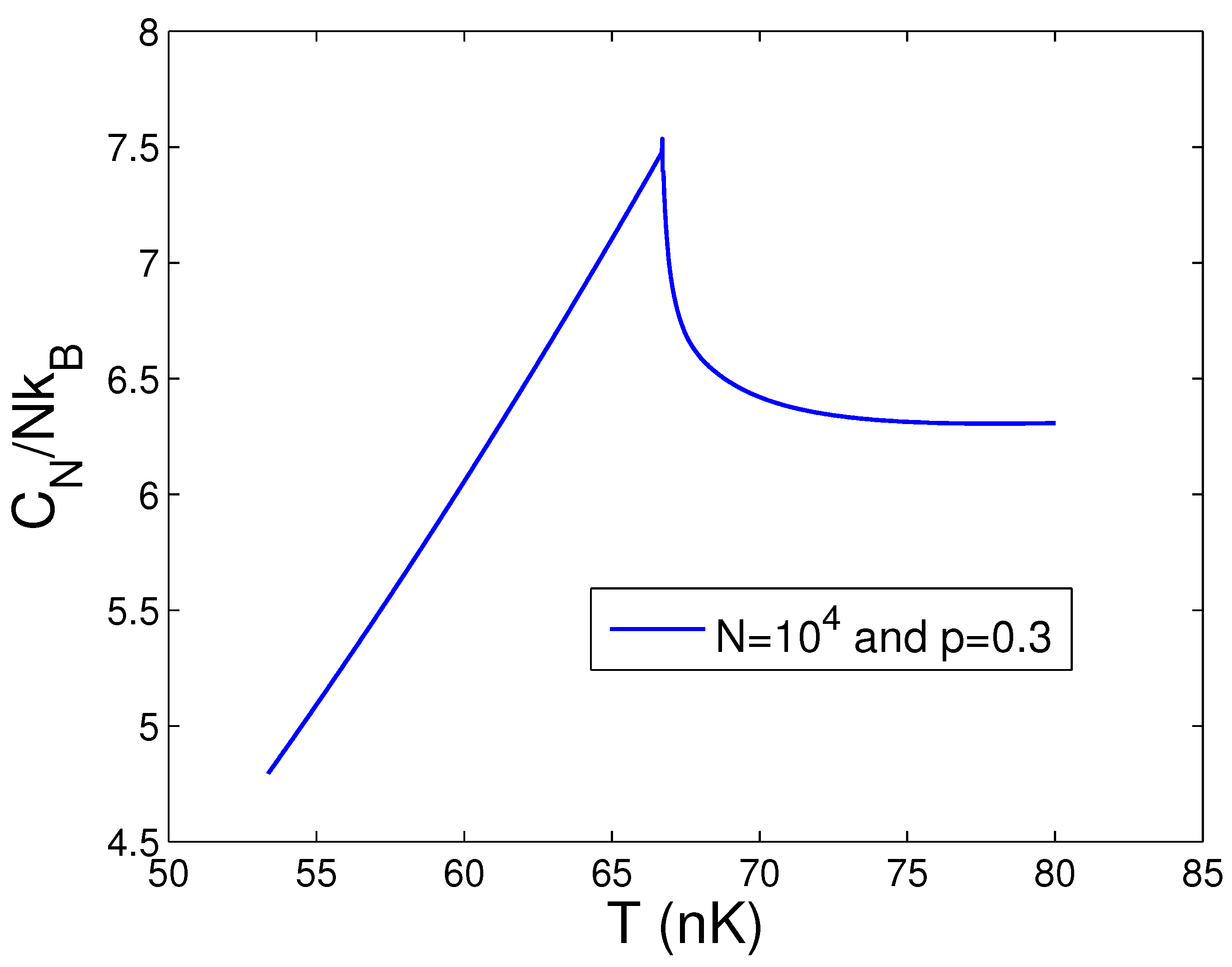
3.4. Heat Capacity at Constant Number
4. Phase Transitions of Ideal Bosons in the Thermodynamic Limit
4.1. Critical Temperature and Reduced Chemical Potential
4.2. Internal Energy, Entropy, and Helmholtz Free Energy
4.3. Heat Capacity at Constant Number
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Anderson, M.H.; Ensher, J.R.; Matthews, M.R.; Wieman, C.E.; Cornell, E.A. Observation of bose-einstein condensation in a dilute atomic vapor. Science 1995, 269, 198. [Google Scholar] [CrossRef]
- Davis, K.B.; Mewes, M.O.; Andrews, M.R.; van Druten, N.J.; Durfee, D.S.; Kurn, D.M.; Ketterle, W. Bose-Einstein condensation in a gas of sodium atoms. Phys. Rev. Lett. 1995, 75, 3969. [Google Scholar] [CrossRef]
- Bradley, C.C.; Sackett, C.A.; Tollett, J.J.; Hulet, R.G. Evidence of Bose-Einstein Condensation in an Atomic Gas with Attractive Interactions. Phys. Rev. Lett. 1995, 75, 1687. [Google Scholar] [CrossRef] [PubMed]
- Butov, L.V.; Lai, C.W.; Ivanov, A.L.; Gossard, A.C.; Chemla, D.S. Towards Bose-Einstein condensation of excitons in potential traps. Nature 2002, 417, 47. [Google Scholar] [CrossRef] [PubMed]
- Eisenstein, J.P.; Macdonald, A.H. Bose-Einstein condensation of excitons in bilayer electron systems. Nature 2004, 432, 691. [Google Scholar] [CrossRef] [PubMed]
- Kasprzak, J.; Richard, M.; Kundermann, S.; Baas, A.; Jeambrun, P.; Keeling, J.M.J.; Marchetti, F.M.; Szymańska, M.H.; André, R.; Staehli, J.; et al. Bose-Einstein condensation of exciton polaritons. Nature 2006, 443, 409. [Google Scholar] [CrossRef]
- Balili, R.; Hartwell, V.; Snoke, D.; Pfeiffer, L.; West, K. Bose-Einstein condensation of microcavity polaritons in a trap. Science 2007, 316, 1007. [Google Scholar] [CrossRef]
- Nikuni, T.; Oshikawa, M.; Oosawa, A.; Tanaka, H. Bose-Einstein Condensation of Dilute Magnons in TlCuCl3. Phys. Rev. Lett. 2000, 84, 5868. [Google Scholar] [CrossRef]
- Demokritov, S.O.; Demidov, V.E.; Dzyapko, O.; Melkov, G.A.; Serga, A.A.; Hillebrands, B.; Slavin, A.N. Bose-Einstein condensation of quasi-equilibrium magnons at room temperature under pumping. Nature 2006, 443, 430. [Google Scholar] [CrossRef]
- Cheng, Z. Bose-Einstein condensation of ideal photons in a one-dimensional barrel cavity. Phys. Rev. A 2016, 93, 023829. [Google Scholar] [CrossRef]
- Klaers, J.; Vewinger, F.; Weitz, M. Thermalization of a two-dimensional photonic gas in a `white wall’photon box. Nat. Phys. 2010, 6, 512. [Google Scholar] [CrossRef]
- Klaers, J.; Schmitt, J.; Vewinger, F.; Weitz, M. Bose-Einstein condensation of photons in an optical microcavity. Nature 2010, 468, 545. [Google Scholar] [CrossRef] [PubMed]
- Klaers, J.; Schmitt, J.; Damm, T.; Vewinger, F.; Weitz, M. Statistical physics of Bose-Einstein-condensed light in a dye microcavity. Phys. Rev. Lett. 2012, 108, 160403. [Google Scholar] [CrossRef] [PubMed]
- Cheng, Z. Exact thermodynamic theory of ideal Bose atoms in a two-dimensional isotropic harmonic trap. Stat. Mech. Theory Exp. 2017, 2017, 113103. [Google Scholar] [CrossRef]
- Cheng, Z.; Man, J.H. Accurate analytic solution for ideal boson gases in a highly anisotropic two-dimensional harmonic trap. Can. J. Phys. 2020, 98, 183. [Google Scholar] [CrossRef]
- Pakuliak, S.; von Gehlen, G. (Eds.) Integrable Structures of Exactly Solvable Two-Dimensional Models of Quantum Field Theory; Springer: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Salasnich, L. Ideal quantum gases in D-dimensional space and power-law potentials. J. Math. Phys. 2000, 41, 8016. [Google Scholar] [CrossRef]
- Öztürk, F.E.; Vewinger, F.; Weitz, M.; Schmitt, J. Fluctuation-dissipation relation for a Bose-Einstein condensate of photons. Phys. Rev. Lett. 2023, 130, 033602. [Google Scholar] [CrossRef]
- Stein, E.; Pelster, A. Thermodynamics of trapped photon gases at dimensional crossover from 2D to 1D. New J. Phys. 2022, 24, 023013. [Google Scholar] [CrossRef]
- Hadzibabic, Z.; Krüger, P.; Cheneau, M.; Battelier, B.; Dalibard, J. Berezinskii–Kosterlitz–Thouless crossover in a trapped atomic gas. Nature 2006, 441, 1118. [Google Scholar] [CrossRef]
- Fletcher, R.J.; Robert-de-Saint-Vincent, M.; Man, J.; Navon, N.; Smith, R.P.; Viebahn, K.G.H.; Hadzibabic, Z. Connecting berezinskii-kosterlitz-thouless and bec phase transitions by tuning interactions in a trapped gas. Phys. Rev. Lett. 2015, 114, 255302. [Google Scholar] [CrossRef]
- Roati, G.; D’Errico, C.; Fallani, L.; Fattori, M.; Fort, C.; Zaccanti, M.; Modugno, G.; Modugno, M.; Inguscio, M. Anderson localization of a non-interacting Bose–Einstein condensate. Nature 2008, 453, 895. [Google Scholar] [CrossRef] [PubMed]
- Mullin, W.J. A Study of Bose-Einstein Condensation in a Two-Dimensional Trapped Gas. J. Low Temp. Phys. 1997, 106, 615. [Google Scholar] [CrossRef]
- Dalfovo, F.; Giorgini, S.; Pitaevskii, L.P.; Stringari, S. Theory of Bose-Einstein condensation in trapped gases. Rev. Mod. Phys. 1999, 71, 463. [Google Scholar] [CrossRef]
- Jackson, F.H. A generalisation of the functions Γ (n) and xn. Proc. Roy. Soc. Lond. A 1904, 74, 64. [Google Scholar]
- Krattenthaler, C.; Srivastava, H.M. Summations for basic hypergeometric series involving a q-analogue of the digamma function. Comput. Math. Appl. 1996, 32, 73. [Google Scholar] [CrossRef]
- Salem, A. Completely monotonic functions related to the q-gamma and the q-trigamma functions. Anal. Appl. 2015, 13, 125. [Google Scholar] [CrossRef]
- Hung, C.L.; Zhang, X.; Gemelke, N.; Chin, C. Observation of scale invariance and universality in two-dimensional Bose gases. Nature 2011, 470, 236. [Google Scholar] [CrossRef]
- Damm, T.; Schmitt, J.; Liang, Q.; Dung, D.; Vewinger, F.; Weitz, M.; Klaers, J. Calorimetry of a Bose–Einstein-condensed photon gas. Nat. Commun. 2016, 7, 11340. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, Z. Accurate Thermodynamic Properties of Ideal Bosons in a Highly Anisotropic 2D Harmonic Potential. Entropy 2023, 25, 1513. https://doi.org/10.3390/e25111513
Cheng Z. Accurate Thermodynamic Properties of Ideal Bosons in a Highly Anisotropic 2D Harmonic Potential. Entropy. 2023; 25(11):1513. https://doi.org/10.3390/e25111513
Chicago/Turabian StyleCheng, Ze. 2023. "Accurate Thermodynamic Properties of Ideal Bosons in a Highly Anisotropic 2D Harmonic Potential" Entropy 25, no. 11: 1513. https://doi.org/10.3390/e25111513
APA StyleCheng, Z. (2023). Accurate Thermodynamic Properties of Ideal Bosons in a Highly Anisotropic 2D Harmonic Potential. Entropy, 25(11), 1513. https://doi.org/10.3390/e25111513




