The Quantum Geometric Tensor in a Parameter-Dependent Curved Space
Abstract
:1. Introduction
2. Quantum Metric Tensor: Geometrical Approach
3. Berry Curvature and Quantum Geometric Tensor
4. Examples of the Quantum Metric Tensor in Curved Space
4.1. Anharmonic Oscillator in One Dimensional Curved Space
4.2. Harmonic Oscillator with a Morse Type Potential
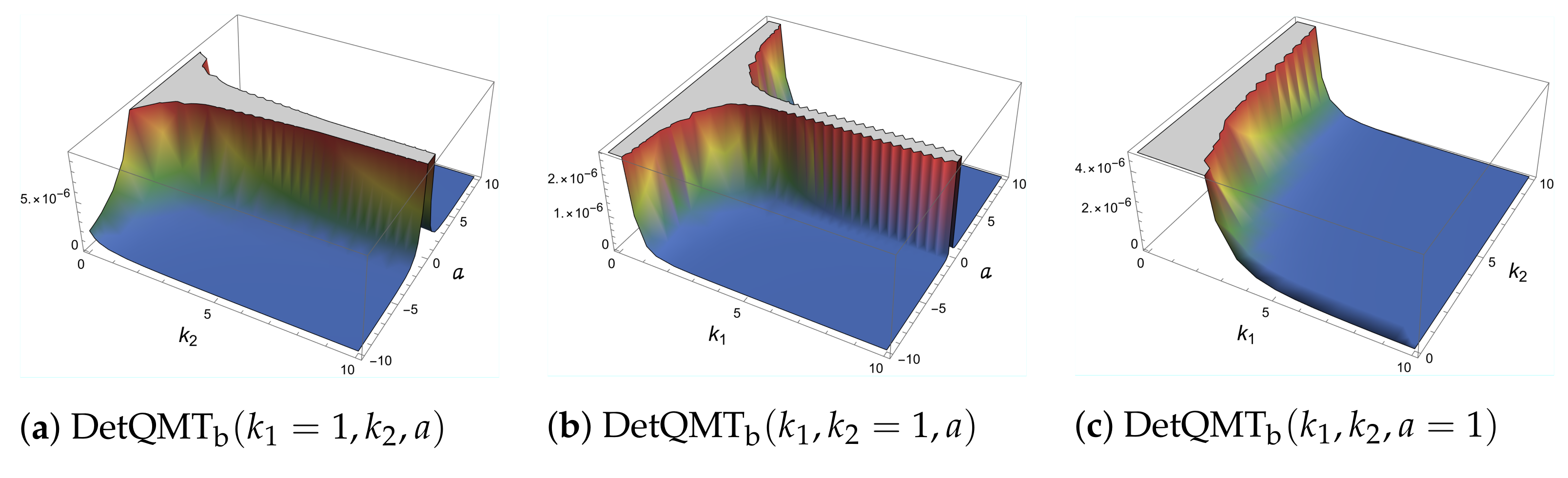
5. QGT Coupled Anharmonic Oscillator
6. Generalized Anharmonic Oscillator in Curved Space
7. Discussion
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| MDPI | Multidisciplinary Digital Publishing Institute |
| QGT | Quantum Geometric Tensor |
| QPT | Quantum Phase Transitions |
| QMT | Quantum Metric Tensor |
Appendix A
References
- Provost, J.P.; Vallee, G. Riemannian structure on manifolds of quantum states. Commun. Math. Phys. 1980, 76, 289–301. [Google Scholar] [CrossRef]
- Sondhi, S.L.; Girvin, S.M.; Carini, J.P.; Shahar, D. Continuous quantum phase transitions. Rev. Mod. Phys. 1997, 69, 315–333. [Google Scholar] [CrossRef]
- Sachdev, S. Quantum Phase Transitions, 2nd ed.; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar] [CrossRef]
- Zanardi, P.; Giorda, P.; Cozzini, M. Information-Theoretic Differential Geometry of Quantum Phase Transitions. Phys. Rev. Lett. 2007, 99, 100603. [Google Scholar] [CrossRef] [PubMed]
- Gu, S.J. Fidelity Approach to Quantum Phase Transitions. Int. J. Mod. Phys. B 2010, 24, 4371–4458. [Google Scholar] [CrossRef]
- Dutta, A.; Aeppli, G.; Chakrabarti, B.K.; Divakaran, U.; Rosenbaum, T.F.; Sen, D. Quantum Phase Transitions in Transverse Field Spin Models: From Statistical Physics to Quantum Information; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar] [CrossRef]
- Carollo, A.; Valenti, D.; Spagnolo, B. Geometry of quantum phase transitions. Phys. Rep. 2020, 838, 1–72. [Google Scholar] [CrossRef]
- Berry, M.V. Classical adiabatic angles and quantal adiabatic phase. J. Phys. A Math. Gen. 1985, 18, 15. [Google Scholar] [CrossRef]
- Chruscinski, D.; Jamiolkowski, A. Geometric Phases in Classical and Quantum Mechanics; Springer Science & Business Media: New York, NY, USA, 2012; Volume 36. [Google Scholar]
- Bengtsson, I.; Zyczkowski, K. Geometry of Quantum States: An Introduction to Quantum Entanglement; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar] [CrossRef]
- Carollo, A.C.M.; Pachos, J.K. Geometric Phases and Criticality in Spin-Chain Systems. Phys. Rev. Lett. 2005, 95, 157203. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhu, S.L. Scaling of Geometric Phases Close to the Quantum Phase Transition in the XY Spin Chain. Phys. Rev. Lett. 2006, 96, 077206. [Google Scholar] [CrossRef]
- Fisher, R.A. On the mathematical foundations of theoretical statistics. Philos. Trans. R. Soc. Lond. Ser. A 1922, 222, 309–368. [Google Scholar] [CrossRef]
- Rao, C.R. Information and the Accuracy Attainable in the Estimation of Statistical Parameters. Bull. Calcutta Math. Soc. 1945, 37, 81–91. [Google Scholar]
- Petz, D. Monotone metrics on matrix spaces. Linear Algebra Appl. 1996, 244, 81–96. [Google Scholar] [CrossRef]
- Balian, R. The Entropy-Based Quantum Metric. Entropy 2014, 16, 3878–3888. [Google Scholar] [CrossRef]
- Ciaglia, F.M.; Jost, J.; Schwachhöfer, L. From the Jordan Product to Riemannian Geometries on Classical and Quantum States. Entropy 2020, 22, 637. [Google Scholar] [CrossRef] [PubMed]
- Ciaglia, F.M.; Di Cosmo, F.; Di Nocera, F.; Vitale, P. Monotone metric tensors in Quantum Information Geometry. arXiv 2022, arXiv:2203.10857. [Google Scholar]
- Díaz, B.; González, D.; Gutiérrez-Ruiz, D.; Vergara, J.D. Classical analogs of the covariance matrix, purity, linear entropy, and von Neumann entropy. Phys. Rev. A 2022, 105, 062412. [Google Scholar] [CrossRef]
- Nielsen, M.; Chuang, I. Quantum Computation and Quantum Information: 10th Anniversary Edition; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Campos Venuti, L.; Zanardi, P. Quantum Critical Scaling of the Geometric Tensors. Phys. Rev. Lett. 2007, 99, 095701. [Google Scholar] [CrossRef] [PubMed]
- Castro Neto, A.H.; Guinea, F.; Peres, N.M.R.; Novoselov, K.S.; Geim, A.K. The electronic properties of graphene. Rev. Mod. Phys. 2009, 81, 109–162. [Google Scholar] [CrossRef]
- Siu, Z.B.; Chang, J.; Tan, S.G. Curvature induced quantum phase transitions in an electron-hole system. Sci. Rep. 2018, 8, 16497. [Google Scholar] [CrossRef]
- Jost, J. Riemannian Geometry and Geometric Analysis; Universitext; Springer International Publishing: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Margalli, C.; Vergara, J.D. From complex holomorphic systems to real systems. Int. J. Mod. Phys. A 2020, 35, 2050065. [Google Scholar] [CrossRef]
- Makarov, D.N. Coupled harmonic oscillators and their quantum entanglement. Phys. Rev. E 2018, 97, 042203. [Google Scholar] [CrossRef]
- Alvarez-Jimenez, J.; Gonzalez, D.; Gutiérrez-Ruiz, D.; Vergara, J.D. Geometry of the Parameter Space of a Quantum System: Classical Point of View. Annalen der Physik 2020, 532, 1900215. [Google Scholar] [CrossRef]
- Jefferson, R.A.; Myers, R.C. Circuit complexity in quantum field theory. J. High Energy Phys. 2017, 2017, 107. [Google Scholar] [CrossRef]
- Alvarez-Jimenez, J.; Dector, A.; Vergara, J.D. Quantum information metric and Berry curvature from a Lagrangian approach. J. High Energy Phys. 2017, 2017, 44. [Google Scholar] [CrossRef]
- Eguchi, T.; Gilkey, P.B.; Hanson, A.J. Gravitation, gauge theories and differential geometry. Phys. Rep. 1980, 66, 213–393. [Google Scholar] [CrossRef]
- Guillarmou, C.; Kupiainen, A.; Rhodes, R.; Vargas, V. Conformal bootstrap in Liouville Theory. arXiv 2020, arXiv:2005.11530. [Google Scholar]
- Reuter, M.; Wolter, F.E.; Shenton, M.; Niethammer, M. Laplace–Beltrami eigenvalues and topological features of eigenfunctions for statistical shape analysis. Comput.-Aided Des. 2009, 41, 739–755. [Google Scholar] [CrossRef] [Green Version]
- Susskind, L. Three Lectures on Complexity and Black Holes. arXiv 2018, arXiv:1810.11563. [Google Scholar]
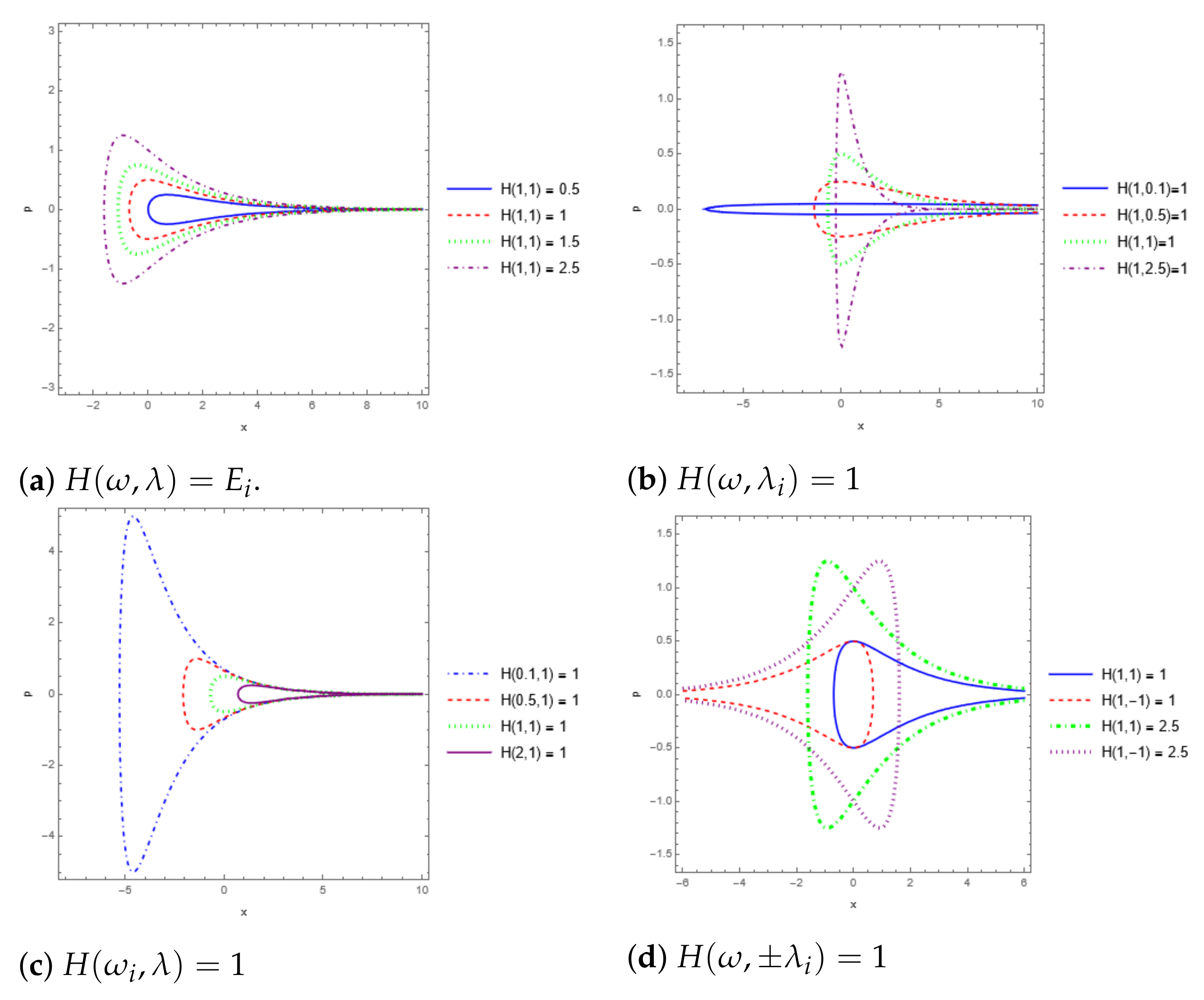
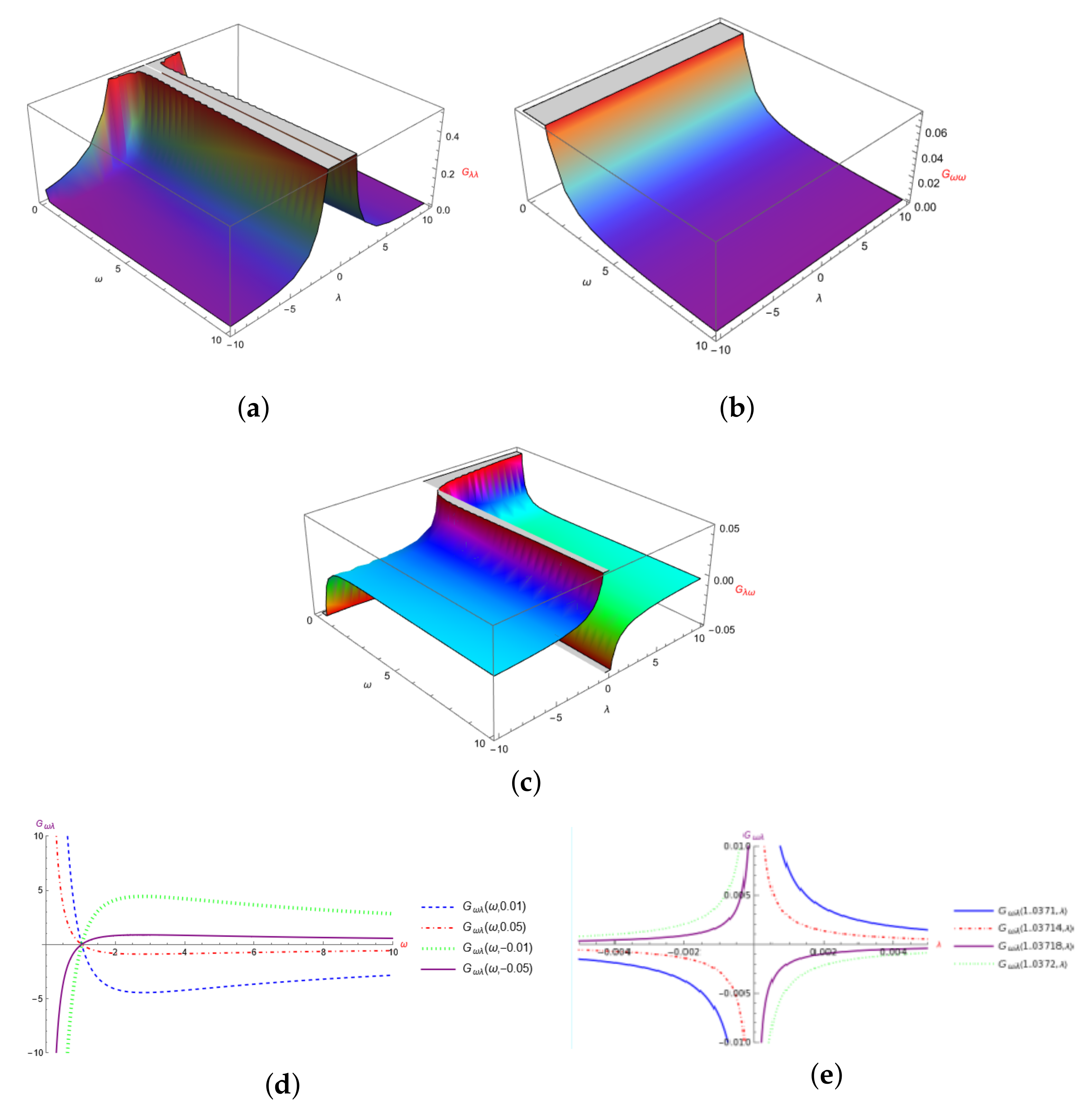

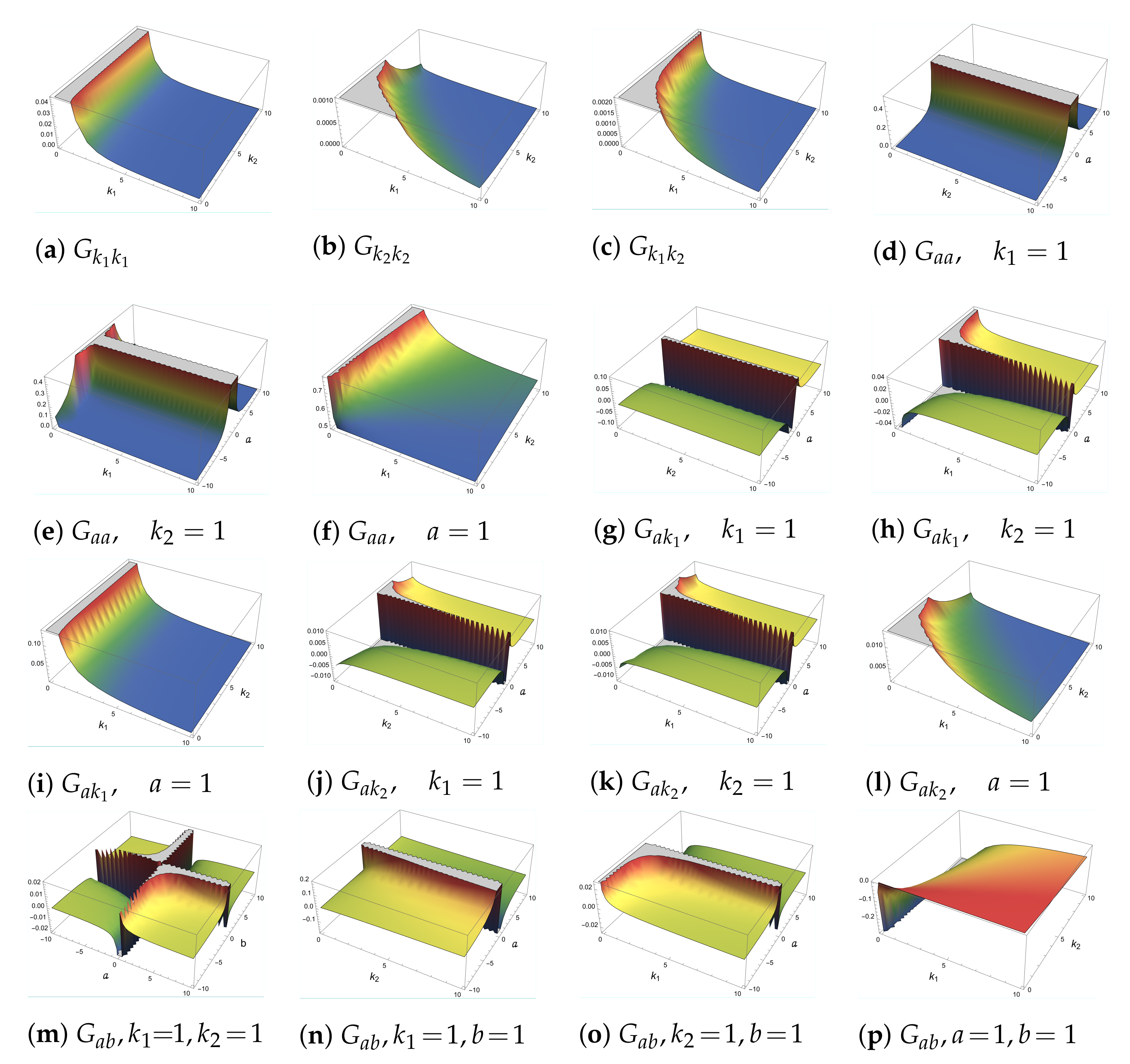
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Austrich-Olivares, J.A.; Vergara, J.D. The Quantum Geometric Tensor in a Parameter-Dependent Curved Space. Entropy 2022, 24, 1236. https://doi.org/10.3390/e24091236
Austrich-Olivares JA, Vergara JD. The Quantum Geometric Tensor in a Parameter-Dependent Curved Space. Entropy. 2022; 24(9):1236. https://doi.org/10.3390/e24091236
Chicago/Turabian StyleAustrich-Olivares, Joan A., and Jose David Vergara. 2022. "The Quantum Geometric Tensor in a Parameter-Dependent Curved Space" Entropy 24, no. 9: 1236. https://doi.org/10.3390/e24091236
APA StyleAustrich-Olivares, J. A., & Vergara, J. D. (2022). The Quantum Geometric Tensor in a Parameter-Dependent Curved Space. Entropy, 24(9), 1236. https://doi.org/10.3390/e24091236





