Quantumness Measures for a System of Two Qubits Interacting with a Field in the Presence of the Time-Dependent Interaction and Kerr Medium
Abstract
1. Introduction
2. Power-Law Potentials
3. System Hamiltonian and Dynamics
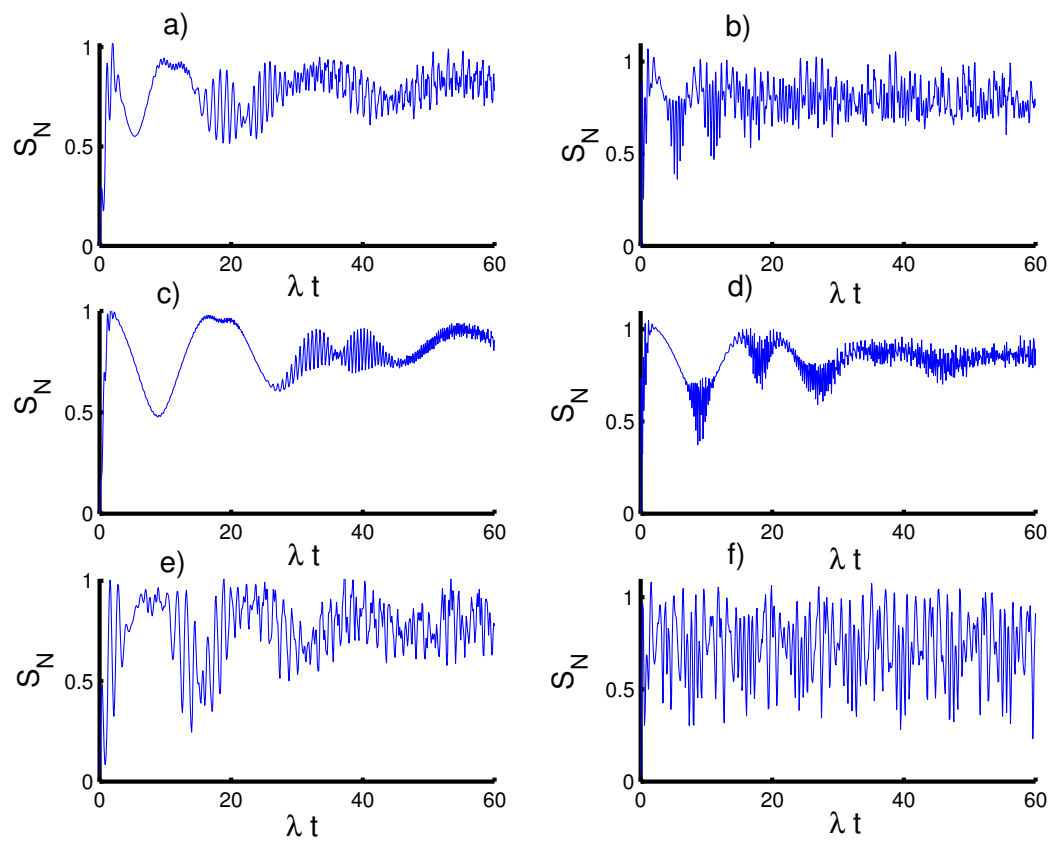
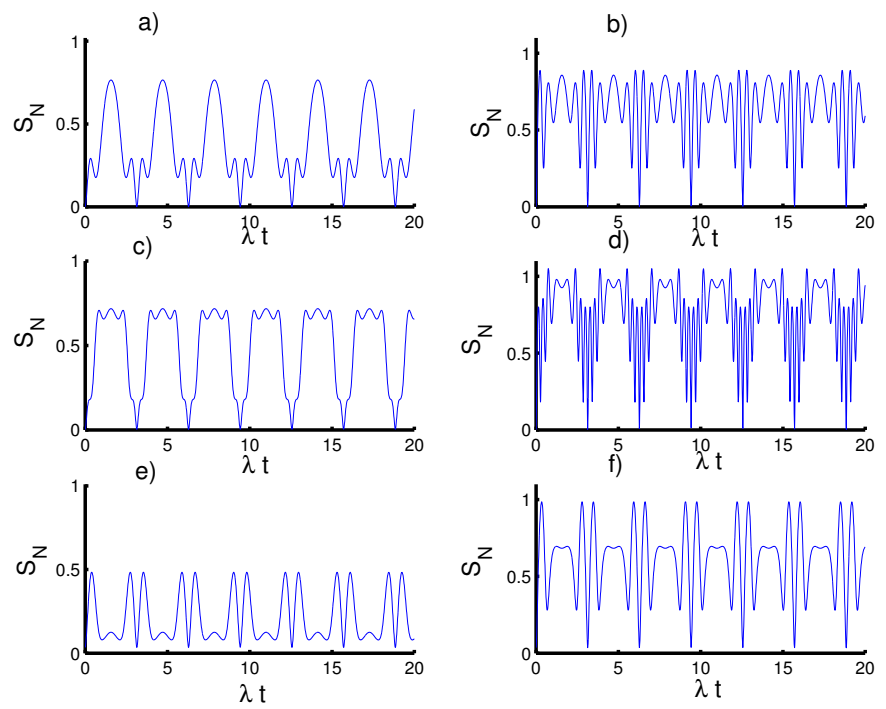
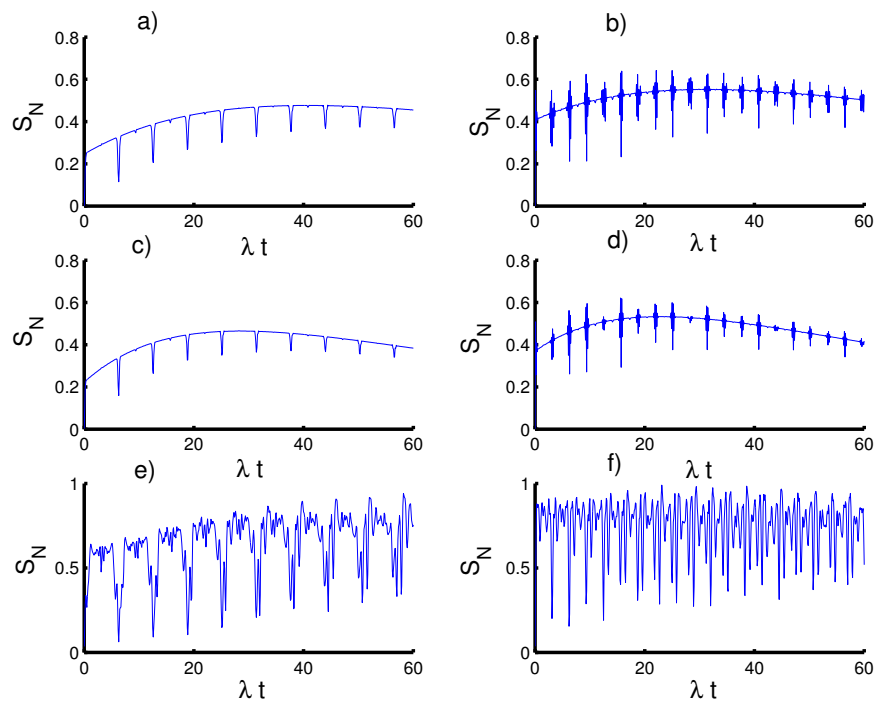
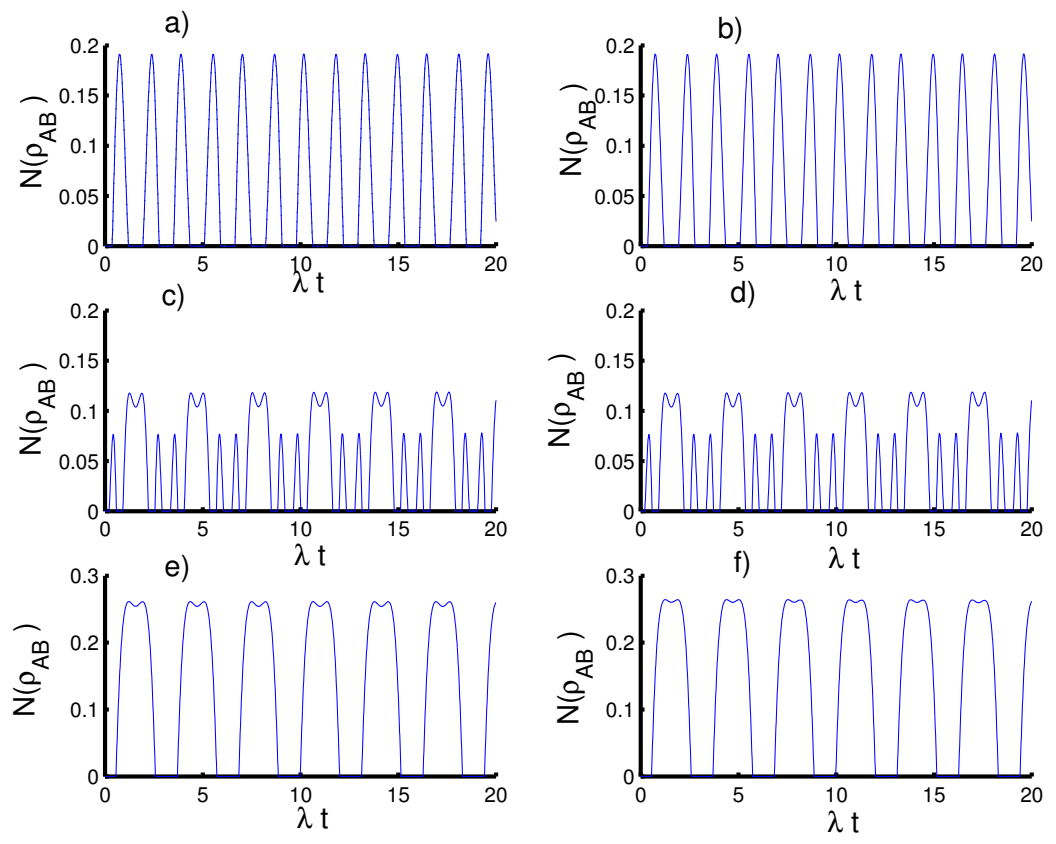
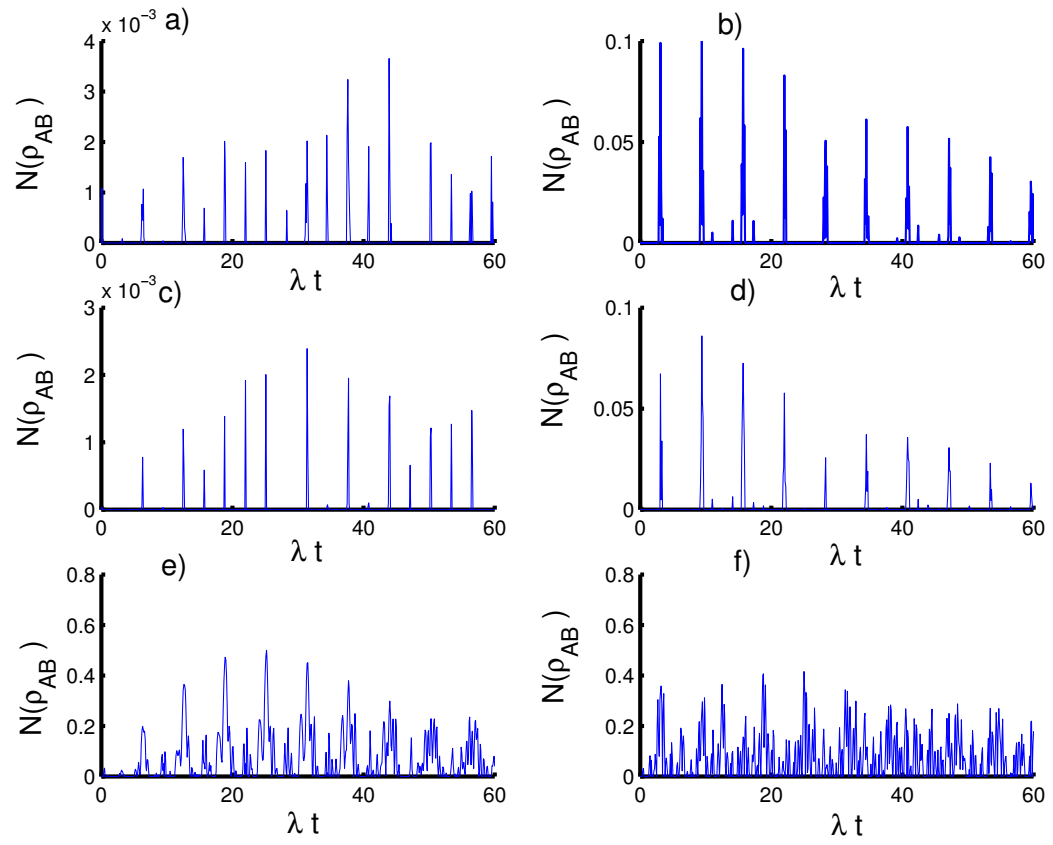
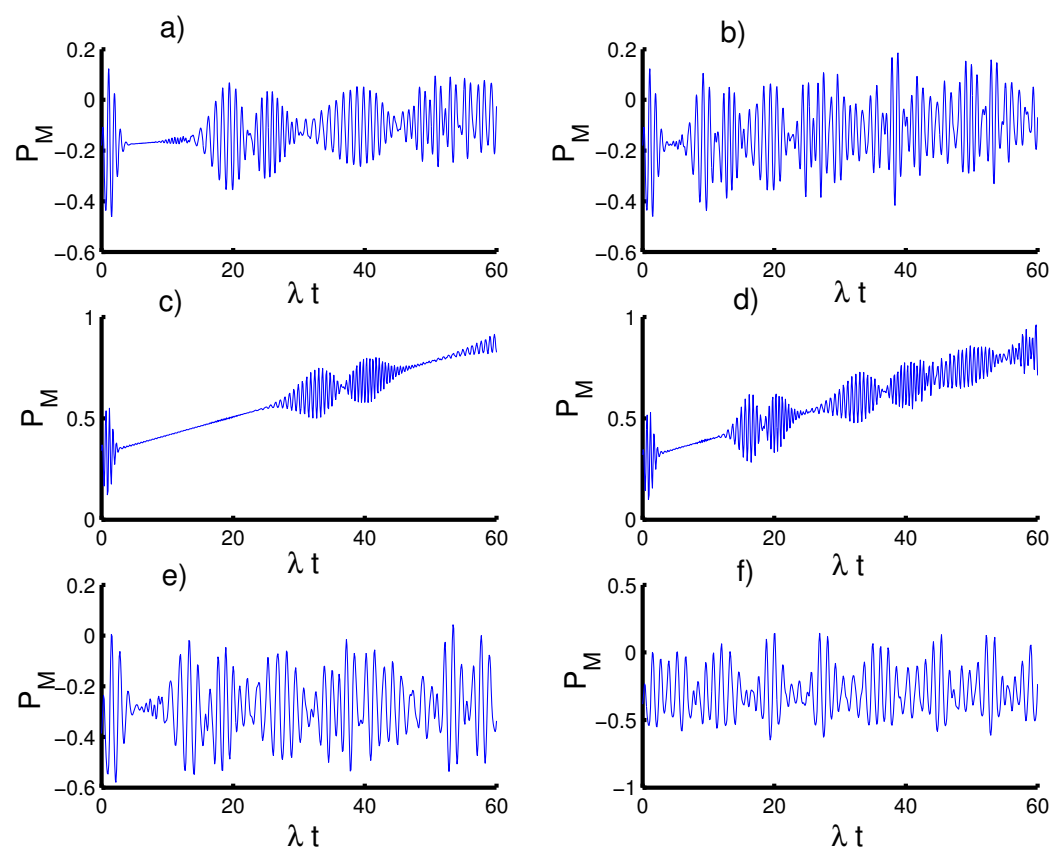
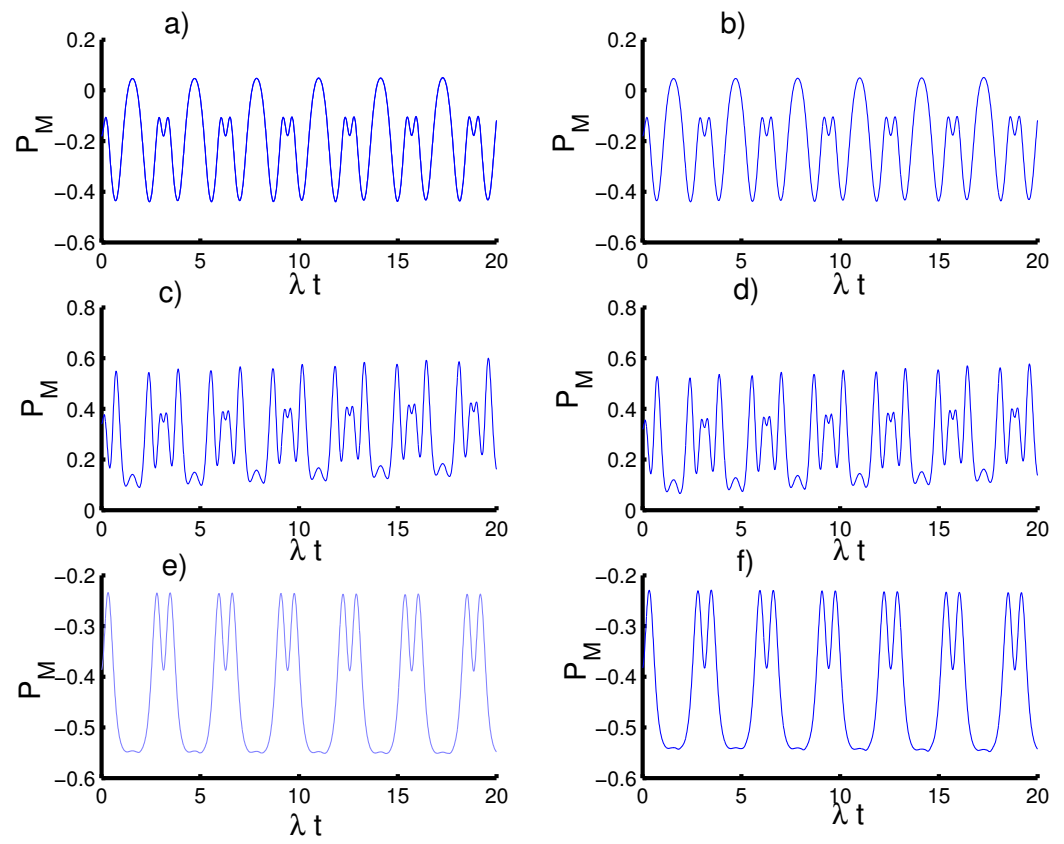
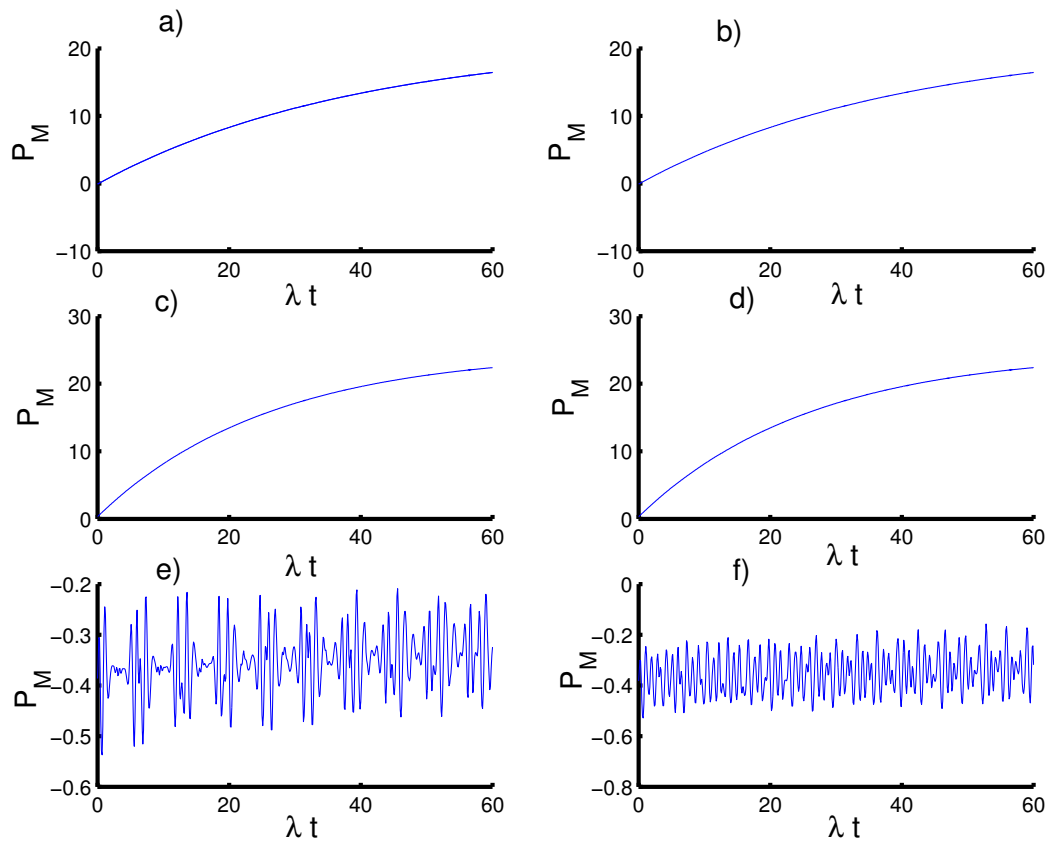
4. Quantum Quantifiers and Main Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Schrödinger, E. The current situation in quantum mechanics. Naturwissenschaften 1935, 23, 807. [Google Scholar] [CrossRef]
- Barenco, A.; Deutsch, D.; Ekert, A.; Jozsa, R. Conditional quantum dynamics and logic gates. Phys. Rev. Lett. 1995, 74, 4083. [Google Scholar] [CrossRef] [PubMed]
- Bennett, C.H.; Brassard, G.; Crepeau, C.; Jozsa, R.; Peres, A.; Wootters, W.K. Teleporting an unknown quantum state via dual classical and Einstein–Podolsky–Rosen channels. Phys. Rev. Lett. 1993, 70, 1895. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Pan, Q.; Jing, J.; Zhang, J.; Xie, C.; Peng, K. Quantum dense coding exploiting a bright Einstein–Podolsky–Rosen beam. Phys. Rev. Lett. 2002, 88, 047904. [Google Scholar] [CrossRef] [PubMed]
- Bennett, C.H. Quantum cryptography using any two non-orthogonal states. Phys. Rev. Lett. 1992, 68, 3121. [Google Scholar] [CrossRef] [PubMed]
- Fakhri, H.; Dehghani, A. Coherency of su(1,1)-Barut-Girardello type and entanglement for spherical harmonics. J. Math. Phys. 2009, 50, 052104. [Google Scholar] [CrossRef]
- Dey, S.; Hussin, V. Entangled squeezed states in noncommutative spaces with minimal length uncertainty relations. Phys. Rev. D 2015, 91, 124017. [Google Scholar] [CrossRef]
- Dey, S.; Fring, A.; Hussin, V. Nonclassicality versus entanglement in a noncommutative space. Int. J. Mod. Phys. B 2017, 31, 1650248. [Google Scholar] [CrossRef]
- Wang, X.G. Two-mode nonlinear coherent states. Opt. Commun. 2000, 178, 365. [Google Scholar] [CrossRef][Green Version]
- Karimi, A. Two-mode photon-added entangled coherent-squeezed states: Their entanglement and nonclassical properties. Appl. Phys. B 2017, 123, 181. [Google Scholar] [CrossRef]
- Hyunseok, J.; Nguyen, B.A. GHZ-type and W-type entangled coherent states: Generation and Bell-type inequality tests without photon counting. Phys. Rev. A 2006, 74, 022104. [Google Scholar]
- Pan, J.W.; Chen, Z.-B.; Lu, C.Y.; Weinfurter, H.; Zeilinger, A.; Zukowski, M. Multiphoton entanglement and interferometry. Rev. Mod. Phys. 2012, 84, 777. [Google Scholar] [CrossRef]
- Simon, D.S.; Sergienko, A.V. High-capacity quantum key distribution via hyperentangled degrees of freedom. New J. Phys. 2014, 16, 063052. [Google Scholar] [CrossRef]
- Bouwmeester, D.; Ekert, A.K.; Zeilinger, A. The Physics of Quantum Information: Quantum Crypthography, Quantum Teleportation, Quantum Computation; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Scully, M.O.; Rathe, U.W.; Su, C.; Agarwal, G.S. On enhancing spectral resolution via correlated spontaneous emission. Opt. Comm. 1997, 136, 39. [Google Scholar] [CrossRef]
- Gatti, A.; Brambilla, E.; Bache, M.; Lugiato, L.A. Correlated imaging, quantum and classical. Phys. Rev. A 2004, 70, 013802. [Google Scholar] [CrossRef]
- Pleinert, M.-O.; von Zanthier, J.; Agarwal, G.S. Phase control of the quantum statistics of collective emission. Phys. Rev. A 2018, 97, 023831. [Google Scholar] [CrossRef]
- Berrada, K.; Eleuch, H. Noncommutative deformed cat states under decoherence. Phys. Rev. D 2019, 100, 016020. [Google Scholar] [CrossRef]
- Raymond Ooi, C.H. Quenching the collective effects on the two-photon correlation from two double-Raman atoms. Phys. Rev. A 2007, 75, 043817. [Google Scholar] [CrossRef]
- Raymond Ooi, C.H.; Sun, Q.; Zubairy, M.S.; Scully, M.O. Correlation of photon pairs from the double Raman amplifier: Generalized analytical quantum Langevin theory. Phys. Rev. A 2007, 75, 013820. [Google Scholar] [CrossRef]
- Klauder, J.R.; Skagerstam, B.-S. Coherent States-Applications in Physics and Mathematical Physics; World Scientific: Singapore, 1985. [Google Scholar]
- Zhang, W.-M.; Feng, D.H.; Gilmore, R. Coherent states: Theory and some applications. Rev. Mod. Phys. 1990, 62, 867. [Google Scholar] [CrossRef]
- Galuber, R.J. The Quantum Theory of Optical Coherence. Phys. Rev. 1963, 130, 2529. [Google Scholar] [CrossRef]
- Walls, D.F. Squeezed states of light. Nature 1983, 306, 141. [Google Scholar] [CrossRef]
- Loudon, R.; Knight, P.L. Squeezed Light. J. Mod. Opt. 1987, 34, 709. [Google Scholar] [CrossRef]
- Berrada, K.; El Baz, M.; Hassouni, Y. Generalized Heisenberg algebra coherent states for power-law potentials. Phys. Lett. A 2011, 375, 298. [Google Scholar] [CrossRef][Green Version]
- Abdel-Khalek, S.; Berrada, K.; Alkhateeb, S.A. Measures of nonclassicality for a two-level atom interacting with power-law potential field under decoherence effect. Laser Phys. 2016, 26, 095201. [Google Scholar] [CrossRef]
- Berrada, K. Improving quantum phase estimation via power-law potential systems. Laser Phys. 2014, 24, 065201. [Google Scholar] [CrossRef]
- Sukhatma, U.P. WKB Energy Levels for a Class of One-Dimensional Potentials. Am. J. Phys. 1973, 41, 1015. [Google Scholar] [CrossRef]
- Liboff, E.I. On the potentialx 2N and the correspondence principle. Int. J. Theor. Phys. 1979, 47, 185. [Google Scholar] [CrossRef]
- Robinett, R.W. Wave packet revivals and quasirevivals in one-dimensional power law potentials. J. Math. Phys. 2000, 41, 1801. [Google Scholar] [CrossRef]
- Glauber, R.J. Coherent and Incoherent States of the Radiation Field. Phys. Rev. 1963, 131, 2766. [Google Scholar] [CrossRef]
- Antoine, J.-P.; Gazeau, J.-P.; Monceau, P.; Klauder, J.R.; Penson, K.A. Temporally stable coherent states for infinite well and Pöschl–Teller potentials. J. Math. Phys. 2001, 42, 2349. [Google Scholar] [CrossRef]
- Iqbal, S.; Saif, F. Gazeau-Klauder Coherent States of the Triangular-Well Potential. J. Russ. Laser Res. 2013, 34, 1. [Google Scholar] [CrossRef]
- Sargent, M.; Scully, M.O.; Lamb, W.E., Jr. Laser Physics; Addison-Wesley Publishing Company: Boston, MA, USA, 1974. [Google Scholar]
- Abdalla, M.S.; Khalil, E.M.; Obada, A.-S.F.; Perina, J.; Krepelka, J. Quantum statistical characteristics of the interaction between two two-level atoms and radiation field. J. Eur. Phys. J. Plus 2015, 130, 227. [Google Scholar]
- Khalil, E.M.; Abdalla, M.S.; Obada, A.-S.F.; Perina, J. Entropic uncertainty in two two-level atoms interacting with a cavity field in presence of degenerate parametric amplifier. J. Opt. Soc. Am. B 2010, 27, 266. [Google Scholar] [CrossRef]
- Buzek, V.; Vidiella-Barranco, A.; Knight, P.L. Superpositions of coherent states: Squeezing and dissipation. Phys. Rev. A 1992, 45, 6570. [Google Scholar] [CrossRef]
- Vidal, G.; Werner, R.F. Computable measure of entanglement. Phys. Rev. A 2002, 65, 032314. [Google Scholar] [CrossRef]
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdel-Khalek, S.; Berrada, K.; Khalil, E.M.; Obada, A.-S.F.; Reda, E.; Eleuch, H. Quantumness Measures for a System of Two Qubits Interacting with a Field in the Presence of the Time-Dependent Interaction and Kerr Medium. Entropy 2021, 23, 635. https://doi.org/10.3390/e23050635
Abdel-Khalek S, Berrada K, Khalil EM, Obada A-SF, Reda E, Eleuch H. Quantumness Measures for a System of Two Qubits Interacting with a Field in the Presence of the Time-Dependent Interaction and Kerr Medium. Entropy. 2021; 23(5):635. https://doi.org/10.3390/e23050635
Chicago/Turabian StyleAbdel-Khalek, Sayed, Kamal Berrada, Eied M. Khalil, Abdel-Shafy F. Obada, Esraa Reda, and Hichem Eleuch. 2021. "Quantumness Measures for a System of Two Qubits Interacting with a Field in the Presence of the Time-Dependent Interaction and Kerr Medium" Entropy 23, no. 5: 635. https://doi.org/10.3390/e23050635
APA StyleAbdel-Khalek, S., Berrada, K., Khalil, E. M., Obada, A.-S. F., Reda, E., & Eleuch, H. (2021). Quantumness Measures for a System of Two Qubits Interacting with a Field in the Presence of the Time-Dependent Interaction and Kerr Medium. Entropy, 23(5), 635. https://doi.org/10.3390/e23050635





