Topological Invariants of Vapor–Liquid, Vapor–Liquid–Liquid and Liquid–Liquid Phase Diagrams
Abstract
:1. Introduction
Topological Concepts Considered
2. Results
2.1. Vapor–Liquid Equilibrium and Vapor–Liquid–Liquid Equilibrium
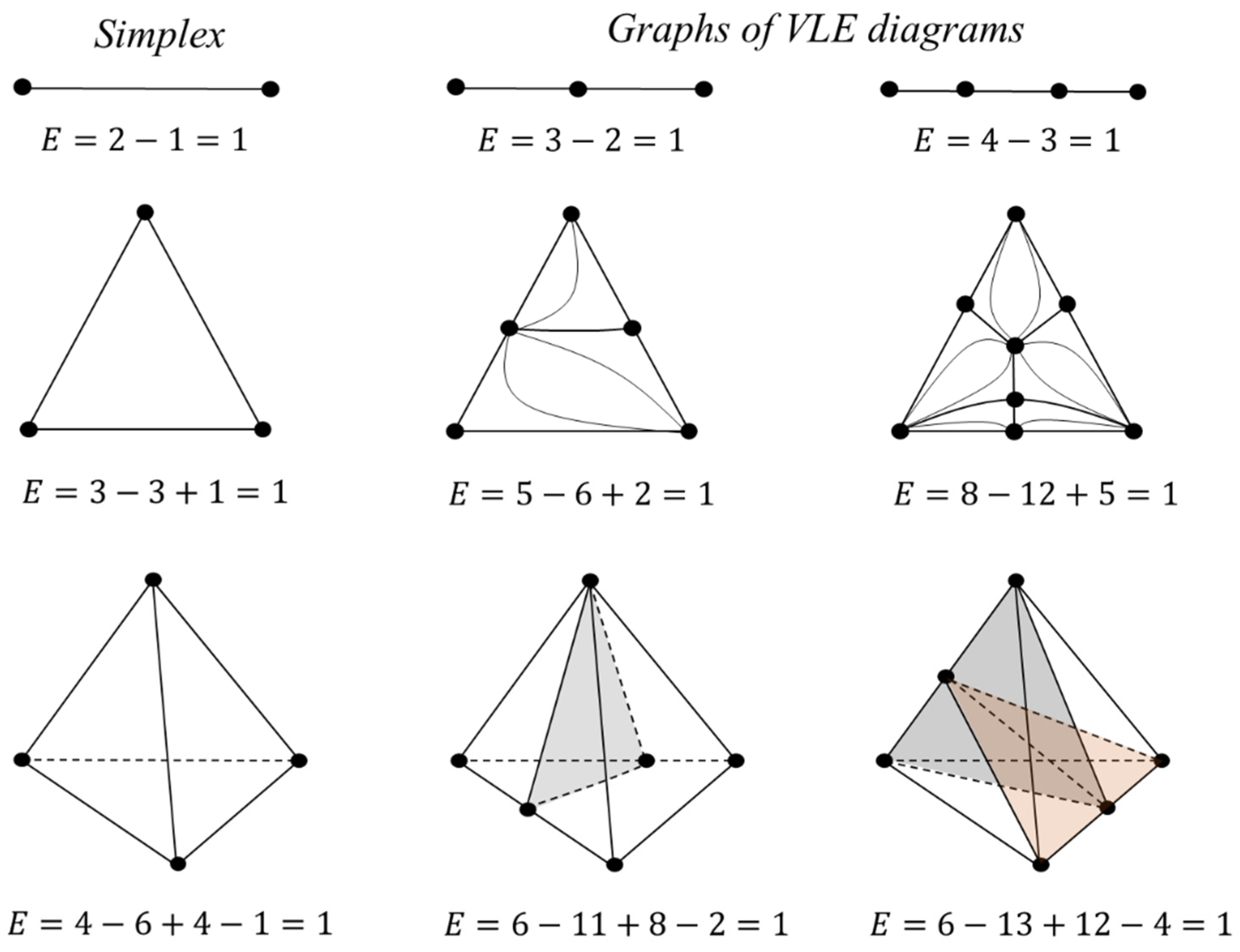
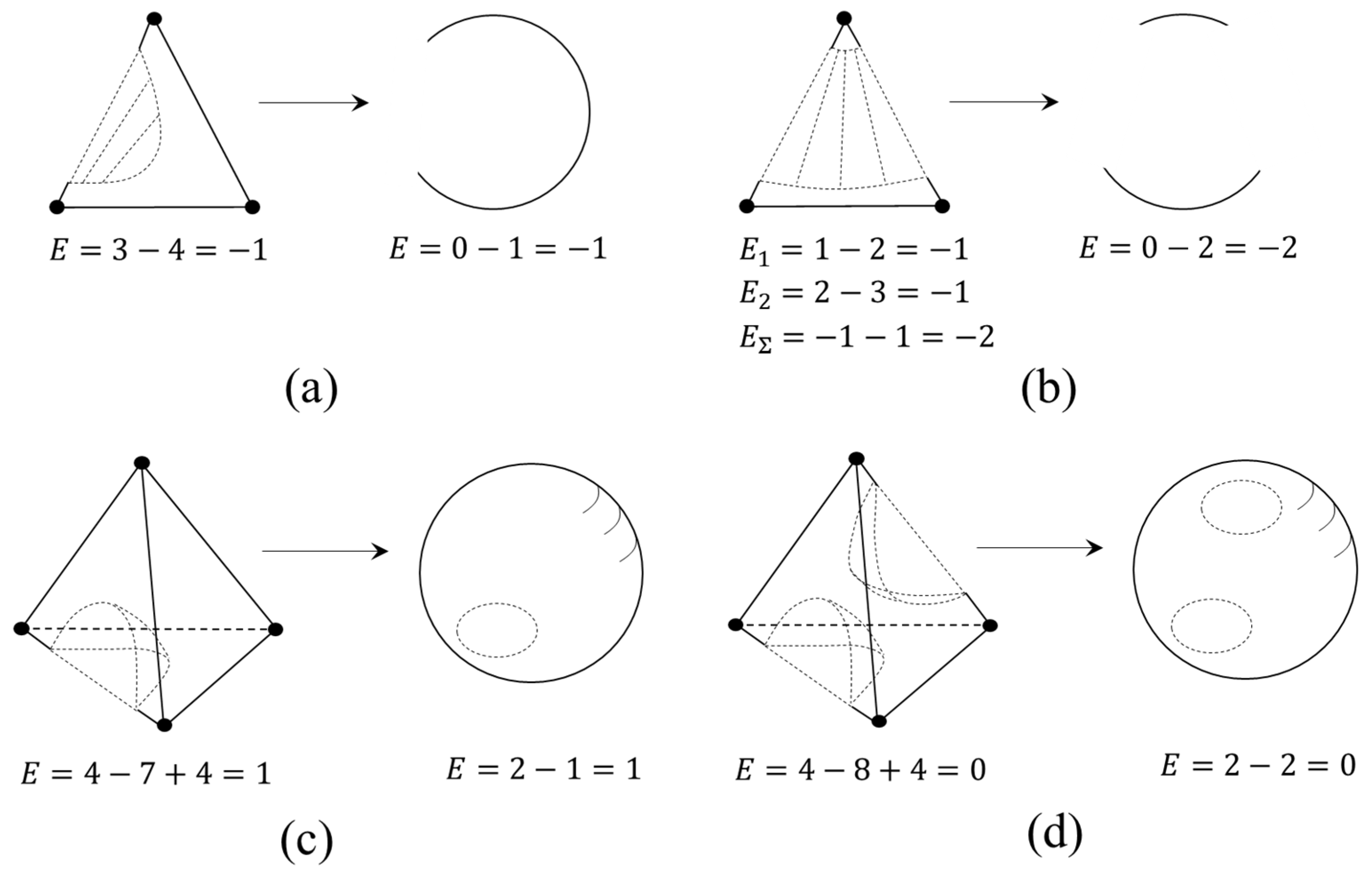
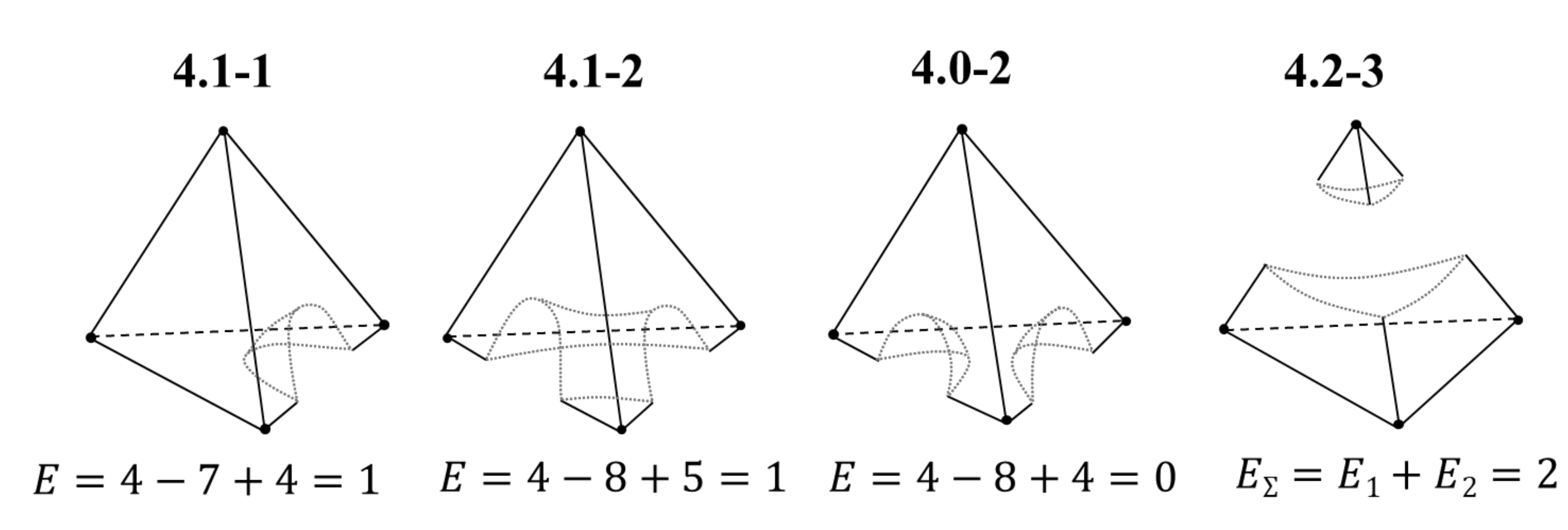
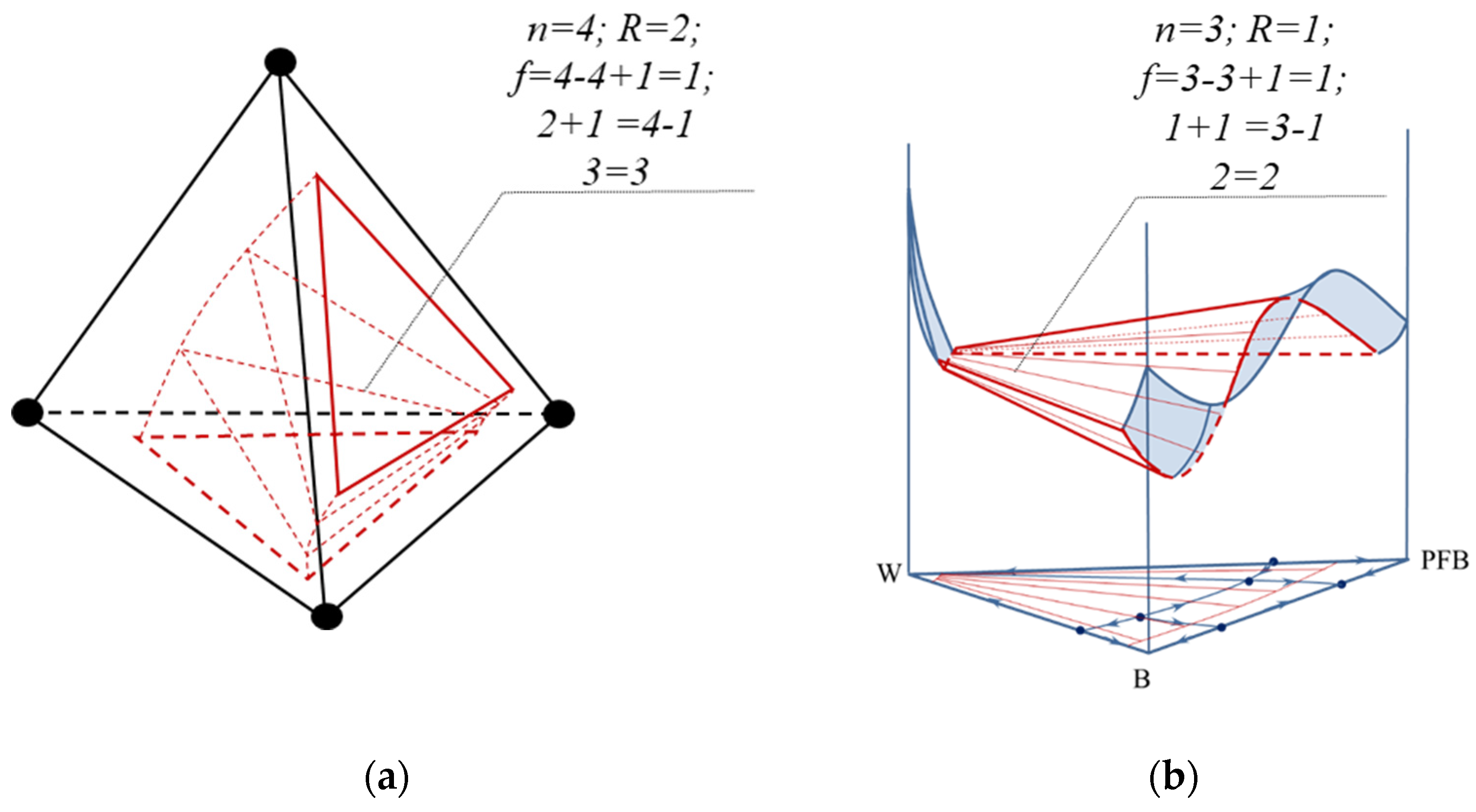
2.1.1. Euler Characteristic and the Balance Rule for Singular-Point Indices
2.1.2. The Balance of Singular-Point Indices Participating in the Bifurcation
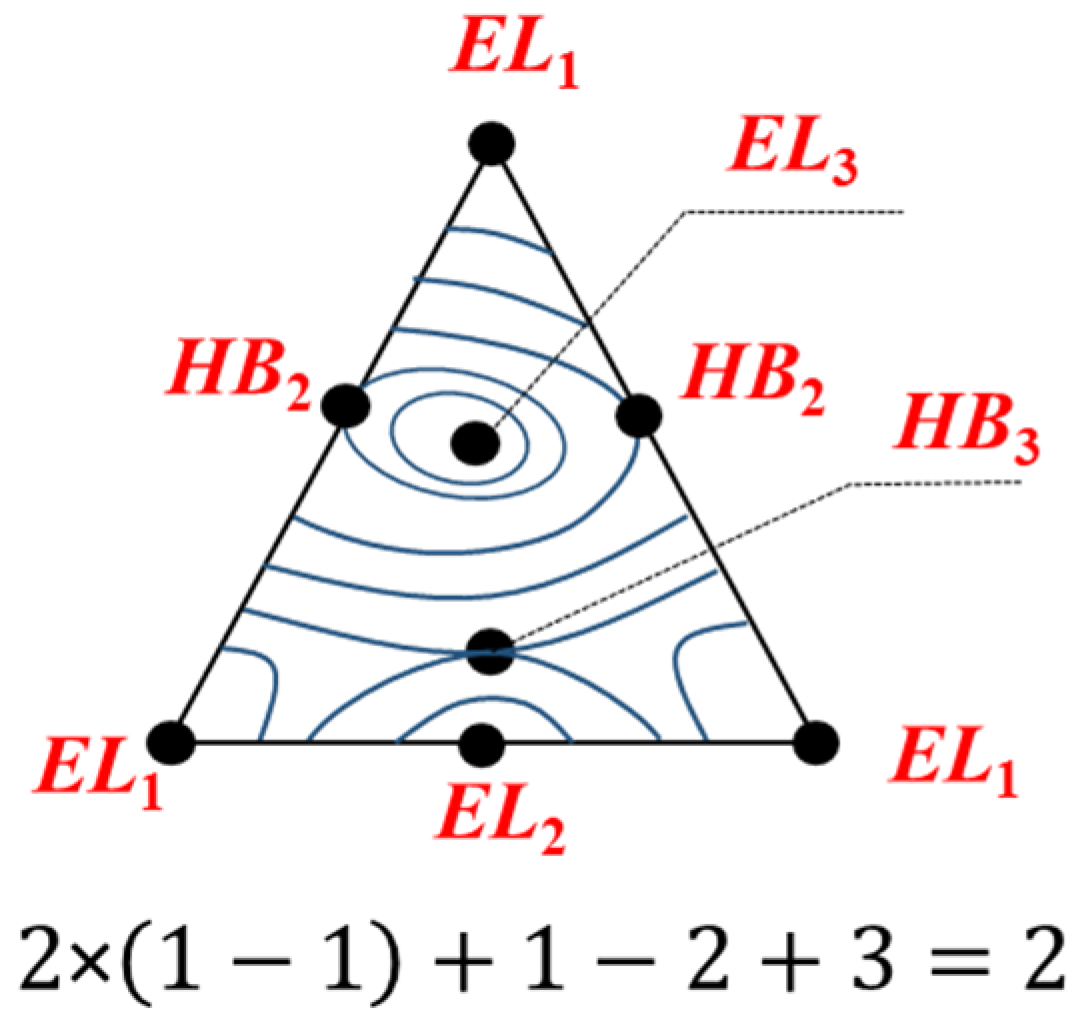
2.1.3. The Euler Characteristic as an Alternative Sum of Elements of Different Dimensions
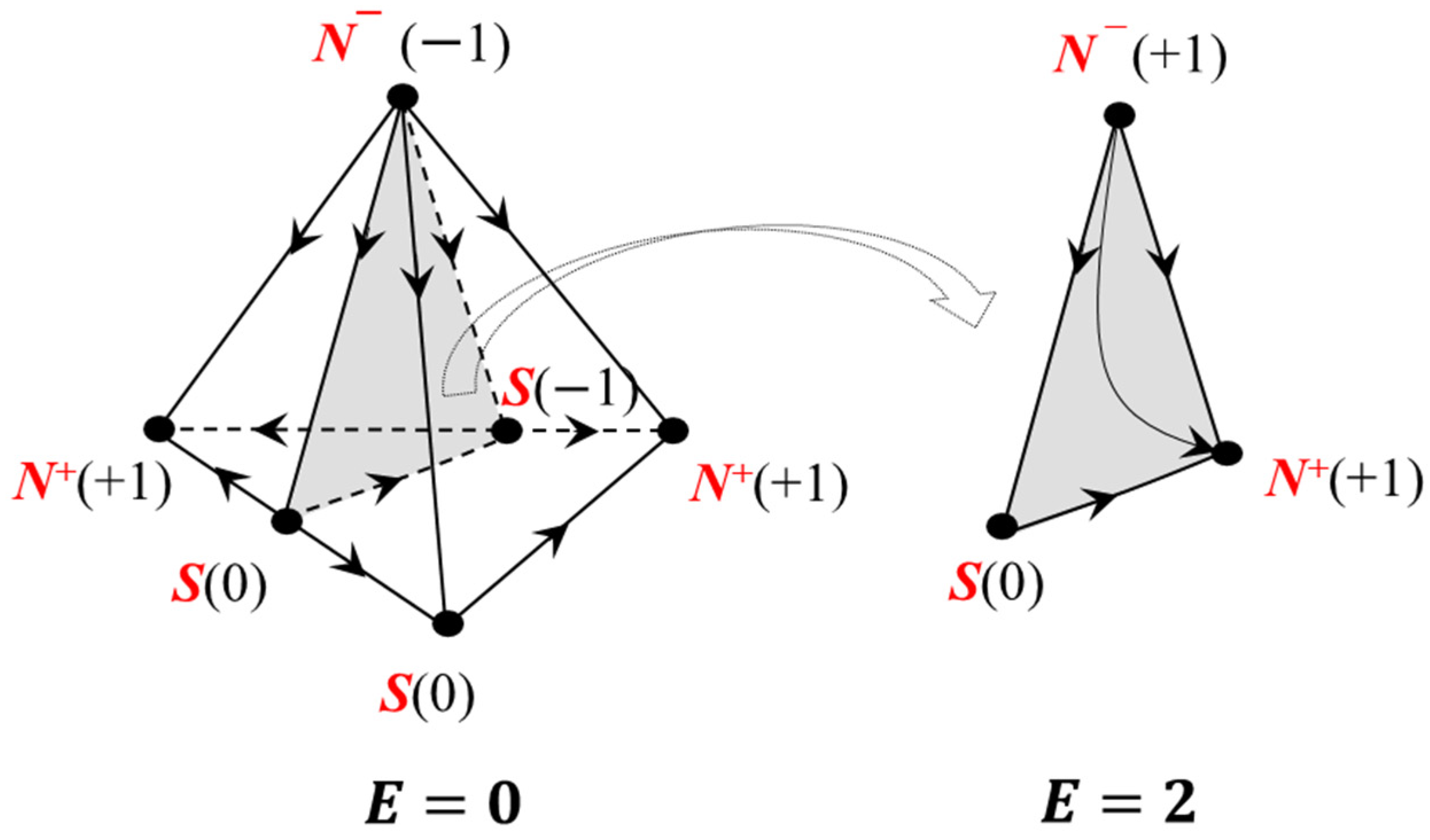
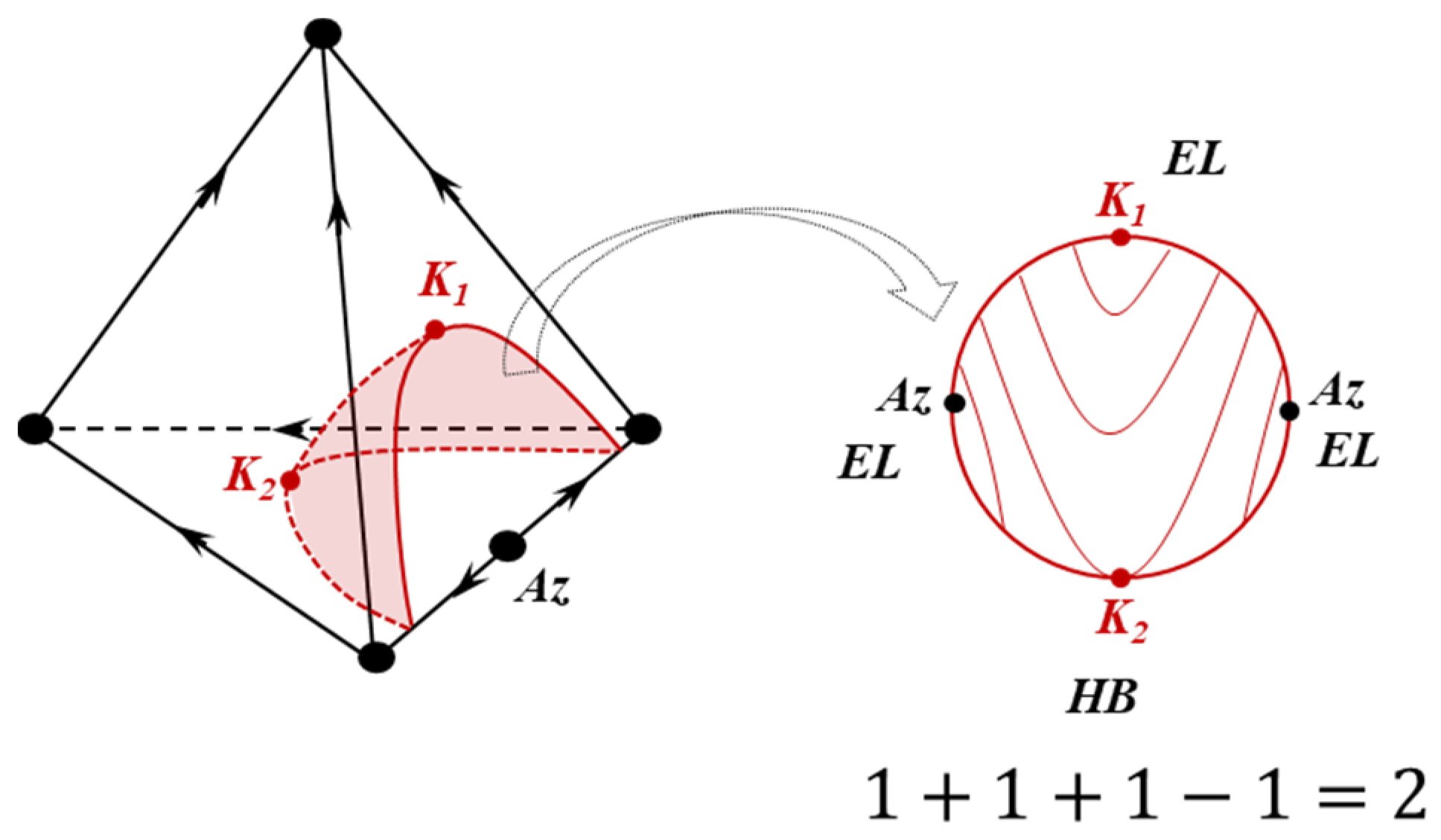
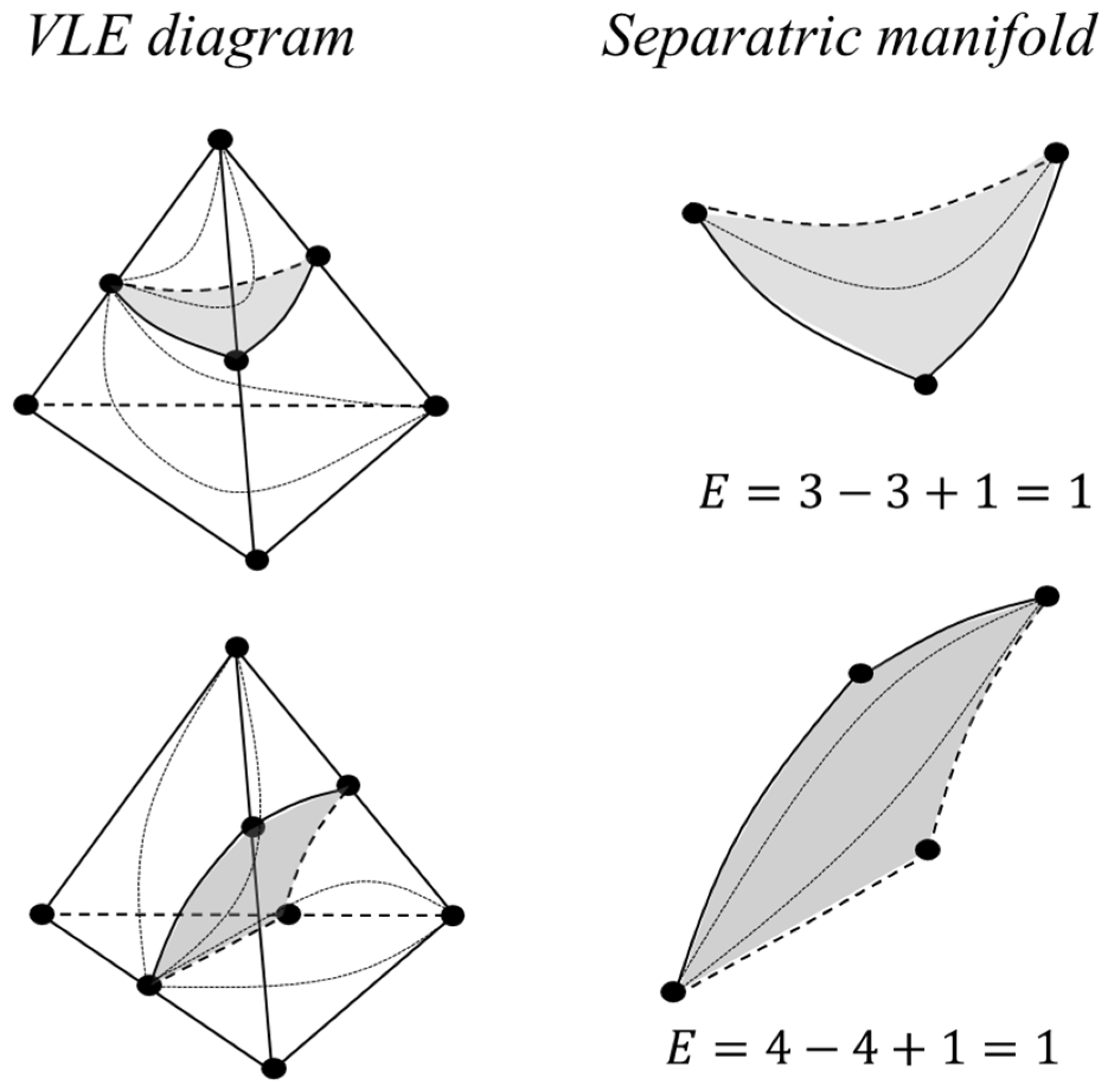
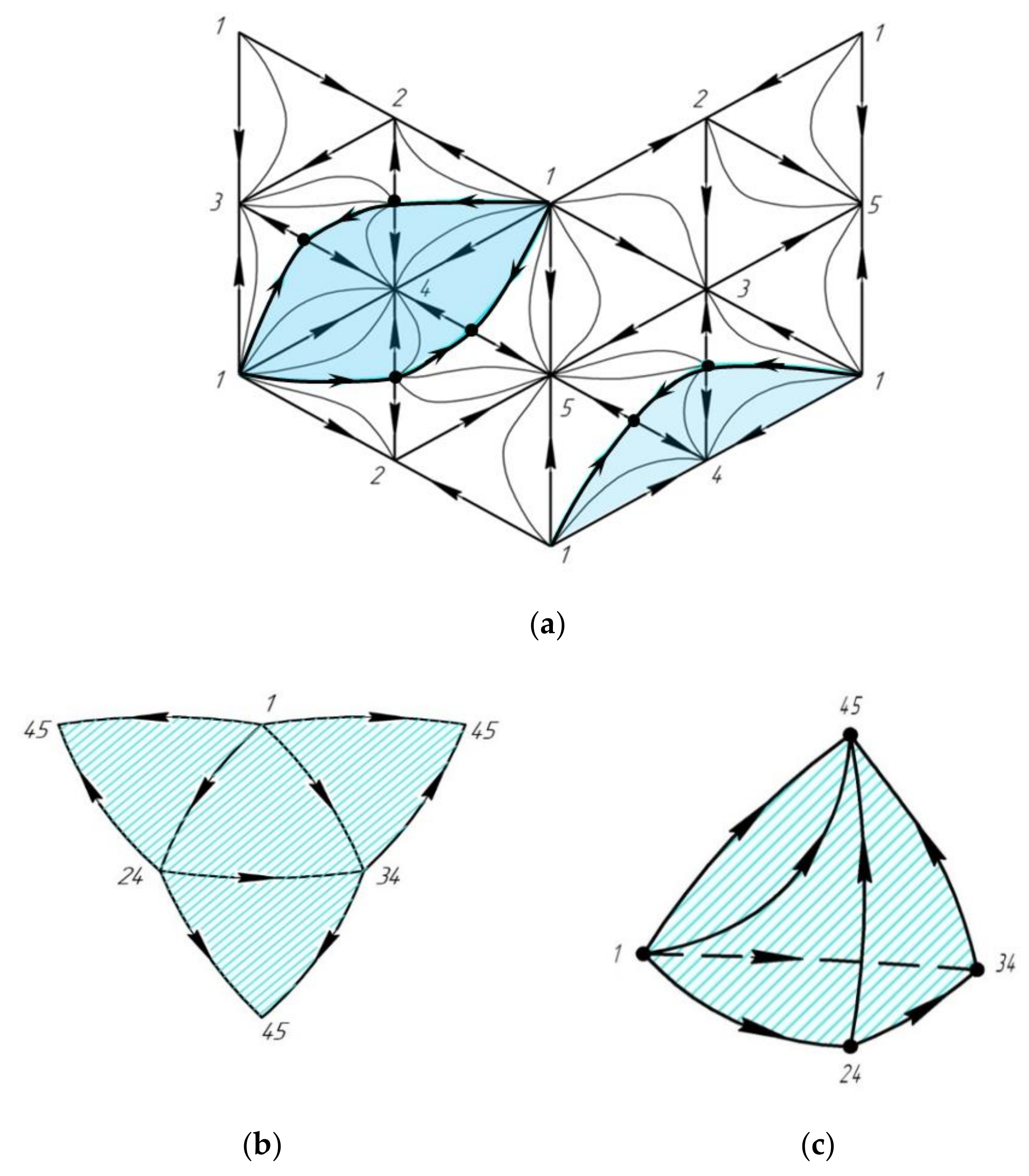
2.1.4. Poincaré Integral Invariant and Separatrix Manifolds
2.2. Liquid–Liquid Equilibrium
2.2.1. Invariant of the LLE Diagram
2.2.2. Invariant of the Liquid-Phase Splitting Region
3. Conclusions
Funding
Conflicts of Interest
References
- Storonkin, A.V. Thermodynamics of Heterogeneous Systems; Leningr. Gos. Univ.: Leningrad, Russia, 1967; p. 447. (In Russian) [Google Scholar]
- Zharov, V.T.; Serafimov, L.A. Physicochemical Foundations of Simple Distillation and Rectification; Chemistry Publishing Co.: Lenningrad, Russia, 1975; p. 240. (In Russian) [Google Scholar]
- Gurikov, Y.V. Structure of the Vapor-Liquid Equilibrium Diagrams of Ternary Homogeneous Solutions. Russ. J. Phys. Chem. 1958, 32, 1980–1996. [Google Scholar]
- Serafimov, L.A. Thermodynamic and topological analysis and problems of the separation of multicomponent polyazeotropic mixtures. Teor. Osn. Khim. Tekhnol. 1987, 21, 74–80. (In Russian) [Google Scholar]
- Serafimov, L.A. Thermodynamic and topological analysis of heterogeneous equilibrium diagrams of multicomponent mixtures. Russ. J. Phys. Chem. A 2002, 76, 1211–1224. [Google Scholar]
- Serafimov, L.A. State of the art in the thermodynamic and topological analysis of phase diagrams. Theor. Found. Chem. Eng. 2009, 43, 268–278. [Google Scholar] [CrossRef]
- Serafimov, L.A.; Zharov, V.T.; Timofeev, V.S. Rectification of Multicomponent Mixtures I. Topological Analysis of Liquid-Vapor Phase Equilibrium Diagrams. Acta Chem. Acad. Sci. Hung. 1971, 69, 383–396. [Google Scholar]
- Toikka, A.M.; Samarov, A.A.; Toikka, M.A. Phase and chemical equilibria in multicomponent fluid systems with a chemical reaction. Russ. Chem. Rev. 2015, 84, 378–392. [Google Scholar] [CrossRef] [Green Version]
- Pisarenko, Y.A.; Serafimov, L.A.; Kulov, N.N. Reactive distillation involving multiple chemical reactions: Principles of statics analysis. Theor. Found. Chem. Eng. 2009, 43, 591–605. [Google Scholar] [CrossRef]
- Kiva, V.N.; Hilmen, E.K.; Skogestad, S. Azeotropic phase equilibrium diagrams: A survey. Chem. Eng. Sci. 2003, 58, 1903–1953. [Google Scholar] [CrossRef]
- Serafimov, L.A.; Frolkova, A.K.; Khakhin, L.A. The euler characterisic as a topological invariant of the azeotropy rules. Vestnik MITHT 2014, 9, 45–55. (In Russian) [Google Scholar]
- Serafimov, L.A.; Frolkova, A.K.; Frolkova, A.V. Poincaré integral invariants and separating manifolds of equilibrium open evaporation diagrams. Theor. Found. Chem. Eng. 2013, 47, 124–127. [Google Scholar] [CrossRef]
- Serafimov, L.A.; Frolkova, A.K. Topological structure of state regions and isothermic-isobaric manifolds for multiphase systems. Theor. Found. Chem. Eng. 2000, 34, 286–291. [Google Scholar]
- Frolkova, A.V.; Serafimov, L.A.; Semin, G.A. Euler characteristic as a structural invariant of the phase diagrams of multicomponent multiphase systems. Theor. Found. Chem. Eng. 2014, 48, 158–166. [Google Scholar] [CrossRef]
- Serafimov, L.A.; Frolkova, A.V.; Semin, G.A. Euler characteristic of splitting diagrams of multicomponent multiphase systems. Fine Chem. Technol. 2015, 10, 39–44. (In Russian) [Google Scholar]
- Kosyakov, V.I. Topological images of phase diagrams. 1. Determination of the topology of phase diagrams. Russ. J. Inorg. Chem. 2010, 55, 1886–1993. (In Russian) [Google Scholar]
- Kosyakov, V.I. Topological images of phase diagrams. 2. Description of the topology of phase diagrams. Russ. J. Inorg. Chem. 2010, 55, 1894–1902. (In Russian) [Google Scholar]
- Kosyakov, V.I.; Bredan, Y.M.; Shestakov, V.A. Informal Mathematical Models in Chemical Thermodynamics; Nauka: Novosibirsk, Russia, 1991; p. 130. [Google Scholar]
- Kosyakov, V.I.; Sinyakova, E.F. Investigation of a monovariant peritectic reaction in a three-component FE-NI-S system by the method of directed crystallization. Russ. J. Inorg. Chem. 1991, 7, 1170–1175. (In Russian) [Google Scholar]
- Serafimov, L.A.; Fedorov, P.P.; Buchinskaya, I.I. Phase portraits of ternary mutual systems with continuous solid phases. Russ. J. Inorg. Chem. 2002, 47, 1371–1377. [Google Scholar]
- Serafimov, L.A.; Fedorov, P.P.; Safonov, V.V. Distribution of singular point over the liquidus surface of phase diagrams of reciprocal systems. Russ. J. Inorg. Chem. 2002, 47, 105–112. [Google Scholar]
- Fedorov, P.P. Thermodynamic-topological analysis of melt solidificaion in the vicinity of singular points in phase diagrams. Russ. J. Inorg. Chem. 2005, 50, 1933–1941. [Google Scholar]
- Cherkasov, D.G.; Il’In, K.K.; Kurskii, V.F. Topological transformation of a phase diagram for the sodium nitrate-water-isopropanol ternary system. Russ. J. Inorg. Chem. 2011, 56, 787–791. [Google Scholar] [CrossRef]
- Kurskii, V.F.; Cherkasov, D.G.; Il’In, K.K. Topological transformation of a phase diagram for the sodium nitrate-water-acetonitrile ternary system. Izv. Sarat. Univ. Chem. Biol. Ecol. 2006, 6, 9–13. (In Russian) [Google Scholar]
- Jiang, H.; Yang, C.; Chen, S. Topological invariants and phase diagrams for one-dimensional two-band non-Hermitian systems without chiral symmetry. Phys. Rev. A 2018, 98, 052116. [Google Scholar] [CrossRef] [Green Version]
- Poincaré, H. Sur le probléme des trois corps et les équations de la ynamique. Acta Math. 1890, 13, 1–270. [Google Scholar]
- Polak, L.S. Variational Principles of Mechanics and Their Development and Application in Physics; LIBROCOM: Moscow, Russia, 2010; p. 600. [Google Scholar]
- Serafmov, L.A. The azeotropic rule and the classification of multicomponent mixtures. IV. Principal equations for the calculation of liquid–vapor phase equilibrium diagrams for four-component mixtures. Russ. J. Phys. Chem. A 1969, 43, 621–624. [Google Scholar]
- Serafimov, L.A.; Chelyuskina, T.V.; Tatsievskaya, G.I. The special features of liquid-vapor phase diagrams and an analysis of the forms of azeotropy rules. Russ. J. Phys. Chem. A 2009, 83, 172–178. [Google Scholar] [CrossRef]
- Serafimov, L.A.; Frolkova, A.V. The law of the algebraic sum of stationary points of vapor-liquid equilibrium diagrams of multicomponent mixtures. Theor. Found. Chem. Eng. 2013, 47, 680–689. [Google Scholar] [CrossRef]
- Serafimov, L.A.; Frolkova, A.V.; Medvedev, D.V.; Semin, G.A. Determining the structure of the distillation line diagram from its geometric development for four-component mixtures. Theor. Found. Chem. Eng. 2012, 46, 120–127. [Google Scholar] [CrossRef]
- Serafimov, L.; Frolkova, A. Determination of vapor-liquid equilibrium diagrams of multicomponent systems. Chem. Pap. 2016, 70, 1578–1589. [Google Scholar] [CrossRef]
- Serafmov, L.A. The azeotropic rule and the classification of multicomponent mixtures VII. Diagrams for ternary mixtures. Russ. J. Phys. Chem. A 1970, 44, 567–571. [Google Scholar]
- Serafimov, L.A.; Razova, O.B.; Frolkova, A.V.; Chelyuskina, T.V. Observance of the Gibbs-Konovalov law at complex singular points of two-phase multicomponent systems. Theor. Found. Chem. Eng. 2008, 42, 415–420. [Google Scholar] [CrossRef]
- Raeva, V.M.; Serafimov, L.A.; Stepanov, V.N. The nonlocal characteristics of diagrams of isolines of the scalar properties of homogeneous three-component mixtures. Russ. J. Phys. Chem. A 2011, 85, 529–536. [Google Scholar] [CrossRef]
- Seifert, G.; Trelfall, F. Topology; GONTI: Moscow-Leningrad, Russia, 1938; p. 400. [Google Scholar]
- Zhuchkov, V.I.; Malyugin, A.A.; Frolkova, A.V.; Frolkova, A.K. Double ternary azeotrope in the benzene + perfluorobenzene + water system at 101 kPa. J. Chem. Eng. Data 2020, 65, 2002–2007. [Google Scholar] [CrossRef]
- Frolkova, A.V.; Ososkova, T.E.; Frolkova, A.K. Thermodynamic and topological analysis of phase diagrams of quaternary systems with internal singular points. Theor. Found. Chem. Eng. 2020, 54, 407–419. [Google Scholar] [CrossRef]
- Frolkova, A.V.; Akishina, A.A.; Frolkova, A.K.; Illarionova, E.V. The method of study of liquid-liquid-liquid equilibrium in quaternary systems. J. Chem. Eng. Data 2017, 62, 1348–1354. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frolkova, A.V. Topological Invariants of Vapor–Liquid, Vapor–Liquid–Liquid and Liquid–Liquid Phase Diagrams. Entropy 2021, 23, 1666. https://doi.org/10.3390/e23121666
Frolkova AV. Topological Invariants of Vapor–Liquid, Vapor–Liquid–Liquid and Liquid–Liquid Phase Diagrams. Entropy. 2021; 23(12):1666. https://doi.org/10.3390/e23121666
Chicago/Turabian StyleFrolkova, Anastasia V. 2021. "Topological Invariants of Vapor–Liquid, Vapor–Liquid–Liquid and Liquid–Liquid Phase Diagrams" Entropy 23, no. 12: 1666. https://doi.org/10.3390/e23121666
APA StyleFrolkova, A. V. (2021). Topological Invariants of Vapor–Liquid, Vapor–Liquid–Liquid and Liquid–Liquid Phase Diagrams. Entropy, 23(12), 1666. https://doi.org/10.3390/e23121666





