Stochastic Collisional Quantum Thermometry
Abstract
:1. Introduction
2. Quantum Thermometry
2.1. Thermal Fisher Information
2.2. Collisional Thermometry
2.3. Dephasing Interactions
2.4. Role of Correlations
2.5. Parameter Dependence
3. Stochastic Approach
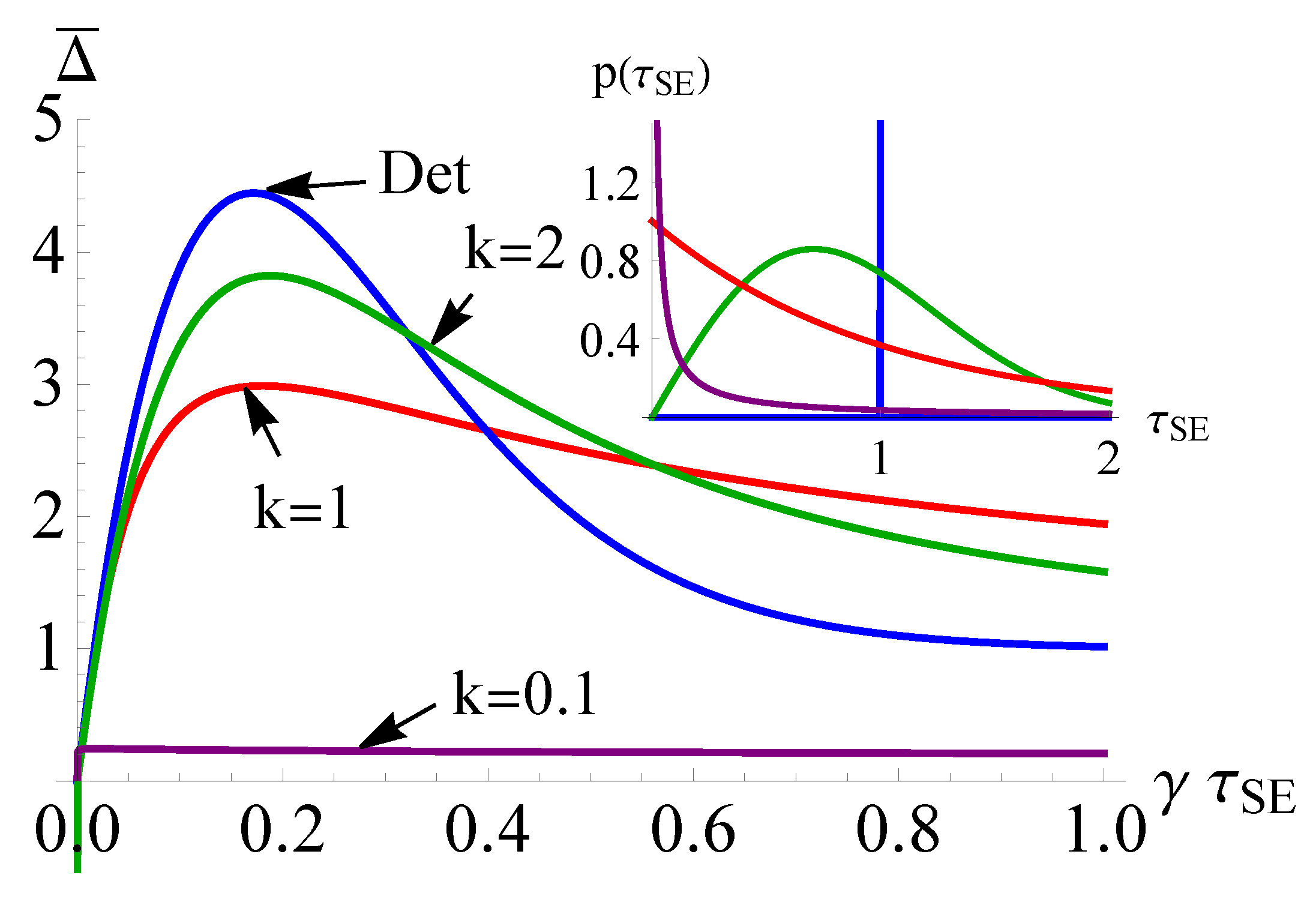
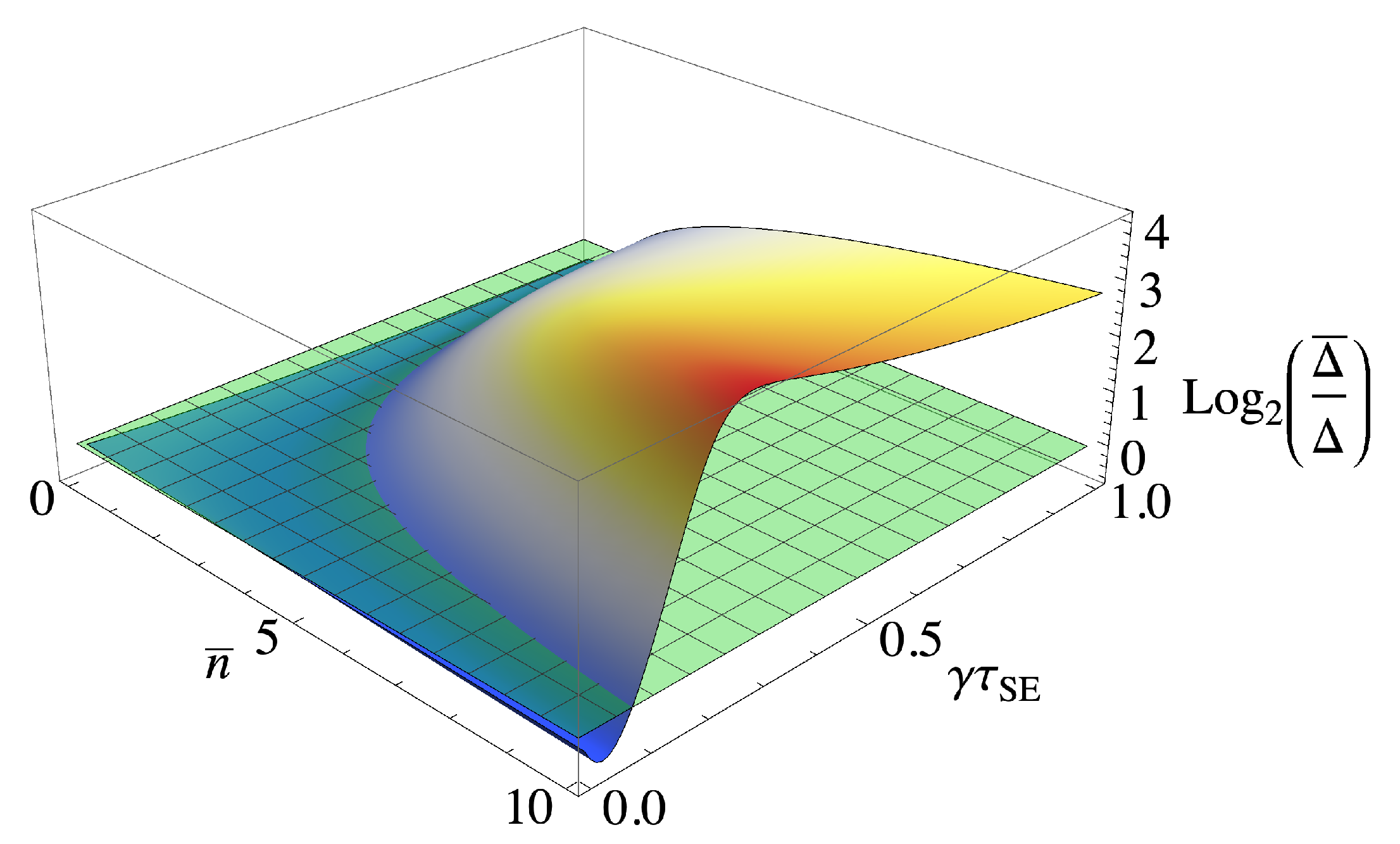
3.1. Random Collision Times
3.2. Optimal Measurements
3.3. Partial Swap Interactions
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Giovannetti, V.; Lloyd, S.; Maccone, L. Quantum metrology. Phys. Rev. Lett. 2006, 96, 010401. [Google Scholar] [CrossRef] [Green Version]
- Acín, A.; Bloch, I.; Buhrman, H.; Calarco, T.; Eichler, C.; Eisert, J.; Esteve, D.; Gisin, N.; Glaser, S.J.; Jelezko, F.; et al. The quantum technologies roadmap: A European community view. New J. Phys. 2018, 20, 080201. [Google Scholar] [CrossRef]
- Braun, D.; Adesso, G.; Benatti, F.; Floreanini, R.; Marzolino, U.; Mitchell, M.W.; Pirandola, S. Quantum-enhanced measurements without entanglement. Rev. Mod. Phys. 2018, 90, 035006. [Google Scholar] [CrossRef] [Green Version]
- Razavian, S.; Benedetti, C.; Bina, M.; Akbari-Kourbolagh, Y.; Paris, M.G. Quantum thermometry by single-qubit dephasing. Eur. Phys. J. Plus 2019, 134, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Correa, L.A.; Mehboudi, M.; Adesso, G.; Sanpera, A. Individual quantum probes for optimal thermometry. Phys. Rev. Lett. 2015, 114, 220405. [Google Scholar] [CrossRef] [Green Version]
- Mitchison, M.T.; Fogarty, T.; Guarnieri, G.; Campbell, S.; Busch, T.; Goold, J. In Situ Thermometry of a Cold Fermi Gas via Dephasing Impurities. Phys. Rev. Lett. 2020, 125, 080402. [Google Scholar] [CrossRef]
- Mehboudi, M.; Sanpera, A.; Correa, L.A. Thermometry in the quantum regime: Recent theoretical progress. J. Phys. A 2019, 52, 303001. [Google Scholar] [CrossRef] [Green Version]
- Maccone, L. Intuitive reason for the usefulness of entanglement in quantum metrology. Phys. Rev. A 2013, 88, 042109. [Google Scholar] [CrossRef] [Green Version]
- Huang, Z.; Macchiavello, C.; Maccone, L. Usefulness of entanglement-assisted quantum metrology. Phys. Rev. A 2016, 94, 012101. [Google Scholar] [CrossRef] [Green Version]
- Micadei, K.; Rowlands, D.A.; Pollock, F.A.; Céleri, L.C.; Serra, R.M.; Modi, K. Coherent measurements in quantum metrology. New J. Phys. 2015, 17, 023057. [Google Scholar] [CrossRef] [Green Version]
- Pires, D.P.; Silva, I.A.; Deazevedo, E.R.; Soares-Pinto, D.O.; Filgueiras, J.G. Coherence orders, decoherence, and quantum metrology. Phys. Rev. A 2018, 98, 032101. [Google Scholar] [CrossRef] [Green Version]
- Castellini, A.; Lo Franco, R.; Lami, L.; Winter, A.; Adesso, G.; Compagno, G. Indistinguishability-enabled coherence for quantum metrology. Phys. Rev. A 2019, 100, 012308. [Google Scholar] [CrossRef]
- Campbell, S.; Mehboudi, M.; Chiara, G.D.; Paternostro, M. Global and local thermometry schemes in coupled quantum systems. New J. Phys. 2017, 19, 103003. [Google Scholar] [CrossRef]
- Jahromi, H.R. Quantum thermometry in a squeezed thermal bath. Phys. Scr. 2020, 95, 035107. [Google Scholar] [CrossRef] [Green Version]
- Correa, L.A.; Perarnau-Llobet, M.; Hovhannisyan, K.V.; Hernández-Santana, S.; Mehboudi, M.; Sanpera, A. Enhancement of low temperature thermometry by strong coupling. Phys. Rev. A 2017, 96, 062103. [Google Scholar] [CrossRef] [Green Version]
- Hovhannisyan, K.V.; Correa, L.A. Measuring the temperature of cold many-body quantum systems. Phys. Rev. B 2018, 98, 045101. [Google Scholar] [CrossRef] [Green Version]
- Ivanov, P.A. Quantum thermometry with trapped ions. Opt. Commun. 2019, 436, 101–107. [Google Scholar] [CrossRef] [Green Version]
- Jevtic, S.; Newman, D.; Rudolph, T.; Stace, T.M. Single-qubit thermometry. Phys. Rev. A 2015, 91, 12331. [Google Scholar] [CrossRef] [Green Version]
- Kiilerich, A.H.; De Pasquale, A.; Giovannetti, V. Dynamical approach to ancilla-assisted quantum thermometry. Phys. Rev. A 2018, 98, 042124. [Google Scholar] [CrossRef] [Green Version]
- Mancino, L.; Sbroscia, M.; Gianani, I.; Roccia, E.; Barbieri, M. Quantum Simulation of Single-Qubit Thermometry Using Linear Optics. Phys. Rev. Lett. 2017, 118, 130502. [Google Scholar] [CrossRef]
- Potts, P.P.; Brask, J.B.; Brunner, N. Fundamental limits on low-temperature quantum thermometry with finite resolution. Quantum 2019, 3, 161. [Google Scholar] [CrossRef] [Green Version]
- Campbell, S.; Vacchini, B. Collision models in open system dynamics: A versatile tool for deeper insights? EPL (Europhys. Lett.) 2021, 133, 60001. [Google Scholar] [CrossRef]
- Ciccarello, F.; Lorenzo, S.; Giovannetti, V.; Palma, G.M. Quantum collision models: Open system dynamics from repeated interactions. arXiv 2021, arXiv:2106.11974. [Google Scholar]
- Lorenzo, S.; Ciccarello, F.; Palma, G.M. Composite quantum collision models. Phys. Rev. A 2017, 96, 032107. [Google Scholar] [CrossRef] [Green Version]
- De Chiara, G.; Antezza, M. Quantum machines powered by correlated baths. Phys. Rev. Res. 2020, 2, 033315. [Google Scholar] [CrossRef]
- Taranto, P.; Bakhshinezhad, F.; Schüttelkopf, P.; Clivaz, F.; Huber, M. Exponential Improvement for Quantum Cooling through Finite-Memory Effects. Phys. Rev. Appl. 2020, 14, 054005. [Google Scholar] [CrossRef]
- Campbell, S.; Ciccarello, F.; Palma, G.M.; Vacchini, B. System-environment correlations and Markovian embedding of quantum non-Markovian dynamics. Phys. Rev. A 2018, 98, 012142. [Google Scholar] [CrossRef]
- McCloskey, R.; Paternostro, M. Non-Markovianity and system-environment correlations in a microscopic collision model. Phys. Rev. A 2014, 89, 052120. [Google Scholar] [CrossRef] [Green Version]
- De Chiara, G.; Landi, G.; Hewgill, A.; Reid, B.; Ferraro, A.; Roncaglia, A.J.; Antezza, M. Reconciliation of quantum local master equations with thermodynamics. New J. Phys. 2018, 20, 113024. [Google Scholar] [CrossRef]
- Grimmer, D.; Layden, D.; Mann, R.B.; Martín-Martínez, E. Open dynamics under rapid repeated interaction. Phys. Rev. A 2016, 94, 032126. [Google Scholar] [CrossRef] [Green Version]
- Scarani, V.; Ziman, M.; Štelmachovič, P.; Gisin, N.; Bužek, V. Thermalizing quantum machines: Dissipation and entanglement. Phys. Rev. Lett. 2002, 88, 097905. [Google Scholar] [CrossRef] [Green Version]
- Strasberg, P.; Schaller, G.; Brandes, T.; Esposito, M. Quantum and information thermodynamics: A unifying framework based on repeated interactions. Phys. Rev. X 2017, 7, 021003. [Google Scholar] [CrossRef] [Green Version]
- Seah, S.; Nimmrichter, S.; Grimmer, D.; Santos, J.P.; Scarani, V.; Landi, G.T. Collisional Quantum Thermometry. Phys. Rev. Lett. 2019, 123, 180602. [Google Scholar] [CrossRef] [Green Version]
- Shu, A.; Seah, S.; Scarani, V. Surpassing the thermal Cramér-Rao bound with collisional thermometry. Phys. Rev. A 2020, 102, 042417. [Google Scholar] [CrossRef]
- Alves, G.O.; Landi, G.T. Bayesian estimation for collisional thermometry. arXiv 2021, arXiv:2106.12072. [Google Scholar]
- Ollivier, H.; Zurek, W.H. Quantum discord: A measure of the quantumness of correlations. Phys. Rev. Lett. 2001, 88, 017901. [Google Scholar] [CrossRef]
- Henderson, L.; Vedral, V. Classical, quantum and total correlations. J. Phys. A 2001, 34, 6899. [Google Scholar] [CrossRef]
- Luiz, F.S.; Junior, A.; Fanchini, F.F.; Landi, G.T. Machine classification for probe based quantum thermometry. arXiv 2021, arXiv:2107.04555. [Google Scholar]
- Liu, J.; Yuan, H.; Lu, X.M.; Wang, X. Quantum Fisher information matrix and multiparameter estimation. J. Phys. A 2019, 53, 023001. [Google Scholar] [CrossRef]
- Strasberg, P. Operational approach to quantum stochastic thermodynamics. Phys. Rev. E 2019, 100, 022127. [Google Scholar] [CrossRef] [Green Version]
- Vacchini, B. Quantum renewal processes. Sci. Rep. 2020, 10, 1–13. [Google Scholar] [CrossRef] [PubMed]
- Chisholm, D.A.; García-Pérez, G.; Rossi, M.A.C.; Palma, G.M.; Maniscalco, S. Stochastic collision model approach to transport phenomena in quantum networks. New J. Phys. 2021, 23, 033031. [Google Scholar] [CrossRef]
- Tabanera, J.; Luque, I.; Jacob, S.L.; Esposito, M.; Barra, F.; Parrondo, J.M.R. Quantum collisional thermostats. arXiv 2021, arXiv:2109.10620. [Google Scholar]
- Ehrich, J.; Esposito, M.; Barra, F.; Parrondo, J.M.R. Micro-reversibility and thermalization with collisional baths. Physica A 2020, 552, 122108. [Google Scholar] [CrossRef] [Green Version]
- Jacob, S.L.; Esposito, M.; Parrondo, J.M.R.; Barra, F. Thermalization Induced by Quantum Scattering. PRX Quantum 2021, 2, 020312. [Google Scholar] [CrossRef]
- Miller, H.J.D.; Anders, J. Energy-temperature uncertainty relation in quantum thermodynamics. Nat. Commun. 2018, 9, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Mok, W.K.; Bharti, K.; Kwek, L.C.; Bayat, A. Optimal probes for global quantum thermometry. Commun. Phys. 2021, 4, 1–8. [Google Scholar] [CrossRef]
- Mehboudi, M.; Jørgensen, M.R.; Seah, S.; Brask, J.B.; Kołodyński, J.; Perarnau-Llobet, M. Fundamental limits in Bayesian thermometry and attainability via adaptive strategies. arXiv 2021, arXiv:2108.05932. [Google Scholar]
- Jørgensen, M.R.; Kołodyński, J.; Mehboudi, M.; Perarnau-Llobet, M.; Brask, J.B. Bayesian quantum thermometry based on thermodynamic length. arXiv 2021, arXiv:2108.05901. [Google Scholar]
- De Pasquale, A.; Yuasa, K.; Giovannetti, V. Estimating temperature via sequential measurements. Phys. Rev. A 2017, 96, 012316. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
O’Connor, E.; Vacchini, B.; Campbell, S. Stochastic Collisional Quantum Thermometry. Entropy 2021, 23, 1634. https://doi.org/10.3390/e23121634
O’Connor E, Vacchini B, Campbell S. Stochastic Collisional Quantum Thermometry. Entropy. 2021; 23(12):1634. https://doi.org/10.3390/e23121634
Chicago/Turabian StyleO’Connor, Eoin, Bassano Vacchini, and Steve Campbell. 2021. "Stochastic Collisional Quantum Thermometry" Entropy 23, no. 12: 1634. https://doi.org/10.3390/e23121634
APA StyleO’Connor, E., Vacchini, B., & Campbell, S. (2021). Stochastic Collisional Quantum Thermometry. Entropy, 23(12), 1634. https://doi.org/10.3390/e23121634





