Topology and Phase Transitions: A First Analytical Step towards the Definition of Sufficient Conditions
Abstract
1. Introduction
2. Entropy Flow
2.1. Introduction to the Geometric Approach
2.2. Entropy Flow Equation
2.2.1. First Variation of Volume
2.2.2. Second Variation of Volume
2.2.3. Entropy Flow
3. A Consistency Check
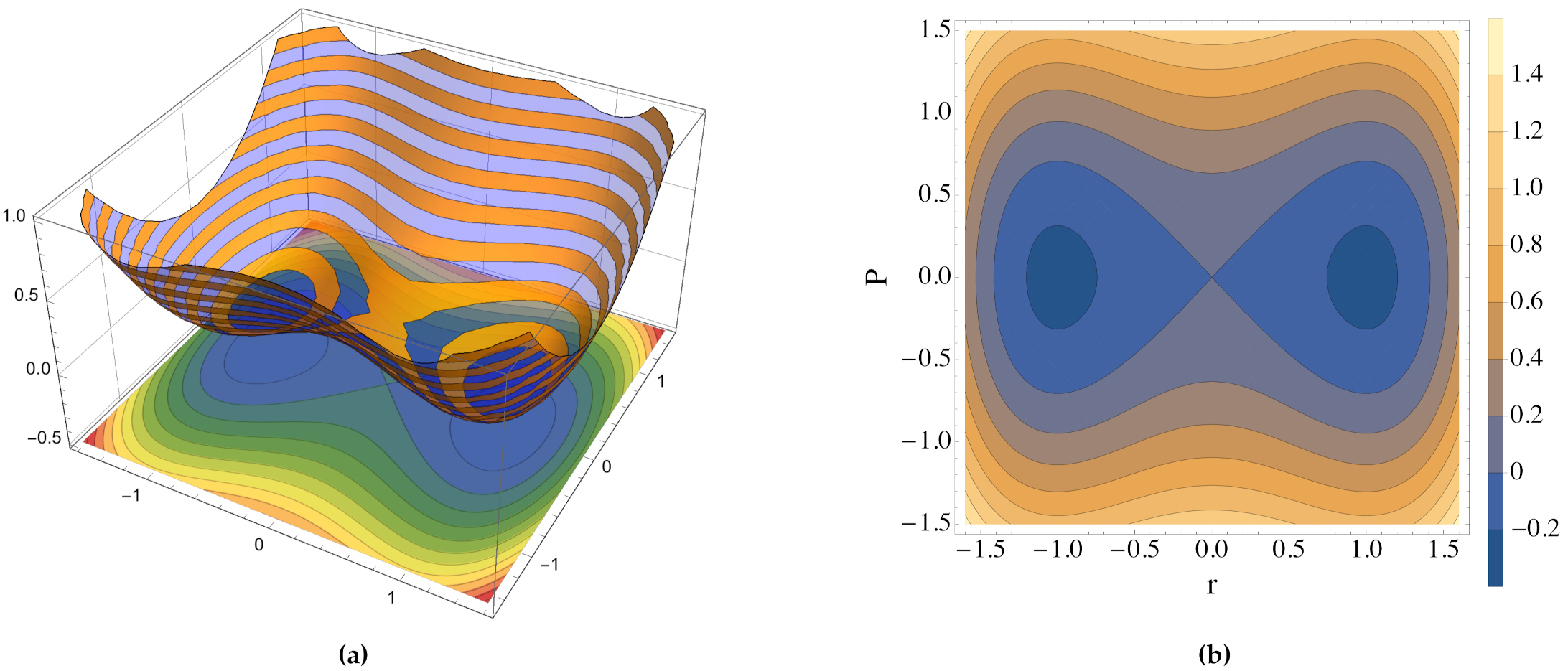
3.1. Harmonic Oscillators and Ginzburg–Landau-Like Potential
3.2. Geometry of the Energy Level Sets
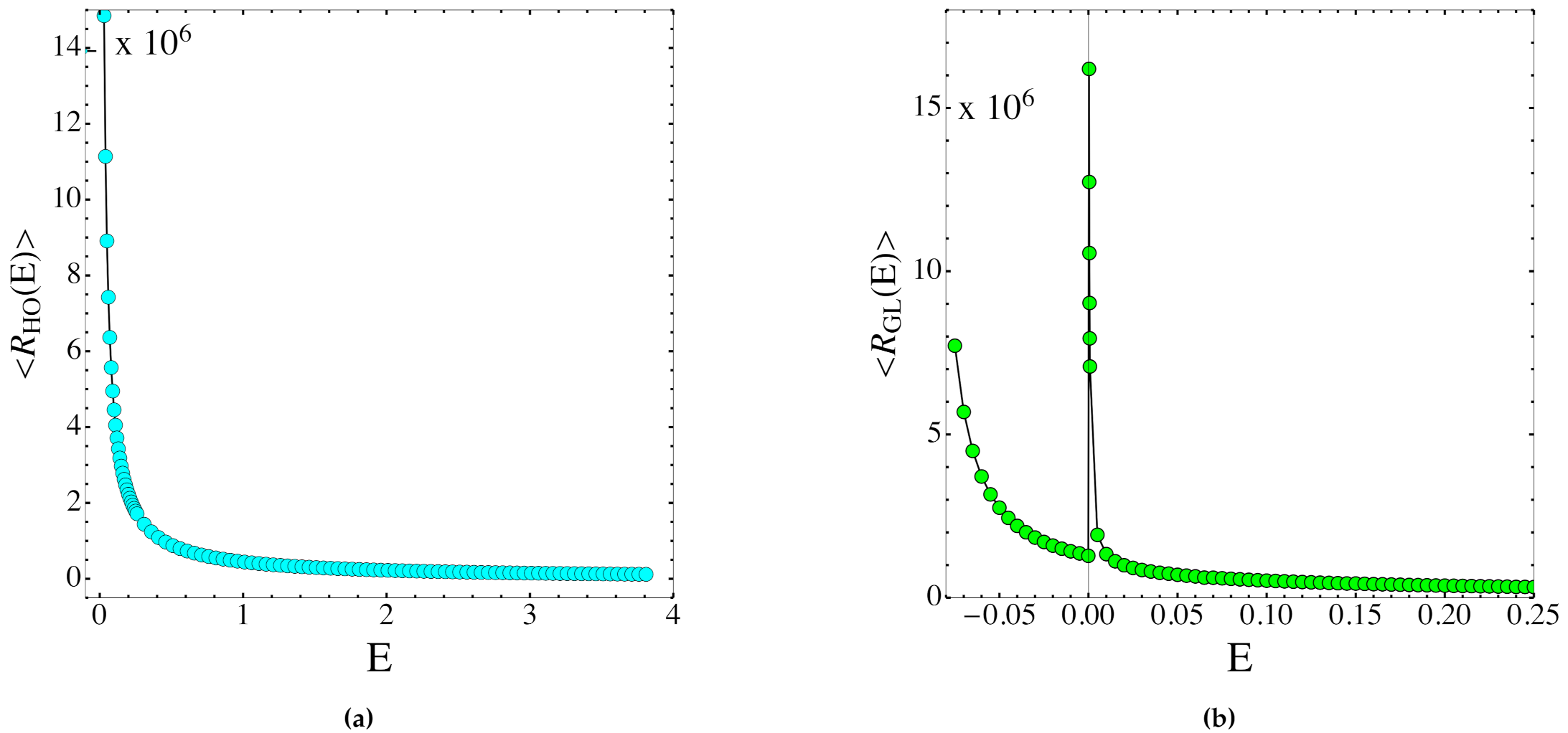
3.3. Numerical Results
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pettini, M. Geometrical hints for a nonperturbative approach to Hamiltonian dynamics. Phys. Rev. E 1993, 47, 828. [Google Scholar] [CrossRef]
- Di Cairano, L.; Matteo, G.; Pettini, M. Coherent Riemannian-geometric description of Hamiltonian order and chaos with Jacobi metric. Chaos 2019, 29, 123134. [Google Scholar] [CrossRef] [PubMed]
- Di Cairano, L.; Matteo, G.; Giulio, P.; Marco, P. Hamiltonian chaos and differential geometry of configuration space–time. Phys. D Nonlinear Phenom. 2021, 422, 132909. [Google Scholar] [CrossRef]
- Pettini, M. Geometry and Topology in Hamiltonian Dynamics and Statistical Mechanics; IAM Series n.33; Springer: New York, NY, USA, 2007. [Google Scholar]
- Caiani, L.; Casetti, L.; Clementi, C.; Pettini, M. Geometry of Dynamics, Lyapunov Exponents, and Phase Transitions. Phys. Rev. Lett. 1997, 79, 4361. [Google Scholar] [CrossRef]
- Cerruti-Sola, M.; Clementi, C.; Pettini, M. Hamiltonian dynamics and geometry of phase transitions in classical XY models. Phys. Rev. E 2000, 61, 5171. [Google Scholar] [CrossRef] [PubMed]
- Caiani, L.; Casetti, L.; Clementi, C.; Pettini, G.; Pettini, M.; Gatto, R. Geometry of dynamics and phase transitions in classical lattice ϕ4 theories. Phys. Rev. E 1998, 57, 3886. [Google Scholar] [CrossRef]
- Casetti, L.; Pettini, M.; Cohen, E.G.D. Geometric approach to Hamiltonian dynamics and statistical mechanics. Phys. Rep. 2000, 337, 237–342. [Google Scholar] [CrossRef]
- Franzosi, R.; Pettini, M. Theorem on the origin of Phase Transitions. Phys. Rev. Lett. 2004, 92, 060601. [Google Scholar] [CrossRef]
- Franzosi, R.; Pettini, M.; Spinelli, L. Topology and Phase Transitions I. Preliminary results. Nucl. Phys. B 2007, 782, 189. [Google Scholar] [CrossRef]
- Franzosi, R.; Pettini, M. Topology and Phase Transitions II. Theorem on a necessary relation. Nucl. Phys. B 2007, 782, 219. [Google Scholar] [CrossRef][Green Version]
- Kastner, M.; Mehta, D. Phase Transitions Detached from Stationary Points of the Energy Landscape. Phys. Rev. Lett. 2011, 107, 160602. [Google Scholar] [CrossRef] [PubMed]
- Gori, M.; Franzosi, R.; Pettini, M. Topological origin of phase transitions in the absence of critical points of the energy landscape. J. Stat. Mech. 2018, 2018, 093204. [Google Scholar] [CrossRef]
- Gori, M.; Franzosi, R.; Pettini, M. Topological theory of phase transitions. in preparation.
- Yang, C.N.; Lee, T.D. Statistical theory of equations of state and phase transitions I. Theory of condensation. Phys. Rev. 1952, 87, 404–409. [Google Scholar] [CrossRef]
- Lee, T.D.; Yang, C.N. Statistical theory of equations of state and phase transitions II. Lattice gas and Ising model. Phys. Rev. 1952, 87, 410–419. [Google Scholar] [CrossRef]
- Georgii, H.O. A comprehensive account of the Dobrushin-Lanford-Ruelle theory and of its developments. In Gibbs Measures and Phase Transitions, 2nd ed.; De Gruyter: Berlin, Germany, 2011. [Google Scholar]
- Gross, D.H.E. Microcanonical Thermodynamics. Phase Transitions in “Small” Systems; World Scientific: Singapore, 2001. [Google Scholar]
- Bachmann, M. Thermodynamics and Statistical Mechanics of Macromolecular Systems; Cambridge University Press: New York, NY, USA, 2014. [Google Scholar]
- Qi, K.; Bachmann, M. Classification of Phase Transitions by Microcanonical Inflection-Point Analysis. Phys. Rev. Lett. 2018, 120, 180601. [Google Scholar] [CrossRef]
- Kurian, P.; Capolupo, A.; Craddock, T.J.A.; Vitiello, G. Water-mediated correlations in DNA-enzyme interactions. Phys. Lett. A 2018, 382, 33. [Google Scholar] [CrossRef]
- Pettini, G.; Gori, M.; Franzosi, R.; Clementi, C.; Pettini, M. On the origin of phase transitions in the absence of symmetry-breaking. Physica A 2018, 516, 376. [Google Scholar] [CrossRef]
- Bel-Hadj-Aissa, G.; Gori, M.; Franzosi, R.; Pettini, M. Geometrical and topological study of the Kosterlitz–Thouless phase transition in the XY model in two dimensions. J. Stat. Mech. 2021, 2021, 023206. [Google Scholar] [CrossRef]
- Rugh, H.H. Dynamical approach to temperature. Phys. Rev. Lett. 1997, 78, 772. [Google Scholar] [CrossRef]
- Rugh, H.H. A geometric, dynamical approach to thermodynamics. J. Phys. A 1998, 31, 7761. [Google Scholar] [CrossRef]
- Rugh, H.H. Microthermodynamic formalism. Phys. Rev. E 2001, 64, 055101. [Google Scholar] [CrossRef]
- Franzosi, R. Microcanonical entropy for classical systems. Physica A 2018, 494, 302. [Google Scholar] [CrossRef]
- Franzosi, R. A microcanonical entropy correcting finite-size effects in small systems. J. Stat. Mech. 2019, 8, 083204. [Google Scholar] [CrossRef]
- Zhou, Y. A simple formula for scalar curvature of level sets in euclidean spaces. arXiv 2013, arXiv:1301.2202. [Google Scholar]
- Hirsch, M.W. Differential Topology; Springer: New York, NY, USA, 2012; Volume 33. [Google Scholar]
- Gromov, M. Four lectures on scalar curvature. arXiv 2019, arXiv:1908.10612. [Google Scholar]
- Polyanin, A.D.; Zaitsev, V.F. Handbook of Exact Solutions for Ordinary Differential Equations, 2nd ed.; Chapman and Hall/CRC: Boca Raton, FL, USA, 2003. [Google Scholar]
- Petersen, P. Riemannian Geometry; Springer: New York, NY, USA, 2006. [Google Scholar]
- Bel-Hadj-Aissa, G.; Gori, M.; Penna, V.; Pettini, G.; Franzosi, R. Geometrical Aspects in the Analysis of Microcanonical Phase-Transitions. Entropy 2020, 22, 380. [Google Scholar] [CrossRef] [PubMed]
- Chern, S.S.; Lashof, R.K. On the total curvature of immersed manifolds. Am. J. Math. 1957, 79, 306–318. [Google Scholar] [CrossRef]
- Casetti, L. Efficient symplectic algorithms for numerical simulations of Hamiltonian flows. Phys. Scr. 1995, 51, 29. [Google Scholar] [CrossRef]
- Fehlberg, E. Low-Order Classical Runge–Kutta Formulas with Stepsize Control and Their Application to Some Heat Transfer Problems; National Aeronautics and Space Administration: Pasadena, CA, USA, 1969; Volume 315. [Google Scholar]
- Shampine, L.F.; Watts, H.A.; Davenport, S.M. Solving non-stiff ordinary differential equations: The state of the art. SIAM Rev. 1976, 18, 376–411. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Di Cairano, L.; Gori, M.; Pettini, M. Topology and Phase Transitions: A First Analytical Step towards the Definition of Sufficient Conditions. Entropy 2021, 23, 1414. https://doi.org/10.3390/e23111414
Di Cairano L, Gori M, Pettini M. Topology and Phase Transitions: A First Analytical Step towards the Definition of Sufficient Conditions. Entropy. 2021; 23(11):1414. https://doi.org/10.3390/e23111414
Chicago/Turabian StyleDi Cairano, Loris, Matteo Gori, and Marco Pettini. 2021. "Topology and Phase Transitions: A First Analytical Step towards the Definition of Sufficient Conditions" Entropy 23, no. 11: 1414. https://doi.org/10.3390/e23111414
APA StyleDi Cairano, L., Gori, M., & Pettini, M. (2021). Topology and Phase Transitions: A First Analytical Step towards the Definition of Sufficient Conditions. Entropy, 23(11), 1414. https://doi.org/10.3390/e23111414





