Minimum Entropy Production Effect on a Quantum Scale
Abstract
:1. Introduction
2. Historical Considerations
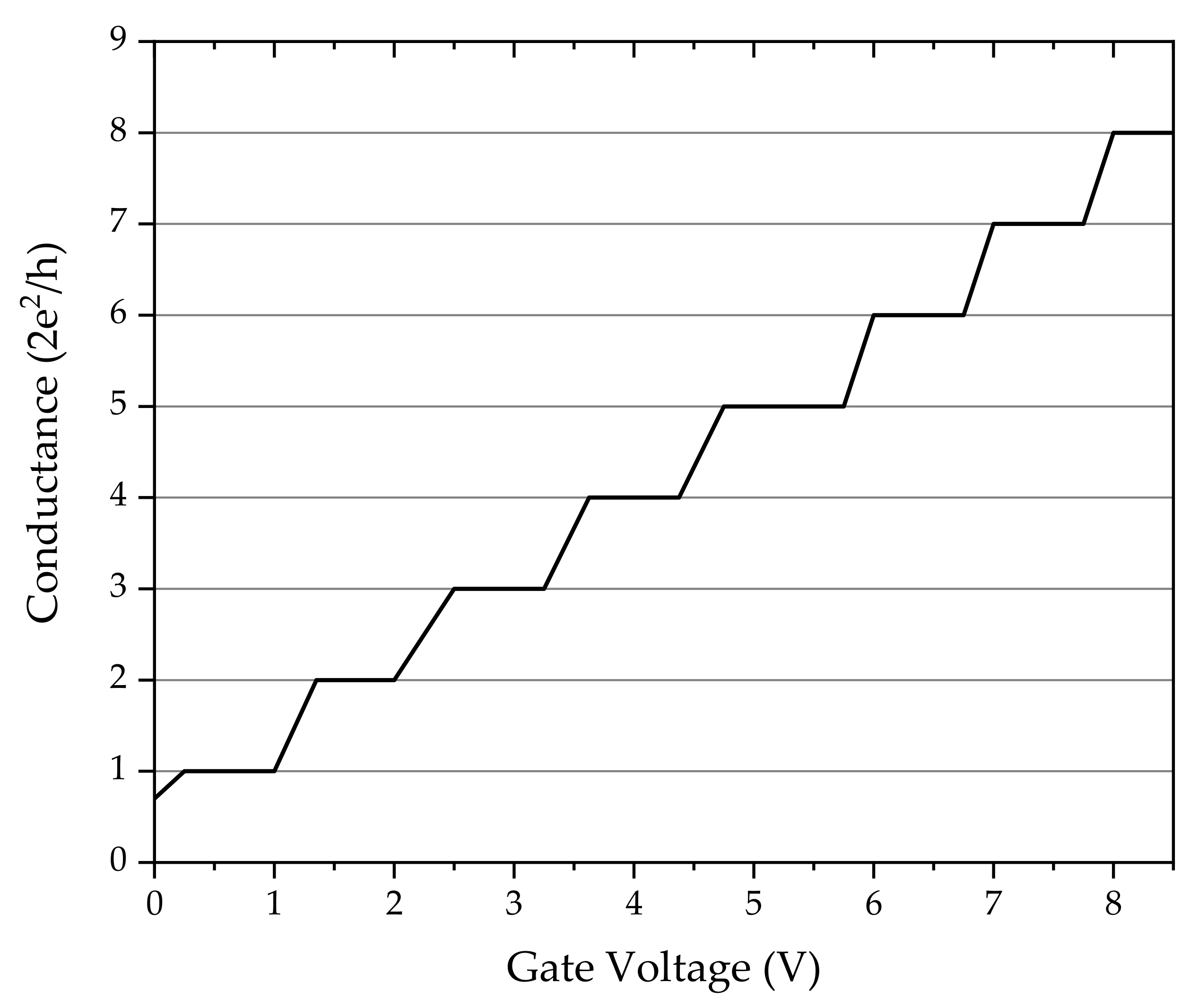
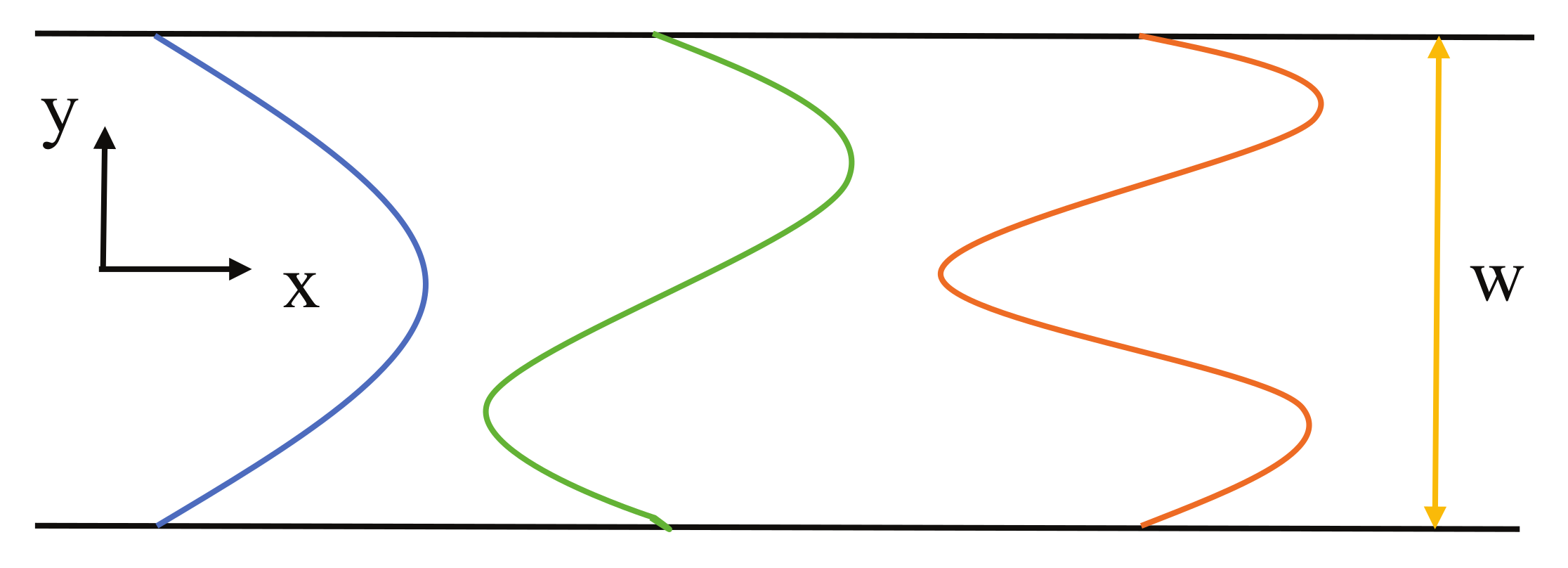
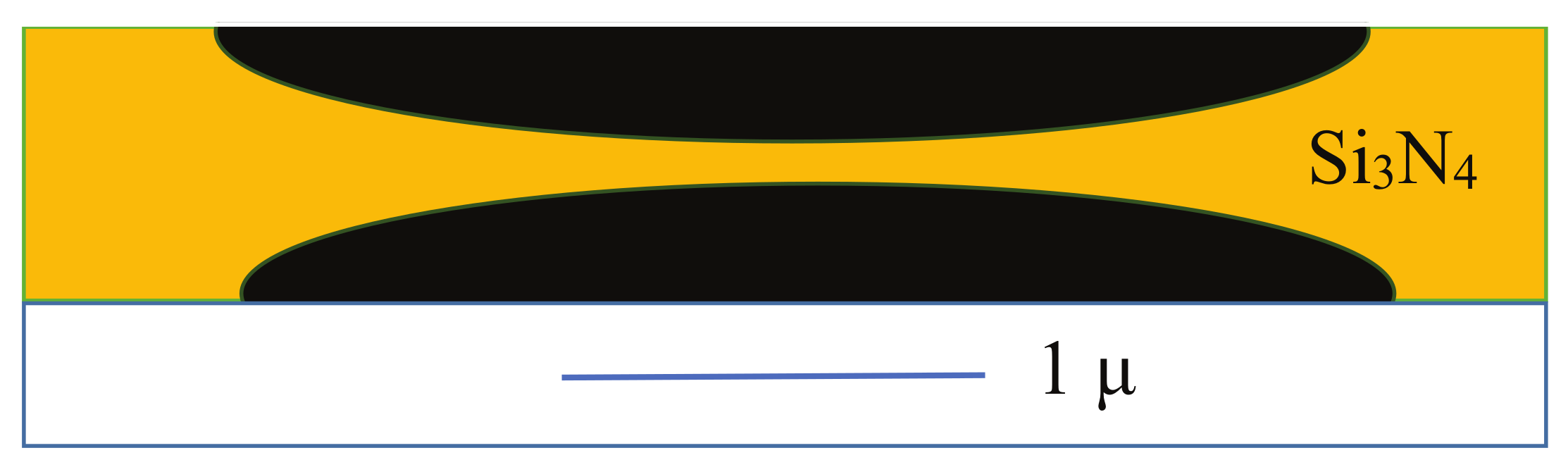
2.1. The Quantized Electric Conductance
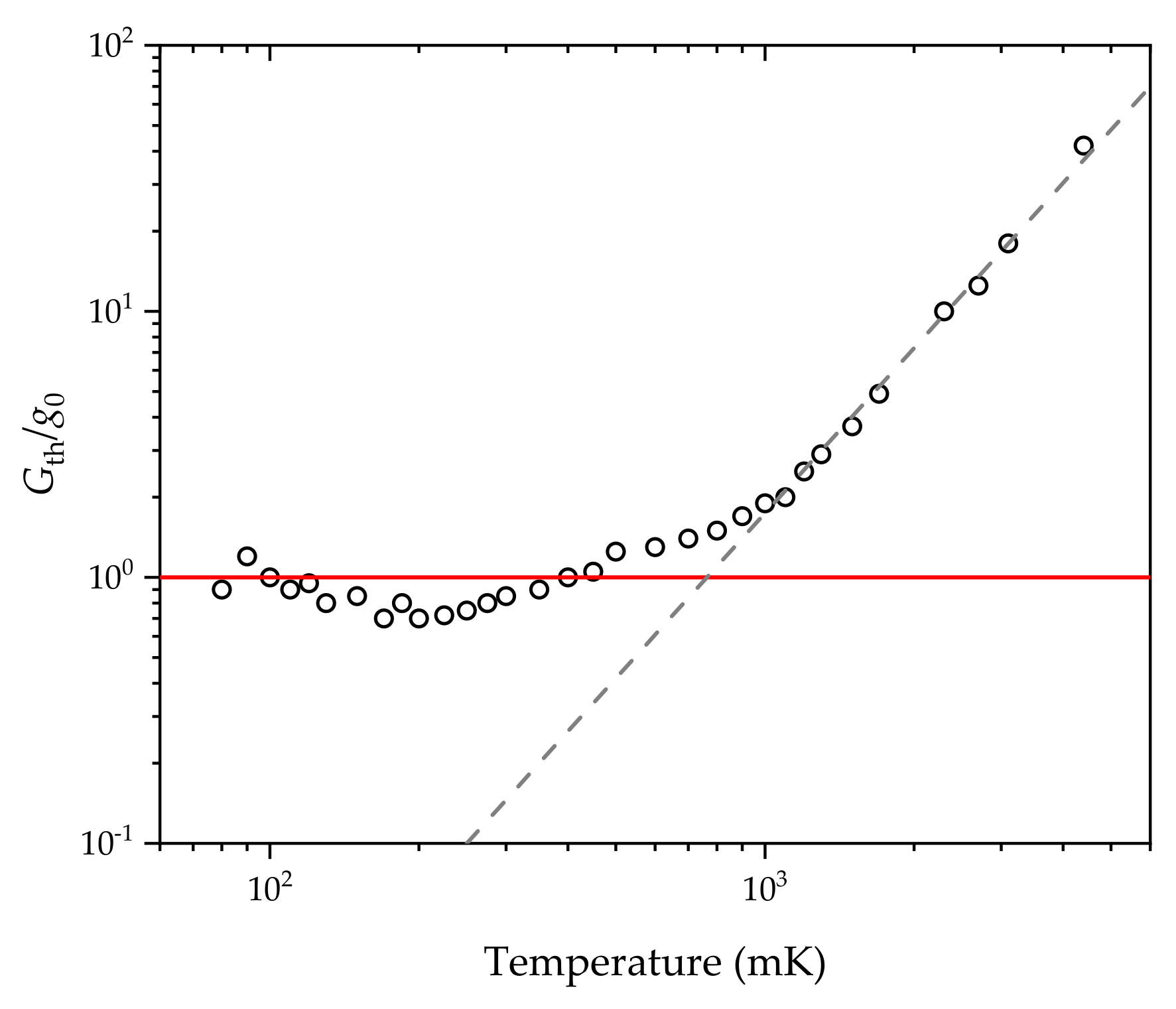
2.2. The Quantized Thermal Conductance
2.3. Lagrangian Description of Heat Conduction
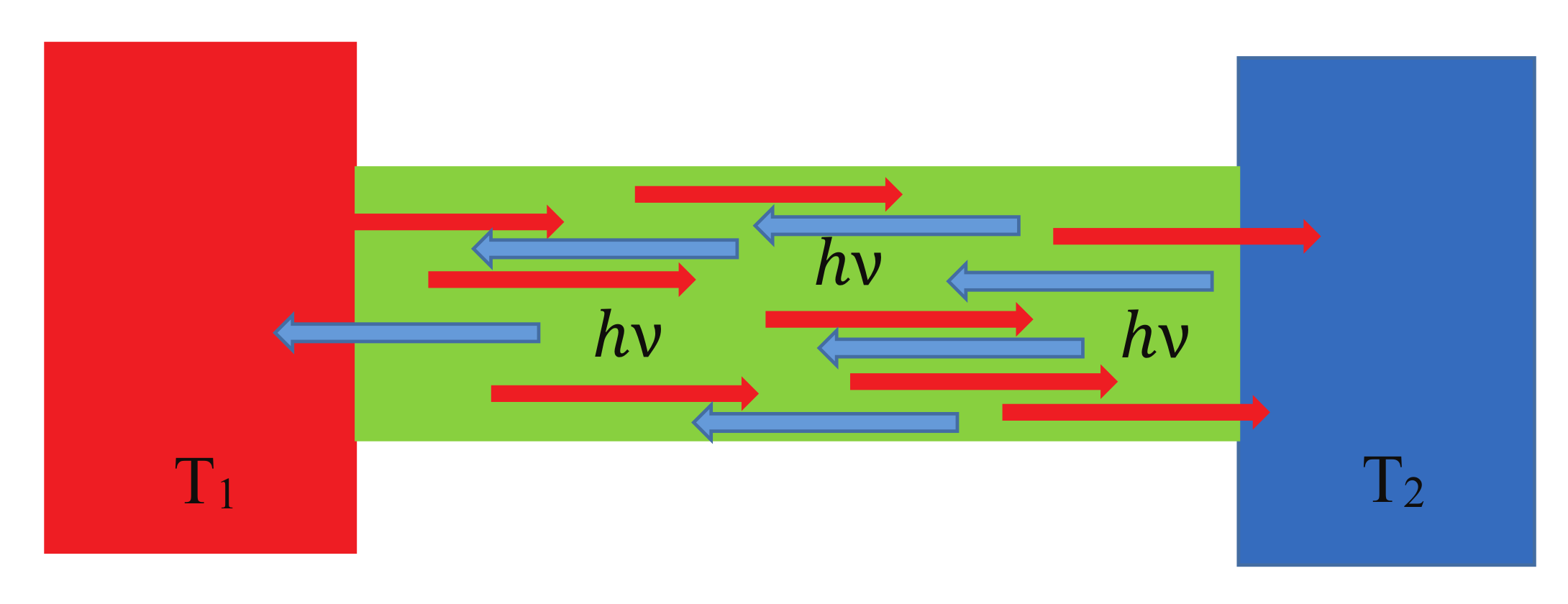
3. The Quantized Behavior of the Conductance of Entropy Current and the Entropy Production
4. Examples and Applications
4.1. Entropy Change during a Single Quantum Transfer
4.2. Spin-Lattice Relaxation
5. An Additional Consequence of the Least Action Principle
6. Summary
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Van Wees, B.J.; van Houten, H.; Beenakker, C.W.J.; Williamson, J.G.; Kouwenhoven, L.P.; van der Marel, D.; Foxon, C.T. Quantized conductance of point contacts in a two-dimensional electron gas. Phys. Rev. Lett. 1988, 60, 848–850. [Google Scholar] [CrossRef] [Green Version]
- Schwab, K.; Henriksen, E.A.; Worlock, J.M.; Roukes, M.L. Measurement of the quantum of thermal conductance. Nature 2000, 404, 974–977. [Google Scholar] [CrossRef] [PubMed]
- Schwab, K.; Arlett, J.L.; Worlock, J.M.; Roukes, M.L. Thermal conductance through discrete quantum channels. Physica E 2001, 9, 60–68. [Google Scholar] [CrossRef]
- Schwab, K. Information on heat. Nature 2006, 444, 161–162. [Google Scholar] [CrossRef] [PubMed]
- Von Klitzing, K.; Dorda, G.; Pepper, M. New method for high-accuracy determination of the fine-structure constant based on quantized hall resistance. Phys. Rev. Lett. 1980, 45, 494–497. [Google Scholar] [CrossRef] [Green Version]
- Tsui, D.C.; Stormer, H.L.; Gossard, A.C. Two-dimensional magnetotransport in the extreme quantum limit. Phys. Rev. Lett. 1982, 48, 1559–1562. [Google Scholar] [CrossRef] [Green Version]
- Laughlin, R.B. Anomalous quantum Hall effect: An incompressible quantum fluid with fractionally charged excitations. Phys. Rev. Lett. 1983, 50, 1395–1398. [Google Scholar] [CrossRef] [Green Version]
- Gulácsi, B.; Dóra, B. Quantum spin Hall insulator interacting with quantum light: Inhomogeneous Dicke model. Phys. Stat. Sol. B 2016, 253, 2468–2472. [Google Scholar] [CrossRef]
- Dóra, B.; Simon, F. Unusual spin dynamics in topological insulators. Sci. Rep. 2015, 5, 14844. [Google Scholar] [CrossRef] [Green Version]
- Dóra, B.; Halbritter, A. Temperature-dependent conductance of deformable molecular devices. Phys.Rev. B 2009, 80, 155402. [Google Scholar] [CrossRef]
- Geresdi, A.; Halbritter, A.; Gyenis, A.; Makk, P.; Mihály, G. From stochastic single atomic switch to nanoscale resistive memory device. Nanoscale 2011, 3, 1504–1507. [Google Scholar] [CrossRef] [Green Version]
- Geresdi, A.; Csontos, M.; Gubicza, Á.; Halbritter, A.; Mihály, G. A fast operation of nanometer-scale metallic memristors: Highly transparent conductance channels in Ag2S devices. Nanoscale 2014, 6, 2613–2617. [Google Scholar] [CrossRef] [Green Version]
- Landauer, R. Spatial variation of currents and fields due to localized scatterers in metallic conduction. IBM J. Res. Dev. 1957, 1, 223–231. [Google Scholar] [CrossRef]
- Landauer, R. Can a length of perfect conductor have a resistance? Phys. Lett. A 1981, 85, 91–93. [Google Scholar] [CrossRef]
- Landauer, R. Conductance determined by transmission: Probes and quantised constriction resistance. J. Phys. Cond. Matter 1989, 1, 8099–8110. [Google Scholar] [CrossRef]
- Nawrocki, W. Electrical and thermal conductance quantization in nanostructure. J. Physics Conf. Ser. 2008, 129, 012023. [Google Scholar] [CrossRef]
- Pendry, J.B. Quantum limits to the flow of information and entropy. J. Phys. A Math. Gen. 1983, 16, 2161–2171. [Google Scholar] [CrossRef] [Green Version]
- Rego, L.G.C.; Kirczenow, G. Quantized thermal conductance of dielectric quantum wires. Phys. Rev. Lett. 1998, 81, 232–235. [Google Scholar] [CrossRef] [Green Version]
- Angelescu, D.E.; Cross, M.C.; Roukes, M.L. Heat transport in mesoscopic systems. Superlatt. Microstruct. 1998, 23, 673–689. [Google Scholar] [CrossRef] [Green Version]
- Nishiguchi, N.; Ando, Y.; Wybourne, M.N. Acoustic phonon modes of rectangular quantum wires. J. Phys. Cond. Matter 1997, 9, 5751–5764. [Google Scholar] [CrossRef]
- Blencowe, M.P. Quantum energy flow in mesoscopic dielectric structures. Phys. Rev. B 1999, 59, 4992–4998. [Google Scholar] [CrossRef] [Green Version]
- Blencowe, M.P. Quantum electromechanical systems. Phys. Rep. 2004, 395, 159–222. [Google Scholar] [CrossRef]
- Li, W.-X.; Chen, K.-Q.; Duan, W.; Du, J.; Gu, B.-L. Phonon transport and thermal conductivity in dielectric quantum wire. J. Phys. D Appl. Phys. 2003, 36, 3027–3033. [Google Scholar] [CrossRef]
- Sólyom, J. Fundamentals of the Physics of Solids Vol. II: Electronic Properties; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Márkus, F.; Gambár, K. A variational principle in thermodynamics. J. Non-Equilib. Thermodyn. 1991, 16, 27–31. [Google Scholar] [CrossRef]
- Gambár, K.; Márkus, F. Hamilton–Lagrange formalism of nonequilibrium thermodynamics. Phys. Rev. E 1994, 50, 1227–1231. [Google Scholar] [CrossRef]
- Gambár, K.; Lendvay, M.; Lovassy, R.; Bugyjás, J. Application of potentials in the description of transport processes. Acta Polytechn. Hung. 2016, 13, 173–184. [Google Scholar]
- Szegleti, A.; Márkus, F. Dissipation in Lagrangian Formalism. Entropy 2020, 22, 930. [Google Scholar] [CrossRef]
- Márkus, F.; Gambár, K. Temperature and entropy production operator in Fourier heat conduction. Phys. Rev. E 1995, 52, 623–626. [Google Scholar] [CrossRef]
- Vázquez, F.; Márkus, F.; Gambár, K. Quantized heat transport in small systems: A phenomenological approach. Phys. Rev. E 2009, 79, 031113. [Google Scholar] [CrossRef]
- de Groot, S.R.; Mazur, P. Non-Equilibrium Thermodynamics; North-Holland: Amsterdam, The Netherlands, 1962. [Google Scholar]
- Lebon, G.; Jou, D.; Casas-Vázquez, J. Understanding Non-Equilibrium Thermodynamics; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Sieniutycz, S. Conservation Laws in Variational Thermo-Hydrodynamics; Springer: Dordrecht, The Netherlands, 1994. [Google Scholar]
- Qin, H.; Holländer, R.B.; Flajšman, L.; Hermann, F.; Dreyer, R.; Woltersdorf, G.; van Dijken, S. Nanoscale magnonic Fabry-Pérot resonator for low-loss spin-wave manipulation. Nat. Commun. 2021, 12, 2293–2302. [Google Scholar] [CrossRef]
- Csosz, G.; Dóra, B.; Simon, F. Entropy in spin relaxation, spintronics, and magnetic resonance. Phys. Stat. Sol. B 2020, 257, 2000301. [Google Scholar] [CrossRef]
- Palma, G.M.; Suominen, K.-A.; Ekert, A.K. Quantum Computers and Dissipation. Proc. R. Soc. A 1996, 452, 567–584. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Márkus, F.; Gambár, K. Minimum Entropy Production Effect on a Quantum Scale. Entropy 2021, 23, 1350. https://doi.org/10.3390/e23101350
Márkus F, Gambár K. Minimum Entropy Production Effect on a Quantum Scale. Entropy. 2021; 23(10):1350. https://doi.org/10.3390/e23101350
Chicago/Turabian StyleMárkus, Ferenc, and Katalin Gambár. 2021. "Minimum Entropy Production Effect on a Quantum Scale" Entropy 23, no. 10: 1350. https://doi.org/10.3390/e23101350
APA StyleMárkus, F., & Gambár, K. (2021). Minimum Entropy Production Effect on a Quantum Scale. Entropy, 23(10), 1350. https://doi.org/10.3390/e23101350




