A Continuous-Time Random Walk Extension of the Gillis Model
Abstract
1. Introduction
2. Review of Previous Work
2.1. Gillis Random Walk
2.2. CTRW
3. Results
3.1. Probability of Being at the Origin
3.1.1. Gillis Way
- is the probability of being (arriving) at j at (within) time t;
- is the probability of arriving at j at time t.
3.1.2. Recurrence Relation: First-Return Time to the Origin
3.1.3. Finite-Mean Waiting-Time Distributions
3.1.4. Infinite-Mean Waiting-Time Distributions
3.2. Survival Probability on the Positive Semi-Axis
3.3. Occupation Times
3.3.1. Occupation Time of the Origin
3.3.2. Occupation Time of the Positive Semi-Axis
3.4. Moments Spectrum
3.5. Statistics of Records
4. Numerical Results
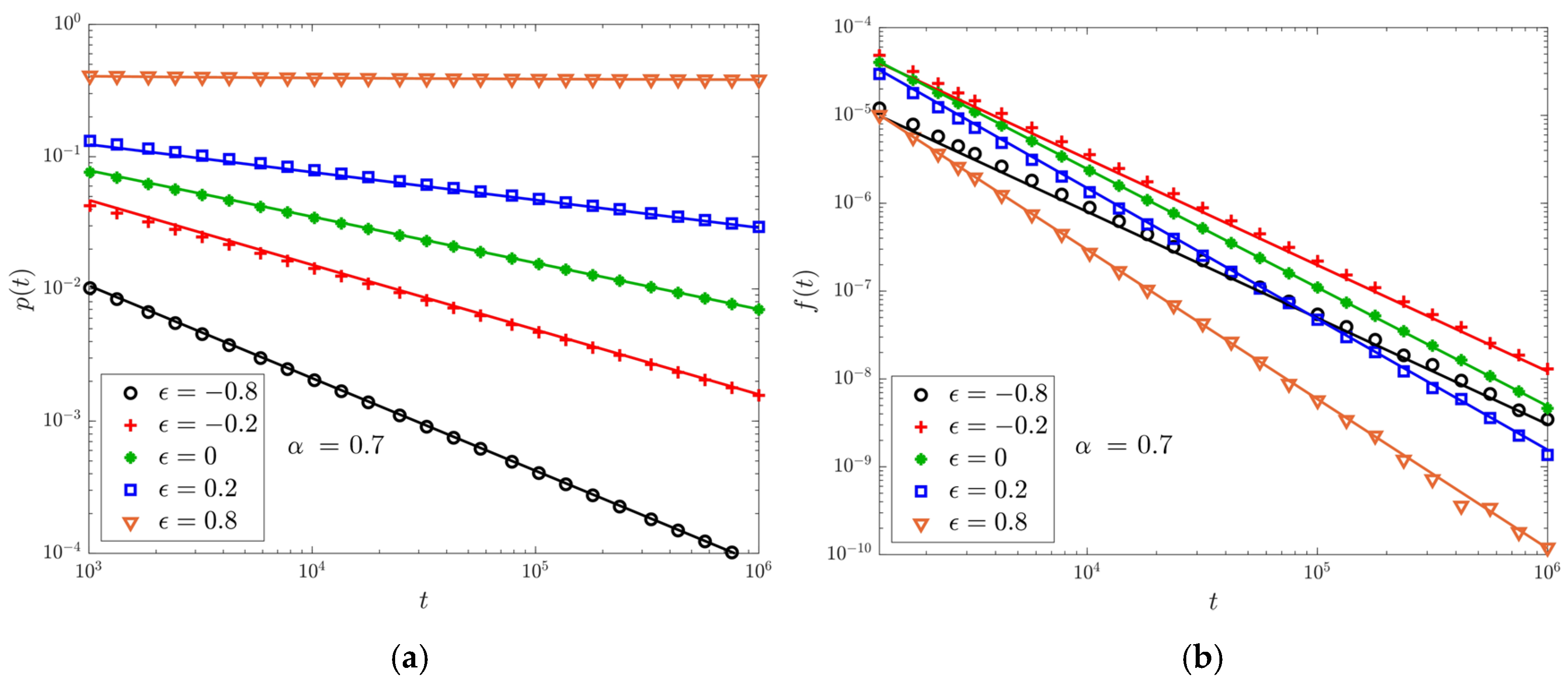
4.1. Return and First-Return Events
4.2. Occupation Times
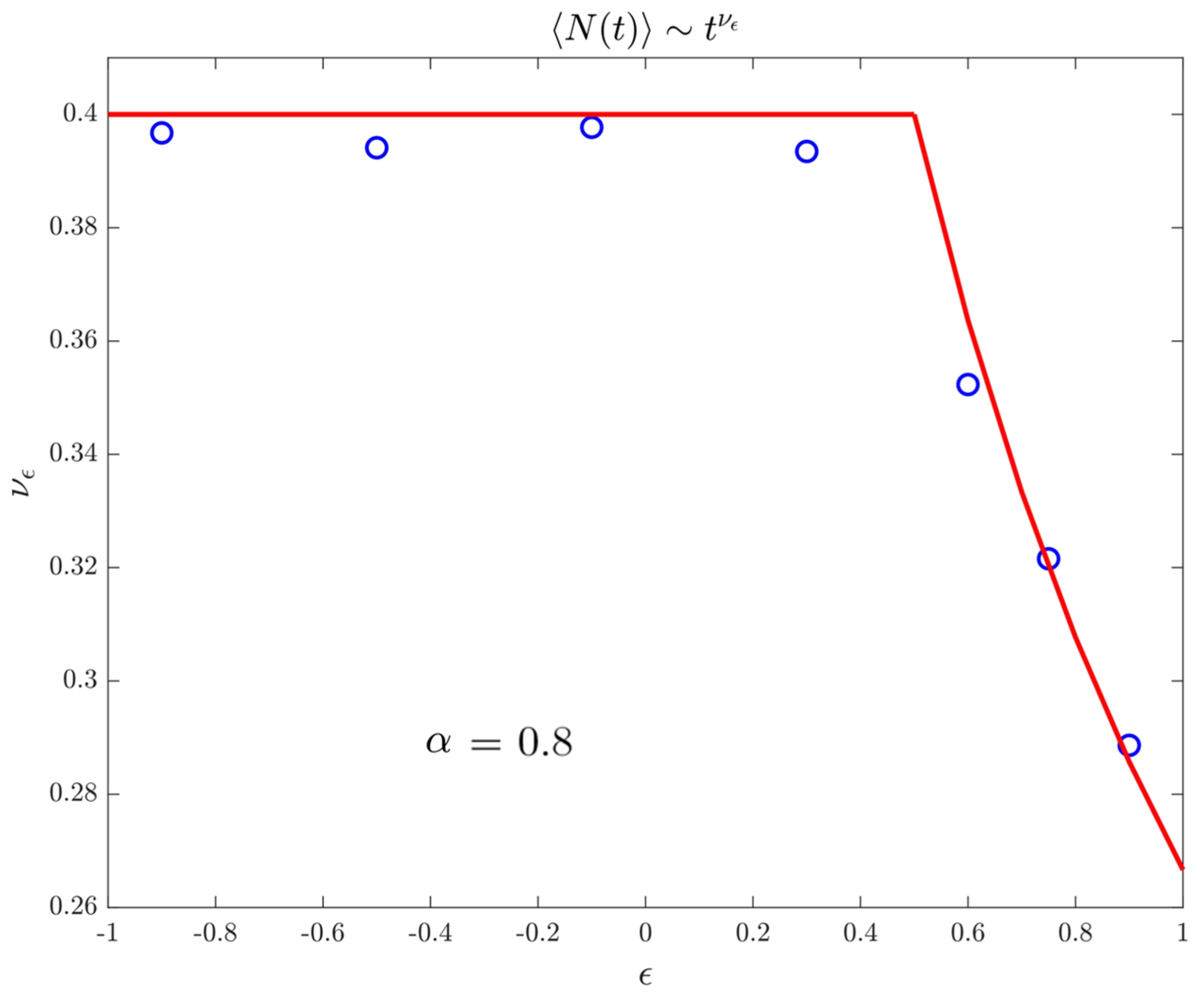
4.3. Moments Spectrum
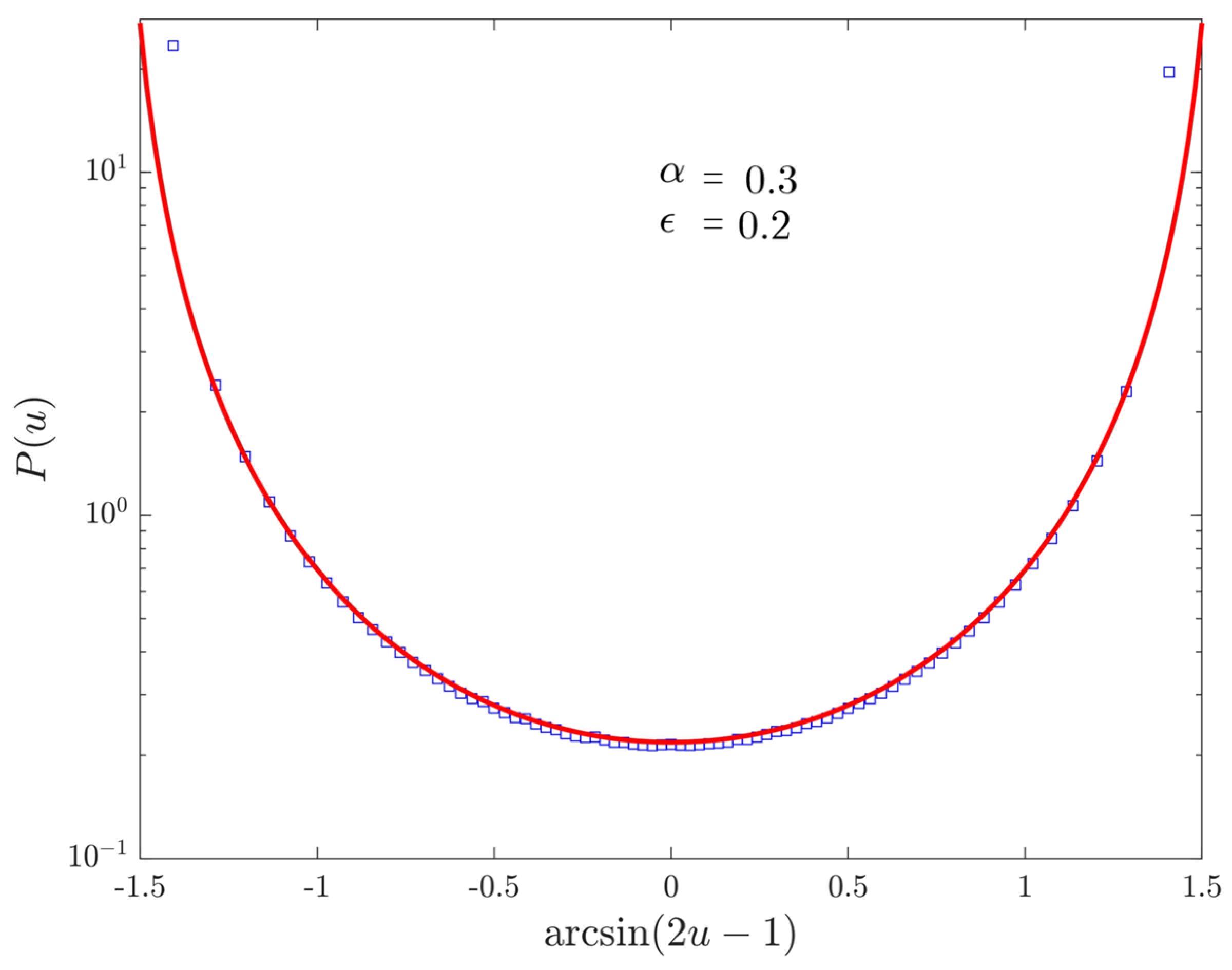
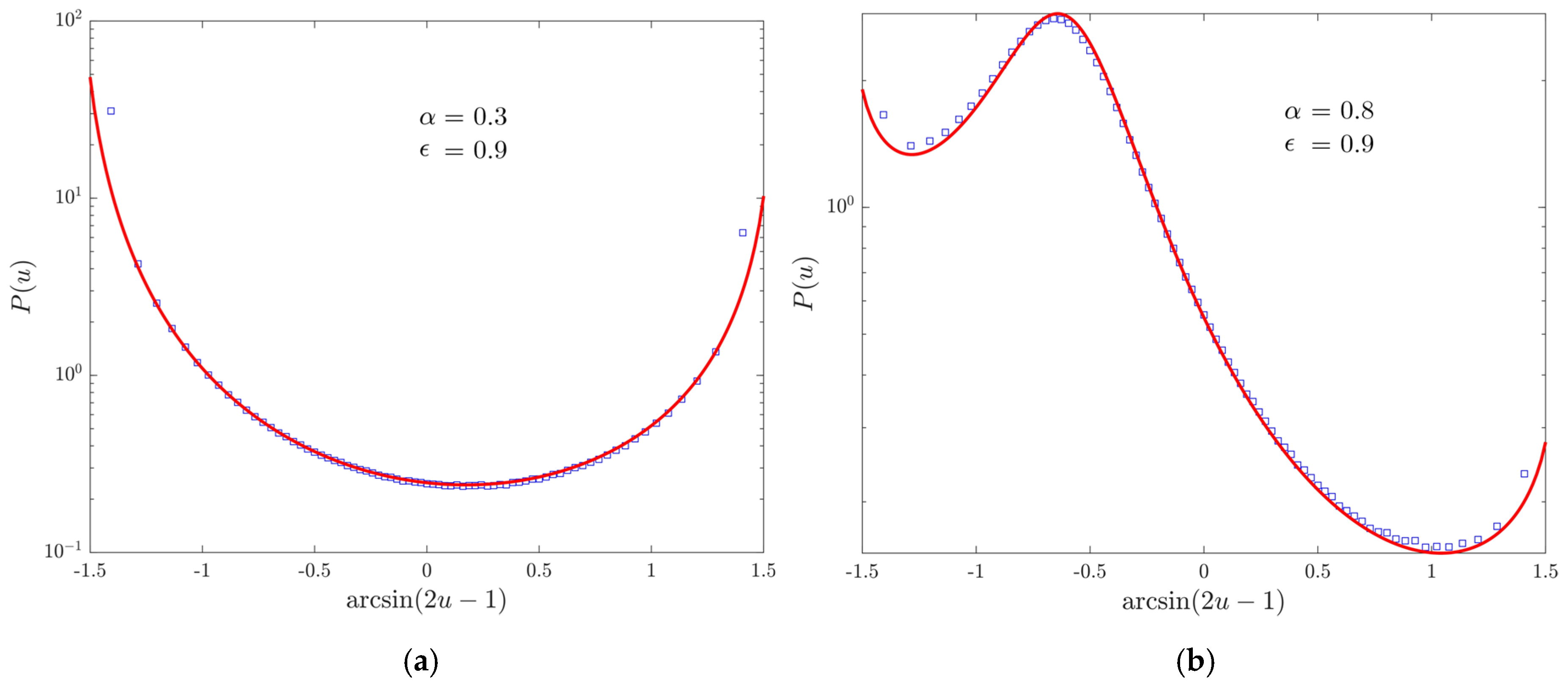
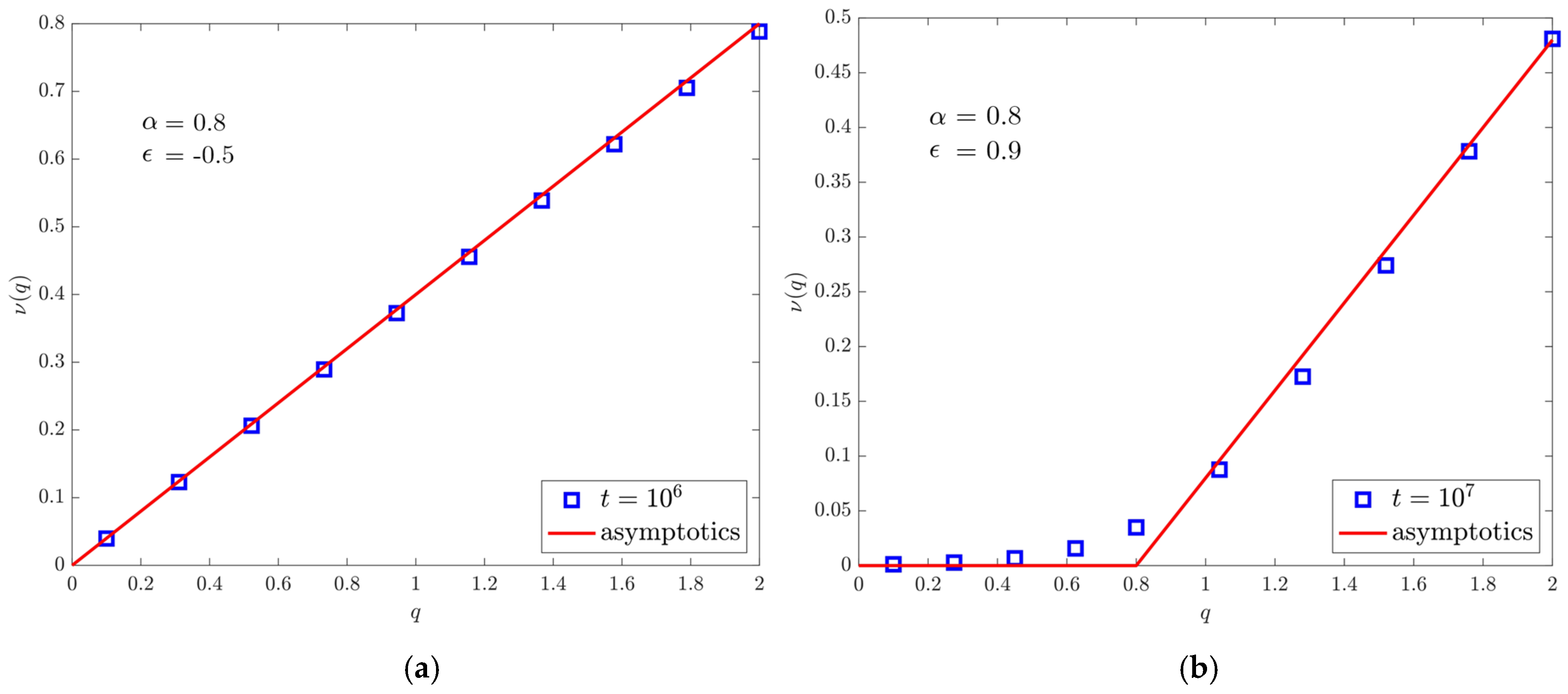
4.4. Records
5. Discussion
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| CTRW | Continuous Time Random Walk |
| Probability Density Function | |
| i.i.d. | Independent Identically Distributed |
Appendix A. Gillis-Type Proof
Appendix B. Hitting Time PDF of the Origin: Exact Results
Appendix C. First-Hitting Time PDF: Exact Results
Appendix C.1. First-Return
Appendix C.2. First-Hitting
Appendix D. CTRW on
References
- Hughes, B.D. Random Walks and Random Environments. Volume I: Random Walks; Clarendon Press: Oxford, UK, 1995. [Google Scholar]
- Menshikov, M.; Popov, S.; Wade, A. Non-Homogeneous Random Walks. Lyapunov Function Methods for Near-Critical Stochastic Systems; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Klages, R.; Radons, G.; Sokolov, I.M. Anomalous Transport: Foundations and Applications; Wiley–VHC: Berlin, Germany, 2008. [Google Scholar]
- Bouchaud, J.P.; Georges, A. Anomalous diffusion in disordered media: Statistical mechanisms, models and physical applications. Phys. Rep. 1990, 195, 127–293. [Google Scholar] [CrossRef]
- Barthelemy, P.; Bertolotti, J.; Wiersma, D.S. A Lévy flight for light. Nature 2008, 453, 495–498. [Google Scholar] [CrossRef] [PubMed]
- Dentz, M.; Cortis, A.; Scher, H.; Berkowitz, B. Time behavior of solute transport in heterogeneous media: Transition from anomalous to normal transport. Adv. Water Resour. 2004, 27, 155–173. [Google Scholar] [CrossRef]
- Cortis, A.; Berkowitz, B. Anomalous transport in “classical” soil and sand columns. Soil Sci. Soc. Am. J. 2004, 68, 1539–1548. [Google Scholar] [CrossRef]
- Kühn, T.; Ihalainen, T.O.; Hyväluoma, J.; Dross, N.; Willman, S.F.; Langowski, J.; Vihinen-Ranta, M.; Timonen, J. Protein Diffusion in Mammalian Cell Cytoplasm. PLoS ONE 2011, 6, e22962. [Google Scholar] [CrossRef]
- Nissan, A.; Berkowitz, B. Inertial Effects on Flow and Transport in Heterogeneous Porous Media. Phys. Rev. Lett. 2018, 120, 054504. [Google Scholar] [CrossRef]
- Barkai, E.; Garini, Y.; Metzler, R. Strange kinetics of single molecules in living cells. Phys. Today 2012, 65, 29–35. [Google Scholar] [CrossRef]
- Metzler, R.; Jeon, J.H.; Cherstvy, A.G.; Barkai, E. Anomalous diffusion models and their properties: Non-stationarity, non-ergodicity, and ageing at the centenary of single particle tracking. Phys. Chem. Chem. Phys. 2014, 16, 24128–24164. [Google Scholar] [CrossRef]
- He, Y.; Burov, S.; Metzler, R.; Barkai, E. Random Time-Scale Invariant Diffusion and Transport Coefficients. Phys. Rev. Lett. 2008, 101, 058101. [Google Scholar] [CrossRef]
- Burov, S.; Jeon, J.H.; Metzler, R.; Barkai, E. Single particle tracking in systems showing anomalous diffusion: The role of weak ergodicity breaking. Phys. Chem. Chem. Phys. 2010, 13, 1800–1812. [Google Scholar] [CrossRef]
- Gillis, J. Centrally biased discrete random walk. Q. J. Math. 1956, 7, 144–152. [Google Scholar]
- Percus, O.E. Phase transition in one-dimensional random walk with partially reflecting boundaries. Adv. Appl. Probab. 1985, 17, 594–606. [Google Scholar] [CrossRef]
- Montroll, E.W. Random Walks on Lattices. III. Calculation of First-Passage Times with Application to Exciton Trapping on Photosynthetic Units. J. Math. Phys. 1969, 10, 153–165. [Google Scholar] [CrossRef]
- Hill, J.M.; Gulati, C.M. The random walk associated by the game of roulette. J. Appl. Probab. 1981, 18, 931–936. [Google Scholar] [CrossRef]
- Hughes, B.D.; Sahimi, M. Random walks on the Bethe lattice. J. Stat. Phys. 1982, 23, 1688–1692. [Google Scholar] [CrossRef]
- Zaburdaev, V.; Denisov, S.; Klafter, J. Lévy walks. Rev. Mod. Phys. 2015, 87, 483. [Google Scholar] [CrossRef]
- Shlesinger, M.F.; Klafter, J. Lévy Walks Versus Lévy Flights. In On Growth and Form. Fractal and Non–Fractal Patterns in Physics; Stanley, H.E., Ostrowsky, N., Eds.; NATO ASI Series E: Applied Sciences-No. 100; Martinus Nijhoff Publihers: Dordrecht, The Netherlands; Boston, MA, USA; Lancaster, UK, 1986; pp. 279–283. [Google Scholar]
- Montroll, E.W.; Weiss, G.H. Random walks on lattices, II. J. Math. Phys. 1965, 6, 167–181. [Google Scholar] [CrossRef]
- Klafter, J.; Sokolov, I.M. First Steps in Random Walks. From Tools to Applications; Oxford University Press Inc.: New York, NY, USA, 2011; pp. 1–51. [Google Scholar]
- Scalas, E. The application of continuous-time random walks in finance and economics. Physica A 2006, 362, 225–239. [Google Scholar] [CrossRef]
- Wolfgang, P.; Baschnagel, J. Stochastic Processes. From Physics to Finance; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Scher, H.; Shlesinger, M.F.; Bendler, J.T. Time-scale invariance in transport and relaxation. Phys. Today 1991, 44, 26–34. [Google Scholar] [CrossRef]
- Berkowitz, B.; Cortis, A.; Dentz, M.; Scher, H. Modeling non-Fickian transport in geological formations as a continuous time random walk. Rev. Geophys. 2006, 44, RG2003. [Google Scholar] [CrossRef]
- Boano, F.; Packman, A.I.; Cortis, A.; Ridolfi, R.R.L. A continuous time random walk approach to the stream transport of solutes. Water Resour. Res. 2007, 43, W10425. [Google Scholar] [CrossRef]
- Geiger, S.; Cortis, A.; Birkholzer, J.T. Upscaling solute transport in naturally fractured porous media with the continuous time random walk method. Water Resour. Res. 2010, 46, W12530. [Google Scholar] [CrossRef]
- Onofri, M.; Pozzoli, G.; Radice, M.; Artuso, R. Exploring the Gillis model: a discrete approach to diffusion in logarithmic potentials. J. Stat. Mech. 2020, 2020, 113201. [Google Scholar] [CrossRef]
- Redner, S. A Guide to First-Passage Processes; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions; Dover Publications Inc.: New York, NY, USA, 1970. [Google Scholar]
- Hughes, B.D. On returns to the starting site in lattice random walks. Physica A 1986, 134, 443–457. [Google Scholar] [CrossRef]
- Castiglione, P.; Mazzino, A.; Muratore-Ginanneschi, P.; Vulpiani, A. On strong anomalous diffusion. Physica D 1999, 134, 75–93. [Google Scholar] [CrossRef]
- Hryniv, O.; Menshikov, M.V.; Wade, A.R. Excursions and path functionals for stochastic processes with asymptotically zero drifts. Stoch. Process. Their Appl. 2013, 123, 1891–1921. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Straka, P. Inverse Stable Subordinators. Math. Model. Nat. Phenom. 2013, 8, 1–16. [Google Scholar] [CrossRef]
- Janson, S. Stable Distributions. (Lecture Notes). arXiv 2011, arXiv:1112.0220v2. [Google Scholar]
- Feller, W. An Introduction to Probability Theory and Its Applications; John Wiley and Sons, Inc.: New York, NY, USA, 1971; Volume II. [Google Scholar]
- Sparre Andersen, E. On the fluctuations of sums of random variables II. Math. Scand. 1954, 2, 195–223. [Google Scholar]
- Mounaix, P.; Majumdar, S.N.; Schehr, G. Statistics of the number of records for random walks and Lévy Flights on a 1D Lattice. J. Phys. A Math. Theor. 2020, 53, 415003. [Google Scholar] [CrossRef]
- Feller, W. An Introduction to Probability Theory and Its Applications; John Wiley and Sons, Inc.: New York, NY, USA, 1968; Volume I. [Google Scholar]
- Artuso, R.; Cristadoro, G.; Degli Esposti, M.; Knight, G.S. Sparre-Andersen theorem with spatiotemporal correlations. Phys. Rev. E 2014, 89, 052111. [Google Scholar] [CrossRef] [PubMed]
- Radice, M.; Onofri, M.; Artuso, R.; Pozzoli, G. Statistics of occupation times and connection to local properties of nonhomogeneous random walks. Phys. Rev. E 2020, 101, 042103. [Google Scholar]
- Darling, D.A.; Kac, M. On occupation times for Markoff processes. Trans. Am. Math. Soc. 1957, 84, 444–458. [Google Scholar] [CrossRef]
- Lamperti, J. An occupation time theorem for a class of stochastic processes. Trans. Am. Math. Soc. 1958, 88, 380–387. [Google Scholar] [CrossRef]
- Godrèche, C.; Luck, J.M. Statistics of the occupation time for renewal processes. J. Stat. Phys. 2001, 104, 489–524. [Google Scholar] [CrossRef]
- Barkai, E. Residence time statistics for normal and fractional diffusion in a force field. J. Stat. Phys. 2006, 123, 883–907. [Google Scholar] [CrossRef]
- Bel, G.; Barkai, E. Occupation time and ergodicity breaking in biased continuous time random walks. J. Phys. Condens. Matter 2005, 17, S4287–S4304. [Google Scholar] [CrossRef][Green Version]
- Bel, G.; Barkai, E. Random walk to a nonergodic equilibrium concept. Phys. Rev. E 2006, 73, 16125. [Google Scholar] [CrossRef]
- Bel, G.; Barkai, E. Weak ergodicity breaking in continuous-time random walk. Phys. Rev. Lett. 2005, 94, 240602. [Google Scholar] [CrossRef]
- Widder, D.V. The Laplace Transform; Princeton University Press: London, UK, 1946. [Google Scholar]
- Dechant, A.; Lutz, E.; Barkai, E.; Kessler, D.A. Solution of the Fokker-Planck Equationation with a Logarithmic Potential. J. Stat. Phys. 2011, 145, 1524–1545. [Google Scholar] [CrossRef]
- Lamperti, J. Criteria for the Recurrence or Transience of Stochastic Process I. J. Math. Anal. Appl. 1960, 1, 314–330. [Google Scholar] [CrossRef]
- Lamperti, J. Criteria for Stochastic Processes II: Passage-Time Moments. J. Math. Anal. Appl. 1963, 7, 127–145. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pozzoli, G.; Radice, M.; Onofri, M.; Artuso, R. A Continuous-Time Random Walk Extension of the Gillis Model. Entropy 2020, 22, 1431. https://doi.org/10.3390/e22121431
Pozzoli G, Radice M, Onofri M, Artuso R. A Continuous-Time Random Walk Extension of the Gillis Model. Entropy. 2020; 22(12):1431. https://doi.org/10.3390/e22121431
Chicago/Turabian StylePozzoli, Gaia, Mattia Radice, Manuele Onofri, and Roberto Artuso. 2020. "A Continuous-Time Random Walk Extension of the Gillis Model" Entropy 22, no. 12: 1431. https://doi.org/10.3390/e22121431
APA StylePozzoli, G., Radice, M., Onofri, M., & Artuso, R. (2020). A Continuous-Time Random Walk Extension of the Gillis Model. Entropy, 22(12), 1431. https://doi.org/10.3390/e22121431




