Subshifts on Infinite Alphabets and Their Entropy
Abstract
1. Introduction
2. Symbolic Dynamics on Finite Alphabets
3. Symbolic Dynamics on Infinite Alphabets
4. Entropy Theory
5. Shifts along Infinite (Directed) Graphs
- (i)
- For all , is not a vertex of G. Then , where v runs through the vertices of G, is an open neighborhood of s which does not intersect .
- (ii)
- There exists such that is not an edge of G but is a vertex of G. Then , where y runs through the vertices of G which fulfill the condition that is an edge of G, which is an open neighborhood of s, does not intersect .
- (iii)
- There exists such that is not an edge of G but is a vertex of G. Then , where y runs through the vertices of G which fulfills the condition that is an edge of G, which is an open neighborhood of s, does not intersect . □
6. Entropy Numbers
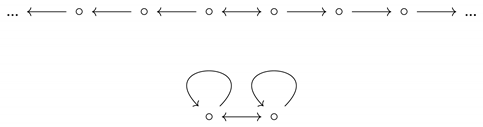
7. Examples

Funding
Conflicts of Interest
References
- Gurevich, B. Topological entropy of enumerable Markov chains. Sov. Math. Dokl. 1969, 10, 911–915. [Google Scholar]
- Petersen, K. Chains, entropy, coding. Ergod. Theory Dyn. Syst. 1986, 6, 415–448. [Google Scholar] [CrossRef]
- Fiebig, D.; Fiebig, U.R. Topological boundaries for countable state Markov shifts. Proc. Lond. Math. Soc. 1995, 70, 625–643. [Google Scholar] [CrossRef]
- Fiebig, D. Factor maps, entropy and fiber cardinality for Markov shifts. Rocky Mt. J. Math. 2001, 31, 955–986. [Google Scholar] [CrossRef]
- Fiebig, D.; Fiebig, U.R. Embedding theorems for locally compact Markov shifts. Ergod. Theory Dyn. Syst. 2005, 25, 107–131. [Google Scholar] [CrossRef]
- Ott, W.; Tomforde, M.; Willis, P. One-sided shift spaces over infinite alphabets. arXiv 2012, arXiv:1209.1760. [Google Scholar]
- Goncalves, D.; Sobottka, M.; Starling, C. Sliding block codes between shift spaces over infinite alphabets. Math. Nachrichten 2016, 289, 2178–2191. [Google Scholar] [CrossRef]
- Goncalves, D.; Sobottka, M.; Starling, C. Two-sided shift spaces over infinite alphabets. J. Aust. Math. Soc. 2017, 103, 357–386. [Google Scholar] [CrossRef]
- Parry, W. Intrinsic Markov chains. Trans. Am. Math. Soc. 1964, 112, 55–66. [Google Scholar] [CrossRef]
- Adler, R.; Konheim, A.; McAndrew, M. Topological entropy. Trans. Am. Math. Soc. 1965, 114, 309–319. [Google Scholar] [CrossRef]
- Hedlund, G. Endomorphisms and automorphisms of the shift dynamical system. Math. Syst. Theory 1969, 3, 320–375. [Google Scholar] [CrossRef]
- Rado, R. Universal graphs and universal functions. Acta Arith. 1964, 9, 331–340. [Google Scholar] [CrossRef]
- Truss, J. The group of the countable universal graph. Math. Proc. Camb. Philos. Soc. 1985, 98, 213–245. [Google Scholar] [CrossRef]
- Mohar, B. The spectrum of an infinite graph. Linear Algebra Its Appl. 1982, 48, 245–256. [Google Scholar] [CrossRef]
- Salama, I. Topological entropy and recurrence of countable chains. Pac. J. Math. 1988, 134, 325–341. [Google Scholar] [CrossRef]
- Lind, D. The entropies of topological Markov shifts and a related class of algebraic integers. Ergod. Theory Dyn. Syst. 1984, 4, 283–300. [Google Scholar] [CrossRef]
- Boyle, M. Symbolic dynamics and matrices. In Combinatorial and Graph-Theoretical Problems in Linear Algebra; Brualdi, R., Ed.; Springer: Berlin/Heidelberg, Germany, 1993; pp. 1–38. [Google Scholar]
- Stevanovich, D. Spectral Radius of Graphs; Elsevier: Amsterdam, The Netherlands, 2014. [Google Scholar]
- Ferenczi, S. Substitution dynamical systems on infinite alphabets. Ann. L’Institut Fourier 2006, 56, 2315–2343. [Google Scholar] [CrossRef]
- Grillenberger, C. Constructions of strictly ergodic systems 1: Positive entropy. Zeitschrift für Wahrscheinlichkeitstheorie und Verwandte Gebiete 1973, 25, 323–334. [Google Scholar] [CrossRef]
- Weiss, B. Subshifts of finite type and sofic systems. Monatshefte für Mathematik 1973, 77, 462–474. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rezagholi, S. Subshifts on Infinite Alphabets and Their Entropy. Entropy 2020, 22, 1293. https://doi.org/10.3390/e22111293
Rezagholi S. Subshifts on Infinite Alphabets and Their Entropy. Entropy. 2020; 22(11):1293. https://doi.org/10.3390/e22111293
Chicago/Turabian StyleRezagholi, Sharwin. 2020. "Subshifts on Infinite Alphabets and Their Entropy" Entropy 22, no. 11: 1293. https://doi.org/10.3390/e22111293
APA StyleRezagholi, S. (2020). Subshifts on Infinite Alphabets and Their Entropy. Entropy, 22(11), 1293. https://doi.org/10.3390/e22111293



