Modeling the Device Behavior of Biological and Synthetic Nanopores with Reduced Models
Abstract
:1. Introduction
1.1. The Device Approach
1.2. Ion Channels and Nanopores as Devices
2. Reduced Models
Our aim with this paper is to illustrate how to accomplish this, with ion channels and nanopores as worked examples.A good reduced model is defined by choosing the important degrees of freedom carefully and constructing sufficiently accurate response functions for the others.
2.1. Ionic Distribution in the Pore as a Determining Factor
| 1. The current carried by an ionic species as a result of a given driving force (conductance) is mainly determined by the axial concentration profile of that species inside the pore. |
2.2. What Determines Local Concentration Inside the Pore?
| 2. We need to build the pore charges into the model properly if we want to reproduce local concentration, and, consequently, device function. |
2.3. Important vs. Unimportant Degrees of Freedom
| 3. Those degrees of freedom are the important ones that depend on the input parameters of the device (voltage and concentration), while those that do not can be replaced by response functions. |
2.4. What Are Good Response Functions?
| 4. When we create a response function, we should choose one whose parameters do not depend on external conditions, or, at least, we should minimize that dependence. In other words, those parameters should be transferable as much as possible. |
3. Case Studies
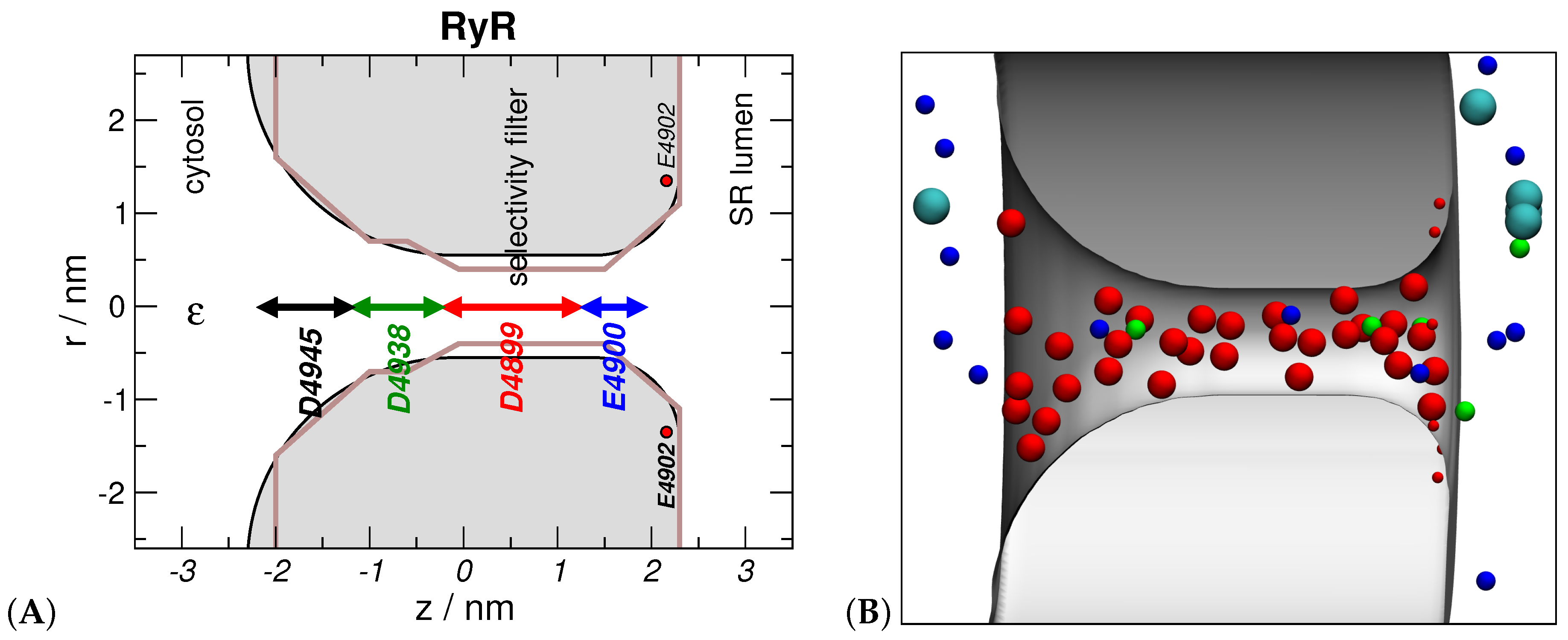
3.1. The Ryanodine Receptor Calcium Channel
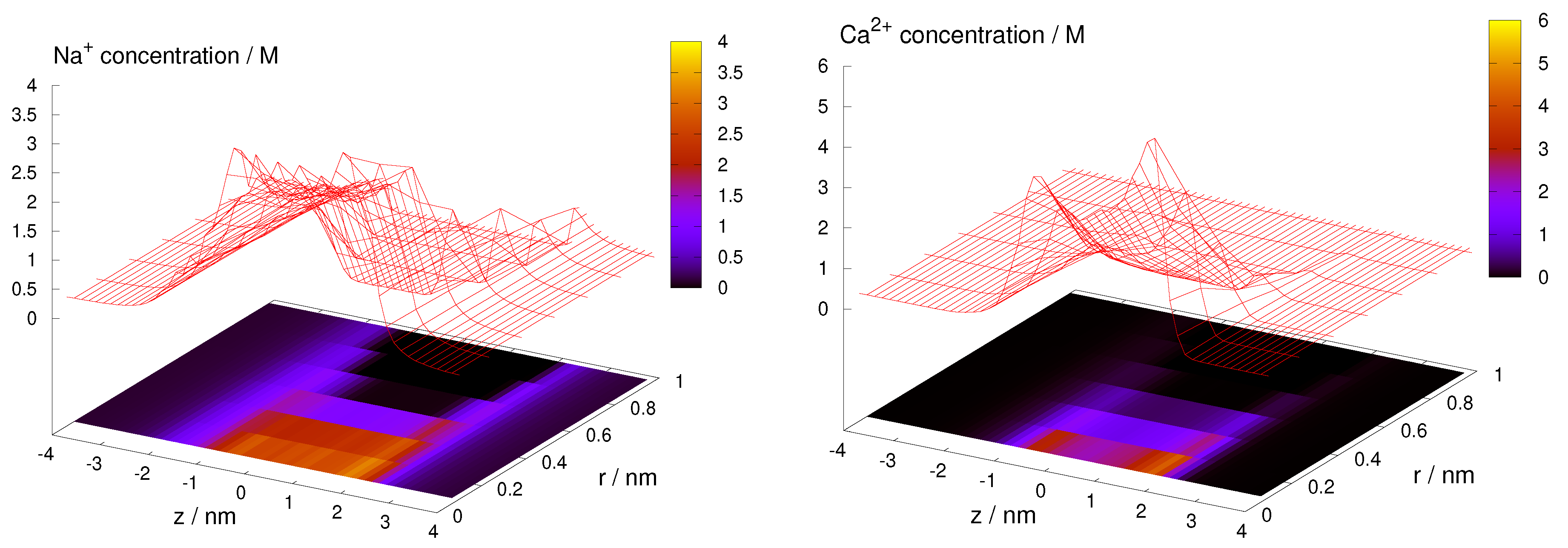
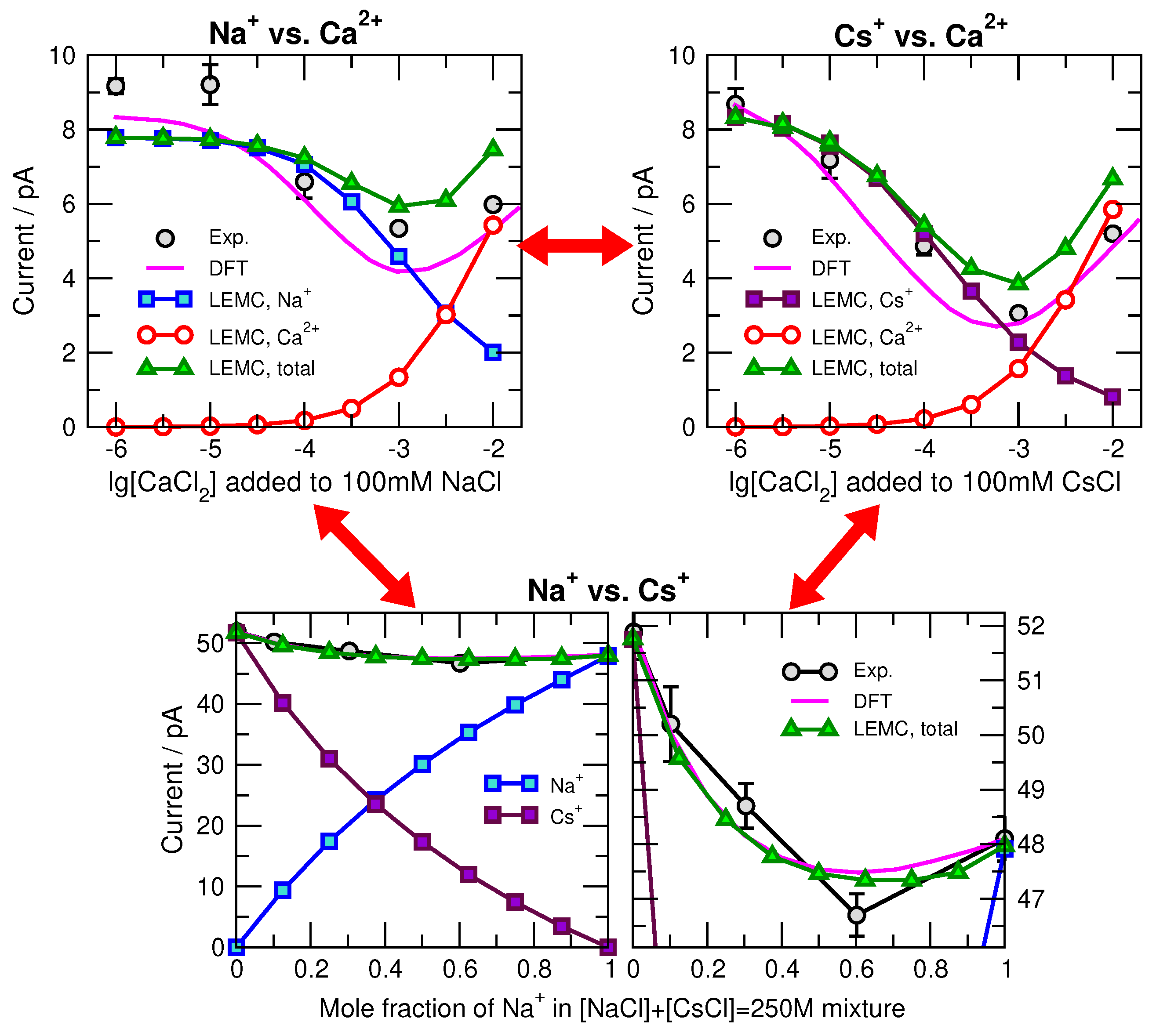
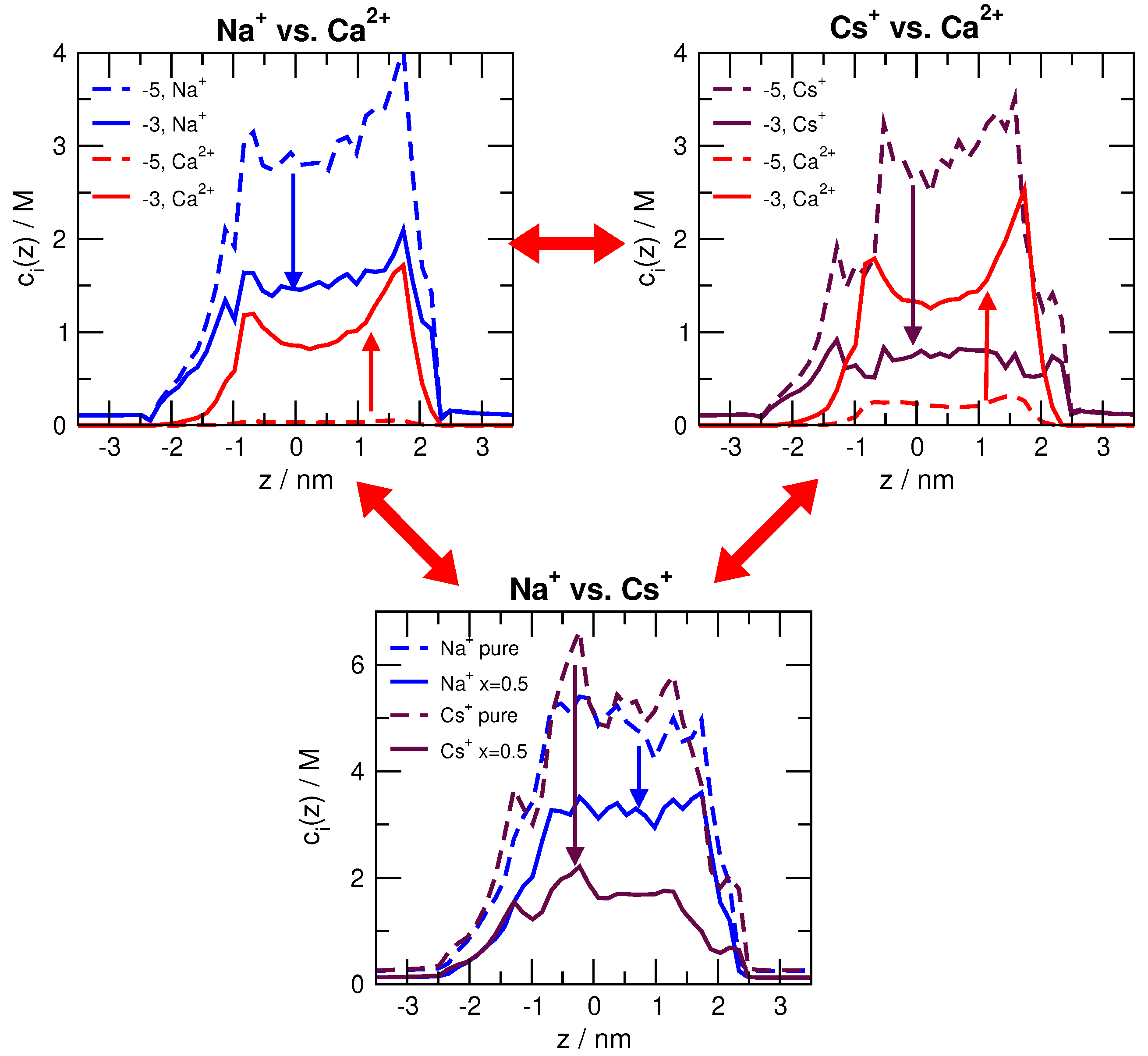
3.1.1. Ionic Concentrations and Current
3.1.2. Accurate Representation of Pore Charges is Important for Reproducing Device Function
3.1.3. Important versus Unimportant Degrees of Freedom
3.1.4. Transferability of Parameters
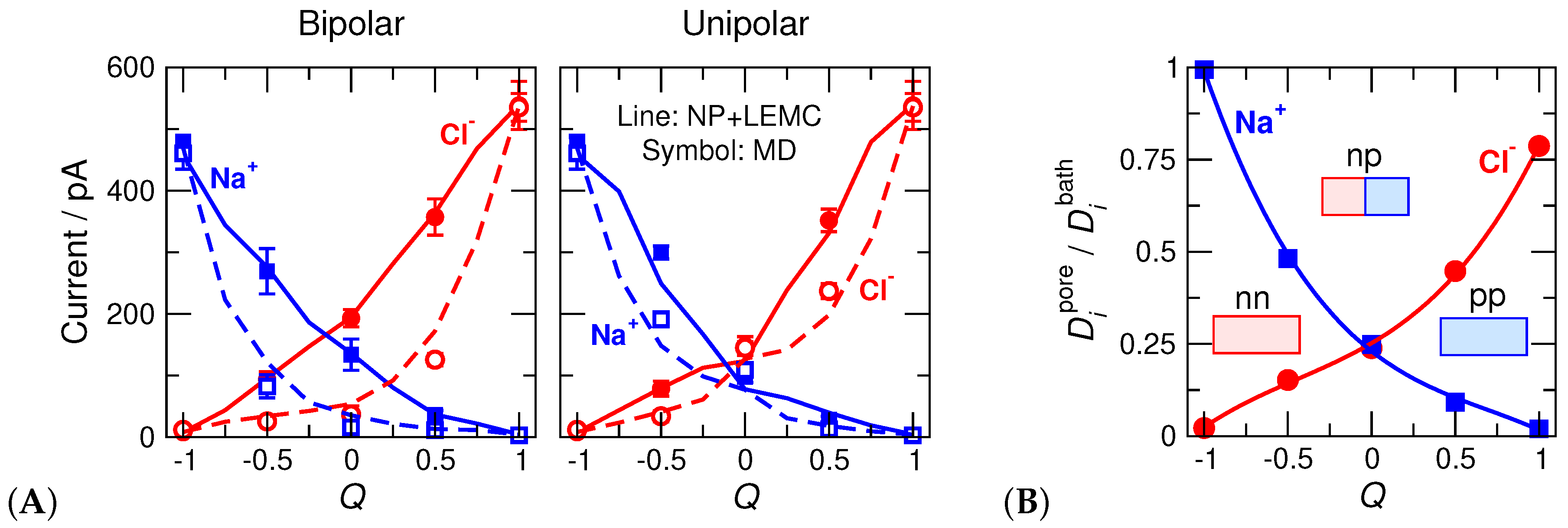
3.2. Nanopores of Different Device Functions from Different Charge Patterns
- We studied how different charge patterns influence concentration profiles, and, through those, device functions (rules of thumb #1 and #2).
- We performed simulations with models of different resolutions and studied the performance of reduced models compared to all-atom MD simulations. Special attention was given to whether water molecules could be treated implicitly, that is, whether they proved to be “unimportant” degrees of freedom (rule of thumb #3).
- We fit the diffusion coefficients in the pore to MD data and investigated their transferability over varying charge patterns (rule of thumb #4).
- Bipolar pores:
- Unipolar pores:
- In the other series, one of the regions was neutral (grey in Figure 5) in the intermediate cases. These are actually two series of experiments. Starting from the ‘nn’ limiting case (from left to right in Figure 5), through unipolar ‘n0’ charge patterns, we reach the ‘00’ limiting case (neutral pore) as changes from 1 to 0. Starting from the ‘pp’ limiting case (from right to left in Figure 5), through unipolar ‘0p’ charge patterns, we reach the ‘00’ limiting case (neutral pore) as changes from 0 to 1. The ‘n0’ (‘0p’) pore, where and ( and ) exhibits rectification due to the asymmetric charge pattern.
- Water is explicit (SPC) in MD, while it is implicit in LEMC.
- The ions have Lennard-Jones cores in MD, while they have hard-sphere cores in LEMC.
- The pore wall is a carbon nanotube (CNT) in MD, while it is a hard wall in LEMC.
- The membrane is confined by carbon nanosheets (CNS) in MD, while with hard walls in LEMC.
- The interior of the membrane is empty (a vacuum) in MD, while it is an region in LEMC.
- The MD simulation cell applies periodic boundary conditions, while the LEMC simulation cell is finite (a cylinder).
3.2.1. Concentration Profiles and Device Functions
3.2.2. Charge Pattern Determines Device Behavior
3.2.3. Water Molecules as Unimportant Degrees of Freedom
3.2.4. Transferability of the Fitted Diffusion Coefficient
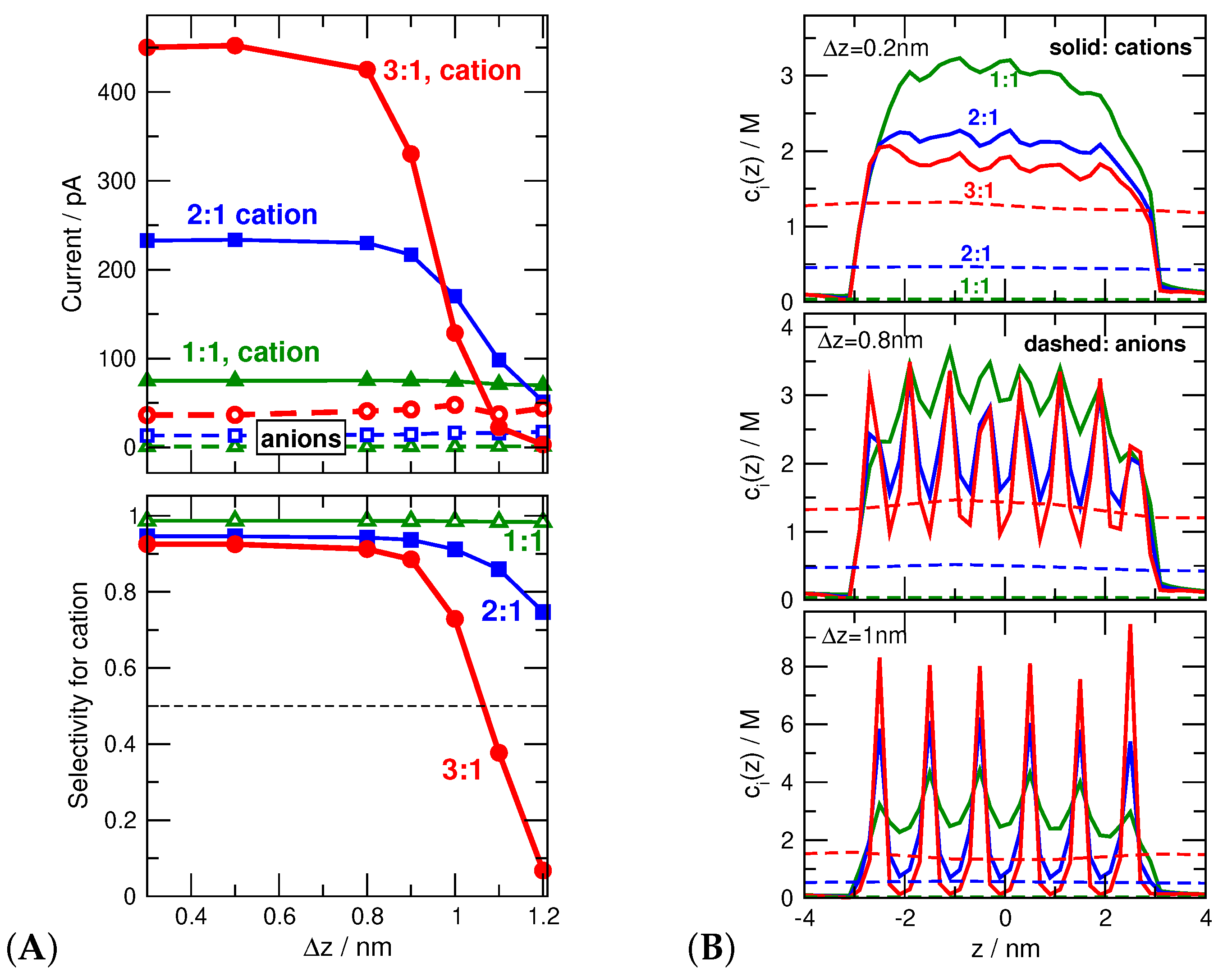
3.3. Selectivity Inversion Due to Charge Inversion
3.3.1. Axial Concentration Profiles Determine Selectivity
3.3.2. Charge Localization Is an Important Degree of Freedom
3.3.3. Future Work
4. Conclusions
- The current carried by an ionic species is mainly determined by the axial concentration profile of that species inside the pore.
- Care must be taken to model the pore charges since they produce the local ion concentrations, and, consequently, device function.
- The important degrees of freedom that must be included in the model are those that depend on the input parameters of the device (voltage and concentration), while those that do not can be replaced by response functions.
- Having the parameters within a response function not depend on external conditions (or at least have minimal dependence) makes those parameters transferable to other conditions, and this makes it possible for the model to make predictions that can be tested.
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Slope Conductance Theory
References
- Eisenberg, R.S. Living Transistors: A Physicist’s View of Ion Channels. Available online: http://arxiv.org/abs/q-bio/0506016v2 (accessed on 12 August 2020).
- Nonner, W.; Eisenberg, B. Ion Permeation and Glutamate Residues Linked by Poisson-Nernst-Planck Theory in L-type Calcium Channels. Biophys. J. 1998, 75, 1287–1305. [Google Scholar] [CrossRef] [Green Version]
- Nonner, W.; Chen, D.P.; Eisenberg, B. Anomalous Mole Fraction Effect, Electrostatics, and Binding in Ionic Channels. Biophys. J. 1998, 74, 2327–2334. [Google Scholar] [CrossRef] [Green Version]
- Nonner, W.; Catacuzzeno, L.; Eisenberg, B. Binding and Selectivity in L-Type Calcium Channels: A Mean Spherical Approximation. Biophys. J. 2000, 79, 1976–1992. [Google Scholar] [CrossRef] [Green Version]
- Boda, D.; Busath, D.D.; Henderson, D.; Sokołowski, S. Monte Carlo simulations of the mechanism for channel selectivity: The competition between volume exclusion and charge neutrality. J. Phys. Chem. B 2000, 104, 8903–8910. [Google Scholar] [CrossRef]
- Nonner, W.; Gillespie, D.; Henderson, D.; Eisenberg, B. Ion accumulation in a biological calcium channel: Effects of solvent and confining pressure. J. Phys. Chem. B 2001, 105, 6427–6436. [Google Scholar] [CrossRef]
- Boda, D.; Henderson, D.; Busath, D.D. Monte Carlo study of the effect of ion and channel size on the selectivity of a model calcium channel. J. Phys. Chem. B 2001, 105, 11574–11577. [Google Scholar] [CrossRef]
- Boda, D.; Busath, D.D.; Eisenberg, B.; Henderson, D.; Nonner, W. Monte Carlo simulations of ion selectivity in a biological Na channel: Charge-space competition. Phys. Chem. Chem. Phys. 2002, 4, 5154–5160. [Google Scholar] [CrossRef]
- Boda, D.; Henderson, D.; Busath, D.D. Monte Carlo study of the selectivity of calcium channels: Improved geometrical model. Mol. Phys. 2002, 100, 2361–2368. [Google Scholar] [CrossRef]
- Miedema, H.; Meter-Arkema, A.; Wierenga, J.; Tang, J.; Eisenberg, B.; Nonner, W.; Hektor, H.; Gillespie, D.; Meijberg, W. Permeation properties of an engineered bacterial OmpF porin containing the EEEE-locus of Ca2+ channels. Biophys. J. 2004, 87, 3137–3147. [Google Scholar] [CrossRef] [Green Version]
- Gillespie, D.; Xu, L.; Wang, Y.; Meissner, G. (De)constructing the Ryanodine Receptor: Modeling Ion Permeation and Selectivity of the Calcium Release Channel. J. Phys. Chem. B 2005, 109, 15598–15610. [Google Scholar] [CrossRef]
- Miedema, H.; Vrouenraets, M.; Wierenga, J.; Gillespie, D.; Eisenberg, B.; Meijberg, W.; Nonner, W. Ca2+ selectivity of a chemically modified OmpF with reduced pore volume. Biophys. J. 2006, 91, 4392–4400. [Google Scholar] [CrossRef] [Green Version]
- Boda, D.; Valiskó, M.; Eisenberg, B.; Nonner, W.; Henderson, D.; Gillespie, D. The effect of protein dielectric coefficient on the ionic selectivity of a calcium channel. J. Chem. Phys. 2006, 125, 034901. [Google Scholar] [CrossRef] [PubMed]
- Boda, D.; Nonner, W.; Valiskó, M.; Henderson, D.; Eisenberg, B.; Gillespie, D. Steric selectivity in Na channels arising from protein polarization and mobile side chains. Biophys. J. 2007, 93, 1960–1980. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Boda, D.; Valiskó, M.; Eisenberg, B.; Nonner, W.; Henderson, D.; Gillespie, D. Combined effect of pore radius and protein dielectric coefficient on the selectivity of a calcium channel. Phys. Rev. Lett. 2007, 98, 168102. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gillespie, D. Energetics of Divalent Selectivity in a Calcium Channel: The Ryanodine Receptor Case Study. Biophys. J. 2008, 94, 1169–1184. [Google Scholar] [CrossRef] [Green Version]
- Gillespie, D.; Boda, D. The anomalous mole fraction effect in calcium channels: A measure of preferential selectivity. Biophys. J. 2008, 95, 2658–2672. [Google Scholar] [CrossRef] [Green Version]
- Gillespie, D.; Boda, D.; He, Y.; Apel, P.; Siwy, Z. Synthetic nanopores as a test case for ion channel theories: The anomalous mole fraction effect without single filing. Biophys. J. 2008, 95, 609–619. [Google Scholar] [CrossRef] [Green Version]
- Gillespie, D.; Fill, M. Intracellular Calcium Release Channels Mediate Their Own Countercurrent: The Ryanodine Receptor Case Study. Biophys. J. 2008, 95, 3706–3714. [Google Scholar] [CrossRef] [Green Version]
- Gillespie, D.; Giri, J.; Fill, M. Reinterpreting the anomalous mole fraction effect: The Ryanodine receptor case study. Biophys. J. 2009, 97, 2212–2221. [Google Scholar] [CrossRef] [Green Version]
- Boda, D.; Valiskó, M.; Henderson, D.; Eisenberg, B.; Gillespie, D.; Nonner, W. Ion selectivity in L-type calcium channels by electrostatics and hard-core repulsion. J. Gen. Physiol. 2009, 133, 497–509. [Google Scholar] [CrossRef]
- He, Y.; Gillespie, D.; Boda, D.; Vlassiouk, I.; Eisenberg, R.S.; Siwy, Z.S. Tuning transport properties of nanofluidic devices with local charge inversion. JACS 2009, 131, 5194–5202. [Google Scholar] [CrossRef] [Green Version]
- Malasics, A.; Gillespie, D.; Nonner, W.; Henderson, D.; Eisenberg, B.; Boda, D. Protein structure and ionic selectivity in calcium channels: Selectivity filter size, not shape, matters. Biochim. Biophys. Acta Biomembr. 2009, 1788, 2471–2480. [Google Scholar] [CrossRef] [Green Version]
- Malasics, M.; Boda, D.; Valiskó, M.; Henderson, D.; Gillespie, D. Simulations of calcium channel block by trivalent ions: Gd3+ competes with permeant ions for the selectivity filter. Biochim. Biophys. Acta Biomembr. 2010, 1798, 2013–2021. [Google Scholar] [CrossRef] [Green Version]
- Rutkai, G.; Boda, D.; Kristóf, T. Relating binding affinity to dynamical selectivity from dynamic Monte Carlo simulations of a model calcium channel. J. Phys. Chem. Lett. 2010, 1, 2179–2184. [Google Scholar] [CrossRef]
- Boda, D.; Giri, J.; Henderson, D.; Eisenberg, B.; Gillespie, D. Analyzing the components of the free energy landscape in a calcium selective ion channel by Widom’s particle insertion method. J. Chem. Phys. 2011, 134, 055102. [Google Scholar] [CrossRef] [Green Version]
- Giri, J.; Fonseca, J.; Boda, D.; Henderson, D.; Eisenberg, B. Self-organized models of selectivity in calcium channels. Phys. Biol. 2011, 8, 026004. [Google Scholar] [CrossRef] [PubMed]
- Gillespie, D. High Energy Conversion Efficiency in Nanofluidic Channels. Nano Lett. 2012, 12, 1410–1416. [Google Scholar] [CrossRef]
- Boda, D.; Gillespie, D. Steady state electrodiffusion from the Nernst-Planck equation coupled to Local Equilibrium Monte Carlo simulations. J. Chem. Theor. Comput. 2012, 8, 824–829. [Google Scholar] [CrossRef]
- Csányi, E.; Boda, D.; Gillespie, D.; Kristóf, T. Current and selectivity in a model sodium channel under physiological conditions: Dynamic Monte Carlo simulations. Biochim. Biophys. Acta Biomembr. 2012, 1818, 592–600. [Google Scholar] [CrossRef] [Green Version]
- Ható, Z.; Boda, D.; Kristóf, T. Simulation of steady-state diffusion: Driving force ensured by Dual Control Volumes or Local Equilibrium Monte Carlo. J. Chem. Phys. 2012, 137, 054109. [Google Scholar] [CrossRef] [PubMed]
- Gillespie, D.; Chen, H.; Fill, M. Is ryanodine receptor a calcium or magnesium channel? Roles of K+ and Mg2+ during Ca2+ release. Cell Calcium 2012, 51, 427–433. [Google Scholar] [CrossRef] [Green Version]
- Hoffmann, J.; Gillespie, D. Ion Correlations in Nanofluidic Channels: Effects of Ion Size, Valence, and Concentration on Voltage- and Pressure-Driven Currents. Langmuir 2013, 29, 1303–1317. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Boda, D.; Henderson, D.; Gillespie, D. The role of solvation in the binding selectivity of the L-type calcium channel. J. Chem. Phys. 2013, 139, 055103. [Google Scholar] [CrossRef]
- Gillespie, D.; Xu, L.; Meissner, G. Selecting Ions by Size in a Calcium Channel: The Ryanodine Receptor Case Study. Biophys. J. 2014, 107, 2263–2273. [Google Scholar] [CrossRef] [Green Version]
- Berti, C.; Furini, S.; Gillespie, D.; Boda, D.; Eisenberg, R.S.; Sangiorgi, E.; Fiegna, C. A 3-D Brownian Dynamics simulator for the study of ion permeation through membrane pores. J. Chem. Theor. Comput. 2014, 10, 2911–2926. [Google Scholar] [CrossRef]
- Boda, D. Chapter 5: Monte Carlo Simulation of Electrolyte Solutions in Biology: In and Out of Equilibrium. In Annual Reports in Computational Chemistry; Wheeler, R.A., Ed.; Elsevier: Amsterdam, The Netherlands, 2014; Volume 10, pp. 127–163. [Google Scholar] [CrossRef]
- Boda, D.; Csányi, E.; Gillespie, D.; Kristóf, T. Dynamic Monte Carlo simulation of coupled transport through a narrow multiply-occupied pore. J. Phys. Chem. C 2014, 118, 700–707. [Google Scholar] [CrossRef] [Green Version]
- Boda, D.; Kovács, R.; Gillespie, D.; Kristóf, T. Selective transport through a model calcium channel studied by Local Equilibrium Monte Carlo simulations coupled to the Nernst-Planck equation. J. Mol. Liq. 2014, 189, 100–112. [Google Scholar] [CrossRef]
- Ható, Z.; Boda, D.; Gillepie, D.; Vrabec, J.; Rutkai, G.; Kristóf, T. Simulation study of a rectifying bipolar ion channel: Detailed model versus reduced model. Cond. Matt. Phys. 2016, 19, 13802. [Google Scholar] [CrossRef] [Green Version]
- Fertig, D.; Mádai, E.; Valiskó, M.; Boda, D. Simulating ion transport with the NP+LEMC method. Applications to ion channels and nanopores. Hung. J. Ind. Chem. 2017, 45, 73–84. [Google Scholar] [CrossRef]
- Ható, Z.; Valiskó, M.; Kristóf, T.; Gillespie, D.; Boda, D. Multiscale modeling of a rectifying bipolar nanopore: Explicit-water versus implicit-water simulations. Phys. Chem. Chem. Phys. 2017, 19, 17816–17826. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mádai, E.; Valiskó, M.; Dallos, A.; Boda, D. Simulation of a model nanopore sensor: Ion competition underlines device behavior. J. Chem. Phys. 2017, 147, 244702. [Google Scholar] [CrossRef] [Green Version]
- Matejczyk, B.; Valiskó, M.; Wolfram, M.T.; Pietschmann, J.F.; Boda, D. Multiscale modeling of a rectifying bipolar nanopore: Comparing Poisson-Nernst-Planck to Monte Carlo. J. Chem. Phys. 2017, 146, 124125. [Google Scholar] [CrossRef] [Green Version]
- Mádai, E.; Matejczyk, B.; Dallos, A.; Valiskó, M.; Boda, D. Controlling ion transport through nanopores: Modeling transistor behavior. Phys. Chem. Chem. Phys. 2018, 20, 24156–24167. [Google Scholar] [CrossRef] [Green Version]
- Valiskó, M.; Matejczyk, B.; Ható, Z.; Kristóf, T.; Mádai, E.; Fertig, D.; Gillespie, D.; Boda, D. Multiscale analysis of the effect of surface charge pattern on a nanopore’s rectification and selectivity properties: From all-atom model to Poisson-Nernst-Planck. J. Chem. Phys. 2019, 150, 144703. [Google Scholar] [CrossRef] [Green Version]
- Mádai, E.; Valiskó, M.; Boda, D. The effect of the charge pattern on the applicability of a nanopore as a sensor. J. Mol. Liq. 2019, 283, 391–398. [Google Scholar] [CrossRef] [Green Version]
- Fertig, D.; Valiskó, M.; Boda, D. Controlling ionic current through a nanopore by tuning pH: A Local Equilibrium Monte Carlo study. Mol. Phys. 2019, 117, 2793–2801. [Google Scholar] [CrossRef] [Green Version]
- Mádai, E.; Valiskó, M.; Boda, D. Application of a bipolar nanopore as a sensor: Rectification as an additional device function. Phys. Chem. Chem. Phys. 2019, 21, 19772–19784. [Google Scholar] [CrossRef] [Green Version]
- Fertig, D.; Matejczyk, B.; Valiskó, M.; Gillespie, D.; Boda, D. Scaling Behavior of Bipolar Nanopore Rectification with Multivalent Ions. J. Phys. Chem. C 2019, 123, 28985–28996. [Google Scholar] [CrossRef] [Green Version]
- Hohl, B.; Mádai, E.; Boda, D.; Valiskó, M. Modeling of a pH-tunable dual-response nanopore sensor. J. Mol. Liq. 2020, 310, 112946. [Google Scholar] [CrossRef]
- Fertig, D.; Valiskó, M.; Boda, D. Rectification of bipolar nanopores in multivalent electrolytes: Effect of charge inversion and strong ionic correlations. Phys. Chem. Chem. Phys. 2020, 22, 19033–19045. [Google Scholar] [CrossRef]
- Eisenberg, B. Ionic Channels as Natural Nanodevices. J. Comput. Electron. 2002, 1, 331–333. [Google Scholar] [CrossRef]
- Eisenberg, B. Asking biological questions of physical systems: The device approach to emergent properties. J. Mol. Liq. 2018, 270, 212–217. [Google Scholar] [CrossRef] [Green Version]
- Hille, B. Ion Channels of Excitable Membranes, 3rd ed.; Sinauer Associates: Sunderland, MA, USA, 2001. [Google Scholar]
- Albrecht, T.; Gibb, T.; Nuttall, P. Ion Transport in Nanopores. In Engineered Nanopores for Bioanalytical Applications; Elsevier BV: Amsterdam, The Netherlands, 2013; pp. 1–30. [Google Scholar] [CrossRef]
- Iqbal, S.M.; Bashir, R. (Eds.) Nanopores; Springer Science and Business Media: Amsterdam, The Netherlands, 2011. [Google Scholar] [CrossRef]
- Kuo, T.C.; Sloan, L.A.; Sweedler, J.V.; Bohn, P.W. Manipulating Molecular Transport through Nanoporous Membranes by Control of Electrokinetic Flow: Effect of Surface Charge Density and Debye Length. Langmuir 2001, 17, 6298–6303. [Google Scholar] [CrossRef]
- Ali, M.; Ramirez, P.; Mafé, S.; Neumann, R.; Ensinger, W. A pH-Tunable Nanofluidic Diode with a Broad Range of Rectifying Properties. ACS Nano 2009, 3, 603–608. [Google Scholar] [CrossRef] [PubMed]
- Vlassiouk, I.; Kozel, T.R.; Siwy, Z.S. Biosensing with Nanofluidic Diodes. J. Am. Chem. Soc. 2009, 131, 8211–8220. [Google Scholar] [CrossRef] [Green Version]
- Ali, M.; Nasir, S.; Ramirez, P.; Cervera, J.; Mafe, S.; Ensinger, W. Calcium Binding and Ionic Conduction in Single Conical Nanopores with Polyacid Chains: Model and Experiments. ACS Nano 2012, 6, 9247–9257. [Google Scholar] [CrossRef] [PubMed]
- Siwy, Z.; Trofin, L.; Kohli, P.; Baker, L.A.; Trautmann, C.; Martin, C.R. Protein Biosensors Based on Biofunctionalized Conical Gold Nanotubes. J. Am. Chem. Soc. 2005, 127, 5000–5001. [Google Scholar] [CrossRef]
- Gracheva, M.E.; Vidal, J.; Leburton, J.P. p-n Semiconductor membrane for electrically tunable ion current rectification and filtering. Nano Lett. 2007, 7, 1717–1722. [Google Scholar] [CrossRef] [Green Version]
- Piruska, A.; Gong, M.; Sweedler, J.V. Nanofluidics in chemical analysis. Chem. Soc. Rev. 2010, 39, 1060–1072. [Google Scholar] [CrossRef]
- Lepoitevin, M.; Ma, T.; Bechelany, M.; Janot, J.M.; Balme, S. Functionalization of single solid state nanopores to mimic biological ion channels: A review. Adv. Coll. Interf. 2017, 250, 195–213. [Google Scholar] [CrossRef]
- Daiguji, H.; Yang, P.; Majumdar, A. Ion Transport in Nanofluidic Channels. Nano Lett. 2004, 4, 137–142. [Google Scholar] [CrossRef]
- Howorka, S.; Siwy, Z. Nanopores: Generation, Engineering, and Singly-Molecule Applications. In Handbook of Single-Molecule Biophysics; Hinterdorfer, P., van Oijen, A., Eds.; Advances in Chemical Physics; Springer: Amsterdam, The Netherlands, 2009; Chapter 1; pp. 293–339. [Google Scholar] [CrossRef]
- Eijkel, J.C.T.; van den Berg, A. Nanofluidics and the Chemical Potential Applied to Solvent and Solute Transport. Chem. Soc. Rev. 2010, 39, 957. [Google Scholar] [CrossRef]
- Gokel, G.W.; Negin, S. Synthetic Ion Channels: From Pores to Biological Applications. Acc. Chem. Res. 2013, 46, 2824–2833. [Google Scholar] [CrossRef] [PubMed]
- Guo, W.; Tian, Y.; Jiang, L. Asymmetric Ion Transport through Ion-Channel-Mimetic Solid-State Nanopores. Acc. Chem. Res. 2013, 46, 2834–2846. [Google Scholar] [CrossRef]
- Makra, I.; Gyurcsányi, R.E. Electrochemical sensing with nanopores: A mini review. Electrochem. Commun. 2014, 43, 55–59. [Google Scholar] [CrossRef] [Green Version]
- Zhang, H.; Tian, Y.; Jiang, L. Fundamental studies and practical applications of bio-inspired smart solid-state nanopores and nanochannels. Nano Today 2016, 11, 61–81. [Google Scholar] [CrossRef]
- Kalman, E.B.; Vlassiouk, I.; Siwy, Z.S. Nanofluidic bipolar transistors. Adv. Mater. 2008, 20, 293–297. [Google Scholar] [CrossRef]
- Duboué-Dijon, E.; Javanainen, M.; Delcroix, P.; Jungwirth, P.; Martinez-Seara, H. A practical guide to biologically relevant molecular simulations with charge scaling for electronic polarization. J. Chem. Phys. 2020, 153, 050901. [Google Scholar] [CrossRef]
- Nernst, W. Zur Kinetik der in Lösung befindlichen Körper. Z. Phys. Chem. 1888, 2. [Google Scholar] [CrossRef] [Green Version]
- Planck, M. Ueber die Erregung von Electricität und Wärme in Electrolyten. Ann. Phys. Chem. 1890, 275, 161–186. [Google Scholar] [CrossRef] [Green Version]
- Varma, S.; Rempe, S.B. Tuning Ion Coordination Architectures to Enable Selective Partitioning. Biophys. J. 2007, 93, 1093–1099. [Google Scholar] [CrossRef] [Green Version]
- Voukadinova, A.; Gillespie, D. Energetics of Counterion Adsorption in the Electrical Double Layer. J. Chem. Phys. 2019, 150, 154706. [Google Scholar] [CrossRef] [PubMed]
- Norman, G.E.; Filinov, V.S. Investigations of Phase Transitions by a Monte-Carlo Method. High Temp. (USSR) 1969, 7, 216–222. [Google Scholar]
- Adams, D. Chemical potential of hard-sphere fluids by Monte Carlo methods. Mol. Phys. 1974, 28, 1241–1252. [Google Scholar] [CrossRef]
- McCleskey, E.W. Ion Channel Selectivity Using an Electric Stew. Biophys. J. 2000, 79, 1691–1692. [Google Scholar] [CrossRef] [Green Version]
- Humphrey, W.; Dalke, A.; Schulten, K. VMD: Visual molecular dynamics. J. Mol. Graphics 1996, 14, 33–38. [Google Scholar] [CrossRef]
- Almers, W.; McCleskey, E.W.; Palade, P.T. A non-selective cation conductance in frog muscle membrane blocked by micromolar external calcium ions. J. Physiol. 1984, 353, 565–583. [Google Scholar] [CrossRef]
- Hess, P.; Lansman, J.B.; Tsien, R.W. Calcium channel selectivity for divalent and monovalent cations. Voltage and concentration dependence of single channel current in ventricular heart cells. J. Gen. Physiol. 1986, 88, 293–319. [Google Scholar] [CrossRef] [Green Version]
- Friel, D.D.; Tsien, R.W. Voltage-gated calcium channels: Direct observation of the anomalous mole fraction effect at the single-channel level. Proc. Natl. Acad. Sci. USA 1989, 86, 5207–5211. [Google Scholar] [CrossRef] [Green Version]
- Yue, D.T.; Marban, E. Permeation in the dihydropyridine-sensitive calcium channel. Multi-ion occupancy but no anomalous mole-fraction effect between Ba2+ and Ca2+. J. Gen. Physiol. 1990, 95, 911–939. [Google Scholar] [CrossRef]
- Prod’hom, B.; Pietrobon, D.; Hess, P. Interactions of protons with single open L-type calcium channels. Location of protonation site and dependence of proton-induced current fluctuations on concentration and species of permeant ion. J. Gen. Physiol. 1989, 94, 23–42. [Google Scholar] [CrossRef] [Green Version]
- Babich, O.; Reeves, J.; Shirokov, R. Block of CaV1.2 Channels by Gd3+ Reveals Preopening Transitions in the Selectivity Filter. J. Gen. Physiol. 2007, 129, 461–475. [Google Scholar] [CrossRef] [Green Version]
- Heinemann, S.H.; Terlau, H.; Stühmer, W.; Imoto, K.; Numa, S. Calcium channel characteristics conferred on the sodium channel by single mutations. Nature 1992, 356, 441–443. [Google Scholar] [CrossRef]
- Xu, L.; Wang, Y.; Gillespie, D.; Meissner, G. Two Rings of Negative Charges in the Cytosolic Vestibule of Type-1 Ryanodine Receptor Modulate Ion Fluxes. Biophys. J. 2006, 90, 443–453. [Google Scholar] [CrossRef] [Green Version]
- Biggin, P.; Smith, G.; Shrivastava, I.; Choe, S.; Sansom, M. Potassium and sodium ions in a potassium channel studied by molecular dynamics simulations. Biochim. Biophys. Acta (BBA) Biomembr. 2001, 1510, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Lansman, J.B.; Hess, P.; Tsien, R.W. Blockade of current through single calcium channels by Cd2+, Mg2+, and Ca2+. Voltage and concentration dependence of calcium entry into the pore. J. Gen. Physiol. 1986, 88, 321–347. [Google Scholar] [CrossRef] [Green Version]
- Shoorideh, K.; Chui, C.O. On the origin of enhanced sensitivity in nanoscale FET-based biosensors. Proc. Natl. Acad. Sci. USA 2014, 111, 5111–5116. [Google Scholar] [CrossRef] [Green Version]



| Ion | (Pauling) | (LEMC) | (DFT) | |
|---|---|---|---|---|
| nm | ms | |||
| Na | ||||
| Cs | ||||
| Ca | ||||
| Cl | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boda, D.; Valiskó, M.; Gillespie, D. Modeling the Device Behavior of Biological and Synthetic Nanopores with Reduced Models. Entropy 2020, 22, 1259. https://doi.org/10.3390/e22111259
Boda D, Valiskó M, Gillespie D. Modeling the Device Behavior of Biological and Synthetic Nanopores with Reduced Models. Entropy. 2020; 22(11):1259. https://doi.org/10.3390/e22111259
Chicago/Turabian StyleBoda, Dezső, Mónika Valiskó, and Dirk Gillespie. 2020. "Modeling the Device Behavior of Biological and Synthetic Nanopores with Reduced Models" Entropy 22, no. 11: 1259. https://doi.org/10.3390/e22111259
APA StyleBoda, D., Valiskó, M., & Gillespie, D. (2020). Modeling the Device Behavior of Biological and Synthetic Nanopores with Reduced Models. Entropy, 22(11), 1259. https://doi.org/10.3390/e22111259






