Gallager Exponent Analysis of Coherent MIMO FSO Systems over Gamma-Gamma Turbulence Channels
Abstract
:1. Introduction
2. The System and Gallager’s Exponent
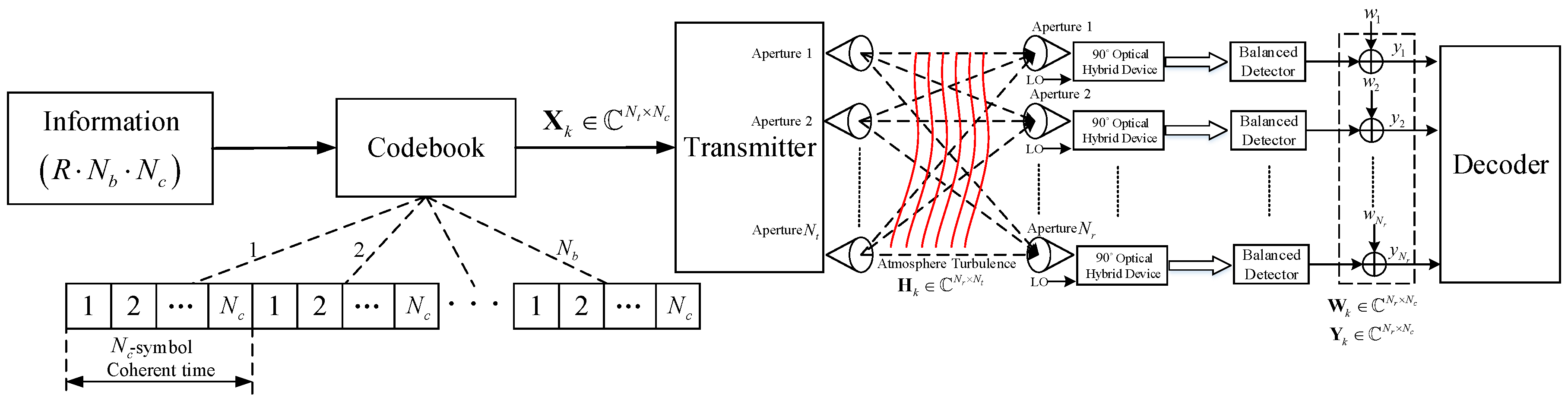
2.1. System Model
2.2. Gallager’s Exponent
3. Gallager’s Exponent for Gamma–Gamma Block Fading Channels
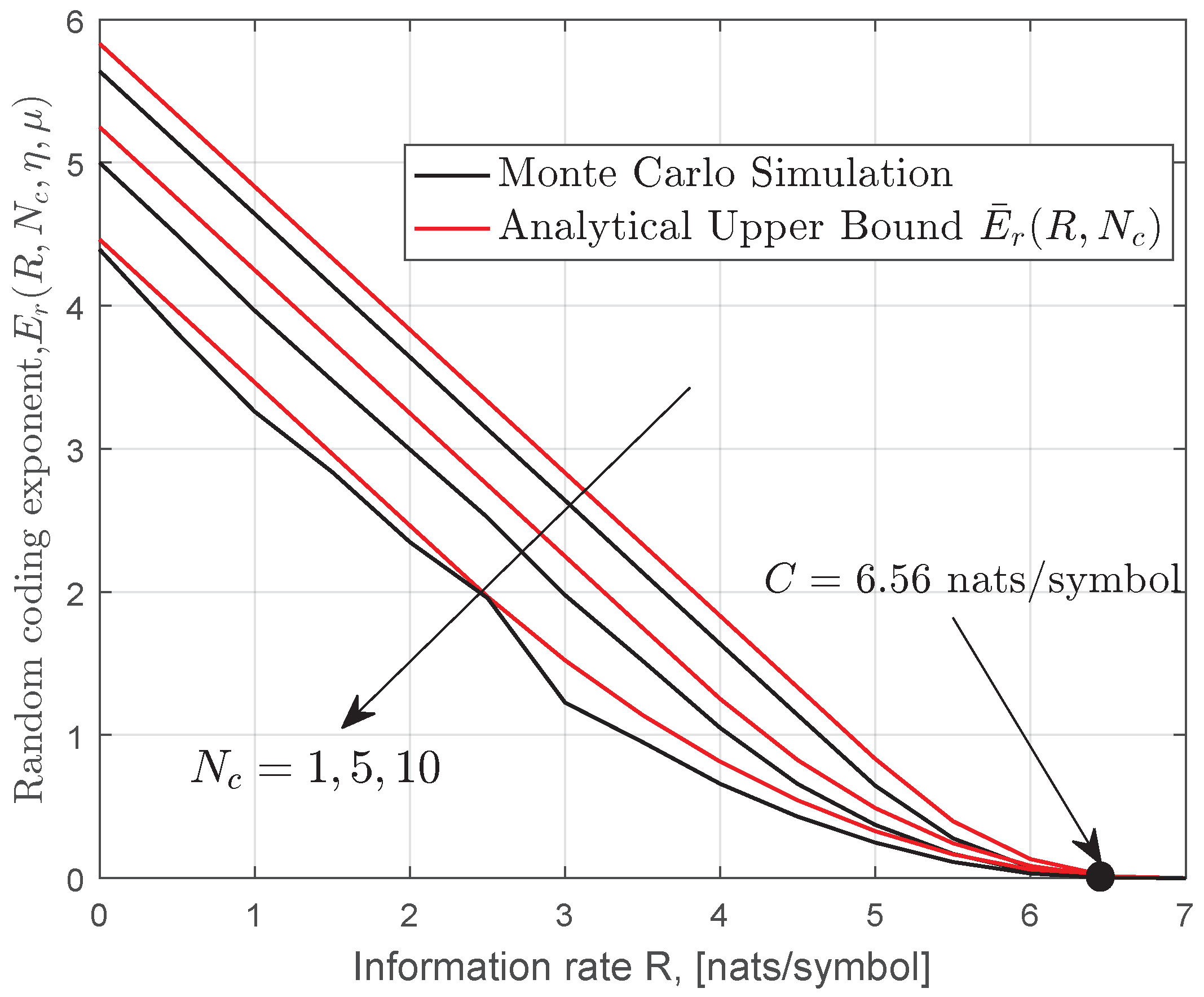
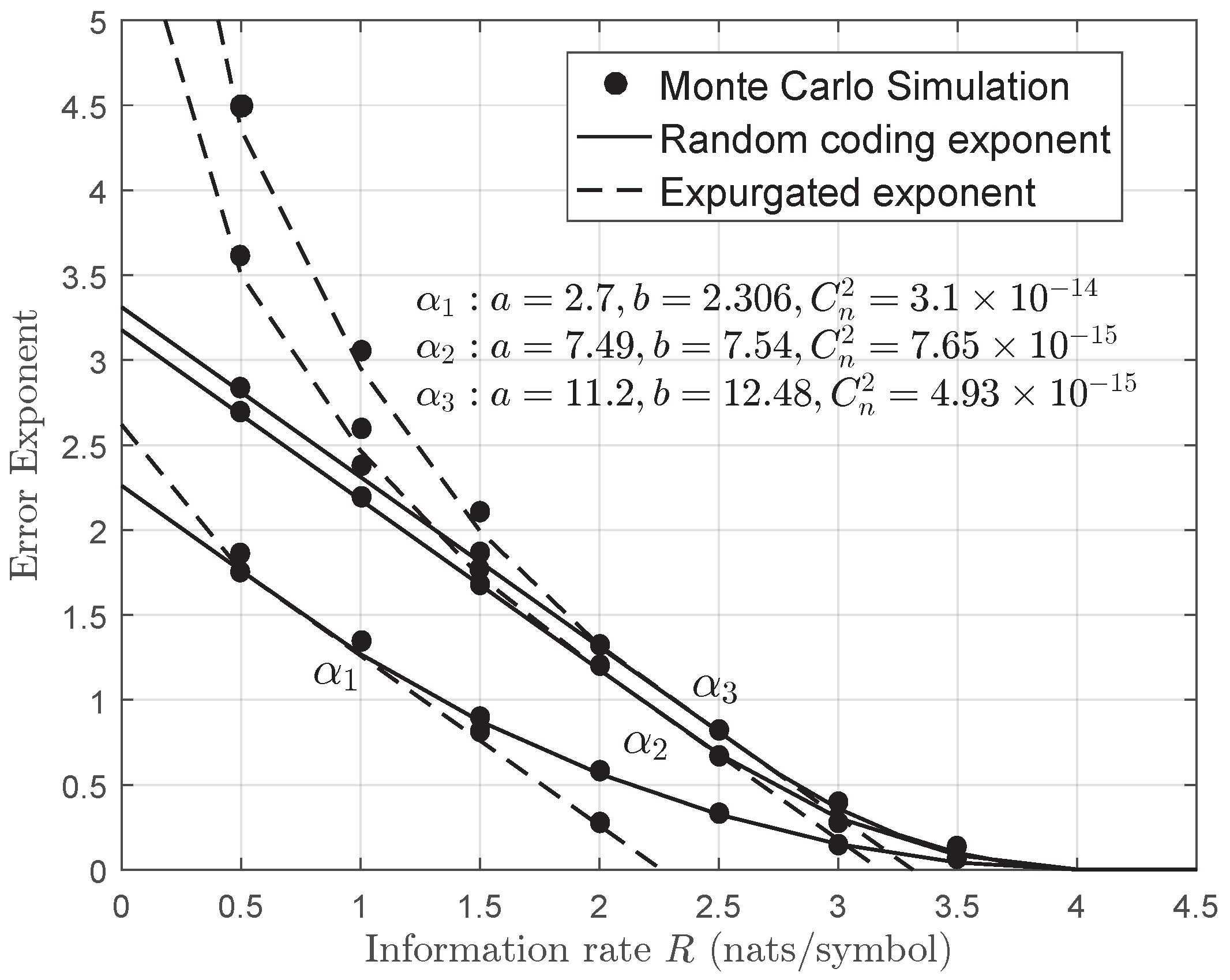
3.1. Random Coding Exponent Analysis
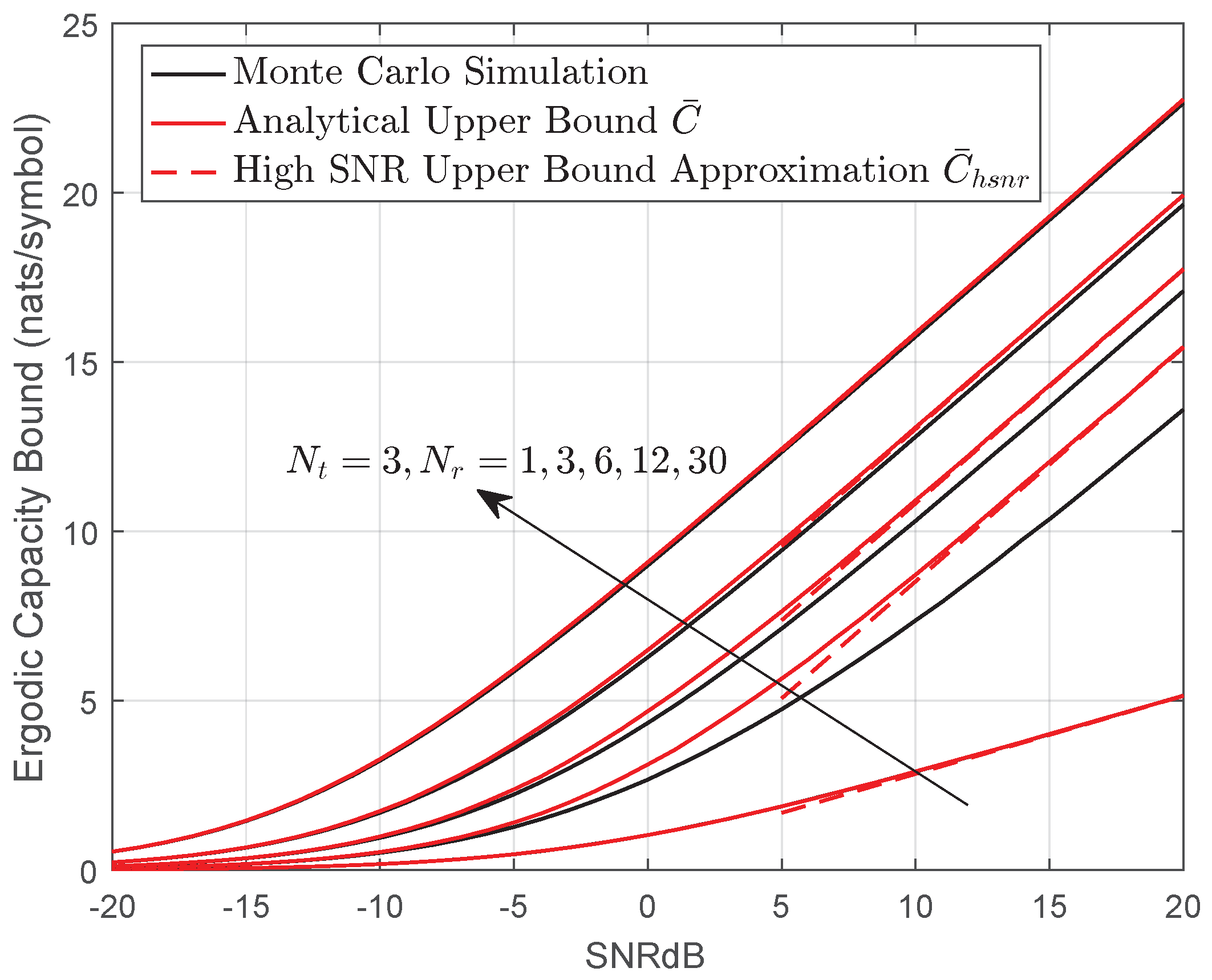
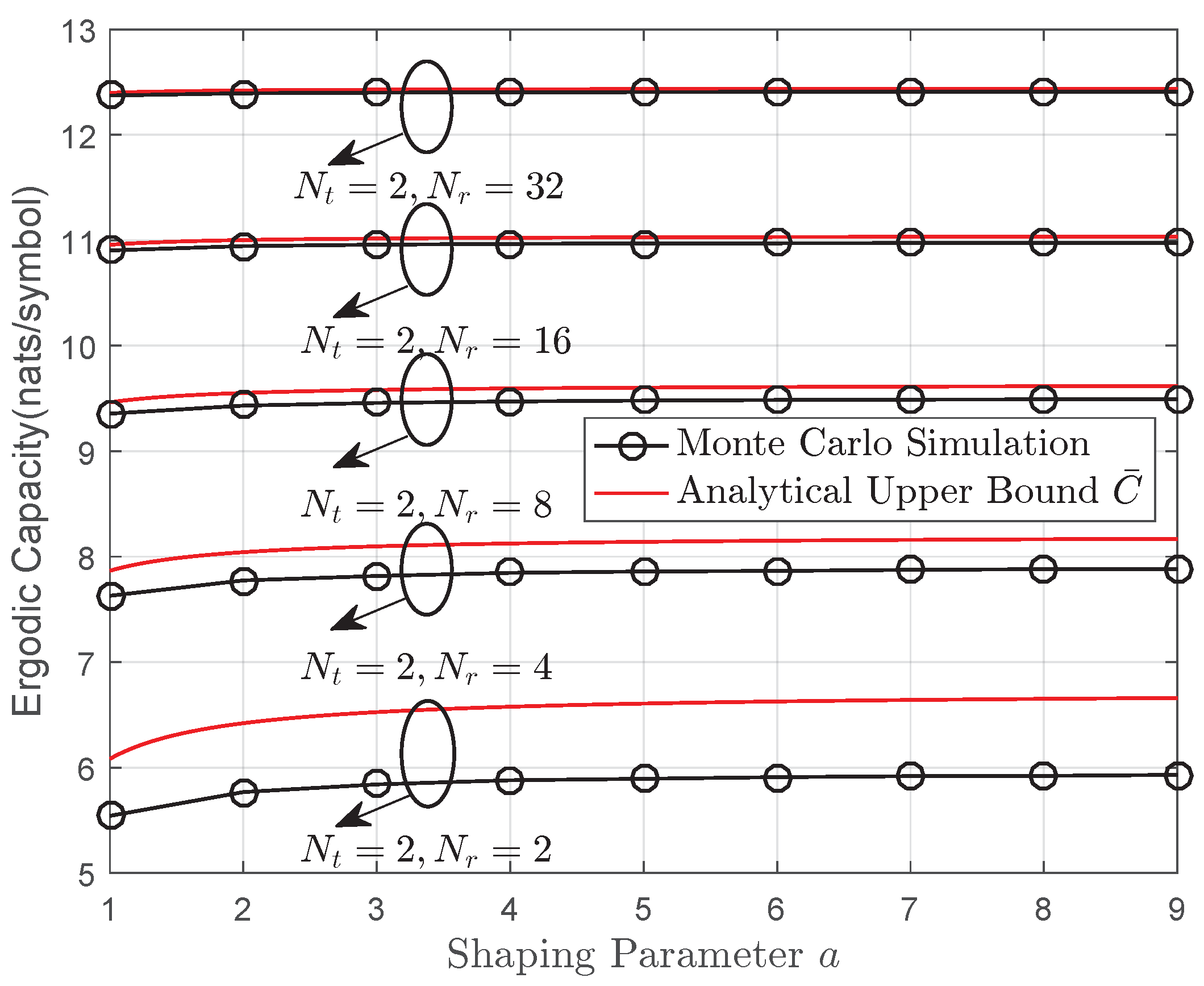
3.2. Ergodic Capacity Analysis
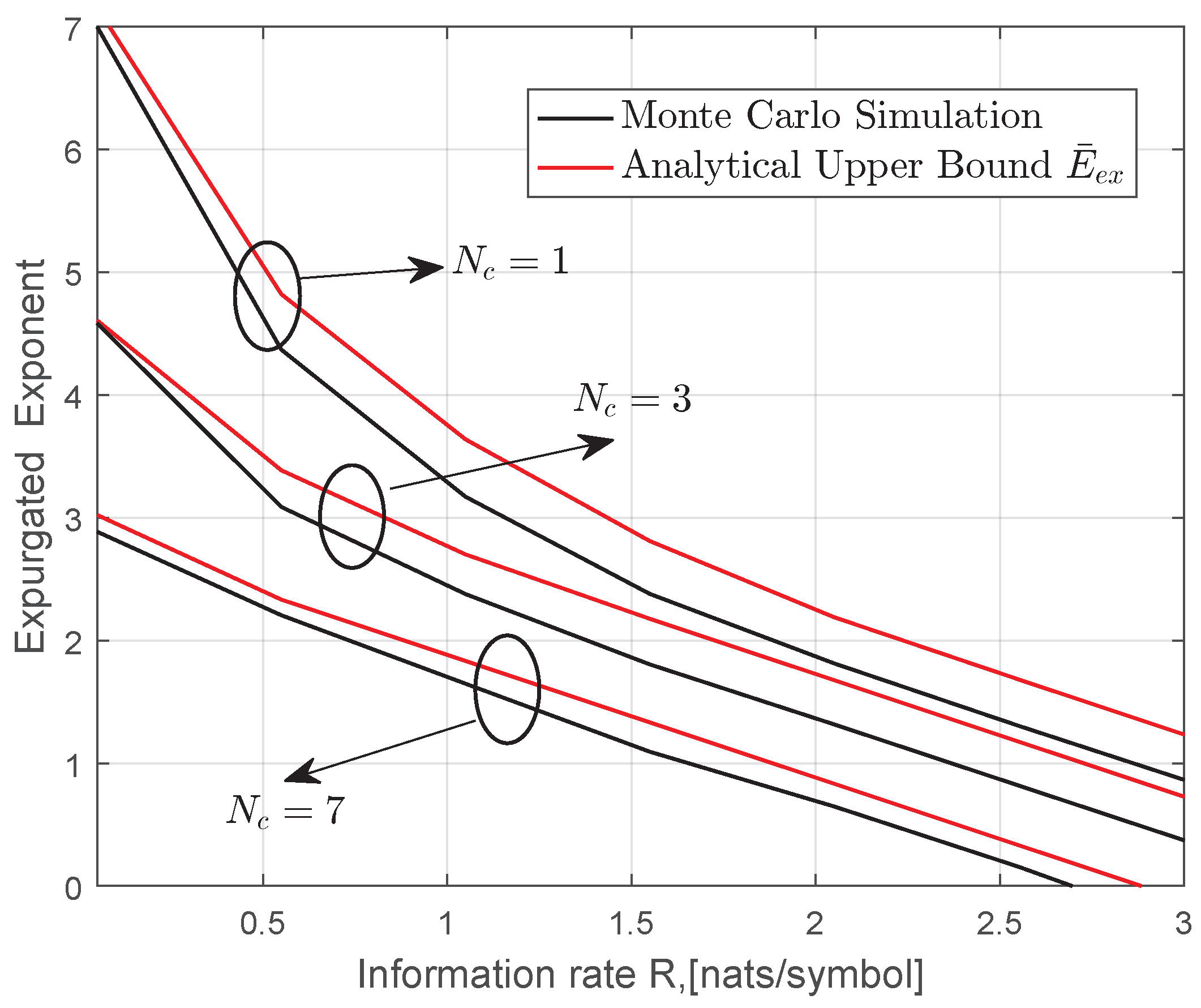
3.3. Expurgated Exponents
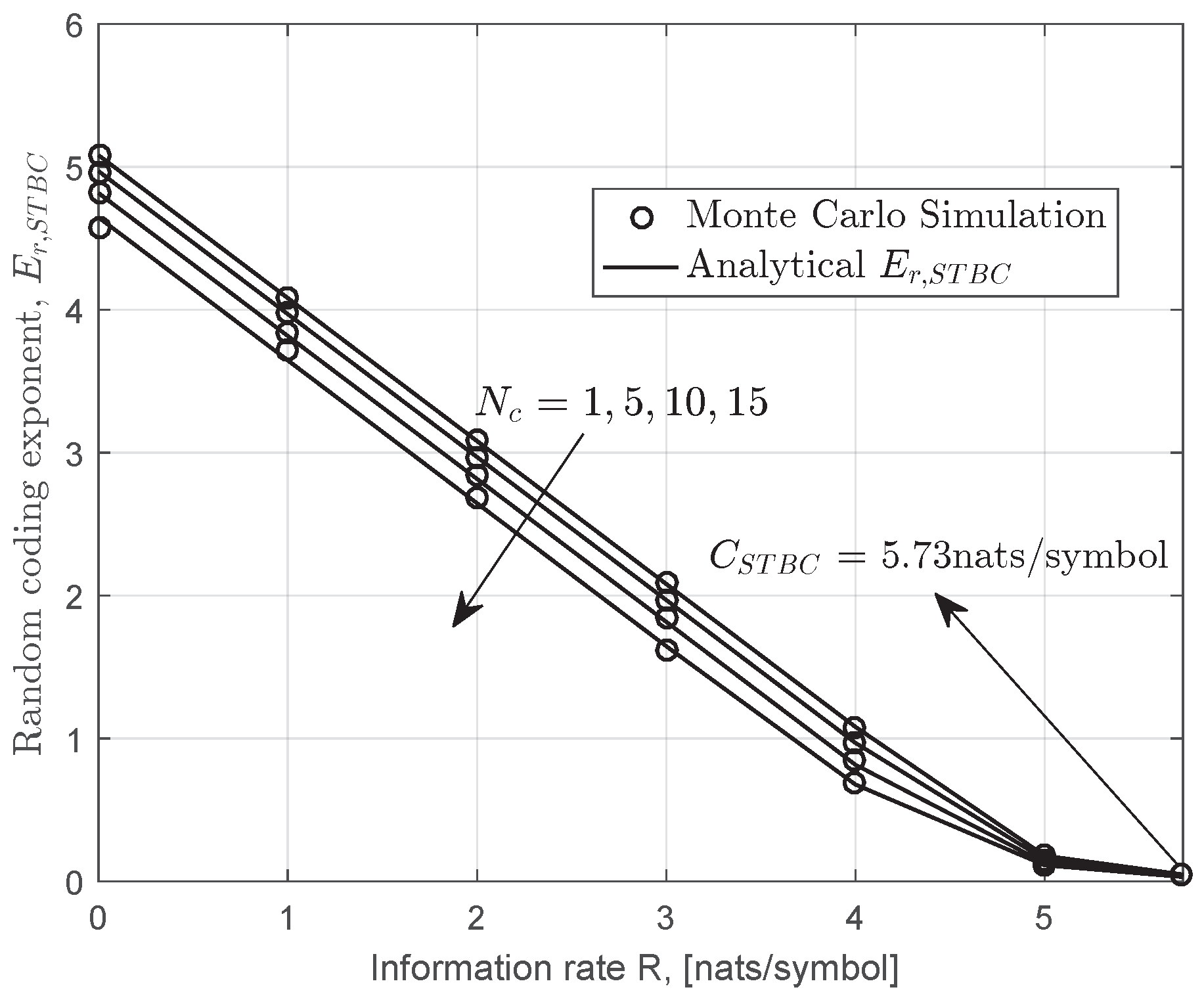
4. Error Exponent for MIMO-STBC Systems
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
Appendix B
References
- Telatar, E. Capacity of Multi-antenna Gaussian Channels. Eur. Trans. Telecommun. 1999, 10, 585–595. [Google Scholar] [CrossRef]
- Kang, M.; Alouini, M.S. Capacity of MIMO Rician channels. IEEE Trans. Wirel. Commun. 2006, 5, 112–122. [Google Scholar] [CrossRef]
- Fraidenraich, G.; Leveque, O.; Cioffi, J.M. On the MIMO Channel Capacity for the Nakagami-m Channel. IEEE Trans. Inf. Theory 2008, 54, 3752–3757. [Google Scholar] [CrossRef]
- Matthaiou, M.; Chatzidiamantis, N.D.; Karagiannidis, G.K.; Nossek, J.A. On the Capacity of Generalized- K Fading MIMO Channels. IEEE Trans. Signal Process. 2010, 58, 5939–5944. [Google Scholar] [CrossRef]
- Zhang, J.; Dai, L.; Zhang, X.; Björnson, E.; Wang, Z. Achievable Rate of Rician Large-Scale MIMO Channels With Transceiver Hardware Impairments. IEEE Trans. Veh. Technol. 2016, 65, 8800–8806. [Google Scholar] [CrossRef] [Green Version]
- Shannon, C.E. Probability of error for optimal codes in a Gaussian channel. Bell Syst. Tech. J. 1959, 38, 611–656. [Google Scholar] [CrossRef]
- Shin, H.; Win, M.Z. Gallager’s exponent for MIMO channels: A reliability-rate tradeoff. IEEE Trans. Commun. 2009, 57, 972–985. [Google Scholar] [CrossRef] [Green Version]
- Gallager, R.G. Information Theory and Reliable Communication; John Wiley and Sons, Inc.: Hoboken, NJ, USA, 1968. [Google Scholar]
- Ahm, W.K.M. Information Theoretic Reliability Function for Flat Fading Channe. Ph.D. Thesis, Queen’s University, Kingston, ON, Canada, 1997. [Google Scholar]
- Ahmed, W.K.M.; McLane, P.J. Random coding error exponents for two-dimensional flat fading channels with complete channel state information. IEEE Trans. Inf. Theory 1999, 45, 1338–1346. [Google Scholar] [CrossRef]
- Kaplan, G.; Shamai, S. Error Exponents And Outage Probabilities For The Block-Fading Gaussian Channel. In Proceedings of the IEEE International Symposium on Personal, Indoor and Mobile Radio Communications, London, UK, 23–25 September 1991; pp. 329–334. [Google Scholar]
- Abou-Faycal, I.; Hochwald, B.M. Coding Requirements for Multiple-Antenna Channels with Unknown Rayleigh Fading; Bell Labs.: Murray Hill, NJ, USA, 2007. [Google Scholar]
- Ebrahimzad, H.; Mohammadi, A.; Khandani, A.K. On the error exponent of MIMO-ARQ system over the fast fading channels. Eur. Trans. Telecommun. 2011, 22, 451–457. [Google Scholar] [CrossRef]
- Xue, J.; Sarkar, M.Z.I.; Ratnarajah, T. Random Coding Error Exponent for OSTBC Nakagami-m Fading MIMO Channel. In Proceedings of the 2011 IEEE 73rd Vehicular Technology Conference, Yokohama, Japan, 15–18 May 2011; pp. 1–5. [Google Scholar]
- Zhang, J.; Matthaiou, M.; Karagiannidis, G.K.; Wang, H.; Tan, Z. Gallager’s Exponent Analysis of STBC MIMO Systems over η-μ and η-μ Fading Channels. IEEE Trans. Commun. 2013, 61, 1028–1039. [Google Scholar] [CrossRef]
- Xue, J.; Ratnarajah, T.; Zhong, C. Error exponents for multi-keyhole MIMO channels. Probl. Inf. Transm. 2015, 51, 19. [Google Scholar] [CrossRef]
- Andrews, L.C.; Phillips, R.L. Laser Beam Propagation through Random Media, 2nd ed.; SPIE Press: Bellingham, WA, USA, 2005. [Google Scholar]
- Humblet, P.A.; Young, J.S. Performance of phase noisy optical systems with frequency stabilization. J. Light. Technol. 1992, 10, 938–946. [Google Scholar] [CrossRef]
- Seimetz, M.; Weinert, C. Options, feasibility, and availability of 2/spl times/4 90/spl deg/hybrids for coherent optical systems. J. Light. Technol. 2006, 24, 1317–1322. [Google Scholar] [CrossRef]
- Deng, P.; Kavehrad, M.; Liu, Z.; Zhou, Z.; Yuan, X. Capacity of MIMO free space optical communications using multiple partially coherent beams propagation through non-Kolmogorov strong turbulence. Opt. Express 2013, 21, 15213–15229. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Gao, C.; Leeson, M.S.; Li, X. Asymptotic analysis of V-BLAST MIMO for coherent optical wireless communications in Gamma-Gamma turbulence. Opt. Express 2018, 26, 27931–27944. [Google Scholar] [CrossRef]
- Chatzidiamantis, N.D.; Karagiannidis, G.K. On the Distribution of the Sum of Gamma-Gamma Variates and Applications in RF and Optical Wireless Communications. IEEE Trans. Commun. 2011, 59, 1298–1308. [Google Scholar] [CrossRef] [Green Version]
- Benkhelifa, F.; Rezki, Z.; Alouini, M. Low SNR Capacity of FSO Links over Gamma-Gamma Atmospheric Turbulence Channels. IEEE Commun. Lett. 2013, 17, 1264–1267. [Google Scholar] [CrossRef] [Green Version]
- Paulraj, A.; Nabar, R.; Gore, D. Introduction to Space-Time Wireless Communications, 1st ed.; Cambridge University Press: Cambridge, MA, USA, 2008. [Google Scholar]
- Zheng, L.; Tse, D.N.C. Diversity and multiplexing: A fundamental tradeoff in multiple-antenna channels. IEEE Trans. Inf. Theory 2003, 49, 1073–1096. [Google Scholar] [CrossRef] [Green Version]
- Prudnikov, A.P.; Bryčkov, Y.A.; Maričev, O.I. Integrals and Series of Special Functions; Science: Moscow, Russia, 1983. [Google Scholar]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products, 7th ed.; Elsevier/Academic Press: Amsterdam, The Netherlands, 2007; p. xlviii+1171. [Google Scholar]
- Glasser, L.; Kohl, K.T.; Koutschan, C.; Moll, V.H.; Straub, A. The integrals in Gradshteyn and Ryzhik. Part 22: Bessel-K functions. Sci. Ser. A Math. Sci. New Ser. 2012, 22, 129–151. [Google Scholar]
- Zhong, C.; Wong, K.; Jin, S. Capacity Bounds for MIMO Nakagami-m Fading Channels. IEEE Trans. Signal Process. 2009, 57, 3613–3623. [Google Scholar] [CrossRef]
- Ando, T. Majorizations and inequalities in matrix theory. Linear Algebra Its Appl. 1994, 199, 17–67. [Google Scholar] [CrossRef] [Green Version]

| SNRdB= 15 dB | Weak Turbulence | Moderate Turbulence | Strong Turbulence |
| Description | |||
| Exact | 435 | 270 | 240 |
| Lower Bound | 342 | 207 | 192 |
| SNRdB= 16 dB | Weak Turbulence | Moderate Turbulence | Strong Turbulence |
| Description | |||
| Exact | 120 | 78 | 75 |
| Lower Bound | 105 | 69 | 63 |
| SNRdB= 17 dB | Weak Turbulence | Moderate Turbulence | Strong Turbulence |
| Description | |||
| Exact | 54 | 36 | 36 |
| Lower Bound | 48 | 33 | 30 |
| Strong Turbulence | Moderate Turbulence | Weak Turbulence | |
| SNRdB | |||
| 14 | 2065 | 800 | 670 |
| 15 | 530 | 250 | 215 |
| 17.5 | 100 | 55 | 50 |
| 20 | 45 | 25 | 20 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Miao, M.; Li, X. Gallager Exponent Analysis of Coherent MIMO FSO Systems over Gamma-Gamma Turbulence Channels. Entropy 2020, 22, 1245. https://doi.org/10.3390/e22111245
Miao M, Li X. Gallager Exponent Analysis of Coherent MIMO FSO Systems over Gamma-Gamma Turbulence Channels. Entropy. 2020; 22(11):1245. https://doi.org/10.3390/e22111245
Chicago/Turabian StyleMiao, Maoke, and Xiaofeng Li. 2020. "Gallager Exponent Analysis of Coherent MIMO FSO Systems over Gamma-Gamma Turbulence Channels" Entropy 22, no. 11: 1245. https://doi.org/10.3390/e22111245
APA StyleMiao, M., & Li, X. (2020). Gallager Exponent Analysis of Coherent MIMO FSO Systems over Gamma-Gamma Turbulence Channels. Entropy, 22(11), 1245. https://doi.org/10.3390/e22111245





