Entropy, Information, and Symmetry: Ordered is Symmetrical
Abstract
1. Introduction
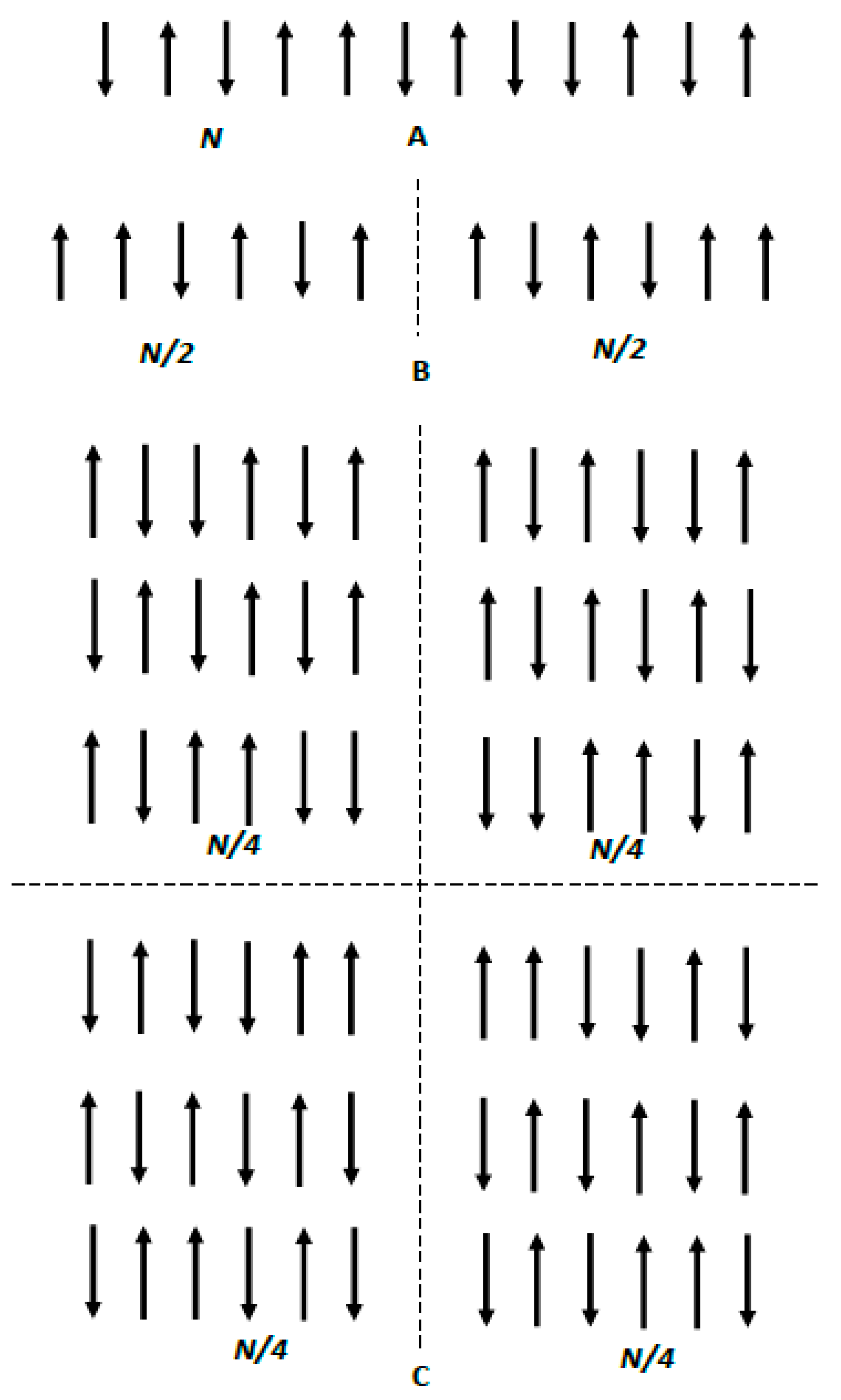
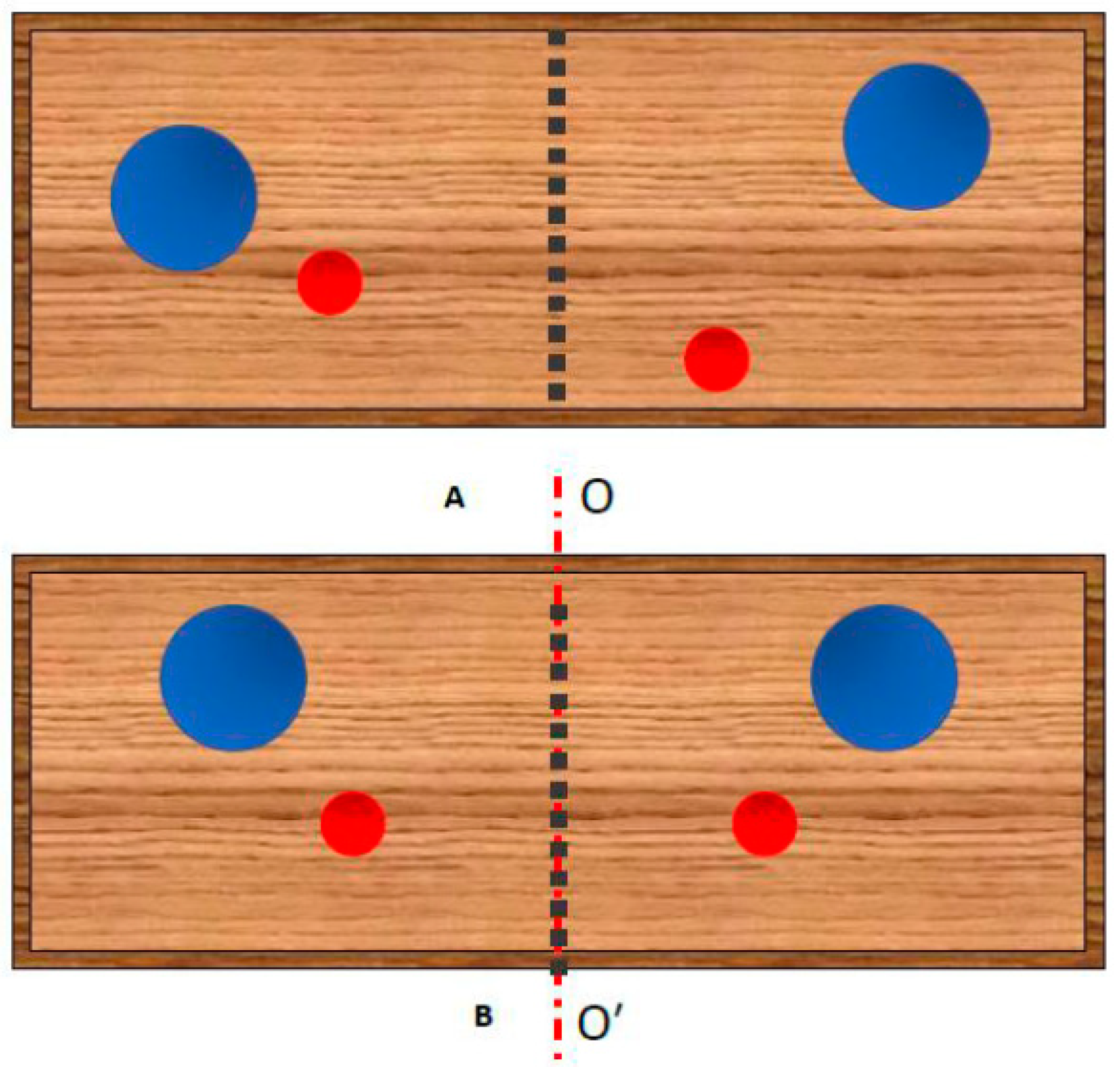
2. Symmetry and Entropy of Binary Systems
3. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Clausius, R. On the Application of the Mechanical theory of Heat to the Steam-Engine. In The Mechanical Theory of Heat—with Its Applications to the Steam Engine and to Physical Properties of Bodies; John van Voorst: London, UK, 1856; 1 Paternoster Row, MDCCCLXVII; pp. 136–207. [Google Scholar]
- Müller, I. A History of Thermodynamics; The Doctrine of Energy and Entropy; Springer: Berlin, Germany, 2007. [Google Scholar]
- Falk, G. Entropy, a resurrection of caloric-a look at the history of thermodynamics. Eur. J. Phys. 1985, 6, 108–115. [Google Scholar] [CrossRef]
- Martin, J.S.; Smith, N.A.; Francis, C.D. Removing the entropy from the definition of entropy: Clarifying the relationship between evolution, entropy, and the second law of thermodynamics. Evol. Educ. Outreach 2013, 6, 30. [Google Scholar] [CrossRef]
- Clausius, R. On Several Convenient Forms of the Fundamental Equations of the Mechanical Theory of Heat. In The Mechanical Theory of Heat with Its Applications to the Steam-Engine and to the Physical Properties of Bodies; Tyndall, J., Translator; John Van Voorst: London, UK, 1867; pp. 327–365. [Google Scholar]
- Gaudenzi, R. Entropy? Exercices de Style. Entropy 2019, 21, 742. [Google Scholar] [CrossRef]
- Baierlein, R. Thermal Physics; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Statistical Physics, 3rd ed.; Elsevier: Oxford, UK, 2011; Course of Theoretical Physics; Volume 5. [Google Scholar]
- Kittel, C. Thermal Physics; J. Wiley & Sons: New York, NY, USA, 1969. [Google Scholar]
- Wright, P.G. Entropy and disorder. Contemp. Phys. 1970, 11, 581–588. [Google Scholar] [CrossRef]
- Styer, D. Entropy as disorder: History of misconception. Phys. Teach. 2019, 57, 454–458. [Google Scholar] [CrossRef]
- Darrigol, O. Number and measure: Hermann von Helmholtz at the crossroads of mathematics, physics, and psychology. Stud. Hist. Philos. Sci. A 2003, 34, 515–573. [Google Scholar] [CrossRef]
- Myszkowski, N.; Storme, M.; Zenasni, F. Order in complexity: How Hans Eysenck brought differential psychology and aesthetics together. Personal. Individ. Differ. 2016, 103, 156–162. [Google Scholar] [CrossRef]
- Shaki, S.; Fischer, M.H.; Petrusic, W.M. Reading habits for both words and numbers contribute to the SNARC effect. Psychon. Bull. Rev. 2009, 16, 328–331. [Google Scholar] [CrossRef] [PubMed]
- Yodogawa, E. Symmetropy, an entropy-like measure of visual symmetry. Percept. Psychophys. 1982, 32, 230–240. [Google Scholar] [CrossRef] [PubMed]
- Zabrodsky, H.; Avnir, D.; Peleg, S. Symmetry as a continuous feature. IEEE Trans. Pattern Anal. Mach. Intell. 1995, 17, 1154–1166. [Google Scholar] [CrossRef]
- Petitjean, M. Chirality and symmetry measures: A transdisciplinary review. Entropy 2003, 5, 271–312. [Google Scholar] [CrossRef]
- Weyl, H. Symmetry; Princeton University Press: Princeton, NJ, USA, 1989. [Google Scholar]
- Van Fraassen, B.C. Laws and Symmetry; Oxford University Press: Oxford, UK, 1989. [Google Scholar]
- Rosen, J. Symmetry in Science: An Introduction to the General Theory; Springer: Berlin, Germany, 1995. [Google Scholar]
- Hall, B.C. Quantum Theory for Mathematicians; Graduate Texts in Mathematics; Springer: Berlin, Germany, 2013. [Google Scholar]
- Chatterjee, S.K. Crystallography and the World of Symmetry; Springer: Berlin, Germany, 2008. [Google Scholar]
- El-Batanouny, M.; Wooten, F. Symmetry and Condensed Matter Physics—A Computational Approach; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Landauer, R. Dissipation and heat generation in the computing process. IBM J. Res. Dev. 1961, 5, 183. [Google Scholar] [CrossRef]
- Landauer, R. Information is physical. Phys. Today 1991, 44, 23–29. [Google Scholar] [CrossRef]
- Landauer, R. Minimal energy requirements in communication. Science 1996, 272, 1914–1918. [Google Scholar] [CrossRef] [PubMed]
- Barthélemy, M. Spatial networks. Phys. Rep. 2011, 499, 1–101. [Google Scholar] [CrossRef]
- Bormashenko, E.; Frenkel, M.; Vilk, A.; Legchenkova, I.; Fedorets, A.; Aktaev, N.; Dombrovsky, L.; Nosonovsky, M. Characterization of self-assembled 2D patterns with Voronoi Entropy. Entropy 2018, 20, 956. [Google Scholar] [CrossRef]
- Fedorets, A.A.; Frenkel, M.; Bormashenko, E.; Nosonovsky, M. Small levitating ordered droplet clusters: Stability, symmetry, and Voronoi Entropy. J. Phys. Chem. Lett. 2017, 8, 5599–5602. [Google Scholar] [CrossRef] [PubMed]
- Bormashenko, E.; Legchenkova, I.; Frenkel, M. Symmetry and Shannon Measure of ordering: Paradoxes of Voronoi tessellation. Entropy 2019, 21, 452. [Google Scholar] [CrossRef]
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bormashenko, E. Entropy, Information, and Symmetry: Ordered is Symmetrical. Entropy 2020, 22, 11. https://doi.org/10.3390/e22010011
Bormashenko E. Entropy, Information, and Symmetry: Ordered is Symmetrical. Entropy. 2020; 22(1):11. https://doi.org/10.3390/e22010011
Chicago/Turabian StyleBormashenko, Edward. 2020. "Entropy, Information, and Symmetry: Ordered is Symmetrical" Entropy 22, no. 1: 11. https://doi.org/10.3390/e22010011
APA StyleBormashenko, E. (2020). Entropy, Information, and Symmetry: Ordered is Symmetrical. Entropy, 22(1), 11. https://doi.org/10.3390/e22010011




