1. Introduction
Past banking regulation has concerned and current banking regulation concerns the measurement of risk needed for capital calculation, which can be computed based on various models. Additionally, banks and financial institutions need to estimate their own exposure to different relevant risk factors in order to understand the overall riskiness of their activities and to help them prepare for undesirable situations. While value-at-risk (VaR) is the most popular risk measure and has been widely used in the past, it is not a subadditive measure (Artzner et al. [
1,
2]) and it is a misleading measure for portfolio optimizers [
3]. Expected shortfall (ES), which has been popularized by Artzner et al. [
1,
2], having the advantage of being a coherent measure [
4], has increased in importance since the Basel Committee on Banking Supervision ([
5,
6]) began to bases its regulatory capital calculations on ES estimates at the 2.5% significance level.
The standard way to estimate ES at level α is to simply compute the weighted average of the historical returns in the α-tail of the returns’ distribution, where the weights are the probabilities associated with the returns estimated from historical data (nonparametric method). However, this often gives an estimate that is inaccurate (see McNeil et al. [
7]), data-sensitive (due to the low number of observations in the tail), and often characterized by an undesirably large variability.
A comprehensive presentation of the modern techniques used in risk management, especially with regard to estimating value-at-risk, expected shortfall, and spectral risk measures, can be found in Guégan et al. [
8].
Several alternatives exist to computing ES, and a comprehensive review can be found in Nadaraj et al. [
9]. Starting with semiparametric methods, a strand of this approach is based on extreme value theory, as in McNeil and Frey [
10], who apply the theoretical framework of Embrechts et al. [
11] and employ the generalized Pareto distribution combined with an ARMA-GARCH(1,1) process. In a later paper, Embrechts et al. [
12] propose an ES estimation method based on the Hill estimator. Another semiparametric ES estimation method, using expectiles, has been proposed by Taylor [
13] based on an asymmetric regression methodology of Aigner et al. [
14] and the CaViaR model of Engle [
15]. A smoothing technique, detailed in Schnabel and Eilers [
16] and based on the algorithm of Schall [
17], can be employed to improve the performance of the ES estimate. Daouia et al. [
18] consider expectile-based ES estimation for extreme percentiles and study their asymptotic behavior.
There are many parametric methods used to estimate ES. Nadaraj et al. [
9,
19] enlist more than 100 approaches, including methods that make different distributional assumptions (Gaussian, Johnson family, Student’s
t, stable distributions, mixture distributions, generalized Pareto and elliptical distributions, etc.)—see also Broda and Paolella [
20]. Many methods employ different econometric formulations (like GARCH, as in a recent paper by Krause and Paolella [
21], or ARMA, or even Bayesian approaches); these methods are mostly applied to historical data, but they can be applied within a Monte Carlo simulation setup as well. All these parametric approaches make an assumption of a particular model, so they bear significant model risk.
Chen [
22] argues that semiparametric or parametric tail-risk estimation models struggle to specify an adequate model for the tail distribution (due to a lack of data), meaning nonparametric models are preferred. In terms of nonparametric ES estimation (which has the advantage of not employing a particular model and hence not being affected by misspecification risk), in addition to the classical historical ES estimation (which is considered the gold standard), the estimator of Yamai and Yoshiba [
3], the estimator of Inui and Kijma [
23], the ES estimator of Chen [
22], and the methodology of Jadhav et al. [
24], which has been shown to perform better than an historical estimator, have all been proposed. Kernel methods based on a kernel-smoothed estimator, as in Scaillet [
25], can be used as well, but as shown by Chen [
22], smoothing negatively affects the accuracy of ES estimates as it introduces a bias and does not reduce the variance of the estimates. To correct this, Alemany et al. [
26] have shown that a double transformed kernel estimator can, in some cases, be a good alternative. Cai and Wang [
27] suggest a nonparametric ES estimator using weighted double kernels and study its finite sample performance. The trimmed kernel method of Hill [
28] is a bias-corrected estimator with a small error only. Another alternative is provided by the method of Richardson [
29] and the algorithm of Fan et al. [
30], which reduce the bias but not the error of the estimation (see Inui and Kijma [
23]). A two-stage estimation method based on extreme value theory has been proposed by Martins-Filho et al. [
31]. In this paper we propose a nonparametric estimator based on tail entropy (TE) and compare it with alternatives.
As entropy is a measure of uncertainty, which is in some ways similar to volatility, it is widely used in physics and the social sciences. In general, the higher the uncertainty of a system, the larger its entropy. The central theme of this paper is risk, which is directly linked to the tail of the distribution of returns (as opposed to its full distribution), so it makes sense to compute entropy based solely on returns that determine risk (that is, the tail of the distribution), which then gives tail entropy, as discussed in detail in this paper. Roughly speaking, tail entropy is defined as the weighted sum of log probabilities in the tail, where the weights are the conditional tail probabilities themselves.
Furthermore, it has to be noted that there is a strong similarity between definitions of ES and tail entropy. While ES computes the weighted average of tail returns, tail entropy calculates the weighted average of the log probabilities of the returns (again, in the tail of the distribution), but the two calculations use the same weights (probabilities). As such, tail entropy is an estimate of tail risk, which is free of the main problem with ES, namely, its direct dependence on the actual observations in the tail, hence the motivation of our paper. Our aim was to study how tail entropy can be used as an efficient measure of risk and how it compares with alternative estimates of ES.
It is natural to compare the theoretical and empirical properties of entropy with those of variance or volatility, with both being measures of uncertainty. While volatility is often considered a measure of risk in finance, here we consider it a measure of uncertainty. To differentiate between the two types of measures, i.e., measures of risk and measures of uncertainty, measures that are symmetric by nature are measures of uncertainty, and (asymmetric) tail measures are considered measures of risk. Thus, tail entropy is a measure of risk. Philippatos and Wilson in 1972 [
32], and, later, Ebrahimi et al. [
33] suggested that entropy compares favorably to volatility/variance as a measure of uncertainty. The same has been shown by Dionisio et al. [
34], with the authors arguing that while variance measures the concentration around the mean, entropy measures the dispersion of the density irrespective of the location of the concentration (also see Ebrahimi et al. [
35] and Allen et al. [
36]). Moreover, the entropy of a distribution function is strongly related to its tails, as shown by Pele et al. [
37], which becomes an important feature for distributions with heavy tails or with an infinite second-order moment for which an estimator of variance is obsolete. Liu et al. [
38] compare an entropy-based measure with the classical coefficient of correlation and conclude that their measure has certain superior characteristics as a descriptor of the relationship between time series when compared with the correlation measure.
The study of the relationship between entropy and financial markets goes back more than a decade. Entropy has been used as a measure of stock market efficiency in Zunino et al. [
39], while Risso [
40] links it to stock market crashes, arguing that the probability of having a crash increases as entropy decreases. Oh et al. [
41] use entropy as a measure of the relative efficiency of the foreign exchange (FX) markets. Based on generalized entropy theory, Wang et al. [
42] analyze the interactions among agents of stock markets. Entropy has also been used as a tool for the predictability of stock market returns. Maasoumi and Racine [
43] show that entropy can capture nonlinear dependence between financial returns. An effective early warning indicator for crisis situations of banking systems has been built by Billio et al. [
44]; this indicator considers different definitions of entropy.
Entropy has also been used in option pricing (Stutzer [
45] and Stutzer and Kitamura [
46]). An application of entropy-based risk measures for decision-making can be found in Yang and Qiu [
47]. Applications of Tsallis entropy in risk management can be found in Gradojevic and Gencay [
48], Gencay and Gradojevic [
49], and Gradojevic and Caric [
50]. Bowden [
51] introduces directional entropy and uses it to improve the performance of VaR in capturing regime changes. Portfolio optimization based on maximum entropy has been discussed by Geman et al. [
52].
The main objectives of this paper were to introduce a measure for expected shortfalls based on tail entropy, to study this measure’s properties, and to compare it with alternative measures of ES. The main advantage of the measure we propose is that it is less sensitive to the actual values of observations in the tail of the distribution (compared to historical ES), making it a more stable measure of tail risk. We use several ES backtests to verify the accuracy of the proposed measure. The rest of the paper is organized as follows.
Section 2 derives the theoretical results specifying the steps in estimating tail entropy as a measure of market risk and details our methodology,
Section 3 presents an empirical application, and
Section 4 concludes.
2. Methodology
For a series of asset prices denoted by
St, in the following we compute and denote log-returns by
X with realizations
, i.e.,
2.1. Measures of Risk: VaR and ES
Given the returns series
X, with
F denoting its cumulative density (or distribution) function, for an
the VaR at level
α is the smallest number with cumulative density at least as big as α, i.e.,
In probabilistic terms we can write that
In addition, VaR is the negative of the quantile, i.e.,
The ES at significance level
α is the average of the returns below the VaR at level
α, as given by
For a continuous variable with density function
f, the above definition for ES can be rewritten as
where
is the left α-quantile.
2.2. Entropy
Entropy is a measure of uncertainty, which is in some ways similar to volatility. Various definitions exist (e.g., Shannon entropy, Tsallis entropy, and Kullback Cross entropy etc.) based on the informational content of a discrete or continuous random variable (see Zhou et al. [
53] for a comprehensive review of entropy measures used in finance). Shannon information entropy is the most commonly used definition of entropy; it quantifies the expected value of the information contained in a discrete distribution.
Definition 1 (Shannon information entropy). For X, a discrete random variable with probability distribution , with , , and , the Shannon information entropy is defined as For a discrete distribution, the entropy reaches its maximum value of for the uniform distribution when all the pi values are the same (i.e., when there is a high level of uncertainty). Similarly, the entropy will reach its minimum value of 0 for a distribution with zero uncertainty (i.e., one of the probabilities pi is 1 and the rest are all 0).
For X, a continuous random variable with probability density function
, the differential entropy is given by
However, unlike with Shannon entropy, differential entropy does not possess certain desirable properties like invariance to linear transformations and non-negativity (Lorentz [
54] and Pele [
55]). A measure of entropy similar to Shannon entropy can be defined via a transformation called quantization, as defined below [
54].
Definition 2 (sampled function). For , a real valued continuous function, , a fixed number, and , , where , the sampled function for is given by (point sampling) For,
which is essentially bounded, the sampled function is (mean sampling) Definition 3 (quantization). The quantization of a function creates a simple function that approximates the original one. Given q > 0, a quantum, the following function defines a quantization of.
Definition 4 (entropy of a function at quantization level q). Let be a measurable and essentially bounded real valued function defined on [a,b] and let q > 0. Let and . Then, the entropy of at quantization level q is given bywhere μ denotes the Lebesgue measure.
Lorentz’s theorem given below calculates the entropy of a continuous function on a compact interval. Eventually, it helps define the entropy of a probability distribution function for a continuous random variable on a compact interval, regardless of the sampling and quantization.
Theorem (Lorentz [54]). Let f be continuous for point sampling, measurable, and essentially bounded for mean sampling. The sampling spacing is 1/n. Let be the corresponding sampling; fix q > 0 and let be the quantization of the samples with resolution q as in Definition 3. The number of occurrences of in is and the relative probability of the occurrence of the value i is denoted by . Then, we have In the following we assume that we are dealing with a continuous random variable X whose support is the set A with the distribution function . If the distribution function is absolutely continuous, then there exists a non-negative, Lebesgue integrable function f which is a probability density function (PDF), i.e.,
- (i)
;
- (ii)
;
- (iii)
.
Assuming that the probability density function f is essentially bounded, we can define the entropy of the probability density function Hq(f) at the quantization level q > 0.
Definition 5 (entropy of a probability density function at quantization level q). For a probability density function which is essentially bounded, let disjoint giving and not necessarily disjoint, with . Then, the entropy of at quantization level q is , where is the Lebesgue measure.
Of note is that in general, for a probability density function defined on set A not necessarily of finite measure, it is possible to consider this function’s restriction on a compact interval, i.e.,
Then, satisfies the conditions of Lorentz’s theorem, meaning the entropy can be defined.
The above framework can be applied to estimation of the entropy of a probability density function of a continuous random variable X.
Next, we present the estimation algorithm of the entropy of a probability density function. Let
be a sample of an
i.i.d. variable with probability density function
f. The Algorithm 1 (see below) estimates the entropy of a probability density function (see Pele et al. [
37]).
| Algorithm 1. Estimation of the entropy of a probability density function. |
Estimate the probability density function, obtaining values for ; Sample from the probability density function using the sampled function for ; Define a quantum ; then , if ; Compute the probabilities ; Estimate the entropy of the probability density function, i.e.,
|
A similar approach can be found in Miśkiewicz [
56], where two possible algorithms for estimating entropy of a continuous probability density function are discussed.
The entropy of the probability density function reaches its maximum value for the uniform distribution. A dimensionless measure of uncertainty, normalized entropy, can be defined as the ratio between the entropy of the probability density function and the entropy of the uniform distribution, i.e.,
In the following we refer to the entropy of the probability density function as the normalized entropy of the probability density function, i.e., .
2.3. Tail Entropy
Similarly to the definition of entropy, we can define tail entropy as being when entropy is computed using observations in the tail only. Shannon tail entropy is defined below.
Definition 6 (Shannon tail entropy). For X, a discrete random variable with probability distribution , with , , and , and α-level value-at-risk for 0 < α < 1, the Shannon tail information entropy at level α is defined by The tail-adjusted probabilities are denoted by , and this normalization is required to make sure that the probabilities in the tail add up to 1. For a discrete distribution, the TE will reach its maximum value for a distribution which has uniform distribution in the α-tail, with all tail observations having the same probability. Similarly, for a given α, the tail entropy will reach its minimum for a distribution with zero uncertainty in the tail (an observation in the tail has a probability of pi = α and the rest of the tail observations have a probability of 0).
For X, the discrete random variable given above, we can compute the expected shortfall based on the variable’s probability distribution at level α; this is denoted by ESPD and given by
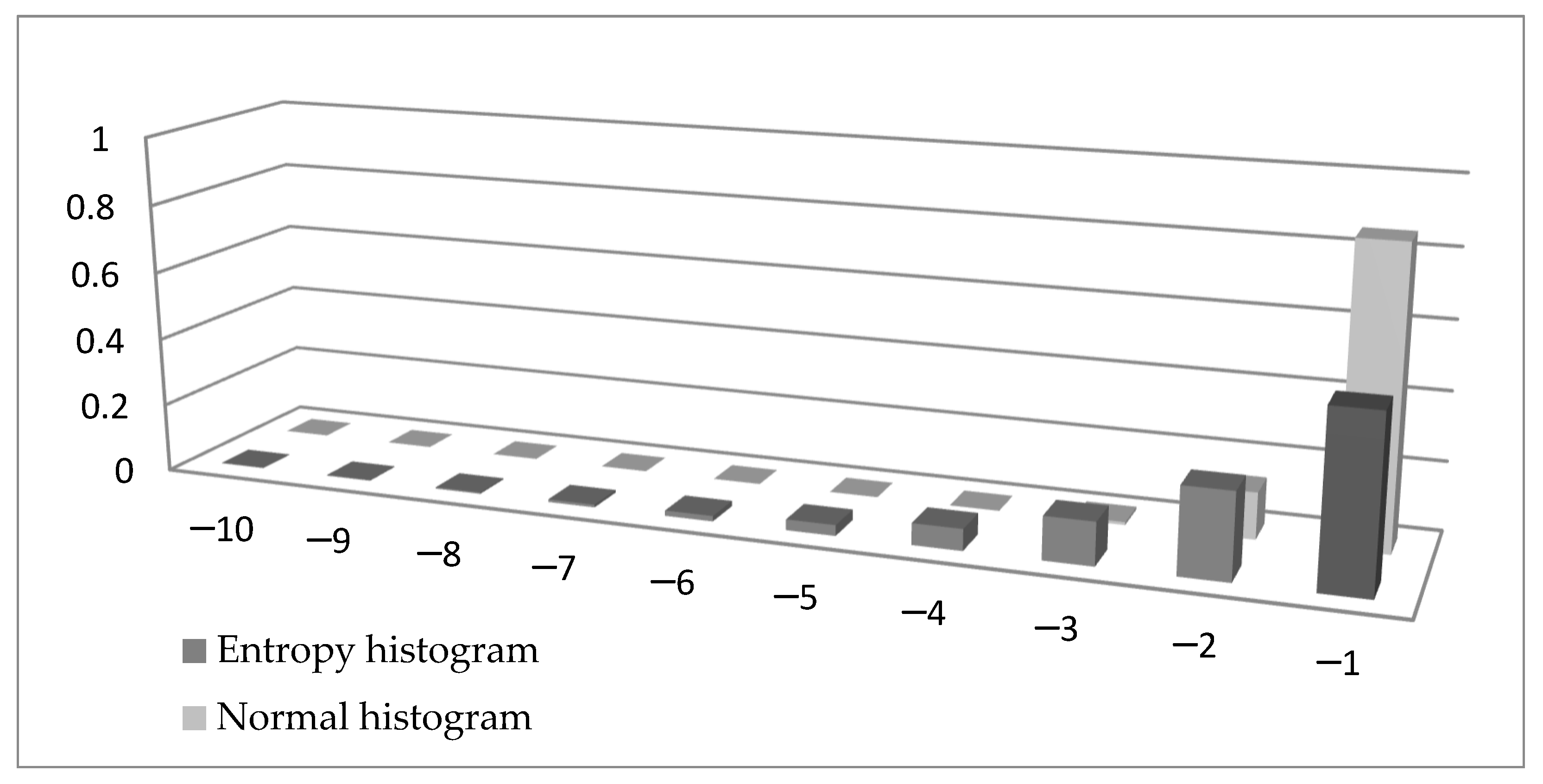
The similarity between Formulas (15) and (16) is obvious. There is a minus sign in Formula (15) to make sure the tail entropy is a positive measure. More importantly, while the entropy is a weighted sum of log probabilities, the ES is the weighted sum of observations in the tail. To visualize the difference between the two formulae, in
Figure 1 we compare the left tail of a histogram of a variable distributed N (0,1) (normalized with the tail probability and using bins of width 1) with the left tail of a histogram built using the entropy measure. In Formula (15) we can denote
by
xi, giving
, and this probability is presented in
Figure 1 in the entropy histogram. It can be seen that the probabilities calculated using entropy are higher (except for when using the value −1) than the probabilities in the normal histogram.
For a continuous random variable with density function,
the differential tail entropy is given by Similarly to differential entropy, tail differential entropy does not possess the properties of invariance to linear transformations and non-negativity. Using quantization, a measure of entropy similar to Shannon tail entropy can be defined as follows.
Definition 7 (tail entropy of a probability density function at quantization level q). For the probability density function , let disjoint giving and not necessarily disjoint, with . Then, the tail entropy of f at level α with quantum q (using the notation * for the Lebesgue measure divided by α and [.] for the integer part of a number) is Of note is that as in Definition 5, for a probability density function defined on set A which is not necessarily of finite measure, we can consider its restriction on a compact interval, i.e.,
The tail entropy of the returns’ distribution can be interpreted as the expected shortfall of the returns, if in the tail of the distribution the probability of losses can be expressed as an exponential function of the size of the losses. The tail entropy is a measure of uncertainty in the tail, with low values of tail entropy being associated with a lower risk in the tail.
This framework can be used to estimate the tail entropy of a distribution function of a continuous random variable X, defined on the support set of X, with values on [0, 1]. The distribution function can be estimated using the histogram estimator of a probability density or kernel density estimation methods as described in the previous section. We proceed by presenting an estimation algorithm of the tail entropy of a distribution function.
Let
be a sample of an
i.i.d. variable with probability density function
f. The Algorithm 2 (see below) estimates the tail entropy of a distribution function.
| Algorithm 2. Estimation of the tail entropy of a distribution function. |
Estimate the probability density function, obtaining values for ; Sample from the probability density function using the sampled function for ; Define a quantum ; then , if ; Compute the probabilities ; Estimate the tail entropy of F as where .
|
The tail entropy of a distribution function reaches its maximum value for a distribution which has uniform distribution in the α-tail.
Property (maximum value of the tail entropy of a probability density function): The α-tail entropy of a distribution function reaches its maximum for a distribution with F(x) = x for x ≤ α, and this maximum value is .
Proof. This is similar to the proof of the maximum Shannon entropy. Indeed, when all probabilities are equal for the observations in the α-tail (= 1/
m), the tail probability can be written as:
□
As such, a dimensionless measure of uncertainty, the normalized tail entropy, can be defined as the ratio between the entropy of the probability density function and its maximum value, i.e.,
We refer below to the tail entropy of the probability density function as the normalized tail entropy of the probability density function: .
In practical applications, the estimated tail entropy may be severely biased for small samples (Liu et al. [
57]). In order to correct for small sample bias we propose a bootstrapping method, following DeDeo et al. [
58]. Thus, we generate
B independent samples of volume
k from a multinomial distribution with probabilities
, and for each sample we estimate the normalized tail entropy
. The unbiased estimator of the normalized tail entropy has the form
The estimated entropy can be influenced by the quantum value
q > 0; as there is no canonical quantum/level of quantization, apparently all results depend on an arbitrarily chosen quantum/quantization level. However, the Lorentz theorem ([
54]) shows that the entropy estimator is consistent regardless of the choice of
q; in practical applications, such as stock market data, we have used
q = 0.2 (see
Section 3). For
q = 0.2, the number of bins used to compute the tail entropy is 1/
q = 5; a smaller value of the quantum
q will increase the number of bins and also the likelihood of having zero probability bins. For example, when the window used for estimation has 1000 observations (roughly 4 years of daily observations) and α = 5%, there are 50 observations in the left tail of the distribution; by decreasing the quantum level and increasing the number of bins, there is a high chance of having bins with zero probability where the term
is undefined.
Unlike expected shortfall, which is not well-defined when the expectation fails to exist (for instance for the Pareto family when the parameter
a ≤ 1), tail entropy does exist for the Pareto distribution for any tail parameter value
a. In general, under the hypothesis of the Lorentz theorem ([
54]), tail entropy exists for any probability density function with bounded support. Even if the probability density function is not bounded, we can consider its restriction on a compact interval in order to fulfil the conditions of the Lorentz theorem. Indeed, tail entropy is sensitive to the choice of the level of the quantum, but in the next section we show how it can be transformed (via normalizing it and using a linear adjustment) into an expected-shortfall-type measure which will be less sensitive to the choice of quantum.
2.3.1. Tail Entropy Expected Shortfall
Next, we turn our attention to the link between tail entropy and measures of risk and uncertainty, seeking to propose a tail-entropy-enhanced ES estimator. First, we perform a Monte Carlo experiment, estimating and comparing tail entropy and historical expected shortfall for several simulated Pareto-like distributions; we report our results for different tail probabilities. Here we use the random variable Y = −X~Pareto(a), which has the following characteristics:
- (i)
Cumulative distribution function: .
- (ii)
Probability density function: .
- (iii)
.
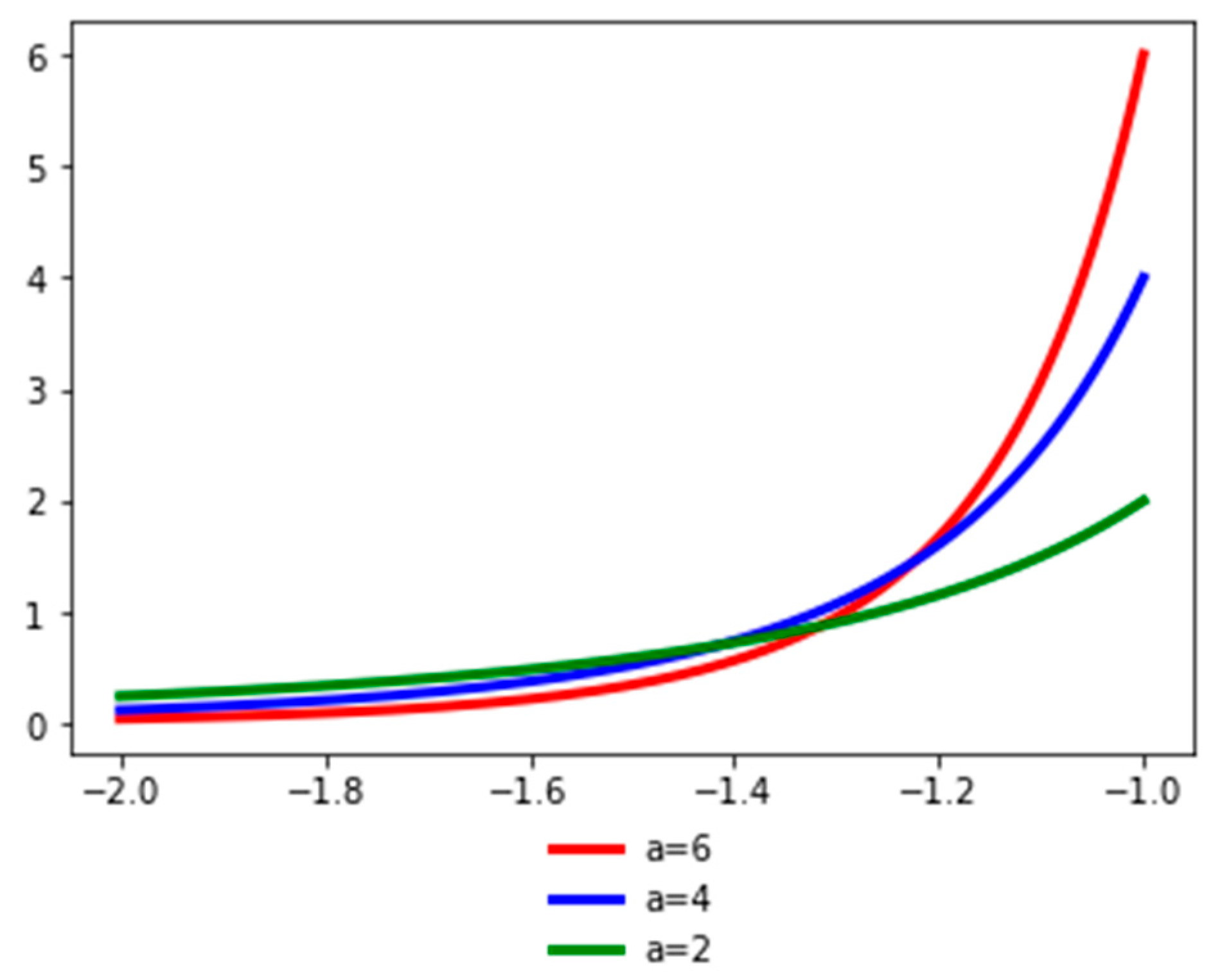
Figure 2 presents the probability density function of the random variable
Y for different values of the
a parameter. The
a parameter (the tail index) is a measure of tail probability: higher values of
a correspond to lower probability in the tail.
In order to assess the relationship between the tail entropy and the historical expected shortfall (ESH), we simulate random variables
, with
.
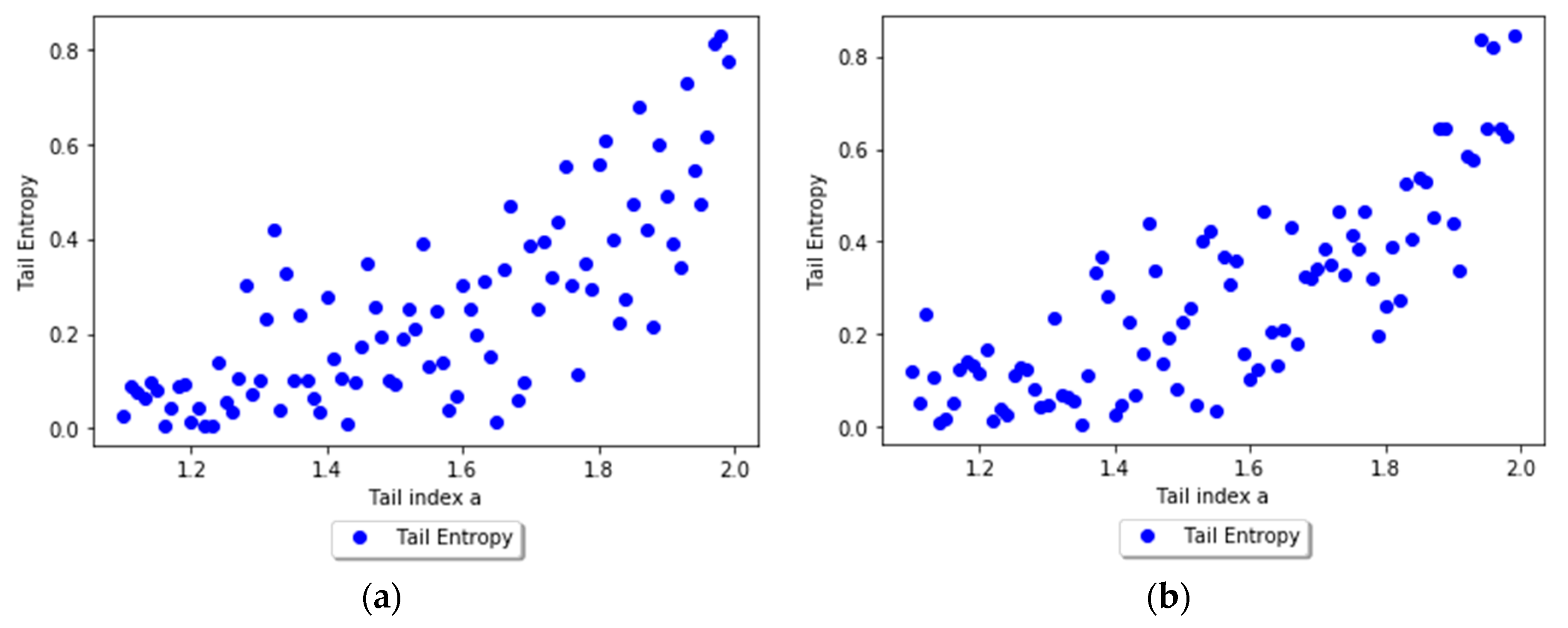
Figure 3 presents the relationship between TE and tail index
a using scatterplots for different levels, i.e., 1% and 2.5%, of
α. As the
a parameter of the simulated distribution decreases, the tail entropy of the distribution decreases, too; as expected, high tail entropy values are associated with heavy-tailed distributions.
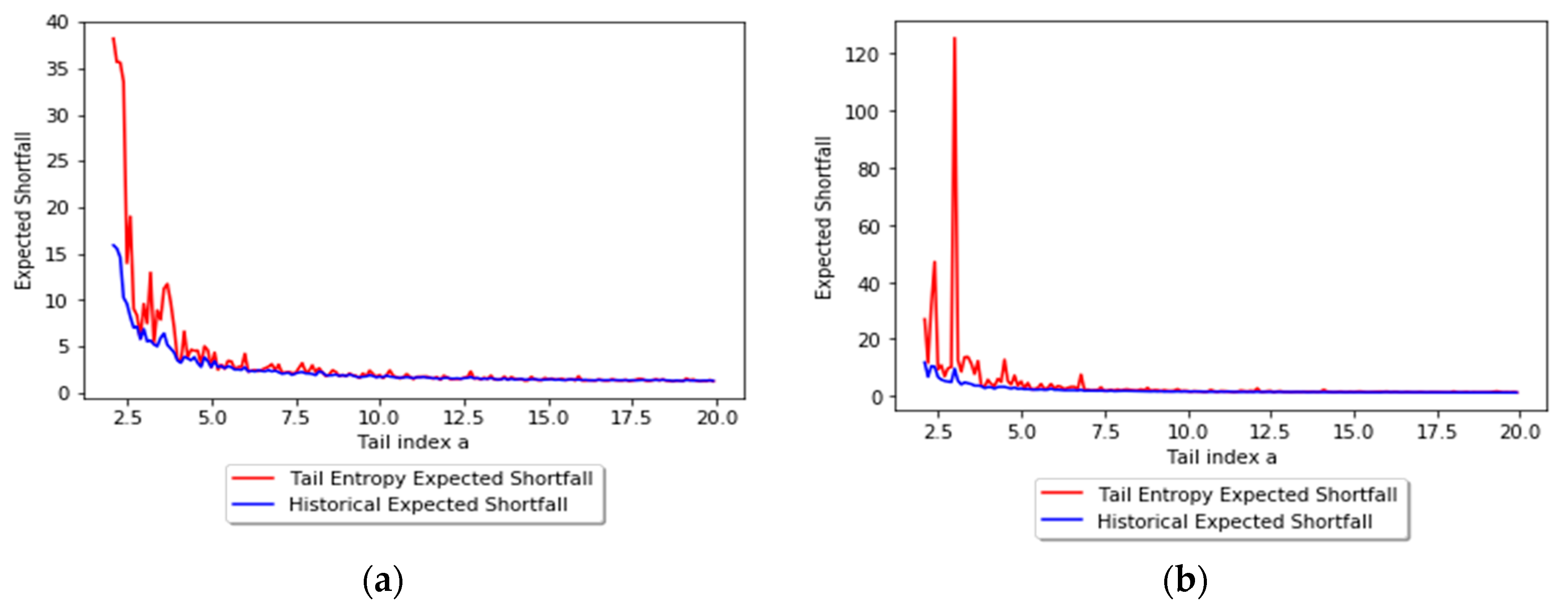
Figure 4 presents the relationship between TE and historical ES, as functions of the
a parameter of the simulated distribution, for 1% and 2.5% levels of
α.
Additionally, we regress the historical ES estimates of the simulated returns above on tail entropy; the results are reported in
Table 1. We estimate the historical ES using the formula
It can be seen that there is a strong linear negative relationship between the tail entropy and ESH, with higher values of tail entropy corresponding to lower values of expected shortfall. The validity of this regression approach depends on the tail behavior of the underlying distribution; without this explicit assumption, one would need regularly varying tails and a particular tail behavior in order to make the regression approach sound. Indeed, for some distributions the relationship between historical ES and tail entropy might not be exactly linear, but we show below how assuming a linear relationship helps us define a new measure of expected shortfall.
A multiple regression with several tail entropies of varying tail levels was also estimated in order to explicitly assess the relationship between historical ES and TE for a Pareto-like simulated distribution, as in the above examples.
Thus, we estimated the following regression model, where the historical ES (1% and 2.5%) is regressed against the tail entropy for significance levels 1%, 2.5%, and 5%, i.e.,
The results of the regression as calculated using Formula (23) are reported in
Table 2. By including varying tail levels for entropy, the performance of the model is significantly improved, with
increasing for α = 1% from 51% (see
Table 1, left panel) to 55%, and for α = 2.5% from 48% (see
Table 1, right panel) to 60%.
A second simulation using α-stable distributions can help understand this behavior. We chose α-stable distributions because they have several interesting properties: they allow for heavy tails and any linear combination of independent stable variables follows a stable distribution for up to a scale and location parameter (Nolan [
59]); additionally, the Gaussian distribution is a particular case of a stable distribution.
We have that a random variable
X which follows an α-stable distribution
if its characteristic function is
In the above expression is the tail index (we obtain for a normal distribution and lower values correspond to heavier tails), is the skewness parameter, is the scale parameter, and is the location parameter. We simulate 1000 observations from an α-stable distribution for and study the relationship between tail index and tail entropy.
The tail entropy reaches its maximum value for a distribution that has uniform distribution in the tail, and as the
a parameter decreases, the tail entropy of the stable distribution decreases, too. As expected, low tail entropy values are associated with heavier-tailed distributions.
Figure 5 presents the relationship between TE and tail index, while
Table 3 present the regression results between TE and tail index.
Based on the results above (and taking into consideration that the tail entropy is between 0 and 1), we perform a linear adjustment on the tail entropy to obtain the tail entropy expected shortfall, i.e.,
where
b0 is the mid-point of the first bin
B0 and
bm is the mid-point of the last bin in the α-tail of the distribution
Bm.
The justification for Formula (25) lies in the following points.
If the entropy reaches its maximum (), which corresponds to the highest uncertainty level, then we have , i.e., the tail entropy expected shortfall is the average of losses below the value-at-risk.
If the entropy reaches its minimum (), which corresponds to the lowest uncertainty level, then we have , i.e., the tail entropy expected shortfall is the minimum value of the distribution.
2.3.2. Tail Entropy Expected Shortfall as a Spectral Risk Measure
According to Guégan et al. [
8], a spectral risk measure can be defined as the function
, where
is a functional space and
In Formula (26) is a positive or null, non-increasing, right-continuous, integrable function defined on [0, 1] such as .
Following Formula (25), the tail entropy expected shortfall can be written as a linear function of the tail entropy (even though for some distributions this is only an approximation), i.e.,
where
and
.
Thus, according to Formula (27), the tail entropy expected shortfall is a spectral risk measure and satisfies the conditions of coherence (see Artzner [
1,
2]), any spectral risk measure being law-invariant and comonotonic additive (see [
8]).
The tail entropy expected shortfall can have interpretability in terms of monetary units, being a linear combination of the expected shortfall and the adimensional normalized entropy which takes values between [0,1], as can be seen from Formula (27).
2.3.3. Backtesting ES
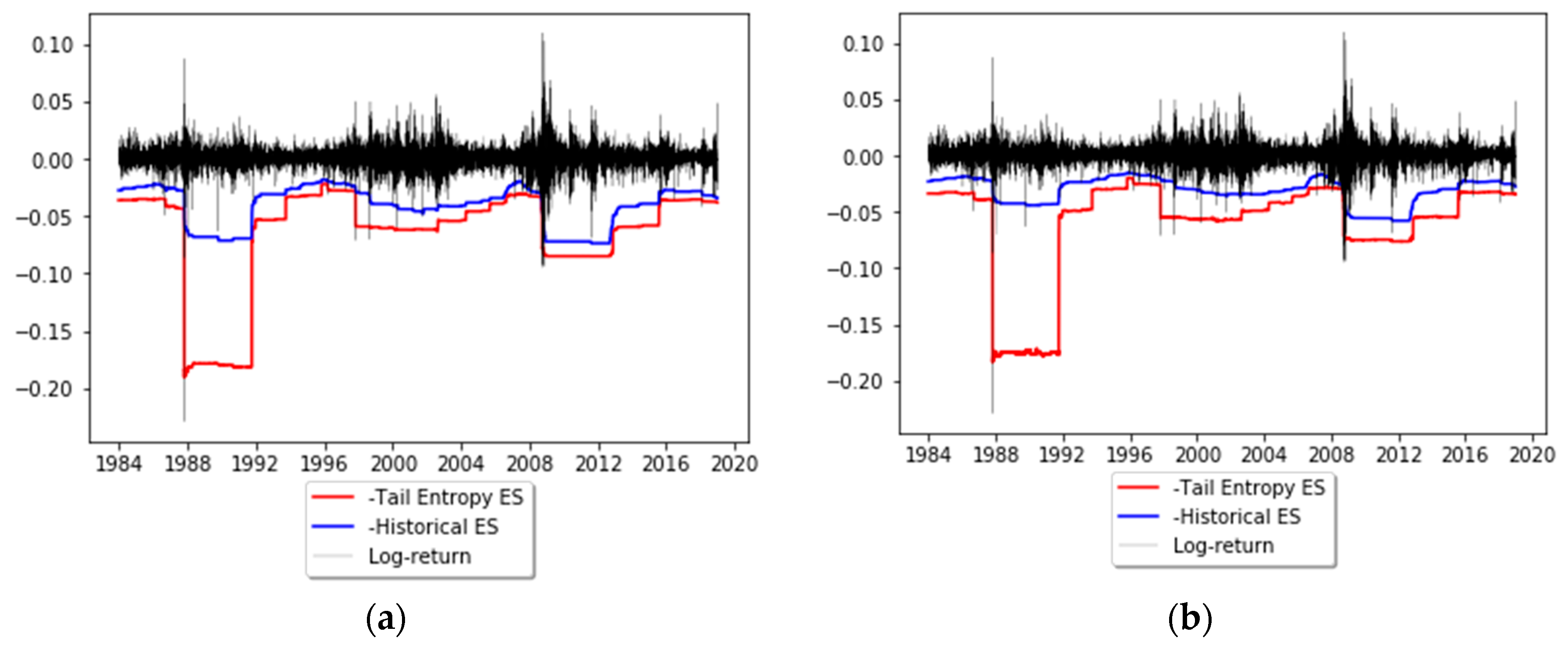
In order to forecast daily ES, non-parametric and parametric models are used based on a rolling window approach. Thus, we compare the ES forecasting ability of the following six models.
Historical ES forecasts: , where is the α-quantile of the daily log-returns distribution;
Tail entropy ES forecasts: ;
Normal GARCH(1,1) ES forecasts: , where , being the Gaussian quantile;
Student’s t GARCH(1,1) ES forecasts: , where , being the Student’s t quantile with df being the estimated degrees of freedom;
Gaussian ES forecasts: , where is the α-quantile of the estimated Gaussian distribution of log-returns;
Student’s t ES forecasts: , where is the α-quantile of the estimated Student’s t distribution of log-returns.
In the above formulae, every risk measure is estimated using a rolling window of length
w, with
,
, and
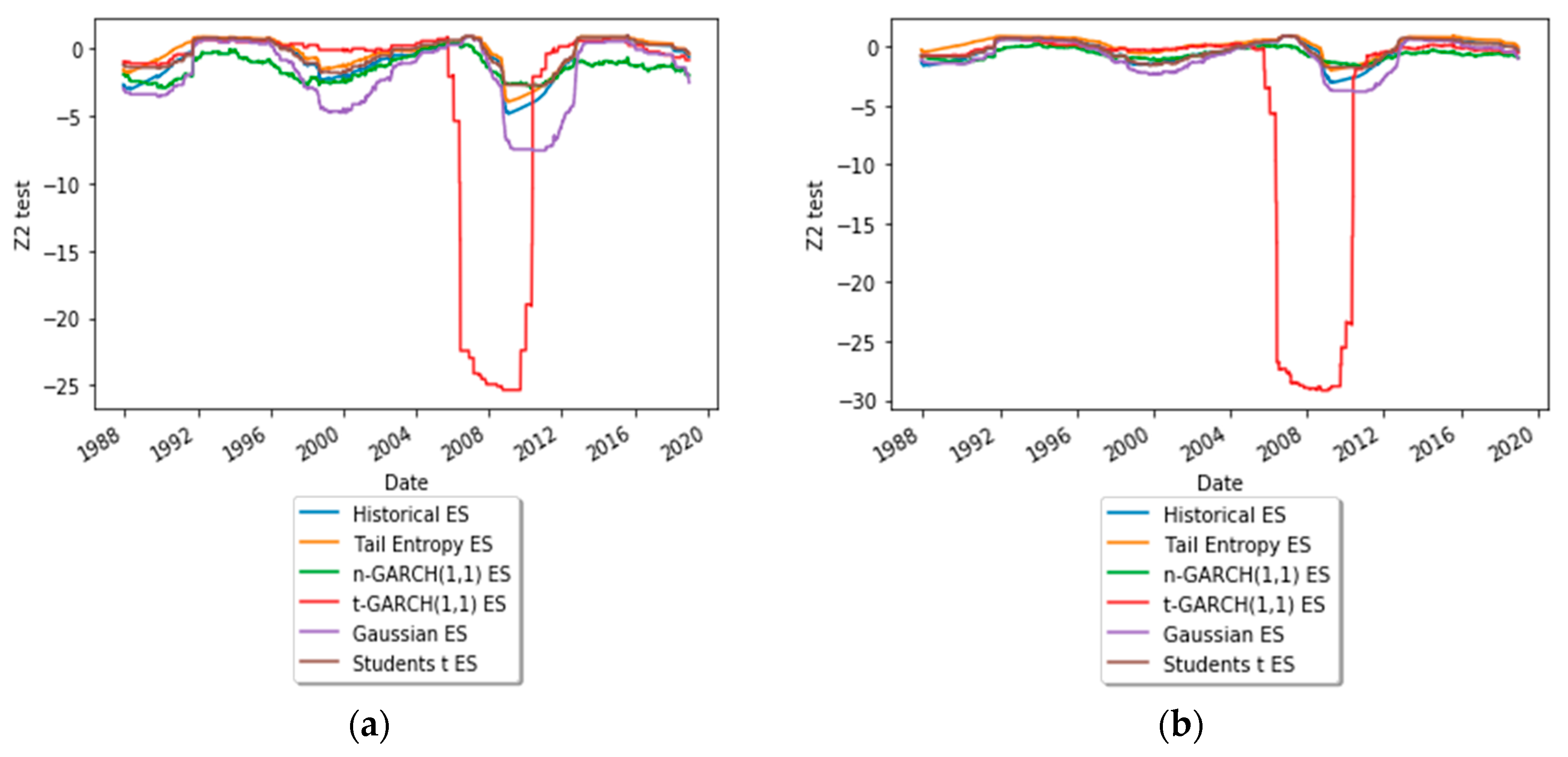
T the number of daily log-returns. To see the ES forecasting performance of the above models, we run several backtests to compare the ES estimates. These are the unconditional exception frequency (UC) test and the conditional independence (CC) test of Du and Escanciano [
60], and the
exception frequency and magnitude test of Acerbi and Szekely [
61]. These are described in
Appendix A and
Appendix B.