Ergodic Rate for Fading Interference Channels with Proper and Improper Gaussian Signaling
Abstract
1. Introduction
1.1. Related Work
1.2. Contribution
- We derive closed-form expressions for the achievable ergodic rate of the PGS and IGS users. We also characterize the achievable ergodic rate region of the mixed PGS/IGS scheme by allowing power control.
- Our proposed scheme incurs no additional overhead at the transmitter side when both users transmit at maximum power. Moreover, in order to operate at a specific Pareto-optimal point, the transmitters (or a central entity implementing the power control module) should be informed about the average gain of the direct and interference links, which are scalar values and can be easily sent to the transmitters. Hence, the PGS/IGS scheme is simple to implement in practice.
- Through numerical examples, we show that our proposed PGS/IGS scheme substantially outperforms the PGS scheme in moderate and strong interference regimes. Moreover, we analyze the maximum sum-rate point for the symmetric 2-user IC when both users transmit at maximum power. Under this condition, the sum-rate point is attained either with PGS or with PGS/MIGS (maximally IGS); that is, one user transmits proper signals while the other user employs maximally improper signaling. Then, the maximum sum-rate point does not require any optimization and/or power control.
- We also compare our results with the more general scheme where both users can employ IGS and their transmission parameters are numerically optimized. Our numerical results show that the union of the PGS and PGS/MIGS schemes with time sharing attains almost the whole Pareto-optimal points in the rate region and little is gained by allowing both users to employ IGS.
1.3. Paper Outline
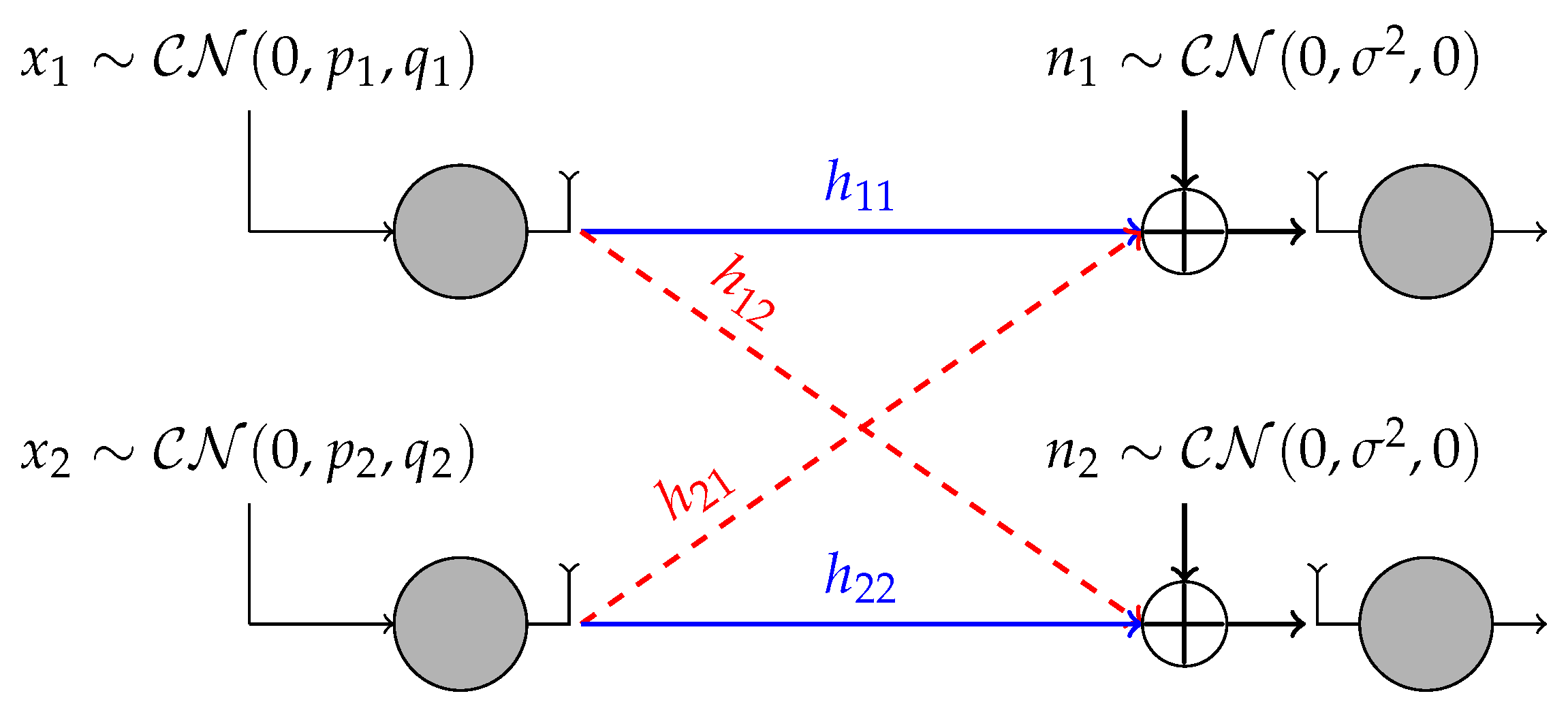
2. Signal Model and Problem Statement
2.1. Notation and Preliminaries
2.2. Signal Model
2.3. Problem Statement
3. PGS/IGS Scheme
- User 1 employs PGS, while user 2 may employ IGS,
- User 2 employs PGS, while user 1 may employ IGS.
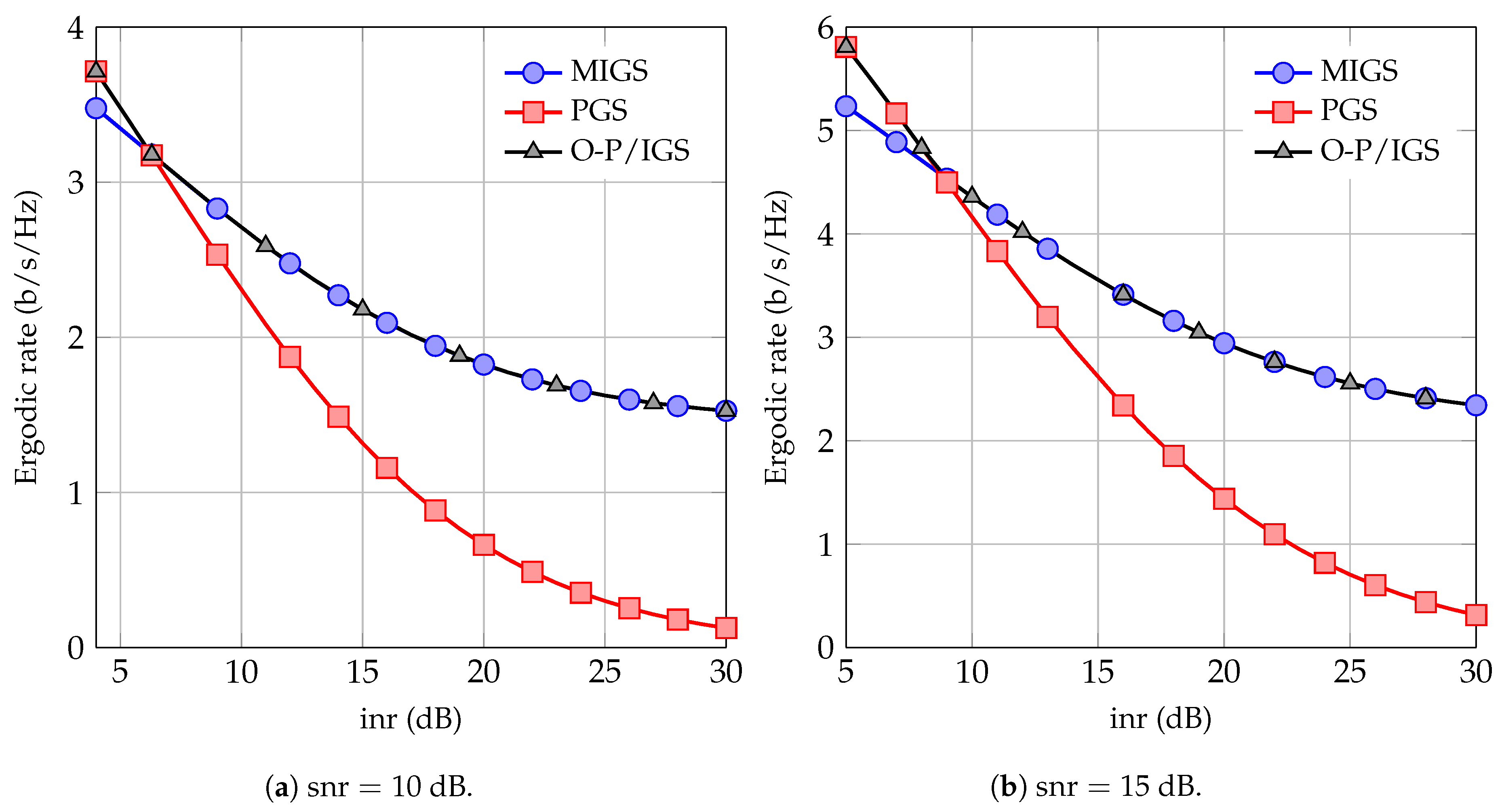
4. Numerical Examples
- PGS: The PGS scheme with .
- MIGS: The PGS/MIGS scheme with .
- E-IGS: The exhaustive search for IGS when both users may employ IGS.
- O-P/IGS: The PGS/IGS scheme with the optimal .
- MIGS-U: The PGS/MIGS scheme when the MIGS user is user i.
- MIGS-TS: The PGS/MIGS scheme with time sharing over the points with maximum power for both users.
5. Conclusions
Future Studies
Author Contributions
Funding
Conflicts of Interest
Appendix A. Proof of Lemma 1
References
- Andrews, J.G.; Buzzi, S.; Choi, W.; Hanly, S.V.; Lozano, A.; Soong, A.C.; Zhang, J.C. What will 5G be? IEEE J. Sel. Areas Commun. 2014, 32, 1065–1082. [Google Scholar] [CrossRef]
- Cover, T.M.; Thomas, J.A. Elements of Information Theory; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Cadambe, V.R.; Jafar, S.A.; Wang, C. Interference alignment with asymmetric complex signaling–Settling the Høst-Madsen-Nosratinia conjecture. IEEE Trans. Inf. Theory 2010, 56, 4552–4565. [Google Scholar] [CrossRef]
- Lameiro, C.; Santamaria, I. Degrees-of-Freedom for the 4-User SISO Interference Channel with Improper Signaling. In Proceedings of the 2013 IEEE International Conference on Communications (ICC), Budapest, Hungary, 9–13 June 2013. [Google Scholar]
- Yang, L.; Zhang, W. Interference alignment with asymmetric complex signaling on MIMO X channels. IEEE Trans. Commun. 2014, 62, 3560–3570. [Google Scholar] [CrossRef]
- Lameiro, C.; Santamaria, I.; Schreier, P.J. Benefits of improper signaling for underlay cognitive radio. IEEE Wireless Commun. Lett. 2015, 4, 22–25. [Google Scholar] [CrossRef]
- Lameiro, C.; Santamaria, I.; Schreier, P.J. Rate region boundary of the SISO Z-interference channel with improper signaling. IEEE Trans. Commun. 2016, 65, 1022–1034. [Google Scholar] [CrossRef]
- Kurniawan, E.; Sun, S. Improper Gaussian signaling scheme for the Z-interference channel. IEEE Trans. Wirel. Commun. 2015, 14, 3912–3923. [Google Scholar] [CrossRef]
- Lagen, S.; Agustin, A.; Vidal, J. On the superiority of improper Gaussian signaling in wireless interference MIMO scenarios. IEEE Trans. Commun. 2016, 64, 3350–3368. [Google Scholar] [CrossRef]
- Ho, Z.K.; Jorswieck, E. Improper Gaussian signaling on the two-user SISO interference channel. IEEE Trans. Wirel. Commun. 2012, 11, 3194–3203. [Google Scholar] [CrossRef]
- Zeng, Y.; Yetis, C.M.; Gunawan, E.; Guan, Y.L.; Zhang, R. Transmit optimization with improper Gaussian signaling for interference channels. IEEE Trans. Signal Process. 2013, 61, 2899–2913. [Google Scholar] [CrossRef]
- Park, H.; Park, S.-H.; Kim, J.-S.; Lee, I. SINR balancing techniques in coordinated multi-cell downlink systems. IEEE Trans. Wirel. Commun. 2013, 12, 626–635. [Google Scholar] [CrossRef]
- Kariminezhad, A.; Sezgin, A. Heterogeneous multi-tier networks: Improper signaling for joint rate-energy optimization. IEEE Trans. Wirel. Commun. 2019, 18, 680–694. [Google Scholar] [CrossRef]
- Javed, S.; Amin, O.; Shihada, B.; Alouini, M.-S. Improper Gaussian signaling for hardware impaired multihop full-duplex relaying systems. IEEE Trans. Commun. 2019, 67, 1858–1871. [Google Scholar] [CrossRef]
- Soleymani, M.; Lameiro, C.; Schreier, P.J.; Santamaria, I. Energy-efficient design for underlay cognitive radio using improper signaling. In Proceedings of the ICASSP 2019—2019 IEEE International Conference on Acoustics, Speech and Signal Processing (ICASSP), Brighton, UK, 12–17 May 2019; pp. 4769–4773. [Google Scholar]
- Soleymani, M.; Lameiro, C.; Santamaria, I.; Schreier, P.J. Improper signaling for SISO two-user interference channels with additive asymmetric hardware distortion. IEEE Trans. Commun. 2019. [Google Scholar] [CrossRef]
- Soleymani, M.; Lameiro, C.; Santamaria, I.; Schreier, P.J. Energy-Efficient Improper Signaling for K-User Interference Channels. In Proceedings of the IEEE European Signal Processing Coference (EUSIPCO), A Coruña, Spain, 2–6 September 2019. [Google Scholar]
- Soleymani, M.; Lameiro, C.; Santamaria, I.; Schreier, P.J. Robust improper signaling for two-user SISO interference channels. IEEE Trans. Commun. 2019, 67, 4709–4723. [Google Scholar] [CrossRef]
- Amin, O.; Abediseid, W.; Alouini, M.-S. Underlay cognitive radio systems with improper Gaussian signaling: Outage performance analysis. IEEE Trans. Wirel. Commun. 2016, 15, 4875–4887. [Google Scholar] [CrossRef]
- Gaafar, M.; Amin, O.; Abediseid, W.; Alouini, M.-S. Underlay spectrum sharing techniques with in-band full-duplex systems using improper Gaussian signaling. IEEE Trans. Wirel. Commun. 2017, 16, 235–249. [Google Scholar] [CrossRef]
- Gaafar, M.; Khafagy, M.G.; Amin, O.; Schaefer, R.F.; Alouini, M.-S. Full-duplex relaying with improper Gaussian signaling over Nakagami-m fading channels. IEEE Trans. Commun. 2018, 66, 64–78. [Google Scholar] [CrossRef]
- Adali, T.; Schreier, P.J.; Scharf, L.L. Complex-valued signal processing: The proper way to deal with impropriety. IEEE Trans. Signal Process. 2011, 59, 5101–5125. [Google Scholar] [CrossRef]
- Schreier, P.J.; Scharf, L.L. Statistical Signal Processing of Complex-Valued Data: The Theory of Improper and Noncircular Signals; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Dysto, A.; Tuninetti, D.; Devroye, N. Interference as noise: Friend or foe? IEEE Trans. Inf. Theory 2016, 62, 3561–3596. [Google Scholar]
- Motahari, A.S.; Khandani, A.K. Capacity bounds for the Gaussian interference channel. IEEE Trans. Inf. Theory 2009, 55, 620–643. [Google Scholar] [CrossRef]
- Haghi, A.; Mohammadizadeh, N.; Khandani, A.K. Delay in Cooperative Communications: Achieving Higher Multiplexing Gain in Gaussian Interference Channels with Full-Duplex Transmitters. IEEE Trans. Inf. Theory 2019. [Google Scholar] [CrossRef]
- Carleial, A. A case where interference does not reduce capacity. IEEE Trans. Inf. Theory 1975, 21, 569–570. [Google Scholar] [CrossRef]
- Sato, H. The capacity of the Gaussian interference channel under strong interference. IEEE Trans. Inf. Theory 1981, 27, 786–788. [Google Scholar] [CrossRef]
- Fasoulakis, M.; Tsiropoulou, E.E.; Papavassiliou, S. Satisfy instead of maximize: Improving operation efficiency in wireless communication networks. Comput. Netw. 2019, 159, 135–146. [Google Scholar] [CrossRef]
- Fasoulakis, M.; Traganitis, A.; Ephremides, A. The Gaussian interference channel revisited as a non-cooperative game with transmission cost. In Proceedings of the 2017 15th International Symposium on Modeling and Optimization in Mobile, Ad Hoc, and Wireless Networks (WiOpt), Paris, France, 15–19 May 2017. [Google Scholar]
- Geng, C.; Naderializadeh, N.; Avestimehr, A.S.; Jafar, S.A. On the optimality of treating interference as noise. IEEE Trans. Inf. Theory 2015, 61, 1753–1767. [Google Scholar] [CrossRef]
- Tsinos, C.G.; Berberidis, K. Blind Opportunistic Interference Alignment in MIMO Cognitive Radio Systems. IEEE J. Emerg. Sel. Top. Circuits Syst. 2013, 3, 626–639. [Google Scholar] [CrossRef]
- Cadambe, V.R.; Jafar, S.A. Interference Alignment and Degrees of Freedom of the K-User Interference Channel. IEEE Trans. Inf. Theory 2008, 54, 3425–3441. [Google Scholar] [CrossRef]
- Tsinos, C.G.; Berberidis, K. Blind opportunistic interference alignment in cognitive radio systems. In Proceedings of the 2013 IEEE International Conference on Communications (ICC), Budapest, Hungary, 9–13 June 2013; pp. 4880–4884. [Google Scholar]
- Perlaza, S.M.; Fawaz, N.; Lasaulce, S.; Debbah, M. From Spectrum Pooling to Space Pooling: Opportunistic Interference Alignment in MIMO Cognitive Networks. IEEE Trans. Signal Process. 2010, 58, 3728–3741. [Google Scholar] [CrossRef]
- Maddah-Ali, M.; Motahari, A.; Khandani, A. Communication over MIMO X channels: Interference alignment, decomposition, performance analysis. IEEE Trans. Inf. Theory 2008, 54, 3457–3470. [Google Scholar] [CrossRef]
- Ding, Z.; Liu, Y.; Choi, J.; Sun, Q.; Elkashlan, M.; Chih-Lin, I.; Poor, V. Application of non-orthogonal multiple access in LTE and 5G networks. IEEE Commun. Mag. 2017, 55, 185–191. [Google Scholar] [CrossRef]
- Ding, Z.; Lei, X.; Karagiannidis, G.K.; Schober, R.; Yuan, J.; Bhargava, V.K. A survey on non-orthogonal multiple access for 5G networks: Research challenges and future trends. IEEE J. Sel. Areas Commun. 2017, 35, 2181–2195. [Google Scholar] [CrossRef]
- Liu, Y.; Qin, Z.; Elkashlan, M.; Ding, Z.; Nallanathan, A.; Hanzo, L. Nonorthogonal multiple access for 5G and beyond. Proc. IEEE 2017, 105, 2347–2381. [Google Scholar] [CrossRef]
- Shen, H.; Wang, J.; Levy, B.C.; Zhao, C. Robust optimization for amplify-and-forward MIMO relaying from a worst-case perspective. IEEE Trans. Signal Process. 2013, 61, 5458–5471. [Google Scholar] [CrossRef]
- Charafeddine, M.A.; Sezgin, A.; Han, Z.; Paulraj, A. Achievable and crystallized rate regions of the interference channel with interference as noise. IEEE Trans. Wirel. Commun. 2012, 11, 1100–1111. [Google Scholar] [CrossRef]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Soleymani, M.; Santamaria, I.; Lameiro, C.; Schreier, P.J. Ergodic Rate for Fading Interference Channels with Proper and Improper Gaussian Signaling. Entropy 2019, 21, 922. https://doi.org/10.3390/e21100922
Soleymani M, Santamaria I, Lameiro C, Schreier PJ. Ergodic Rate for Fading Interference Channels with Proper and Improper Gaussian Signaling. Entropy. 2019; 21(10):922. https://doi.org/10.3390/e21100922
Chicago/Turabian StyleSoleymani, Mohammad, Ignacio Santamaria, Christian Lameiro, and Peter J. Schreier. 2019. "Ergodic Rate for Fading Interference Channels with Proper and Improper Gaussian Signaling" Entropy 21, no. 10: 922. https://doi.org/10.3390/e21100922
APA StyleSoleymani, M., Santamaria, I., Lameiro, C., & Schreier, P. J. (2019). Ergodic Rate for Fading Interference Channels with Proper and Improper Gaussian Signaling. Entropy, 21(10), 922. https://doi.org/10.3390/e21100922




