Extremal Matching Energy of Random Polyomino Chains
Abstract
:1. Introduction
- (i)
- with probability ,
- (ii)
- with probability ,
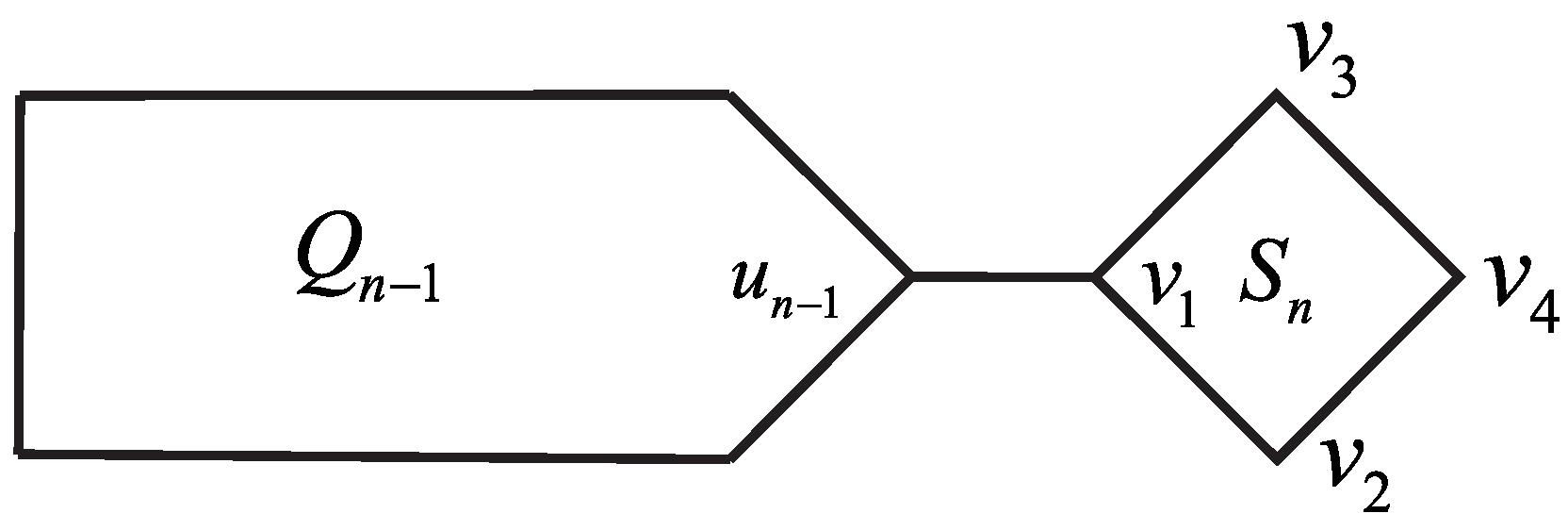
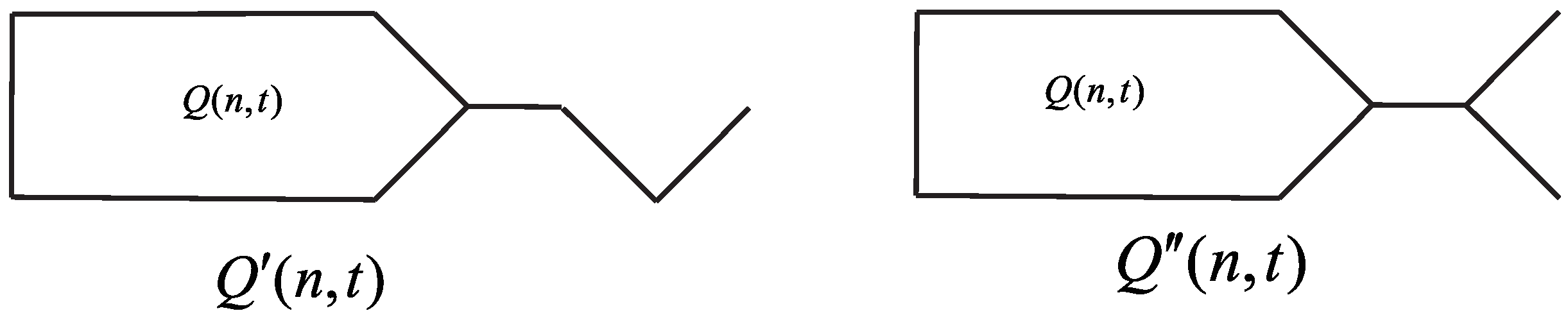
2. Preliminaries
3. Main Result
4. Discussion
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Berge, C.; Chen, C.C.; Chvatal, V.; Seow, C.S. Combinatorial properties of polyominoes. Combinatorics 1981, 1, 217–224. [Google Scholar] [CrossRef]
- Cockayne, E.J. Chessboard domination problems. Discrete Math. 1990, 86, 13–20. [Google Scholar] [CrossRef]
- Harary, F.; Mezey, P.G. Cell-shedding transformations, equivalence relations, and similarity measures for square-cell configurations. Int. Quant. Chem. 1997, 62, 353–361. [Google Scholar] [CrossRef]
- Fisher, M.E. Statistical mechanics of dimers on a plane lattice. Phys. Rev. 1961, 124, 1664–1672. [Google Scholar] [CrossRef]
- Grinstead, C.M.; Hahne, B.; Stone, D.V. On the queen domination problem. Discret. Math. 1990, 86, 21–26. [Google Scholar] [CrossRef]
- Kasteleyn, P.W. The statistics of dimers on a lattice: I. The number of dimer arrangements on a quadratic lattice. Physica 1961, 27, 1209–1225. [Google Scholar] [CrossRef]
- Liu, S.; Ou, J. On maximal resonance of polyomino graphs. J. Math. Chem. 2013, 51, 603–619. [Google Scholar] [CrossRef]
- Motoyama, A.; Hosoya, H. King and domino polyominals for polyomino graphs. J. Math. Phys. 1997, 18, 1485–1490. [Google Scholar]
- Pachter, L.; Kim, P. Forcing matchings on square grids. Discret. Math. 1998, 190, 287–294. [Google Scholar] [CrossRef]
- Wei, S.; Ke, X. Elementary components of essentially disconnected polyomino graphs. J. Math. Chem. 2010, 47, 496–504. [Google Scholar]
- Yan, W.; Yeh, Y.N.; Zhang, F. Dimer problem on the cylinder and torus. Phys. A 2008, 387, 6069–6078. [Google Scholar] [CrossRef]
- Zhang, H. The connectivity of Z-transformation graphs of perfect matchings of polyominoes. Discret. Math. 1996, 158, 257–272. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, F. Perfect matchings of polyomino graphs. Graphs Comb. 1997, 13, 295–304. [Google Scholar] [CrossRef]
- Zhang, H.; Zhou, X. A maximum resonant set of polyomino graphs. Discus. Math. Graph Theory 2016, 36, 323–337. [Google Scholar]
- Liu, W.; Guo, Q.; Zhang, Y.; Feng, L.; Gutman, I. Further results on the largest matching root of unicyclic graphs. Discret. Appl. Math. 2017, 221, 82–88. [Google Scholar] [CrossRef]
- Shi, Y.; Dehmer, M.; Li, X.; Gutman, I. Graph Polynomials; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Yan, W.; Yeh, Y. On the matching polynomial of subdivision graphs. Discret. Appl. Math. 2009, 157, 196–200. [Google Scholar] [CrossRef]
- Chauvin, R.; Lepetit, C.; Fowler, P.W.; Malrieu, J. The chemical roots of the matching polynomial. Phys. Chem. Chem. Phys. 2010, 12, 5295–5306. [Google Scholar] [CrossRef] [PubMed]
- Gutman, I.; Wagner, S. The matching energy of a graph. Discrete Appl. Math. 2012, 160, 2177–2187. [Google Scholar] [CrossRef]
- Gutman, I.; Mohar, B. More difficulties with topological resonance energy. Chem. Phys. Lett. 1981, 77, 567–570. [Google Scholar] [CrossRef]
- Gutman, I. Acyclic systems with extremal Hückel π-electron energy. Theor. Chim. Acta 1977, 45, 79–87. [Google Scholar] [CrossRef]
- Chen, L.; Liu, J.; Shi, Y. Matching energy of unicyclic and bicyclic graphs with a given diameter. Complexity 2015, 21, 224–238. [Google Scholar] [CrossRef]
- Chen, L.; Liu, J. The bipartite unicyclic graphs with the first largest matching energies. Appl. Math. Comput. 2015, 268, 644–656. [Google Scholar] [CrossRef]
- Chen, L.; Shi, Y. The maximal matching energy of tricyclic graphs. MATCH Commun. Math. Comput. Chem. 2015, 73, 105–119. [Google Scholar]
- Chen, X.; Li, X.; Lian, H. The matching energy of random graphs. Discrete Appl. Math. 2015, 193, 102–109. [Google Scholar] [CrossRef]
- Chen, L.; Liu, J.; Shi, Y. Bounds on the matching energy of unicyclic odd-cycle graphs. MATCH Commun. Math. Comput. Chem. 2016, 75, 315–330. [Google Scholar]
- Dehmer, M.; Li, X.; Shi, Y. Connections between generalized graph entropies and graph energy. Complexity 2015, 21, 35–41. [Google Scholar] [CrossRef]
- Huo, B.; Li, X.; Shi, Y. Complete solution to a conjecture on the maximal energy of unicyclic graphs. Eur. J. Comb. 2011, 32, 662–673. [Google Scholar] [CrossRef]
- Ji, S.; Li, X.; Shi, Y. Extremal matching energy of bicyclic graphs. MATCH Commun. Math. Comput. Chem. 2013, 70, 697–706. [Google Scholar]
- Ji, S.; Ma, H.; Ma, G. The matching energy of graphs with given edge connectivity. J. Inequal. Appl. 2015, 2015, 415. [Google Scholar]
- Li, S.; Yan, W. The matching energy of graphs with given parameters. Discret. Appl. Math. 2014, 162, 415–420. [Google Scholar] [CrossRef]
- Wang, W.; So, W. On minimum matching energy of graphs. MATCH Commun. Math. Comput. Chem. 2015, 74, 399–410. [Google Scholar]
- Wu, T. Two classes of topological indices of phenylene molecule graphs. Math. Probl. Eng. 2016, 2016, 8421396. [Google Scholar] [CrossRef]
- Wu, T.; Yan, W.; Zhang, H. Extremal matching energy of complements of trees. Disscu. Math. Graph Theory 2016, 36, 505–522. [Google Scholar] [CrossRef]
- Xu, K.; Das, K.C.; Zheng, Z. The minimal matching energy of (n,m)-graphs with a given matching number. MATCH Commun. Math. Comput. Chem. 2015, 73, 93–104. [Google Scholar]
- Xu, K.; Zheng, Z.; Das, K.C. Extremal t-apex trees with respect to matching energy. Complexity 2016, 21, 238–247. [Google Scholar]


© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, T.; Lü, H.; Zhang, X. Extremal Matching Energy of Random Polyomino Chains. Entropy 2017, 19, 684. https://doi.org/10.3390/e19120684
Wu T, Lü H, Zhang X. Extremal Matching Energy of Random Polyomino Chains. Entropy. 2017; 19(12):684. https://doi.org/10.3390/e19120684
Chicago/Turabian StyleWu, Tingzeng, Huazhong Lü, and Xuexin Zhang. 2017. "Extremal Matching Energy of Random Polyomino Chains" Entropy 19, no. 12: 684. https://doi.org/10.3390/e19120684
APA StyleWu, T., Lü, H., & Zhang, X. (2017). Extremal Matching Energy of Random Polyomino Chains. Entropy, 19(12), 684. https://doi.org/10.3390/e19120684




