Entropy-Based Modeling of Velocity Lag in Sediment-Laden Open Channel Turbulent Flow
Abstract
:1. Introduction
2. Entropy Theory-Based Methodology
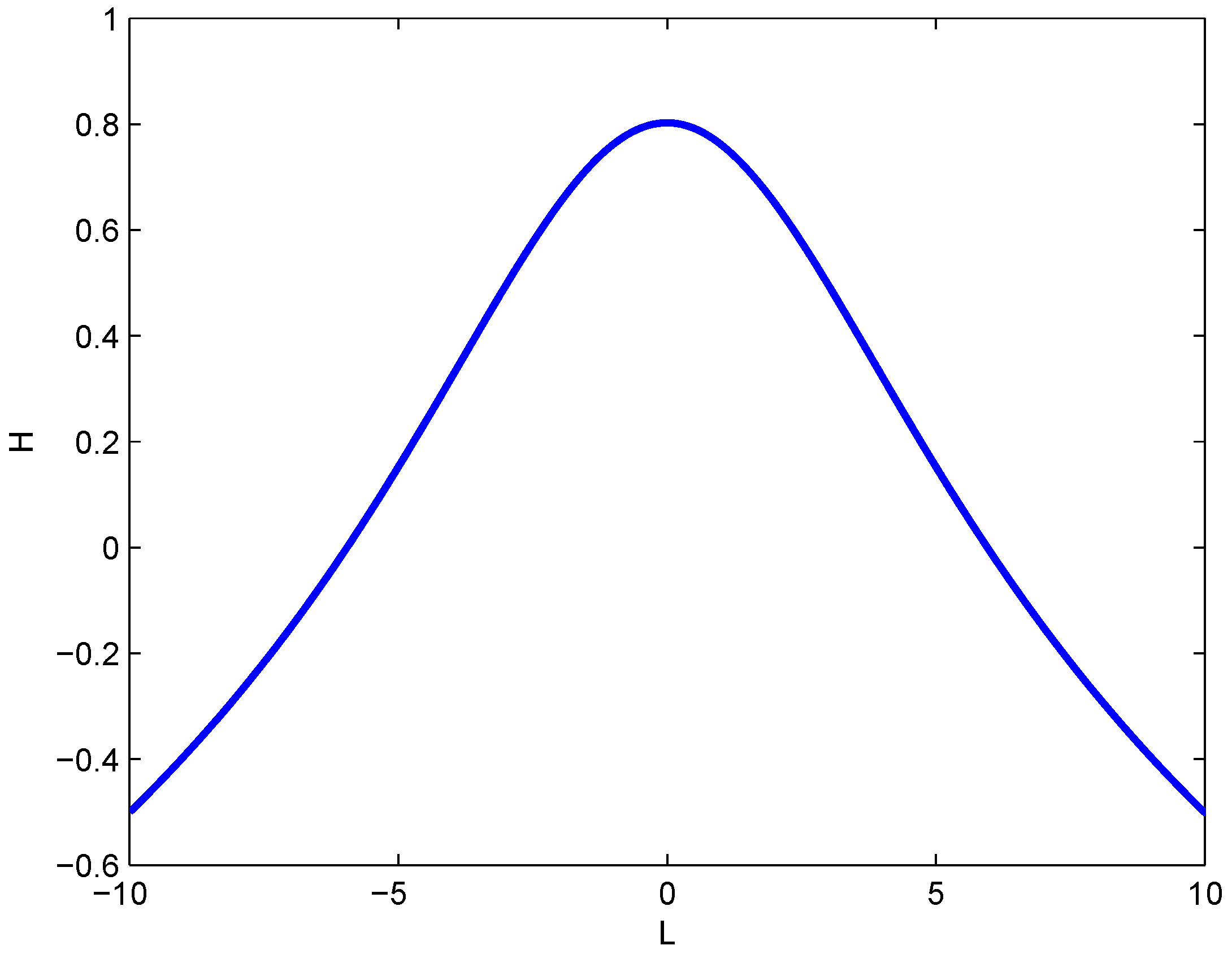
2.1. Definition of Entropy
2.2. Specification of Constraints
2.3. Maximization of Entropy
2.4. Calculation of Lagrange Multipliers
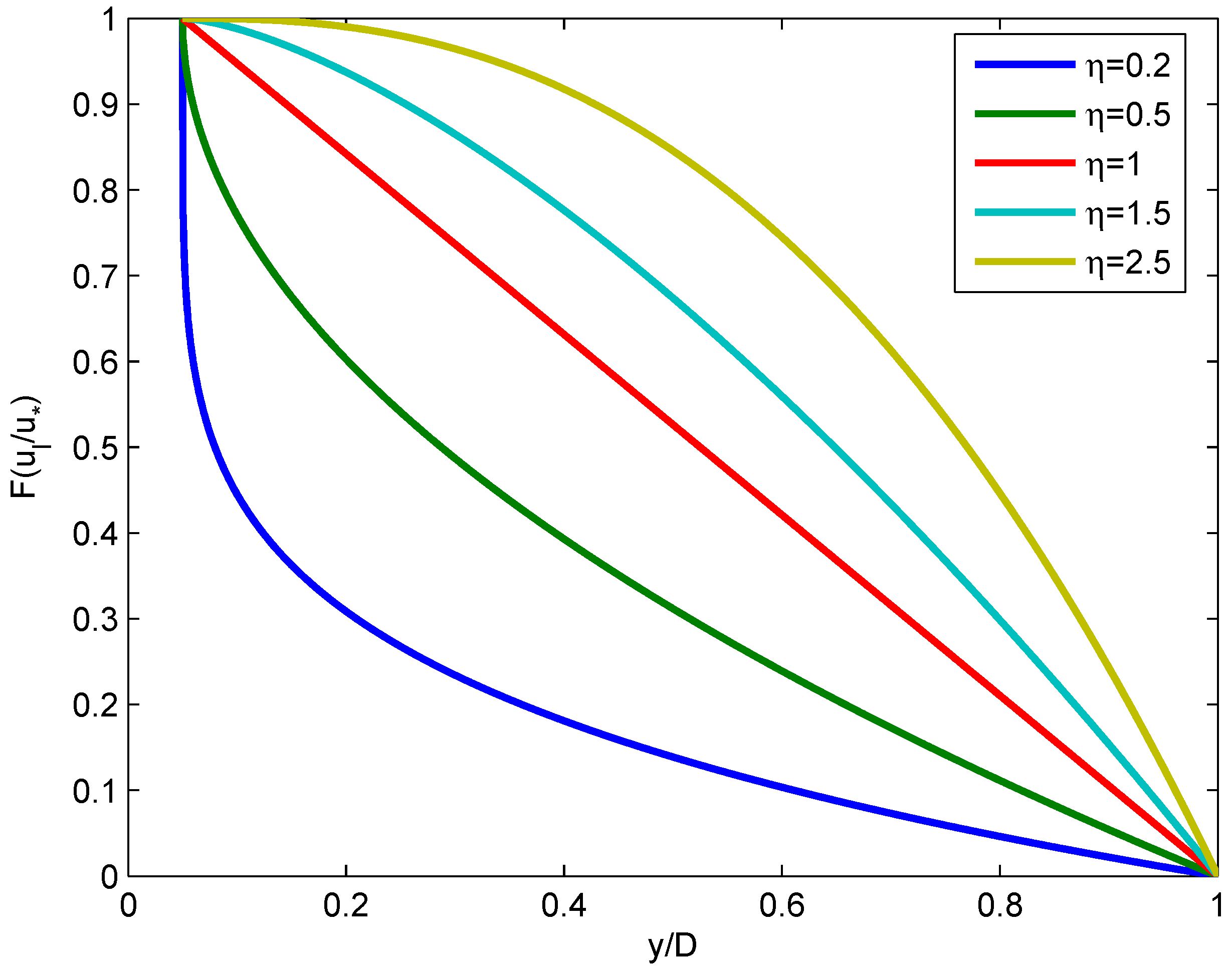
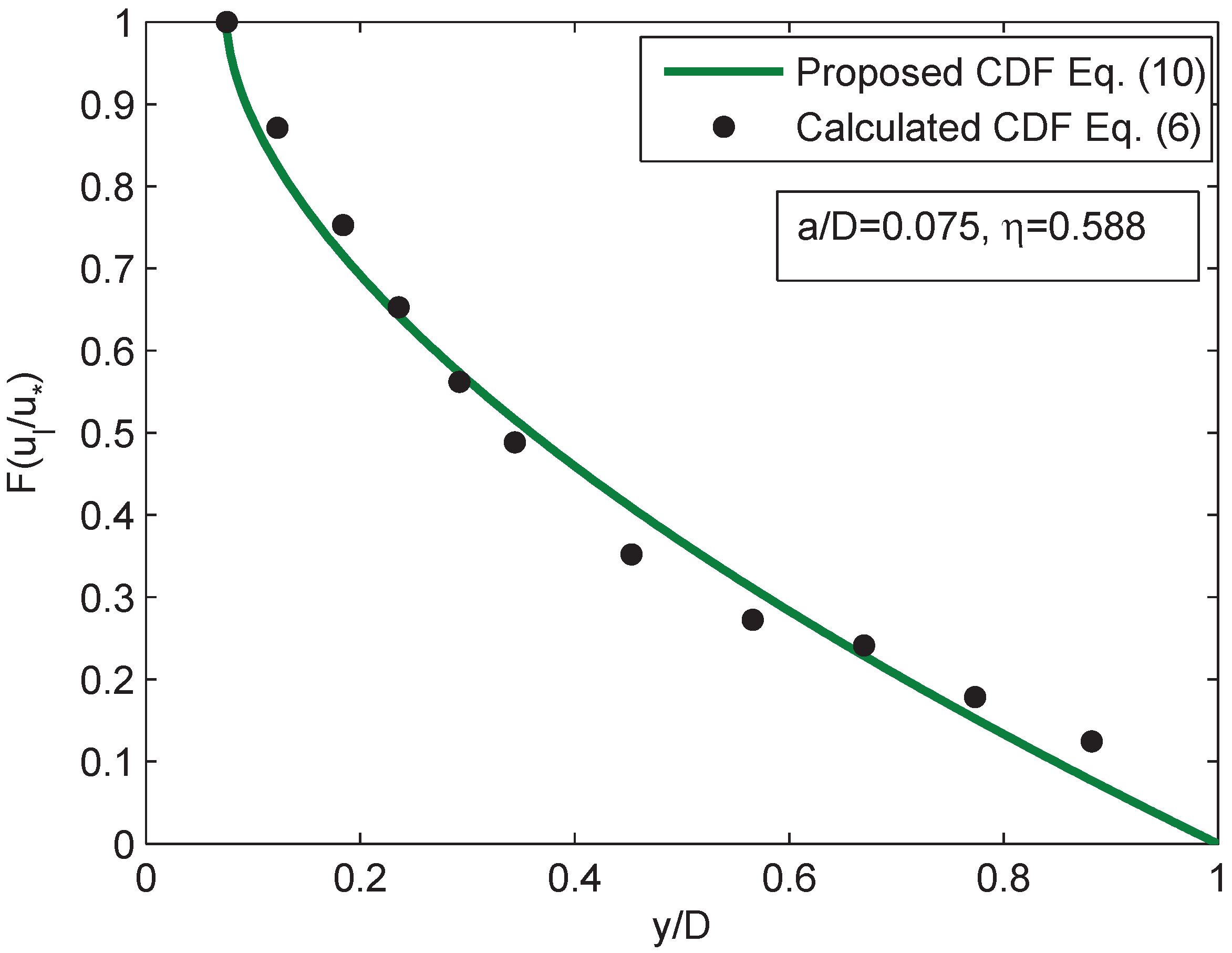
2.5. Cumulative Distribution Function
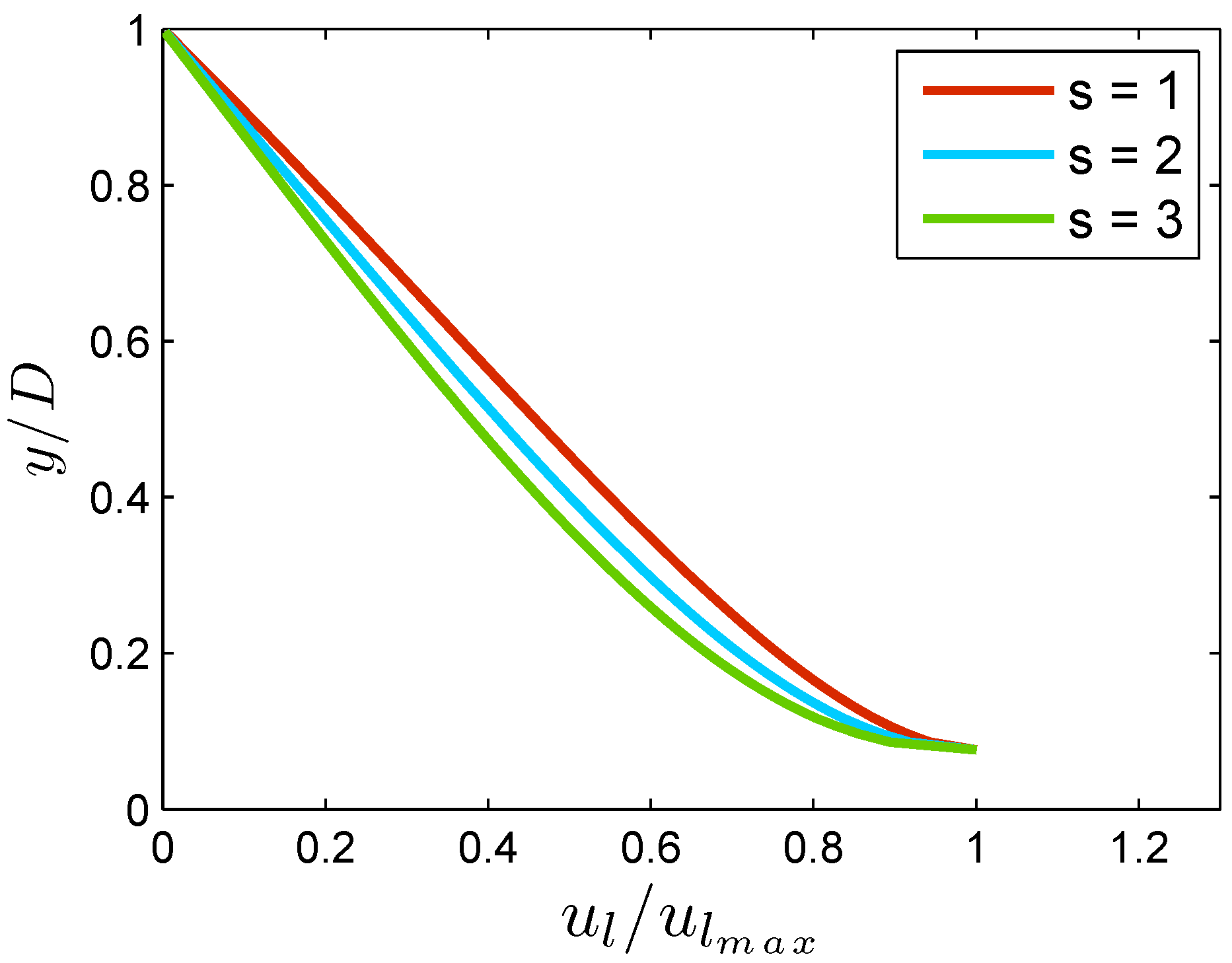
2.6. Derivation of Velocity Lag
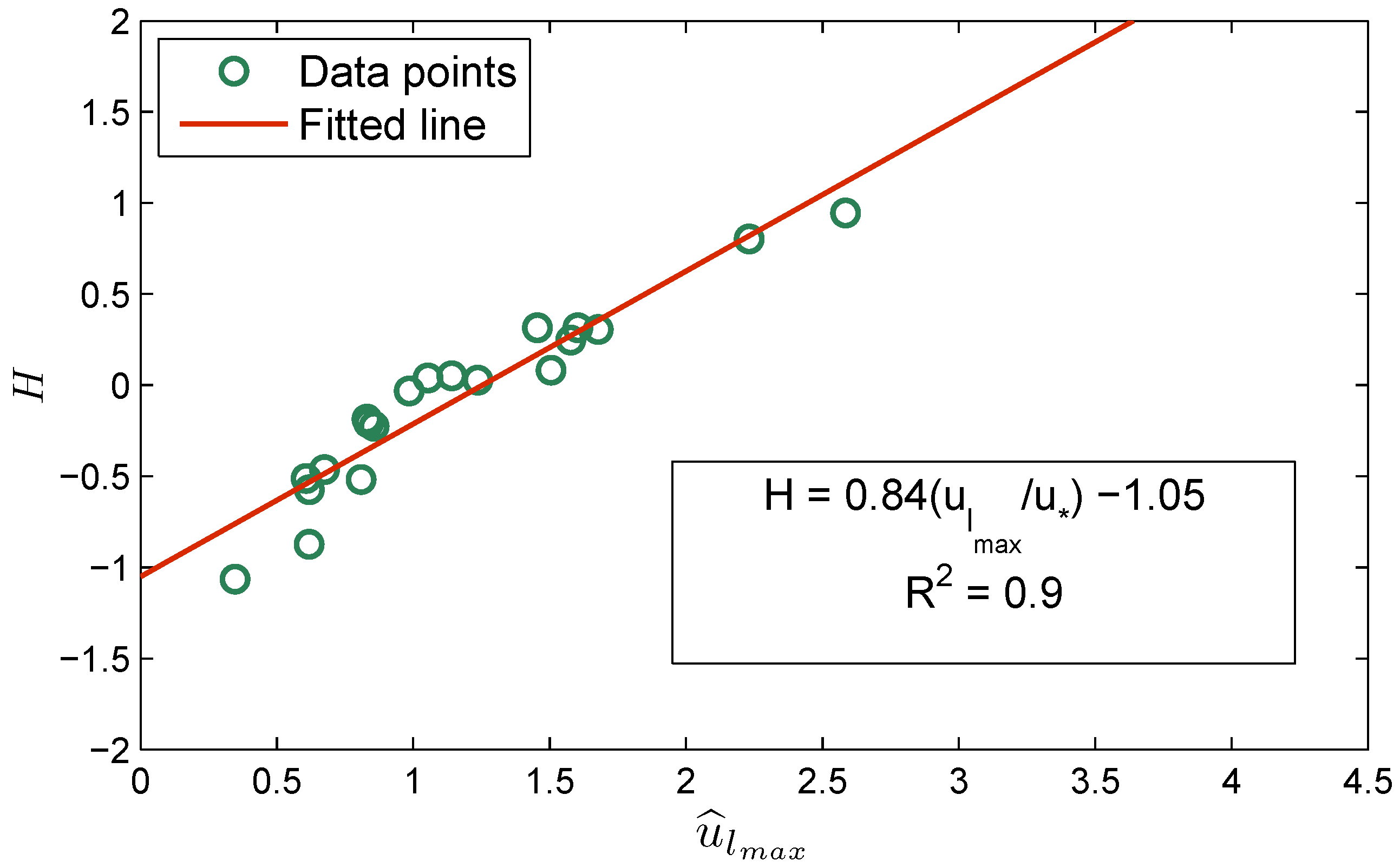
2.7. Re-Parametrization
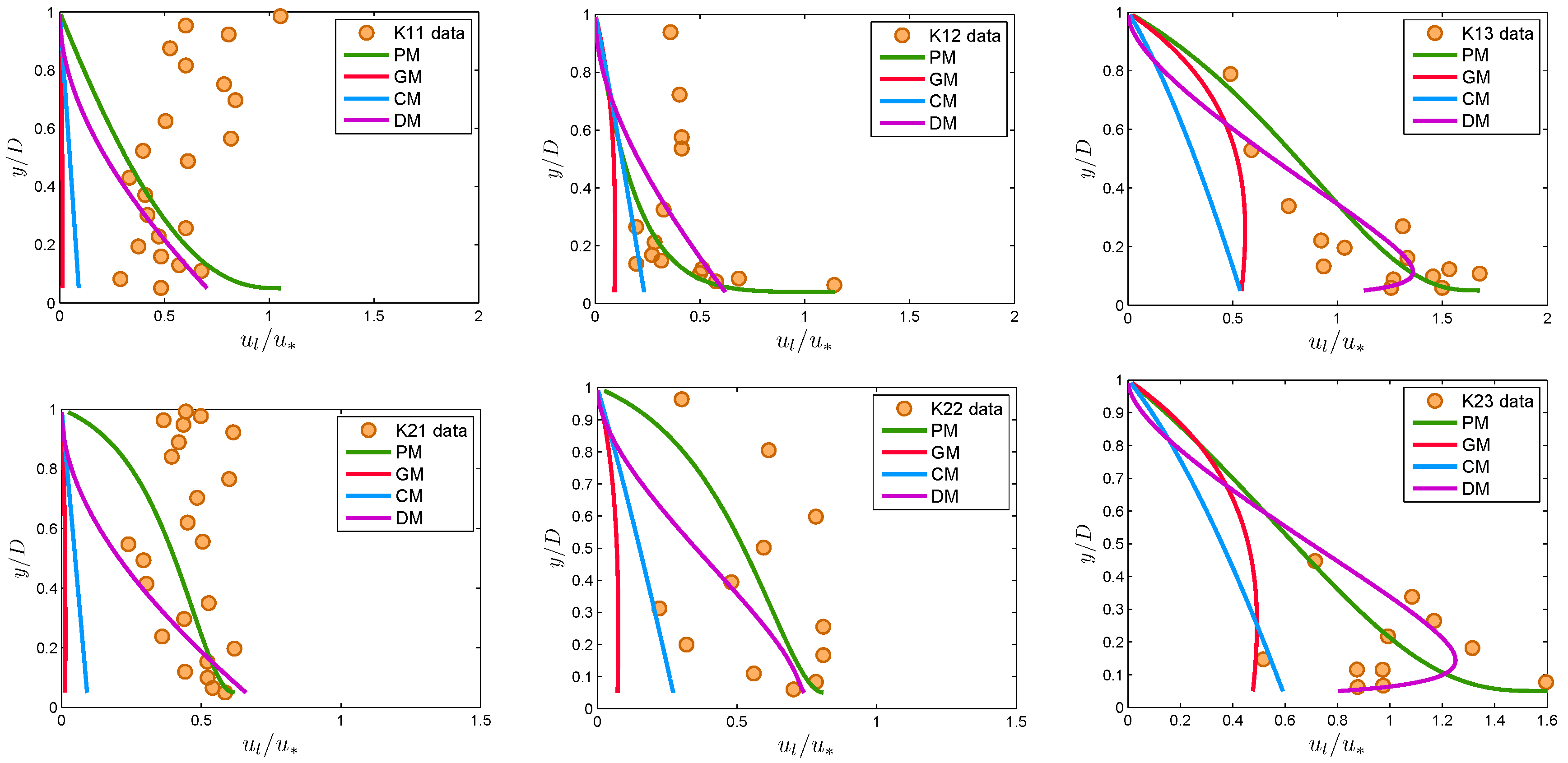
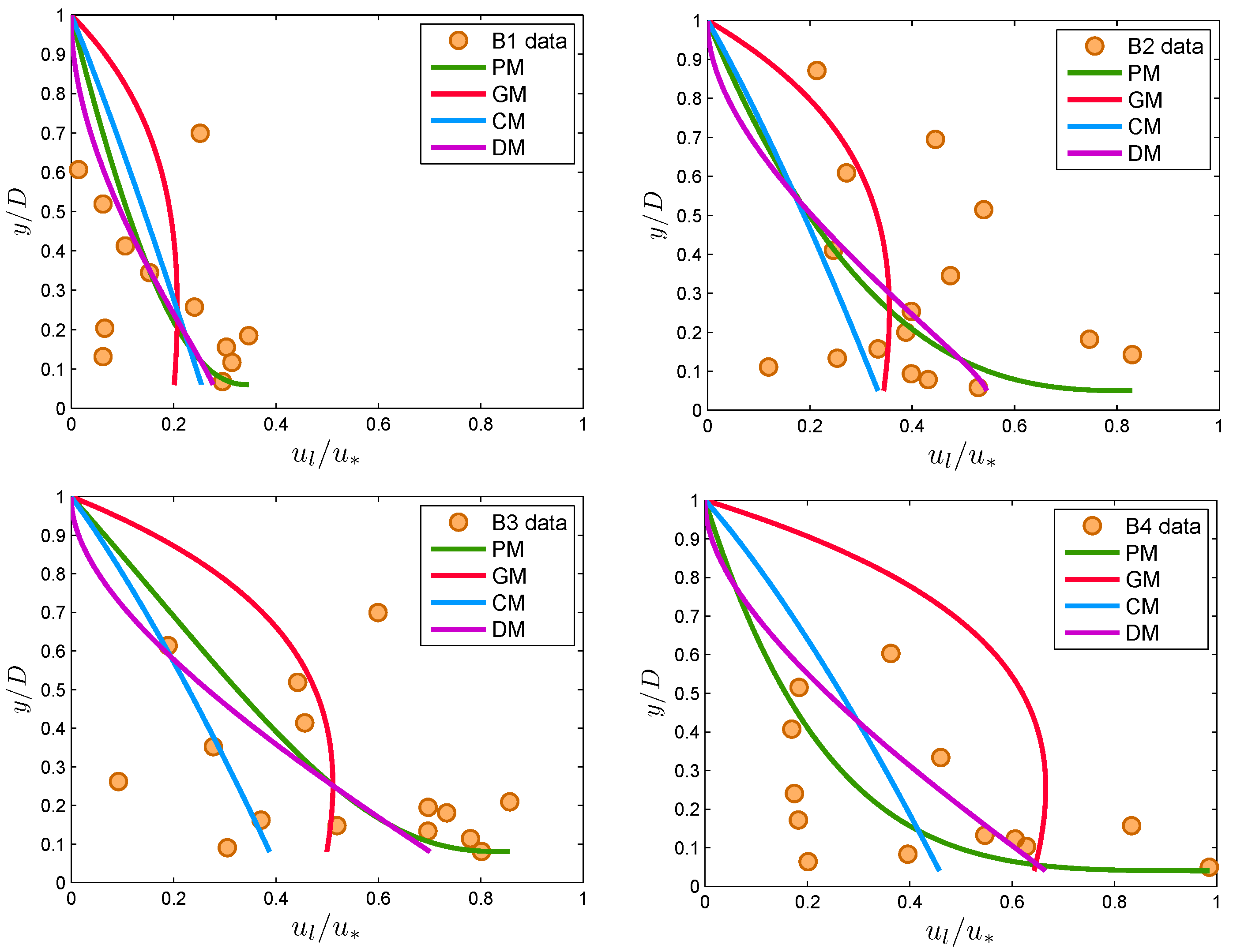
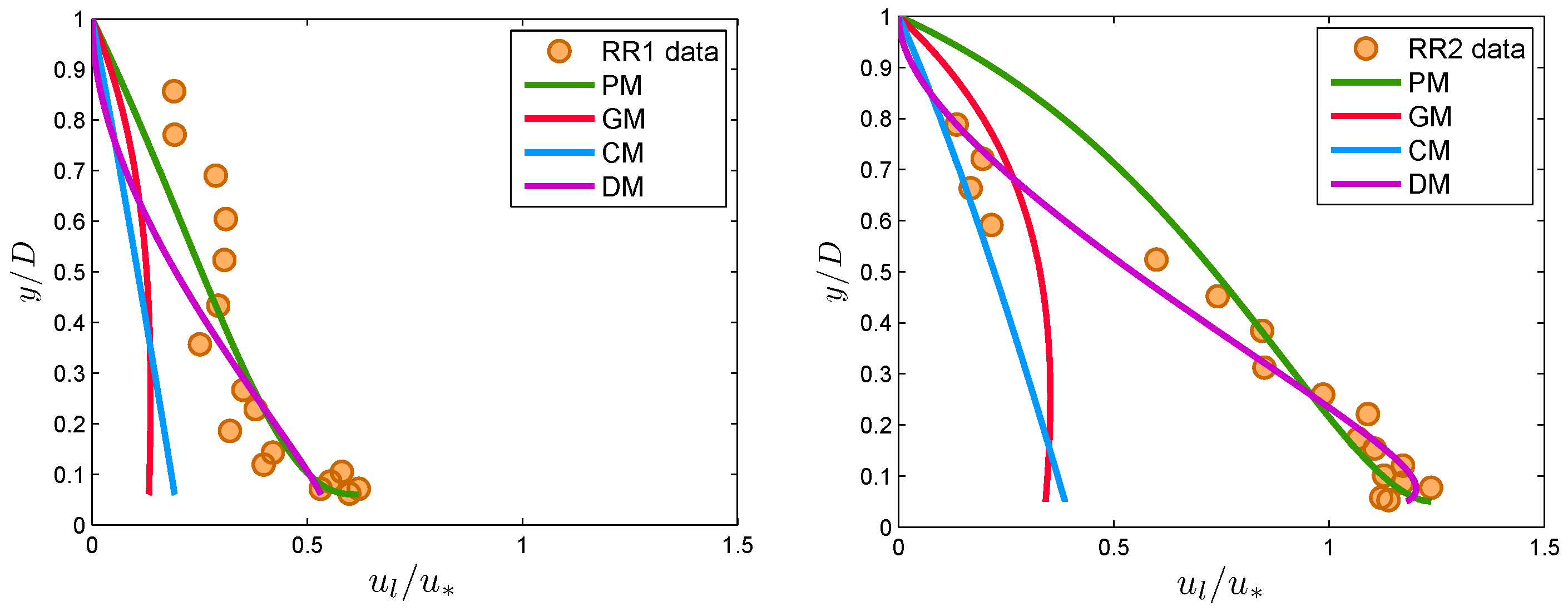
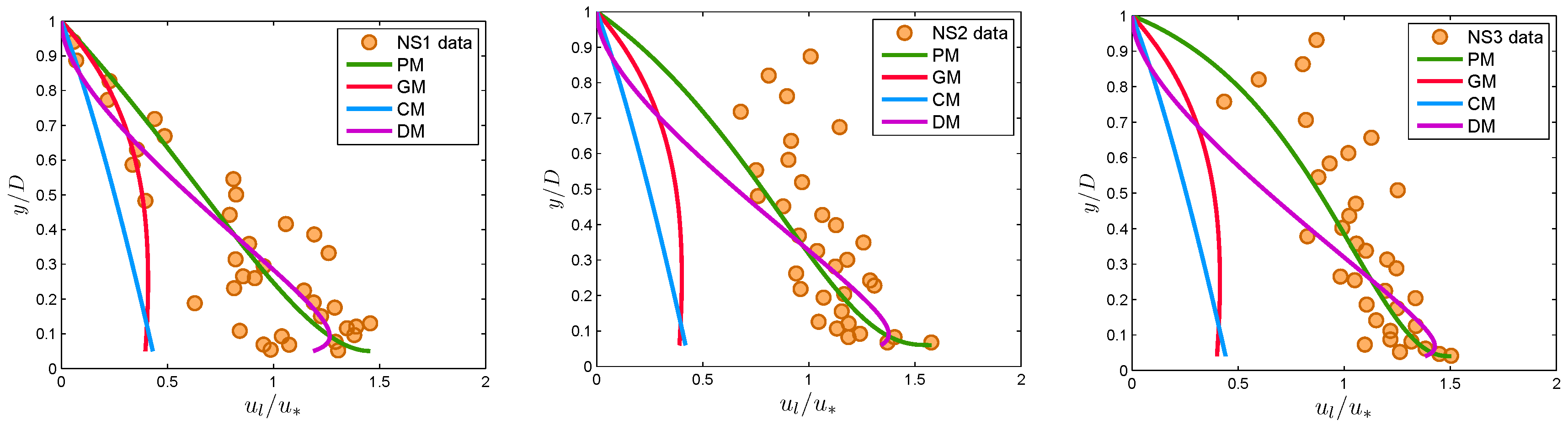
3. Comparison with Experimental Data and Other Models
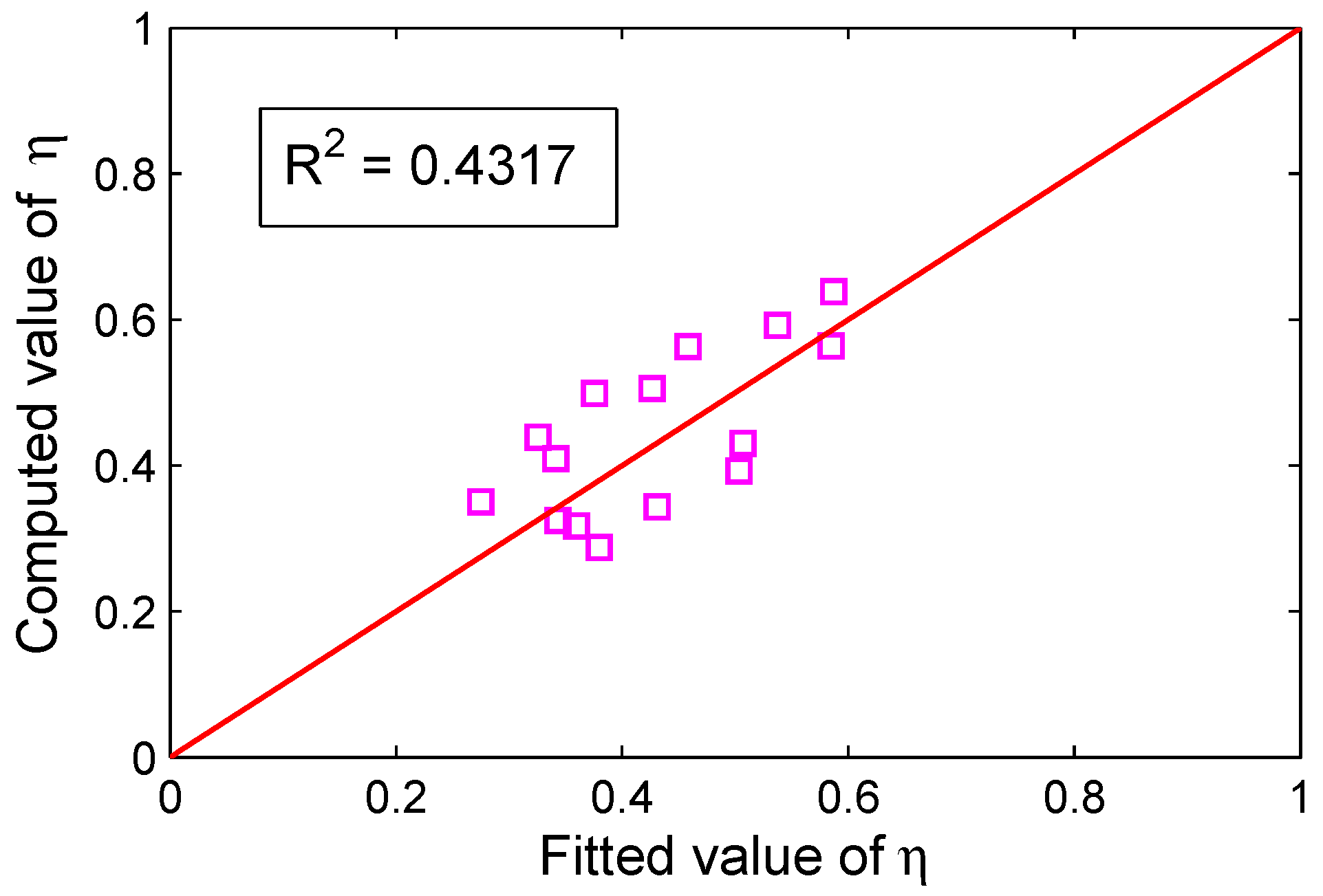
4. Discussion
5. Conclusions
Author Contributions
Conflicts of Interest
References
- Bagnold, R.A. The nature of saltation and bedload transport in water. Proc. R. Soc. Lond. Ser. A 1973, 332, 473–504. [Google Scholar] [CrossRef]
- Muste, M.; Patel, V.C. Velocity profiles for particles and liquid in open-channel flow with suspended sediment. J. Hydraul. Eng. 1997, 123, 742–751. [Google Scholar] [CrossRef]
- Best, J.; Bennett, S.; Bridge, J.; Leeder, M. Turbulence modulation and particle velocities over flat sand beds at low transport rates. J. Hydraul. Eng. 1997, 123, 1118–1129. [Google Scholar] [CrossRef]
- Rashidi, M.; Hetsroni, G.; Banerjee, S. Particle-turbulence interaction in a boundary layer. Int. J. Multiph. Flow 1990, 16, 935–949. [Google Scholar] [CrossRef]
- Taniere, A.; Oesterle, B.; Monnier, J.C. On the behavior of solid particles in a horizontal boundary layer with turbulence and saltation effects. Exp. Fluids 1997, 23, 463–471. [Google Scholar]
- Kiger, K.T.; Pan, C. Suspension and turbulence modification effects of solid particulates on a horizontal turbulent channel flow. J. Turbul. 2002. [Google Scholar] [CrossRef]
- Chauchat, J.; Guillou, S. On turbulence closures for two-phase sediment-laden flow models. J. Geophys. Res. Oceans 2008. [Google Scholar] [CrossRef]
- Bombardelli, F.A.; Jha, S.K. Hierarchical modeling of the dilute transport of suspended sediment in open channels. Environ. Fluid Mech. 2009, 9, 207–235. [Google Scholar] [CrossRef]
- Jha, S.K.; Bombardelli, F.A. Toward two-phase flow modeling of nondilute sediment transport in open channels. J. Geophys. Res. 2010, 115. [Google Scholar] [CrossRef]
- Greimann, B.P.; Muste, M.; Holly, F.M. Two-phase formulation of suspended sediment transport. J. Hydraul. Res. 1999, 37, 479–500. [Google Scholar] [CrossRef]
- Jiang, J.S.; Law, A.W.K.; Cheng, N.S. Two-phase modeling of suspended sediment distribution in open channel flows. J. Hydraul. Res. 2004, 42, 273–281. [Google Scholar] [CrossRef]
- Cheng, N.S. Analysis of velocity lag in sediment-laden open channel flows. J. Hydraul. Eng. 2004, 130, 657–666. [Google Scholar] [CrossRef]
- Pal, D.; Jha, S.K.; Ghoshal, K. Velocity lag between particle and liquid in sediment-laden open channel turbulent flow. Eur. J. Mech. B Fluids 2016, 56, 130–142. [Google Scholar] [CrossRef]
- Vowinckel, B.; Kempe, T.; Fröhlich, J. Fluid–particle interaction in turbulent open channel flow with fully-resolved mobile beds. Adv. Water Resour. 2014, 72, 32–44. [Google Scholar] [CrossRef]
- Shao, X.; Wu, T.; Yu, Z. Fully resolved numerical simulation of particle-laden turbulent flow in a horizontal channel at a low Reynolds number. J. Fluid Mech. 2012, 693, 319–344. [Google Scholar] [CrossRef]
- Derksen, J.J. Simulations of granular bed erosion due to a mildly turbulent shear flow. J. Hydraul. Res. 2015, 53, 622–632. [Google Scholar] [CrossRef]
- Finn, J.R.; Li, M. Regimes of sediment-turbulence interaction and guidelines for simulating the multiphase bottom boundary layer. Int. J. Multiph. Flow 2016, 85, 278–283. [Google Scholar] [CrossRef]
- Chiu, C.L. Entropy and probability concepts in hydraulics. J. Hydraul. Eng. 1987, 113, 583–600. [Google Scholar] [CrossRef]
- Cui, H.; Singh, V.P. Two-dimensional velocity distribution in open channels using the Tsallis entropy. J. Hydrol. Eng. 2012, 18, 331–339. [Google Scholar] [CrossRef]
- Cui, H.; Singh, V.P. One-dimensional velocity distribution in open channels using Tsallis entropy. J. Hydrol. Eng. 2013, 19, 290–298. [Google Scholar] [CrossRef]
- Kumbhakar, M.; Ghoshal, K. One-Dimensional velocity distribution in open channels using Renyi entropy. Stoch. Environ. Res. Risk Assess. 2016. [Google Scholar] [CrossRef]
- Kumbhakar, M.; Ghoshal, K. Two dimensional velocity distribution in open channels using Renyi entropy. Phys. A Stat. Mech. Appl. 2016, 450, 546–559. [Google Scholar] [CrossRef]
- Chiu, C.; Jin, W.; Chen, Y. Mathematical models for distribution of sediment concentration. J. Hydraul. Eng. 2000, 126, 16–23. [Google Scholar] [CrossRef]
- Cui, H.; Singh, V.P. Suspended sediment concentration in open channels using Tsallis entropy. J. Hydrol. Eng. 2013, 19, 966–977. [Google Scholar] [CrossRef]
- Singh, V.P.; Cui, H. Modeling sediment concentration in debris flow by Tsallis entropy. Phys. A Stat. Mech. Appl. 2015, 420, 49–58. [Google Scholar] [CrossRef]
- Righetti, M.; Romano, G.P. Particle-fluid interactions in a plane near-wall turbulent flow. J. Fluid Mech. 2004, 505, 93–121. [Google Scholar] [CrossRef]
- Shannon, C.E. The mathematical theory of communications, I and II. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Jaynes, E. Information theory and statistical mechanics: I. Phys. Rev. 1957, 106, 620–930. [Google Scholar] [CrossRef]
- Jaynes, E. Information theory and statistical mechanics: II. Phys. Rev. 1957, 108, 171–190. [Google Scholar] [CrossRef]
- Jaynes, E. On the rationale of maximum entropy methods. Proc. IEEE 1982, 70, 939–952. [Google Scholar] [CrossRef]
- Kaftori, D.; Hetsroni, G.; Banerjee, S. Particle behavior in the turbulent boundary layer velocity and distribution profiles. Phys. Fluids 1995, 7, 1107–1121. [Google Scholar] [CrossRef]
- Muste, M.; Yu, K.; Fujita, I.; Ettema, R. Two-phase versus mixed-flow perspective on suspended sediment transport in turbulent channel flows. Water Resour. Res. 2005, 41. [Google Scholar] [CrossRef]







| Literature | Run | D | Shields | ||||||
|---|---|---|---|---|---|---|---|---|---|
| (mm) | (cm) | (cm/s) | (cm/s) | (cm/s) | Parameter θ | ||||
| Rashidi et al. [4] | R1 | 0.120 | 0.03 | 2.75 | 15.60 | 0.90 | 0.0084 | 22.3 | 0.067 |
| R2 | 0.220 | 0.03 | 2.75 | 15.60 | 0.90 | 0.0084 | 40.9 | 0.036 | |
| R3 | 0.650 | 0.03 | 2.75 | 15.60 | 0.90 | 0.0084 | 120.9 | 0.012 | |
| R4 | 1.100 | 0.03 | 2.75 | 15.60 | 0.90 | 0.0084 | 204.5 | 0.007 | |
| Kaftori et al. [31] | K11 | 0.100 | 0.05 | 3.25 | 24.50 | 1.28 | 0.0080 | 30.5 | 0.159 |
| K12 | 0.275 | 0.05 | 3.27 | 24.10 | 1.29 | 0.0079 | 83.5 | 0.059 | |
| K13 | 0.900 | 0.05 | 3.27 | 24.85 | 1.34 | 0.0081 | 276.1 | 0.019 | |
| K21 | 0.100 | 0.05 | 3.52 | 31.65 | 1.60 | 0.0081 | 39.2 | 0.249 | |
| K22 | 0.275 | 0.05 | 3.51 | 32.10 | 1.60 | 0.0080 | 110.9 | 0.090 | |
| K23 | 0.900 | 0.05 | 3.77 | 29.45 | 1.55 | 0.0078 | 339.8 | 0.026 | |
| Best et al. [3] | B1 | 0.125 | 1.60 | 5.75 | 58.00 | 3.40 | 0.0083 | 87.0 | 0.363 |
| B2 | 0.175 | 1.60 | 5.75 | 58.00 | 3.40 | 0.0083 | 121.8 | 0.259 | |
| B3 | 0.225 | 1.60 | 5.75 | 58.00 | 3.40 | 0.0083 | 156.7 | 0.201 | |
| B4 | 0.275 | 1.60 | 5.75 | 58.00 | 3.40 | 0.0083 | 191.5 | 0.165 | |
| Righetti and Romano [26] | RR1 | 0.100 | 1.60 | 2.30 | 57.00 | 3.29 | 0.0090 | 63.3 | 0.424 |
| RR2 | 0.200 | 1.60 | 2.00 | 60.00 | 3.97 | 0.0094 | 127.7 | 0.309 | |
| Muste and Patel [2] | SL01 | 0.230 | 1.65 | 12.9 | 62.90 | 3.02 | 0.0103 | 140.5 | 0.153 |
| SL02 | 0.230 | 1.65 | 12.9 | 62.90 | 3.05 | 0.0103 | 140.5 | 0.156 | |
| SL03 | 0.230 | 1.65 | 12.8 | 63.30 | 3.13 | 0.0105 | 138.7 | 0.164 | |
| Muste et al. [32] | NS1 | 0.230 | 1.65 | 2.10 | 81.30 | 4.20 | 0.0093 | 200.4 | 0.295 |
| NS2 | 0.230 | 1.65 | 2.10 | 79.60 | 4.20 | 0.0096 | 191.7 | 0.295 | |
| NS3 | 0.230 | 1.65 | 2.10 | 79.30 | 4.20 | 0.0091 | 200.2 | 0.295 |
| Literature | Run | PM | GM | CM | DM |
|---|---|---|---|---|---|
| Rashidi et al. [4] | R1 | 0.0756 | 1.2849 | 1.2488 | 0.1445 |
| R2 | 0.1098 | 1.4848 | 1.4396 | 0.2132 | |
| R3 | 0.2266 | 1.9072 | 1.9723 | 0.6268 | |
| R4 | 0.2788 | 1.7828 | 2.0818 | 0.8430 | |
| Kaftori et al. [31] | K11 | 0.4477 | 0.5959 | 0.5639 | 0.4662 |
| K12 | 0.2365 | 0.4214 | 0.3458 | 0.2495 | |
| K13 | 0.2105 | 0.6945 | 0.7492 | 0.2310 | |
| K21 | 0.2251 | 0.4627 | 0.4277 | 0.3127 | |
| K22 | 0.2284 | 0.5525 | 0.4411 | 0.2908 | |
| K23 | 0.3263 | 0.6519 | 0.6083 | 0.3901 | |
| Best et al. [3] | B1 | 0.1023 | 0.1139 | 0.1039 | 0.1049 |
| B2 | 0.2318 | 0.1938 | 0.2419 | 0.2225 | |
| B3 | 0.2289 | 0.2316 | 0.3002 | 0.2369 | |
| B4 | 0.2284 | 0.3272 | 0.2417 | 0.2345 | |
| Righetti and Romano [26] | RR1 | 0.0745 | 0.2927 | 0.2666 | 0.1066 |
| RR2 | 0.1740 | 0.6152 | 0.6192 | 0.0760 | |
| Muste and Patel [2] | SL01 | 0.1043 | 0.2666 | 0.1439 | 0.1268 |
| SL02 | 0.1020 | 0.2034 | 0.1612 | 0.1201 | |
| SL03 | 0.1530 | 0.2213 | 0.2246 | 0.1758 | |
| Muste et al. [32] | NS1 | 0.2166 | 0.6070 | 0.6378 | 0.2103 |
| NS2 | 0.2505 | 0.7320 | 0.7899 | 0.3469 | |
| NS3 | 0.1994 | 0.7448 | 0.7954 | 0.3469 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kumbhakar, M.; Kundu, S.; Ghoshal, K.; Singh, V.P. Entropy-Based Modeling of Velocity Lag in Sediment-Laden Open Channel Turbulent Flow. Entropy 2016, 18, 318. https://doi.org/10.3390/e18090318
Kumbhakar M, Kundu S, Ghoshal K, Singh VP. Entropy-Based Modeling of Velocity Lag in Sediment-Laden Open Channel Turbulent Flow. Entropy. 2016; 18(9):318. https://doi.org/10.3390/e18090318
Chicago/Turabian StyleKumbhakar, Manotosh, Snehasis Kundu, Koeli Ghoshal, and Vijay P. Singh. 2016. "Entropy-Based Modeling of Velocity Lag in Sediment-Laden Open Channel Turbulent Flow" Entropy 18, no. 9: 318. https://doi.org/10.3390/e18090318
APA StyleKumbhakar, M., Kundu, S., Ghoshal, K., & Singh, V. P. (2016). Entropy-Based Modeling of Velocity Lag in Sediment-Laden Open Channel Turbulent Flow. Entropy, 18(9), 318. https://doi.org/10.3390/e18090318






