Analytical Modeling of MHD Flow over a Permeable Rotating Disk in the Presence of Soret and Dufour Effects: Entropy Analysis
Abstract
:1. Introduction
2. Mathematical Formulation
3. Entropy Generation Analysis
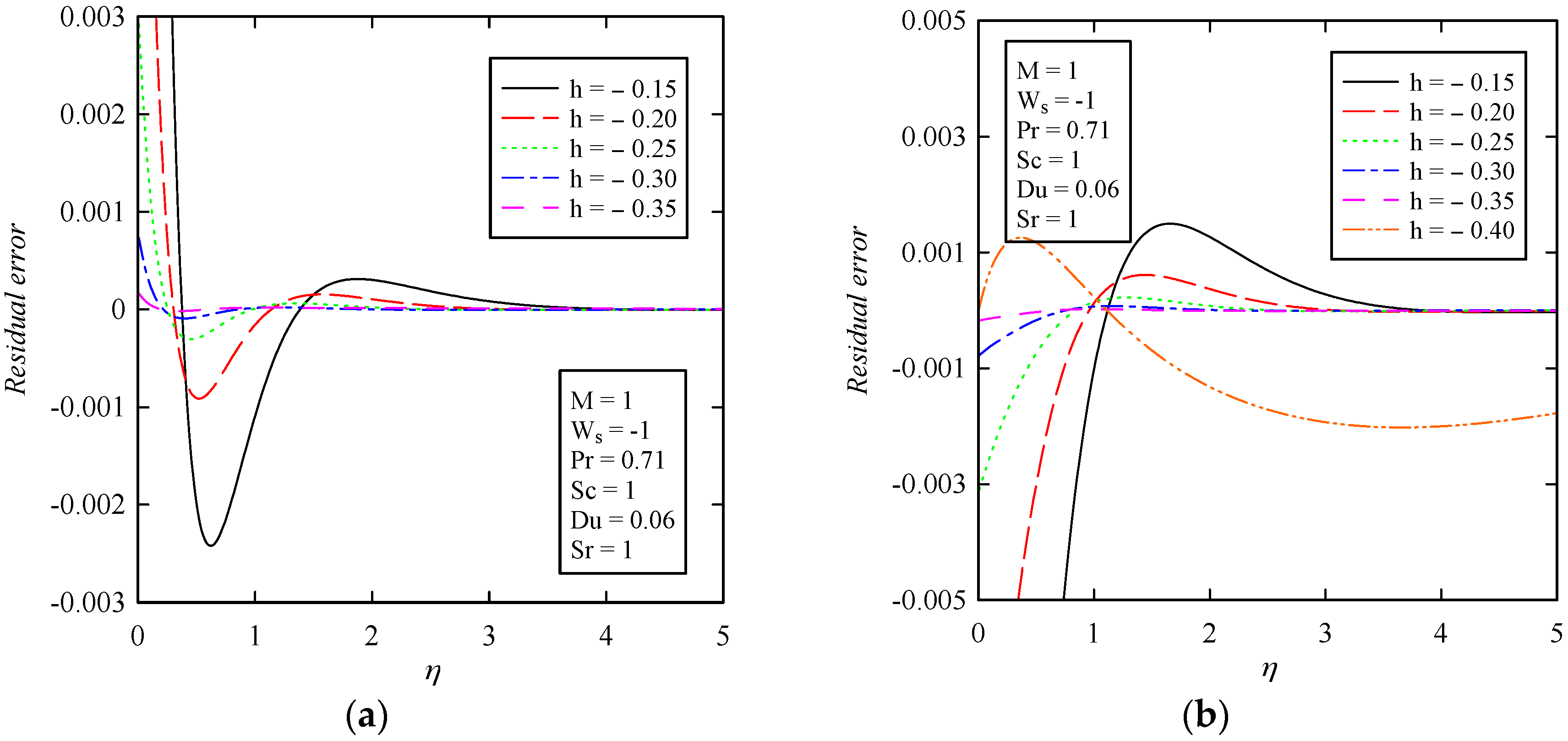
4. HAM Solution
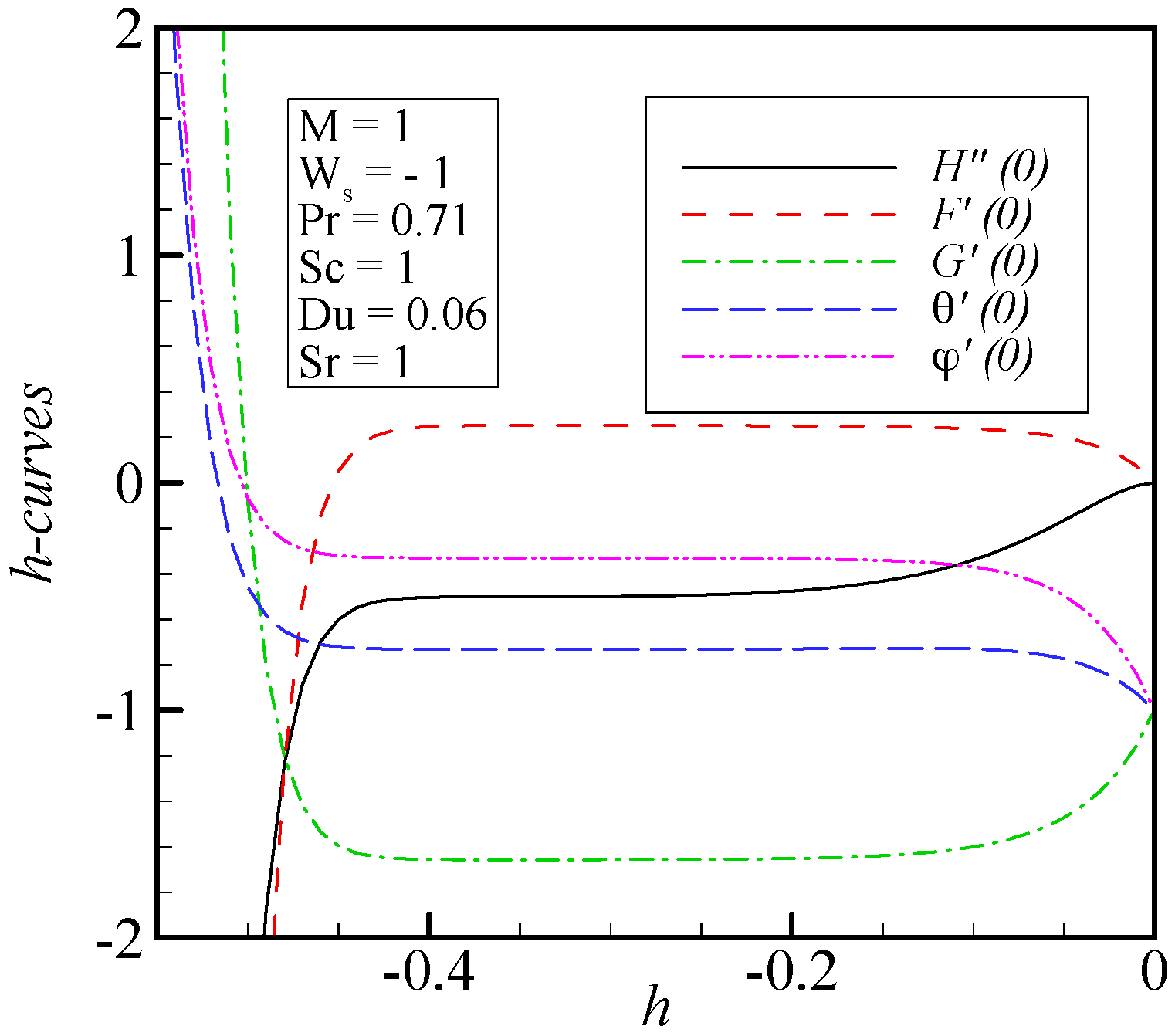
5. Optimal Convergence Control Parameters
6. Results and Discussion
7. Conclusions
- (a)
- HAM is shown to demonstrate excellent potential, convergence and accuracy for simulating flow over rotating disk problems.
- (b)
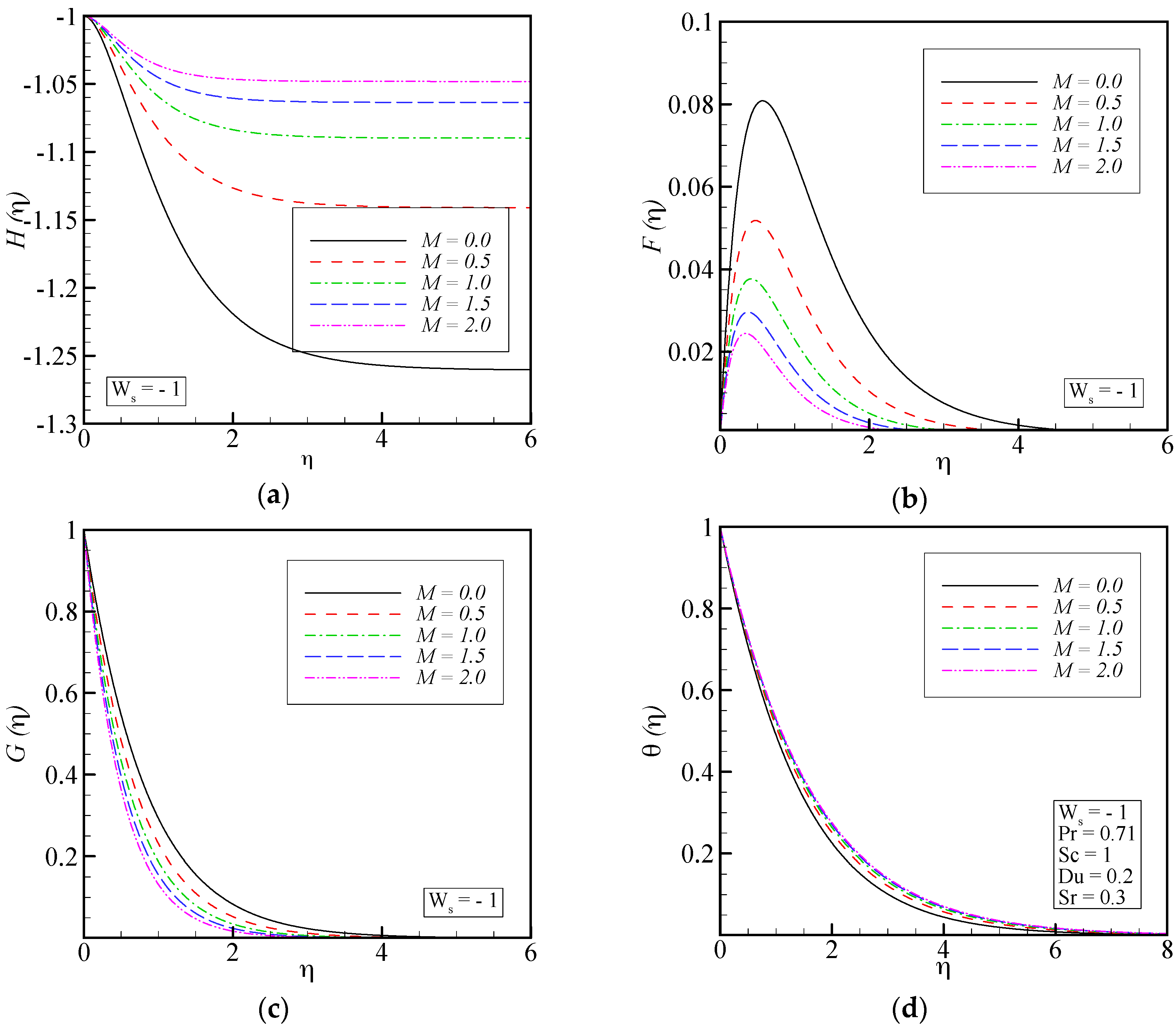
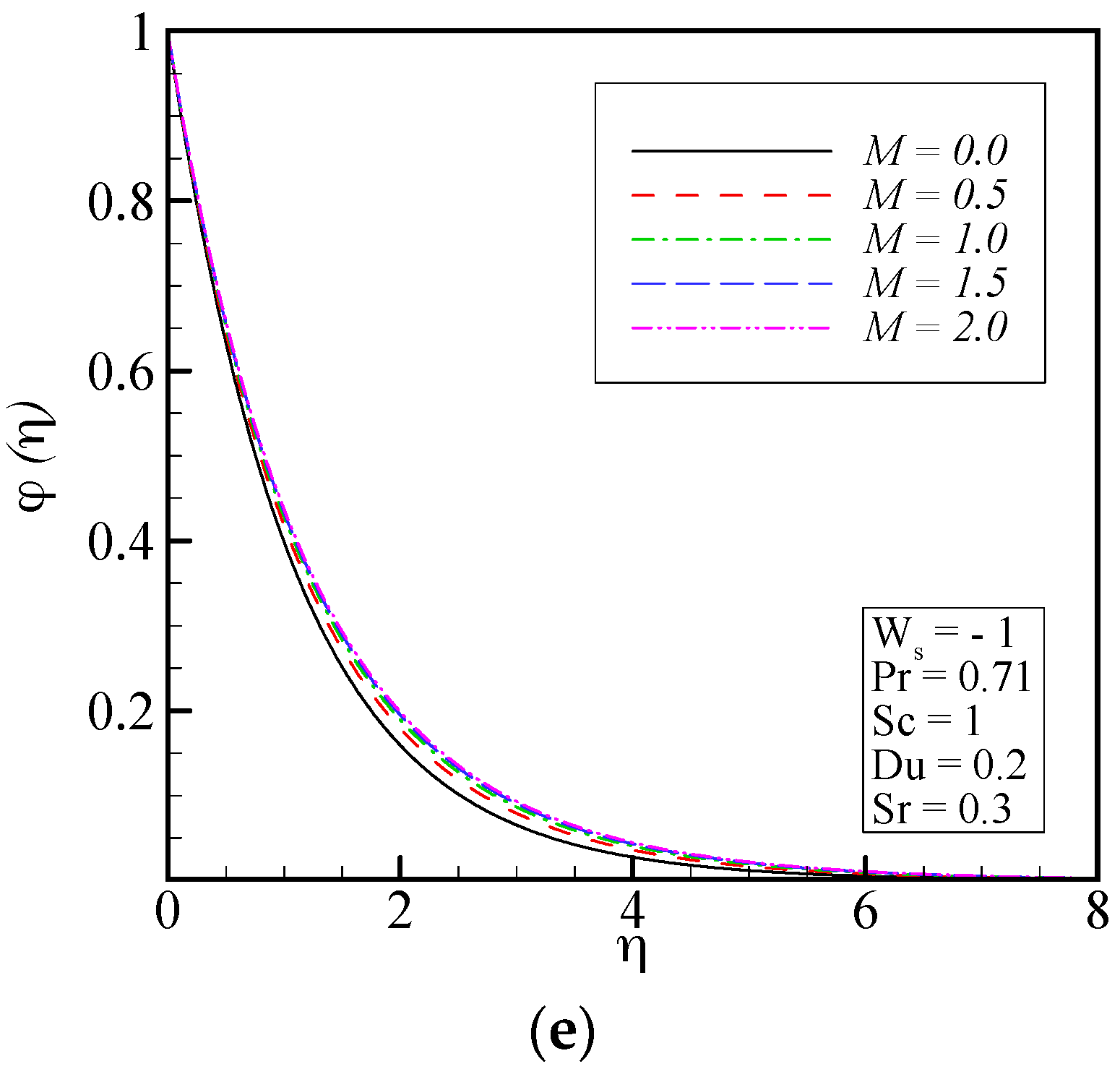
- As the magnetic field becomes stronger, the velocity profiles in radial, tangential and axial directions decrease and the thermal boundary layer and concentration field increase.
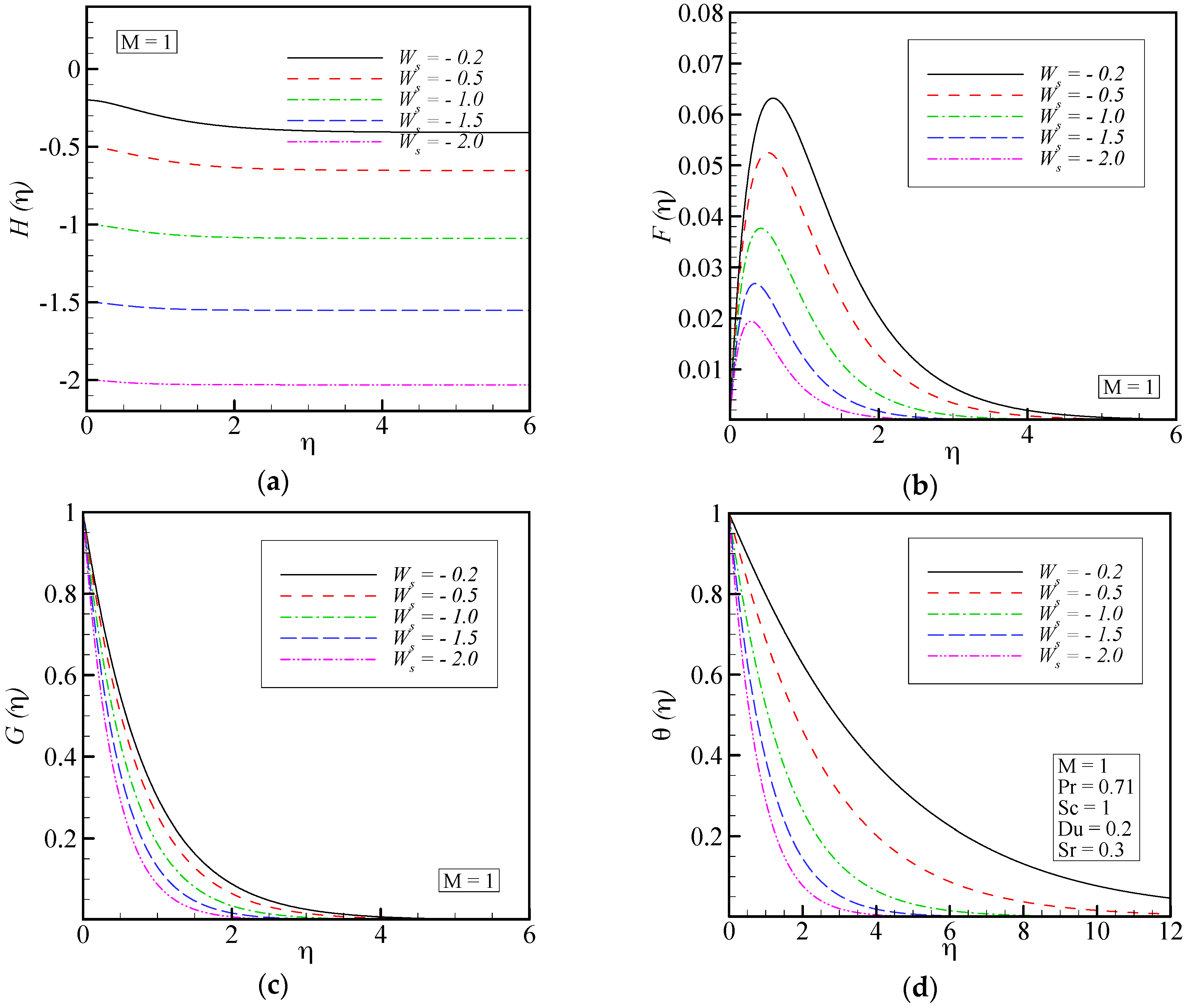
- (c)
- When suction is applied at the disk surface, the radial, tangential and axial velocity profiles decrease. The usual decay of temperature and concentration profiles occurs for larger values of the suction parameter.
- (d)
- The thermal boundary-layer thickness decreases with increasing Prandtl number. Furthermore, as the Schmidt number increases, the concentration boundary layer thickness decreases.
- (e)
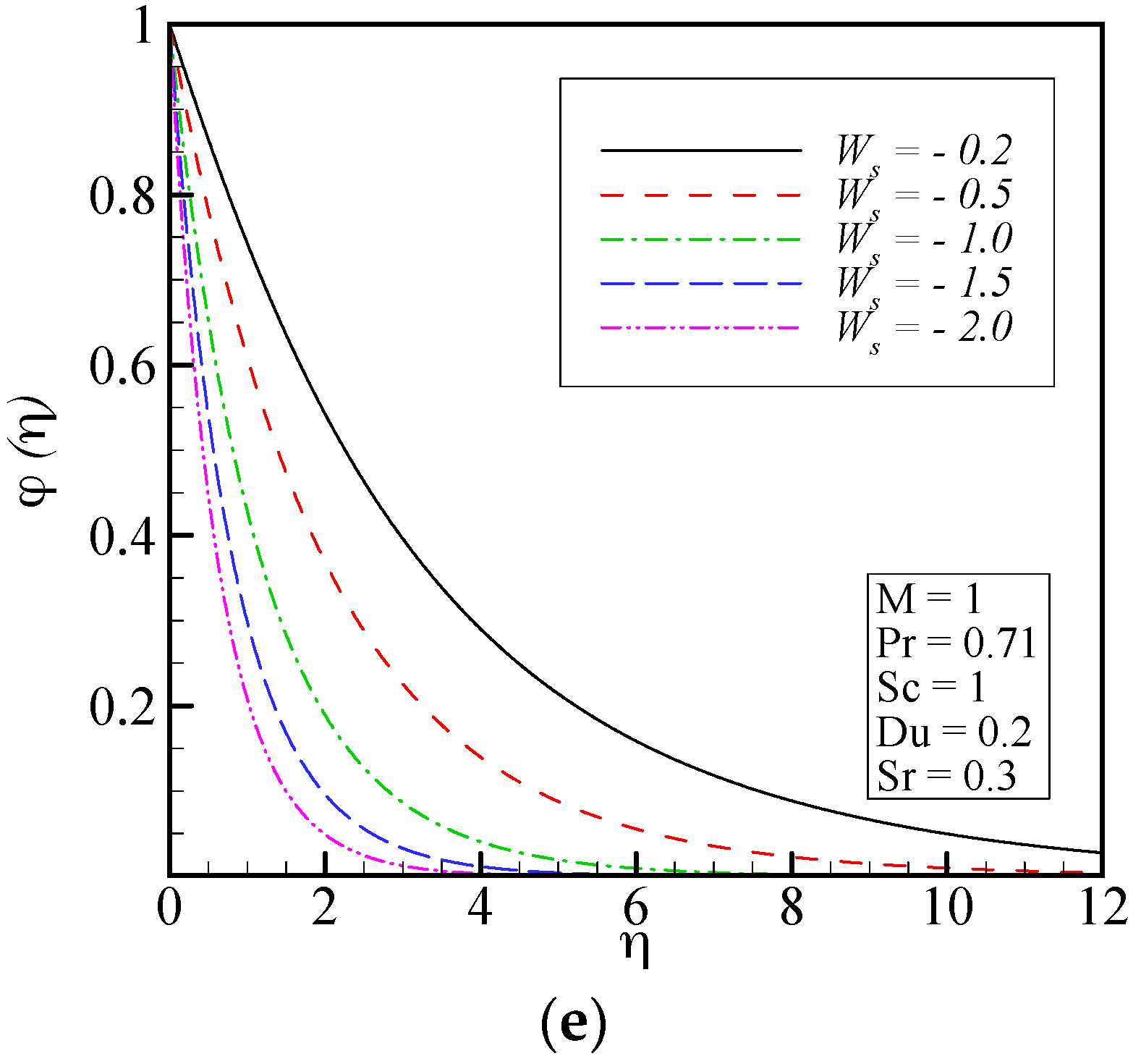
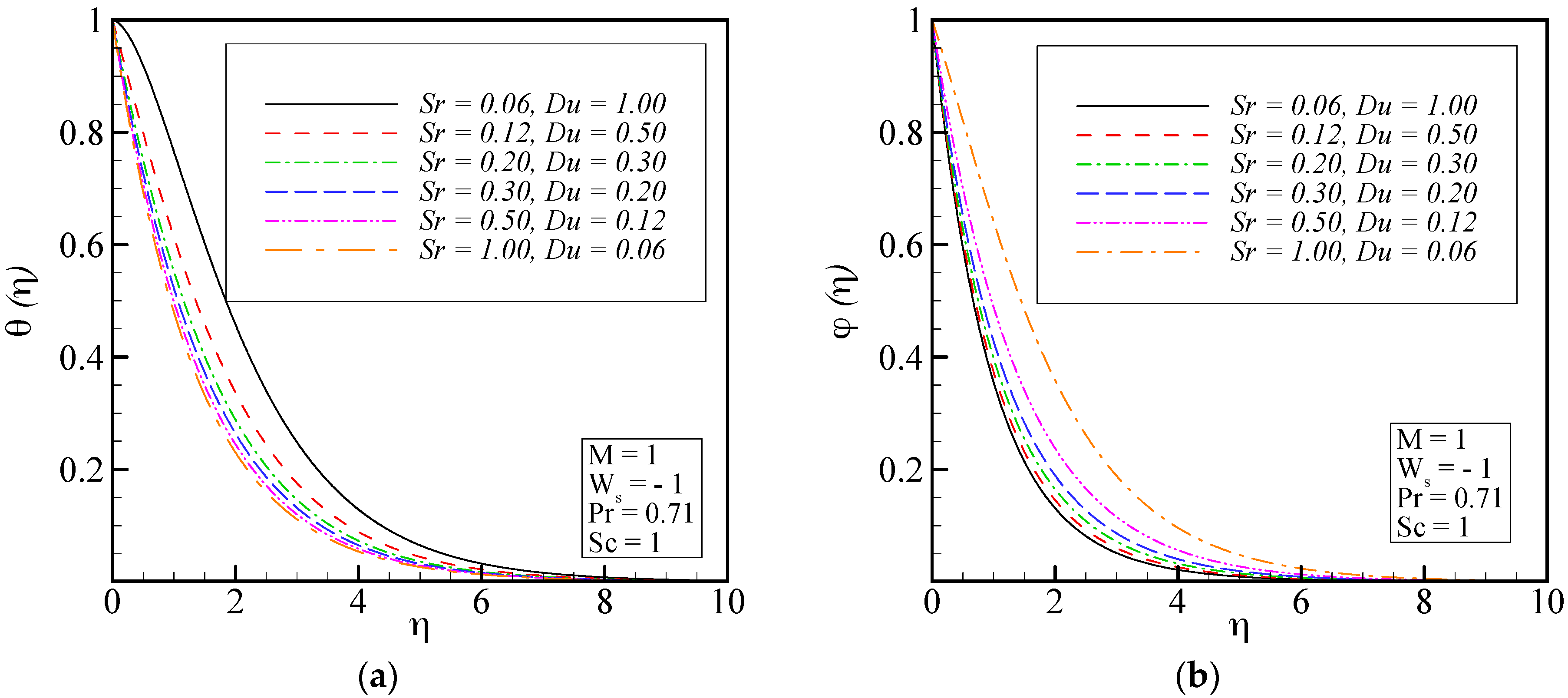
- The thermal boundary layer increases by increasing Dufour number or simultaneously decreasing Soret number. As the Dufour number increases or Soret number decreases, the rate of mass transfer (concentration boundary layer thickness) decreases at the disk.
- (f)
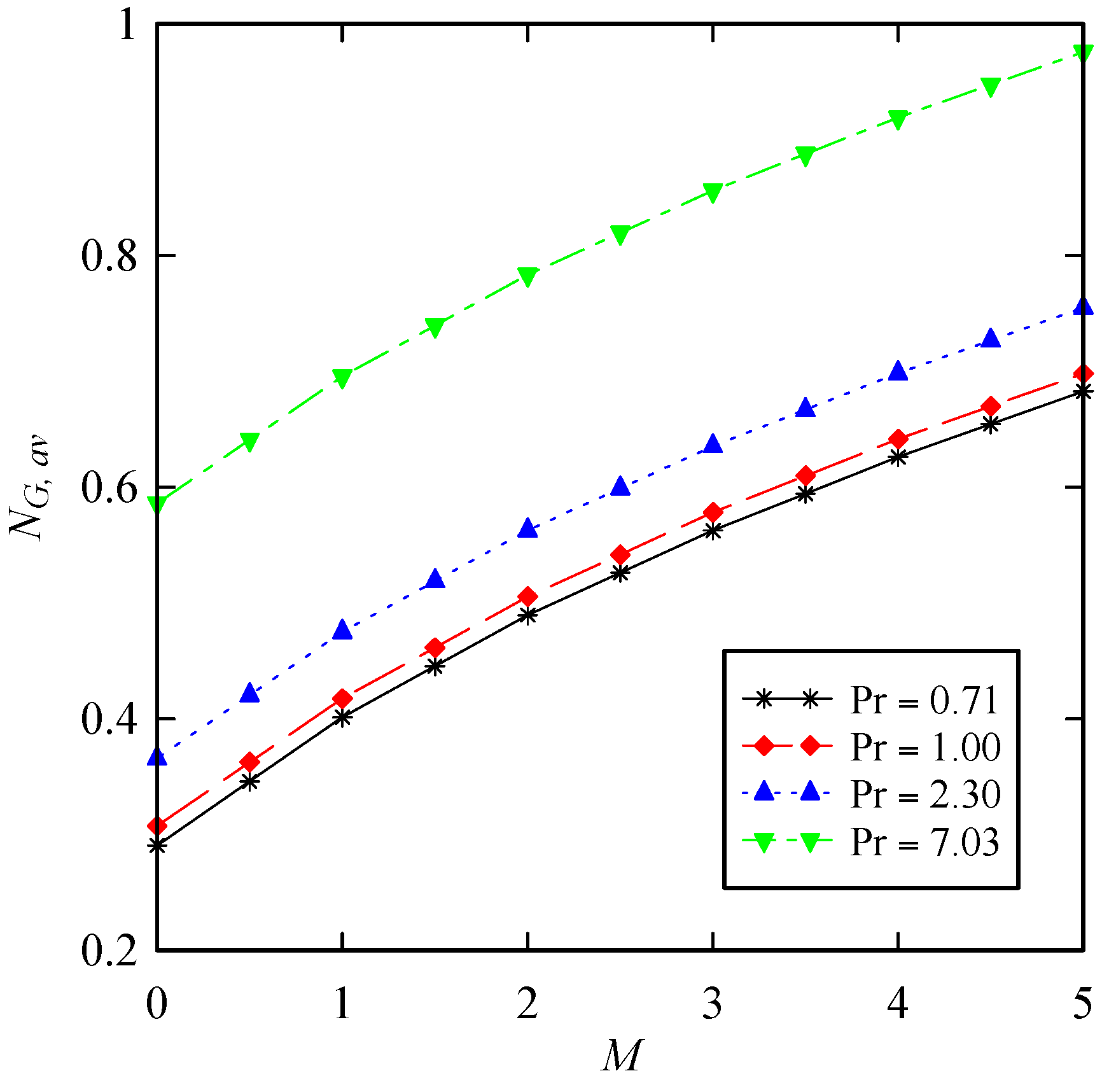
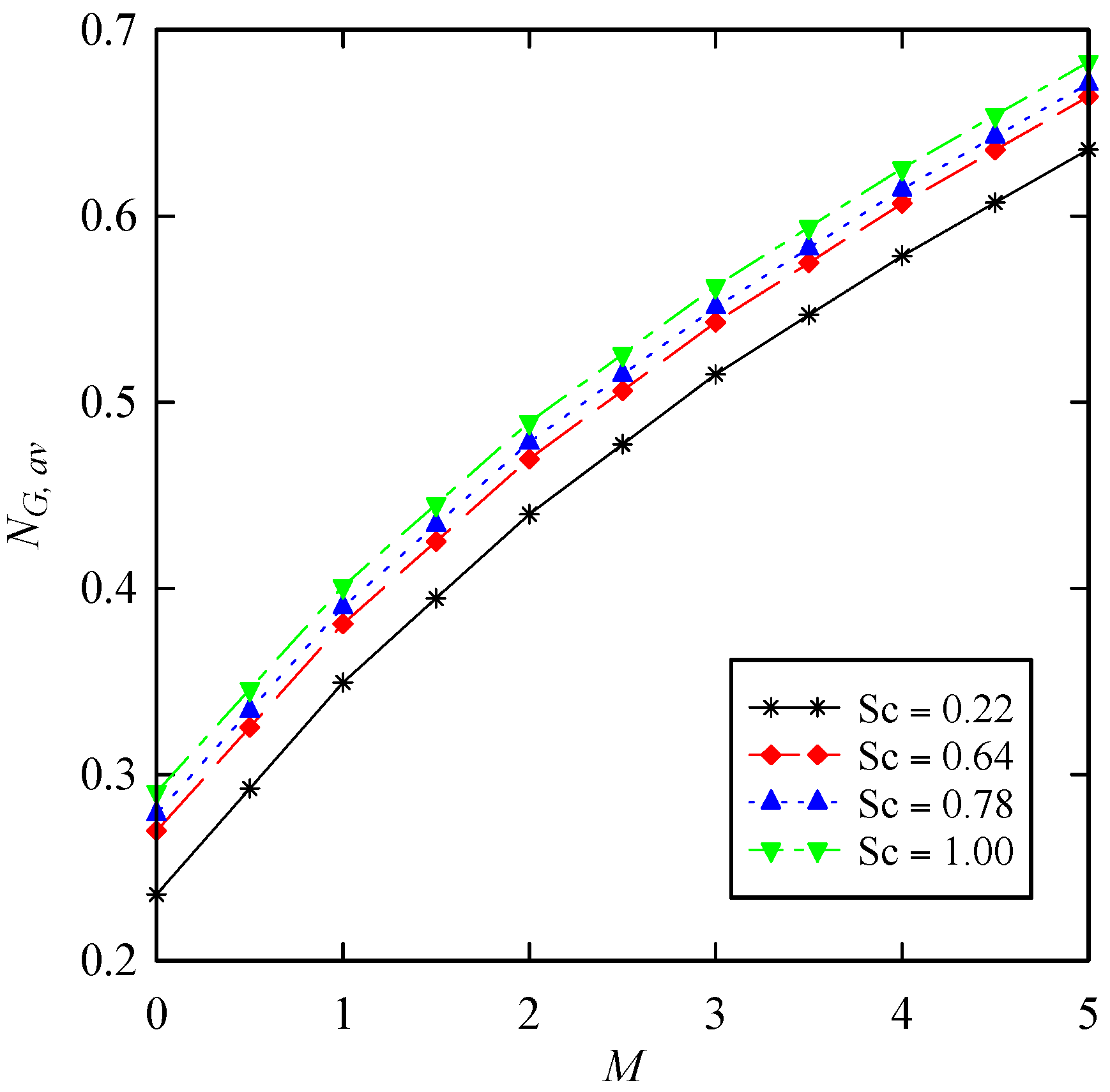
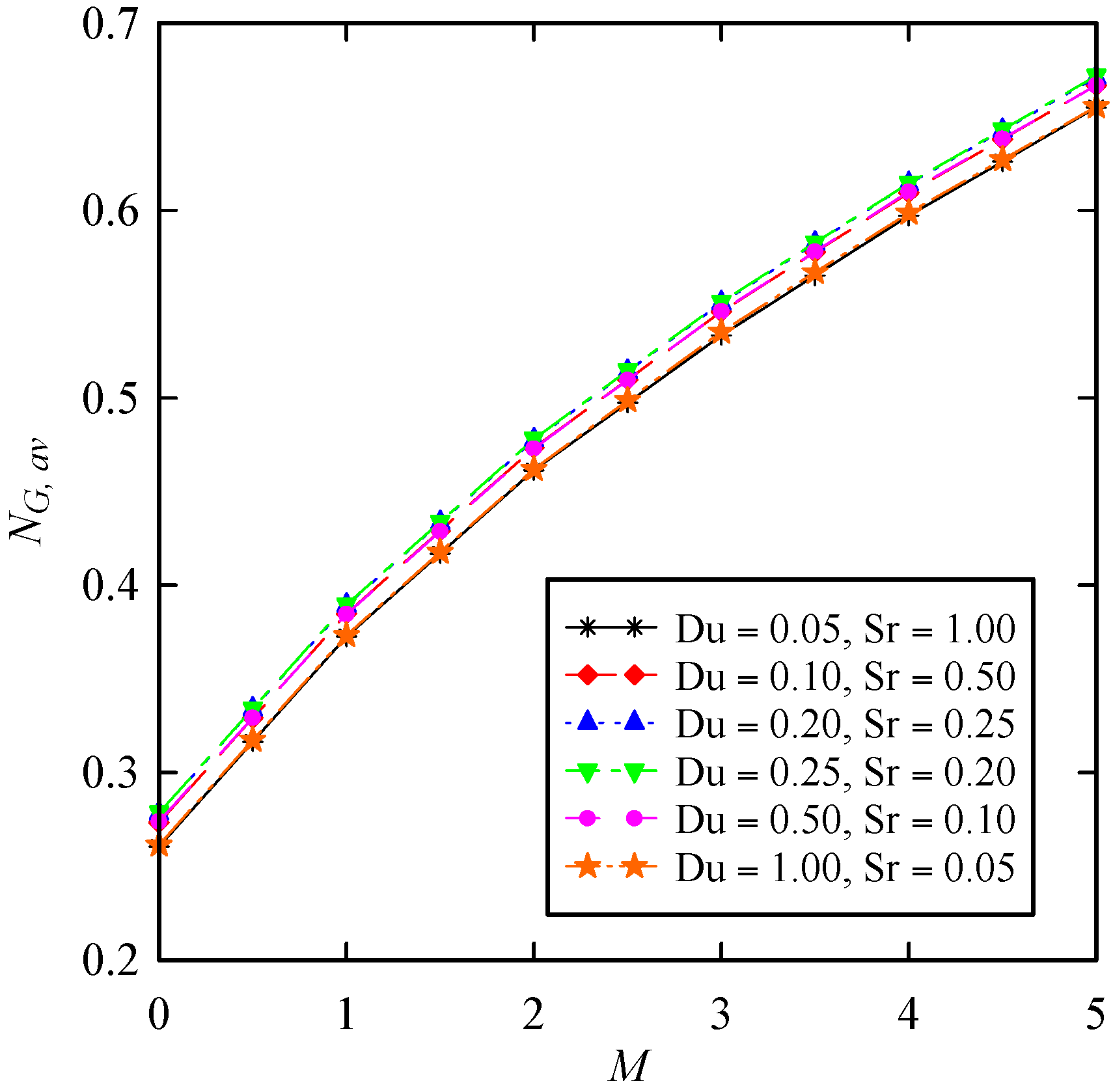
- The averaged entropy generation number increases by increasing the magnetic interaction parameter, suction parameter, Prandtl number, and Schmidt number. In addition, the maximum values of averaged entropy generation number occur when the values of both Soret and Dufour numbers are maximized simultaneously.
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| B | external uniform magnetic field |
| B0 | constant magnetic flux density |
| C | fluid concentration |
| cp | specific heat at constant pressure |
| Cs | concentration susceptibility |
| D | molecular diffusion coefficient |
| E | electric field |
| F | self-similar radial velocity |
| G | self-similar tangential velocity |
| H | self-similar axial velocity |
| J | current density field |
| k | thermal conductivity |
| KT | thermal diffusion ratio |
| L | characteristic length |
| P | pressure |
| Q | electric charge density |
| r | radial direction in cylindrical polar coordinates |
| Rg | ideal gas constant |
| volumetric rate of local entropy generation | |
| characteristic entropy generation rate | |
| T | fluid temperature |
| u | velocity component in the radial directio |
| v | velocity component in the tangential direction |
| w | velocity component in the axial direction |
| w0 | uniform suction |
| z | normal direction in cylindrical polar coordinates |
| Dimensionless parameters | |
| Br | rotational Brinkman number |
| Du | Dufour number |
| NG | entropy generation number |
| M | magnetic interaction parameter |
| Pr | Prandtl number |
| R | dimensionless radial coordinate |
| Re | rotational Reynolds number |
| Sc | Schmidt number |
| Sr | Soret number |
| Ws | suction parameter |
| Greek symbols | |
| α | dimensionless temperature difference |
| β | dimensionless concentration difference |
| λ | diffusive constant parameter |
| η | a scaled boundary-layer coordinate |
| θ | self-similar temperature |
| μ | dynamic viscosity |
| ν | kinematic viscosity |
| ρ | density |
| σ | electrical conductivity |
| φ | self-similar concentration |
| ϕ | tangential direction in cylindrical polar coordinates |
| Φ | viscous dissipation function |
| Ω | angular velocity of the disk |
| volume | |
| Subscripts | |
| av | average condition |
| m | mean condition |
| w | condition of the wall |
| condition of the free steam | |
References
- Kármán, T.V. Über laminare und turbulente reibung. Zeitschrift für Angew volumetric rate of local entropy generation te Mathematik und Mechanik 1921, 1, 233–252. (In German) [Google Scholar] [CrossRef]
- Hayat, T.; Hendi, F.A. Thermal-diffusion and diffusion-thermo effects on MHD three-dimensional axisymmetric flow with hall andion-slip currents. J. Am. Sci. 2012, 8, 284–294. [Google Scholar]
- Devi, S.P.A.; Devi, R.U. Soret and dufour effects on MHD slip flow with thermal radiation over a porous rotating infinite disk. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1917–1930. [Google Scholar] [CrossRef]
- Osalusi, E.; Side, J.; Harris, R. Thermal-diffusion and diffusion-thermo effects on combined heat and mass transfer of a steady MHD convective and slip flow due to a rotating disk with viscous dissipation and ohmic heating. Int. Commun. Heat Mass Transf. 2008, 35, 908–915. [Google Scholar] [CrossRef]
- Das, S.S.; Satapathy, A.; Das, J.K.; Panda, J.P. Mass transfer effects on MHD flow and heat transfer past a vertical porous plate through a porous medium under oscillatory suction and heat source. Int. J. Heat Mass Transf. 2009, 52, 5962–5969. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Hayat, T.; Erfani, E.; Pour, S.A.M.; Hendi, A.A. Simultaneous effects of partial slip and thermal-diffusion and diffusion-thermo on steady MHD convective flow due to a rotating disk. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 4303–4317. [Google Scholar] [CrossRef]
- Hayat, T.; Nawaz, M.; Asghar, S.; Mesloub, S. Thermal-diffusion and diffusion-thermo effects on axisymmetric flow of a second grade fluid. Int. J. Heat Mass Transf. 2011, 54, 3031–3041. [Google Scholar] [CrossRef]
- Pal, D.; Talukdar, B. Influence of fluctuating thermal and mass diffusion on unsteady MHD buoyancy-driven convection past a vertical surface with chemical reaction and soret effects. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 1597–1614. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M.; Pop, I. Soret and heat source effects on the unsteady radiative MHD free convection flow from an impulsively started infinite vertical plate. Int. J. Heat Mass Transf. 2012, 55, 7635–7644. [Google Scholar] [CrossRef]
- Malvandi, A.; Hedayati, F.; Ganji, D.D. Thermodynamic optimization of fluid flow over an isothermal moving plate. Alex. Eng. J. 2013, 52, 277–283. [Google Scholar] [CrossRef]
- Bejan, A. Entropy Generation Minimization: The Method of Thermodynamic Optimization of Finite-Size Systems and Finite-Time Processes; CRC Press: Boca Raton, FL, USA, 1996. [Google Scholar]
- Bejan, A. Second-Law Analysis in Heat Transfer and Thermal Design. In Advances in Heat Transfer; James, P.H., Thomas, F.I., Eds.; Elsevier: Amsterdam, The Netherlands, 1982; Volume 15, pp. 1–58. [Google Scholar]
- Mahian, O.; Mahmud, S.; Heris, S.Z. Analysis of entropy generation between co-rotating cylinders using nanofluids. Energy 2012, 44, 438–446. [Google Scholar] [CrossRef]
- Bejan, A. Second law analysis in heat transfer. Energy 1980, 5, 720–732. [Google Scholar] [CrossRef]
- Oztop, H.F.; Al-Salem, K. A review on entropy generation in natural and mixed convection heat transfer for energy systems. Renew. Sustain. Energy Rev. 2012, 16, 911–920. [Google Scholar] [CrossRef]
- Aïboud, S.; Saouli, S. Second law analysis of viscoelastic fluid over a stretching sheet subject to a transverse magnetic field with heat and mass transfer. Entropy 2010, 12, 1867–1884. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Abelman, S.; Freidoonimehr, N. Entropy generation in steady MHD flow due to a rotating porous disk in a nanofluid. Int. J. Heat Mass Transf. 2013, 62, 515–525. [Google Scholar] [CrossRef]
- Jafari, S.; Freidoonimehr, N. Second law of thermodynamics analysis of hydro-magnetic nano-fluid slip flow over a stretching permeable surface. J. Braz. Soc. Mech. Sci. Eng. 2015, 37, 1245–1256. [Google Scholar] [CrossRef]
- Abolbashari, M.H.; Freidoonimehr, N.; Nazari, F.; Rashidi, M.M. Entropy analysis for an unsteady MHD flow past a stretching permeable surface in nano-fluid. Powder Technol. 2014, 267, 256–267. [Google Scholar] [CrossRef]
- Abolbashari, M.H.; Freidoonimehr, N.; Nazari, F.; Rashidi, M.M. Analytical modeling of entropy generation for casson nano-fluid flow induced by a stretching surface. Adv. Powder Technol. 2015, 26, 542–552. [Google Scholar] [CrossRef]
- Ellahi, R.; Hassan, M.; Zeeshan, A. Shape effects of nanosize particles in nanofluid on entropy generation. Int. J. Heat Mass Transf. 2015, 81, 449–456. [Google Scholar] [CrossRef]
- Freidoonimehr, N.; Rashidi, M.M.; Mahmud, S. Unsteady MHD free convective flow past a permeable stretching vertical surface in a nano-fluid. Int. J. Therm. Sci. 2015, 87, 136–145. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Mahmud, S.; Freidoonimehr, N.; Rostami, B. Analysis of entropy generation in an MHD flow over a rotating porous disk with variable physical properties. Int. J. Exergy 2015, 16, 481–503. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Kavyani, N.; Abelman, S.; Uddin, M.J.; Freidoonimehr, N. Double diffusive magnetohydrodynamic (MHD) mixed convective slip flow along a radiating moving vertical flat plate with convective boundary condition. PLoS ONE 2014, 9, e109404. [Google Scholar] [CrossRef] [PubMed]
- Rashidi, M.M.; Freidoonimehr, N.; Momoniat, E.; Rostami, B. Study of nonlinear mhd tribological squeeze film at generalized magnetic reynolds numbers using dtm. PLoS ONE 2015, 10, e0135004. [Google Scholar] [CrossRef] [PubMed]
- Freidoonimehr, N.; Rostami, B.; Rashidi, M.M.; Momoniat, E. Analytical modelling of three-dimensional squeezing nanofluid flow in a rotating channel on a lower stretching porous wall. Math. Probl. Eng. 2014, 2014, 692728. [Google Scholar] [CrossRef]
- Liao, S.J. Beyond Perturbation: Introduction to the Homotopy Analysis Method; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Liao, S.J. On the homotopy analysis method for nonlinear problems. Appl. Math. Comput. 2004, 147, 499–513. [Google Scholar] [CrossRef]
- Liao, S.J. An explicit, totally analytic approximation of blasius viscous flow problems. Int. J. Non-Linear Mech. 1999, 34, 759–778. [Google Scholar] [CrossRef]
- Awad, M.M. Heat transfer from a rotating disk to fluids for a wide range of Prandtl numbers using the asymptotic model. J. Heat Transfer 2008, 130, 014505. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Ali, M.; Freidoonimehr, N.; Rostami, B.; Hossain, M.A. Mixed convective heat transfer for MHD viscoelastic fluid flow over a porous wedge with thermal radiation. Adv. Mech. Eng. 2014, 2014, 735939. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Rostami, B.; Freidoonimehr, N.; Abbasbandy, S. Free convective heat and mass transfer for MHD fluid flow over a permeable vertical stretching sheet in the presence of the radiation and buoyancy effects. Ain Shams Eng. J. 2014, 5, 901–912. [Google Scholar] [CrossRef]
- Freidoonimehr, N.; Rostami, B.; Rashidi, M.M. Predictor homotopy analysis method for nanofluid flow through expanding or contracting gaps with permeable walls. Int. J. Biomath. 2015, 8, 1550050. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Analytic approximate solutions of rotating disk boundary layer flow subject to a uniform suction or injection. Int. J. Mech. Sci. 2010, 52, 1735–1744. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ellahi, R. Electrohydrodynamic nanofluid hydrothermal treatment in an enclosure with sinusoidal upper wall. Appl. Sci. 2015, 5, 294–306. [Google Scholar] [CrossRef]
- Rashidi, S.; Dehghan, M.; Ellahi, R.; Riaz, M.; Jamal-Abad, M.T. Study of stream wise transverse magnetic fluid flow with heat transfer around an obstacle embedded in a porous medium. J. Magn. Magn. Mater. 2015, 378, 128–137. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ellahi, R. Three dimensional mesoscopic simulation of magnetic field effect on natural convection of nanofluid. Int. J. Heat Mass Transf. 2015, 89, 799–808. [Google Scholar] [CrossRef]
- Ellahi, R.; Hassan, M.; Zeeshan, A. Study of natural convection MHD nanofluid by means of single and multi-walled carbon nanotubes suspended in a salt-water solution. IEEE Trans. Nanotechnol. 2015, 14, 726–734. [Google Scholar] [CrossRef]
- Akbar, N.S.; Raza, M.; Ellahi, R. Influence of induced magnetic field and heat flux with the suspension of carbon nanotubes for the peristaltic flow in a permeable channel. J. Magn. Magn. Mater. 2015, 381, 405–415. [Google Scholar] [CrossRef]
- Ellahi, R.; Rahman, S.U.; Nadeem, S.; Vafai, K. The blood flow of Prandtl fluid through a tapered stenosed arteries in permeable walls with magnetic field. Commun. Theor. Phys. 2015, 63, 353–358. [Google Scholar] [CrossRef]
- Ellahi, R.; Shivanian, E.; Abbasbandy, S.; Hayat, T. Analysis of some magnetohydrodynamic flows of third-order fluid saturating porous space. J. Porous Media 2015, 18, 89–98. [Google Scholar] [CrossRef]
- Kandelousi, M.S.; Ellahi, R. Simulation of ferrofluid flow for magnetic drug targeting using the lattice boltzmann method. Zeitschrift für Naturforschung A 2015, 70, 115–124. [Google Scholar] [CrossRef]
- Bejan, A. Entropy generation minimization: The new thermodynamics of finite-size devices and finite-time processes. J. Appl. Phys. 1996, 79, 1191–1218. [Google Scholar] [CrossRef]
- Bejan, A. Entropy Generation through Heat and Fluid Flow; Wiley: New York, NY, USA, 1982. [Google Scholar]
- Magherbi, M.; Abbassi, H.; Hidouri, N.; Brahim, A. Second law analysis in convective heat and mass transfer. Entropy 2006, 8, 1–17. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. An effective approach for approximate analytical solutions of the damped duffing equation. Phys. Scr. 2012, 86, 015301. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. The Airy equation and its alternative analytic solution. Phys. Scr. 2012, 86, 055004. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Freidoonimehr, N.; Hosseini, A.; Bég, O.A.; Hung, T.K. Homotopy simulation of nanofluid dynamics from a non-linearly stretching isothermal permeable sheet with transpiration. Meccanica 2014, 49, 469–482. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Purely analytic solutions of magnetohydrodynamic swirling boundary layer flow over a porous rotating disk. Comput. Fluid. 2010, 39, 793–799. [Google Scholar] [CrossRef]
- Kelson, N.; Desseaux, A. Note on porous rotating disk flow. ANZIAM J. 2000, 42, 837–855. [Google Scholar]
- Postelnicu, A. Influence of a magnetic field on heat and mass transfer by natural convection from vertical surfaces in porous media considering soret and dufour effects. Int. J. Heat Mass Transf. 2004, 47, 1467–1472. [Google Scholar] [CrossRef]
- Kafoussias, N.G.; Williams, E.W. Thermal-diffusion and diffusion-thermo effects on mixed free-forced convective and mass transfer boundary layer flow with temperature dependent viscosity. Int. J. Eng. Sci. 1995, 33, 1369–1384. [Google Scholar] [CrossRef]



© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Freidoonimehr, N.; Rashidi, M.M.; Abelman, S.; Lorenzini, G. Analytical Modeling of MHD Flow over a Permeable Rotating Disk in the Presence of Soret and Dufour Effects: Entropy Analysis. Entropy 2016, 18, 131. https://doi.org/10.3390/e18050131
Freidoonimehr N, Rashidi MM, Abelman S, Lorenzini G. Analytical Modeling of MHD Flow over a Permeable Rotating Disk in the Presence of Soret and Dufour Effects: Entropy Analysis. Entropy. 2016; 18(5):131. https://doi.org/10.3390/e18050131
Chicago/Turabian StyleFreidoonimehr, Navid, Mohammad Mehdi Rashidi, Shirley Abelman, and Giulio Lorenzini. 2016. "Analytical Modeling of MHD Flow over a Permeable Rotating Disk in the Presence of Soret and Dufour Effects: Entropy Analysis" Entropy 18, no. 5: 131. https://doi.org/10.3390/e18050131
APA StyleFreidoonimehr, N., Rashidi, M. M., Abelman, S., & Lorenzini, G. (2016). Analytical Modeling of MHD Flow over a Permeable Rotating Disk in the Presence of Soret and Dufour Effects: Entropy Analysis. Entropy, 18(5), 131. https://doi.org/10.3390/e18050131








