Rényi Divergences, Bures Geometry and Quantum Statistical Thermodynamics
Abstract
:1. Introduction
2. The Space of Density Operators
2.1. The Bures Geometry
2.2. The Rényi α-Divergences
3. Results
3.1. Fundamental Relations
3.2. Geometry, Entropy, and the Thermodynamical Free Energy
3.3. Work and Distance
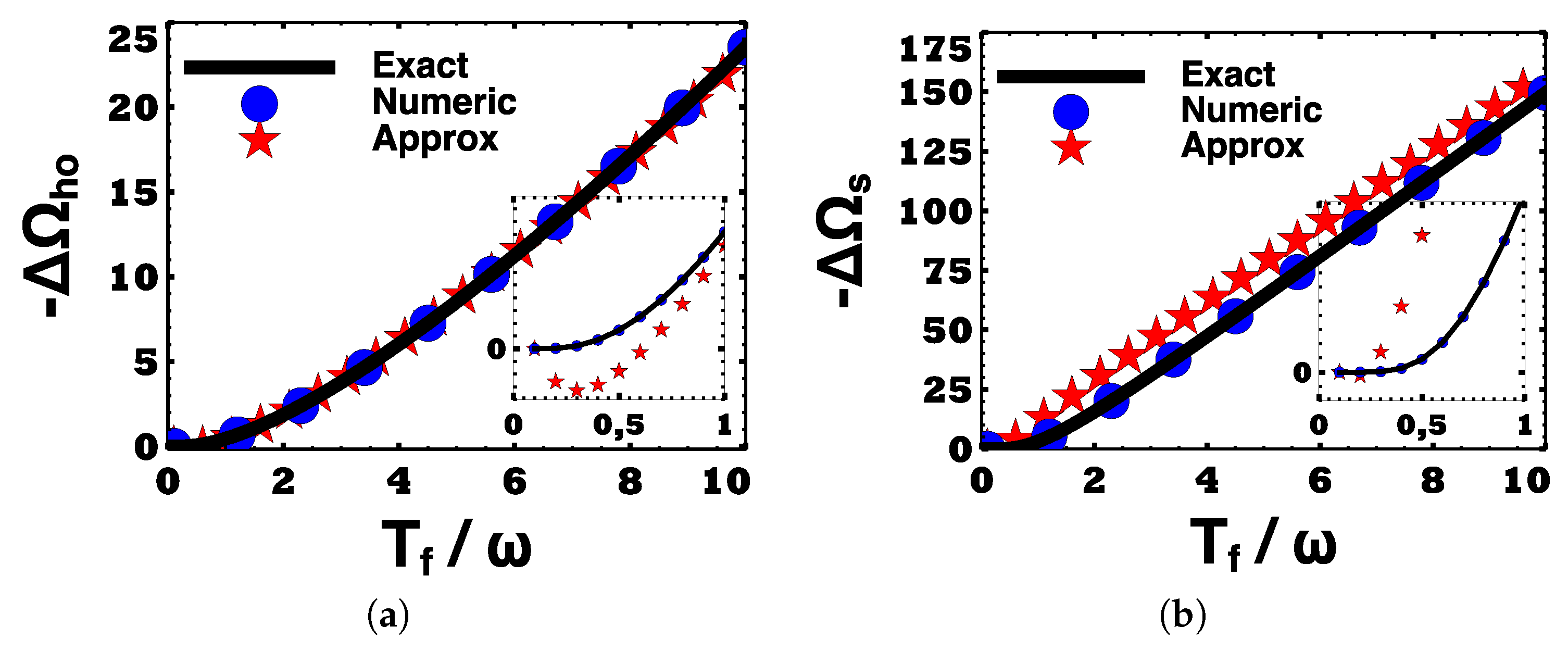
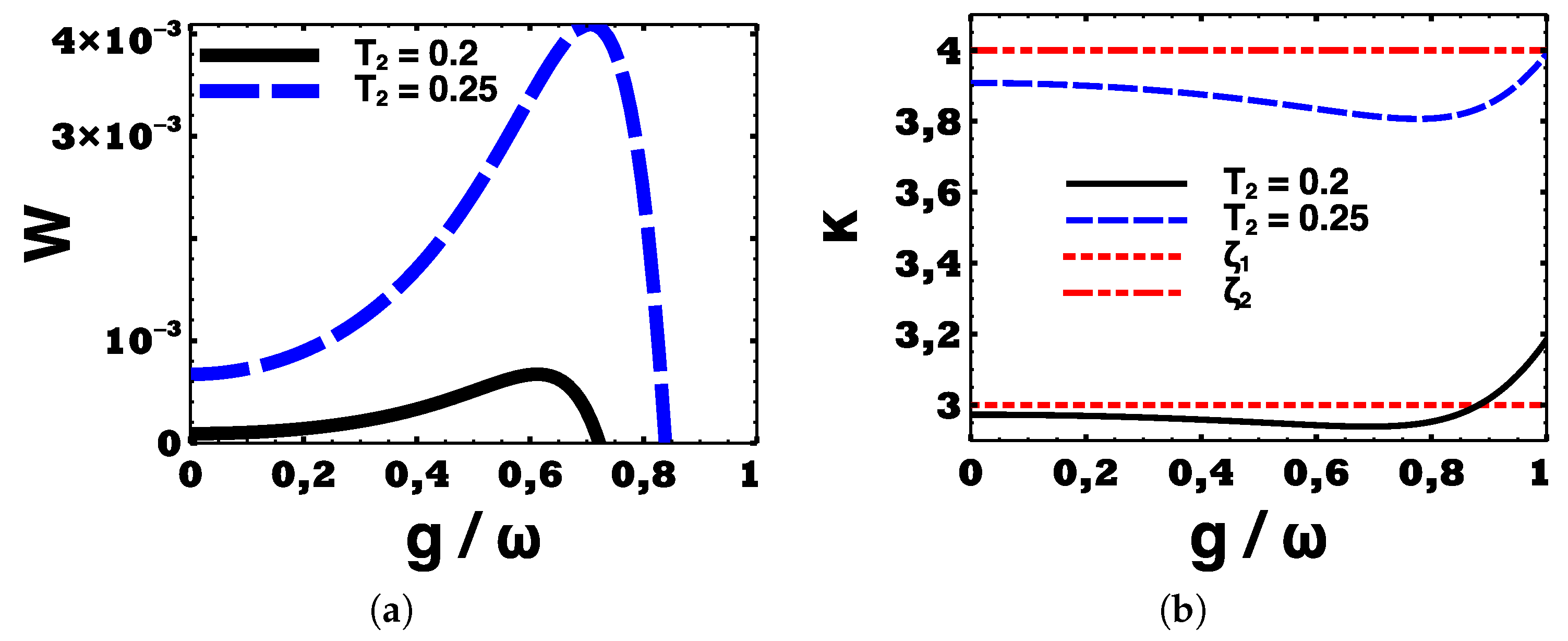
3.4. Examples
4. Concluding Remarks
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Galilei, G.; Drake, S. Il Saggiatore (The Assayer) in Discoveries and Opinions of Galileo; Anchor Books: New York, NY, USA, 1957. [Google Scholar]
- Gibbs, J.W. Graphical methods in the thermodynamics of fluids. Trans. Conn. Acad. 1873, 2, 309–342. [Google Scholar]
- Gibbs, J.W. A Method of geometrical representation of the thermodynamic properties of substances by means of surfaces. Trans. Conn. Acad. 1873, 2, 382–404. [Google Scholar]
- Weinhold, F. Metric geometry of equilibrium thermodynamics. J. Chem. Phys. 1975, 63, 2479–2483. [Google Scholar] [CrossRef]
- Ruppeiner, G. Thermodynamics: A Riemannian geometric model. Phys. Rev. A 1979, 20, 1608. [Google Scholar] [CrossRef]
- Ruppeiner, G. Application of Riemannian geometry to the thermodynamics of a simple fluctuating magnetic system. Phys. Rev. A 1981, 24, 488–492. [Google Scholar] [CrossRef]
- Ruppeiner, G. Riemannian geometry in thermodynamic fluctuation theory. Rev. Mod. Phys. 1995, 67, 605–659. [Google Scholar] [CrossRef]
- Descartes, R.; Maclean, I. A Discourse on the Method; Oxford University Press: New York, NY, USA, 2006. [Google Scholar]
- Bengtsson, I.; Zyczkowski, K. Geometry of Quantum States: An Introduction to Quantum Entanglement; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Bures, D. An extension of Kakutani’s theorem on infinite product measures to the tensor product of semifinite W*-algebras. Trans. Am. Math. Soc. 1969, 135, 199–212. [Google Scholar]
- Twamley, J. Bures and statistical distance for squeezed thermal states. J. Phys. A 1996, 29, 3723. [Google Scholar] [CrossRef]
- Deffner, S.; Lutz, E. Nonequilibrium entropy production for open quantum systems. Phys. Rev. Lett. 2011, 107, 140404. [Google Scholar] [CrossRef] [PubMed]
- Deffner, S.; Lutz, E. Thermodynamic length for far-from-equilibrium quantum systems. Phys. Rev. E 2013, 87, 022143. [Google Scholar] [CrossRef] [PubMed]
- Zanardi, P.; Venuti, L.C.; Giorda, P. Bures metric over thermal state manifolds and quantum criticality. Phys. Rev. A 2007, 76, 062318. [Google Scholar] [CrossRef]
- Zanardi, P.; Giorda, P.; Cozzini, M. Information-theoretic differential geometry of quantum phase transitions. Phys. Rev. Lett. 2007, 99, 100603. [Google Scholar] [CrossRef] [PubMed]
- You, W.L.; Li, Y.W.; Gu, S.J. Fidelity, dynamic structure factor, and susceptibility in critical phenomena. Phys. Rev. E 2007, 76, 022101. [Google Scholar] [CrossRef] [PubMed]
- Plastina, F.; Alecce, A.; Apollaro, T.; Falcone, G.; Francica, G.; Galve, F.; Gullo, N.L.; Zambrini, R. Irreversible work and inner friction in quantum thermodynamic processes. Phys. Rev. Lett. 2014, 113, 260601. [Google Scholar] [CrossRef] [PubMed]
- Renyi, A. On measures of entropy and information. Berkeley Symp. Math. Stat. Probab. 1961, 1, 547–561. [Google Scholar]
- Müller-Lennert, M.; Dupuis, F.; Szehr, O.; Fehr, S.; Tomamichel, M. On quantum Rényi entropies: A new generalization and some properties. J. Math. Phys. 2013, 54, 122203. [Google Scholar] [CrossRef]
- Plastino, A.; Plastino, A.R. On the universality of thermodynamics’ Legendre transform structure. Phys. Lett. A 1997, 226, 257–263. [Google Scholar] [CrossRef]
- Lenzi, E.; Mendes, R.; Da Silva, L. Statistical mechanics based on Renyi entropy. Physica A 2000, 280, 337–345. [Google Scholar] [CrossRef]
- Misra, A.; Singh, U.; Bera, M.N.; Rajagopal, A. Quantum Rényi relative entropies affirm universality of thermodynamics. Phys. Rev. E 2015, 92, 042161. [Google Scholar] [CrossRef] [PubMed]
- Horodecki, M.; Oppenheim, J. Fundamental limitations for quantum and nanoscale thermodynamics. Nat. Commun. 2013, 4, 2059. [Google Scholar] [CrossRef] [PubMed]
- Brandão, F.; Horodecki, M.; Ng, N.; Oppenheim, J.; Wehner, S. The second laws of quantum thermodynamics. Proc. Natl. Acad. Sci. USA 2015, 112, 3275–3279. [Google Scholar] [CrossRef] [PubMed]
- Lostaglio, M.; Jennings, D.; Rudolph, T. Description of quantum coherence in thermodynamic processes requires constraints beyond free energy. Nat. Commun. 2015, 6, 6383. [Google Scholar] [CrossRef] [PubMed]
- Uhlmann, A. The metric of Bures and the geometric phase. In Groups and Related Topics; Springer: Berlin/Heidelberg, Germany, 1992; pp. 267–274. [Google Scholar]
- Uhlmann, A. Density operators as an arena for differential geometry. Rep. Math. Phys. 1993, 33, 253–263. [Google Scholar] [CrossRef]
- Uhlmann, A. Spheres and hemispheres as quantum state spaces. J. Geom. Phys. 1996, 18, 76–92. [Google Scholar] [CrossRef]
- Uhlmann, A. On Berry phases along mixtures of states. Annalen der Physik 1989, 501, 63–69. [Google Scholar] [CrossRef]
- Viyuela, O.; Rivas, A.; Martin-Delgado, M. Symmetry-protected topological phases at finite temperature. 2D Mater. 2015, 2, 034006. [Google Scholar] [CrossRef]
- Uhlmann, A. An energy dispersion estimate. Phys. Lett. A 1992, 161, 329–331. [Google Scholar] [CrossRef]
- Uhlmann, A.; Crell, B. Geometry of state spaces. In Entanglement and Decoherence; Springer: Berlin/Heidelberg, Germany, 2009; pp. 1–60. [Google Scholar]
- Wilde, M.M.; Winter, A.; Yang, D. Strong converse for the classical capacity of entanglement-breaking and Hadamard channels via a sandwiched Rényi relative entropy. Commun. Math. Phys. 2014, 331, 593–622. [Google Scholar] [CrossRef]
- Mosonyi, M.; Ogawa, T. Quantum hypothesis testing and the operational interpretation of the quantum Rényi relative entropies. Commun. Math. Phys. 2014, 334, 1617–1648. [Google Scholar] [CrossRef]
- Wootters, W.K. Statistical distance and Hilbert space. Phys. Rev. D 1981, 23, 357–362. [Google Scholar] [CrossRef]
- Jarzynski, C. Nonequilibrium equality for free energy differences. Phys. Rev. Lett. 1997, 78, 2690. [Google Scholar] [CrossRef]
- Francica, G.; Montangero, S.; Paternostro, M.; Plastina, F. The driven Dicke Model: Time-dependent mean field and quantum fluctuations in a non-equilibrium quantum many-body system. arXiv 2016. [Google Scholar]
- Altintas, F.; Hardal, A.Ü.C.; Müstecaplıoğlu, Ö.E. Rabi model as a quantum coherent heat engine: From quantum biology to superconducting circuits. Phys. Rev. A 2015, 91, 023816. [Google Scholar] [CrossRef]
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hardal, A.Ü.C.; Müstecaplıoğlu, Ö.E. Rényi Divergences, Bures Geometry and Quantum Statistical Thermodynamics. Entropy 2016, 18, 455. https://doi.org/10.3390/e18120455
Hardal AÜC, Müstecaplıoğlu ÖE. Rényi Divergences, Bures Geometry and Quantum Statistical Thermodynamics. Entropy. 2016; 18(12):455. https://doi.org/10.3390/e18120455
Chicago/Turabian StyleHardal, Ali Ümit Cemal, and Özgür Esat Müstecaplıoğlu. 2016. "Rényi Divergences, Bures Geometry and Quantum Statistical Thermodynamics" Entropy 18, no. 12: 455. https://doi.org/10.3390/e18120455
APA StyleHardal, A. Ü. C., & Müstecaplıoğlu, Ö. E. (2016). Rényi Divergences, Bures Geometry and Quantum Statistical Thermodynamics. Entropy, 18(12), 455. https://doi.org/10.3390/e18120455




