Entropy Content During Nanometric Stick-Slip Motion
Abstract
:1. Introduction
2. Methods
2.1. Molecular Dynamics
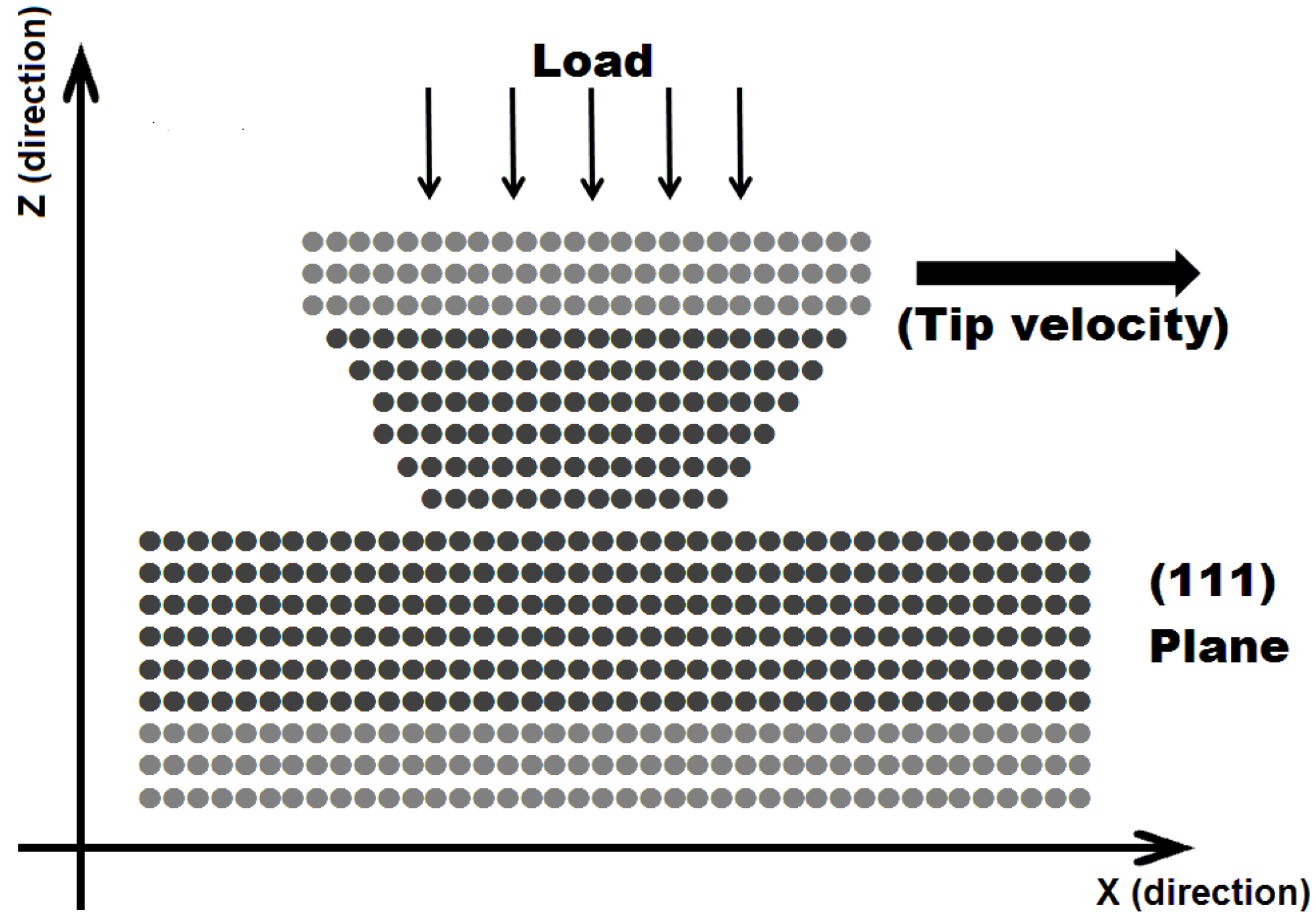
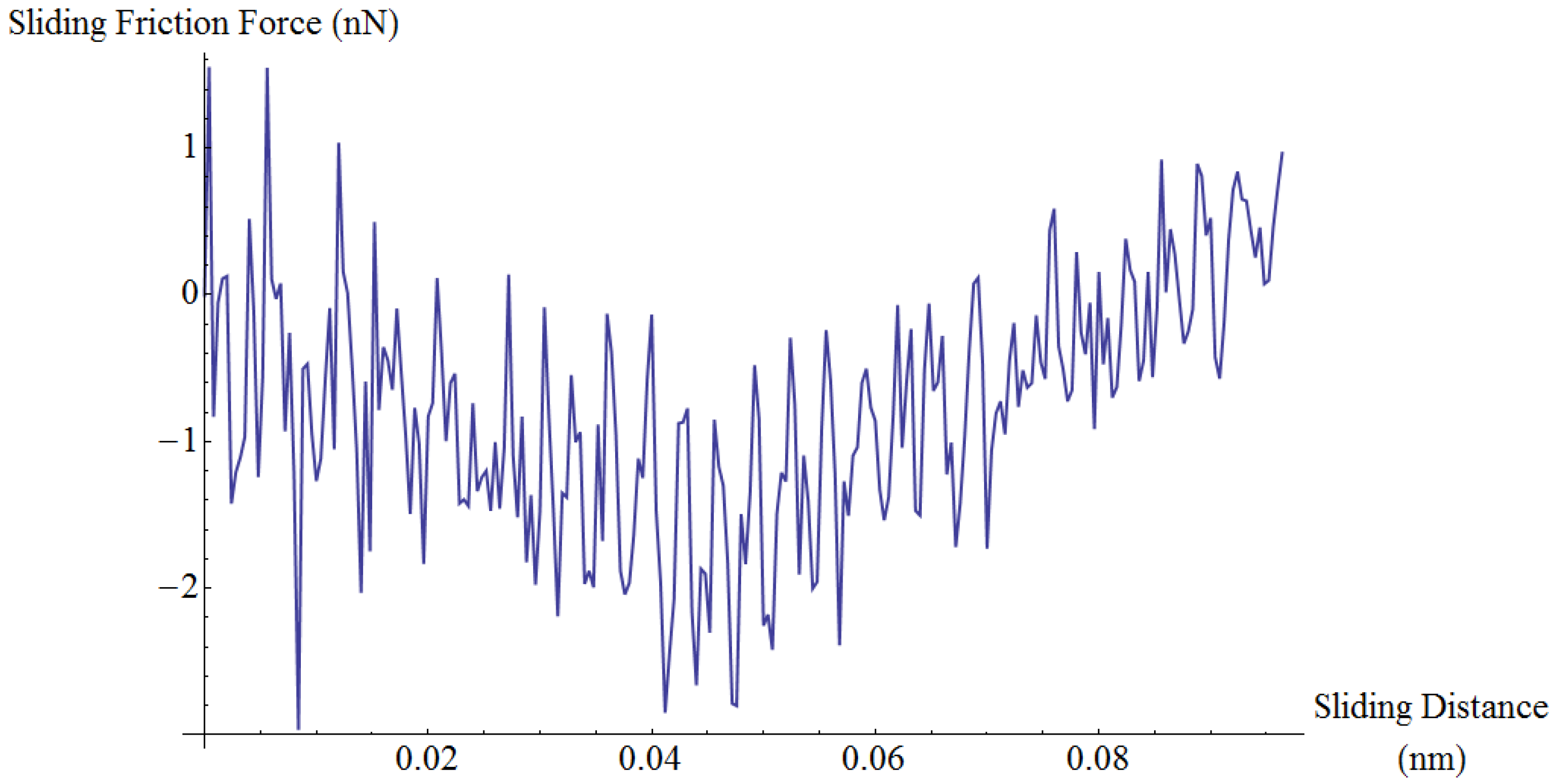
2.2. Stick-Slip Statistics
2.3. Tip-Sample Entropy
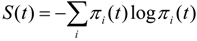
3. Results
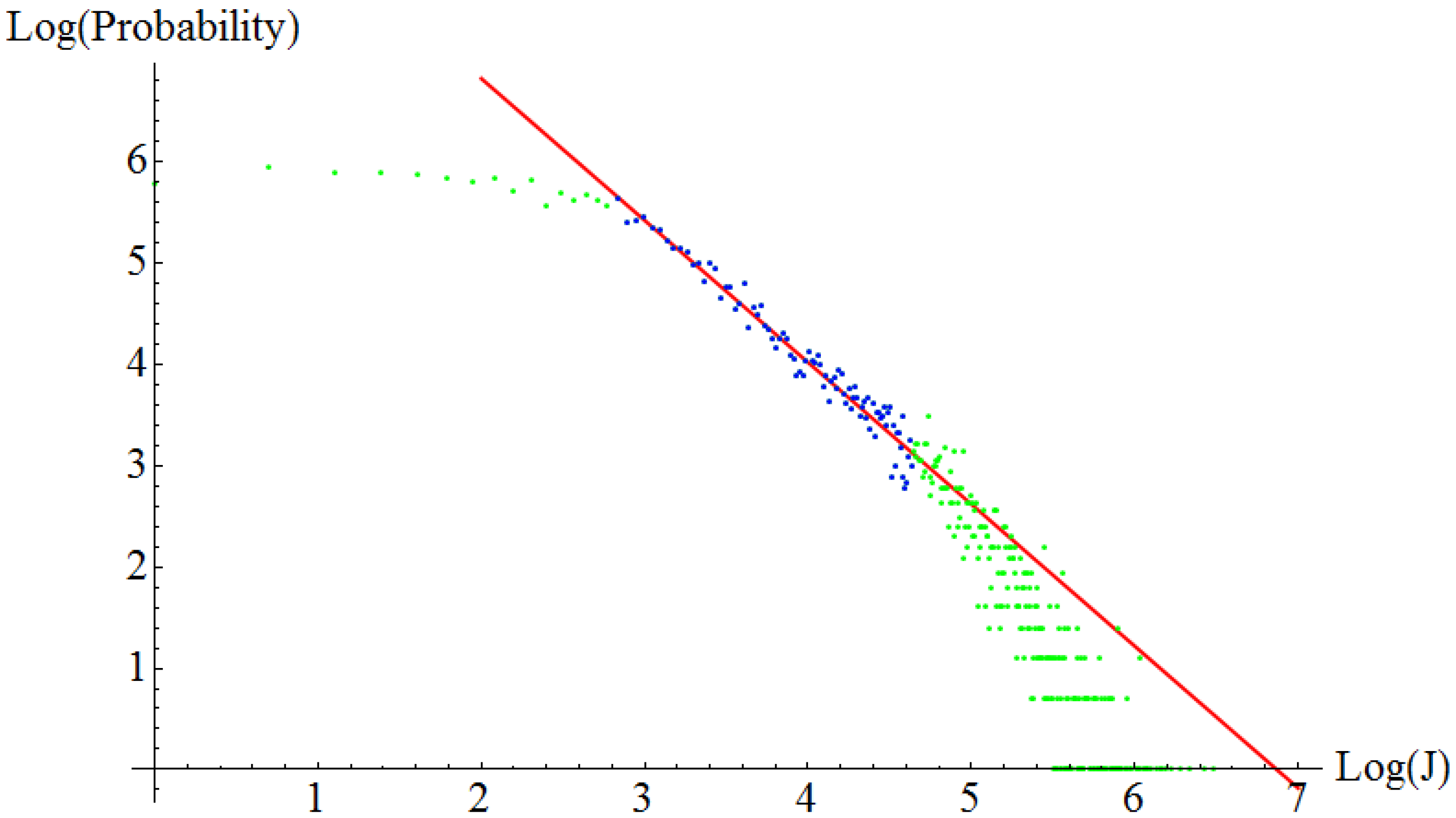
3.1. Probability of Jumps
| Load | Material | |
|---|---|---|
| Aluminum | Copper | |
| 1.0 nN | 1.40 | 0.64 |
| 1.5 nN | 0.99 | 1.16 |
| 2.0 nN | 1.00 | 0.74 |
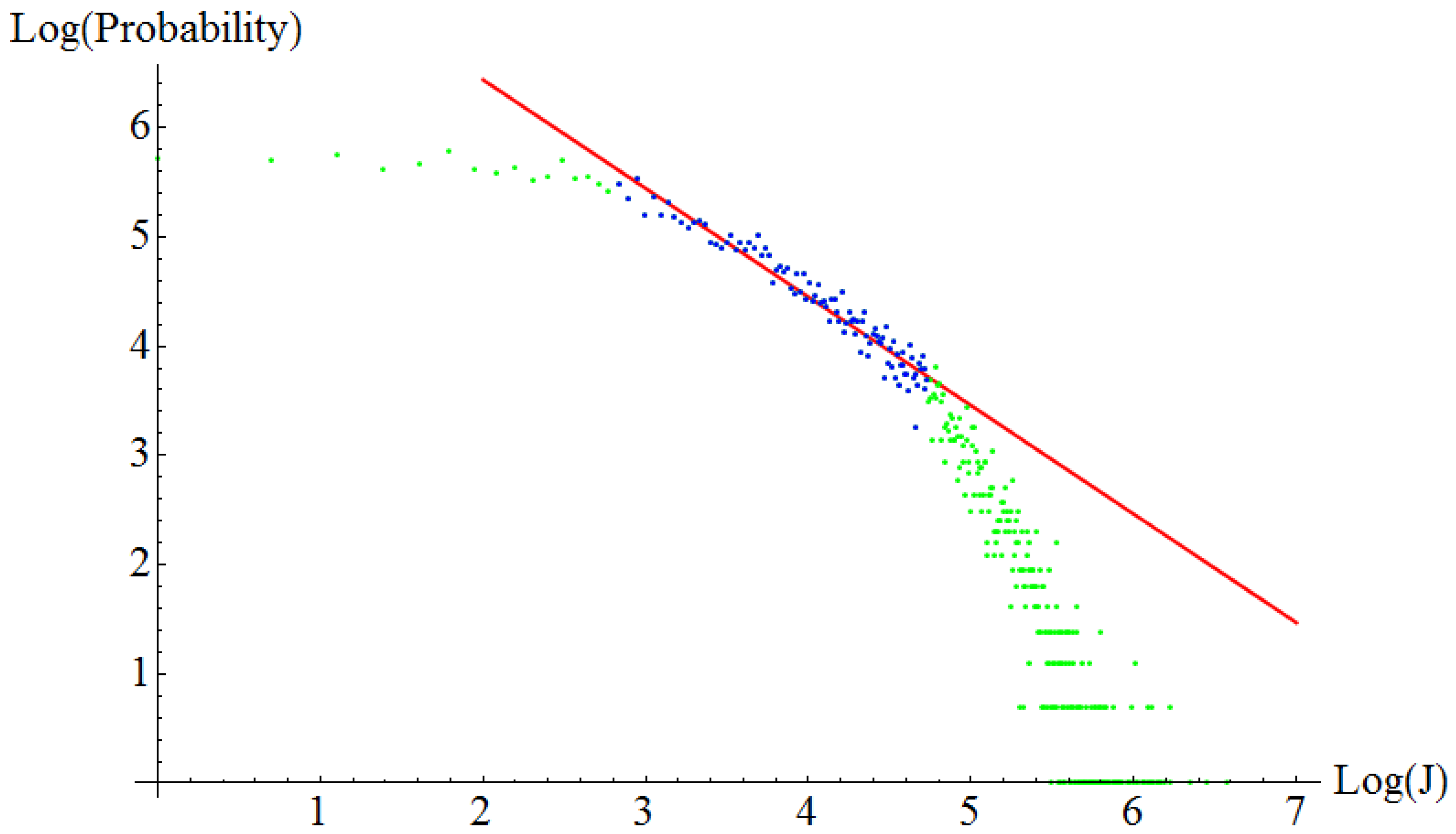
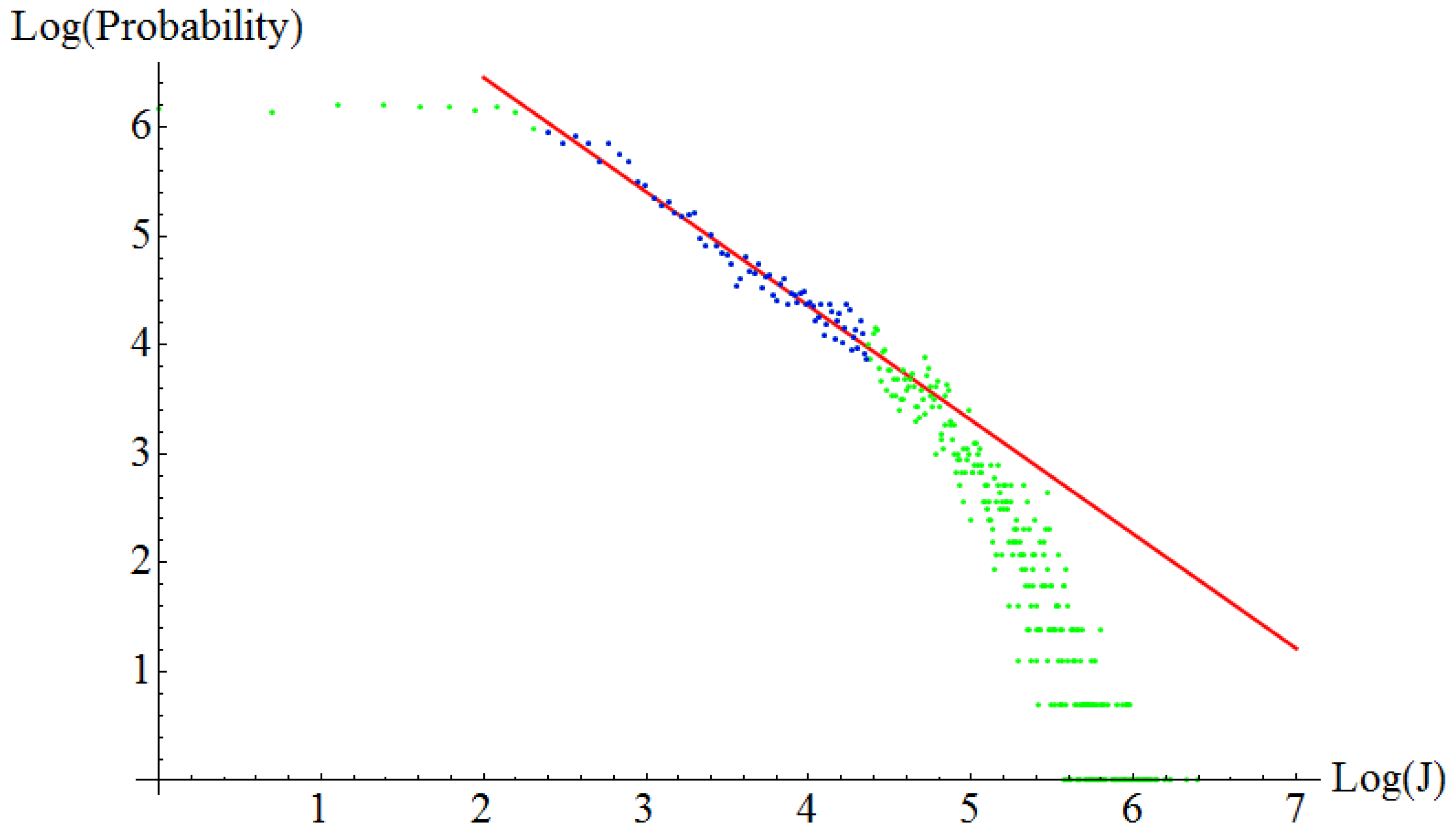
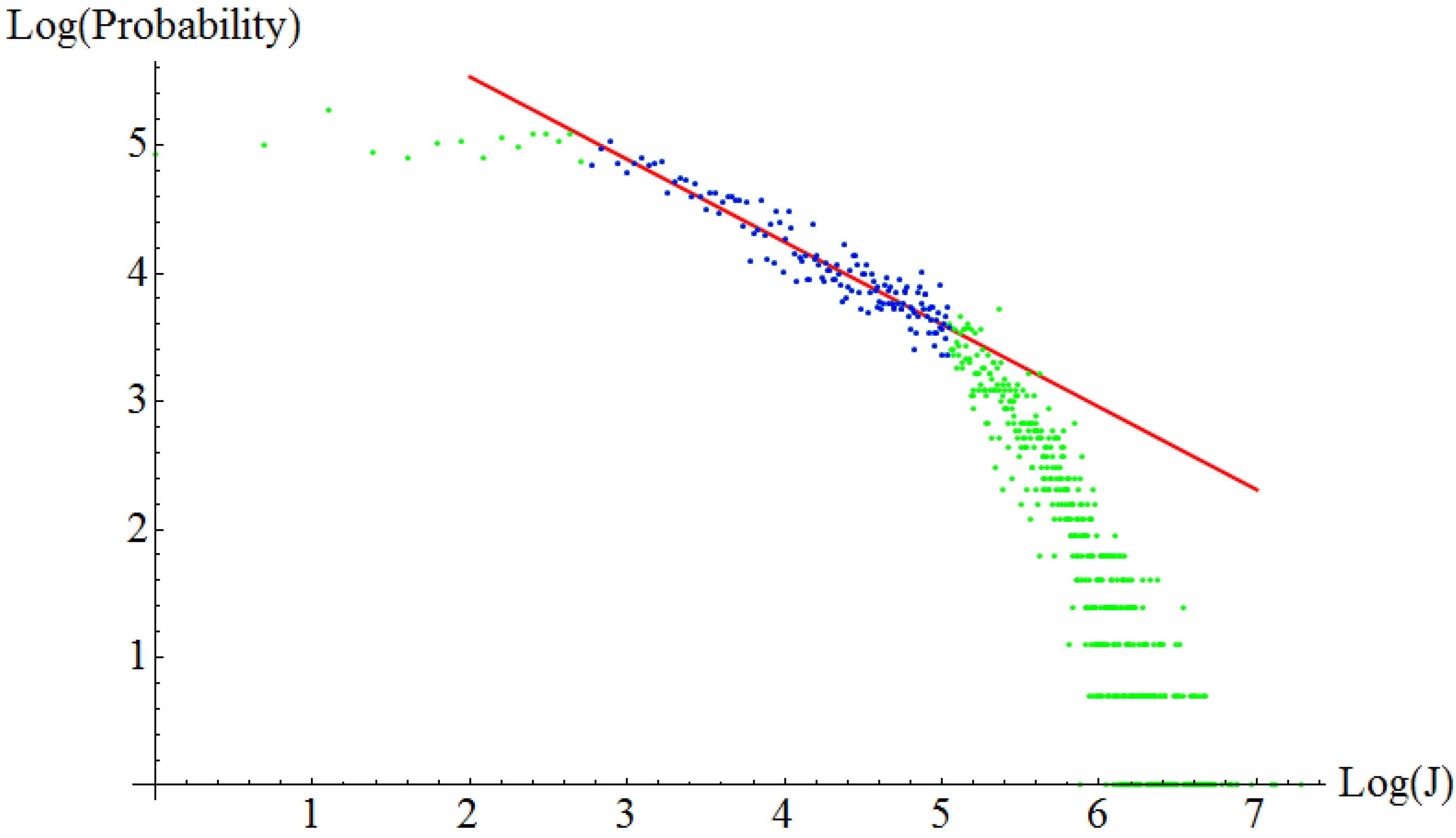
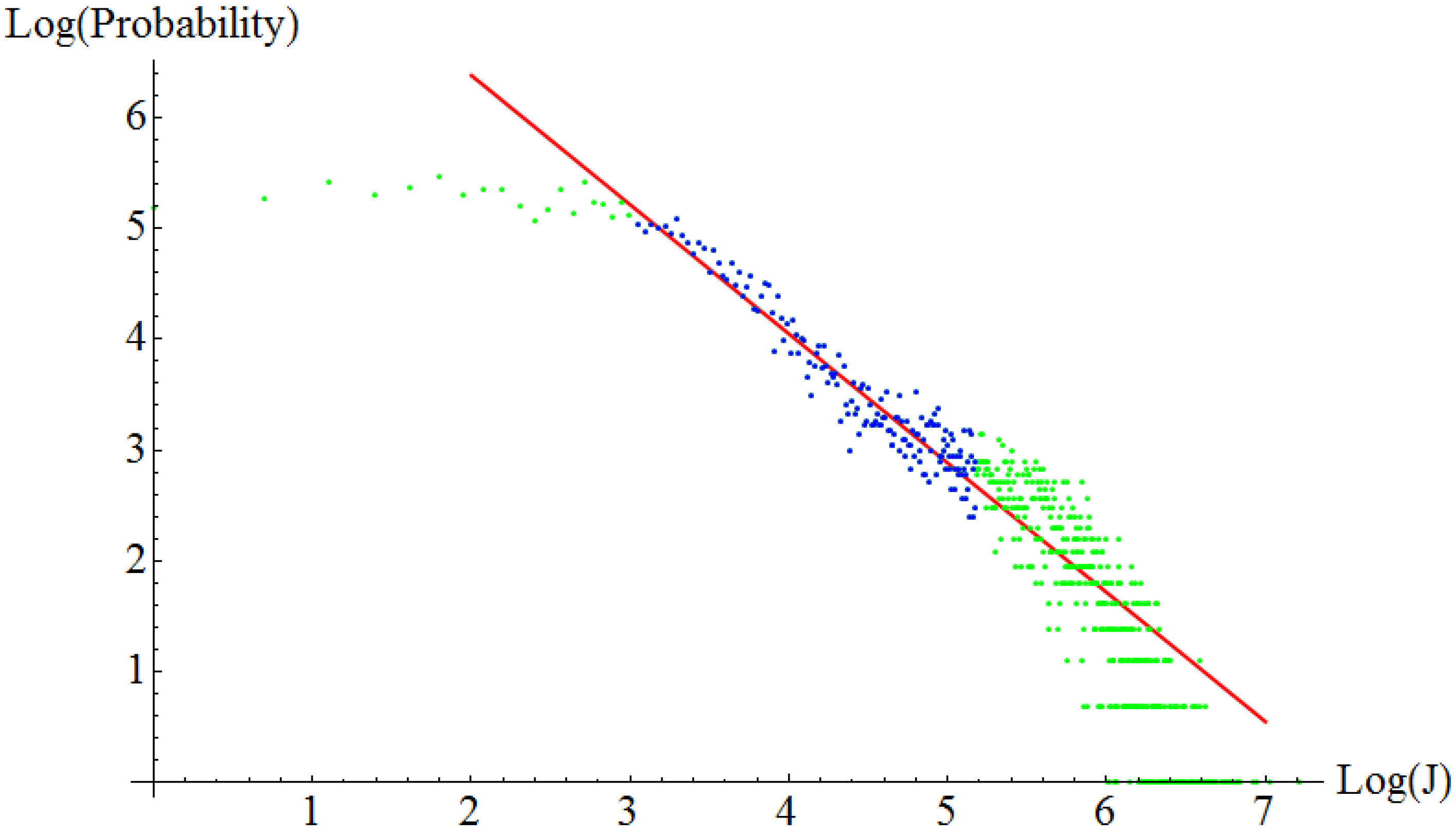
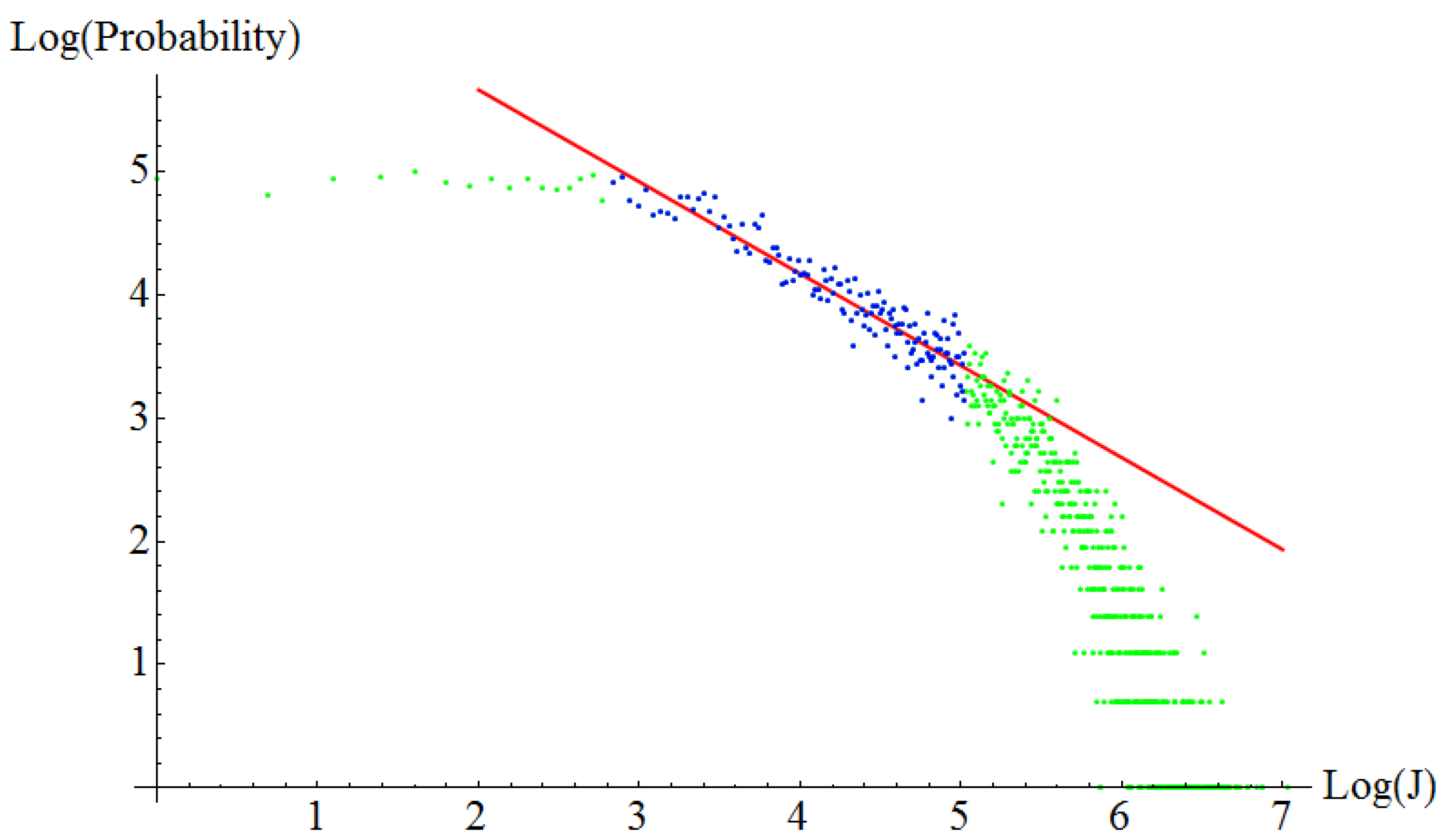
3.2. Entropy of Jittering
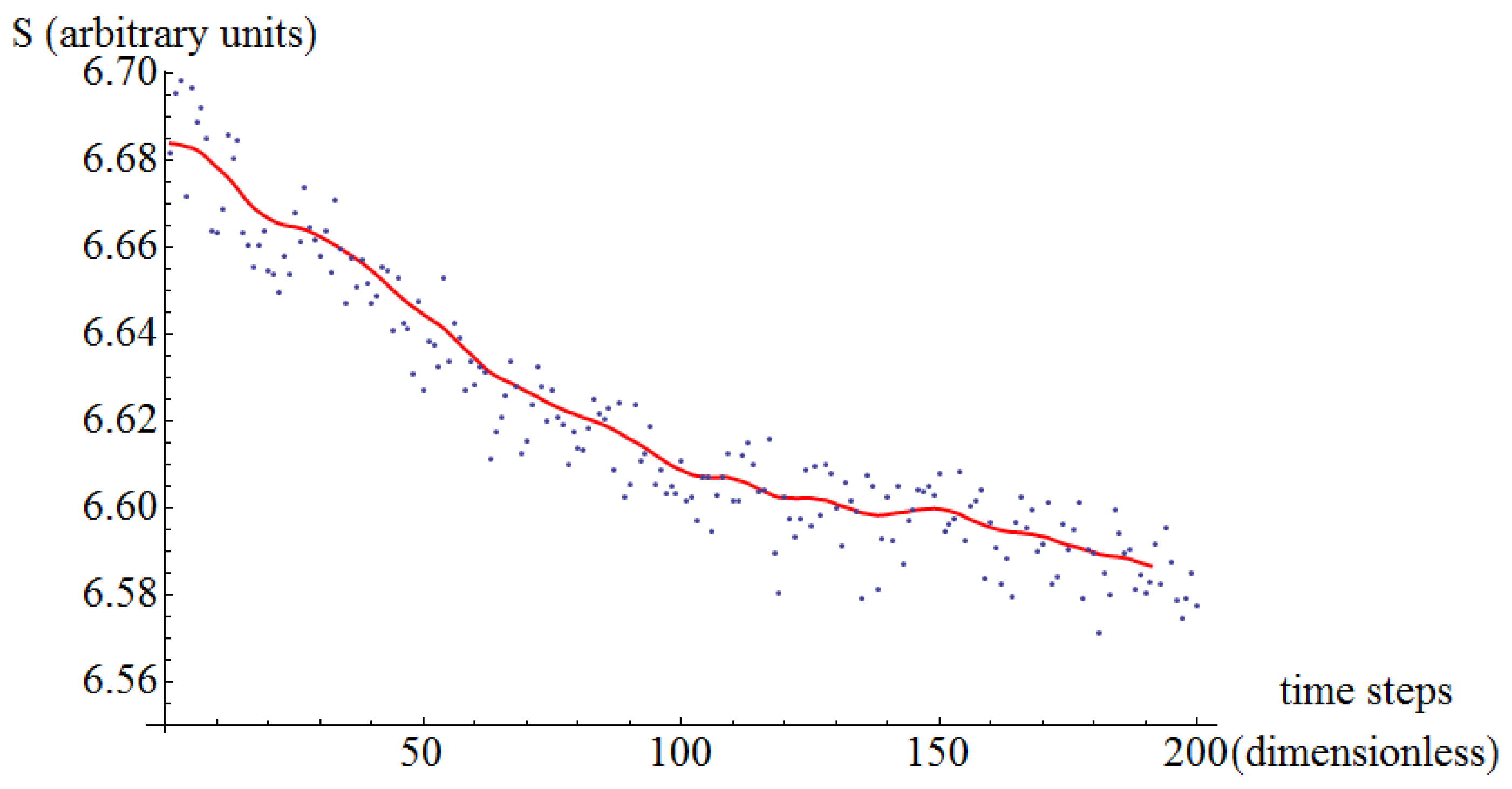
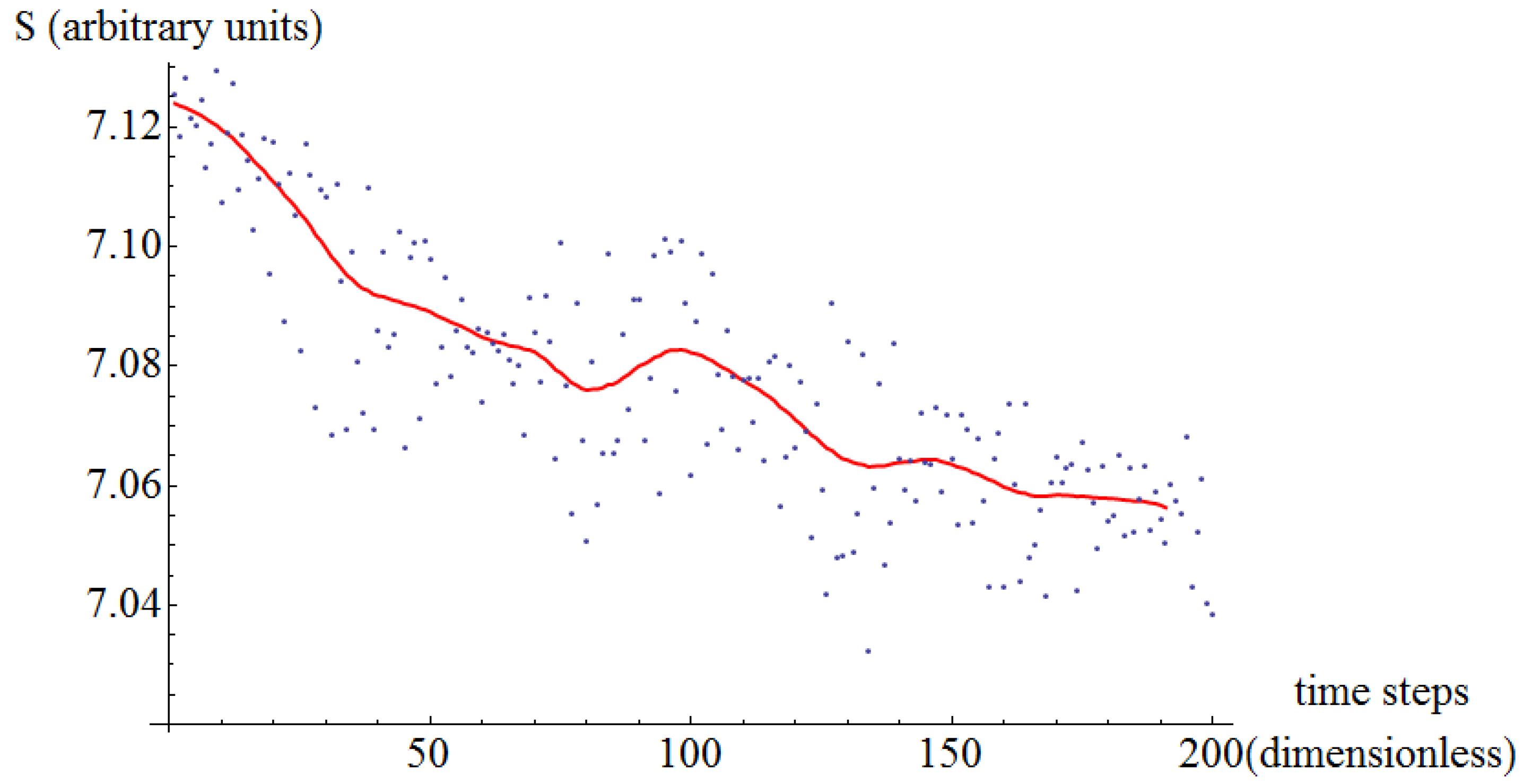
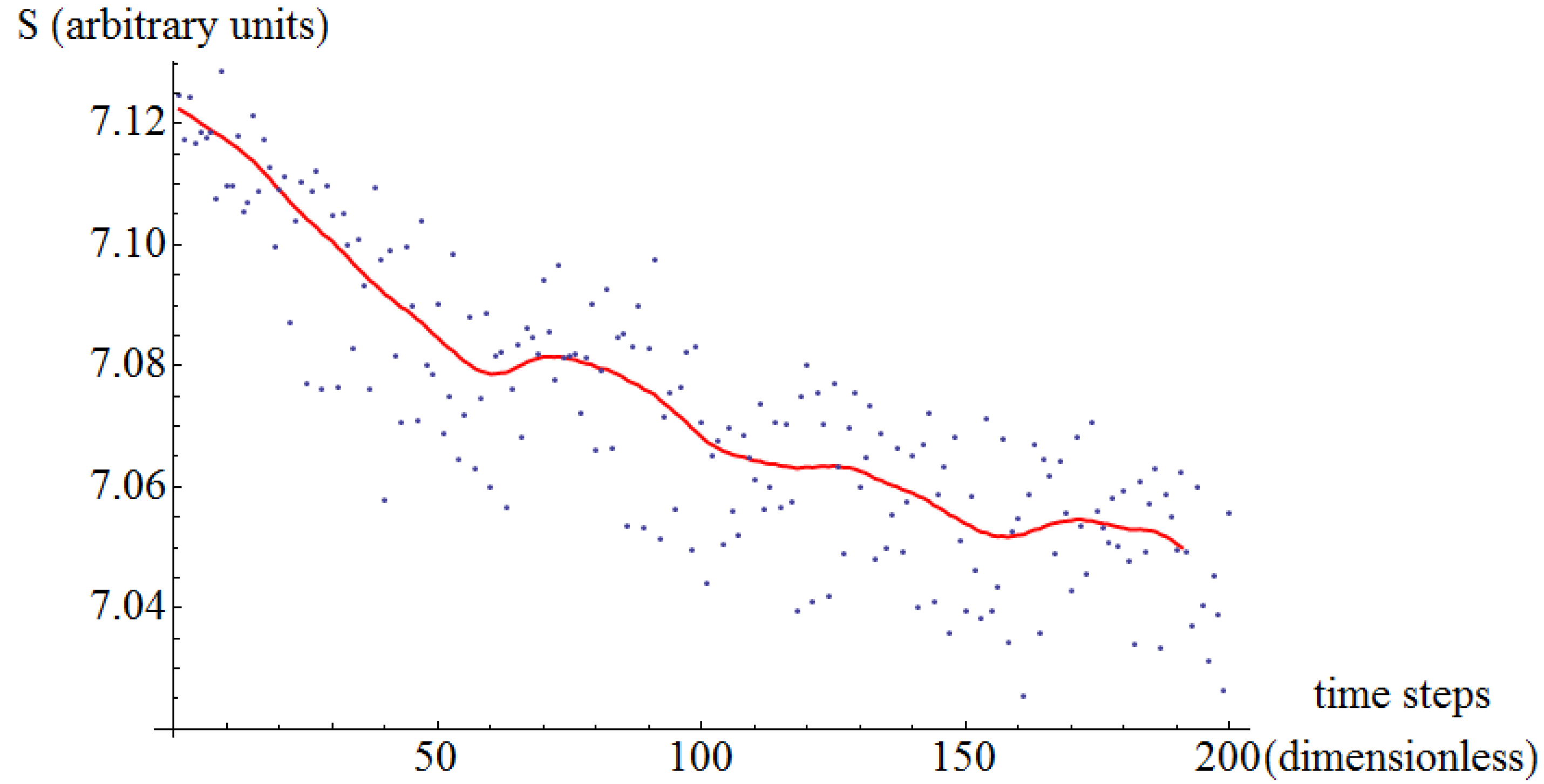
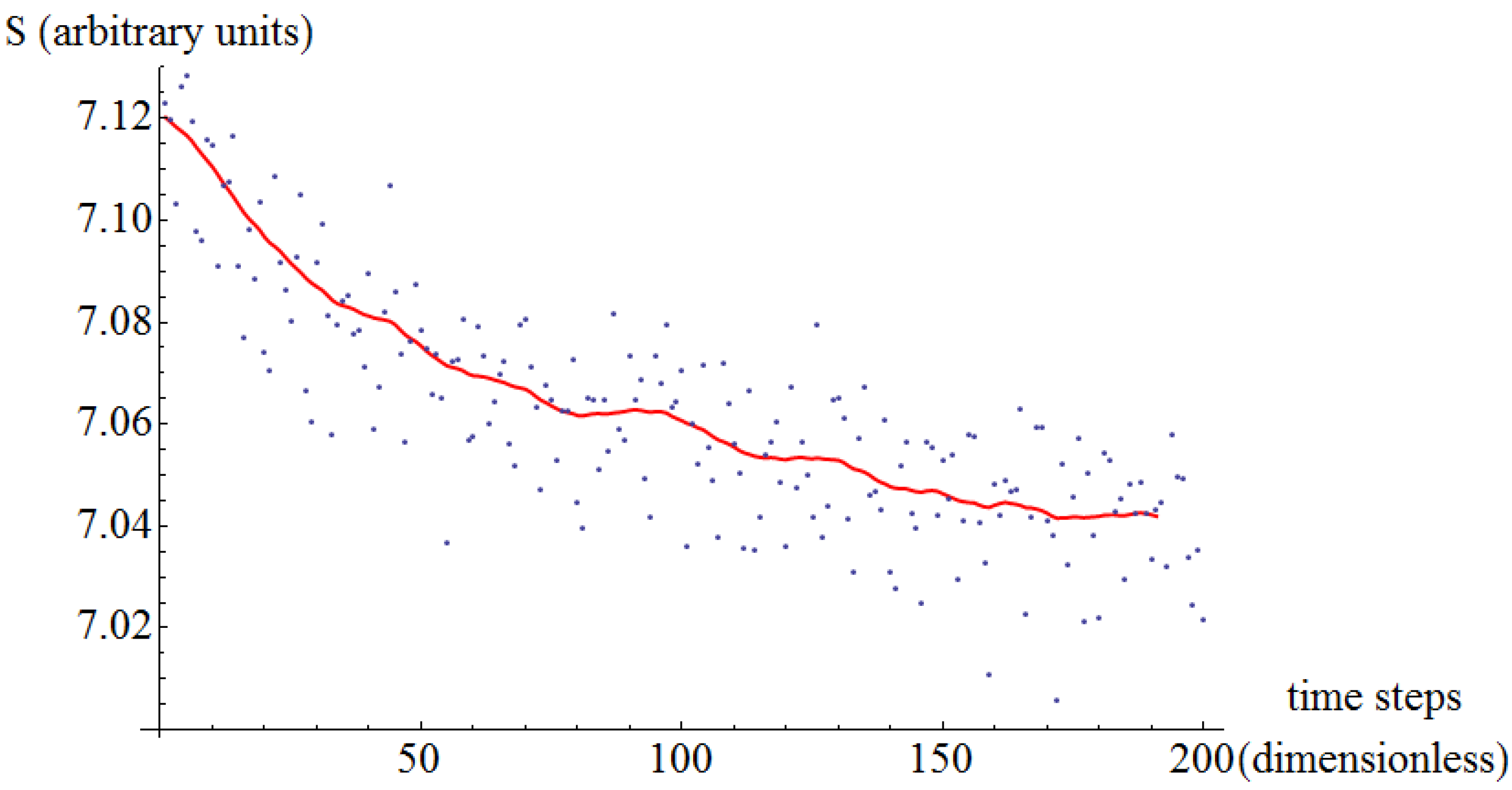
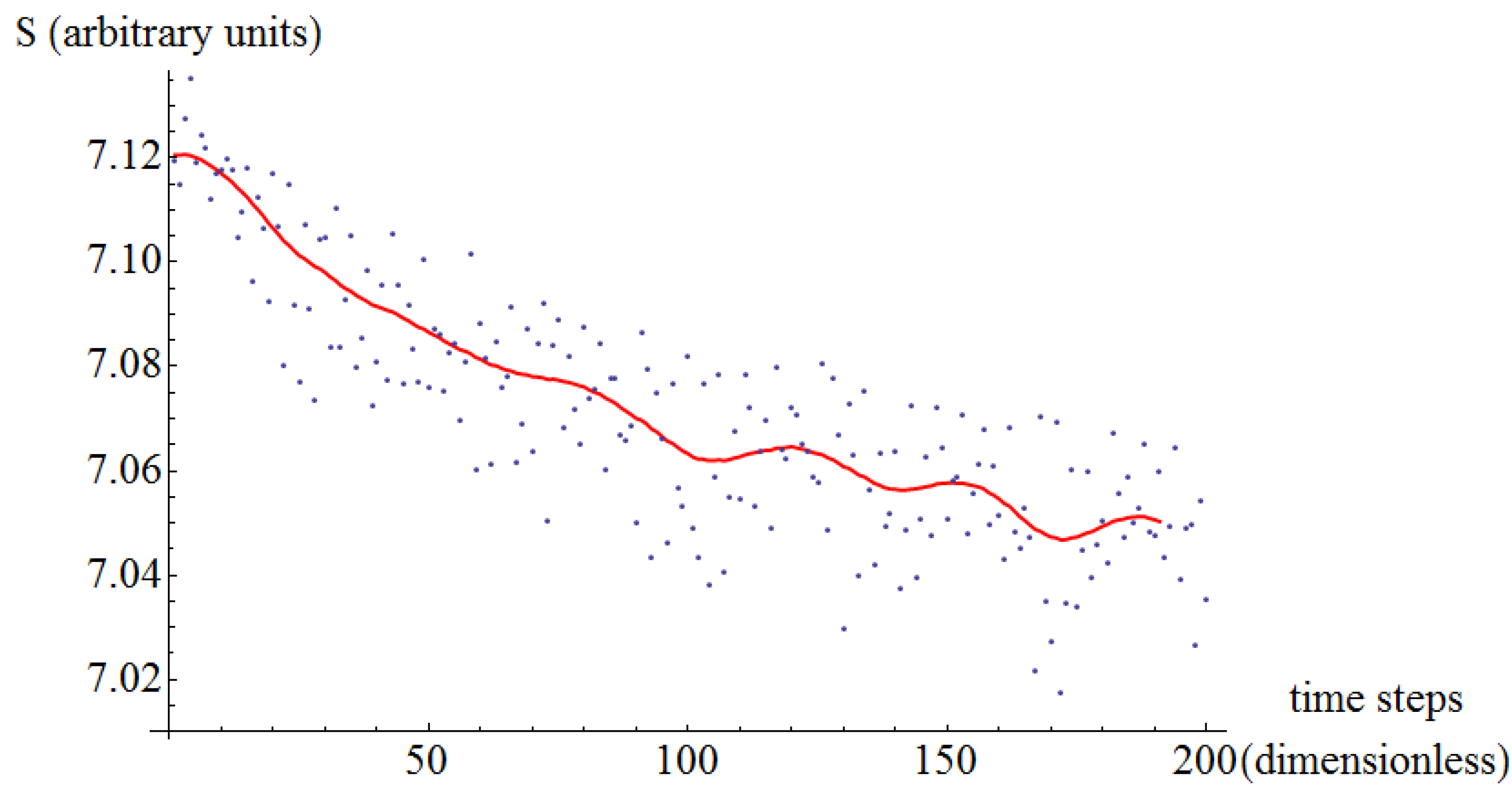
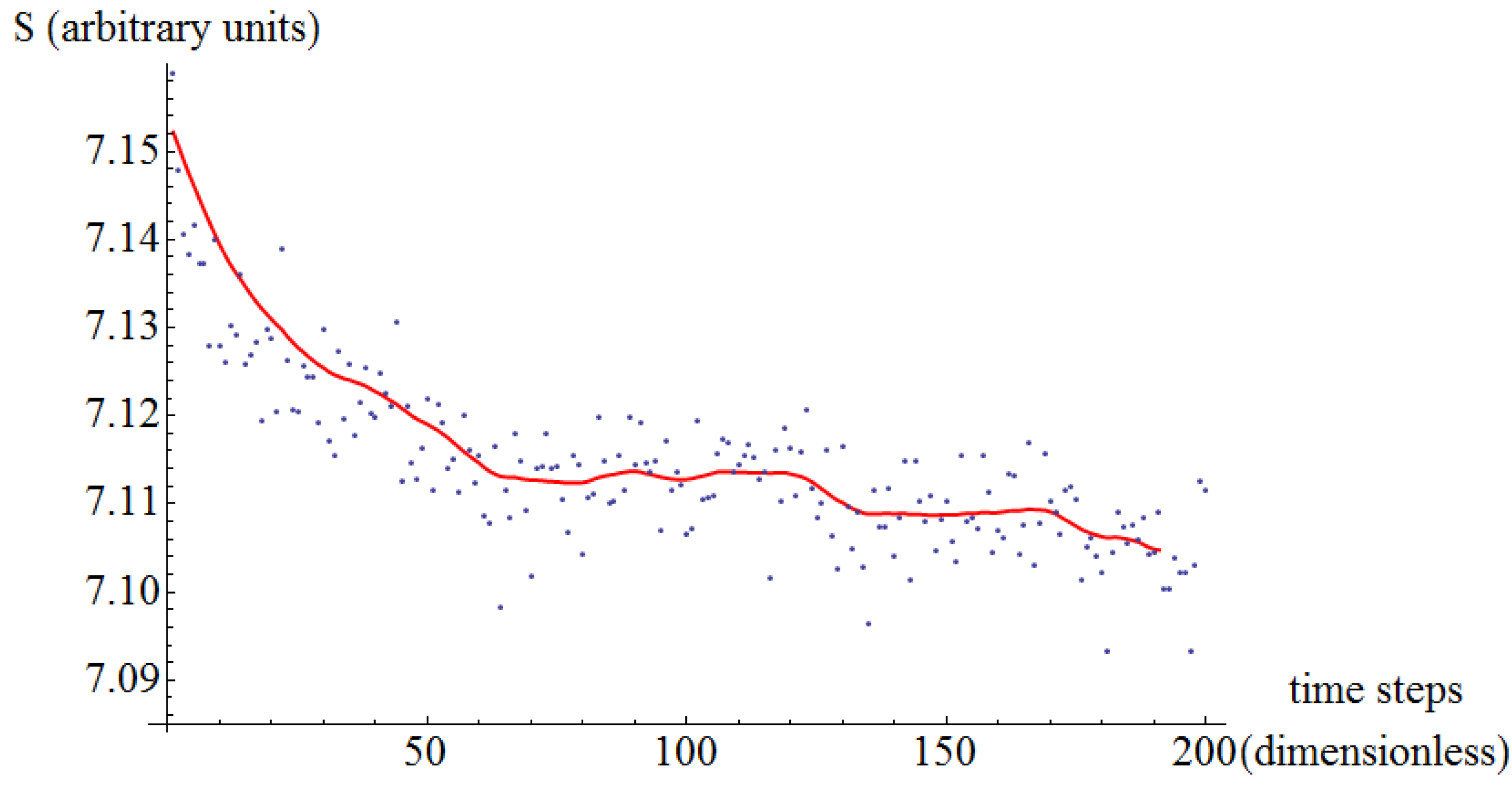
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Baumberger, T. Dry Friction Dynamics at Low Velocities. In Physics of Sliding Friction; NATO ASI Series; Springer: Dordrecht, The Netherlands, 1996; Volume 311, pp. 1–26. [Google Scholar]
- Bowden, F.P.; Tabor, D. The Friction and Lubrication of Solids; Oxford University: New York, NY, USA, 1950. [Google Scholar]
- Nosonovsky, M.; Mortazavi, V. Friction-Induced Vibrations and Self-Organization; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar]
- Trabesinger, A. Complexity. Nat. Phys. 2012, 8. [Google Scholar] [CrossRef]
- Carpick, R.W.; Salmeron, M. Scratching the Surface: Fundamental Investigations of Tribology with Atomic Force Microscopy. Chem. Rev. 1997, 97, 1163–1194. [Google Scholar] [CrossRef]
- Baykara, M.Z.; Schwarz, U.D. Noncontact atomic force microscopy II. Beilstein J. Nanotechnol. 2014, 5, 289–290. [Google Scholar] [CrossRef]
- Miller, B.; Krim, J. Quartz Crystal Microbalance (QCM) Applications to Tribology. In Encyclopedia of Tribology; Springer: New York, NY, USA, 2013; pp. 2727–2733. [Google Scholar]
- Israelachvili, J. Intermolecular and Surface Forces, 2nd ed.; Academic Press: New York, NY, USA, 2011. [Google Scholar]
- Gottlieb, S.; Sterling, T. Exascale Computing. Comput. Sci. Eng. 2013, 15, 12–15. [Google Scholar]
- Plimpton, S.J.; Thompson, A.P. Computational Aspects of Many-body Potentials. MRS Bull. 2012, 37, 513–521. [Google Scholar] [CrossRef]
- Becker, C.A.; Tavazza, F.; Trautt, Z.T.; Buarque de Macedo, R.A. Considerations for choosing and using force fields and interatomic potentials in materials science and engineering. Curr. Opin. Solid State Mater. Sci. 2013, 17, 277–283. [Google Scholar] [CrossRef]
- Carlson, J.N.; Langer, J.S. Properties of earthquakes generated by fault dynamics. Phys. Rev. Lett. 1989, 62, 2632–2635. [Google Scholar] [CrossRef]
- Carlson, J.M.; Langer, J.S.; Shaw, B.E. Dynamics of earthquake faults. Rev. Mod. Phys. 1994, 66, 657–670. [Google Scholar] [CrossRef]
- Burridge, R.; Knopoff, L. Model and theoretical seismicity. Bull. Seismol. Soc. Am. 1967, 57, 3411–3471. [Google Scholar]
- Carlson, J.N.; Langer, J.S.; Shaw, B.E.; Tang, C. Intrinsic properties of a Burridge-Knopoff model of an earthquake fault. Phys. Rev. A 1991, 44, 884–897. [Google Scholar] [CrossRef]
- Fox-Rabinovich, G.S.; Gershman, I.S.; Yamamoto, K.; Biksa, A.; Veldhuis, S.C.; Beake, B.D.; Kovalev, A.I. Self-Organization during Friction in Complex Surface Engineered Tribosystems. Entropy 2010, 12, 275–288. [Google Scholar] [CrossRef]
- Kagan, E. Turing Systems, Entropy, and Kinetic Models for Self-Healing Surfaces. Entropy 2010, 12, 554–569. [Google Scholar] [CrossRef]
- Nosonovsky, M. Entropy in Tribology: in the Search for Applications. Entropy 2010, 12, 1345–1390. [Google Scholar] [CrossRef]
- LAMMPS Molecular Dynamics Simulator. Available online: http://lammps.sandia.gov/ (accessed on 28 May 2014).
- Zypman, F.; Ferrante, J.; Jansen, M.; Scanlon, K.; Abel, P. Evidence of self-organized criticality in dry sliding friction. J. Phys. Cond. Matt. Lett. 2003, 15. [Google Scholar] [CrossRef]
- Adler, M.; Ferrante, J.; Schilowitz, A.; Yablon, D.; Zypman, F. Self-organized criticality in nanotribology. In MRS Proceedings; Cambridge University Press: Cambridge, UK, 2003; Volume 782. [Google Scholar]
- Buldyrev, J.; Ferrante, F.; Zypman, F. Dry friction avalanches: Experiment and theory. Phys. Rev. E 2006, 74, 066110. [Google Scholar] [CrossRef]
- Fleurquin, P.; Fort, H.; Kornbluth, M.; Sandler, R.; Segall, M.; Zypman, F. Negentropy generation and fractality in dry friction of polished surfaces. Entropy 2010, 12, 480–489. [Google Scholar]
- Schneider, T.; Stoll, E. Molecular-dynamics study of a three-dimensional one-component model for distortive phase transitions. Phys. Rev. B 1978, 17, 1302–1323. [Google Scholar] [CrossRef]
- Martens, C.C. Qualitative dynamics of generalized Langevin equations and the theory of chemical reaction rates. J. Chem. Phys. 2002, 116, 2516–2528. [Google Scholar]
- Wang, J. Quantum Thermal Transport from Classical Molecular Dynamics. Phys. Rev. Lett. 2007, 99, 160601. [Google Scholar] [CrossRef]
- Kantorovich, L. Generalized Langevin equation for solids I. Rigorous derivation and main properties. Phys. Rev. B 2008, 78, 094304. [Google Scholar] [CrossRef]
- Martyna, G.J.; Tobias, D.J.; Klein, M.L. Constant pressure molecular dynamics algorithms. J. Chem. Phys. 1994, 101, 4177–4189. [Google Scholar]
- Feller, S.E.; Zhang, Y.; Pastor, R.W.; Brooks, B.R. Constant pressure molecular dynamics simulation: The Langevin piston method. J. Chem. Phys. 1995, 103, 4613–4621. [Google Scholar] [CrossRef]
- Daw, M.S.; Baskes, M. Embedded-atom method: Derivation and application to impurities, surfaces, and other defects in metals. Phys. Rev. B 1984, 29, 6443–6453. [Google Scholar] [CrossRef]
- Plimpton, S.J. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Clauset, A.; Shalizi, C.R.; Newman, M.E.J. Data Analysis, Statistics and Probability. SIAM Rev. 2009, 51, 661–703. [Google Scholar] [CrossRef]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Creeger, P.; Zypman, F. Entropy Content During Nanometric Stick-Slip Motion. Entropy 2014, 16, 3062-3073. https://doi.org/10.3390/e16063062
Creeger P, Zypman F. Entropy Content During Nanometric Stick-Slip Motion. Entropy. 2014; 16(6):3062-3073. https://doi.org/10.3390/e16063062
Chicago/Turabian StyleCreeger, Paul, and Fredy Zypman. 2014. "Entropy Content During Nanometric Stick-Slip Motion" Entropy 16, no. 6: 3062-3073. https://doi.org/10.3390/e16063062
APA StyleCreeger, P., & Zypman, F. (2014). Entropy Content During Nanometric Stick-Slip Motion. Entropy, 16(6), 3062-3073. https://doi.org/10.3390/e16063062




