Global Stability Analysis of a Curzon–Ahlborn Heat Engine under Different Regimes of Performance
Abstract
:1. Introduction
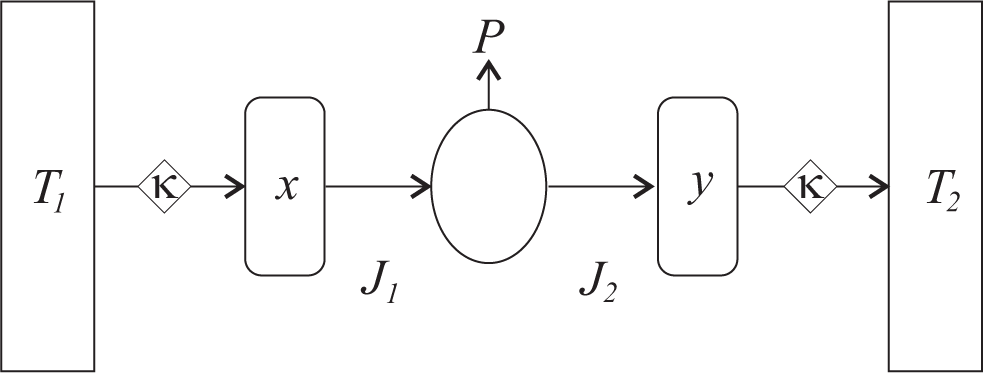
2. Steady-State Characteristics of a Curzon–Ahlborn Engine Model for Different Regimes of Performance
3. Global Stability Analysis
3.1. Dynamical CA-Engine Model
3.2. Lyapunov Functions of the CA-Engine Model under Both Maximum Efficient Power and Maximum Ecological Function
- V (x, y) must be positive definite in a region around the steady state,
- must be negative definite, that is, .
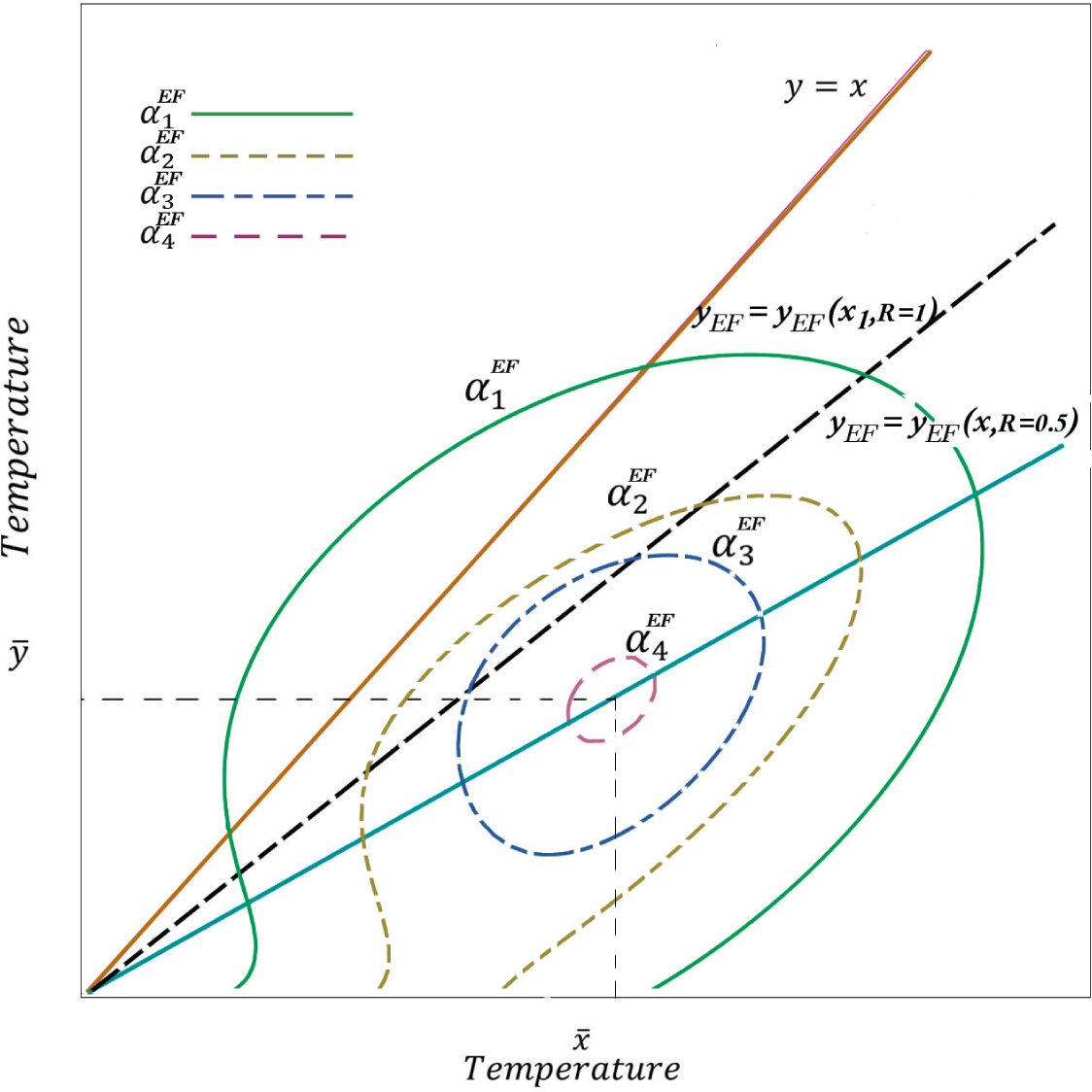
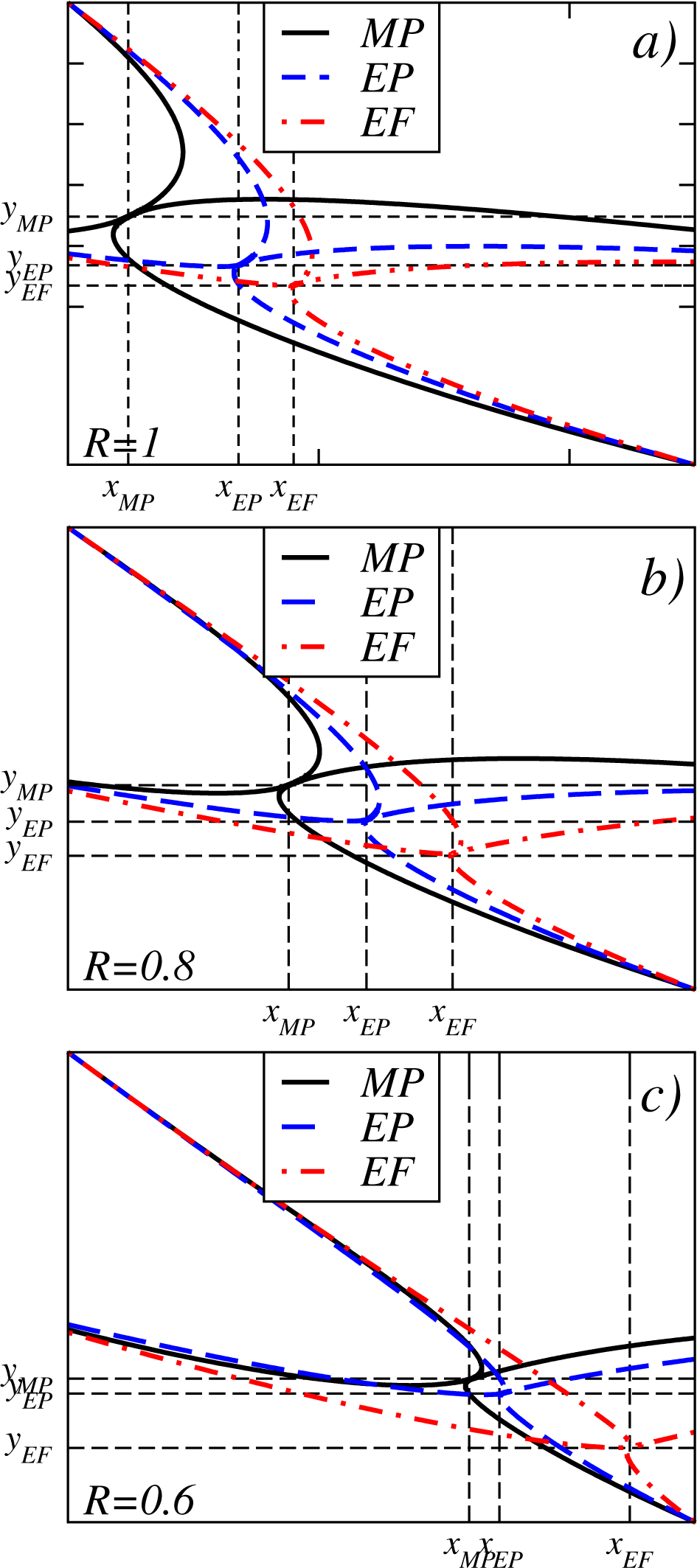
3.3. Maximum Efficient Power Regime
3.4. Maximum Ecological Function Regime
4. Discussion
5. Concluding Remarks
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Curzon, F.; Ahlborn, B. Efficiency of a Carnot engine at maximum power output. Am. J. Phys. 1975, 43, 22–24. [Google Scholar]
- Sieniutycs, S.; Salamon, P. Finite Time Thermodynamics and Themoeconomics; Taylor and Francis: London, UK, 1990. [Google Scholar]
- De Vos, A. Endorreversible Thermodynamics of Solar Energy Conversion; Oxford University Press: Oxford, UK, 1992. [Google Scholar]
- Wu, C.; Chen, L.; Chen, J. Recent Advances in Finite-Time Thermodynamics; Nova Science: New York, NY, USA, 1999. [Google Scholar]
- Chen, L.; Sun, F. Advances in Finite Time Thermodynamics: Analysis and Optimization; Nova Science: New York, NY, USA, 2004. [Google Scholar]
- Andresen, B. Current trends in finite-time thermodynamics. Angew. Chem. Int. Ed. 2011, 50, 2690–2704. [Google Scholar]
- De Vos, A. Efficiency of some heat engines at maximum-power conditions. Am. J. Phys. 1985, 53, 570–573. [Google Scholar]
- Yilmaz, T. A new performance criterion for heat engine: efficient power. J. Energy Inst. 2006, 79, 38–41. [Google Scholar]
- Arias-Hernández, L.A.; Barranco-Jiménez, M.A.; Angulo-Brown, F. Comparative analysis of two ecological types modes of performance for a simple energy converters. J. Energy Inst. 2009, 82, 223–227. [Google Scholar]
- Angulo-Brown, F. An ecological optimization criterion for finite-time heat engines. J. Appl. Phys. 1991, 69. [Google Scholar] [CrossRef]
- Velasco, S.; Roco, J.M.M.; Medina, A.; White, J.A.; Calvo-Hernández, A. Optimization of heat engines including the saving of natural resources and the reduction of thermal pollution. J. Phys. D 2000, 33, 355–359. [Google Scholar]
- Gordon, J.M.; Huleihil, M. On Optimizing maximum-power heat engines. J. Appl. Phys. 1991, 69. [Google Scholar] [CrossRef]
- Hoffmann, K.H.; Burzler, J.M.; Schubert, S. Endoreversible Thermodynamics. J. Non-Equilib. Thermodyn. 1997, 22, 311–355. [Google Scholar]
- Barranco-Jiménez, M.A.; Angulo-Brown, F. Thermo-economical optimization of an endoreversible power plant model. Rev. Mex. Fis. 2005, 51, 49–56. [Google Scholar]
- Arias-Hernández, L.A.; Ares de Parga, G.A.; Angulo-Brown, F. On Some Nonendoreversible engine models with Nonlinear heat transfer laws. Open Sys. Inf. Dyn. 2003, 10, 351–375. [Google Scholar]
- Santillán, M.; Maya-Aranda, G.; Angulo-Brown, F. Local stability analysis of an endoreversible Curzon–Ahlborn-Novikov engine working in a maximum-power like regime. J. Phys. D 2001, 34, 2068–2072. [Google Scholar]
- Guzmán-Vargas, L.; Reyes-Ramírez, I.; Sánchez-Salas, N. The effect of heat transfer laws and thermal conductances on the local stability of an endoreversible heat engine. J. Phys. D 2005, 38, 1282–1291. [Google Scholar]
- Páez-Hernández, R.T.; Angulo-Brown, F.; Santillán, M. Dynamic robustness and thermodynamic optimization in a non-endoreversible Curzon–Ahlborn engine. J. Non-Equilib. Thermodyn. 2006, 31, 173–188. [Google Scholar]
- Barranco-Jiménez, M.A.; Páez-Hernández, R.T.; Reyes-Ramírez, I.; Guzmán-Vargas, L. Local stability analysis of a thermo-economic model of a Chambadal-Novikov-Curzon–Ahlborn heat engine. Entropy 2011, 13, 1584–1594. [Google Scholar]
- Reyes-Ramírez, I.; Barranco-Jiménez, M.A.; Rojas-Pacheco, A.; Guzmán-Vargas, L. Global stability analysis of a Curzon–Ahlborn heat engine using the Lyapunov method. Physica A 2014, 399, 98–105. [Google Scholar]
- Chen, J. The maximum power output and maximum efficiency of an irreversible Carnot heat engine. J. Phys. D 1994, 27, 1144–1149. [Google Scholar]
- Ozcaynak, S.; Goktan, S.; Yavuz, H. Finite-time thermodynamics analysis of a radiative heat engine with internal irreversibility. J. Phys. D 1994, 27, 1139–1143. [Google Scholar]
- Barranco-Jiménez, M.A.; Sánchez-Salas, N.; Angulo-Brown, F. Finite-time themoeconomic optimization of a solar-driven heat engine model. Entropy 2011, 13, 171–183. [Google Scholar]
- Krasovskii, N.N. Stability of Motion; Stanford University Press: Stanford, CA, USA, 1963. [Google Scholar]
- Shampine, L.F.; Thompson, S. Solving DDEs in matlab. Appl. Numer. Math. 2001, 37, 441–458. [Google Scholar]
- Bogacki, P.; Shampine, L.F. A 3(2) pair of RungeâǍŞKutta, formulas. Appl. Math. Lett. 1989, 2, 321–325. [Google Scholar]




© 2014 by the authors; licensee MDPI, Basel, Switzerland This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Reyes-Ramírez, I.; Barranco-Jiménez, M.A.; Rojas-Pacheco, A.; Guzmán-Vargas, L. Global Stability Analysis of a Curzon–Ahlborn Heat Engine under Different Regimes of Performance. Entropy 2014, 16, 5796-5809. https://doi.org/10.3390/e16115796
Reyes-Ramírez I, Barranco-Jiménez MA, Rojas-Pacheco A, Guzmán-Vargas L. Global Stability Analysis of a Curzon–Ahlborn Heat Engine under Different Regimes of Performance. Entropy. 2014; 16(11):5796-5809. https://doi.org/10.3390/e16115796
Chicago/Turabian StyleReyes-Ramírez, Israel, Marco A. Barranco-Jiménez, Adolfo Rojas-Pacheco, and Lev Guzmán-Vargas. 2014. "Global Stability Analysis of a Curzon–Ahlborn Heat Engine under Different Regimes of Performance" Entropy 16, no. 11: 5796-5809. https://doi.org/10.3390/e16115796
APA StyleReyes-Ramírez, I., Barranco-Jiménez, M. A., Rojas-Pacheco, A., & Guzmán-Vargas, L. (2014). Global Stability Analysis of a Curzon–Ahlborn Heat Engine under Different Regimes of Performance. Entropy, 16(11), 5796-5809. https://doi.org/10.3390/e16115796



