Holographic Dark Information Energy: Predicted Dark Energy Measurement
Abstract
:1. Introduction
2. The HDIE Model
2.1. Stellar Heated Gas and Dust
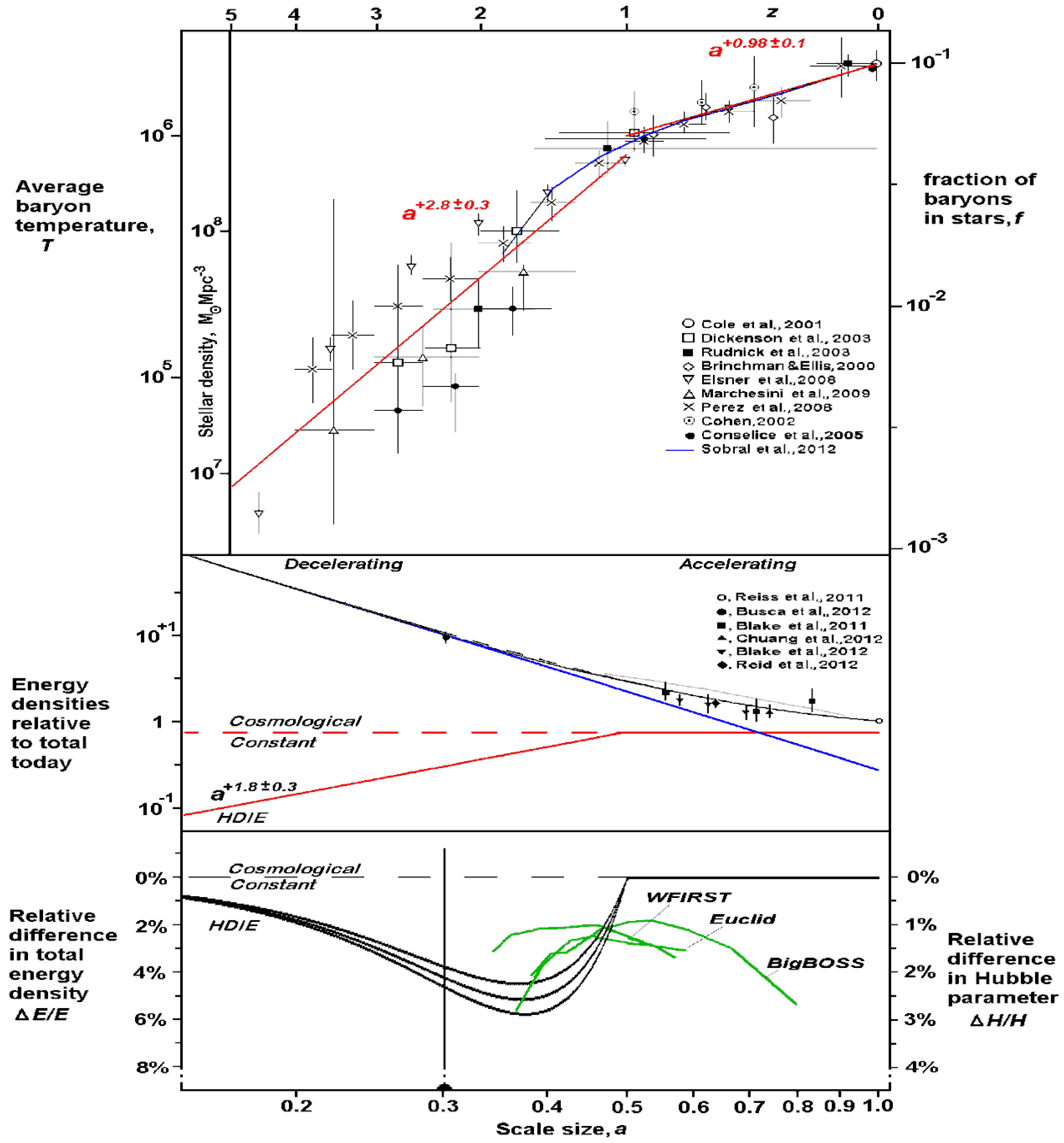
2.2. Dependence on the Holographic Principle
2.3. Dark Energy Predictions for z > 1
2.4. Measurement Capabilities of Next Generation Instruments
2.5. Characteristic Energy
3. Gedanken Experiment
3.1. Hypothetical Computer Simulation
3.2. Algorithmic Information Content
4. Implications for the cosmos
5. Summary
References
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Gilliland, R.L.; Hogan, C.J.; Jha, S.; Kirshner, R.P.; et al. Observational evidence from supernovae for an accelerating universe and a cosmological constant. Astron. J. 1998, 116, 1009–1038. [Google Scholar] [CrossRef]
- Perlmutter, S.; Aldering, G.; Goldhaber, G.; Knop, R.A.; Nugent, P.; Castro, P.G.; Deustua, S.; Fabbro, S.; Goobar, A.; Groom, D.E.; et al. Measurements of Ω and Λ from 42 high-redshift supernovae. Astrophys. J. 1999, 517, 565–586. [Google Scholar] [CrossRef]
- Riess, A.G.; Strolger, L.-G.; Castertano, S.; Ferguson, H.C.; Mobasher, B.; Gold, B.; Challis, P.J.; Filippenko, A.V.; Jha, S.; Li, W.; et al. New Hubble space telescope discoveries of type 1a supernovae at z ≥ 1; narrowing constraints on the early behaviour of dark energy. Astrophys. J. 2007, 659, 98–121. [Google Scholar] [CrossRef]
- Bacon, D.J.; Refregier, A.R.; Ellis, R.S. Detection of weak gravitational lensing by large-scale structure. Mon. Not. R. Astron. Soc. 2000, 318, 625–640. [Google Scholar] [CrossRef]
- Beutler, F.; Blake, C.; Colless, M.; Jones, D.H.; Staveley-Smith, L.; Campbell, L.; Parker, Q.; Saunders, W.; Watson, F. The 6dF galaxy survey: Baryon acoustic oscillations and the local Hubble constant. Mon. Not. R. Astron. Soc. 2011, 416, 3017–3032. [Google Scholar] [CrossRef]
- Sherwin, B.D; Dunkley, J.; Das, S.; Appel, J.W.; Bond, J.R.; Carvalho, C.S.; Delvin, M.J.; Dunner, R.; Essinger-Hileman, T.; et al. Evidence for dark energy from the cosmic microwave background alone using the Atacama Cosmology Telescope lensing measurements. Phys. Rev. Lett. 2011, 107, 021302. [Google Scholar] [CrossRef] [PubMed]
- Blake, C.; Brough, S.; Colless, M.; Contreras, C.; Couch, W.; Croom, S.; Davis, T.; Drinkwater, M.J.; Forster, K.; Gilbank, D.; et al. The WiggleZ dark energy survey: The growth rate of cosmic structure since z = 0.9. Mon. Not. R. Astron. Soc. 2011, 415, 2876–2891. [Google Scholar] [CrossRef]
- Carroll, S.M. Why is the universe accelerating? In Carnegie Observatories Astrophysics Series, Vol. 2, Measuring and Modeling the Universe; Freedmann, W.L., Ed.; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Frieman, J.A.; Turner, M.S.; Huterer, D. Dark energy and the accelerating universe. Ann. Rev. Astron. Astrophys. 2008, 46, 385–432. [Google Scholar] [CrossRef]
- Thompson, R.I.; Martins, C.J.A.P.; Vielveuf, P.E. Constraining cosmologies with fundamental constants I. quintessence and K-essence. Mon. Not. R. Astron. Soc. 2013, 428, 2232–2240. [Google Scholar] [CrossRef]
- Tegmark, M.; Eisenstein, D.J.; Strauss, M.A.; Weinberg, D.H.; Blanton, M.R.; Frieman, J.A.; Fukugita, M.; Gunn, J.E.; Hamilton, A.J.S.; Knapp, G.R.; et al. Cosmological constraints from the SDSS luminous red galaxies. Phys. Rev. D 2006, 74, 123507. [Google Scholar] [CrossRef]
- Weinberg, S. The cosmological constant problem. Rev. Mod. Phys. 1989, 61, 1–23. [Google Scholar] [CrossRef]
- Li, M. A model of holographic dark energy. Phys. Lett. B 2004, 603, 1–5. [Google Scholar] [CrossRef]
- Gong, Y.; Wang, B.; Zhang, Y.-Z. Holographic dark energy reexamined. Phys. Rev. D 2005, 72, 043510. [Google Scholar] [CrossRef]
- Fischler, W.; Susskind, L. Holography and cosmology. 1998. [Google Scholar]
- Hooft, G. Obstacles on the way towards the quantization of space, time and matter- and possible solutions. Stud. Hist. Phil. Mod. Phys. 2001, 32, 157–180. [Google Scholar] [CrossRef]
- Gong, Y.; Zhang, Y.-Z. Holography and holographic dark energy model. Class. Quantum Grav. 2005, 22, 4895–4901. [Google Scholar] [CrossRef]
- Lee, J.W.; Lee, J.; Kim, H.-C. Dark energy from vacuum entanglement. J. Cosmol. Astropart. P. 2007, 08, 005. [Google Scholar] [CrossRef]
- Landauer, R. Irreversibility and heat generation in the computing process. IBM J. Res. Dev. 1961, 3, 183–191. [Google Scholar] [CrossRef]
- Gough, M.P. Holographic Dark Information Energy. Entropy 2011, 13, 924–935. [Google Scholar] [CrossRef]
- Piechocinska, B. Information erasure. Phys. Rev. A 2000, 61, 062314. [Google Scholar] [CrossRef]
- Landauer, R. Dissipation and noise immunity in computation and communication. Nature 1988, 335, 779–784. [Google Scholar] [CrossRef]
- Maxwell’s Demon 2: Entropy. In Classical and Quantum Information; Leff, H.S.; Rex, A.F. (Eds.) Computing IOP Publishing Ltd: London, UK, 2003.
- Hilbert, M.; López, P. The world’s technological capacity to store, communicate, and compute information. Science 2011, 332, 60–65. [Google Scholar] [CrossRef] [PubMed]
- Toyabe, S.; Sagawa, T.; Ueda, M.; Muneyuki, E.; Sano, M. Experimental demonstration of information-to-energy conversion and validation of the generalized Jarzynski equality. Nat. Phys. 2010, 6, 988–992. [Google Scholar] [CrossRef]
- Berut, A.; Arakelyan, A.; Petrosyan, A.; Ciliberto, S.; Dillenschneider, R.; Lutz, E. Experimental verification of Landauer’s principle linking information and thermodynamics. Nature 2012, 483, 187–189. [Google Scholar] [CrossRef] [PubMed]
- Maruyama, K.; Nori, F.; Vlatko, V. The physics of Maxwell’s demon and information. Rev. Mod. Phys. 2009, 81, 1–23. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Black holes and entropy. Phys. Rev. D 1973, 7, 2333–2346. [Google Scholar] [CrossRef]
- Susskind, L. The world as a hologram. J. Math. Phys. 1995, 36, 6377–6396. [Google Scholar] [CrossRef]
- Frampton, P.H.; Hsu, S.D.H.; Kephart, T.W.; Reeb, D. What is the entropy of the universe? Classical Quant. Grav. 2009, 26, 145005. [Google Scholar] [CrossRef]
- Egan, C.A.; Lineweaver, C.H. A larger estimate of the entropy of the universe. Astrophys. J. 2010, 710, 1825–1834. [Google Scholar] [CrossRef]
- Buosso, R.; Harnik, R.; Kribs, G.D.; Perez, G. Predicting the cosmological constant from the causal entropic principle. 2007. [Google Scholar]
- Ruffini, R.; Wheeler, J.A. Introducing the black hole. Phys. Today 1971, 24, 30–41. [Google Scholar] [CrossRef]
- Cole, S.; Norberg, P.; Baugh, C.M.; Frenk, C.S.; Bland-Hawthorn, J.; Bridges, T.; Cannon, R.; Colless, M.; Collins, C.; Couch, W.; et al. The 2dF galaxy redshift survey. Mon. Not. R. Astron. Soc. 2001, 326, 255–273. [Google Scholar] [CrossRef]
- Dickinson, M.; Papovich, C.; Ferguson, H.C.; Budavari, T. The evolution of the global stellar mass density at 0 < z < 3. Astrophys. J. 2003, 587, 25–40. [Google Scholar]
- Rudnick, G.; Rix, H-W.; Franx, M.; Labbe, I.; Blanton, M.; Daddi, E.; Schreiber, N.M.F.; Moorwood, A.; Rottgering, H.; Trujillo, I.; et al. The rest-frame optical uminosity density, color, and stellar mass density of the universe from z = 0 to z = 3. Astrophys. J. 2003, 599, 847–864. [Google Scholar] [CrossRef]
- Brinchmann, J.; Ellis, R.S. The mass assembly and star formation characterisatics of field galaxies of known morphology. Astrophys. J. 2000, 536, L77–L80. [Google Scholar] [CrossRef] [PubMed]
- Elsner, F.; Feulner, G.; Hopp, U. The impact of Spitzer infrared data on stellar mass estimates. Astron. Astrophys. 2008, 477, 503–512. [Google Scholar] [CrossRef]
- Marchesini, D.; Van Dokkum, P.G.; Schreiber, N.M. F.; Franx, M.; Labbe, I.; Wuyts, S. Evolution of the stellar mass function of galaxies from z = 4.0. Astrophys. J. 2009, 701, 1765–1796. [Google Scholar] [CrossRef]
- Perez-Gonzalez, P.G.; Rieke, G.H.; Villar, V.; Barro, G.; Blaylock, M.; Egami, E.; Gallego, J.; Gil de Paz, A.; Pascual, S.; Zamorano, J.; et al. The stellar mass assembly of galaxies from z = 0 to z = 4.0. Astrophys. J. 2008, 675, 234–261. [Google Scholar] [CrossRef]
- Cohen, J.G. Caltech faint galaxy redshift survey. Astrophys. J. 2002, 567, 672–701. [Google Scholar] [CrossRef]
- Conselice, C.J.; Blackburne, J.A.; Papovich, C. The luminosity, stellar mass, and number density evolution of field galaxies. Astrophys. J. 2005, 620, 564–583. [Google Scholar] [CrossRef]
- Sobral, D.; Smail, I.; Best, P.N.; Geach, J.E.; Matsuda, Y.; Stott, J.P.; Cirasuolo, M.; Kurk, J. A large Hα survey at z=2.23, 1.47, 0.84 & 0.40: The 11 Gyr evolution of star-forming galaxies from HiZELS. 2012. [Google Scholar]
- Buosso, R. The holographic principle. Rev. Mod. Phys. 2002, 74, 825–874. [Google Scholar] [CrossRef]
- Maldacena, J. The large N limit of superconformal field theories and supergravity. 1998. [Google Scholar]
- Verlinde, E. On the origin of gravity and the laws of Newton. 2010. [Google Scholar]
- Cho, A. Sparks fly over shoestring test of the holographic principle. Science 2012, 336, 147–149. [Google Scholar] [CrossRef] [PubMed]
- Friedman, A. On the curvature of space. Gen. Relat. Gravit. 1999, 31, 1991–2000. [Google Scholar] [CrossRef]
- Busca, N.G.; Delubac, T.; Rich, J.; Bailey, S.; Font-Ribera, A.; Kirkby, D.; Le Goff, J.M.; Pieri, M.M.; Slosar, A.; Auborg, E.; et al. Baryon acoustic oscillations in the Lyα forest of Boss quasars. 2012. [Google Scholar]
- Reiss, A.G.; Macri, L.; Casertano, S.; Lampeitl, H.; Ferguson, H.C.; Filippenko, A.V.; Jha, S.W.; Li, W.; Chornock, R. A 3% solution: Determination of the Hubble constant with the Hubble Space Telescope and wide field camera. Astrophys. J. 2011, 730, 119. [Google Scholar] [CrossRef]
- Blake, C.; Glazebrook, K.; Davis, T.M.; Brough, S.; Colless, M.; Contreras, C.; Couch, W.; Croom, S.; Drinkwater, M.J.; Forster, K; et al. The WiggleZ dark energy survey: Measuring the cosmic expansion history using the Alcock-Paczynski test and distant supernovae. Mon. Not. R. Astron. Soc. 2011, 418, 1725–1735. [Google Scholar] [CrossRef]
- Chuang, C.-H.; Wang, Y. Measurements of H(z) and Da(z) from the two-dimensional two-point correlation function of Sloan Digital Sky Survey luminous red galaxies. Mon. Not. R. Astron. Soc. 2012, 426, 226–236. [Google Scholar] [CrossRef]
- Blake, C.; Brough, S.; Colless, M.; Contreras, C.; Couch, W.; Croom, S.; Croton, D.; Davis, T.M.; Drinkwater, M.J.; Forster, K.; et al. The WiggleZ dark energy survey: Joint measurements of the expansion and growth history at z <1. Mon. Not. R. Astron. Soc. 2012, 425, 405–414. [Google Scholar]
- Reid, B.A.; Samushia, L.; White, M.; Percival, W.J.; Manera, M.; Padmanabhan, N.; Ross, A.J.; Sanchez, A.G.; Bailey, S.; Bizyaev, D.; et al. The clustering of galaxies in the SDSS-III Baryon oscillation spectroscopic survey. 2012. [Google Scholar]
- Laureijs, R.; Amiaux, J.; Arduini, S.; Augueres, J.L.; Brinchmann, J.; Cole, R.; Cropper, M.; Dabin, C.; Duvet, L.; Ealet, A.; et al. Euclid definition study report. 2011. [Google Scholar]
- NASA WFIRST Final Report. Available online: http://www.wfirst.gsfc.nasa.gov/science/sdt_public/WFIRST_SDT_Final_Report.pdf (accessed on 26 November 2012).
- Schlegel, D.J.; Bebek, C.; Heetderks, H.; Ho, S.; Lampton, M.; Levi, M.; Mostek, N.; Padmanabhan, N.; Perlmutter, S.; Roe, N.; et al. The ground-based StageIV BAO Experiment. 2009. [Google Scholar]
- Ivezic, Z.; Tyson, J.A.; Acosta, E.; Allsman, R.; Anderson, S.F.; Andrew, J.; Axelrod, T.; Barr, J.D.; Becker, A.C.; et al. LSST: From science drivers to reference design and anticipated data products. 2011. [Google Scholar]
- Dark Energy Survey proposal. Available online: http://www.darkenergysurvey.org/reports/proposal-standalone.pdf (accessed on 15 January 2013).
- Popper, K. The Logic of Scientific Discovery; Routledge: London, UK, 1959. [Google Scholar]
- Gough, M.P.; Carozzi, T.; Buckley, A.M. On the similarity of information energy to dark energy. 2006. [Google Scholar]
- Gough, M.P. Information equation of state. Entropy 2008, 10, 150–159. [Google Scholar] [CrossRef]
- Peebles, P.J.E. Principles of Physical Cosmology; Princeton University Press: Princeton, NJ, USA, 1993. [Google Scholar]
- Zurek, W.H. Thermodynamic cost of computation, algorithmic complexity and the information metric. Nature 1989, 341, 119–124. [Google Scholar] [CrossRef]
- Devine, S. The insights of Algorithmic Entropy. Entropy 2009, 11, 85–110. [Google Scholar] [CrossRef]
- Penrose, R. The Road to Reality; Jonathan Cape: London, UK, 2004. [Google Scholar]
- Guzzo, L.; Pierleoni, M.; Meneux, B.; Branchini, E.; Le Fevre, O.; Marinoni, C.; Garilli, B.; Blaizot, J.; De Lucia, G.; Pollo, A.; et al. A test of the nature of cosmic acceleration using galaxy redshift distortions. Nature 2008, 451, 541–544. [Google Scholar] [CrossRef] [PubMed]
- Nagamine, K.; Loeb, A. Future evolution of the intergalactic medium in a universe dominated by a cosmological constant. New Astron. 2004, 9, 573–583. [Google Scholar] [CrossRef]
- Landauer, R. Information is physical. Phys. Today. 1991, 44, 23. [Google Scholar] [CrossRef]
- Wheeler, J.A. Information, physics, quantum: The search for links. Zurek, W., Ed.; In Complexity, Entropy, and the Physics of Information; Addison-Wesley: Redwood City, California, USA, 1990. [Google Scholar]
© 2013 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Gough, M.P. Holographic Dark Information Energy: Predicted Dark Energy Measurement. Entropy 2013, 15, 1135-1151. https://doi.org/10.3390/e15031135
Gough MP. Holographic Dark Information Energy: Predicted Dark Energy Measurement. Entropy. 2013; 15(3):1135-1151. https://doi.org/10.3390/e15031135
Chicago/Turabian StyleGough, Michael Paul. 2013. "Holographic Dark Information Energy: Predicted Dark Energy Measurement" Entropy 15, no. 3: 1135-1151. https://doi.org/10.3390/e15031135
APA StyleGough, M. P. (2013). Holographic Dark Information Energy: Predicted Dark Energy Measurement. Entropy, 15(3), 1135-1151. https://doi.org/10.3390/e15031135



