Information, Deformed қ-Wehrl Entropies and Semiclassical Delocalization
Abstract
:1. Introduction
- choosing an escort order q and
- “deforming" the Wehrl entropy (see Sect. 4) up to a degree, say, .
2. Preliminary materials
2.1. Escort distributions
2.2. Semi-classical Husimi distributions, Wehrl entropy, and Fisher information
3. Participation ratio
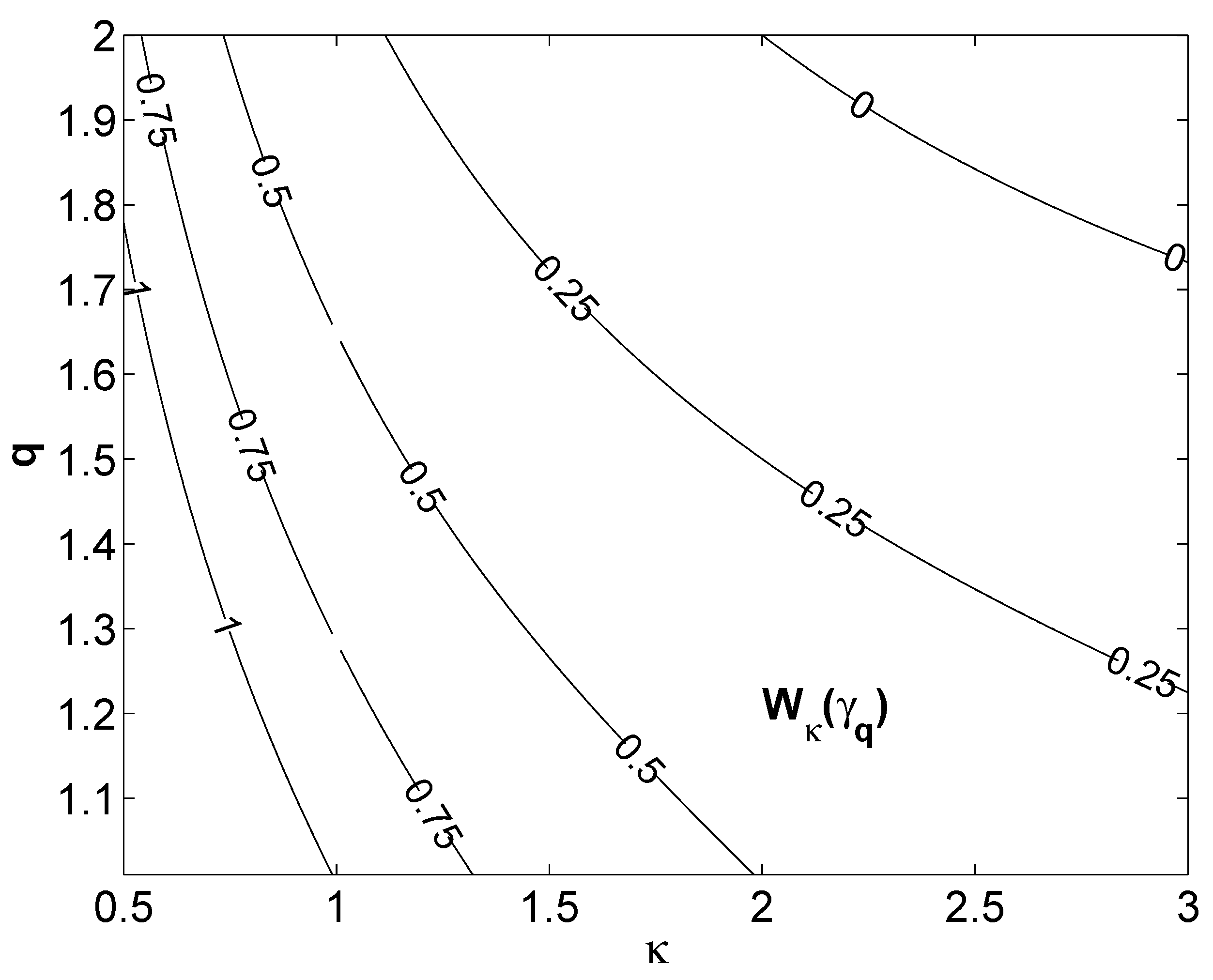
4. Deformed -Wehrl measures
5. Conclusions
- the escort order q and
- the degree of deformation, , of a Wehrl entropy.
- We have obtained the inequalitythat sets an upper bound to the possible escort degrees q of a Husimi distribution. For one has . As T grows, so does .
- For our deformed Wehrl functions we findequality holding for . Since can be greater than unity, we are definitely improving upon the celebrated Lieb’s bound .
Acknowledgements
References
- Wehrl, A. On the relation between classical and quantum–mechanical entropy. Rep. Math. Phys. 1979, 16, 353–358. [Google Scholar] [CrossRef]
- Lieb, E.H. Proof of an entropy conjecture of Wehrl. Commun. Math. Phys. 1978, 62, 35–41. [Google Scholar] [CrossRef]
- Beck, C.; Schlögl, F. Thermodynamics of chaotic systems; Cambridge University Press: Cambridge, England, 1993. [Google Scholar]
- Tsallis, C. Introduction to Nonextensive Statistical Mechanics: Approaching a Complex World; Springer–Verlag: New York, 2009. [Google Scholar]
- Pennini, F.; Plastino, A.; Ferri, G.L. Semiclassical information from deformed and escort information measures. Phys. A 2007, 383, 782–796. [Google Scholar] [CrossRef]
- Wigner, E.P. On the Quantum Correction For Thermodynamic Equilibrium. Phys. Rev. 1932, 40, 749–759. [Google Scholar] [CrossRef]
- Lee, H.W. Theory and application of the quantum phase space distribution functions. Phys. Rep. 1995, 259, 147–211. [Google Scholar] [CrossRef]
- Wlodarz, J.J. Entropy and Wigner distribution functions revisited. Int. J. Theor. Phys. 2003, 42, 1075–1084. [Google Scholar] [CrossRef]
- Husimi, K. Some formal properties of the density matrix. Proc. Phys. Math. Soc. Japan 1940, 22, 264. [Google Scholar]
- Note that the Husimi distribution function is not, strictly speakig, a probability density because the marginal distribution on each variable is not the squared modulus of a wave function.
- O’ Connel, R.F.; Wigner, E.P. Some properties of a non–negative quantum–mechanical distribution function. Phys. Lett. A 1981, 85, 121–126. [Google Scholar] [CrossRef]
- Mizrahi, S.S. Quantum mechanics in the Gaussian wave–packet phase space representation. Physica A 1984, 127, 241–264. [Google Scholar] [CrossRef]
- Anderson, A.; Halliwell, J.J. Information–theoretic measure of uncertainty due to quantum and thermal fluctuations. Phys. Rev. D 1993, 48, 2753–2765. [Google Scholar] [CrossRef]
- Zureck, W.H. Decoherence, einselection, and the quantum origins of the classical. Rev. Mod. Phys. 2003, 75, 715–775. [Google Scholar] [CrossRef]
- Korsch, H.J.; Leyes, W. Quantum and classical phase space evolution: a local measure of delocalization. New Journal of Physics 2002, 4, 62:1–62:15. [Google Scholar] [CrossRef]
- Baranger, M.; de Aguiar, M.A.M.; Keck, F.; Korsch, H.J.; Schellhaass, B. Semiclassical approximations in phase space with coherent states. J. Phys. A: Math. Gen. 2001, 34, 7227–7286. [Google Scholar] [CrossRef]
- Jacquod, Ph.; Whitney, R.S. Semiclassical Theory of Quantum Chaotic Transport: Phase–Space Splitting, Coherent Backscattering and Weak Localization. [arXiv:cond–mat/0512662].
- Schoendorff, J.L.; Korsch, H.J.; Moiseyev, N. Semiclassical quantization of a system with mixed regular/chaotic dynamics. Europhys. Lett. 1998, 44, 290–295. [Google Scholar] [CrossRef]
- Pennini, F.; Plastino, A.R.; Plastino, A. Renyi entropies and fisher informations as measures of nonextensivity in a Tsallis setting. Physica A 1998, 258, 446–457. [Google Scholar] [CrossRef]
- Di Sisto, R.P.; Martinez, S.; Orellana, R.B.; Plastino, A.R.; Plastino, A. General thermostatistical formalisms, invariance under uniform spectrum translations, and Tsallis Q–additivity. Physica A 1999, 265, 590. [Google Scholar] [CrossRef]
- Pennini, F.; Plastino, A. Escort Husimi distributions, Fisher information and nonextensivity. Phys. Lett. A 2004, 326, 20–26. [Google Scholar] [CrossRef]
- Gnuzmann, S.; Zyczkowski, K. Renyi–Wehrl entropies as measures of localization in phase space. J. Phys. A 2001, 34, 10123–10139. [Google Scholar] [CrossRef]
- Glauber, R.J. Coherent and Incoherent States of the Radiation Field. Phys. Rev. 1963, 131, 2766–2788. [Google Scholar] [CrossRef]
- Klauder, J.R.; Skagerstam, B.S. Coherent states; World Scientific: Singapore, 1985. [Google Scholar]
- Schnack, J. Thermodynamics of the harmonic oscillator using coherent states. Europhys. Lett. 1999, 45, 647–652. [Google Scholar] [CrossRef]
- (a) Jaynes, E.T. In Statistical Physics, Ford, W.K., Ed. Benjamin: New York, 1963. (b) Katz, A. Statistical Mechanics, Freeman: San Francisco, 1967.
- Pathria, R.K. Statistical Mechanics; Pergamon Press: Exeter, 1993. [Google Scholar]
- Pennini, F.; Plastino, A. Heisenberg–Fisher thermal uncertainty measure. Phys. Rev. E 2004, 69, 057101:1–057101:4. [Google Scholar] [CrossRef] [PubMed]
- Frieden, B.R. Physics from Fisher information; Cambridge University Press: Cambridge, England, 1998; Science from Fisher information; Cambridge University Press: Cambridge, England, 2004. [Google Scholar]
- Hall, J.W.M. Quantum properties of classical Fisher information. Phys. Rev. A 2000, 62, 012107:1–012107:6. [Google Scholar] [CrossRef]
- Munro, W.J.; James, D.F.V.; White, A.G.; Kwiat, P.G. Maximizing the entanglement of two mixed qubits. Phys. Rev. A 2004, 64, 030302:1–030302:4. [Google Scholar] [CrossRef]
- Batle, J.; Plastino, A.R.; Casas, M.; Plastino, A. Conditional q–entropies and quantum separability: a numerical exploration. J. Phys. A: Math. Gen. 2002, 35, 10311–10324. [Google Scholar] [CrossRef]
- Pennini, F.; Plastino, A.; Ferri, G.L.; Olivares, F. Semiclassical localization and uncertainty principle. Phys. Lett. A 2008, 372, 4870–4873. [Google Scholar] [CrossRef]
© 2009 by the authors; licensee Molecular Diversity Preservation International, Basel, Switzerland. This article is an open-access article distributed under the terms and conditions of the Creative Commons Attribution license (https://creativecommons.org/licenses/by/3.0/).
Share and Cite
Pennini, F.; Plastino, A.; Ferri, G.L.; Olivares, F.; Casas, M. Information, Deformed қ-Wehrl Entropies and Semiclassical Delocalization. Entropy 2009, 11, 32-41. https://doi.org/10.3390/e11010032
Pennini F, Plastino A, Ferri GL, Olivares F, Casas M. Information, Deformed қ-Wehrl Entropies and Semiclassical Delocalization. Entropy. 2009; 11(1):32-41. https://doi.org/10.3390/e11010032
Chicago/Turabian StylePennini, Flavia, Angelo Plastino, Gustavo L. Ferri, Felipe Olivares, and Montse Casas. 2009. "Information, Deformed қ-Wehrl Entropies and Semiclassical Delocalization" Entropy 11, no. 1: 32-41. https://doi.org/10.3390/e11010032
APA StylePennini, F., Plastino, A., Ferri, G. L., Olivares, F., & Casas, M. (2009). Information, Deformed қ-Wehrl Entropies and Semiclassical Delocalization. Entropy, 11(1), 32-41. https://doi.org/10.3390/e11010032



