Stress Field Approach for Prediction of End Concrete Cover Separation in RC Beams Strengthened with FRP Reinforcement
Abstract
:1. Introduction
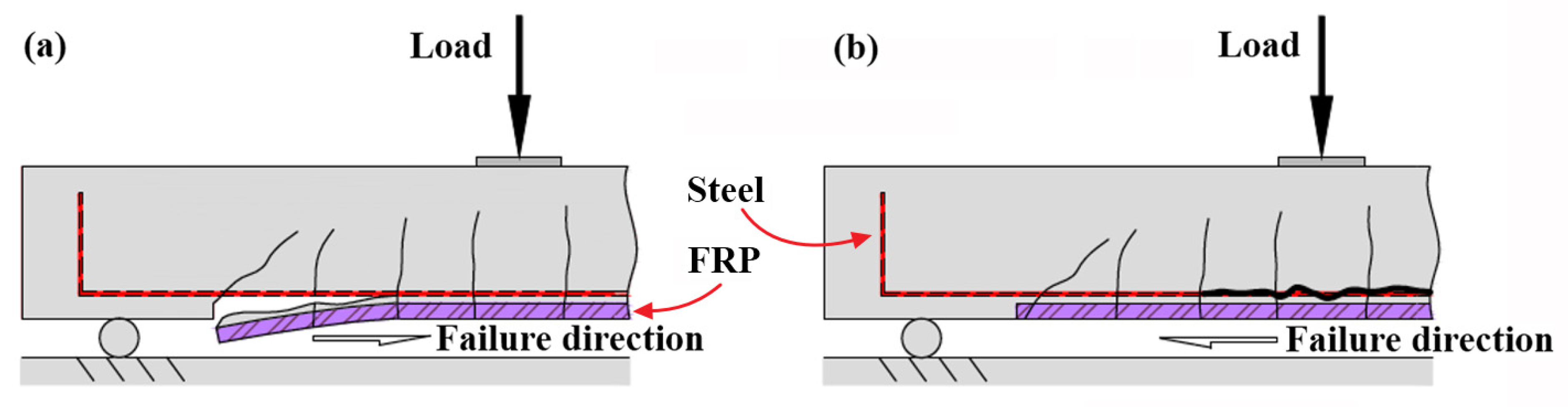
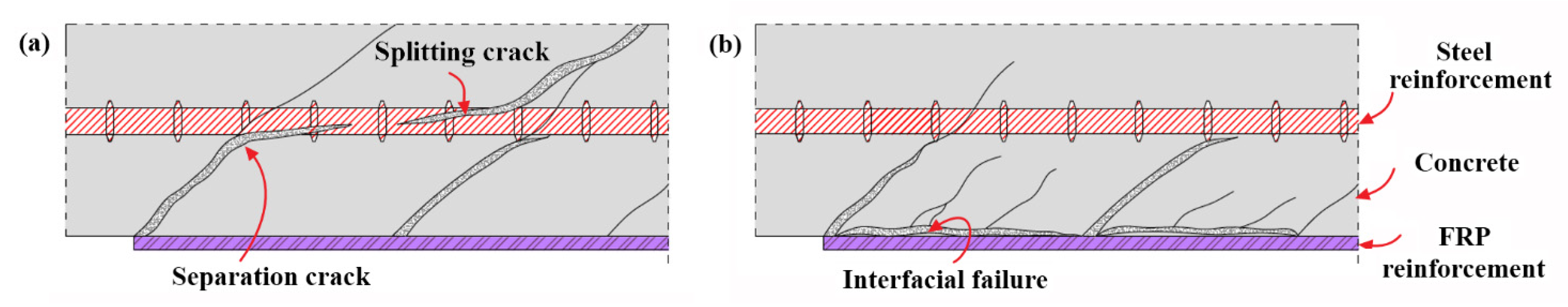
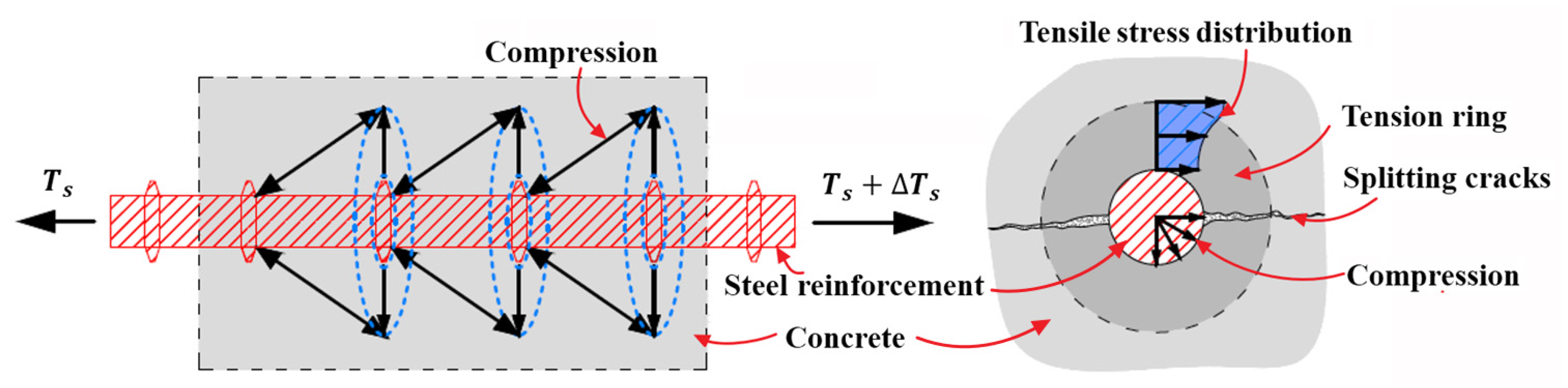
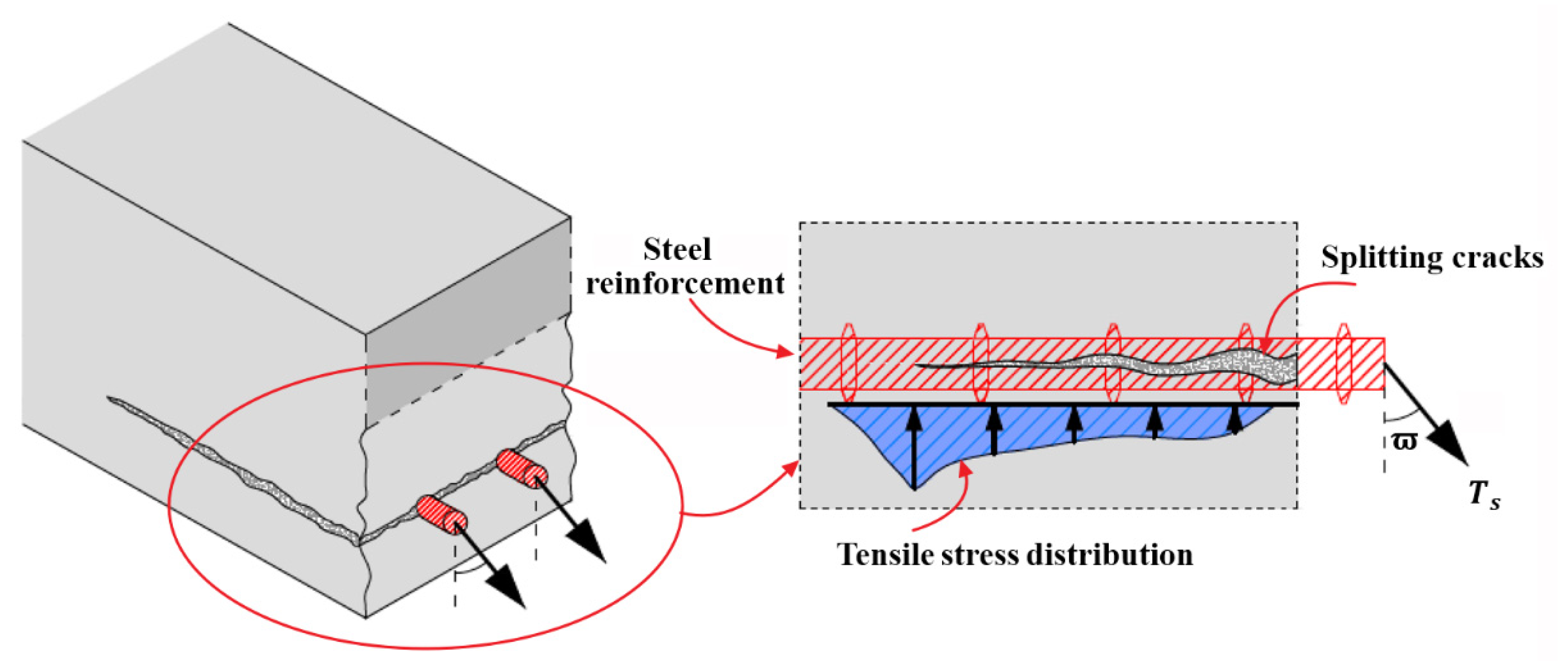
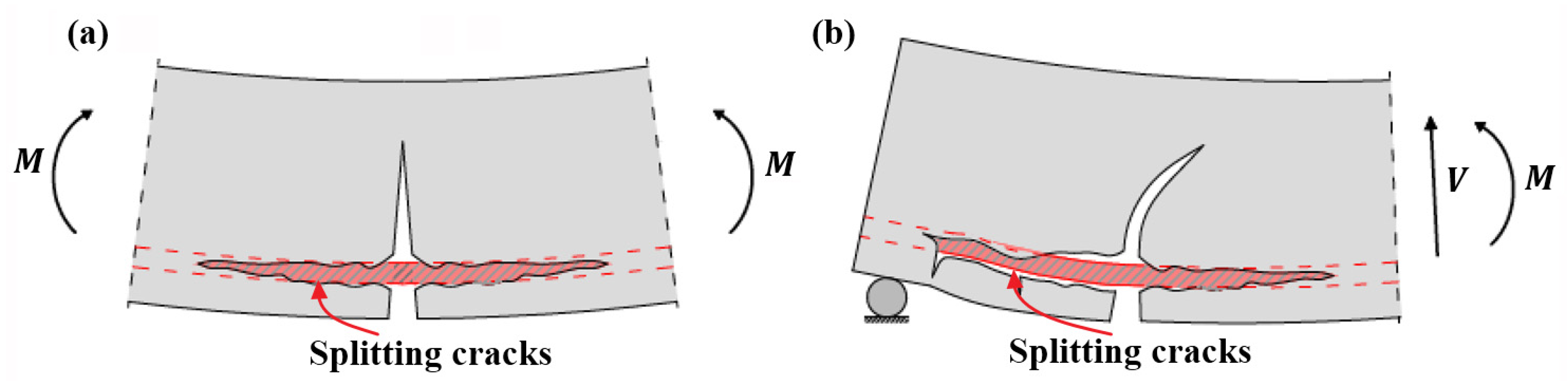
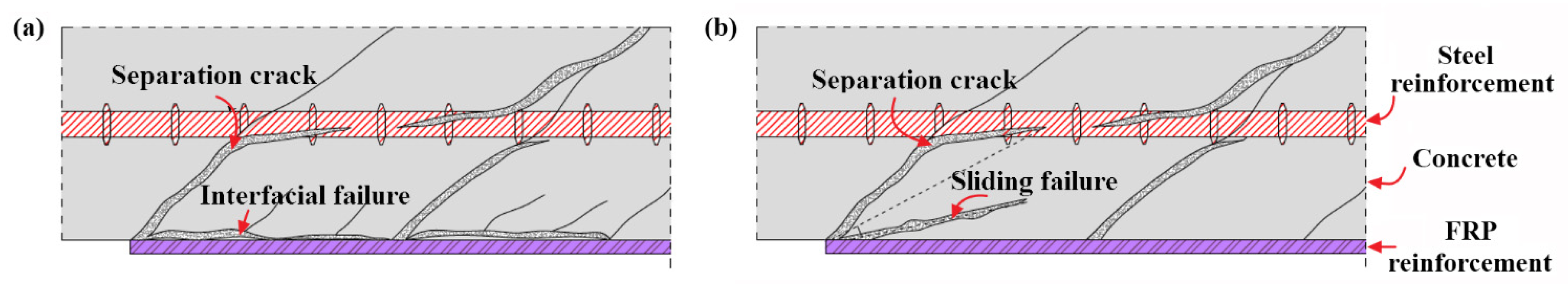
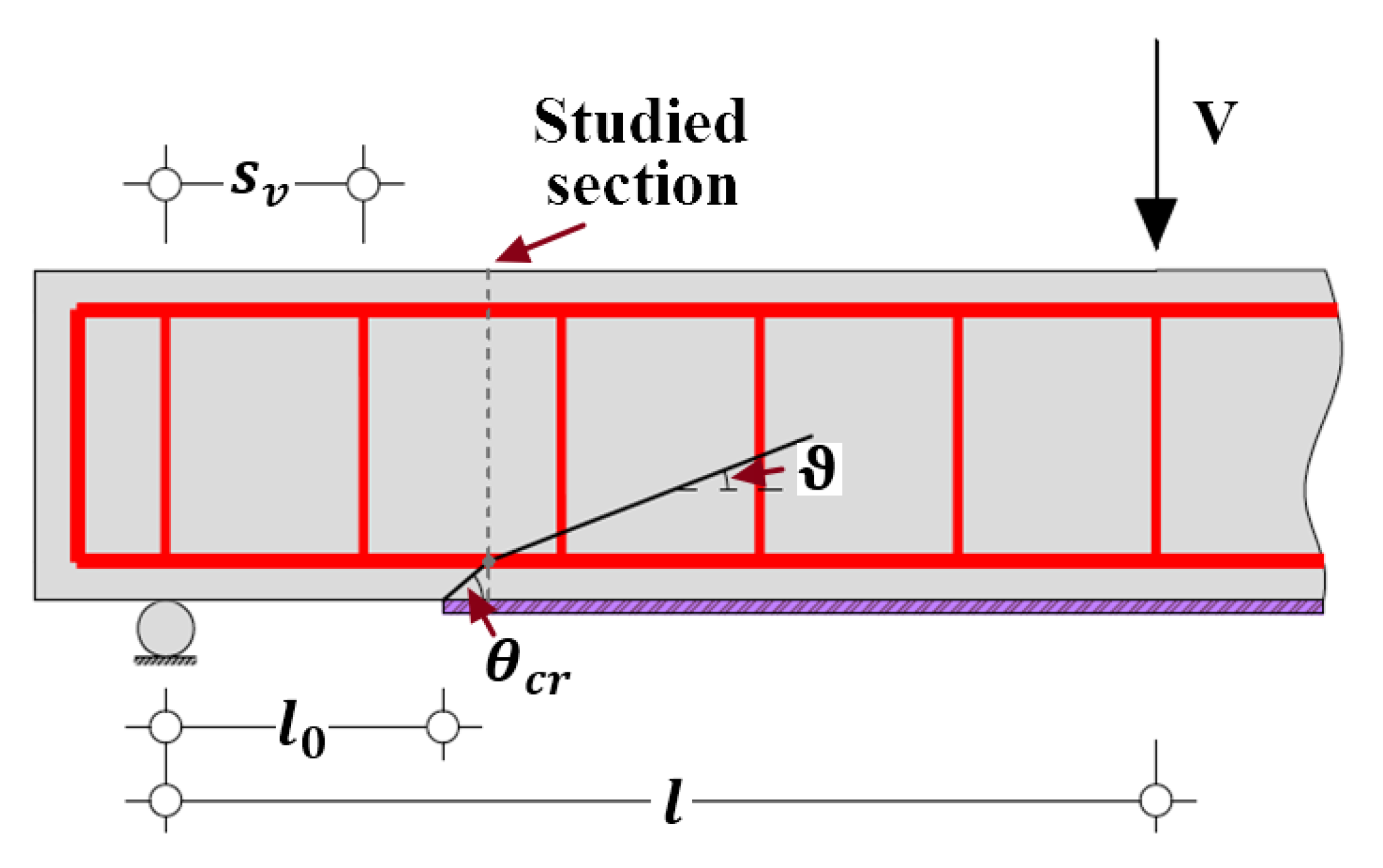
2. Analysis of Mechanical State and Failure Mechanism of Concrete Cover Separation
3. Analytical Model to Predict Concrete Cover Separation
3.1. Assumptions and Simplifications
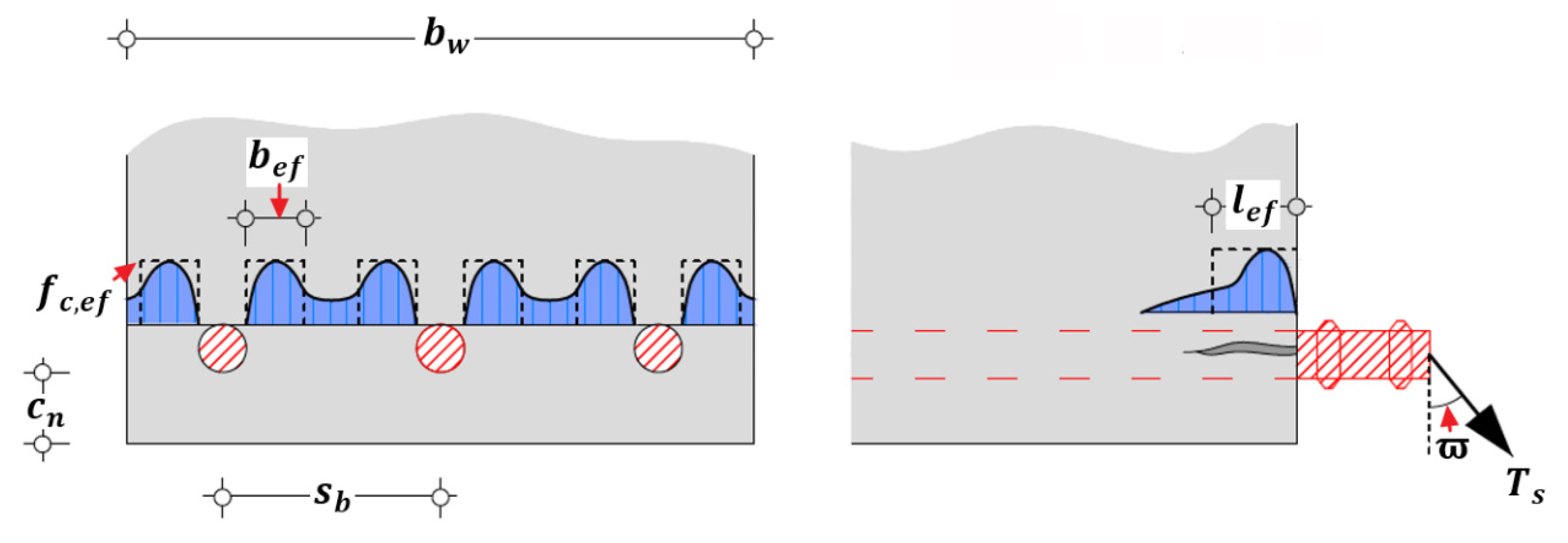
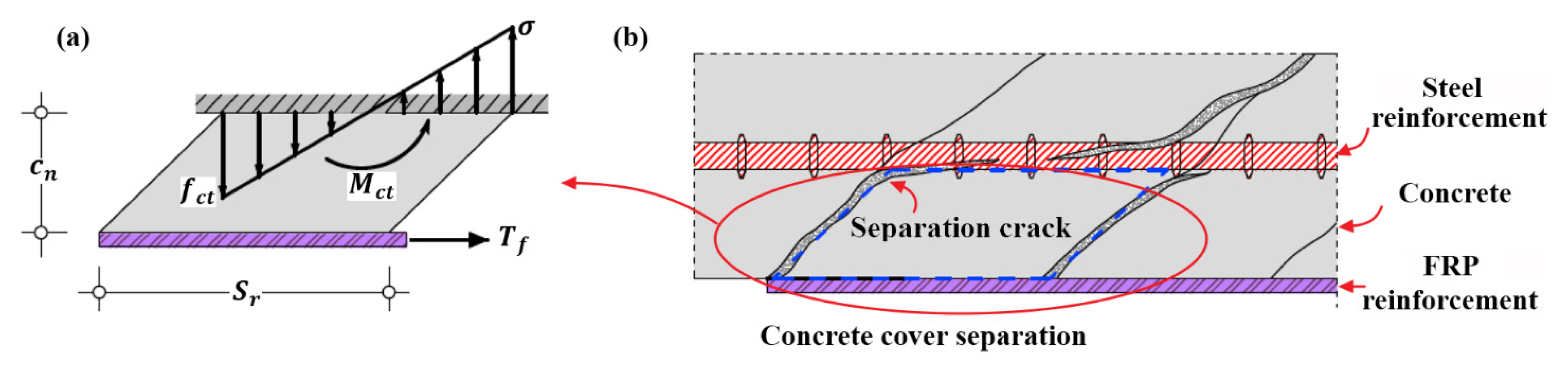
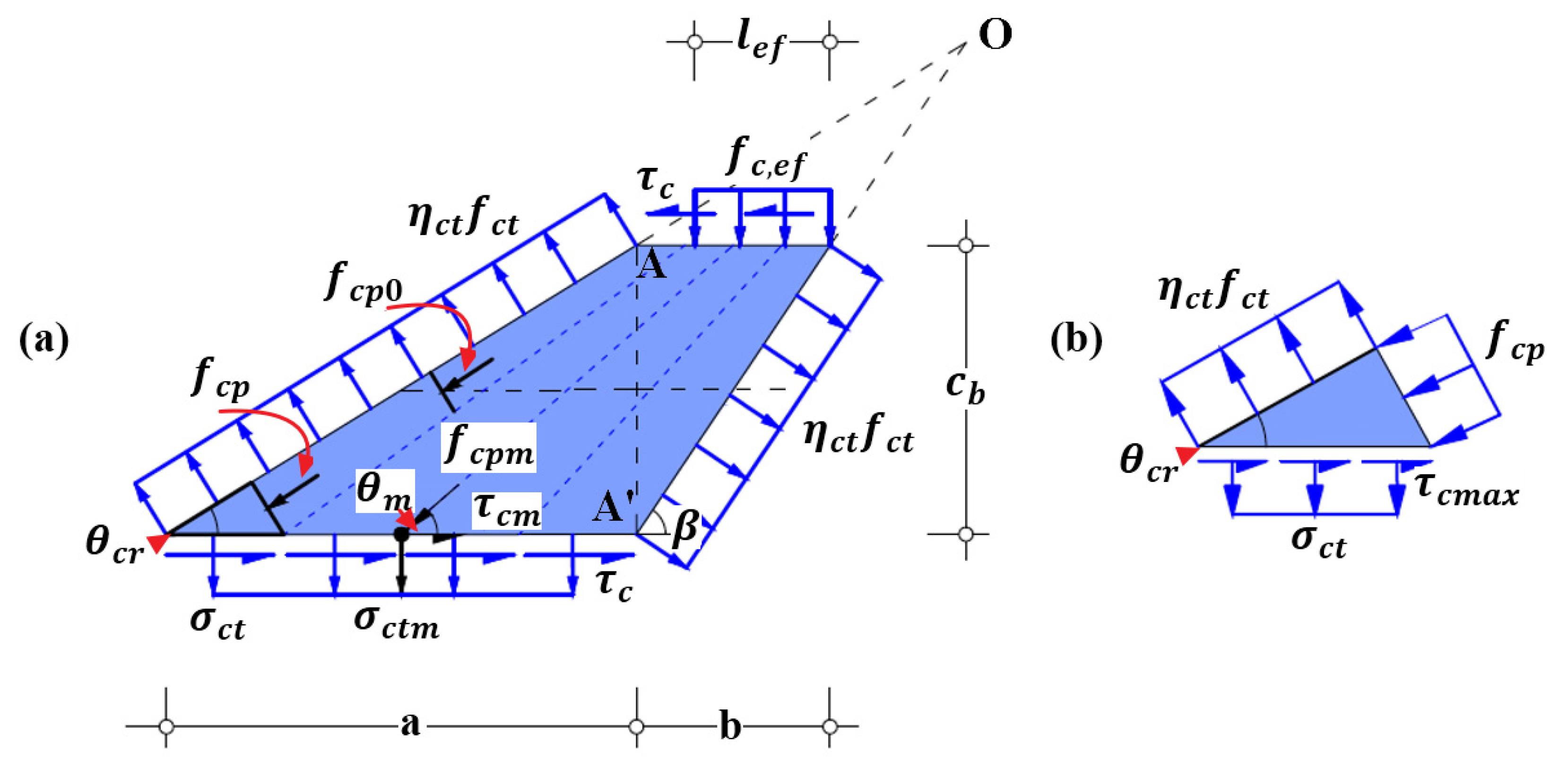
3.2. Specifications of the Mechanical and Geometrical Conditions of Stress Field for Cracked Concrete Block
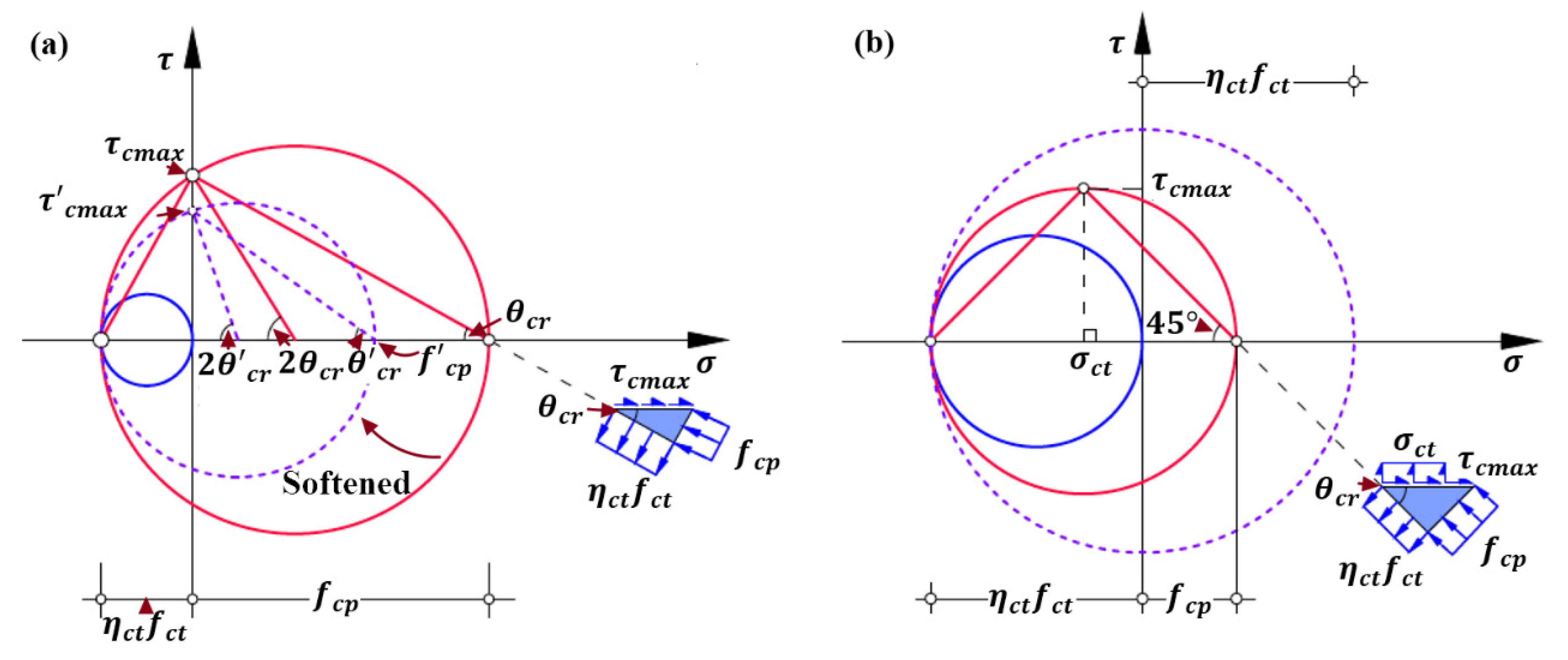
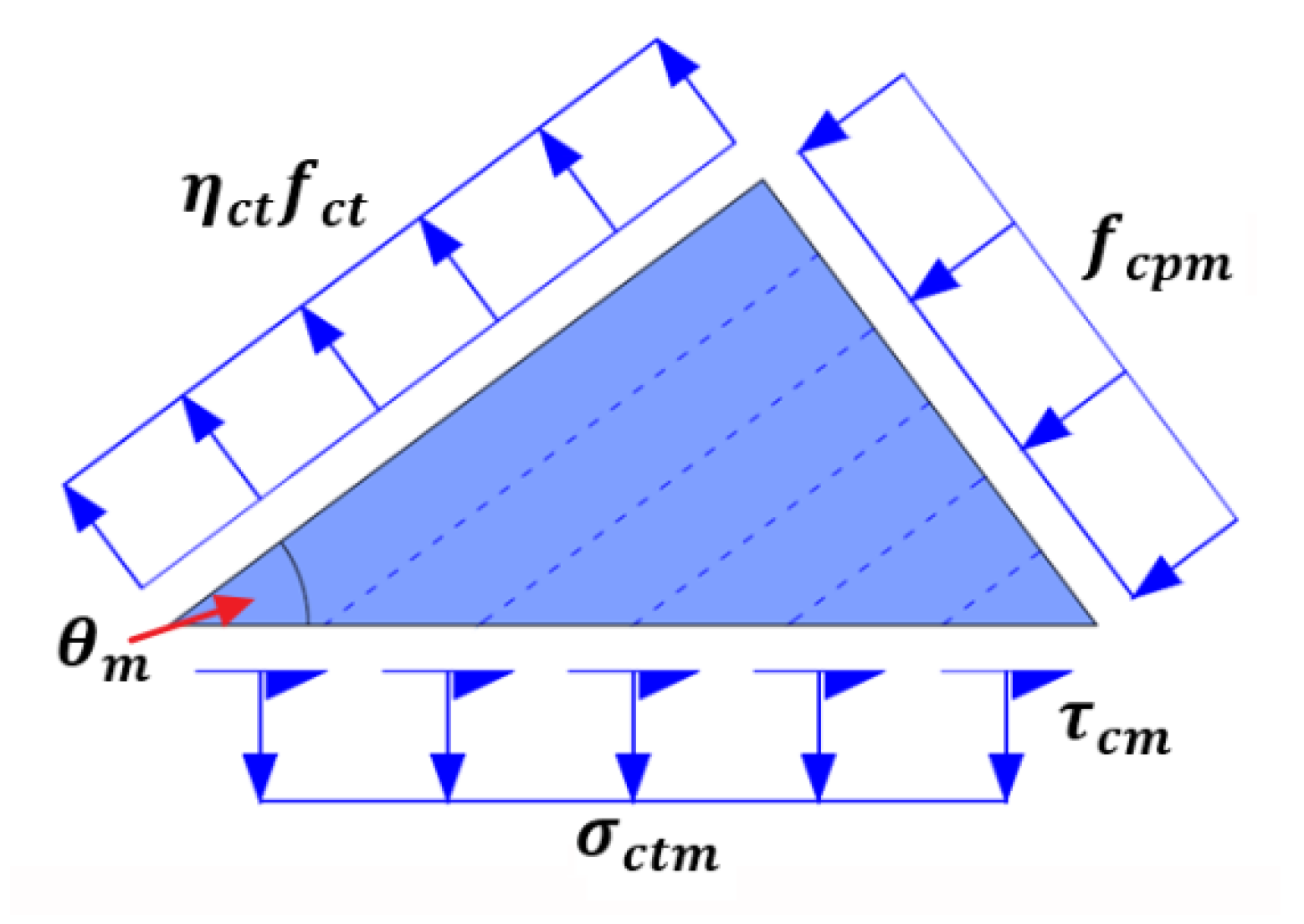
3.3. Failure Criterion
3.3.1. Critical Mechanical State of Concrete Cover Separation
3.3.2. Effective Compressive Strength of Concrete
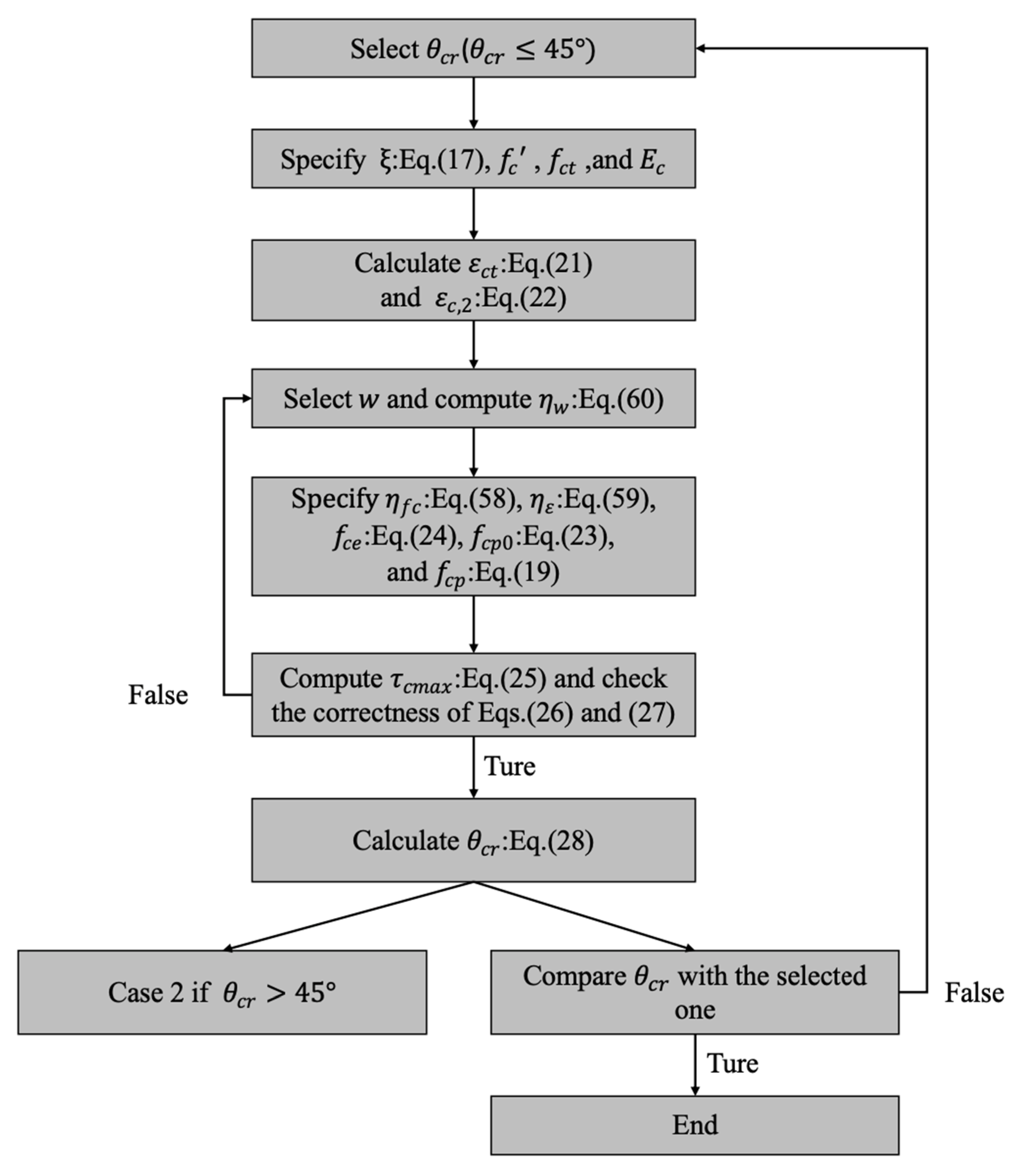
3.3.3. Failure Strength of Concrete Cover Separation and Cracking Angle of Concrete Block
- Select a cracking angle ( <);
- Specify the geometrical parameter , cylinder compressive strength , tensile strength , and static Young’s modulus of concrete ;
- Calculate the cracking strain of tensile concrete and the principal compressive strain ;
- Select a splitting cracking width of and compute the cracked concrete influential coefficient ;
- Figure out the plasticity of concrete coefficient , transverse strain influential coefficient , effective compressive strength of concrete , and principal compressive stresses and ;
- Check the correctness of principal compressive stress according to the maximum local shear stress , estimated by Equations (25) and (26), and the maximum principal compressive stress , derived by Equation (27);
- If Step 6 is false, adjust the value of and then repeat Steps 4–6;
- If Step 6 is true, calculate the cracking angle from Equation (28) using the obtained material properties and relevant parameters;
- If the computed cracking angle in step 8 is larger than , calculate the cracking angle and maximum shear stress defined in Case 2;
- Check the computed cracking angle in step 8 with the assumed one in Step 1;
3.3.4. Effective Tensile Strain of FRP Reinforcement Corresponding to Concrete Cover Separation
4. Analytical Model of Carrying Capacity of the FRP-Strengthened RC Beams That Failed in Concrete Cover Separation
4.1. Background to the Proposed Model
4.2. Analytical Model
4.2.1. Flexural Behavior
4.2.2. Shear Behavior
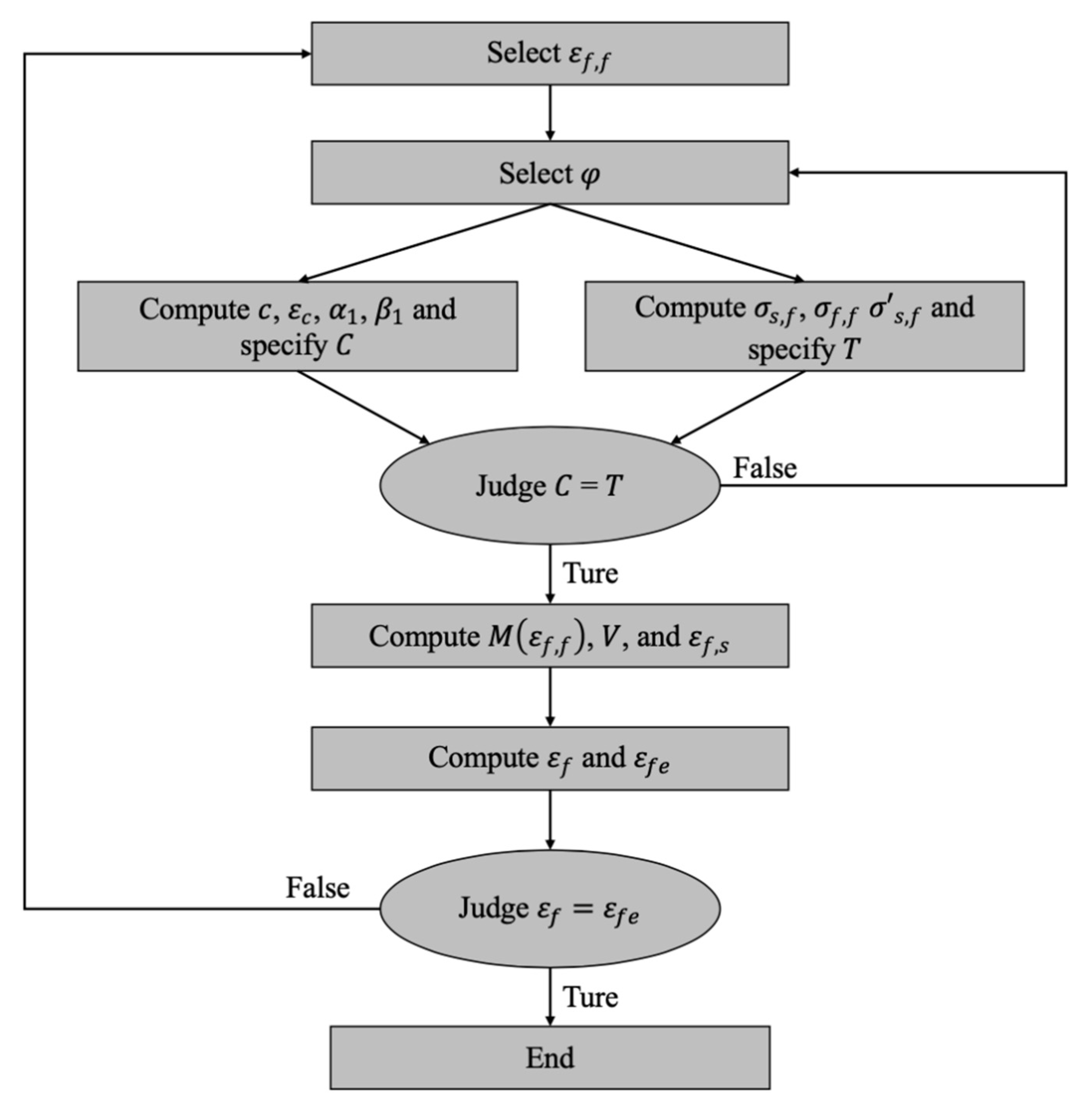
4.3. Analytical Process
- Select a flexural strain ;
- Select a curvature ;
- Figure out the depth of compressive region , the strain of concrete extreme compression fiber , and the parameters of the equivalent stress block and ;
- Specify the reinforcement stresses of , and in flexural behavior;
- Compute the resultant force at tensile region and at compressive region;
- If is not equal to , repeat steps 2–5;
- If is equal to , compute the moment , the shear , and the strain ;
- Compute the strain of FRP reinforcement under flexural–shear action and the effective tensile strain of FRP reinforcement ;
- If is not equal to , repeat steps 1–8; and
- If is equal to , obtain the desired carrying capacity of and of a strengthened RC beam.
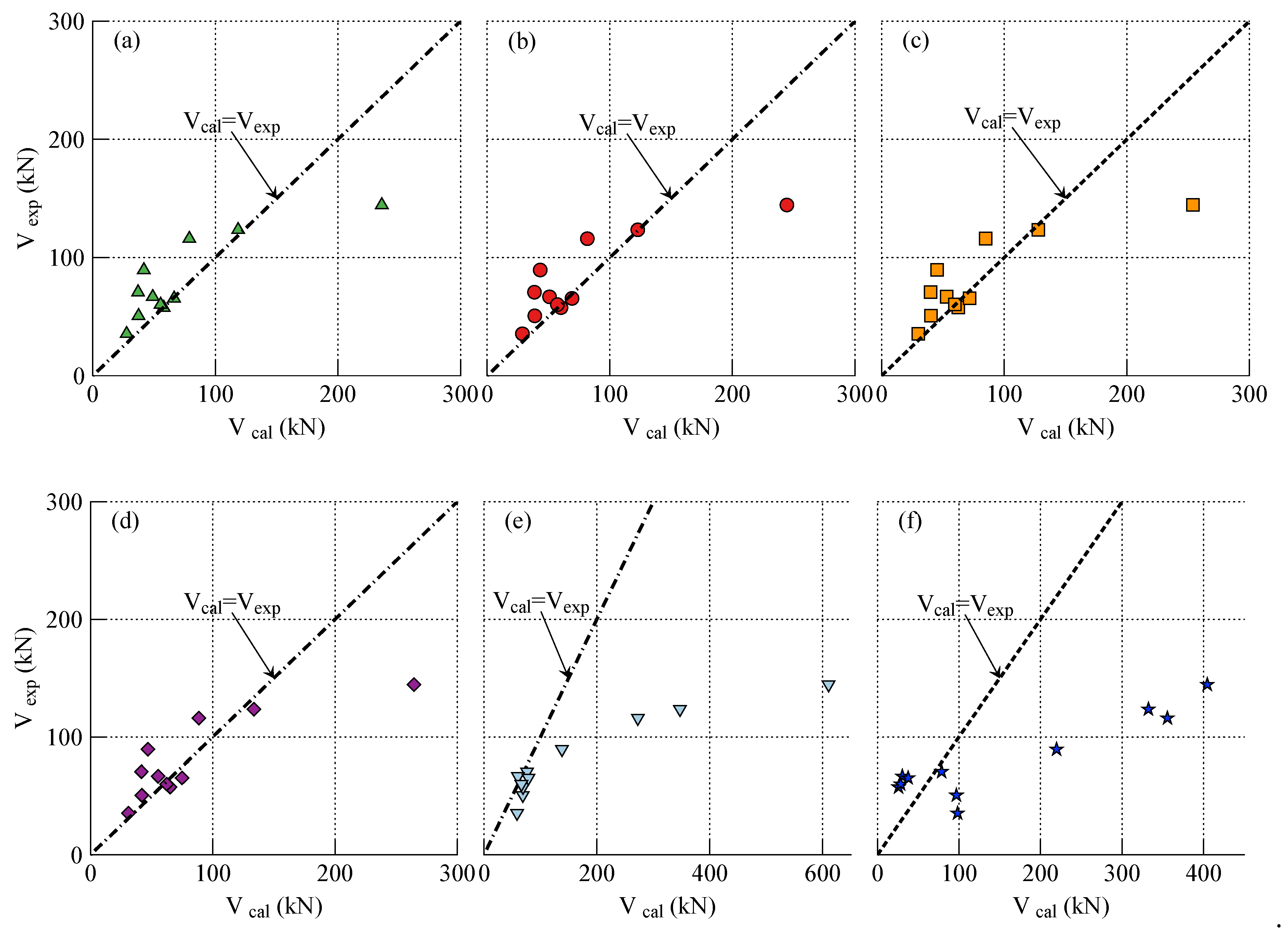
5. Validations and Discussions
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Reducing Coefficients of Compressive Strength of Concrete
- The plasticity of concrete coefficientTo consider the plasticity of concrete, Muttoni et al. [60] presented the reducing coefficient, expressed by Equation (A1), to modify the compressive strength of concrete.
- The transverse strain influential coefficientThe effective compressive strength of concrete in biaxial stressed state is highly sensitive to its transverse strain [58,59,60,61,62,63,64,65]. Kaufmann et al. [61] proposed a coefficient to reflect the softening effect of transverse strain of stress field for reinforced concrete on compressive strength of concrete, which is represented by Equation (A2):where is the transverse strain of stress field for reinforced concrete and is assigned with the transverse strain of concrete strut in this study.
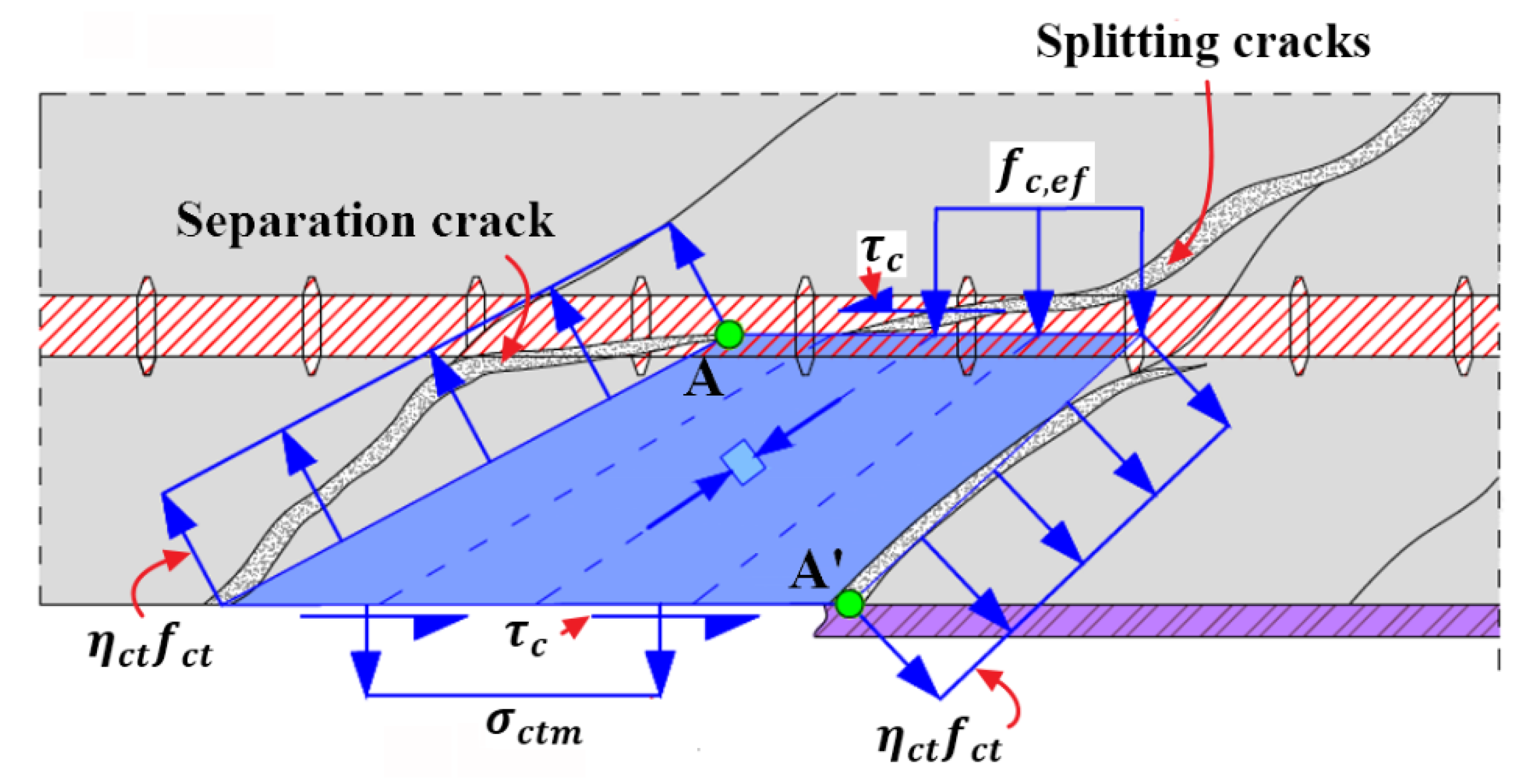
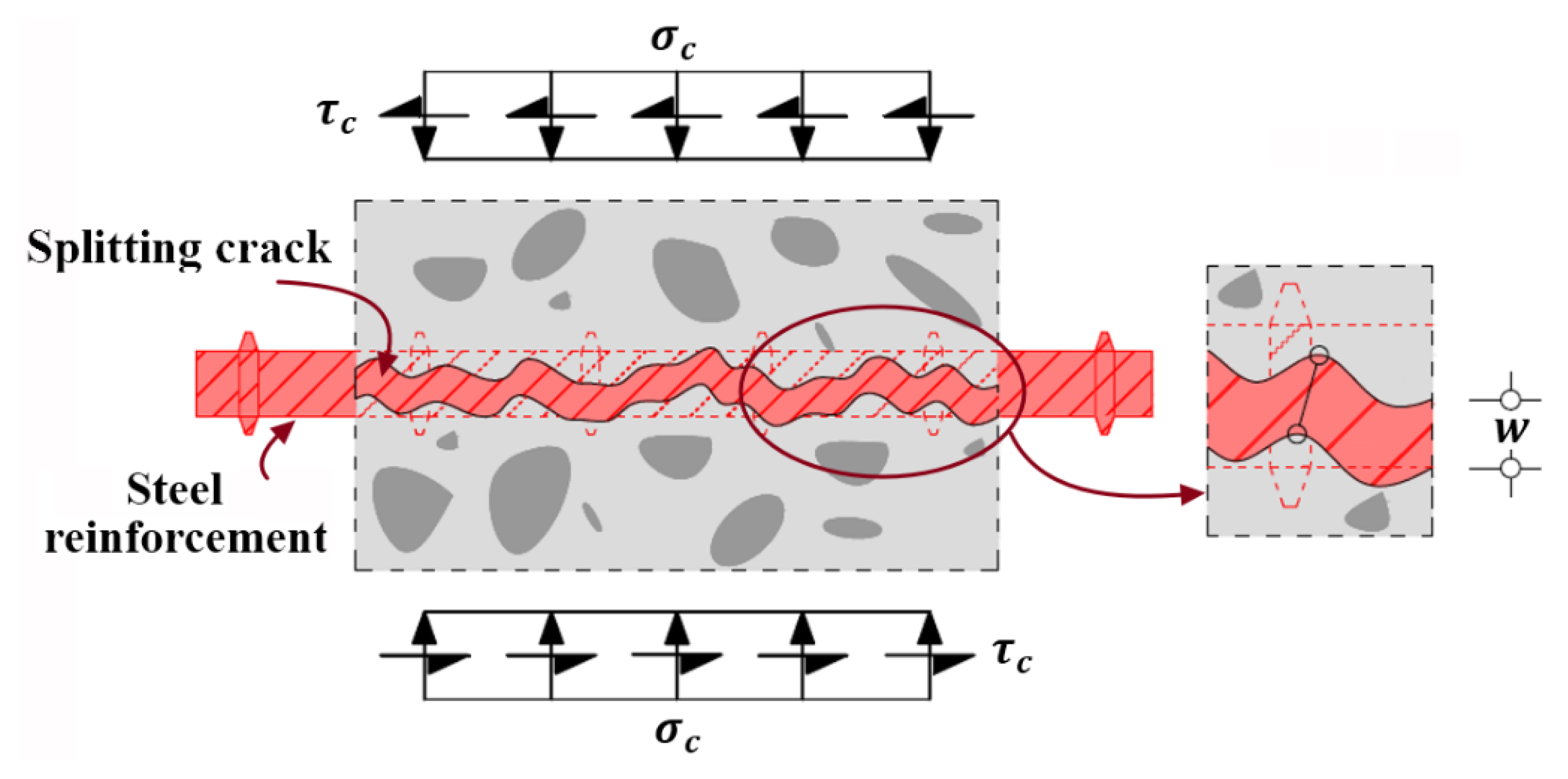
- The cracked concrete influential coefficientThe cracked concrete is still able to transfer the shear stress and the normal compressive or tensile stress ; furthermore, the ultimate capacity depends on the crack width, as schematically shown in Figure 10 [71]. Through the experimental and analytical investigations about aggregate interlock of cracked concrete, Fernández Ruiz [71] presented a cracked concrete influential coefficient to consider the influence of crack width on compressive strength and shear capacity of cracked concrete. The formulation of reducing coefficient is expressed by Equations (A3) and (A4) [71]:where is the crack with of cracked concrete and refers to the width of splitting crack in this study (Figure 10); is the constant parameter and assigned with 100 [56,71,72]; is the equivalent surface roughness and not larger than 40 mm; is the reference aggregate size and equal to 16 mm; and is the maximum aggregate size.
References
- Meier, U. Carbon fiber reinforced polymers, modern materials in bridge engineering. Struct. Eng. Int. 1992, 2, 7–12. [Google Scholar] [CrossRef]
- Teng, J.G.; Chen, J.F.; Smith, S.T.; Lam, L. FRP-Strengthened RC Structures; John Wiley & Sons Ltd: Chichester, UK, 2002. [Google Scholar]
- El Hacha, R.; Rizkalla, S.H. Near-surface-mounted fiber-reinforced polymer reinforcements for flexural strengthening of concrete structures. ACI Struct. J. 2004, 101, 717–726. [Google Scholar]
- De Lorenzis, L.; Teng, J.G. Near-surface mounted FRP reinforcement: An emerging technique for strengthening structures. Compos. B Eng. 2007, 38, 119–143. [Google Scholar] [CrossRef]
- Bencardino, F.; Spadea, G.; Swamy, R.N. Strength and ductility of reinforced concrete beams externally reinforced with carbon fiber fabric. Struct. J. 2002, 99, 163–171. [Google Scholar]
- Aram, M.R.; Czaderski, C.; Motavalli, M. Debonding failure modes of flexural FRP-strengthened RC beam. Compos. B Eng. 2008, 39, 826–841. [Google Scholar] [CrossRef]
- Zhang, S.S.; Yu, T.; Chen, G.M. Reinforced concrete beams strengthened in flexure with near-surface mounted (NSM) CFRP strips: Current status and research needs. Compos. B Eng. 2017, 131, 30–42. [Google Scholar] [CrossRef] [Green Version]
- Smith, S.T.; Teng, J.G. FRP-strengthened RC beams II: Assessment of debonding strength models. Eng. Struct. 2002, 24, 397–417. [Google Scholar] [CrossRef]
- Smith, S.T.; Teng, J.G. Shear-bending interaction in debonding failures of FRP-plated RC beams. Adv. Struct. Eng. 2003, 6, 183–199. [Google Scholar] [CrossRef]
- Teng, J.G.; De Lorenzis, L.; Wang, B.; Rong, L.; Wong, T.N.; Lam, L. Debonding failures of RC beams strengthened with near-surface mounted CFRP strips. J. Compos. Constr. 2006, 10, 92–105. [Google Scholar] [CrossRef]
- Oehlers, D.J.; Rashid, R.; Seracino, R. IC debonding resistance of groups of FRP NSM strips in reinforced concrete beams. Constr. Build. Mater. 2008, 22, 1574–1582. [Google Scholar] [CrossRef]
- Barros, J.A.O.; Fortes, A.S. Flexural strengthening of concrete beams with CFRP laminates bonded into slits. Cem. Concr. Compos. 2005, 27, 471–480. [Google Scholar] [CrossRef] [Green Version]
- Barros, J.A.O.; Dias, S.J.E.; Lima, J.L.T. Efficacy of CFRP-based techniques for the flexural and shear strengthening of concrete beams. Cem. Concr. Compos. 2007, 29, 203–217. [Google Scholar] [CrossRef]
- Esfahani, M.R.; Kianoush, M.R.; Tajari, A.R. Flexural behaviour of reinforced concrete beams strengthened by CFRP sheets. Eng. Struct. 2007, 29, 2428–2444. [Google Scholar] [CrossRef]
- Garden, H.N.; Quantrill, R.J.; Hollaway, L.C.; Thorne, A.M.; Parke, G.A.R. An experimental study of the anchorage length of carbon fibre composite plates used to strengthen reinforced concrete beams. Constr. Build. Mater. 1998, 12, 203–219. [Google Scholar] [CrossRef]
- Yao, J.; Teng, J.G. Plate end debonding in FRP-plated RC beams—I: Experiments. Eng. Struct. 2007, 29, 2457–2471. [Google Scholar] [CrossRef]
- Aprile, A.; Benedetti, A. Coupled flexural-shear design of R/C beams strengthened with FRP. Compos. B Eng. 2004, 35, 1–25. [Google Scholar] [CrossRef]
- Aprile, A.; Luciano, F. Concrete cover rip-off of R/C beams strengthened with FRP composites. Compos. B Eng. 2007, 38, 759–771. [Google Scholar] [CrossRef]
- De Lorenzis, L.; Nanni, A. Bond between near-surface mounted fiber-reinforced polymer rods and concrete in structural strengthening. ACI Struct. J. 2002, 99, 123–132. [Google Scholar]
- Bilotta, A.; Ceroni, F.; Nigro, E.; Pecce, M. Efficiency of CFRP NSM strips and EBR plates for flexural strengthening of RC beams and loading pattern influence. Compos. Struct. 2015, 124, 163–175. [Google Scholar] [CrossRef]
- Czaderski, C. Strengthening of Reinforced Concrete Members by Prestressed Externally Bonded Reinforcement with Gradient Method. Ph.D. Thesis, ETH Zürich, Zurich, Switzerland, 2012. [Google Scholar]
- Sabzi, J.; Esfahani, M.R. Effects of tensile steel bars arrangement on concrete cover separation of RC beams strengthened by CFRP sheets. Constr. Build. Mater. 2018, 162, 470–479. [Google Scholar] [CrossRef]
- Sabzi, J.; Esfahani, M.R.; Ozbakkaloglu, T.; Farahi, B. Effect of concrete strength and longitudinal reinforcement arrangement on the performance of reinforced concrete beams strengthened using EBR and EBROG methods. Eng. Struct. 2020, 205, 110072. [Google Scholar] [CrossRef]
- Sharaky, I.A.; Selmy, S.A.I.; El-Attar, M.M.; Sallam, H.E.M. The influence of interaction between NSM and internal reinforcements on the structural behavior of upgrading RC beams. Compos. Struct. 2020, 234, 111751. [Google Scholar] [CrossRef]
- Al-Saawani, M.; El-Sayed, A.; Al-Negheimish, A. Effect of shear-span/depth ratio on debonding failures of FRP-strengthened RC beams. J. Build. Eng. 2020, 32, 101771. [Google Scholar] [CrossRef]
- Rezazadeh, M.; Barros, J.A.O.; Ramezansefat, H. End concrete cover separation in RC structures strengthened in flexure with NSM FRP: Analytical design approach. Eng. Struct. 2016, 128, 415–427. [Google Scholar] [CrossRef] [Green Version]
- Gao, B.; Leung, C.K.Y.; Kim, J.K. Prediction of concrete cover separation failure for RC beams strengthened with CFRP strips. Eng. Struct. 2005, 27, 177–189. [Google Scholar] [CrossRef]
- Pham, H.; Al-Mahaidi, R. Prediction models for debonding failure loads of carbon fiber reinforced polymer retrofitted reinforced concrete beams. J. Compos. Construct. 2006, 10, 48–59. [Google Scholar] [CrossRef]
- Raoof, M.; Zhang, S. An insight into the structural behaviour of reinforced concrete beams with externally bonded plates. Proc. Inst. Civ. Eng. Struct. Build. 1997, 122, 477–492. [Google Scholar] [CrossRef]
- Raoof, M.; Hassanen, M.A.H. Peeling failure of reinforced concrete beams with fibre-reinforced plastic or steel plates glued to their soffits. Proc. Inst. Civ. Eng. Struct. Build. 2000, 140, 291–305. [Google Scholar] [CrossRef]
- Chaallal, O.; Nollet, M.J.; Perraton, D. Strengthening of reinforced concrete beams with externally bonded fiber-reinforced-plastic plates: Design guidelines for shear and flexure. Can. J. Civ. Eng. 1998, 25, 692–704. [Google Scholar] [CrossRef]
- Teng, J.G.; Zhang, S.S.; Chen, J.F. Strength model for end cover separation Failure in RC beams strengthened with near-surface mounted (NSM) FRP strips. Eng. Struct. 2016, 110, 222–232. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.S.; Teng, J.G. Finite element analysis of end cover separation in RC beams strengthened in flexure with FRP. Eng. Struct. 2014, 75, 550–560. [Google Scholar] [CrossRef]
- Zhang, S.S.; Teng, J.G. End cover separation in RC beams strengthened in flexure with bonded FRP reinforcement: Simplified finite element approach. Mater. Struct. 2016, 49, 2223–2236. [Google Scholar] [CrossRef]
- Hawileh, R. Nonlinear finite element modeling of RC beams strengthened with NSM FRP rods. Constr. Build. Mater. 2011, 27, 461–471. [Google Scholar] [CrossRef]
- Camata, G.; Spacone, E.; Zarnic, R. Experimental and nonlinear finite element studies of RC beams strengthened with FRP plates. Compos. B Eng. 2007, 38, 277–288. [Google Scholar] [CrossRef]
- Zhang, D.; Shi, H.; Zhu, J.; Su, M.; Jin, W.L. Cover separation of CFRP strengthened beam type cantilevers with steel bolt anchorage. Eng. Struct. 2018, 156, 224–234. [Google Scholar] [CrossRef] [Green Version]
- Radfar, S.; Foret, G.; Saeedi, N.; Sab, K. Simulation of concrete cover separation failure in FRP plated RC beams. Constr. Build. Mater. 2012, 37, 791–800. [Google Scholar] [CrossRef]
- De Maio, U.; Fabbrocino, F.; Greco, F.; Leonetti, L.; Lonetti, P. A study of concrete cover separation failure in FRP-plated RC beams via an inter-element fracture approach. Compos. Struct. 2019, 212, 625–636. [Google Scholar] [CrossRef]
- Roberts, T.M. Approximate analysis of shear and normal stress concentrations in the adhesive layer of plated RC beams. Struct. Eng. 1989, 67, 229–233. [Google Scholar]
- Malek, A.M.; Saadatmanesh, H.; Ehsani, M.R. Prediction of failure load of R/C beams strengthened with FRP plate due to stress concentration at the plate end. ACI Struct. J. 1998, 95, 142–152. [Google Scholar]
- Oehlers, D.J.; Park, S.M.; Mohamed Ali, M.S. A structural engineering approach to adhesive bonding longitudinal plates to RC beams and slabs. Compos. Part A 2003, 34, 887–897. [Google Scholar] [CrossRef]
- Jansze, W. Strengthening of RC Members in Bending by Externally Bonded Steel Plates. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1997. [Google Scholar]
- Ziraba, Y.N.; Baluch, M.H.; Basunbul, I.A.; Sharif, A.M.; Azad, A.K.; Al-Sulaimani, G.J. Guidelines towards the design of reinforced concrete beams with external plates. ACI Struct. J. 1994, 91, 639–646. [Google Scholar]
- Sallam, H.E.M.; Saba, A.M.; Shaheen, H.H.; Abdel-Raouf, H. Prevention of peeling failure in plated beams. J. Adv. Concr. Technol. 2004, 2, 419–429. [Google Scholar] [CrossRef] [Green Version]
- Narayanamurthy, V.; Chen, J.F.; Cairns, J.; Oehlers, D.J. Plate end debonding in the constant bending moment zone of plated beams. Compos. B Eng. 2012, 43, 3361–3373. [Google Scholar] [CrossRef]
- Buyukozturk, O.; Gunes, O.; Karaca, E. Progress on understanding debonding problems in reinforced concrete and steel members strengthened using FRP composites. Constr. Build. Mater. 2004, 18, 9–19. [Google Scholar] [CrossRef]
- Tepfers, R.; Olsson, P.A. Ring test for evaluation of bond properties of reinforcing bars. In Proceedings of the International Conference from Research to Practice-Bond in Concrete (CEB), Riga, Latvia, 15–17 October 1992; pp. 89–99. [Google Scholar]
- Fernández Ruiz, M.; Plumey, S.; Muttoni, A. Interaction between bond and deviation forces in spalling failures of arch-shaped members without transverse reinforcement. ACI Struct. J. 2010, 107, 346–354. [Google Scholar]
- Moccia, F.; Fernández Ruiz, M.; Muttoni, A. Spalling of concrete cover induced by reinforcement. Eng. Struct. 2021, 19, 112188. [Google Scholar] [CrossRef]
- Baumann, T.; Rüsch, H. Tests on Dowelling Action of Flexural Reinforcement of Reinforced Concrete Beams (in German: Versuche zum Studium der Verdubelungswirkung der Biegezugbewehrung eines Stahlbetonbalkens); Deutscher Ausscuss für Stahlbeton; Wilhelm Ernst & Sohn: Berlin, Germany, 1970; Volume 210, pp. 42–83. [Google Scholar]
- Giuriani, E.; Plizzari, G.; Schumm, C. Role of stirrups and residual tensile strength of cracked concrete on bond. J. Struct. Eng. 1991, 117, 1–18. [Google Scholar] [CrossRef]
- Giuriani, E.; Plizzari, G. Interrelation of splitting and flexural cracks in RC beams. J. Struct. Eng. 1998, 124, 1032–1049. [Google Scholar] [CrossRef]
- Fernández Ruiz, M.; Mirzaei, Y.; Muttoni, A. Post-punching behavior of flat slabs. ACI Struct. J. 2013, 110, 801–812. [Google Scholar]
- Fernández Ruiz, M.; Muttoni, A.; Sagaseta, J. Shear strength of concrete members without transverse reinforcement: A mechanical approach to consistently account for size and strain effects. Eng. Struct. 2015, 99, 360–372. [Google Scholar] [CrossRef] [Green Version]
- Cavagnis, F.; Simões, J.T.; Fernández Ruiz, M.; Muttoni, A. Shear strength of members without transverse reinforcement based on development of critical shear crack. ACI Struct. J. 2020, 117, 103–118. [Google Scholar] [CrossRef]
- Zhou, B.; Wu, R.; Feng, J. Analytical model for rotation response of singly reinforced flexural members. ACI Struct. J. 2018, 115, 789–799. [Google Scholar] [CrossRef]
- Vecchio, F.J.; Collins, M.P. The modified compression-field theory for reinforced concrete elements subjected to shear. ACI J. Proc. 1986, 83, 219–231. [Google Scholar]
- Sigrist, V. Deformation Capacity of Reinforced Concrete Beams (In German: Zum Verformungsvermögen von Stahlbetonträgern). Ph.D. Thesis, ETH Zurich, Zurich, Switzerland, 1995. [Google Scholar]
- Muttoni, A.; Schwartz, J.; Thürlimann, B. Design of Concrete Structures with Stress Fields; Birkhäuser: Boston, MA, USA, 1996. [Google Scholar]
- Kaufmann, W.; Marti, P. Structural concrete: Cracked membrane model. J. Struct. Eng. 1998, 24, 1467–1475. [Google Scholar] [CrossRef]
- Bentz, E.C.; Vecchio, F.J.; Collins, M.P. Simplified modified compression field theory for calculating shear strength of reinforced concrete elements. ACI Struct. J. 2006, 103, 614–624. [Google Scholar]
- Sigrist, V. Generalized stress field approach for analysis of beams in shear. ACI Struct. J. 2011, 108, 479–487. [Google Scholar]
- Nielsen, M.P.; Hoang, L.C. Limit Analysis and Concrete Plasticity, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2011. [Google Scholar]
- Muttoni, A.; Fernández Ruiz, M.; Niketic, F. Design versus assessment of concrete structures using stress fields and strut-and-tie models. ACI Struct. J. 2015, 112, 605–615. [Google Scholar] [CrossRef] [Green Version]
- Tirassa, M.; Fernández Ruiz, M.; Muttoni, A. An interlocking approach for the rebar-to-concrete contact in bond. Mag. Concr. Res. 2020, 73, 379–393. [Google Scholar] [CrossRef]
- Lydon, F.D.; Balendran, R.V. Some observations on elastic properties of plain concrete. Cem. Concr. Res. 1986, 16, 314–324. [Google Scholar] [CrossRef]
- Kaneko, Y.; Connor, J.J.; Triantafillou, T.C.; Leung, C.L. Fracture mechanics approach for failure of concrete shear key, I: Theory. J. Eng. Mech. 1993, 119, 681–700. [Google Scholar] [CrossRef]
- Hsu, T.T.C.; Mau, S.T.; Chen, B. Theory on shear transfer strength of reinforced concrete. ACI Struct. J. 1987, 84, 149–160. [Google Scholar]
- Hognestad, E. A Study of Combined Bending and Axial Load in Reinforced Concrete Members; University of Illinois Engineering Experimental Station: Urbana, IL, USA, 1951. [Google Scholar]
- Fernández Ruiz, M. The influence of the kinematics of rough surface engagement on the transfer of forces in cracked concrete. Eng. Struct. 2021, 231, 111650. [Google Scholar] [CrossRef]
- Muttoni, A.; Fernández Ruiz, M. Shear strength of members without transverse reinforcement as function of critical shear crack width. ACI Struct. J. 2008, 105, 163–172. [Google Scholar]
- Walraven, J.C. Fundamental analysis of aggregate interlock. J. Struct. Divis. 1981, 107, 2245–2270. [Google Scholar] [CrossRef]
- Gambarova, P.G.; Rosati, G.P.; Zasso, B. Steel-to-concrete bond after concrete splitting: Test results. Mater. Struct. 1989, 22, 35–47. [Google Scholar] [CrossRef]
- Gambarova, P.G.; Rosati, G.P.; Zasso, B. Steel-to-concrete bond after concrete splitting: Constitutive laws and interface deterioration. Mater. Struct. 1989, 22, 347–356. [Google Scholar] [CrossRef]
- Gambarova, P.G.; Rosati, G.P. Bond and splitting in bar pull-out: Behavioural laws and concrete cover role. Mag. Concr. Res. 1997, 49, 99–110. [Google Scholar] [CrossRef]
- Gambarova, P.G.; Rosati, G.P. Bond and splitting in reinforced concrete: Test results on bar pull-out. Mater. Struct. 1996, 29, 267–276. [Google Scholar] [CrossRef]
- Dei Poli, S.; Di Prisco, M.; Gambarova, P.G. Cover and stirrup effects on the shear response of dowel bar embedded in concrete. ACI Struct. J. 1993, 90, 441–450. [Google Scholar]
- Herbrand, M.; Hegger, J. Querkraftmodell für Bauteile ohne Schubbewehrung unter Druck- und Zug- beanspruchung. Beton Stahlbetonbau 2017, 112, 704–713. [Google Scholar] [CrossRef]
- Nardone, F.; Lignola, G.P.; Prota, A.; Manfredi, G.; Nanni, A. Modeling of flexural behavior of RC beams strengthened with mechanically fastened FRP strips. Compos. Struct. 2011, 93, 1973–1985. [Google Scholar] [CrossRef]
- Grelle, S.V.; Sneed, L.H. Review of Anchorage Systems for Externally-Bonded FRP Laminates. Int. J. Concr. Struct. Mater. 2013, 7, 17–33. [Google Scholar] [CrossRef] [Green Version]
- Colombi, P.; Fava, G.; Poggi, C. End debonding of CFRP wraps and strips for the strengthening of concrete structures. Compos. Struct. 2014, 111, 510–521. [Google Scholar] [CrossRef]
- Brosens, K. Anchorage of Externally Bonded Steel Plates and CFRP Laminates for the Strengthening of Concrete Structures. Ph.D. Thesis, KU Leuven, Leuven, Belgium, 2001. [Google Scholar]
- Spinella, N. Modeling of shear behavior of reinforced concrete beams strengthened with FRP. Compos. Struct. 2019, 215, 351–364. [Google Scholar] [CrossRef]
- Mostafaei, H.; Vecchio, F.J. Uniaxial shear-flexure model for reinforced concrete elements. J. Struct. Eng. 2008, 134, 1538–1547. [Google Scholar] [CrossRef] [Green Version]
- Collins, M.P. Toward a rational theory for R/C members in shear. ASCE Proc. Struct. Div. 1978, 104, 649–666. [Google Scholar] [CrossRef]
- Zhou, B.; Wu, R.; Lu, S.; Yin, S. A general numerical model for predicting the flexural behavior of hybrid FRP-steel reinforced concrete beams. Eng. Struct. 2021, 239, 112293. [Google Scholar] [CrossRef]
- Fédération Internationale du Béton (FIB). fib Model Code for Concrete Structures 2010; Ernst & Sohn: Berlin, Germany, 2013. [Google Scholar]
- Holzenkämpfer, P. Ingenieur Modelle des Verbundes Geklebter Bewehrung für Betonbauteile. Ph.D. Thesis, TU Braunschweig, Braunschweig, Germany, 1994. [Google Scholar]
- FIB (International Federation for Structural Concrete). Externally bonded FRP reinforcement for RC structures: Basis of design and safety concept. FIB Bull. 2001, 14, 43–44. [Google Scholar]
- Al-Mahmoud, F.; Castel, A.; François, R.; Tourneur, C. RC beams strengthened with NSM CFRP rods and modeling of peeling-off failure. Compos. Struct. 2010, 92, 1920–1930. [Google Scholar] [CrossRef]
- Pham, H.; Al-Mahaidi, R. Assessment of available prediction models for the strength of FRP retrofitted RC beams. Compos. Struct. 2004, 66, 601–610. [Google Scholar] [CrossRef]




| Reference | Specimen | Geometries | Mechanical Properties of Concrete | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| l (mm) | h (mm) | |||||||||
| Smith et al. [9] | 1B | 25 | 500 | 250 | 205 | 45 | 45 | 31.5 | 2.4 | 23.3 |
| 2B | 125 | 500 | 250 | 205 | 45 | 45 | 48.6 | 3.6 | 28.8 | |
| 3B | 50 | 500 | 250 | 205 | 45 | 45 | 45.3 | 3.2 | 29.0 | |
| 6B | 75 | 500 | 250 | 205 | 45 | 45 | 41.0 | 2.9 | 29.4 | |
| Esfahani et al. [14] | B3 | 100 | 600 | 200 | 166 | 34 | 25 | 25.2 | 2.6 | 23.7 |
| Yao et al. [16] | CS-L3-B | 50 | 500 | 253 | 217 | 36 | 35 | 26.3 | 3.5 | 27.2 |
| CS-W100-B | 50 | 500 | 254 | 214 | 41 | 35 | 30.2 | 3.3 | 24.3 | |
| CP-B | 50 | 500 | 253 | 218 | 35 | 35 | 26.2 | 3.8 | 27.4 | |
| Sabzi et al. [22] | 5D18-F25-G | 150 | 800 | 300 | 251 | 49 | 41 | 25.0 | 2.6 | 23.7 |
| 5D10-F25-G | 150 | 800 | 300 | 267 | 33 | 39 | 25.0 | 2.6 | 23.7 | |
| Sabzi et al. [23] | 2D22-NSG-G | 150 | 800 | 300 | 251 | 49 | 44 | 25.0 | 2.6 | 23.7 |
| 5D14-NSC-G | 150 | 800 | 300 | 255 | 45 | 44 | 25.0 | 2.6 | 23.7 | |
| Pham et al. [27] | E1a | 150 | 700 | 260 | 220 | 40 | 52 | 53.7 | 4.3 | 34.7 |
| Specimen | Tensile Steel Longitudinal Reinforcement | Compressive Steel Longitudinal Reinforcement | Steel Transverse Reinforcement | Geometries of FRP | Mechanical Properties of FRP | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1B | 2 | 10 | 207 | 506 | 157.1 | 207 | 506 | 157.1 | 100 | 207 | 506 | 150 | 1.77 | 271 | 3720 |
| 2B | 2 | 10 | 207 | 506 | 157.1 | 207 | 506 | 157.1 | 100 | 207 | 506 | 148 | 1.70 | 271 | 3720 |
| 3B | 2 | 10 | 207 | 506 | 157.1 | 207 | 506 | 157.1 | 100 | 207 | 506 | 147 | 1.87 | 257 | 4591 |
| 6B | 2 | 10 | 207 | 506 | 157.1 | 207 | 506 | 157.1 | 100 | 207 | 506 | 145 | 1.81 | 257 | 4591 |
| B3 | 2 | 12 | 200 | 400 | 157.1 | 200 | 365 | 100.5 | 80 | 200 | 350 | 150 | 0.35 | 237 | 2845 |
| CS-L3-B | 2 | 10 | 199 | 536 | 157.1 | 199 | 536 | 157.1 | 100 | 199 | 536 | 148 | 2.63 | 256 | 4114 |
| CS-W100-B | 2 | 10 | 199 | 536 | 157.1 | 199 | 536 | 157.1 | 100 | 199 | 536 | 100 | 1.95 | 256 | 4114 |
| CP | 2 | 10 | 199 | 536 | 157.1 | 199 | 536 | 157.1 | 100 | 199 | 536 | 148 | 1.20 | 165 | 2800 |
| 5D18-F25-G | 5 | 18 | 223 | 367 | 226.2 | 210 | 412 | 157.1 | 80 | 190 | 462 | 160 | 0.17 | 240 | 3600 |
| 5D10-F25-G | 5 | 10 | 190 | 462 | 226.2 | 210 | 412 | 100.5 | 120 | 190 | 462 | 160 | 0.17 | 240 | 3600 |
| 2D22-NSG-G | 2 | 22 | 204 | 376 | 226.2 | 210 | 412 | 100.5 | 100 | 190 | 462 | 160 | 0.17 | 240 | 4950 |
| 5D14-NSC-G | 5 | 14 | 205 | 423 | 226.2 | 210 | 412 | 100.5 | 100 | 190 | 462 | 160 | 0.17 | 240 | 4950 |
| E1a | 3 | 12 | 205 | 551 | 226.2 | 205 | 551 | 157.1 | 100 | 204 | 334 | 100 | 1.06 | 209 | 3900 |
| Specimen | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 1B | 37 | 0.67 | 66.80 | 0.73 | 0.76 | 0.79 | 0.83 | 0.90 | 0.46 |
| 2B | 51 | 0.47 | 57.60 | 1.01 | 1.04 | 1.09 | 1.13 | 1.20 | 0.45 |
| 3B | 34 | 0.71 | 65.40 | 1.02 | 1.06 | 1.10 | 1.14 | 1.21 | 0.57 |
| 6B | 34 | 0.71 | 60.20 | 0.92 | 0.95 | 0.99 | 1.03 | 1.10 | 0.47 |
| B3 | 31 | 0.95 | 35.47 | 0.78 | 0.81 | 0.84 | 0.88 | 1.65 | 2.77 |
| CS-L3-B | 37 | 0.83 | - | - | - | - | - | - | - |
| CS-W100-B | 39 | 0.99 | - | - | - | - | - | - | - |
| CP | 42 | 0.73 | 50.70 | 0.74 | 0.77 | 0.80 | 0.83 | 1.36 | 1.91 |
| 5D18-F25-G | 42 | 0.93 | 144.50 | 1.63 | 1.69 | 1.76 | 1.83 | 4.22 | 2.80 |
| 5D10-F25-G | 43 | 0.91 | 89.50 | 0.47 | 0.48 | 0.51 | 0.53 | 1.54 | 2.45 |
| 2D22-NSG-G | 44 | 0.89 | 116.00 | 0.68 | 0.70 | 0.73 | 0.76 | 2.35 | 3.07 |
| 5D14-NSC-G | 43 | 0.91 | 123.50 | 0.96 | 0.99 | 1.03 | 1.08 | 2.81 | 2.69 |
| E1a | 39 | 0.84 | 70.70 | 0.52 | 0.54 | 0.57 | 0.59 | 1.08 | 1.11 |
| Average | 0.81 | 0.86 | 0.89 | 0.93 | 0.97 | 1.77 | 1.71 | ||
| Standard deviation | 0.15 | 0.32 | 0.33 | 0.34 | 0.35 | 1.00 | 1.10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, B.; Wu, R.-Y.; Yin, S. Stress Field Approach for Prediction of End Concrete Cover Separation in RC Beams Strengthened with FRP Reinforcement. Polymers 2022, 14, 988. https://doi.org/10.3390/polym14050988
Zhou B, Wu R-Y, Yin S. Stress Field Approach for Prediction of End Concrete Cover Separation in RC Beams Strengthened with FRP Reinforcement. Polymers. 2022; 14(5):988. https://doi.org/10.3390/polym14050988
Chicago/Turabian StyleZhou, Binbin, Ruo-Yang Wu, and Shiping Yin. 2022. "Stress Field Approach for Prediction of End Concrete Cover Separation in RC Beams Strengthened with FRP Reinforcement" Polymers 14, no. 5: 988. https://doi.org/10.3390/polym14050988
APA StyleZhou, B., Wu, R.-Y., & Yin, S. (2022). Stress Field Approach for Prediction of End Concrete Cover Separation in RC Beams Strengthened with FRP Reinforcement. Polymers, 14(5), 988. https://doi.org/10.3390/polym14050988