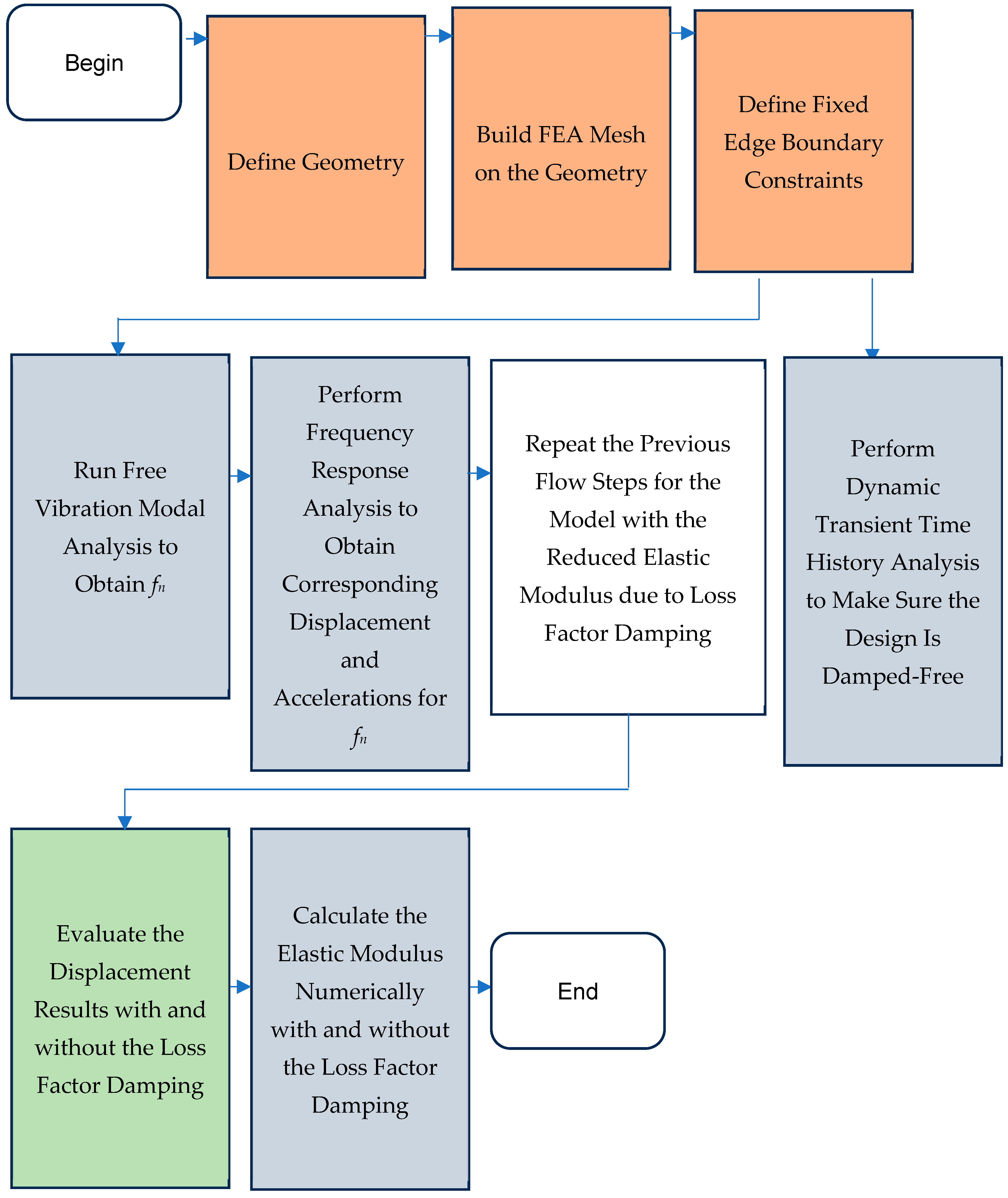
Proof of Concept for Determination of Static–Dynamic Material Loss Factor Damping via Simulation and Numerical Methods
Abstract
1. Introduction
2. Validation of the Elastic Modulus and Loss Factor Damping per the ASTM Standards
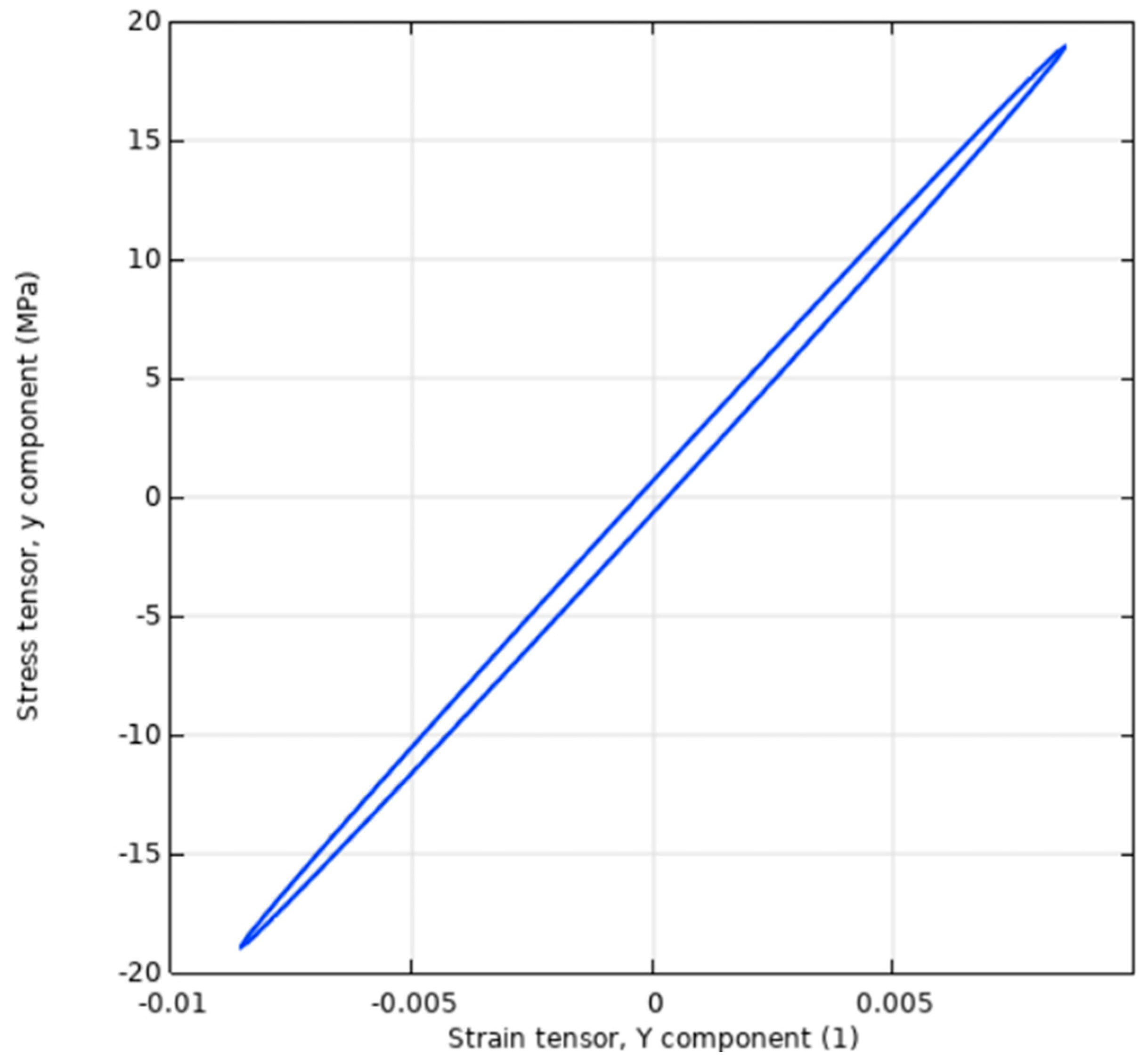
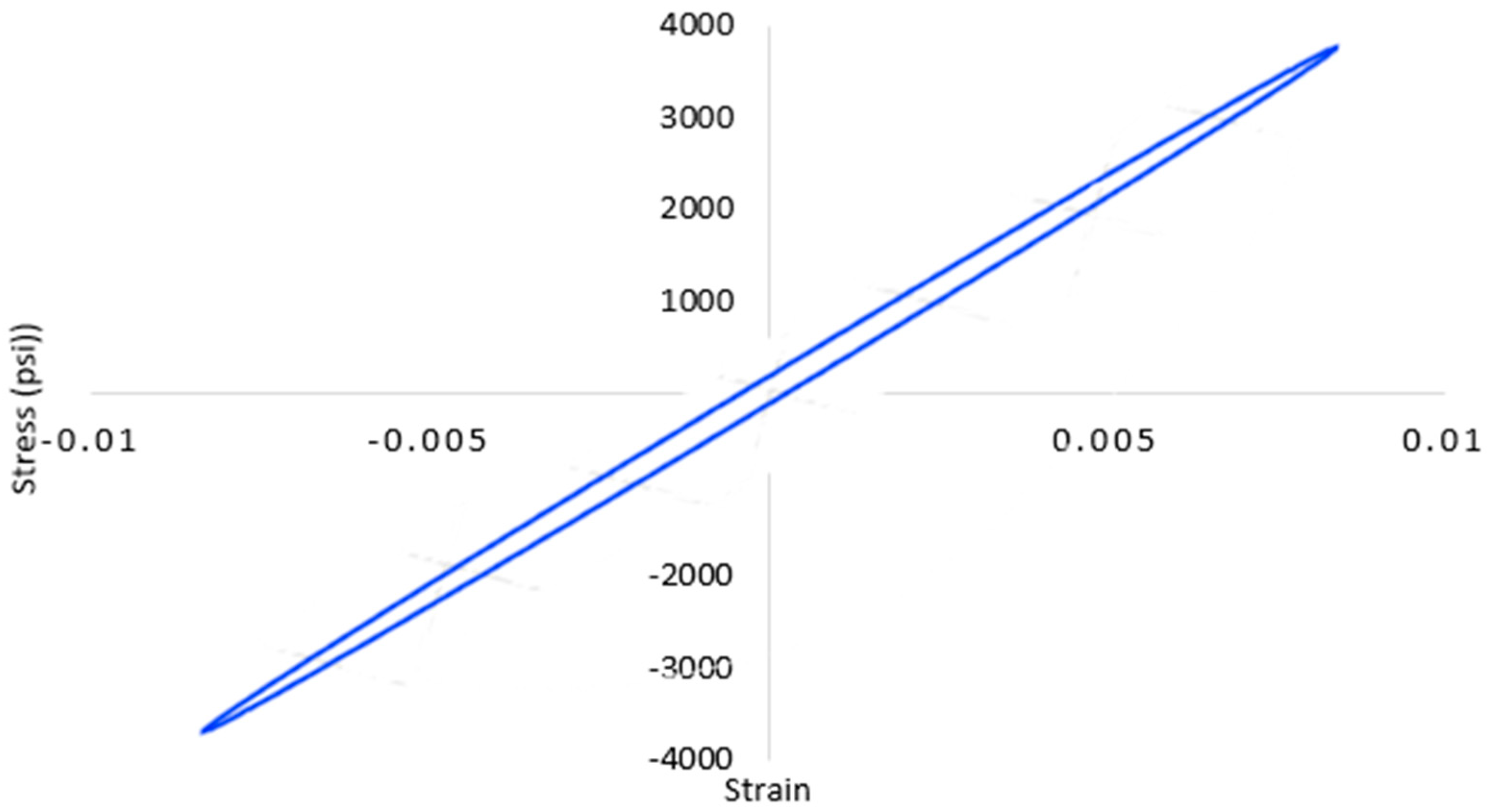
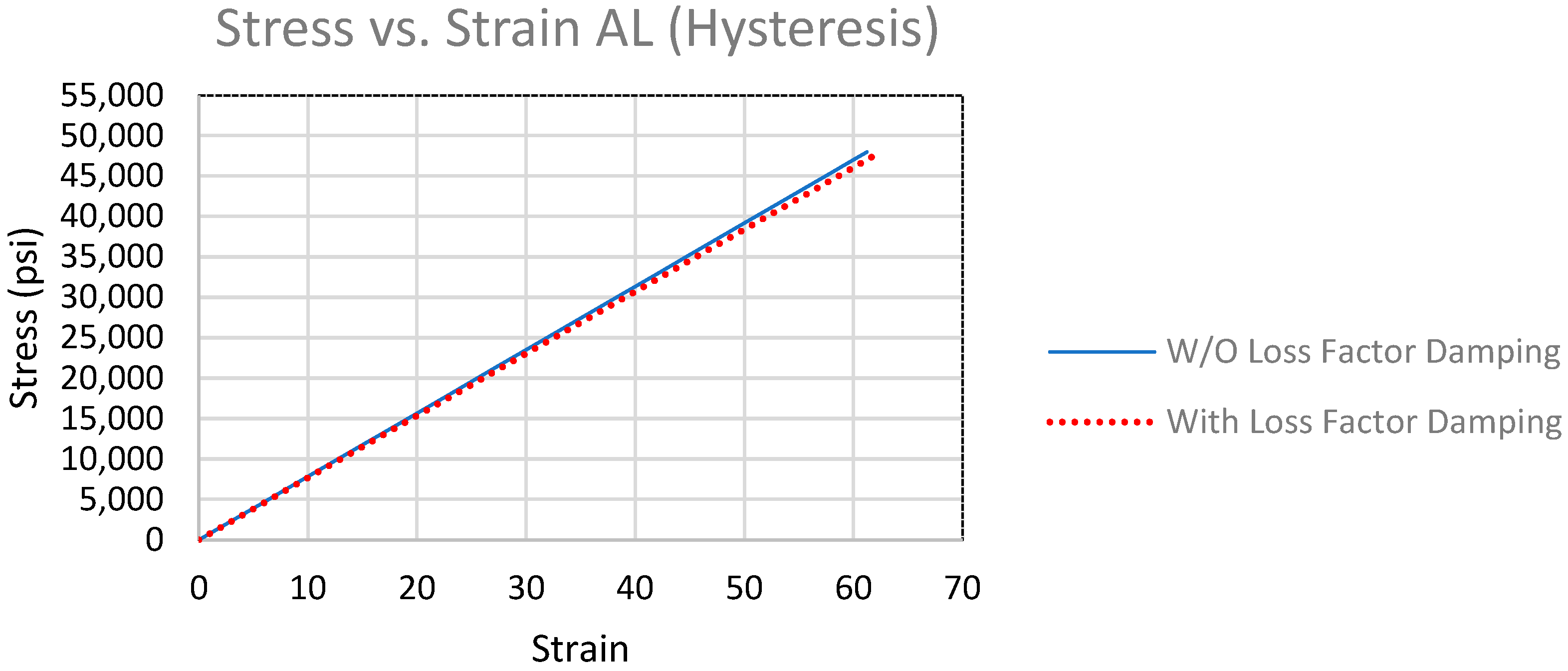
3. Numerical Case (Static Hysteresis)
4. Frequency Response and Time Analysis for Loss Factor Damping
5. Fatigue Aspects of the Loss Factor Damping
6. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| c | viscous damping |
| C | specific heat |
| Cn | coefficient associated with mode n |
| d | diameter of the circular plate (2r) |
| D | stiffness of the plate |
| E | elastic modulus |
| Ed | elastic modulus due to loss factor damping |
| E′ | storage modulus |
| E″ | difference in elastic modulus |
| f | fatigue frequency |
| fn | resonance frequency, n mode |
| G | shear modulus |
| h | coefficient of heat transfer |
| H | plate thickness |
| k | spring stiffness |
| l | beam length |
| m | mass |
| r | radius of the circular plate |
| v | Poisson’s ratio |
| t | time |
| T | temperature |
| Tamb | ambient temperature |
| wd | dissipation energy |
| d | loss angle |
| e | strain |
| ρ | density |
| h | loss factor damping |
| σ | stress |
| wn | angular natural frequency, n-mode |
| z | coefficient of viscous damping factor |
Appendix A
| Material | Loss Factor |
|---|---|
| Aluminum | 0.0001–0.02 |
| Concrete | 0.02–0.05 |
| Copper | 0.001-0.05 |
| Glass | 0.0001–0.005 |
| Rubber | 0.05–2 |
| Steel | 0.0001–0.01 |
References
- Carden, E.P.; Fanning, P. Vibration based condition monitoring: A review. Struct. Health Monit. 2004, 3, 355–377. [Google Scholar] [CrossRef]
- Yan, Y.J.; Cheng, L.; Wu, Z.Y.; Yam, L.H. Development in vibration-based structural damage detection technique. Mech. Syst. Signal Process. 2007, 21, 2198–2211. [Google Scholar] [CrossRef]
- Fan, W.; Qiao, P. Vibration-based damage identification methods: A review and comparative study. Struct. Health Monit. 2011, 10, 83–111. [Google Scholar] [CrossRef]
- Salawu, O.S. Detection of structural damage through changes in frequency: A review. Eng. Struct. 1997, 19, 718–723. [Google Scholar] [CrossRef]
- Sapri, R.C. Mechanical Vibrations: Measurement, Effects and Control; Nova Science Publishers, Inc.: New York, NY, USA, 2009; pp. 643–702, INBS 978 1-60692-037-4. [Google Scholar]
- Zou, Y.; Tong, L.; Steven, G.P. Vibration-based model dependent damage (delamination) identification and health monitoring for composite structures—A review. J. Sound Vib. 2000, 230, 357–378. [Google Scholar] [CrossRef]
- Chang, P.C.; Flatau, A.; Liu, S.C. Review paper: Health monitoring of civil structures. Int. J. Struct. Health Monit. 2003, 2, 257–267. [Google Scholar] [CrossRef]
- Montalvao, D.; Maia, N.M.M.; Ribeiro, A.M.R. A review of vibration-based structural health monitoring with special emphasis on composite materials. Shock Vib. Dig. 2006, 38, 295–326. [Google Scholar] [CrossRef]
- Pandey, A.K.; Biswas, M.; Samman, M.M. Damage detection from changes in curvature mode shapes. J. Sound Vib. 1991, 145, 321–332. [Google Scholar] [CrossRef]
- Zhang, J.; Jin, Z.; Teng, S.; Chen, G.; Bassir, D. Structural damage detection based on decision-level fusion with multi-vibration signals. Meas. Sci. Technol. 2022, 33, 105112. [Google Scholar] [CrossRef]
- Cao, M.; Xu, W.; Ostachowicz, W.; Su, Z. Damage identification for beams in noisy conditions based on Teager energy operator-wavelet transform modal curvature. J. Sound Vib. 2014, 333, 1543–1553. [Google Scholar] [CrossRef]
- Xu, W.; Cao, M.; Ostachowicz, W.; Radzieński, M.; Xia, N. Two-dimensional curvature mode shape method based on wavelets and Teager energy for damage detection in plates. J. Sound Vib. 2015, 347, 266–278. [Google Scholar] [CrossRef]
- Cao, M.; Radzieński, M.; Xu, W.; Ostachowicz, W. Identification of multiple damage in beams based on robust curvature mode shapes. Mech. Syst. Signal Process. 2014, 46, 468–480. [Google Scholar] [CrossRef]
- Cao, M.S.; Xu, W.; Ren, W.X.; Ostachowicz, W.; Sha, G.G.; Pan, L.X. A concept of complex-wavelet modal curvature for detecting multiple cracks in beams under noisy conditions. Mech. Syst. Signal Process. 2016, 76, 555–575. [Google Scholar] [CrossRef]
- Farrar, C.R.; Jauregui, D.A. Comparative study of damage identification algorithms applied to a bridge: I. Experiment. Smart Mater. Struct. 1998, 7, 704–719. [Google Scholar] [CrossRef]
- Baruch, M.; Ratan, S. Damage Detetction in Flexible Strctures. J. Sound Vibrtion 1993, 166, 21–30. [Google Scholar] [CrossRef]
- Dammika, A.J.; Kawarai, K.; Yamaguchi, H.; Matsumoto, Y.; Yoshioka, T. Experimental-analytical framework for damping change-based structural health monitoring of bridges. In Research Report of Department of Civil and Environmental Engineering; Saitama University: Saitama, Japan, 2015; Volume 41. [Google Scholar]
- Nashif, A.D.; Jones, D.I.; Henderson, J.P. Vibration Damping; Wiley: New York, NY, USA, 1985. [Google Scholar]
- Beards, C. Structural Vibration: Analysis and Damping; Arnold: London, UK, 1996. [Google Scholar]
- Jeary, A.P. Damping in structures. J. Wind Eng. Ind. Aerodyn. 2017, 72, 345–355. [Google Scholar] [CrossRef]
- Slavič, J.; Simonovski, I.; Boltežar, M. Damping identification using a continuous wavelet transform: Application to real data. J. Sound Vib. 2003, 262, 291–307. [Google Scholar] [CrossRef]
- Zhang, H.; Yang, J.; Xiao, L. Damping ratio identification using a continuous wavelet transform to vortex-induced motion of a Truss Spar. Ships Offshore Struct. 2014, 9, 596–604. [Google Scholar] [CrossRef]
- Schaller, C. Polymer Chemstry; Saint John’s University, LibreTexts: 2024. Available online: https://chem.libretexts.org/Bookshelves/Organic_Chemistry/Polymer_Chemistry_(Schaller) (accessed on 1 September 2024).
- Sonnerlind, H. Damping in Structural Dynamics: Theory and Sources; COMSOL: Stockholm, Sweden, 2019. [Google Scholar]
- Liu, X.; Feng, Q. Statistical energy analysis of tire/road noise. In Automotive Tire Noise and Vibrations; Butterworth-Heinemann Publishing: Oxford, UK, 2020. [Google Scholar]
- Pereira, R.; Arenas, J.P.; Zumelzu, E. Comparison of four test methods to measure damping properties of materials by using piezoelectric transducers. J. Mater. Des. 2011, 32, 2423–2428. [Google Scholar] [CrossRef]
- ASTM E756-04; Standard Test Method for Measuring Vibration-Damping Properties of Materials. American Society for Testing and Materials: West Conshohocken, PA, USA, 2004.
- Kelly, P.A. Mechanics. Lecture Notes: An Introduction to Solid Mechanics. 2024. Available online: http://homepages.engineering.auckland.ac.nz/~pkel015/SolidMechanicsBooks/index.html (accessed on 1 September 2024).
- Khoshmanesh, S.; Watson, S.J.; Zarouchas, D. The effect of the fatigue damage accumulation process on the damping and stiffness properties of adhesively bonded composite structures. J. Compos. Struct. 2022, 287, 115328. [Google Scholar] [CrossRef]
- Incropera, F.P.; DeWitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer; Wiley: New York, NY, USA, 1996; Volume 6. [Google Scholar]
- Radziemska, E.; Lewandowski, W.M. Experimental Investigations of Natural Convection from Circular Plates at Variable Inclination. J. Thermophys. Heat Transf. 2007, 21, 813–816. [Google Scholar] [CrossRef]


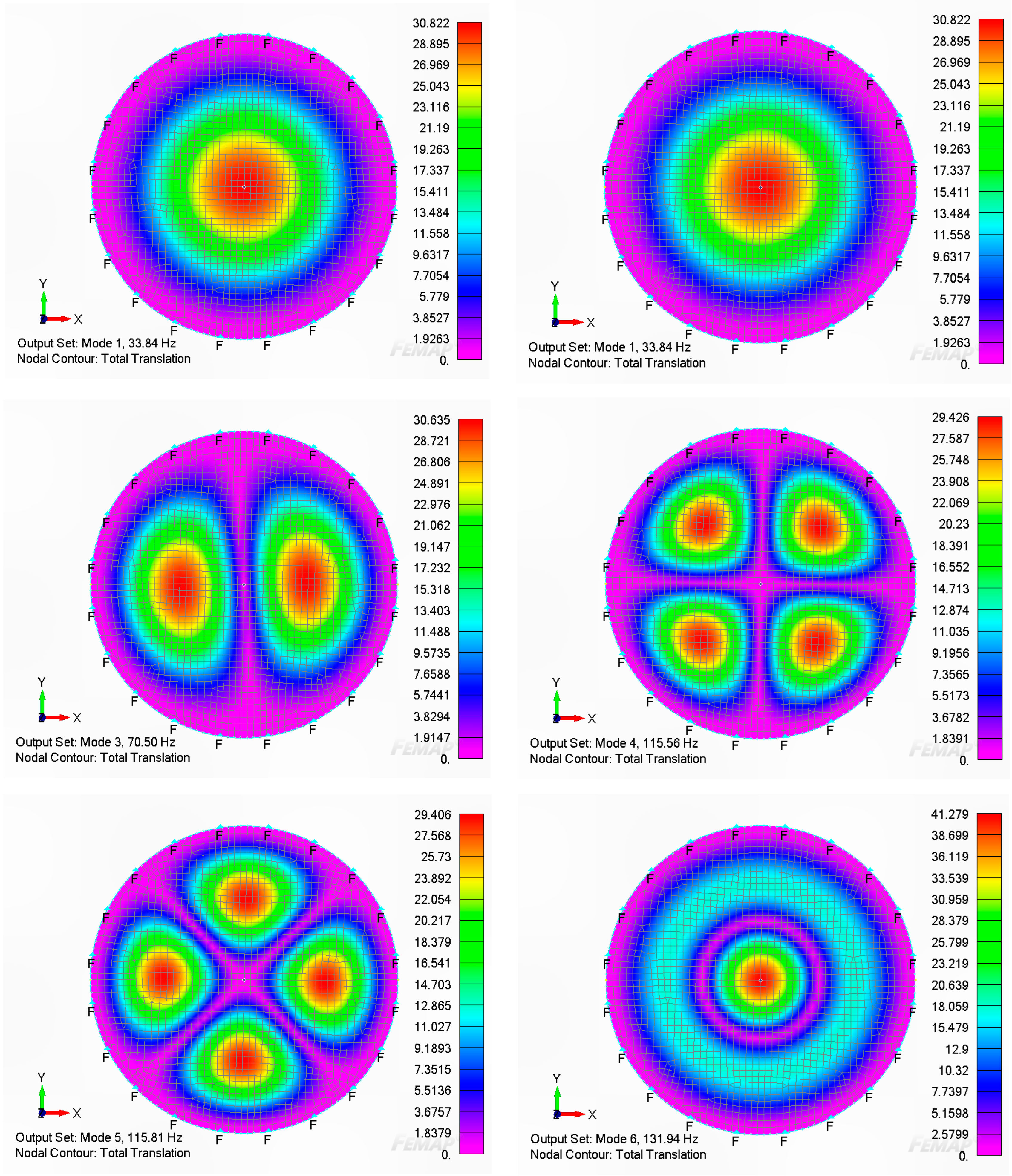
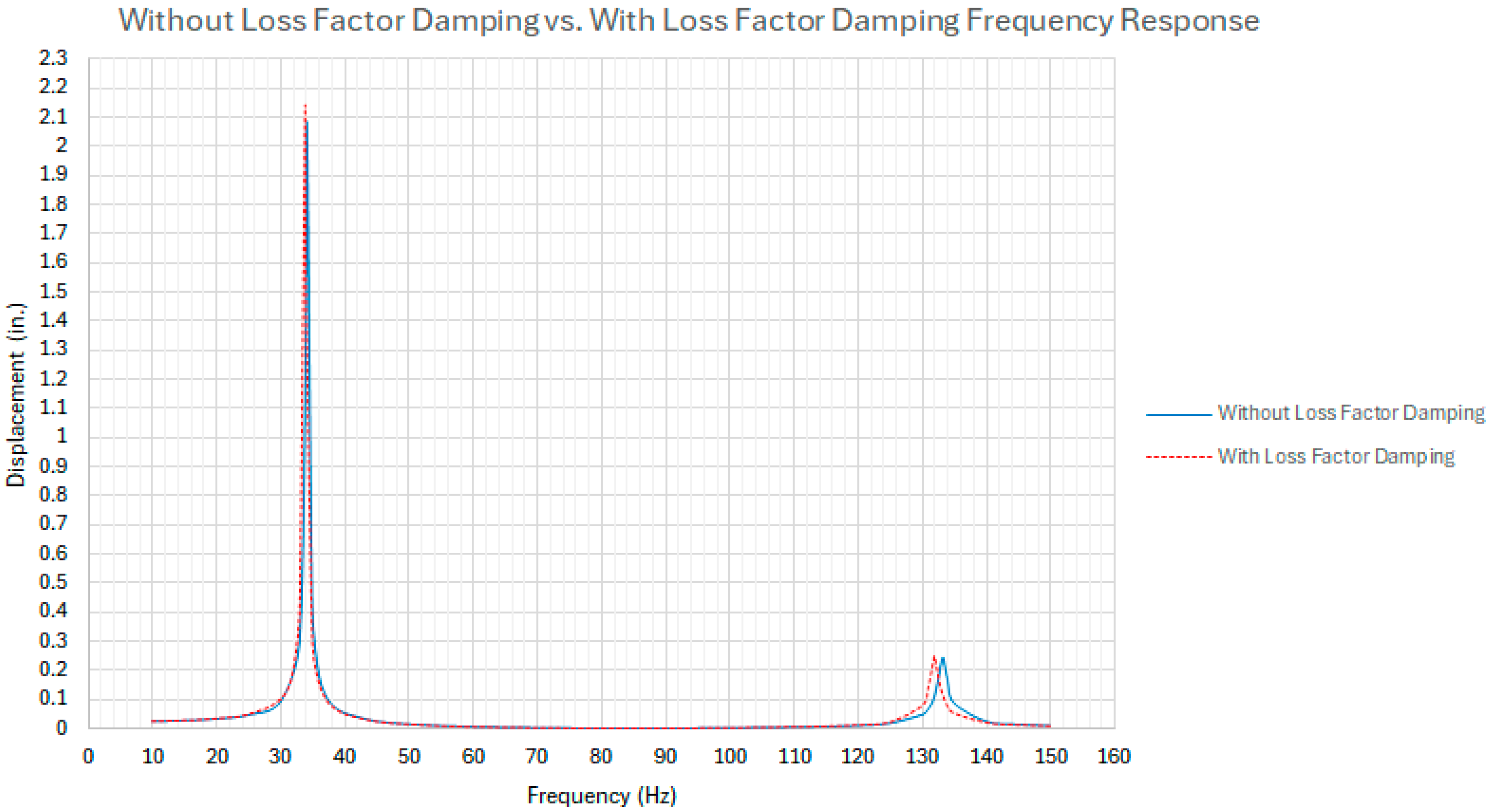

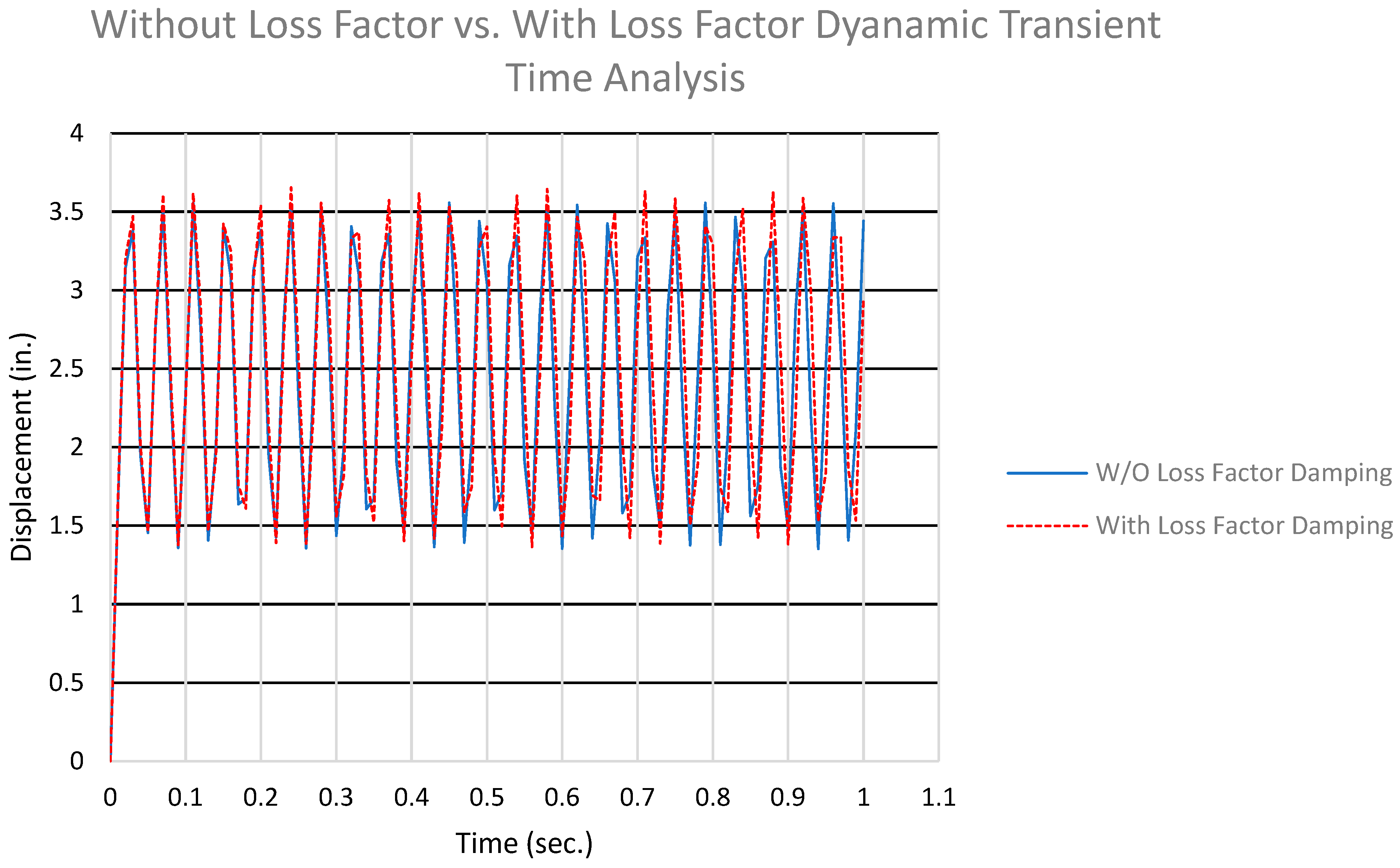

| Resonance | Without Loss Factor Damping | With Loss Factor Damping | ||
|---|---|---|---|---|
| Frequency (Hz) | Displacement (in.) | Frequency (Hz) | Displacement (in.) | |
| 1 | 34.19 | 2.0608 | 33.84 | 2.1028 |
| 2 | 71.19 | 0.0027 | 70.48 | 0.0028 |
| 3 | 71.22 | 0.0027 | 70.50 | 0.0028 |
| 4 | 116.74 | 0.0085 | 115.57 | 0.0087 |
| 5 | 116.99 | 0.0087 | 115.81 | 0.0089 |
| 6 | 133.28 | 0.2438 | 131.94 | 0.2488 |
| Resonance | Without Loss Factor Damping | With Loss Factor Damping | ||
|---|---|---|---|---|
| Frequency (Hz) | Accel (in./sec2) | Frequency (Hz) | Accel (in./sec2) | |
| 1 | 34.19 | 95,111.72 | 33.84 | 95,111.52 |
| 2 | 71.19 | 553.91 | 70.48 | 553.91 |
| 3 | 71.22 | 553.09 | 70.5 | 553.10 |
| 4 | 116.74 | 4589.19 | 115.57 | 4589.23 |
| 5 | 116.99 | 4693.00 | 115.81 | 4692.98 |
| 6 | 133.28 | 170,971.48 | 131.94 | 170,971.27 |
| Resonance | Without Loss Factor Damping | With Loss Factor Damping | ||
|---|---|---|---|---|
| Frequency (Hz) | Elastic Modulus (psi) | Frequency (Hz) | Elastic Modulus (psi) | |
| 1 | 34.19 | 10,000,000 | 33.84 | 9,799,000 |
| 2 | 71.19 | 10,000,000 | 70.48 | 9,800,000 |
| 3 | 71.22 | 10,000,000 | 70.50 | 9,800,000 |
| 4 | 116.74 | 10,000,000 | 115.57 | 9,800,000 |
| 5 | 116.99 | 10,000,000 | 115.81 | 9,799,000 |
| 6 | 133.28 | 10,000,000 | 131.94 | 9,799,000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Javidinejad, A. Proof of Concept for Determination of Static–Dynamic Material Loss Factor Damping via Simulation and Numerical Methods. J. Exp. Theor. Anal. 2025, 3, 9. https://doi.org/10.3390/jeta3010009
Javidinejad A. Proof of Concept for Determination of Static–Dynamic Material Loss Factor Damping via Simulation and Numerical Methods. Journal of Experimental and Theoretical Analyses. 2025; 3(1):9. https://doi.org/10.3390/jeta3010009
Chicago/Turabian StyleJavidinejad, Amir. 2025. "Proof of Concept for Determination of Static–Dynamic Material Loss Factor Damping via Simulation and Numerical Methods" Journal of Experimental and Theoretical Analyses 3, no. 1: 9. https://doi.org/10.3390/jeta3010009
APA StyleJavidinejad, A. (2025). Proof of Concept for Determination of Static–Dynamic Material Loss Factor Damping via Simulation and Numerical Methods. Journal of Experimental and Theoretical Analyses, 3(1), 9. https://doi.org/10.3390/jeta3010009





