The Odd Beta Prime-G Family of Probability Distributions: Properties and Applications to Engineering and Environmental Data †
Abstract
:1. Introduction
2. Linear Representations
3. Statistical Properties
3.1. Moments
3.2. Moment-Generating Function
3.3. Entropy
4. The Odd Beta Prime Exponential Distribution
5. Applications
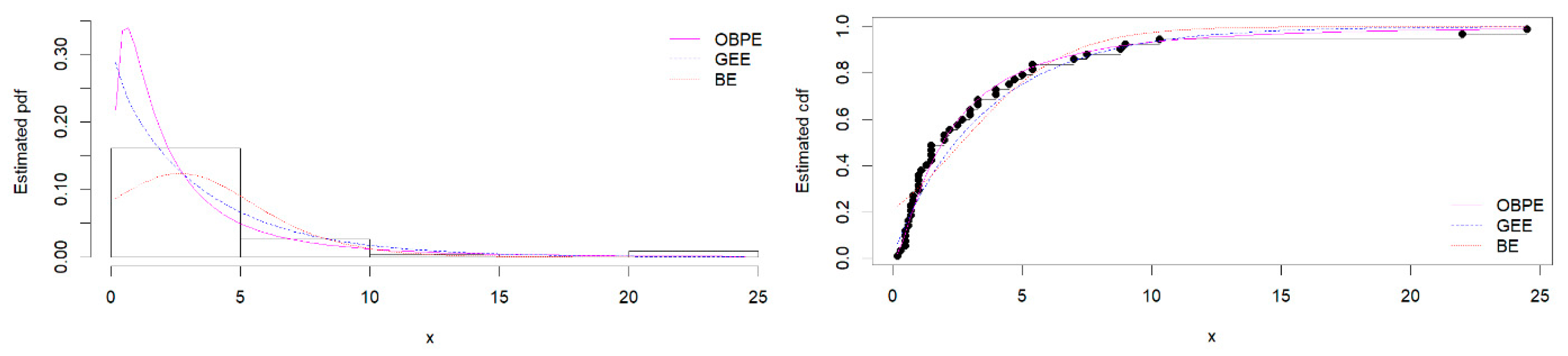
5.1. The Airborne Communications Transceiver Data
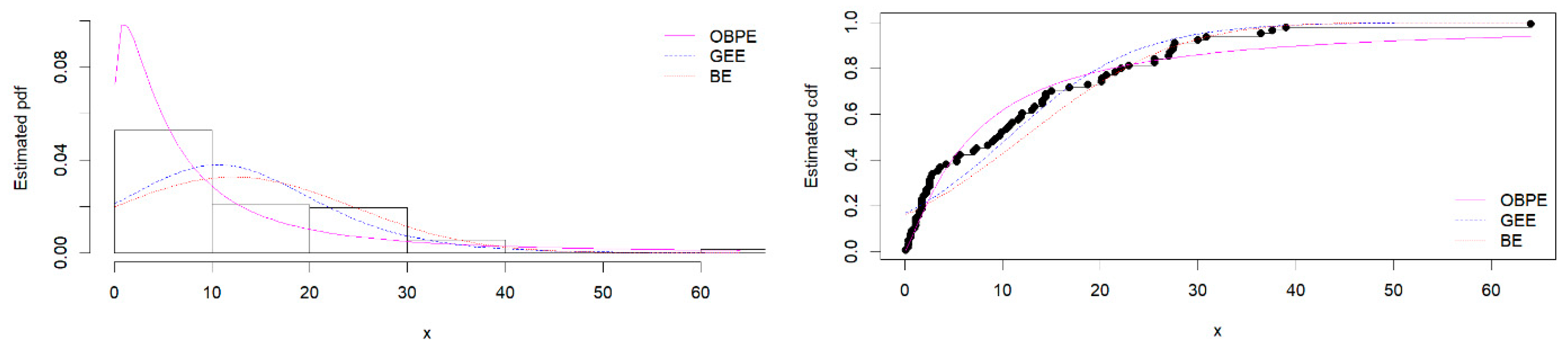
5.2. Exceedances of Wheaton River Flood Data
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Alzaatreh, A.; Lee, C.; Famoye, F. A new method for generating families of continuous distributions. Metron 2013, 71, 63–79. [Google Scholar] [CrossRef]
- Aljarrah, M.A.; Lee, C.; Famoye, F. On generating TX family of distributions using quantile functions. J. Stat. Distrib. Appl. 2014, 1, 1–17. [Google Scholar] [CrossRef]
- Eugene, N.; Lee, C.; Famoye, F. Beta-normal distribution and its applications. Commun. Stat.-Theory Methods 2002, 31, 497–512. [Google Scholar] [CrossRef]
- Cordeiro, G.M.; de Castro, M. A new family of generalized distributions. J. Stat. Comput. Simul. 2011, 81, 883–898. [Google Scholar] [CrossRef]
- Torabi, H.; Montazeri, N.H. The logistic-uniform distribution and its applications. Commun. Stat.-Simul. Comput. 2014, 43, 2551–2569. [Google Scholar] [CrossRef]
- Bourguignon, M.; Silva, R.B.; Cordeiro, G.M. The Weibull-G family of probability distributions. J. Data Sci. 2014, 12, 53–68. [Google Scholar] [CrossRef]
- Afify, A.Z.; Cordeiro, G.M.; Maed, M.E.; Alizadeh, M.; Al-Mofleh, H.; Nofal, Z.M. The generalized odd Lindley-G family: Properties and applications. An. Acad. Bras. Ciências 2019, 91, e20180040. [Google Scholar] [CrossRef] [PubMed]
- Ishaq, A.I.; Abiodun, A.A. The Maxwell–Weibull distribution in modeling lifetime datasets. Ann. Data Sci. 2020, 7, 639–662. [Google Scholar] [CrossRef]
- Rényi, A. On measures of entropy and information. In Proceedings of the Fourth Berkeley Symposium on Mathematical Statistics and Probability, Berkeley, CA, USA, 20 June–30 July 1961; Volume 1, pp. 547–561. [Google Scholar]
- Refaie, M.K. Burr X exponentiated exponential distribution. J. Stat. Appl. 2018, 1, 71–88. [Google Scholar]
- Ristić, M.M.; Balakrishnan, N. The gamma-exponentiated exponential distribution. J. Stat. Comput. Simul. 2012, 82, 1191–1206. [Google Scholar] [CrossRef]
- Nadarajah, S.; Kotz, S. The beta exponential distribution. Reliab. Eng. Syst. Saf. 2006, 91, 689–697. [Google Scholar] [CrossRef]
- Singh, V.V.; Suleman, A.A.; Ibrahim, A.; Abdullahi, U.A.; Suleiman, S.A. Assessment of probability distributions of groundwater quality data in Gwale area, north-western Nigeria. Ann. Optim. Theory Pract. 2020, 3, 37–46. [Google Scholar]
- Abdullahi, U.A.; Suleiman, A.A.; Ishaq, A.I.; Usman, A.; Suleiman, A. The Maxwell–Exponential Distribution: Theory and Application to Lifetime Data. J. Stat. Model. Anal. 2021, 3, 65–80. [Google Scholar] [CrossRef]
- Choulakian, V.; Stephens, M.A. Goodness-of-fit tests for the generalized Pareto distribution. Technometrics 2001, 43, 478–484. [Google Scholar] [CrossRef]
| Distribution | MLE and SE in () | AIC | BIC | CM | AD | |||
|---|---|---|---|---|---|---|---|---|
| OBPE | c = 0.6583 (0.1625) | d = 1.1018 (0.1149) | b = 1.4863 (4.5831) | 100.0163 | 204.032 | 207.689 | 0.054 | 0.337 |
| GEE | α = 0.9323 (0.1701) | θ = 0.2585 (0.0615) | λ = 0.3685 (0.7650) | 104.9309 | 213.861 | 217.519 | 0.175 | 1.103 |
| BE | a = 2.6732 (0.4920) | b = 2.0190 (0.2613) | λ = 1.1455 (0.4016) | 128.48 | 260.960 | 264.617 | 0.409 | 3.007 |
| Distribution | MLE and SE in () | AIC | BIC | CM | AD | |||
|---|---|---|---|---|---|---|---|---|
| OBPE | c = 1.2126 (0.1166) | d = 6.7584 (1.1659) | b = 1.6077 (0.3743) | 257.839 | 519.6782 | 524.231 | 0.232 | 1.472 |
| GEE | α = 10.6378 (1.3609) | θ = 6.6058 (0.6475) | λ = 2.9650 (0.4475) | 279.958 | 563.9169 | 568.470 | 0.330 | 2.478 |
| BE | a = 12.2041 (1.4391) | b = 12.2115 (1.0176) | λ = 5.1753 (0.2352) | 282.334 | 568.669 | 573.223 | 0.469 | 2.891 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suleiman, A.A.; Daud, H.; Othman, M.; Ishaq, A.I.; Indawati, R.; Abdullah, M.L.; Husin, A. The Odd Beta Prime-G Family of Probability Distributions: Properties and Applications to Engineering and Environmental Data. Comput. Sci. Math. Forum 2023, 7, 20. https://doi.org/10.3390/IOCMA2023-14429
Suleiman AA, Daud H, Othman M, Ishaq AI, Indawati R, Abdullah ML, Husin A. The Odd Beta Prime-G Family of Probability Distributions: Properties and Applications to Engineering and Environmental Data. Computer Sciences & Mathematics Forum. 2023; 7(1):20. https://doi.org/10.3390/IOCMA2023-14429
Chicago/Turabian StyleSuleiman, Ahmad Abubakar, Hanita Daud, Mahmod Othman, Aliyu Ismail Ishaq, Rachmah Indawati, Mohd Lazim Abdullah, and Abdullah Husin. 2023. "The Odd Beta Prime-G Family of Probability Distributions: Properties and Applications to Engineering and Environmental Data" Computer Sciences & Mathematics Forum 7, no. 1: 20. https://doi.org/10.3390/IOCMA2023-14429
APA StyleSuleiman, A. A., Daud, H., Othman, M., Ishaq, A. I., Indawati, R., Abdullah, M. L., & Husin, A. (2023). The Odd Beta Prime-G Family of Probability Distributions: Properties and Applications to Engineering and Environmental Data. Computer Sciences & Mathematics Forum, 7(1), 20. https://doi.org/10.3390/IOCMA2023-14429







