Possible Tests of Fundamental Physics with GINGER

Abstract

The GINGER (gyroscopes in general relativity) project foresees the construction of an array of large frame ring laser gyroscopes, rigidly connected to the Earth. Large frame ring laser gyroscopes are high-sensitivity instruments used to measure angular velocity with respect to the local inertial frame. In particular, they can provide sub-daily variations in the Earth rotation rate, a measurement relevant for geodesy and for fundamental physics at the same time. Sensitivity is the key point in determining the relevance of this instrument for fundamental science. The most recent progress in sensitivity evaluation, obtained on a ring laser prototype, indicates that GINGER should reach the level of 1 part in ${10}^{11}$ of the Earth’s rotation rate. The impact on fundamental physics of this kind of apparatus is reviewed.

1. Introduction

It has been always true that observation of reality with a ‘magnifying glass’ provides new information. The key point is how great the magnification is or, in other words, what is the maximum sensitivity achievable by the experimental apparatus. Sagnac interferometers are commonly used to measure inertial angular velocity, in particular the angular rotation of the Earth [1]. The Sagnac effect largely dominates all other effects and it is generally exploited for inertial navigation [2,3]. The active large frame Sagnac interferometer, “Ring Laser Gyroscope” (RLG) is by far the most sensitive instrument used to measure angular velocity. A sensitivity of the order of prad/s with a large dynamic range has been extensively demonstrated in long-term continuous operation [4,5].

• The RLG is a specific kind of interferometer built as a closed path optical cavity, usually defined by four mirrors located at the vertices of a square: two counter-propagating laser beams are excited inside the cavity. Interference of the beams transmitted by each mirror gives information on the non-reciprocal effects experienced by the two counter-propagating beams caused by the geometry or the laser dynamics. Since the interferometer has two equal paths, the differences due to such non-reciprocity effects are extremely small. However, there are other non-reciprocal effects related to the spacetime structure or to fundamental asymmetries, which make RLGs suitable for fundamental physics investigations.

2. Ring Laser

In general, a large frame RLG has a square optical cavity, with a side length above 3–4 m, and it operates rigidly attached to the ground. Figure 1 shows the general scheme of our RLG prototypes, based on the simple mechanical design of a four-mirror optical cavity under vacuum. In general, the cavity is attached to a granite monument to make it rigid and suitably oriented with respect to the rotation axis of the Earth.

An RLG senses the component of the angular velocity vector $\overrightarrow{\Omega }$ along the axis of the closed polygonal cavity, defined by the area vector. The relationship between the Sagnac frequency ${\omega }_s$ and the angular rotation rate $\Omega$ reads

$${\omega }_s=4\frac{A}{\lambda L}\Omega cos\theta ,$$

(1)

where A is the area enclosed by the optical path, L is its perimeter, $\lambda$ is the wavelength of the light, and $\theta$ is the angle between the area vector $\overrightarrow{A}$ and $\overrightarrow{\Omega }$. Equation (1) can be interpreted as the scalar product between $\overrightarrow{A}$ and $\overrightarrow{\Omega }$. In general, $\overrightarrow{\Omega }$ is the sum of several different components, the major ones being

$$\overrightarrow{\Omega }={\overrightarrow{\Omega }}_{\oplus }+{\overrightarrow{\Omega }}_{loc}+{\overrightarrow{\Omega }}_{dS}+{\overrightarrow{\Omega }}_{LT}+{\overrightarrow{\Omega }}_I$$

(2)

where ${\overrightarrow{\Omega }}_{\oplus }$ indicates the Earth rotation rate with all known contributions from tides and polar motions; ${\overrightarrow{\Omega }}_{dS}$ and ${\overrightarrow{\Omega }}_{LT}$ are the relativistic terms (subscripts stand for de Sitter (dS) and Lense–Thirring (LT), respectively); ${\overrightarrow{\Omega }}_{loc}$ indicates local deformations [6]; and ${\overrightarrow{\Omega }}_I$ are effects associated with any spurious rotation of the apparatus due to external perturbations.

Typically, the amplitude of ${\overrightarrow{\Omega }}_{\oplus }$ is higher than the other terms by more than 8 orders of magnitude. The Thomas precession also makes a similar contribution: while its amplitude is certainly below the components in Equation (2), the Thomas precession is worth mentioning, since it may provide evidence of new symmetries.

The GINGER project [7] foresees three RLGs attached to the Earth’s crust; however, in this first stage only two of them will be built. The main objective of the instrument is to reconstruct the total angular velocity vector $\overrightarrow{\Omega }$, which, as shown in Equation (2), contains, besides the kinematic term ${\overrightarrow{\Omega }}_{\oplus }$, contributions due to gravity; in particular, de Sitter and Lense–Thirring effects are seen by an RLG as two very small angular rotation vectors in the meridian plane [8,9]. As we will discuss in the following, the angular velocity vector, in principle, contains effects due to gravitational theories other than general relativity (GR), such as those related to Lorentz violation in the framework of standard model extension (SME). The kinematic local and global contributions are derived from geophysics and geodesy, as polar motion and tides [10], and, thanks to the very high sensitivity of RLGs, the sub-daily component of the length of day (LOD) is expected to be measurable. These kinematic terms are continuously monitored by the International Earth Rotation System (IERS) [11,12] with very high accuracy; therefore, gravitational theories can be tested by comparing the independent measurements of RLGs and IERS.

The effectiveness of GINGER for fundamental physics investigations depends on its sensitivity, which quite often is expressed as relative precision in the measurement of the Earth angular rotation rate. It can be said that an accuracy of 1 part in ${10}^9$ is the target to be meaningful for fundamental physics. At present, 1 part in ${10}^{11}$ seems feasible for GINGER [8,13,14,15]. The experimental setup plays an important role, since the response depends on the geometry, and it is necessary to avoid spurious rotations of the apparatus induced by environmental disturbances. The first working RLGs were based on monolithic structures made of very low thermal expansion ceramic materials, and most of the small sized gyroscopes were monolithic [10]. This choice is quite challenging in terms of cost and space. A heterolytic cavity is composed of different mechanical components, whose relative orientation can be modified. Therefore, rigidity and geometrical stability must be ensured by using active control with piezoelectric actuators (PZT). The most recent large frame RLGs have been heterolytic [16,17,18]. Two prototypes have been built and extensively studied by our group: GINGERINO [19], placed in the Gran Sasso underground INFN laboratories, and GP2 [20], located in Pisa INFN laboratories. Our experimental work has indicated that GINGER can be realized in a heterolytic structure and that an underground location is particularly recommended, since it takes advantage of the natural thermal stability and of reduced environmental noise. Unattended continuous operation for months, a typical sub-prad/s sensitivity in 1 s of measurement time, large bandwidth, and fast response, in principle as fast as milliseconds, have been proven in the experiments carried out so far.

Sensitivity is therefore a key point. The limiting noise is determined by the shot noise of the apparatus, which is a function of the cavity losses. By using the parameters of our prototype GINGERINO, the classical shot noise model [4,21] estimates 50 prad/s in a 1 s measurement. Recently, we have been able to directly evaluate the limiting noise of the GINGERINO prototype, demonstrating, thanks to a new detection scheme, that the limiting noise floor is in the prad/s Hz^−1/2 range (at frequency $<0.1$ Hz), more than a factor of 10 below the expected one [22]. This experimental result is clearly not compatible with the conventional shot noise evaluation, which assumes the two counter-propagating beams are independent and does not take into account couplings between them.In a forthcoming study, we intend to develop a model that, tracing back from the detector scheme, accounts for all the complex interdependent dynamics of the counter-propagating beams with the laser medium and the mirrors. Nonetheless, the reported experimental noise level limit suggests that a realistic final sensitivity target of GINGER should be around 1 part in ${10}^{11}$ of the Earth rotation rate [22].

So far, the sensitivity of RLG has been investigated, but it is important to remark that accuracy is also necessary, especially for GR tests, requiring that an independent measurement of the Earth rotation rate is subtracted or compared. For this purpose, it is necessary to carefully check the geometry of the ring cavity, and the laser dynamic has to be taken into account in reconstructing the Sagnac frequency. The specifications of the GINGER experiment are listed and discussed in the literature [23], where the analysis methods used to eliminate the disturbance induced by laser dynamics are illustrated, a pictorial view of GINGER is shown in Figure 2. The comparison of the GINGER data with available independently measured geodesic and geophysical signals will provide the possibility of testing the analysis procedure. GINGERINO has been a valuable test bench for GINGER, it has certainly shown the advantage of a quiet underground location, being the first non-monolithic RLG suitable for rotational seismology. It has also shown the weak points of the mechanical scheme, indicating the improvements necessary to obtain a rigid cavity, and it has allowed developing and testing the analysis procedure using real data. Some tests have already been performed by making a comparison of GINGERINO data with the GNSS antennas located on top of the Gran Sasso massif, looking for local deformation of the crust [6,7].

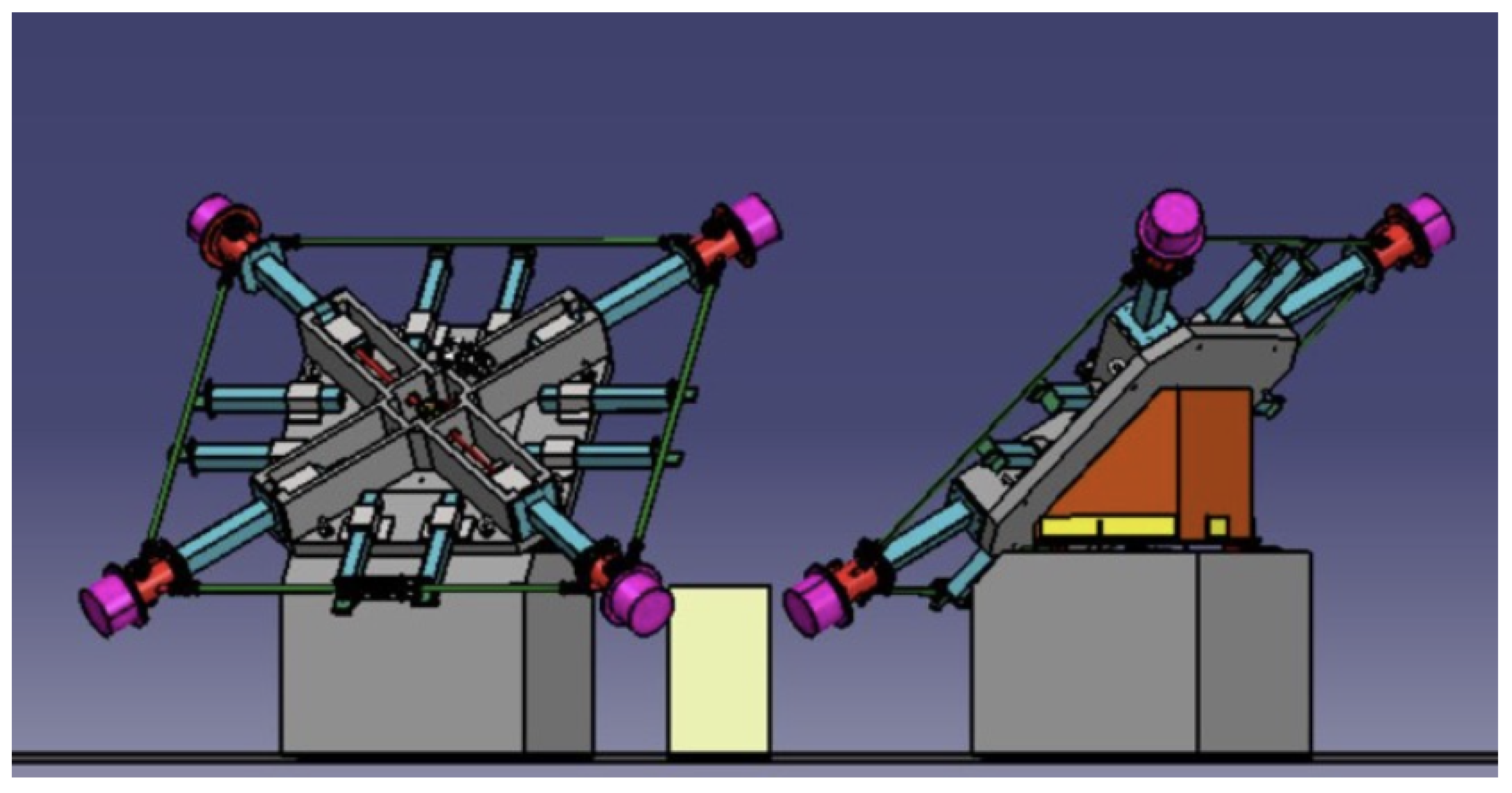
3. GINGER: Fundamental Physics Issues

It is known that general relativity currently constitutes the theory with the widest consensus in the scientific community for describing gravitational phenomena at all experimentally accessible scales. However, the theory is not perfectly adequate in describing Nature at the ultraviolet (UV) and far-infrared (IR) scales. Concerning the UV regime (small spatial scales, high-energy), there is currently no known coherent and self-consistent reformulation of GR as a standard quantum field theory (QFT). On the other hand, the formation and dynamics of cosmic structures and the evolution of the observable Universe can be described by GR only if we assume the existence of dark matter and dark energy, whose fundamental nature is currently unknown. Alternative theories have been proposed. In general, metric-affine theories are used; i.e., theories in which gravitation is described by a (Lorentzian) metric and/or by a linear connection defined on a spacetime manifold. Other theories of gravity, instead, explicitly break some basic assumptions of GR, eventually inspired by other fundamental theories, for instance Horava–Lifschitz theory and the standard model extension; in both cases, local Lorentz symmetry is broken. It is necessary to develop experiments aimed at constraining their free parameters and discarding non-viable models. An overview (although not exhaustive) of these theories that extend or modify GR is shown in Figure 3, which has been taken from a recent paper [23], where a more detailed discussion of the possible impact of GINGER in testing these theories can be found.

Measurements of gravitomagnetic effects, i.e., gravitational phenomena generated by rotating masses, can provide valuable tests. Prominent among these phenomena is the Lense–Thirring effect, which consists of the precession of the axis of test gyroscopes near spinning masses. The Lense–Thirring effect is a peculiar general relativistic effect; indeed, it cannot be described in Newtonian gravity where mass currents are not sources of the gravitational field. However, it naturally emerges in a relativistic framework and is one of the most striking manifestations of general relativity, evident even at the Earth’s surface level. A thorough discussion of the measurements of this effect can be found in the previously mentioned paper [23] and in the reviews [24,25,26,27]. The GINGER experiment provides data for the direct measurement of the Lense–Thirring effect. The major differences with data coming from space experiments [28,29,30,31] are that GINGER does not require the reconstruction of the gravitational map, as it is attached to the Earth’s surface; and being at a fixed latitude, it does not have to average the Lense–Thirring value at different latitudes, as required for satellites. In addition, violations of Lorentz symmetry can be revealed by GINGER as additional contributions to the rotation rate. Light serves as a relativistic probe for exploring both classical and quantum aspects of spacetime, and RLGs are interferometers.

Figure 3. Modified gravity roadmap summarizing the possible extensions of general relativity [32].

Figure 3. Modified gravity roadmap summarizing the possible extensions of general relativity [32].

GINGER is strategically positioned within this framework, as its anticipated sensitivity could be harnessed to achieve various scientific goals:

• The detection of effects due to spacetime curvature around the Earth (de Sitter effect) and Earth mass rotation (Lense–Thirring effect). This measurement requires comparing the IERS Earth rotation vector with the corresponding GINGER rotation vector. Testing extensions/modifications of general relativity by using PPN formalism [14,33]. Some expected measurements could be seen as upper limits; thus, any enhancement in sensitivity and accuracy may pave the way for further theoretical insights beyond general relativity in gravitational theories. The interplay between gravitomagnetism and fundamental physics tests has a large impact; recent reviews about gravitomagnetism and related theories and tests are now available [26,34].
• In principle, tests could also be performed on metric-affine theories, e.g.; teleparallel gravity [35] theories, which assume that the connection on the spacetime manifold constitutes a fundamental field variable and that is independent from the metric.
• Testing Lorentz violations described by the standard model extension (SME) [15,36]. It has been highlighted that SME terms with dimensions d = 4 and d = 5 can disrupt symmetry for counter-propagating beams in a RLG, and GINGER could significantly contribute to the quest for Lorentz violation. In this case, the signal could also be inferred by comparing GINGER with IERS data. Notably, this test is based on observations at fixed frequency rather than a DC level, so high accuracy is not imperative.
• Investigating whether fluctuations stemming from spacetime granularity could potentially exhibit observable signatures in high-frequency RLG spectra [37,38]. Intuitively, the natural length and time scales linked with spacetime quantum nature are the Planck length, and its fluctuations generate white noise, which is investigable using a frequency comb with harmonics at integer multiples of the RLG free spectral range. This point is linked more to the development of RLG, interferometers very different from the ones based on the Michelson scheme.
• Gravitational waves might excite Earth’s normal modes. Detecting such signals seems feasible theoretically, provided the sensitivity exceeds ${10}^{-16}$ rad/s. Recently, in a proposal, Marletto and Vedral highlighted the possibility of exploring, via a quantum version of the Sagnac interferometer, the quantum nature of gravity, assuming the validity of the equivalence principle in its quantum version [39].
• Sagnac corrections to time delay have been derived within the context of Horava– Lifshitz gravity [40,41], a power-counting renormalizable theory, thus considered a candidate for the UV completion of GR.
• The unification of GR and quantum mechanics remains an unresolved issue in contemporary physics. Experimental techniques in quantum optics have recently achieved the precision necessary to investigate quantum systems under the influence of non-inertial motion, such as being stationary in gravitational fields or experiencing uniform accelerations. In this context, exploring entanglement phenomena or quantum mechanics tests in non-inertial reference frames would be intriguing [37,42].
• Mechanical rotation modifies the manifestation of photon entanglement [42].
• The impact of light scalars coupled conformally and disformally to matter on the geodetic and frame-dragging has been recently evaluated [43]. This has shown that GINGER could provide measurements of $\Lambda >3.1$∼${10}^{-17}$ eV.

4. Conclusions

By the end of 2023, the executive design of GINGER will have been completed, and construction is expected to start at the end of 2024 inside the Gran Sasso laboratory. The GINGER project is co-financed by INGV and INFN as part of the the multi-components Underground Geophysics Observatory at Gran Sasso (UGGS)1 The whole experimental setup has been developed based on the experience acquired on the GINGERINO apparatus, and details of the experimental layout can be found in the literature [23]. Figure 2 shows a pictorial view of GINGER, which will be placed inside the corridor between Node A and Node B of the Gran Sasso laboratory. The first proposal was for Node B, an area larger than the corridor, so that the project would have to be re-scaled in order to fit in the new area. The plan is to build only two RLGs, named RLX and RLO, keeping GINGERINO as the third component (RLH), and with a planned perimeter of each square optical cavity of 12 m. RLX has area vector parallel to the Earth rotation axis and RLO outside the meridian plane by approximately 35 degrees; this configuration will provide a first test of the Lense–Thirring effect [9]. GINGER is intended as an interdisciplinary project, with significant expected results not only in the field of fundamental physics, but also in geodesy and in geophysics. It should give complementary information to the GNNS and VLBI networks about the length of the day and the Earth polar motion. For geophysical applications, it will provide rotational seismic information to the multi-components geophysical observatory UGGS.