Numerical Modeling and Experimental Validation of Heat Transfer Characteristics in Small PTCs with Nonevacuated Receivers
Abstract
1. Introduction
2. Materials and Methods
2.1. Description of Collector
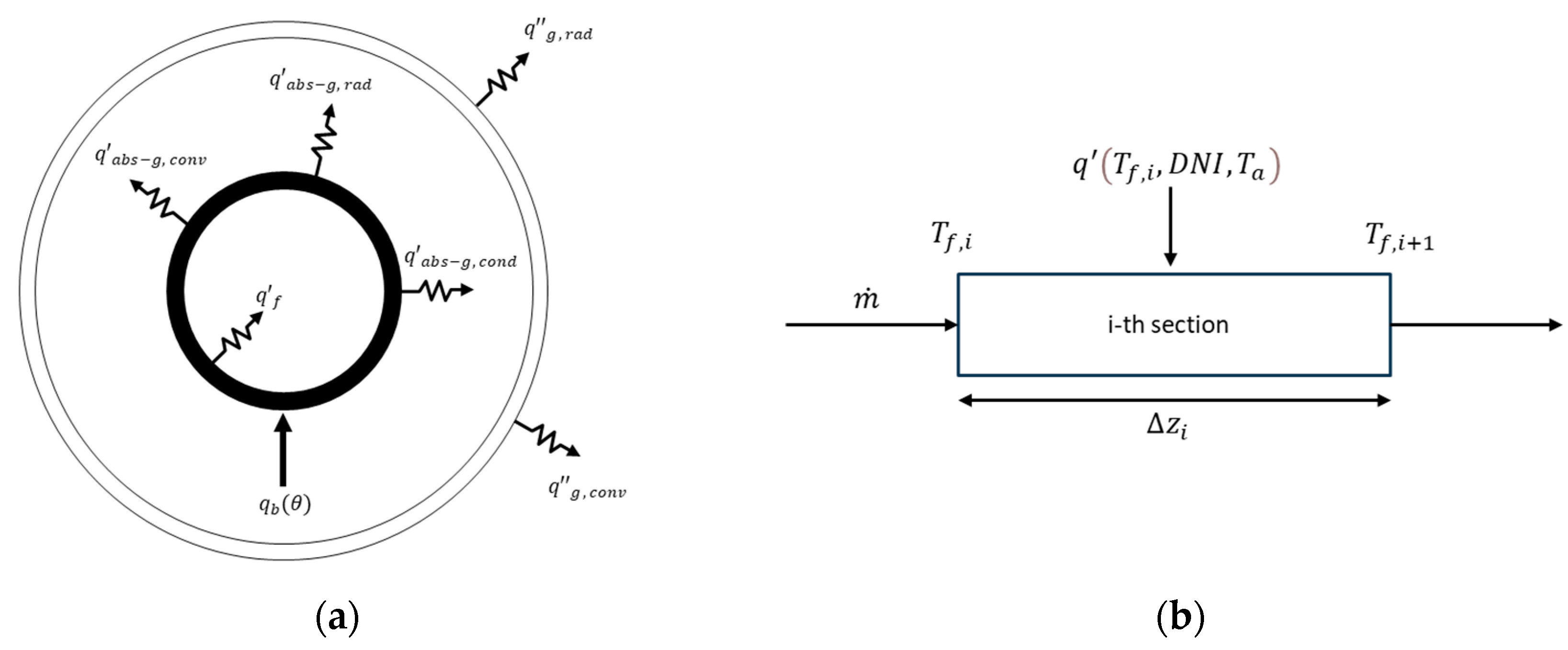
2.2. Heat Transfer in Nonevacuated Parabolic Trough Receiver
- Direct normal irradiance (DNI) is concentrated using the parabolic reflecting mirrors. In this study, the slope, specular and tracking errors were considered by introducing an appropriate increase in the angular divergence of the solar beam.
- The concentrated solar radiation flux is transmitted through the glass envelope and reaches the absorber tube. During this step, a small part of the concentrated solar radiation energy is absorbed by the glass envelope. This amount of energy was considered in the present study.
- The absorber tube absorbs the concentrated solar flux through the selective coating deposited on the outer surface of the absorber. The angular distribution of the absorbed solar flux was considered in this study.
- The heat absorbed by the selective coating is conducted to the inner surface of the absorber tube and then transferred to the HTF through convection. At the same time, the selective coating exchanges energy with the inner surface of glass envelope through conduction, convection and radiative exchange in the annular air gap. All these phenomena were considered in this study.
- The outer surface of the glass envelope dissipates heat towards the environment through convective and radiative exchanges.
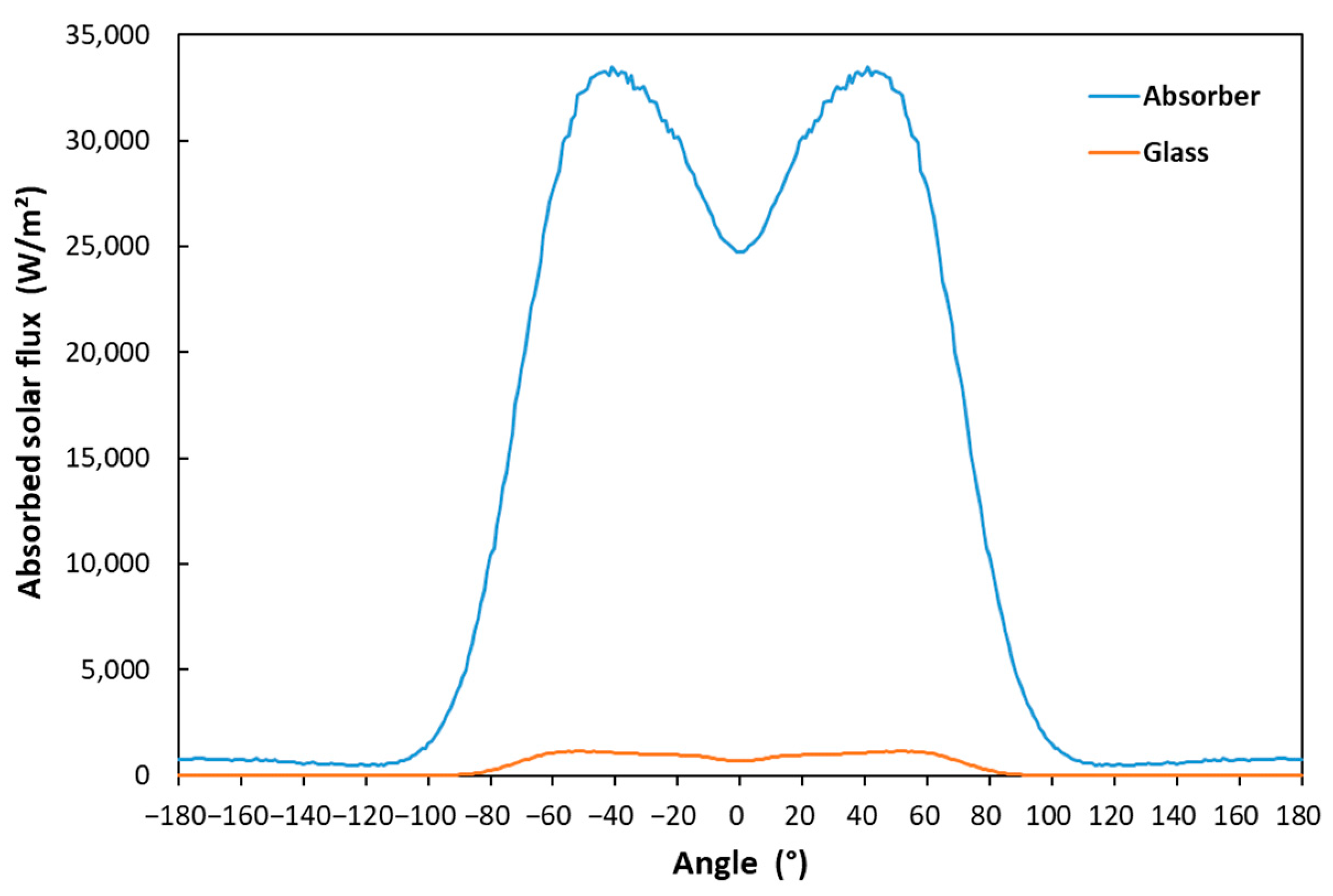
2.3. Concentrated Solar Flux
- Method used: Monte Carlo ray-tracing (MCRT) simulation;
- Grid pattern: dithered rectangular in which for each cell of the rectangular grid, the starting point of each ray is chosen randomly within the cell with a uniform distribution;
- Spatial and angular distributions of the solar beam: the rays are distributed uniformly over the grid dimensions, while the direction of each ray is chosen randomly within a Gaussian angular distribution with a half-angle [31] equal to the assigned divergence of solar beam θsb.
- Sun shape error = 2.6 mrad;
- Slope error = 1.9 mrad;
- Specularity error = 2 mrad;
- Tracking error = 1 mrad.
- Factory settings of optical parameters;
- Reflection and refraction of the rays on the glass envelope;
- Partial reflection of the rays on the absorber surface.
2.4. One-Dimensional Energy Balance Model
3. Model Equations
3.1. Equations Applied to the Heat Transfer Fluid Domain
3.2. Equations Applied to the Air Gap Fluid Domain
3.3. Equations Applied to Solid Domains
3.4. Radiation Heat Transfer
3.5. Boundary Conditions
- Diathermic oil velocity at the inlet of the receiver [41]:
- Turbulence intensity entering the receiver, defined through the following equation [19]:
- Characteristic scale of the turbulence at the inlet, LT0 = 0.07D [30], where D is the inner diameter of the absorber tube;
- Turbulent kinetic energy dissipation in the input, defined by the following expression depending on k0 and LT0:
- Gradients of k and ε in the outlet section in the z direction equal to 0, namely:
- Conductive flux gradients in the z direction, in the outlet and inlet sections, equal to 0;
- Temperature of the diathermic oil entering the absorber tube, variable from 100 to 250 °C;
- Concentrated solar flux absorbed by the absorber tube, , where θ is the angular; coordinate. This flux was deduced from the ray-tracing analysis described in Section 2.3;
- Absolute pressure in the air gap varying from 1.19 to 1.42 bar depending on the temperature of the diathermic oil entering the receiver tube (as described in Section 3.7);
- For fluid domains, the inlet flow rates and the outlet pressures are specified;
- Thermal loss from the glass tube to the ambient air given by the following formula:
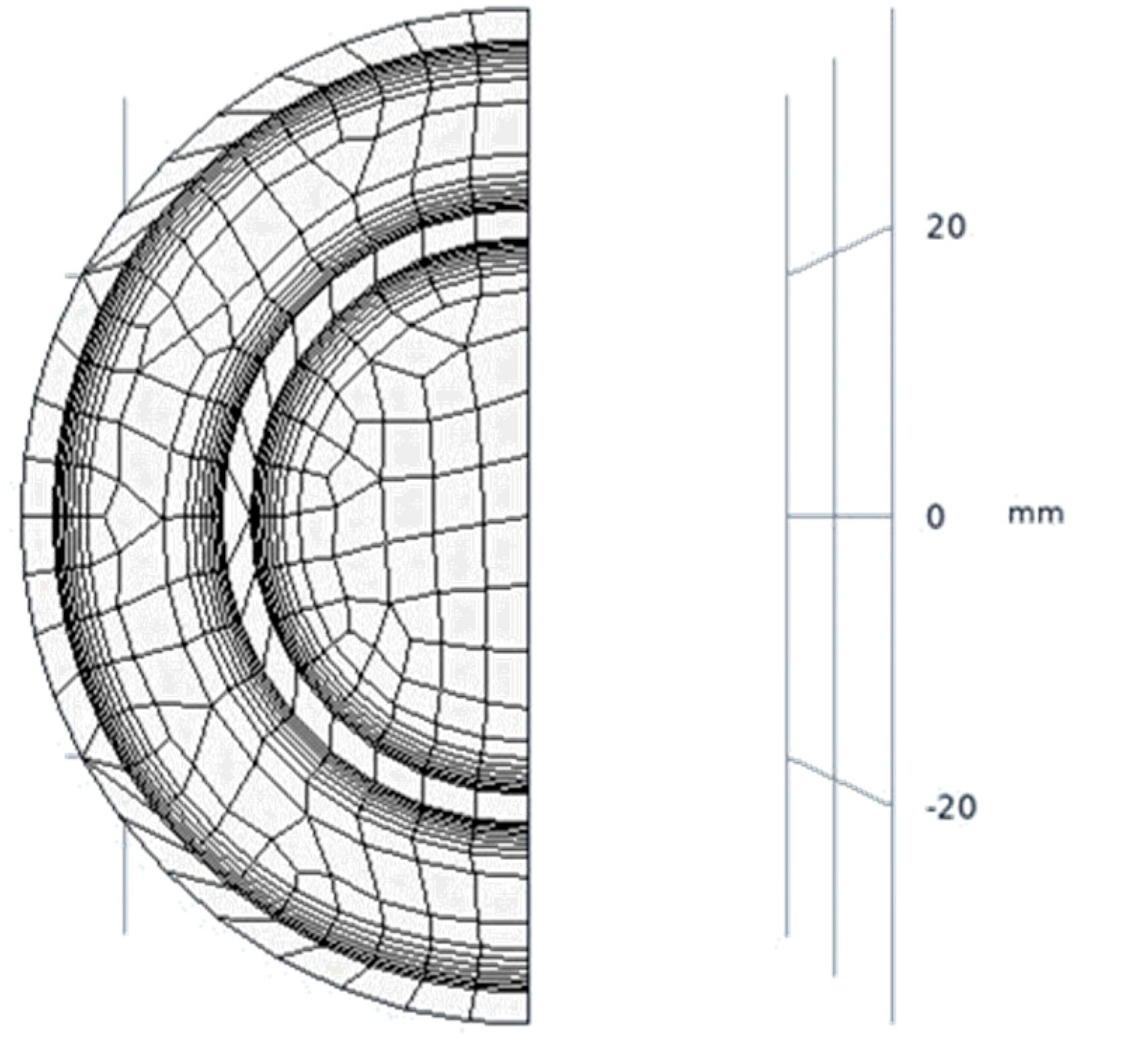
3.6. Mesh and Solver Implemented
3.7. Pressure Variation in the Air Gap
4. Results and Discussion
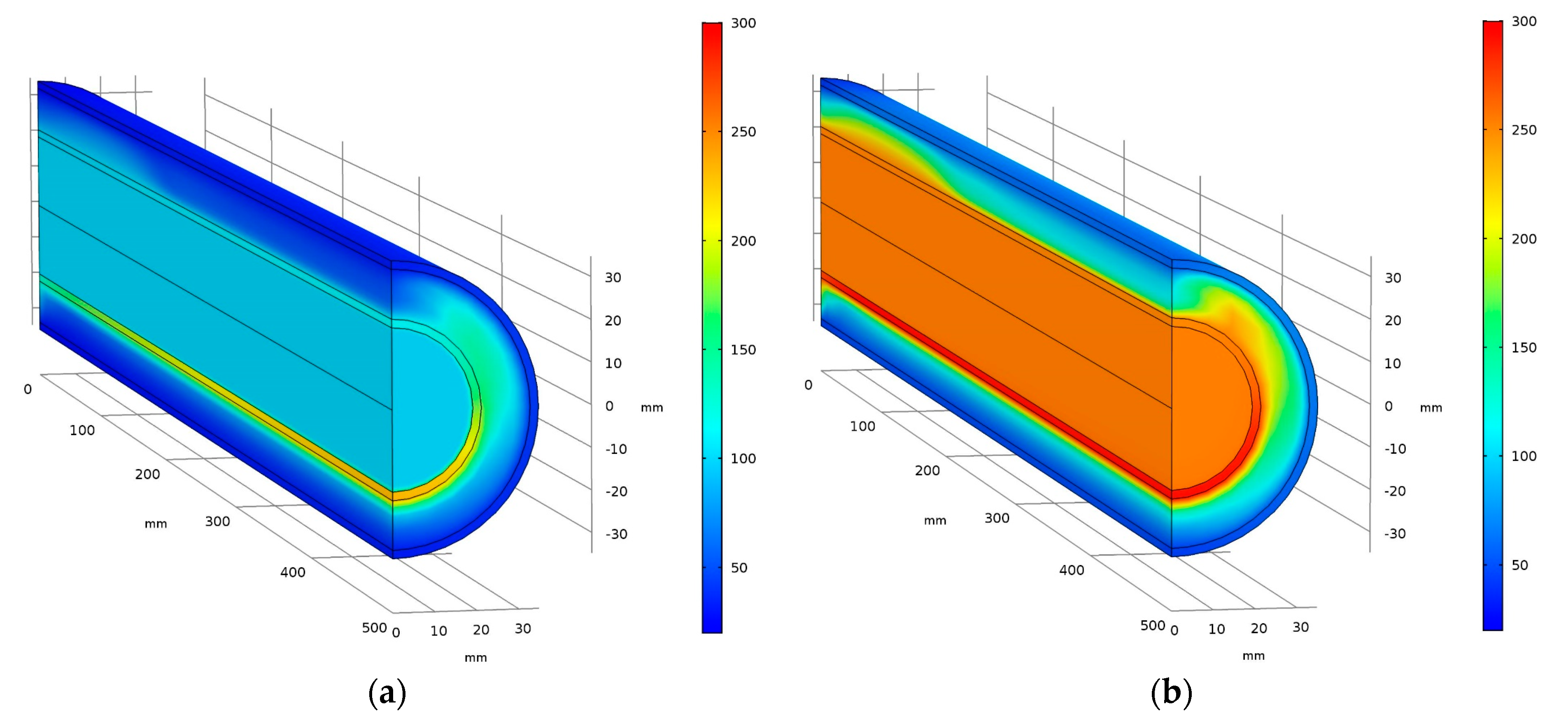
4.1. Three-Dimensional Thermo-Fluid Dynamic Analysis
- Fluid domains are modeled through conjugate heat transfer and nonisothermal flow, which incorporate basic computational fluid dynamic (CFD) equations;
- Laminar and turbulent flow are both supported and can be modeled with natural and forced convection;
- Turbulence can be modeled using Reynolds-averaged Navier–Stokes equations trough “k-ε” or “low Reynolds k-ε” models;
- Pressure work and viscous dissipation can be considered to evaluate the effects on the temperature distribution.
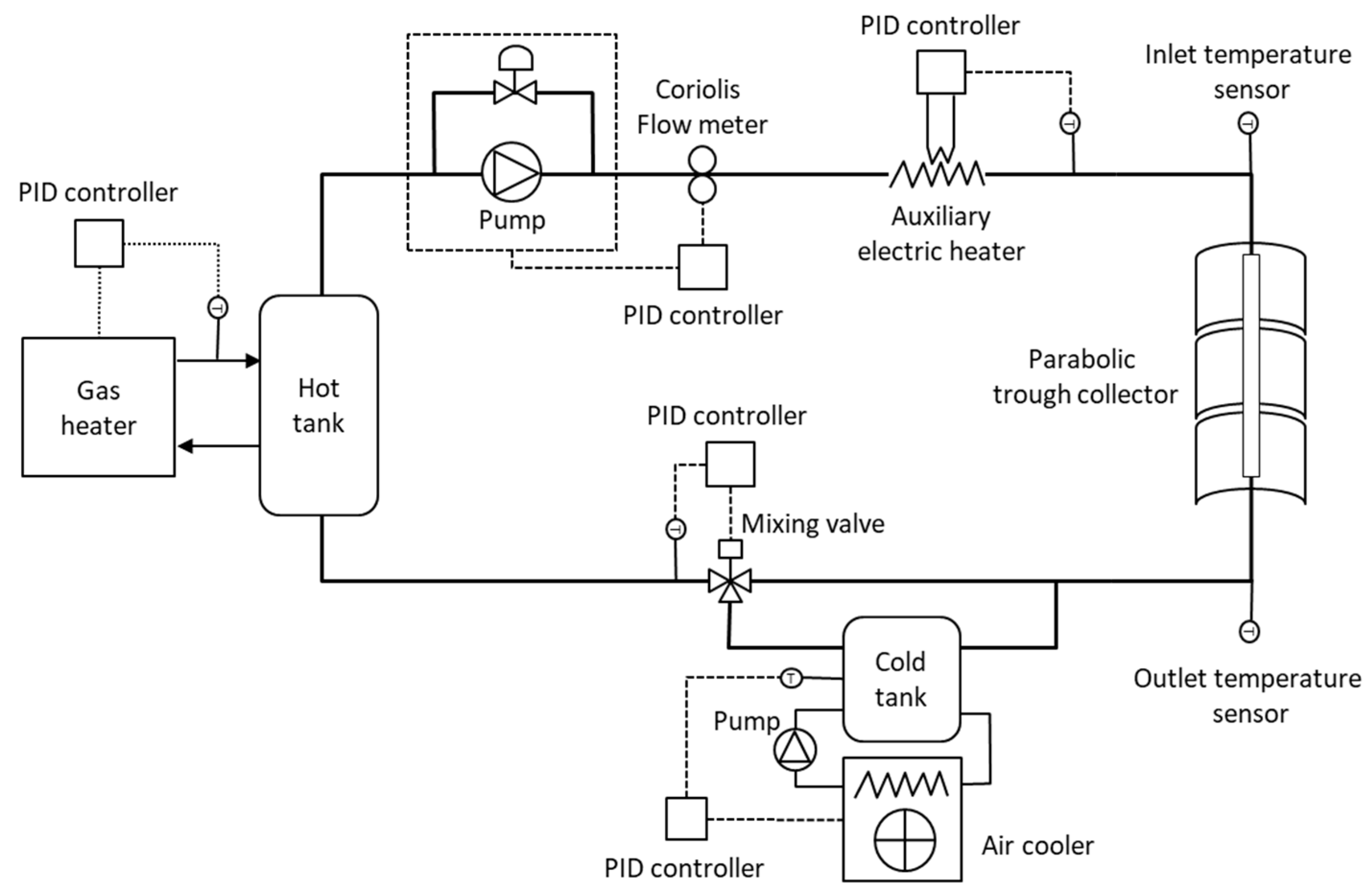
4.2. Experimental Set-Up and Results Obtained
- Temperature: u = 0.08 °C;
- Flow rate: u = 0.1%;
- Direct solar irradiance: u = 6 W/m2;
- Ambient temperature: u = 0.3 °C.
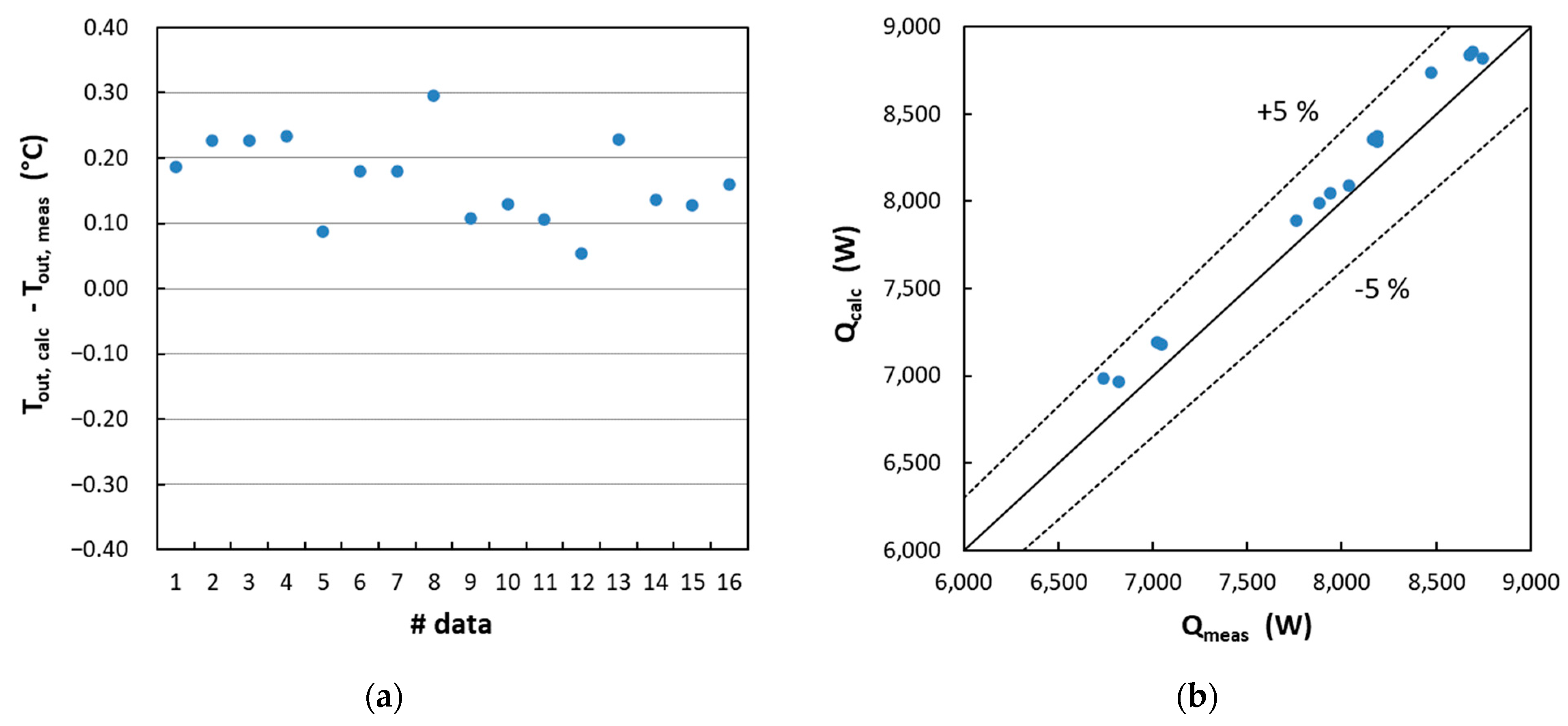
4.3. Comparison between Theoretical and Experimental Data
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | section of the tube (m2) |
| Aa | aperture area of the concentrator (m2) |
| a1, a2 | regression parameters |
| b0, b1, b2 | regression parameters |
| cp,m | specific heat at constant pressure (J/(kg K)) |
| Cµ, Cc1, Cc2 | parameters of the k-ε model |
| D | inner diameter of the receiver tube (m) |
| Dabs | outer diameter of absorber tube (m) |
| Dg | outer diameter of glass envelope (m) |
| DNI | direct normal irradiance (W/m2) |
| f | friction factor |
| fc, fµ | damping functions of Low Reynolds k-ε model |
| g | gravity acceleration (m/s2) |
| G | variable that allows the determination of the wall distance (m−1) |
| Gr | Grashof number |
| k | turbulent kinetic energy (J/kg) |
| k | thermal conductivity (W/(m2 K)) |
| k(l) | laminar conductivity (W/(m K)) |
| k(t) | turbulent conductivity (W/(m K)) |
| hf | convective heat coefficient from absorber tube to diathermic oil (W/(m2 K)) |
| hw | convective heat coefficient from glass tube to ambient air (W/(m2 K)) |
| I | identity matrix |
| l | tube length (m) |
| lref | distance beyond which the objects are described more thoroughly (m) |
| Iw | distance from the receiver wall (wall distance) that satisfies the Eikonal equation (m) |
| flow rate (kg/s) | |
| n | unit vector normal to the tube surface |
| Nu | Nusselt number |
| Pr | Prandtl number |
| pA | absolute pressure (Pa) |
| Reynolds-averaged pressure (Pa) | |
| q | concentrated solar flux on the receiver tube (W/m2) |
| qb(θ) | concentrated solar flux on the outer surface of the absorber tube in function of the angular coordinate θ (W/m2) |
| q′ | thermal power per unit length from the absorber tube to the thermal fluid (W/m) |
| qz′ | thermal power per unit length transferred to the thermal fluid (W/m) |
| heat flux from the glass envelope to the ambient air (W/m2) | |
| heat flux from the absorber tube to the diathermal oil (W/m2) | |
| Q | useful thermal power extracted from the collector (W) |
| r | radial coordinate of the receiver (m) |
| Re | Reynolds number |
| Ri | Richardson number |
| rt | inner radius of the receiver tube (m) |
| tabs | thickness of the absorber tube (m) |
| tglass | thickness of the glass tube (m) |
| Reynolds-averaged temperature (°C) | |
| Tabs | absorber temperature (°C) |
| Ta | ambient air temperature (°C) |
| Tg | glass envelope temperature (°C) |
| Tin | heat transfer fluid temperature at the inlet of the collector (°C) |
| Tout | heat transfer fluid temperature at the outlet of the collector (°C) |
| Tm | mean temperature of the heat transfer fluid (°C) |
| Tf | average mass temperature of the heat transfer fluid (°C) |
| Tst | internal temperatures of the steel tube (°C) |
| Tsky | apparent sky temperature (°C) |
| U | velocity vector (m/s) |
| Reynolds-averaged velocity vector (m/s) | |
| u | standard uncertainty |
| uz,in | diathermic oil velocity at the inlet (m/s) |
| average diathermic oil velocity at the inlet (m/s) | |
| vw | wind velocity (m/s) |
| z | axial receiver coordinate (m) |
| Greek symbols | |
| α | absorber solar absorbance |
| τ | glass solar transmittance |
| ρ | mirror solar reflectance |
| ρ | density of the heat transfer fluid (kg/m3) |
| ε | turbulent kinetic energy dissipation (J/(kg s)) |
| ε | absorber emissivity |
| εg | glass emissivity |
| θ | angular coordinate (rad) |
| θsb | angular divergence of solar beam (rad) |
| µ | viscosity (Pa s) |
| turbulent viscosity (Pa s) | |
| µwall | wall viscosity (Pa s) |
| σ | Boltzmann constant (W/(m2 K4)) |
| σk, σε, σw | low Reynolds k-ε model parameters |
| Subscripts | |
| abs | absorber |
| a | ambient air |
| b | beam |
| calc | calculated |
| cond | conductive |
| conv | convective |
| meas | measured |
| rad | radiative |
| f | fluid |
| g | glass |
| in | inlet |
| out | outlet |
| sb | solar beam |
| st | steel tube |
| w | wind |
| Abbreviations | |
| 2D | two dimensional |
| 3D | three dimensional |
| CFD | computation fluid dynamic |
| DAQ | data acquisition system |
| DCS | distributed control system |
| DNI | direct normal irradiance |
| FEM | finite element method |
| FVM | finite volume method |
| HTF | heat transfer fluid |
| IAM | incident angle modifier |
| MCRT | Monte Carlo ray-tracing |
| PTC | parabolic trough collector |
| RMSE | root mean square error |
References
- Duffie, J.A.; Beckman, W.A. Solar Engineering of Thermal Processes; John Wiley & Sons: Hoboken, NJ, USA, 2006. [Google Scholar]
- Cheng, Z.D.; He, Y.L.; Cui, F.Q.; Xu, R.J.; Tao, Y.B. Numerical simulation of a parabolic trough solar collector with non-uniform solar flux conditions by coupling FVM and MCRT method. Sol. Energy 2012, 86, 1770–1784. [Google Scholar] [CrossRef]
- Cheng, Z.D.; He, Y.L.; Xiao, J.; Tao, Y.B.; Xu, R.J. Three-dimensional Numerical study of heat transfer characteristics in the receiver tube of parabolic trough solar collector. Int. Commun. Heat Mass Transf. 2010, 37, 782–787. [Google Scholar] [CrossRef]
- Ansys Fluent Software. Available online: https://www.ansys.com/Products/Fluids/ANSYS-Fluent (accessed on 10 May 2023).
- Eymard, R.; Gallouët, T.R.; Herbin, R. The Finite Volume Method Handbook of Numerical Analysis; Elsevier: Amsterdam, NL, USA, 2000; Volume 7, pp. 713–1018. [Google Scholar]
- Cheng, Z.D.; He, Y.L.; Cui, F.Q. A new modelling method and unified code with MCRT for concentrating solar collectors and its applications. Appl. Energy 2013, 101, 686–698. [Google Scholar] [CrossRef]
- Wu, Z.; Li, Y.S.; Yuan, G.; Lei, D.; Wang, Z. Three-dimensional Numerical study of heat transfer characteristics of parabolic trough receiver. Appl. Energy 2014, 113, 902–911. [Google Scholar] [CrossRef]
- Fahim, T.; Laouedj, S.; Abderrahmane, A.; Alotaibi, S.; Younis, O.; Muhammad Ali, H. Heat Transfer Enhancement in Parabolic through Solar Receiver: A Three-Dimensional Numerical Investigation. Nanomaterials 2022, 12, 419. [Google Scholar] [CrossRef]
- Sandà, A.; Moya, S.L.; Valenzuela, L.; Cundapí, R. Three-dimensional thermal modelling and heat transfer analysis in the heat collector element of parabolic-trough solar collectors. Appl. Therm. Eng. 2021, 189, 116457. [Google Scholar] [CrossRef]
- Knysh, L. Comprehensive mathematical model and efficient numerical analysis of the design parameters of the parabolic trough receiver. Int. J. Therm. Sci. 2021, 162, 106777. [Google Scholar] [CrossRef]
- Hachicha, A.A.; Rodriguez, I.; Capdevila, R.; Oliva, A. Heat transfer Analysis and numerical simulation of a parabolic trough solar collector. Appl. Energy 2013, 11, 581–592. [Google Scholar] [CrossRef]
- Sandà, A.; Moya, S.L.; Valenzuela, L.; Cundapí, R. Coupling of 3D thermal with 1D thermohydraulic model for single-phase flows of direct steam generation in parabolic-trough solar collectors. Appl. Therm. Eng. 2023, 229, 120614TR. [Google Scholar] [CrossRef]
- Anderson, D.A.; Tannehill, J.C.; Pletcher, R.H. Computational Fluid Mechanics and Heat Transfer; McGraw-Hill Book Company: New York, NY, USA, 1999. [Google Scholar]
- Padilla, R.V.; Demirkaya, G.; Goswami, D.Y.; Stefanakos, E.; Rahman, M.M. Heat Transfer analysis of parabolic trough solar receiver. Appl. Energy 2011, 88, 5097–5110. [Google Scholar] [CrossRef]
- El Kouche, A.; Ortegon Gallego, F. Modeling and numerical simulation of a parabolic trough collector using an HTF with temperature dependent physical properties. Math. Comput. Simul. 2022, 192, 430–451. [Google Scholar] [CrossRef]
- Kalogirou, S.A. A detailed thermal model of a parabolic trough collector receiver. Energy 2012, 48, 298–306. [Google Scholar] [CrossRef]
- EES: Engineering Equation Solver. Available online: http://www.fchart.com/ees/demo.php (accessed on 10 May 2023).
- Heinrich, J.C.; Pepper, D.W. Intermediate Finite Element Method: Fluid Flow and Heat Transfer Applications; Taylor & Francis: London, UK, 1999. [Google Scholar]
- Cesari, F. Il Metodo Degli Elementi Finiti Applicato al Moto dei Fluidi; Pitagora: Bologna, Italy, 1986. [Google Scholar]
- Eck, M.; Feldhoff, J.F.; Uhlig, R. Thermal modelling and simulation of parabolic trough receiver tubes. In Proceedings of the ASME 2010 International Conference of Energy Sustainability, ES2010-90402, Phoenix, AZ, USA, 17–22 May 2010; pp. 659–666. [Google Scholar]
- Dudley, V.E.; Kolb, G.J.; Mahoney, A.R.; Mancini, T.R.; Matthews, C.W.; Sloan, M.; Kearney, D. Tests Results—SEGS LS-2 Solar Collector; Sandia: Albuquerque, NM, USA, 1994. [Google Scholar]
- Singh, M.; Sharma, M.K.; Bhattacharya, J. Design methodology of a parabolic trough collector field for maximum annual energy yield. Renew. Energy 2021, 177, 229–241. [Google Scholar] [CrossRef]
- Salvestroni, M.; Pierucci, G.; Fagioli, F.; Pourreza, A.; Messeri, M.; Taddei, F.; Hosouli, S.; Rashidi, H.; De Lucia, M. Design of a small size PTC: Computational model for the receiver tube and validation with heat loss test. IOP Conf. Ser. Mater. Sci. Eng. 2019, 556, 012025. [Google Scholar] [CrossRef]
- ISO Standards 9806:2017; Solar Energy—Solar Thermal Collectors—Test Methods. ISO: Geneva, Switzerland, 2017.
- Behar, O.; Khellaf, A.; Mohammedi, K. A novel parabolic trough solar collector model—Validation with experimental data and comparison to Engineering Equation Solver (EES). Energy Convers. Manag. 2015, 106, 268–281. [Google Scholar] [CrossRef]
- Liang, H.; You, S.; Zhang, H. Comparison of different heat transfer models for parabolic trough solar collectors. Appl. Energy 2015, 148, 105–114. [Google Scholar] [CrossRef]
- Al-Ansary, H.; Zeitoun, O. Numerical study of conduction and convection heat losses from a half-insulated air-filled annulus of the receiver of a parabolic trough collector. Sol. Energy 2011, 85, 3036–3045. [Google Scholar] [CrossRef]
- Wang, P.; Liu, D.Y.; Xua, C.; Zhou, L.; Xia, L. Conjugate heat transfer modeling and asymmetric characteristic analysis of the heat collecting element for a parabolic trough collector. Int. J. Therm. Sci. 2016, 101, 68–84. [Google Scholar] [CrossRef]
- Ciofalo, M. La turbolenza e i suoi modelli. In Fondamenti di Termofluidodinamica Computazionale; Comini, G., Ed.; S.G.E.: Padova, Italy, 2004; pp. 183–248. [Google Scholar]
- COMSOL Multiphysiscs, Version 6.1; COMSOL, Inc.: Burlington, MA, USA, 2023.
- TRACEPRO, Version 7.4; Lambda Research: Ahmedabad, India, 2015.
- ISO Standard 11146:2021; Lasers and Laser-Related Equipment—Test Methods for Laser Beam Widths, Divergence Angles and Beam Propagation Ratios. ISO: Geneva, Switzerland, 2021.
- Pottler, K.; Ulmer, S.; Lüpfert, E.; Landmann, M.; Röger, M.; Prahl, C. Ensuring performance by geometric quality control and specifications for parabolic trough solar fields. Energy Proc. 2014, 49, 2170–2179. [Google Scholar] [CrossRef][Green Version]
- Mwesigye, A.; Huan, Z.; Bello-Ochende, T.; Meyer, J.P. Influence of optical errors on the thermal and thermodynamic performance of a solar parabolic trough receiver. Solar Energy 2016, 135, 703–718. [Google Scholar] [CrossRef]
- MATLAB, version 7.13.0 (R2011b); The MathWorks Inc.: Natick, MA, USA, 2011.
- Forristall, R. Heat Transfer Analysis and Modeling of a Parabolic through Solar Receiver Implemented in Engineering Equation Solver; NREL/TP-550-34169; National Renewable Energy Laboratory: Golden, CO, USA, 2003. [Google Scholar]
- Incropera, F.P.; Dewitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer, 6th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Kays, W.M. Turbulent Prandtl Number—Where Are We? ASME J. Heat Transf. 1994, 116, 284–295. [Google Scholar] [CrossRef]
- Kays, W.M.; Crawford, M.; Weigand, B. Convective Heat and Mass Transfer, 4th ed.; McGraw-Hill: New York, NY, USA, 2005. [Google Scholar]
- Kreith, F.; Manglik, R.M. Principles of Heat Transfer; Cengage Learning: Boston, MA, USA, 2016. [Google Scholar]
- Bird, R.B.; Stewart, W.E.; Lightfoot, E.N. Transport Phenomena; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Ponti, G.; Palombi, F.; Abate, D.; Ambrosino, F.; Aprea, G.; Bastianelli, T.; Beone, F.; Bertini, R.; Bracco, G.; Caporicci, M.; et al. The role of medium size facilities in the HPC ecosystem: The case of the new CRESCO4 cluster integrated in the ENEAGRID infrastructure. In Proceedings of the 2014 International Conference on High Performance Computing and Simulation (HPCS), Bologna, Italy, 21–25 July 2014; Art. No. 6903807. pp. 1030–1033. [Google Scholar]
- Patnode, A.M. Simulation and Performance Evaluation of Parabolic Trough Solar Power Plants. Master’s Thesis, University of Wisconsin-Madison, Madison, WI, USA, 2006. [Google Scholar]
- Press, W.H.; Teukolsky, S.A.; Vetterling, W.T.; Flannery, B.P. Numerical Recipes 3rd Edition: The Art of Scientific Computing, 3rd ed.; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Therminol® 66 DataSheet. Available online: https://www.therminol.com (accessed on 10 May 2023).


| Parameter | Symbol | Value |
|---|---|---|
| Aperture area | Aa | 13.6 m2 |
| Aperture of optical system | W | 2.37 m |
| Focal length | fc | 0.82 m |
| Rim Angle | ϕr | 72.68° |
| Mirrors length | L | 6 m |
| Outer diameter of absorber tube | Dabs | 42.4 mm |
| Thickness of absorber tube | tabs | 2 mm |
| Outer diameter of glass tube | Dglass | 70 mm |
| Thickness of glass tube | tglass | 2.2 mm |
| Description | Symbol | Value |
|---|---|---|
| Direct normal irradiance on the aperture area | DNI | 1000 W/m2 |
| Mirrors solar reflectance | ρ | 0.94 |
| Absorber tube solar absorbance | α | 0.93 |
| Glass solar transmittance | τ | 0.92 |
| Glass solar absorptance | αg | 0.04 |
| Direction of solar rays | - | On-axis |
| Angular distribution of solar flux | - | Gaussian |
| Assigned angular divergence of solar beam | θsb | 10 mrad |
| Parameter | Mesh 1 | Mesh 2 | Mesh 3 |
|---|---|---|---|
| Type of mesh | Coarse | Normal | Fine |
| Domain elements per unit length | 18,432 | 33,840 | 54,782 |
| Boundary elements per unit length | 8256 | 14,294 | 19,894 |
| Edge elements per unit length | 1412 | 2064 | 2578 |
| Thermal power per unit length q’ (W/m) | 1388.2 | 1376.5 | 1375.3 |
| Relative difference (%) | - | 0.8% | 0.09% |
| Parameter | Values | ||||||
|---|---|---|---|---|---|---|---|
| Tf (°C) | 100 | 125 | 150 | 175 | 200 | 225 | 250 |
| pair gap (bar) | 1.19 | 1.21 | 1.25 | 1.27 | 1.34 | 1.36 | 1.42 |
| Parameter | Symbol | Value |
|---|---|---|
| Ambient air temperature | Ta | 20 °C |
| Apparent sky temperature | Tsky | 10 °C |
| Wind speed | vw | 3 m/s |
| Mass flow rate of diathermic oil | 0.441 kg/s | |
| Absorber thermal conductivity | kabs | 14.8 + 0.0153 (Tabs + 275.15) W/(m K) |
| Absorber hemispherical emittance | εabs | 0.05 + 0.001 Tabs |
| Glass thermal conductivity | kg | 1.38 W/(m K) |
| Glass hemispherical emittance | εg | 0.89 |
| T (°C) | DNI (W/m2) | ||||
|---|---|---|---|---|---|
| 0 | 250 | 500 | 750 | 1000 | |
| 100 | −59.59 | 354.8 | 766.5 | 1176 | 1582 |
| 125 | −87.27 | 330.2 | 745.4 | 1158 | 1569 |
| 150 | −119.5 | 299.7 | 716.9 | 1132 | 1545 |
| 175 | −155.9 | 265.0 | 684.2 | 1102 | 1518 |
| 200 | −199.3 | 222.2 | 642.4 | 1061 | 1478 |
| 225 | −246.3 | 175.1 | 595.3 | 1014 | 1432 |
| 250 | −302.3 | 119.1 | 539.4 | 958.5 | 1377 |
| Parameter | Unit | Value | Standard Error | T-Ratio |
|---|---|---|---|---|
| a1 | W m−1 K−1 | −0.38 | 0.01 | 25.6 |
| a2 | W m−1 K−2 | −0.00401 | 0.00008 | 50.9 |
| b0 | m | 1.571 | 0.005 | 291.1 |
| b1 | m K−1 | 0.00109 | 0.00008 | 14.1 |
| b2 | m K−2 | −0.0000027 | 0.0000003 | 10.4 |
| Parameter | Value |
|---|---|
| Site | ENEA Research Centre Trisaia |
| Latitude | 40°09′ N |
| Longitude | 16°38′ E |
| Inclination and azimuth | Single-axis tracking oriented in E–W direction |
| Heat transfer fluid | Diathermic oil—Therminol® 66 [45] |
| Average flow rate | 0.441 kg/s |
| Mean DNI | 910 W/m2 |
| DNI (W/m2) | Ta (°C) | Tin (°C) | Tout (°C) | (kg/s) | Tm (°C) | cp,m (J/(kg K)) | Qmeas (W) | |
|---|---|---|---|---|---|---|---|---|
| Day 1 | 884 | 23.2 | 105.3 | 115.3 | 0.437 | 110.3 | 1872 | 8204 |
| 887 | 23.4 | 105.1 | 115.1 | 0.437 | 110.1 | 1871 | 8199 | |
| 885 | 23.5 | 105.1 | 115.1 | 0.436 | 110.1 | 1872 | 8158 | |
| 886 | 23.4 | 105.3 | 115.2 | 0.436 | 110.3 | 1872 | 8109 | |
| Day 2 | 955 | 23.3 | 151.8 | 161.6 | 0.436 | 156.7 | 2037 | 8652 |
| 957 | 23.4 | 152.3 | 161.9 | 0.446 | 157.1 | 2039 | 8717 | |
| 959 | 23.6 | 153.2 | 162.7 | 0.446 | 158.0 | 2042 | 8631 | |
| 947 | 23.1 | 151.9 | 161.5 | 0.436 | 156.7 | 2037 | 8527 | |
| Day 3 | 916 | 21.0 | 201.4 | 209.2 | 0.448 | 205.3 | 2214 | 7688 |
| 904 | 21.4 | 199.8 | 207.8 | 0.448 | 203.8 | 2208 | 7876 | |
| 918 | 21.3 | 198.2 | 206.2 | 0.448 | 202.2 | 2203 | 7862 | |
| 921 | 21.2 | 196.7 | 204.6 | 0.449 | 200.7 | 2197 | 7769 | |
| Day 4 | 874 | 23.7 | 251.5 | 258.2 | 0.437 | 254.8 | 2399 | 7040 |
| 871 | 23.5 | 250.5 | 257.0 | 0.436 | 253.7 | 2394 | 6777 | |
| 892 | 23.4 | 250.1 | 256.8 | 0.437 | 253.4 | 2393 | 7004 | |
| 895 | 24.5 | 251.3 | 257.8 | 0.435 | 254.6 | 2398 | 6800 |
| Tout, meas (°C) | Tout, calc (°C) | Difference % | Qmeas (W) | Qcalc (W) | Difference % | |
|---|---|---|---|---|---|---|
| Day 1 | 115.3 | 115.49 | 0.16% | 8204 | 8342 | 1.85% |
| 115.1 | 115.33 | 0.20% | 8199 | 8373 | 2.26% | |
| 115.1 | 115.33 | 0.20% | 8158 | 8353 | 2.26% | |
| 115.2 | 115.53 | 0.20% | 8109 | 8362 | 2.33% | |
| Day 2 | 161.6 | 161.69 | 0.05% | 8652 | 8821 | 0.88% |
| 161.9 | 161.98 | 0.11% | 8717 | 8839 | 1.87% | |
| 162.7 | 162.88 | 0.11% | 8631 | 8855 | 1.88% | |
| 161.5 | 161.70 | 0.18% | 8527 | 8740 | 3.11% | |
| Day 3 | 209.2 | 209.41 | 0.05% | 7688 | 7989 | 1.35% |
| 207.8 | 207.73 | 0.06% | 7876 | 7890 | 1.65% | |
| 206.2 | 206.31 | 0.05% | 7862 | 8045 | 1.32% | |
| 204.6 | 204.85 | 0.03% | 7769 | 8090 | 0.64% | |
| Day 4 | 258.2 | 258.13 | 0.09% | 7040 | 6983 | 3.58% |
| 257.0 | 257.14 | 0.05% | 6777 | 6964 | 2.09% | |
| 256.8 | 256.93 | 0.05% | 7004 | 7178 | 1.91% | |
| 257.8 | 258.16 | 0.06% | 6800 | 7193 | 2.39% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ebolese, A.; Marano, D.; Copeta, C.; Bruno, A.; Sabatelli, V. Numerical Modeling and Experimental Validation of Heat Transfer Characteristics in Small PTCs with Nonevacuated Receivers. Solar 2023, 3, 544-565. https://doi.org/10.3390/solar3040030
Ebolese A, Marano D, Copeta C, Bruno A, Sabatelli V. Numerical Modeling and Experimental Validation of Heat Transfer Characteristics in Small PTCs with Nonevacuated Receivers. Solar. 2023; 3(4):544-565. https://doi.org/10.3390/solar3040030
Chicago/Turabian StyleEbolese, Amedeo, Domenico Marano, Carlo Copeta, Agatino Bruno, and Vincenzo Sabatelli. 2023. "Numerical Modeling and Experimental Validation of Heat Transfer Characteristics in Small PTCs with Nonevacuated Receivers" Solar 3, no. 4: 544-565. https://doi.org/10.3390/solar3040030
APA StyleEbolese, A., Marano, D., Copeta, C., Bruno, A., & Sabatelli, V. (2023). Numerical Modeling and Experimental Validation of Heat Transfer Characteristics in Small PTCs with Nonevacuated Receivers. Solar, 3(4), 544-565. https://doi.org/10.3390/solar3040030






