Porous and Magnetic Effects on Modified Stokes’ Problems for Generalized Burgers’ Fluids
Abstract
:1. Introduction
2. Governing Equations
3. Analytical Expressions for the Dimensionless Steady-State Solutions
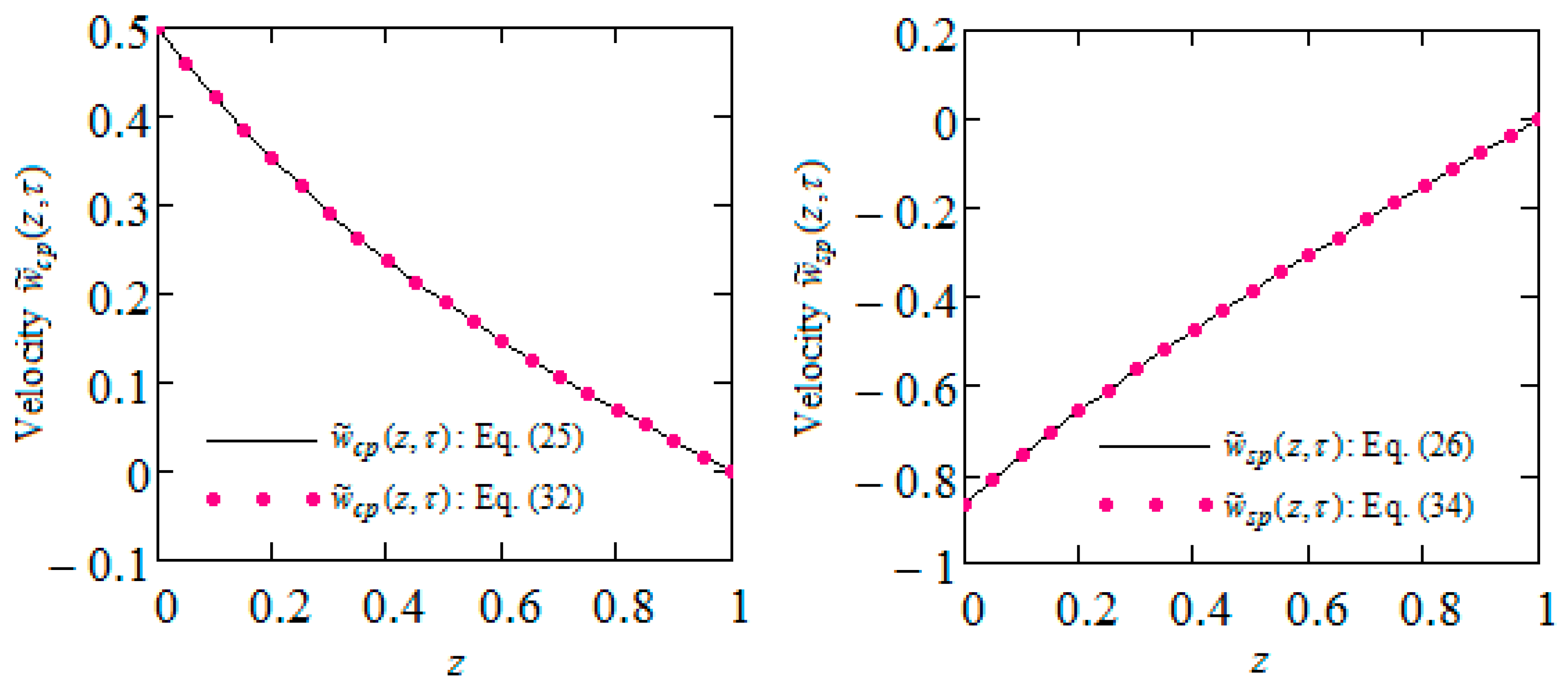
3.1. Analytical Expressions for and
3.2. Exact Expressions for and
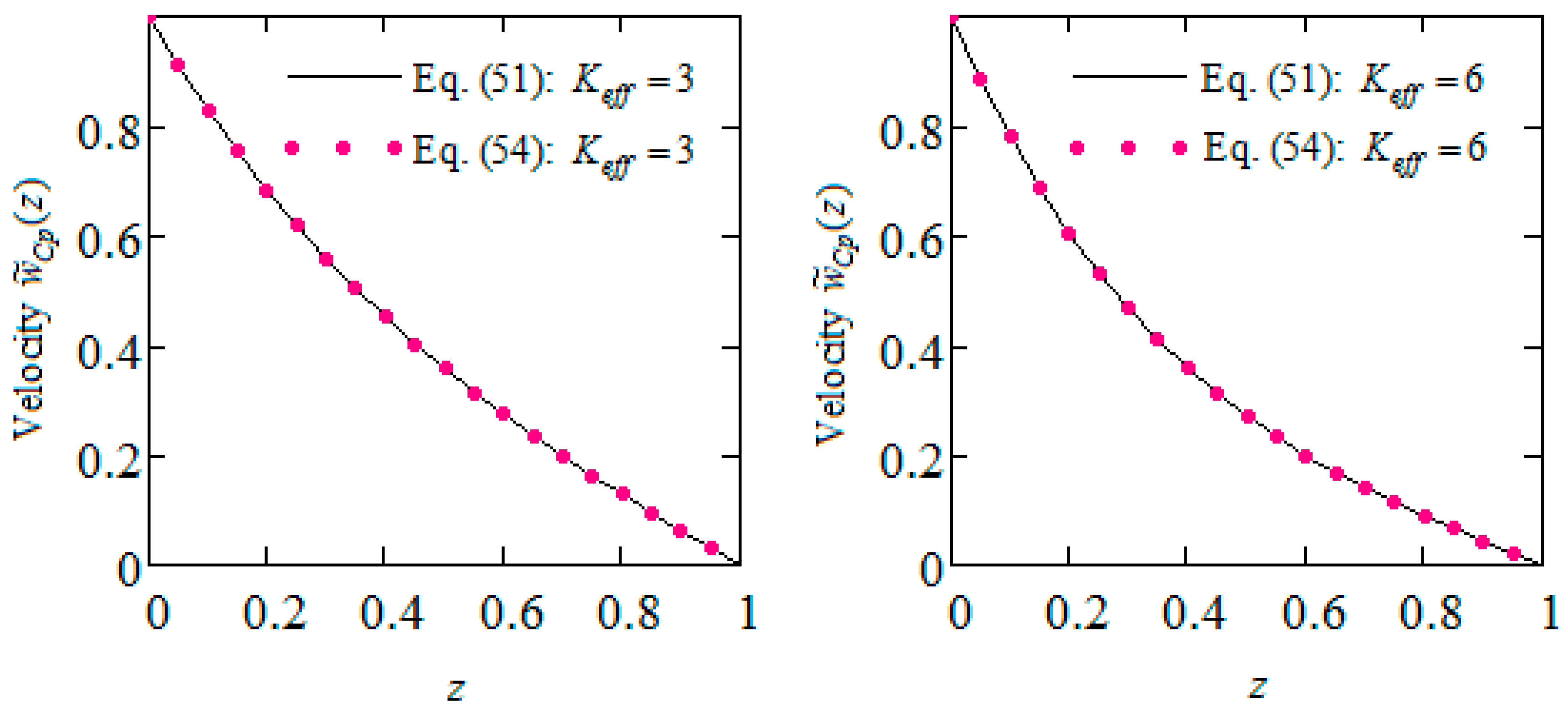
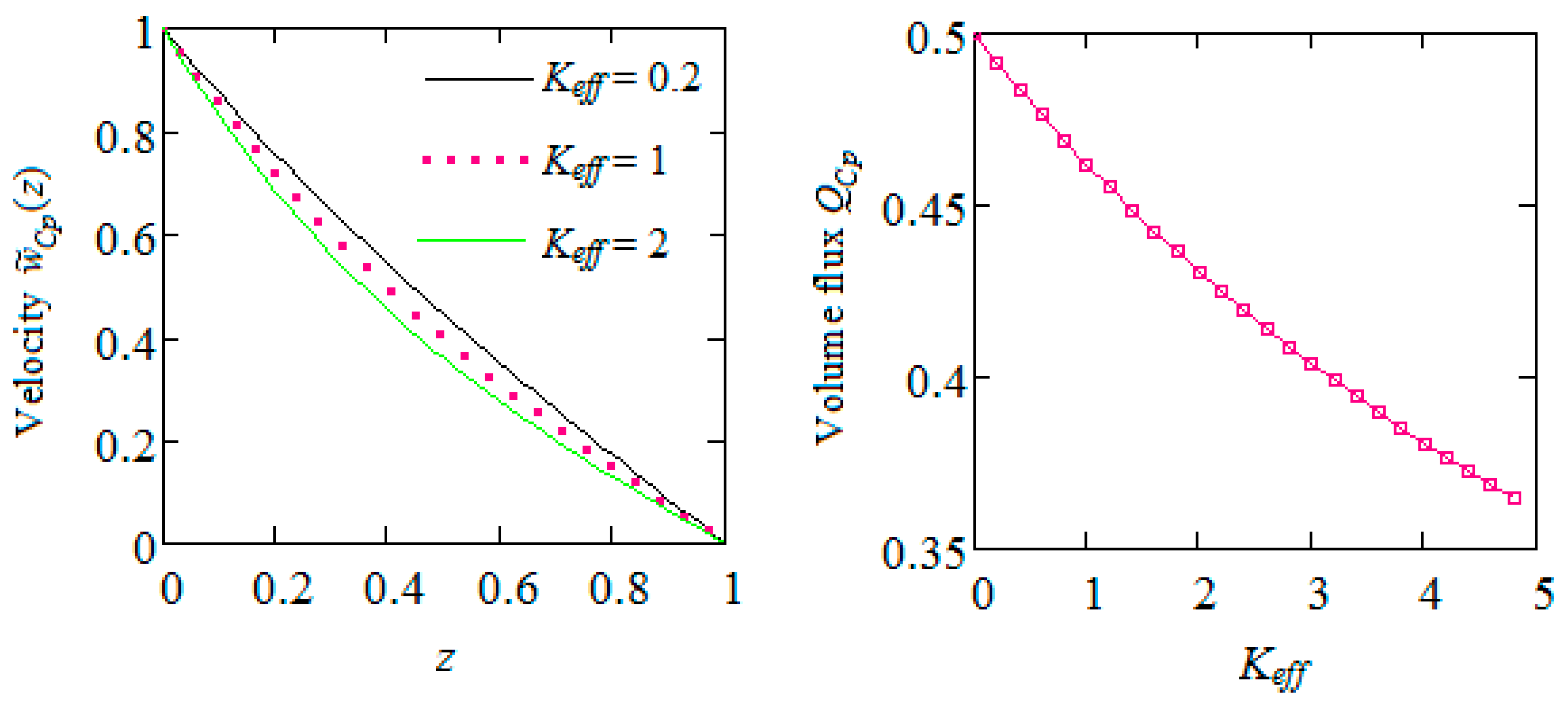
3.3. Limiting Case (Modified Stokes’ First Problem)
3.4. A Simple but Useful Observation Regarding Governing Equations for Velocity and Shear Stress
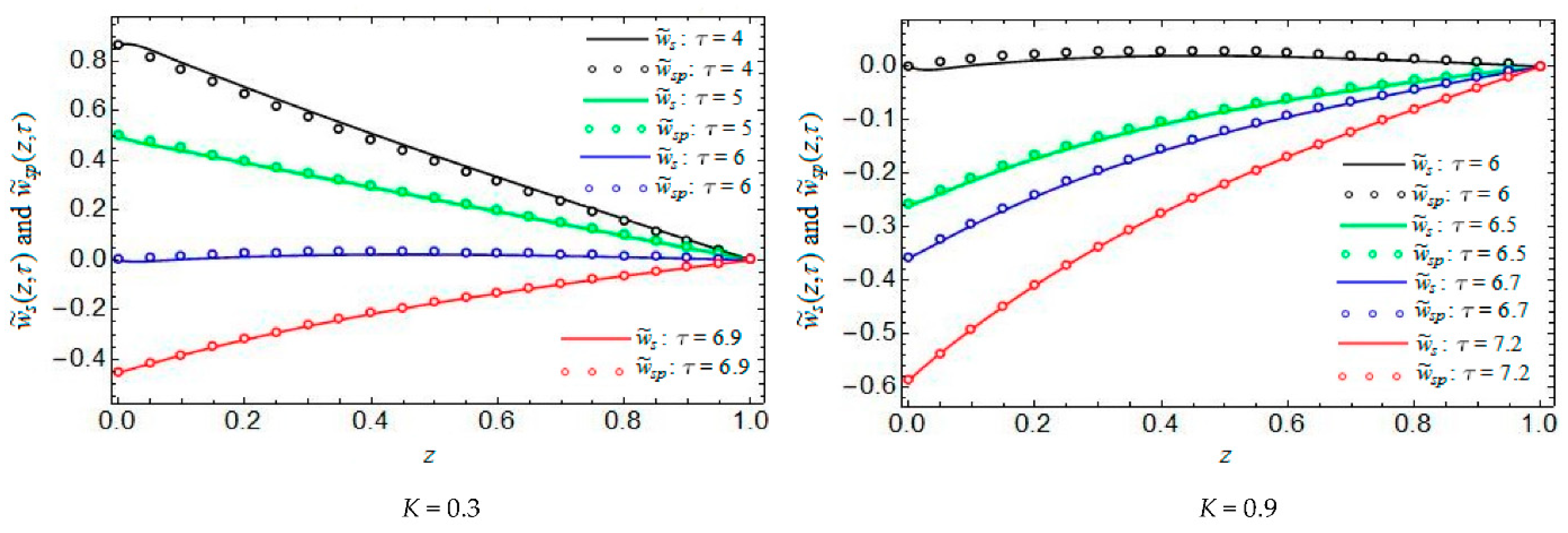
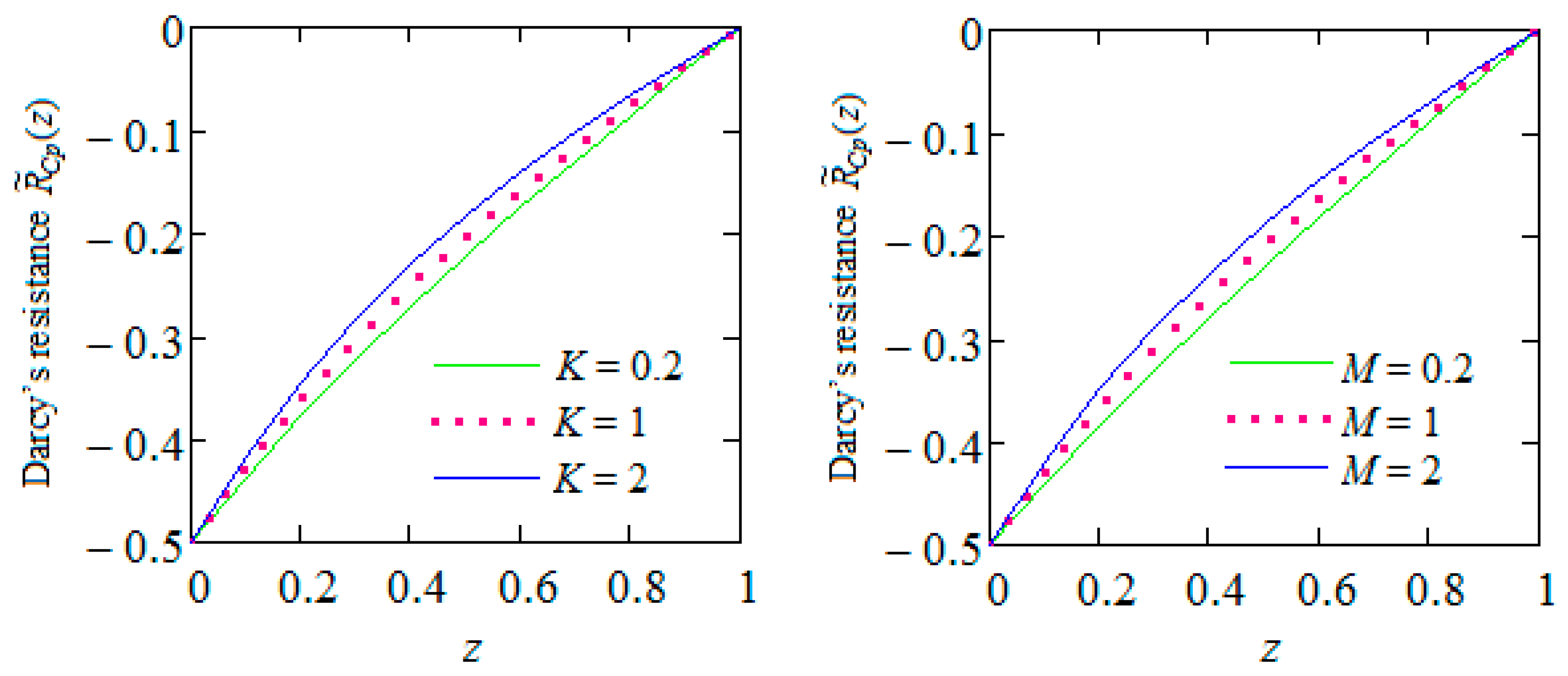
4. Some Numerical Results and Discussion
5. Conclusions
- Closed-form expressions are provided for the dimensionless steady-state velocity, shear stress and Darcy’s resistance of MHD modified Stokes’s problems for IGBFs through a porous medium. For validation, the fluid velocities are presented in equivalent forms.
- The obtained expressions can be immediately particularized to find similar solutions for Burgers, Oldroyd-B, Maxwell, second-grade and Newtonian fluids subject to same motions.
- Convergence of the starting velocities (numerical solutions) to their steady components is graphically proven, and the necessary time to reach a steady state is found.
- This time proportionally increases with the augmentation of the porous and magnetic parameters K and M, respectively. Consequently, the establishment of a steady state is more expeditiously achieved in the absence of a porous medium or a magnetic field.
- The flow resistance of fluid exhibits a propensity to escalate in the presence of a porous medium or magnetic field. This results in a decelerated flow rate of the fluid within a porous medium or in the presence of a magnetic field.
- The governing equations for the fluid velocity and the non-trivial shear stress corresponding to the MHD motions of IGBFs between parallel plates are identical in form. Consequently, MHD motion problems with shear stress on the boundary can be solved for incompressible rate-type fluids.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| T | Cauchy stress tensor |
| S | Extra-stress tensor |
| A | First Rivlin–Ericksen tensor |
| I | Identity tensor |
| Hydrostatic pressure | |
| Amplitude of the oscillations | |
| Material constants | |
| Dynamic viscosity | |
| Kinematic viscosity | |
| Time | |
| Cartesian coordinates | |
| Frequency of oscillations | |
| Velocity vector | |
| Fluid velocity | |
| Tangential shear stress | |
| Darcy’s resistance | |
| d | Distance between plates |
| Fluid density | |
| Electrical conductivity | |
| Porosity | |
| k | Permeability of porous medium |
| Q | Volume flux |
| Dimensionless constants | |
| M | Magnetic parameter |
| K | Porous parameter |
| B | Magnitude of magnetic field |
| Effective permeability |
References
- Fetecau, C.; Hayat, T.; Fetecau, C. Seady-state solutions for some simple flows of generalized Burgers fluids. Int. J. Non-Linear Mech. 2006, 41, 880–887. [Google Scholar] [CrossRef]
- Ravindran, P.; Krishnan, J.M.; Rajagopal, K.R. A note on the flow of a Burgers’ fluid in an orthogonal rheometer. Int. J. Eng. Sci. 2004, 42, 1973–1985. [Google Scholar] [CrossRef]
- Zheng, L.C.; Zhao, F.F.; Zhang, X.X. An exact solution for an unsteady flow of a generalized Burgers’ fluid induced by an accelerating plate. Int. J. Nonlinear Sci. Numer. Simul. 2010, 11, 457–464. [Google Scholar] [CrossRef]
- Jamil, M. First problem of Stokes for generalized Burgers’ fluids. Int. Sch. Res. Netw. ISRN Math. Phys. 2012, 2012, 831063. [Google Scholar] [CrossRef]
- Fetecau, C.; Fetecau, C.; Akhtar, S. Permanent solutions for some axial motions of generalized Burgers fluids in cylindrical domains. Ann. Acad. Rom. Sci. Ser. Math. Appl. 2015, 7, 271–284. [Google Scholar]
- Khan, I.; Hussanan, A.; Salleh, M.Z.; Tahar, R.M. Exact solutions of accelerated flows for a generalized Burgers’ fluid, I: The case. In Proceedings of the 4th International Conference on Computer Science and Computational Mathematics (ICCSCM 2015), Langkawi, Malaysia, 7–8 May 2015; pp. 47–52. [Google Scholar]
- Tong, D. Starting solutions for oscillating motions of a generalized Burgers’ fluid in cylindrical domains. Acta Mech. 2010, 214, 395–407. [Google Scholar] [CrossRef]
- Kiema, D.W.; Manyonge, W.A.; Bitok, J.K.; Adenyah, R.K.; Barasa, J.S. On the steady Couette flow between two infinite parallel plates in an uniform transverse magnetic field. J. Appl. Math. Bioinform. 2015, 5, 87–99. [Google Scholar]
- Sultan, Q.; Nazar, M.; Imran, M.; Ali, U. Flow of generalized Burgers fluid between parallel walls induced by rectified sine pulses stress. Bound. Value Probl. 2014, 2014, 152. [Google Scholar] [CrossRef]
- Sultan, Q.; Nazar, M. Flow of generalized Burgers’ fluid between side walls induced by sawtooth pulses stress. J. Appl. Fluid Mech. 2016, 9, 2195–2204. [Google Scholar] [CrossRef]
- Khan, M.; Malik, R.; Anjum, A. Exact solutions of MHD second Stokes’ flow of generalized Burgers fluid. Appl. Math. Mech. Engl. Ed. 2015, 36, 211–224. [Google Scholar] [CrossRef]
- Abro, K.A.; Hussain, M.; Baig, M.M. Analytical solution of magnetohydrodynamics generalized Burgers’ fluid embedded with porosity. Int. J. Adv. Appl. Sci. 2017, 4, 80–89. [Google Scholar] [CrossRef]
- Alqahtani, A.M.; Khan, I. Time-dependent MHD flow of non-Newtonian generalized Burgers’ fluid (GBF) over a suddenly moved plate with generalized Darcy’s law. Front. Phys. 2020, 7, 214. [Google Scholar] [CrossRef]
- Hussain, M.; Qayyum, M.; Afzal, S. Modeling and analysis of MHD oscillatory flows of generalized Burgers’ fluid in a porous medium using Fourier transform. J. Math. 2022, 2022, 2373084. [Google Scholar] [CrossRef]
- Fetecau, C.; Morosanu, C. Steady state solutions of MHD Stokes problems for generalized Burgers fluids through porous media. Bul. Instit. Polit. Iasi 2023, submitted.
- Rajagopal, K.R.; Saccomandi, G.; Vergori, L. Unsteady flows of fluids with pressure dependent viscosity. J. Math. Anal. Appl. 2013, 404, 362–372. [Google Scholar] [CrossRef]
- Cramer, K.R.; Pai, S.I. Magnetofluid Dynamics for Engineers and Applied Physicists; McGraw-Hill: New York, NY, USA, 1973. [Google Scholar]
- Prusa, V. Revisiting Stokes first and second problems for fluids with pressure-dependent viscosities. Int. J. Eng. Sci. 2010, 48, 2054–2065. [Google Scholar] [CrossRef]
- Sneddon, I.N. Fourier Transforms; McGraw Hill, Book Company, Inc.: New York, NY, USA; Toronto, ON, Canada; London, UK, 1951. [Google Scholar]
- Joseph, D.D. Fluid Dynamics of Viscoelastic Liquids; Springer-Verlag: New York, NY, USA, 1990. [Google Scholar]
- Erdogan, M.E. On the unsteady unidirectional flows generated by impulsive motion of a boundary or sudden application of a pressure gradient. Int. J. Non-Linear Mech. 2002, 37, 1091–1106. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fetecau, C.; Akhtar, S.; Moroşanu, C. Porous and Magnetic Effects on Modified Stokes’ Problems for Generalized Burgers’ Fluids. Dynamics 2023, 3, 803-819. https://doi.org/10.3390/dynamics3040044
Fetecau C, Akhtar S, Moroşanu C. Porous and Magnetic Effects on Modified Stokes’ Problems for Generalized Burgers’ Fluids. Dynamics. 2023; 3(4):803-819. https://doi.org/10.3390/dynamics3040044
Chicago/Turabian StyleFetecau, Constantin, Shehraz Akhtar, and Costică Moroşanu. 2023. "Porous and Magnetic Effects on Modified Stokes’ Problems for Generalized Burgers’ Fluids" Dynamics 3, no. 4: 803-819. https://doi.org/10.3390/dynamics3040044
APA StyleFetecau, C., Akhtar, S., & Moroşanu, C. (2023). Porous and Magnetic Effects on Modified Stokes’ Problems for Generalized Burgers’ Fluids. Dynamics, 3(4), 803-819. https://doi.org/10.3390/dynamics3040044





