Self-Organised Critical Dynamics as a Key to Fundamental Features of Complexity in Physical, Biological, and Social Networks
Abstract
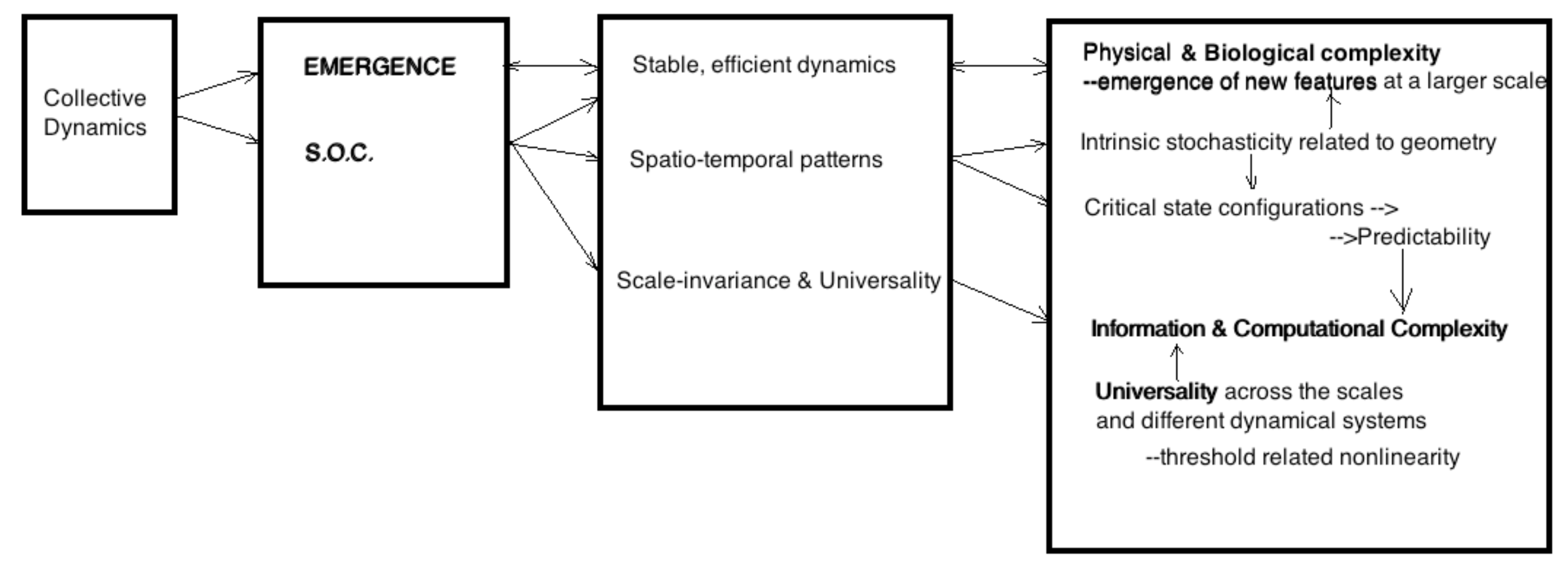
1. Introduction: Self-Organised Criticality and Complexity
2. Properties of the Self-Organised Critical States
3. Self-Organised Critical Systems and Their Networks at Different Scales
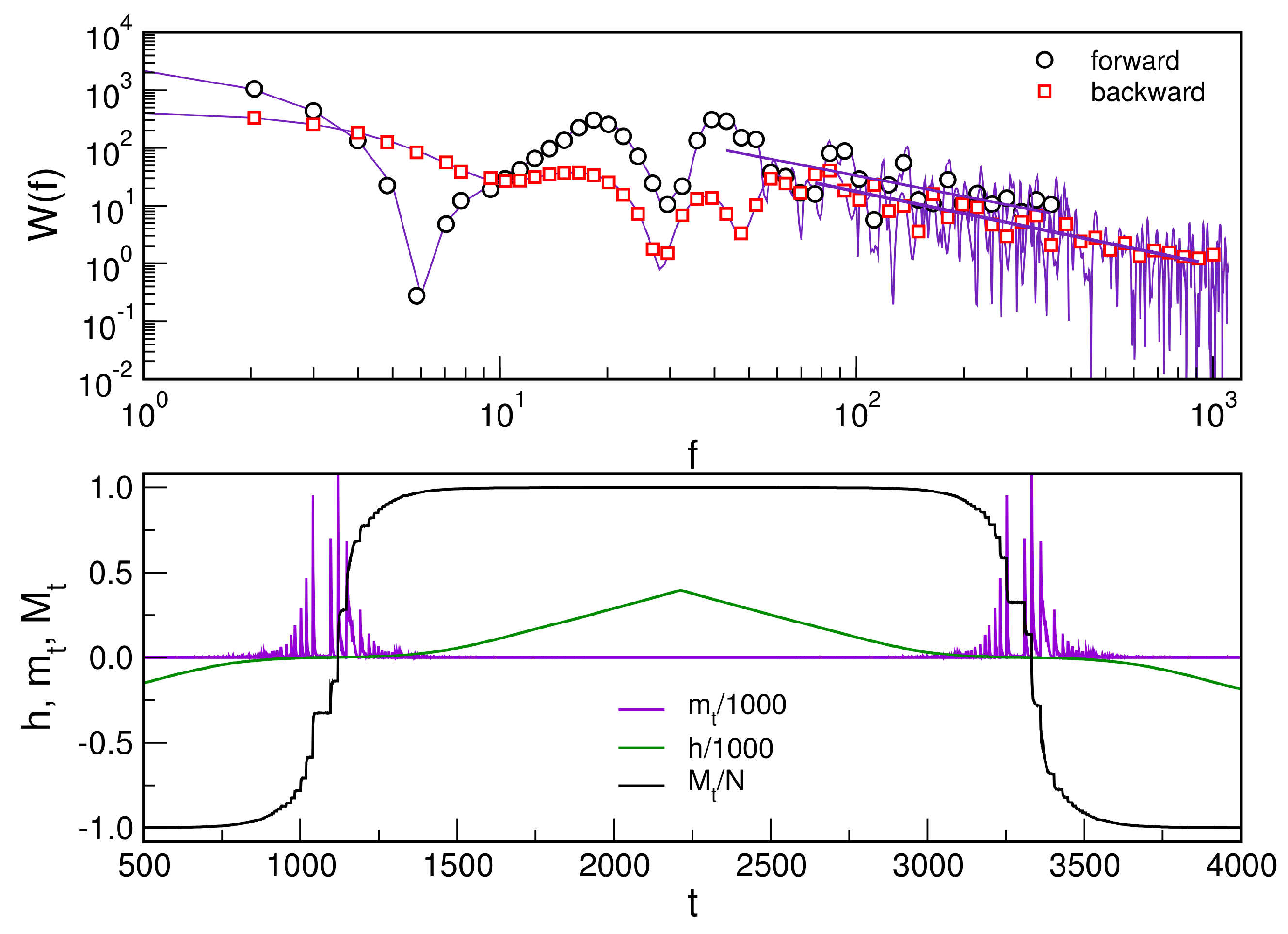
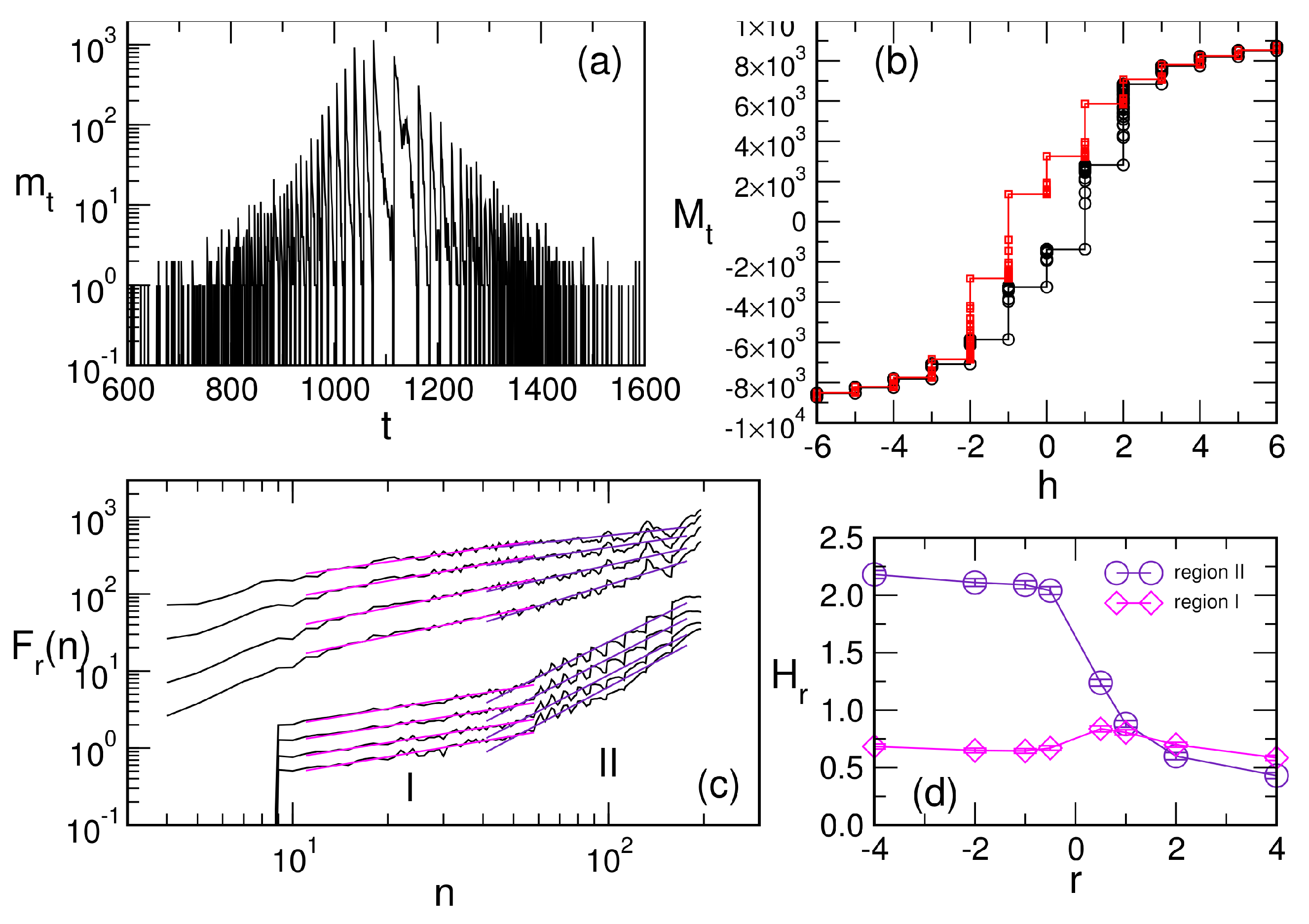
4. Hysteresis-Loop Criticality in Nanonetworks with Simplicial Complexes
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jensen, H.J. Self-Organized Criticality: Emergent Complex Behavior in Physical and Biological Systems; Cambridge Lecture Notes in Physics; Cambridge University Press: Cambridge, UK, 1998. [Google Scholar]
- Aschwanden, M.J. Self-Organized Criticality Systems; Open Academic Press: Berlin, Germany; Warsaw, Poland, 2013. [Google Scholar]
- Bak, P.; Tang, C.; Wiesenfeld, K. Self-organized criticality: An explanation of 1/f noise. Phys. Rev. Lett. 1987, 59, 381–384. [Google Scholar] [CrossRef] [PubMed]
- Marković, D.; Gros, C. Power laws and self-organized criticality in theory and nature. Phys. Rep. 2014, 536, 41–74. [Google Scholar] [CrossRef]
- McAteer, R.; Aschwanden, M.; Dimitropoulou, M.; Georgoulis, M.; Pruessner, G.; Morales, L.; Ireland, J.; Abramenko, V. 25 years of self-organized criticality: Numerical detection methods. Space Sci. Rev. 2016, 198, 217–266. [Google Scholar] [CrossRef]
- Watkins, N.W.; Pruessner, G.; Chapman, S.C.; Crosby, N.B.; Jensen, H.J. 25 years of self-organized criticality: Concepts and controversies. Space Sci. Rev. 2016, 198, 3–44. [Google Scholar] [CrossRef]
- Dhar, D. Self-organized critical state of sandpile automaton models. Phys. Rev. Lett. 1990, 64, 1613–1616. [Google Scholar] [CrossRef] [PubMed]
- Manna, S.S. Two-state model of self-organized criticality. J. Phys. Math. Gen. 1991, 24, L363–L369. [Google Scholar] [CrossRef]
- Tadić, B.; Dhar, D. Emergent spatial structures in critical sandpiles. Phys. Rev. Lett. 1997, 79, 1519. [Google Scholar] [CrossRef]
- Milshtein, E.; Buham, O.; Solomon, S. Universality classes in isotropic, Abelian, and non-Abelian sandpile models. Phys. Rev. E 1998, 58, 303. [Google Scholar] [CrossRef]
- Bak, P.; Paczuski, M. Complexity, contingency, and criticality. Proc. Natl. Acad. Sci. USA 1995, 92, 6689–6696. [Google Scholar] [CrossRef]
- Wolf, Y.I.; Katsnelson, M.K.; Koonin, E.V. Physical foundations of biological complexity. Proc. Natl. Acad. Sci. USA 2018, 115, E8678–E8687. [Google Scholar] [CrossRef]
- Braverman, M. Information complexity and applications. Jap. J. Math. 2019, 14, 27–65. [Google Scholar] [CrossRef]
- Song, J.; Marsili, M.; Jo, J. Resolution and relevance trade-offs in deep learning. J. Stat. Mech. Theory Exp. 2018, 2018, 123406. [Google Scholar] [CrossRef]
- Moore, C.; Nilsson, M. The computational complexity of sandpiles. J. Stat. Phys. 2020, 96, 205–224. [Google Scholar] [CrossRef]
- Goles, E.; Montealegre, P.; Perrot, K. Freezing sandpiles and Boolean threshold networks: Equivalence and complexity. Adv. Appl. Math. 2021, 125, 102161. [Google Scholar] [CrossRef]
- Formenti, E.; Perrot, K. How hard is it to predict sandpiles on lattices? Fundam. Inform. 2019, 171, 189–219. [Google Scholar] [CrossRef]
- Shapoval, A.; Savostianova, D.; Shnirman, M. Predictability and scaling in btw sandpile on a self-similar lattice. J. Stat. Phys. 2021, 183, 1–15. [Google Scholar] [CrossRef]
- Haken, H.; Portugali, J. Information and self-organization. Entropy 2017, 19, 18. [Google Scholar] [CrossRef]
- Mancini, S.; Marmo, G.; Pascazio, S. Quantum information and complexity. Eur. J. Phys. Plus 2014, 129, 269. [Google Scholar] [CrossRef][Green Version]
- Cubero, R.J.; Jo, J.; Marsili, M.; Roudi, Y.; Song, J. Statistical criticality arises in most informative representations. J. Stat. Mech. Theory Exp. 2019, 2019, 063402. [Google Scholar] [CrossRef]
- Moore, C. Majority-vote cellular automata, Ising dynamics, and p-completeness. J. Stat. Phys. 1997, 88, 795–805. [Google Scholar] [CrossRef]
- Zachariou, N.; Expert, P.; Takayashu, M.; Christensen, K. Generalized sandpile dynamics on artificial and real-world networks. PLoS ONE 2015, 10, e0142685. [Google Scholar] [CrossRef]
- Shapoval, A.; Shnirman, M. The BTW mechanism on a self-similar image of a square: A path to unexpected exponents. Phys. A 2020, 391, 15–20. [Google Scholar] [CrossRef]
- Bhaumik, H.; Santra, S.B. Critical properties of deterministic and stochastic sandpile models on two-dimensional percolation backbone. Phys. Stat. Mech. Its Appl. 2020, 548, 124318. [Google Scholar] [CrossRef]
- Najafi, M.N.; Cheraghalizadeh, J.; Luković, M.; Herrmann, H.J. Geometry-induced nonequilibrium phase transition in sandpiles. Phys. Rev. E 2020, 101, 032116. [Google Scholar] [CrossRef]
- Fersula, J.; Nous, C.; Perrot, K. Sandpile toppling on Penrose tilings: Identity and isotropic dynamics. arXiv 2020, arXiv:2006.06254v1. [Google Scholar]
- Kalinin, N.; Guzman-Saenz, A.; Prieto, Y.; Shkolnikov, M.; Kalinina, V.; Lupercio, E. Self-organized criticality and pattern emergence through the lens of tropical geometry. Proc. Natl. Acad. Sci. USA 2018, 115, E8135–E8142. [Google Scholar] [CrossRef] [PubMed]
- Tadić, B.; Priezzhev, V. Scaling of avalanche queues in directed dissipative sandpiles. Phys. Rev. E 2000, 62, 3266–3275. [Google Scholar] [CrossRef]
- Tadić, B. Disorder-induced critical behavior in driven diffusive systems. Phys. Rev. E 1998, 58, 168–173. [Google Scholar] [CrossRef]
- Antonov, N.V.; Gulitskiy, N.M.; Kakin, P.I.; Serov, V.D. Effects of turbulent environment and random noise on self-organized critical behavior: Universality versus nonuniversality. Phys. Rev. E 2021, 103, 042106. [Google Scholar] [CrossRef] [PubMed]
- Hwa, T.; Kardar, M. Avalanches, hydrodynamics, and discharge events in models of sandpiles. Phys. Rev. A 1992, 45, 7002. [Google Scholar] [CrossRef]
- Drossel, B. Scaling behavior of the Abelian sandpile model. Phys. Rev. E 2000, 61, R2168. [Google Scholar] [CrossRef]
- Bargov, A.A.; Iakovlev, I.A.; Iliasov, A.A.; Katsnelson, M.K.; Mazurenko, V.V. Multiscale structural complexity of natural patterns. Proc. Natl. Acad. Sci. USA 2020, 117, 30241–30251. [Google Scholar]
- Spasojević, D.; Janićević, S.; Knežević, M. Analysis of spanning avalanches in the two-dimensional nonequilibrium zero-temperature random-field Ising model. Phys. Rev. E 2014, 89, 012118. [Google Scholar] [CrossRef] [PubMed]
- Tadić, B.; Mijatović, S.; Janićević, S.; Spasojević, D.; Rodgers, G.J. The critical Barkhausen avalanches in thin random-field ferromagnets with an open boundary. Sci. Rep. 2019, 9, 6340. [Google Scholar] [CrossRef]
- Tadić, B. Dynamical implications of sample shape for avalanches in 2-dimensional random-field Ising model with saw-tooth domain wall. Phys. Stat. Mech. Its Appl. 2018, 493, 330–341. [Google Scholar] [CrossRef]
- Tadić, B.; Mitrović Dankulov, M.; Melnik, R. Mechanisms of self-organized criticality in social processes of knowledge creation. Phys. Rev. E 2017, 96, 032307. [Google Scholar] [CrossRef] [PubMed]
- Pavlov, A.N.; Anishchenko, V.S. Multifractal analysis of complex signals. Phys. Uspekhi 2007, 50, 819–834. [Google Scholar] [CrossRef]
- Kantelhardt, J.W.; Zschiegner, S.A.; Koscielny-Bunde, E.; Havlin, S.; Bunde, A.; Stanley, H.E. Multifractal detrended fluctuation analysis of nonstationary time series. Phys. Stat. Mech. Its Appl. 2002, 316, 87–114. [Google Scholar] [CrossRef]
- Tadić, B. Multifractal analysis of Barkhausen noise reveals the dynamic nature of criticality at hysteresis loop. J. Stat. Mech. Theory Exp. 2016, 6, 063305. [Google Scholar] [CrossRef]
- Perez-Reche, F.J.; Tadić, B.; Manosa, L.; Planes, A.; Vives, E. Driving rate effects in avalanche-mediated first-order phase transitions. Phys. Rev. Lett. 2004, 93, 195701. [Google Scholar] [CrossRef]
- Privman, V. (Ed.) Finite Size Scaling and Numerical Simulation of Statistical Systems; World Scientific: Singapore, 1990. [Google Scholar]
- Tadić, B.; Nowak, U.; Usadel, K.D.; Ramaswamy, R.; Padlewski, S. Scaling behavior in disordered sandpile automata. Phys. Rev. A 1992, 45, 8536–8545. [Google Scholar] [CrossRef] [PubMed]
- Spasojević, D.; Mijatović, S.; Navas-Portella, V.; Vives, E. Crossover from three-dimensional to two-dimensional systems in the nonequilibrium zero-temperature random-field Ising model. Phys. Rev. E 2018, 97, 012109. [Google Scholar] [CrossRef]
- Tebaldi, C.; Menech, M.D.; Stella, A.L. Multifractal scaling in the Bak-Tang-Wiesenfeld sandpile and edge events. Phys. Rev. Lett. 1999, 83, 3952–3955. [Google Scholar] [CrossRef]
- Chang, T.; Wu, C.-C. Rank-ordered multifractal spectrum for intermittent fluctuations. Phys. Rev. E 2008, 77, 045401. [Google Scholar] [CrossRef]
- Ivashkevich, E.V.; Ktitarev, V.; Priezzhev, D.V.B. Waves of topplings in an Abelian sandpile. Phys. Stat. Mech. Its Appl. 1994, 209, 347–360. [Google Scholar] [CrossRef]
- Tadić, B. Temporally disordered granular flow: A model of landslides. Phys. Rev. E 1998, 57, 4375–4381. [Google Scholar] [CrossRef]
- Corral, Á. Local distributions and rate fluctuations in a unified scaling law for earthquakes. Phys. Rev. E 2003, 68, 035102. [Google Scholar] [CrossRef] [PubMed]
- Mitrović Dankulov, M.; Tadić, B.; Melnik, R. The dynamics of meaningful social interactions and the emergence of collective knowledge. Sci. Rep. 2015, 5, 12197. [Google Scholar] [CrossRef] [PubMed]
- Schwab, D.J.; Nemenman, I.; Mehta, P. Zipf’s law and criticality in multivariate data without fine tuning. Phys. Rev. Lett. 2014, 113, 068102. [Google Scholar] [CrossRef]
- Corominas-Murtra, B.; Hanel, R.; Thurner, S. Understanding scaling through history-dependent processes with collapsing sample space. Proc. Natl. Acad. Sci. USA 2015, 112, 5348–5353. [Google Scholar] [CrossRef]
- Andjelković, M.; Tadić, B.; Melnik, R. The topology of higher-order complexes associated with brain hubs in human connectomes. Sci. Rep. 2020, 10, 17320. [Google Scholar] [CrossRef]
- Šuvakov, M.; Andjelković, M.; Tadić, B. Hidden geometries in networks arising from cooperative self-assembly. Sci. Rep. 2018, 8, 1987. [Google Scholar] [CrossRef] [PubMed]
- Šuvakov, M.; Mitrović, M.; Gligorijević, V.; Tadić, B. How the online social networks are used: Dialogs-based structure of Myspace. J. Roy. Soc. Interface 2012, 10, 20120819. [Google Scholar] [CrossRef]
- Tadić, B.; Melnik, R. Modeling latent infection transmissions through biosocial stochastic dynamics. PLoS ONE 2020, 15, e0241163. [Google Scholar] [CrossRef] [PubMed]
- Chialvo, D.R. Emergent complex neural dynamics. Nat. Phys. 2010, 6, 744–750. [Google Scholar] [CrossRef]
- Plenz, D.; Niebur, E. (Eds.) Criticality in Neural Systems; Wiley-VCH Verlag GmbH & Co., KGaA: Weinheim, Germany, 2014. [Google Scholar]
- Atasoy, S.; Donelly, I.; Pearson, J. Human brain networks function in connectome-specific harmonic waves. Nat. Commun. 2015, 7, 10340. [Google Scholar] [CrossRef] [PubMed]
- Rubinov, M.; Sporns, O.; Thivierge, J.; Breakspear, M. Neurobiologically realistic determinants of self-organized criticality in networks of spiking neurons. PLoS Comput. Biol. 2011, 7, e1002038. [Google Scholar] [CrossRef] [PubMed]
- Maslenikov, O.V.; Shchapin, D.S.; Nekorkin, V.I. Transient sequences in a hypernetwork generated by an adaptive network of spiking neurons. Phylos. Trans. R. Soc. A 2016, 375, 20160288. [Google Scholar] [CrossRef] [PubMed]
- Tadić, B.; Andjelković, M.; Melnik, R. Functional geometry of human connectomes. Sci. Rep. 2019, 9, 12060. [Google Scholar] [CrossRef]
- Reimann, M.W.; Nolte, M.; Scolamiero, M.; Turner, K.; Perin, R.; Chindemi, G.; Markram, H. Cliques of neurons bound into cavities provide missing link between structure and function. Front. Comput. Neiurosci. 2017, 11, 48. [Google Scholar] [CrossRef]
- Gros, C. A devil’s advocate view on self-organized brain criticality. J. Phys. Complex. 2021, 2, 031001. [Google Scholar] [CrossRef]
- Plenz, D.; Ribeiro, T.L.; Miller, S.R.; Kells, P.A.; Vakili, A.; Capek, E.L. Self-Organized Criticality in the Brain. Front. Phys. 2021, 9, 639389. [Google Scholar] [CrossRef]
- Zimmern, V. Why Brain Criticality is Clinically Relevant: A Scoping Review. Front. Neural Circuits 2020, 14, 54. [Google Scholar] [CrossRef] [PubMed]
- Valderhaug, V.D.; Heiney, K.; Ramstad, O.H.; Brathen, G.; Kuan, W.-L.; Nichele, S.; Sandvig, A.; Sandvig, I. Early functional changes associated with alpha-synuclein proteinopathy in engineered neural networks. A J. Physiol. Cell Physiol. 2021, 320, C1141–C1152. [Google Scholar] [CrossRef]
- Ritacco, H.A. Complexity and self-organized criticality in liquid foams, a short review. Adv. Colloid Interface Sci. 2020, 285, 102282. [Google Scholar] [CrossRef]
- Shur, V.Y. Kinetics of ferroelectric domains: Application of general approach to LiNbO3 and LiTaO3. Front. Ferroelectr. 2006, 41, 199–210. [Google Scholar] [CrossRef]
- Salje, E.K.H.; Xue, D.; Ding, X.; Dahmen, K.A.; Scott, J.F. Ferroelectric switching and scale invariant avalanches in BaTiO3. Phys. Rev. Mater. 2019, 3, 014415. [Google Scholar] [CrossRef]
- Nataf, G.F.; Salje, E.K.H. Avalanches in ferroelectric, ferroelastic and coelastic materials: Phase transition, domain switching and propagation. Ferroelectrics 2020, 569, 82–107. [Google Scholar] [CrossRef]
- Gallardo, M.C.; Manchado, J.; Romero, F.J.; del Cerro, J.; Salje, E.K.H.; Planes, A.; Vives, E.; Romero, R.; Stipcich, M. Avalanche criticality in the martensitic transition of Cu67.64Zn16.71Al15.65 shape-memory alloy: A calorimetric and acoustic emission study. Phys. Rev. B 2010, 81, 174102. [Google Scholar] [CrossRef]
- Malamud, B.; Morein, G.; Turcotte, D. Forest fires: An example of self-organized critical behavior. Science 1998, 281, 1840–1842. [Google Scholar] [CrossRef]
- Corral, Á. Long-term clustering, scaling, and universality in the temporal occurrence of earthquakes. Phys. Rev. Lett. 2004, 92, 108501. [Google Scholar] [CrossRef] [PubMed]
- Smyth, W.D.; Nash, J.D.; Moum, J.N. Self-organized criticality in geophysical turbulence. Sci. Rep. 2019, 9, 3743. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.; Dai, Z. Self-organized criticality in X-ray flares of gamma-ray-burst afterglows. Nat. Phys. 2013, 9, 465. [Google Scholar] [CrossRef]
- Diep, H.T. Frustrated Spin Systems; World Scientific: Singapore, 2005; Available online: https://www.worldscientific.com/doi/pdf (accessed on 20 October 2021).
- Petit, S.; Guitteny, S.; Robert, J.; Bonville, P.; Decorse, C.; Ollivier, J.; Mutka, H.; Mirebeau, I. Spin dynamics in highly frustrated pyrochlore magnets. Eur. Phys. J. Web Conf. 2015, 83, 03012. [Google Scholar] [CrossRef]
- Vanderstraeten, L.; Vanhecke, B.; Verstraete, F. Residual entropies for three-dimensional frustrated spin systems with tensor networks. Phys. Rev. E 2018, 98, 042145. [Google Scholar] [CrossRef]
- Banas, L.; Gess, B.; Neuss, M. Stochastic partial differential equations arising in self-organized criticality. arXiv 2021, arXiv:2104.13336v1. [Google Scholar]
- Zhang, Y.-C. Scaling theory of self-organized criticality. Phys. Rev. Lett. 1989, 63, 470. [Google Scholar] [CrossRef]
- Tadić, B.; Gligorijević, V.; Mitrović, M.; Šuvakov, M. Co-evolutionary mechanisms of emotional bursts in online social dynamics and networks. Entropy 2013, 12, 5084–5120. [Google Scholar] [CrossRef]
- Tadić, B. Self-organised criticality and emergent hyperbolic networks: Blueprint for complexity in social dynamics. Eur. J. Phys. 2019, 40, 024002. [Google Scholar] [CrossRef]
- Dmitriev, A.; Dmitriev, V. Identification of self-organized critical state on Twitter based on the retweets’ time series analysis. Complexity 2021, 6612785, 2021. [Google Scholar]
- Loretto, V.; Steels, L. Emergence of language. Nat. Phys. 2007, 3, 758. [Google Scholar] [CrossRef]
- Gromov, V.; Migrina, A. A language as a self-organized critical system. Complexity 2017, 2017, 9212538. [Google Scholar] [CrossRef]
- Zhukov, D.; Kunavin, K.; Lyamin, S. Online rebellion: Self-organized criticality of contemporary protest movements. SAGE Open 2020, 10, 2158244020923354. [Google Scholar] [CrossRef]
- Roberts, D.; Turcotte, D. Fractality and self-organized criticality of wars. Fractals 1998, 6, 351–357. [Google Scholar] [CrossRef]
- Zhukov, D.S.; Kanishchev, V.V.; Lyamin, S. Application of the theory of self-organized criticality to the investigation of historical processes. SAGE Open 2016, 6, 2158244016683216. [Google Scholar] [CrossRef]
- Sun, R. (Ed.) Grounding Social Sciences in Cognitive Sciences; MIT Press: Cambridge, MA, USA, 2012. [Google Scholar]
- Kitchener, R.F. Piaget’s Social Epistemology. In Social Interactions and the Development of Knowledge; Carpendale, J.I.M., Müller, U., Eds.; Lawrence Erlbaum Associates, Inc.: Mahwah, NJ, USA, 2013; pp. 45–66. [Google Scholar]
- Seraj, M. We create, we connect, we respect, therefore we are: Intellectual, social, and cultural value in online communities. J. Interact. Mark. 2012, 26, 209–222. [Google Scholar] [CrossRef]
- Andjelković, M.; Tadić, B.; Mitrović Dankulov, M.; Rajković, M.; Melnik, R. Topology of innovation spaces in the knowledge networks emerging through questions-and-answers. PLoS ONE 2016, 11, e0154655. [Google Scholar] [CrossRef]
- Tadić, B.; Melnik, R. Microscopic dynamics modeling unravels the role of asymptomatic virus carriers in SARS-CoV-2 epidemics at the interplay between biological and social factors. Comput. Biol. Med. 2021, 133, 104422. [Google Scholar] [CrossRef]
- Laredo, J.L.J.; Guinand, F.; Oliver, D.; Bouvry, P. Load balancing at the edge of chaos: How self-organized criticality can lead to energy-efficient computing. HAL Arch. 2019, 01960412, 2019. [Google Scholar] [CrossRef]
- Gasior, J.; Seredynski, F. A sandpile cellular automata-based approach to dynamic job scheduling in cloud environment. Lect. Note Comput. Sci. Book Ser. Parallel Process. Appl. Math. 2016, 9574, 46. [Google Scholar]
- Martucci, R.; Mascia, C.; Simeoni, C.; Tassi, F. Hospital management in the COVID-19 emergency: Abelian sandpile paradigm and beyond. arXiv 2021, arXiv:2102.11974v1. [Google Scholar]
- Chen, Y.; Zhou, Y. Scaling laws and indicators of self-organized criticality in urban systems. Chais Solitons Fractals 2006, 35, 85–98. [Google Scholar] [CrossRef]
- Bartolozzi, M.; Leinweber, D.B.; Thomas, A.W. Self-organized criticality and stock market dynamics: An empirical study. Phys. A 2006, 350, 451–465. [Google Scholar] [CrossRef]
- Gilbert, I.; Nisoli, C.; Schiffer, P. Frustration by design. Phys. Today 2016, 69, 54. [Google Scholar] [CrossRef]
- Jungwirth, T.; Marti, X.; Wadley, P.; Wunderlich, J. Antiferromagnetic spintronics. Nat. Nanotechnol. 2016, 11, 231. [Google Scholar] [CrossRef]
- Sloetjes, S.D.; Urdahl, H.H.; Grepstad, J.K.; Folven, E. Tailoring the magnetic order in a supermagnetic metamaterial. AIP Adv. 2017, 7, 056325. [Google Scholar] [CrossRef]
- Tadić, B.; Šuvakov, M.; Andjelković, M.; Rodgers, G.J. Large-scale influence of defect bonds in geometrically constrained self-assembly. Phys. Rev. E 2020, 102, 032307. [Google Scholar] [CrossRef]
- Šuvakov, M.; Andjelković, M.; Tadić, B. Applet: Simplex Aggregated Growing Graph. 2017. Available online: http://suki.ipb.rs/ggraph/ (accessed on 20 October 2021).
- Tadić, B.; Gupte, N. Hidden geometry and dynamics of complex networks: Spin reversal in nanoassemblies with pairwise and triangle-based interactions. EPL (Europhys. Lett.) 2020, 132, 60008. [Google Scholar] [CrossRef]
- Chutani, M.; Tadić, B.; Gupte, N. Hysteresis and synchronization processes of kuramoto oscillators on high-dimensional simplicial complexes with the competing simplex-encoded couplings. Phys. Rev. E 2021, 104, 034206. [Google Scholar] [CrossRef]
- Beaumont, J.R.; Gatrell, A.C. An Introduction to Q-Analysis; Geo Abstracts; Norwich-Printed by Edmund Nome Press: Norwich, UK, 1982. [Google Scholar]
- Skardal, P.S.; Arenas, A. Abrupt desynchronization and extensive multistability in globally coupled oscillator simplexes. Phys. Rev. Lett. 2019, 122, 248301. [Google Scholar] [CrossRef]
- Tadić, B.; Andjelković, M.; Šuvakov, M.; Rodgers, G.J. Magnetisation processes in geometrically frustrated spin networks with self-assembled cliques. Entropy 2020, 22, 336. [Google Scholar] [CrossRef] [PubMed]
- Brunt, D.; Balakrishnan, G.; Mayoh, D.A.; Lees, M.R.; Gobrunov, D.; Qureshi, N.; Petrenko, O.A. Magnetisation process in the rare earth tetraborides, NdB4 and HoB4. Sci. Rep. 2018, 8, 232. [Google Scholar] [CrossRef]
- Pérez-Reche, F.-J.; Truskinovsky, L.; Zanzotto, G. Driving-induced crossover: From classical criticality to self-organized criticality. Phys. Rev. Lett. 2008, 101, 230601. [Google Scholar] [CrossRef] [PubMed]
- Krasnytska, M.; Berche, B.; Holovatch, Y.; Kenna, R. Ising model with variable spin/agent strengths. J. Phys. Complex. 2020, 1, 035008. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tadić, B.; Melnik, R. Self-Organised Critical Dynamics as a Key to Fundamental Features of Complexity in Physical, Biological, and Social Networks. Dynamics 2021, 1, 181-197. https://doi.org/10.3390/dynamics1020011
Tadić B, Melnik R. Self-Organised Critical Dynamics as a Key to Fundamental Features of Complexity in Physical, Biological, and Social Networks. Dynamics. 2021; 1(2):181-197. https://doi.org/10.3390/dynamics1020011
Chicago/Turabian StyleTadić, Bosiljka, and Roderick Melnik. 2021. "Self-Organised Critical Dynamics as a Key to Fundamental Features of Complexity in Physical, Biological, and Social Networks" Dynamics 1, no. 2: 181-197. https://doi.org/10.3390/dynamics1020011
APA StyleTadić, B., & Melnik, R. (2021). Self-Organised Critical Dynamics as a Key to Fundamental Features of Complexity in Physical, Biological, and Social Networks. Dynamics, 1(2), 181-197. https://doi.org/10.3390/dynamics1020011






